Crystal Plasticity Modeling to Capture Microstructural Variations in Cold-Sprayed Materials
Abstract
1. Introduction
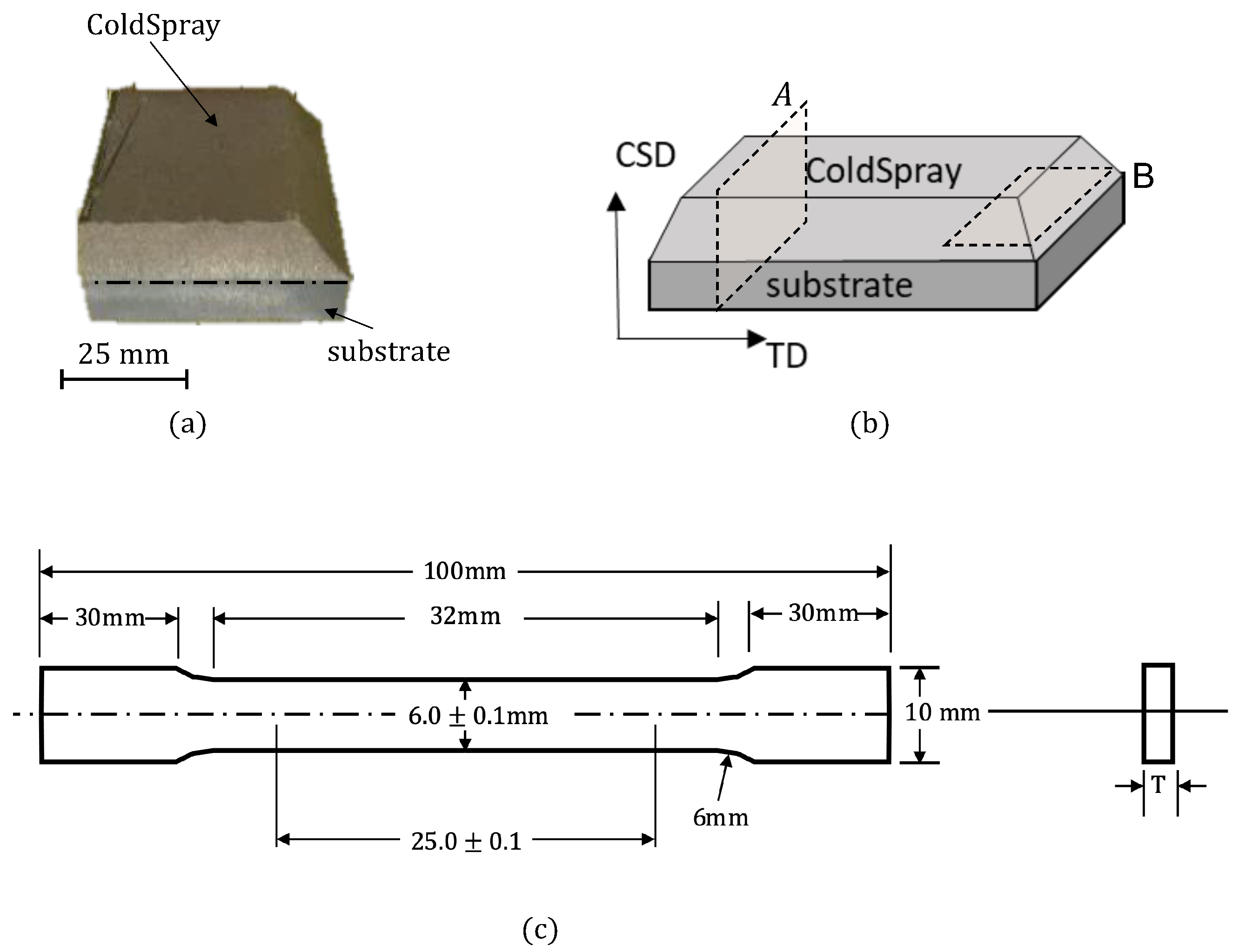
2. Experimental Methods
3. Experimental Results and Discussion
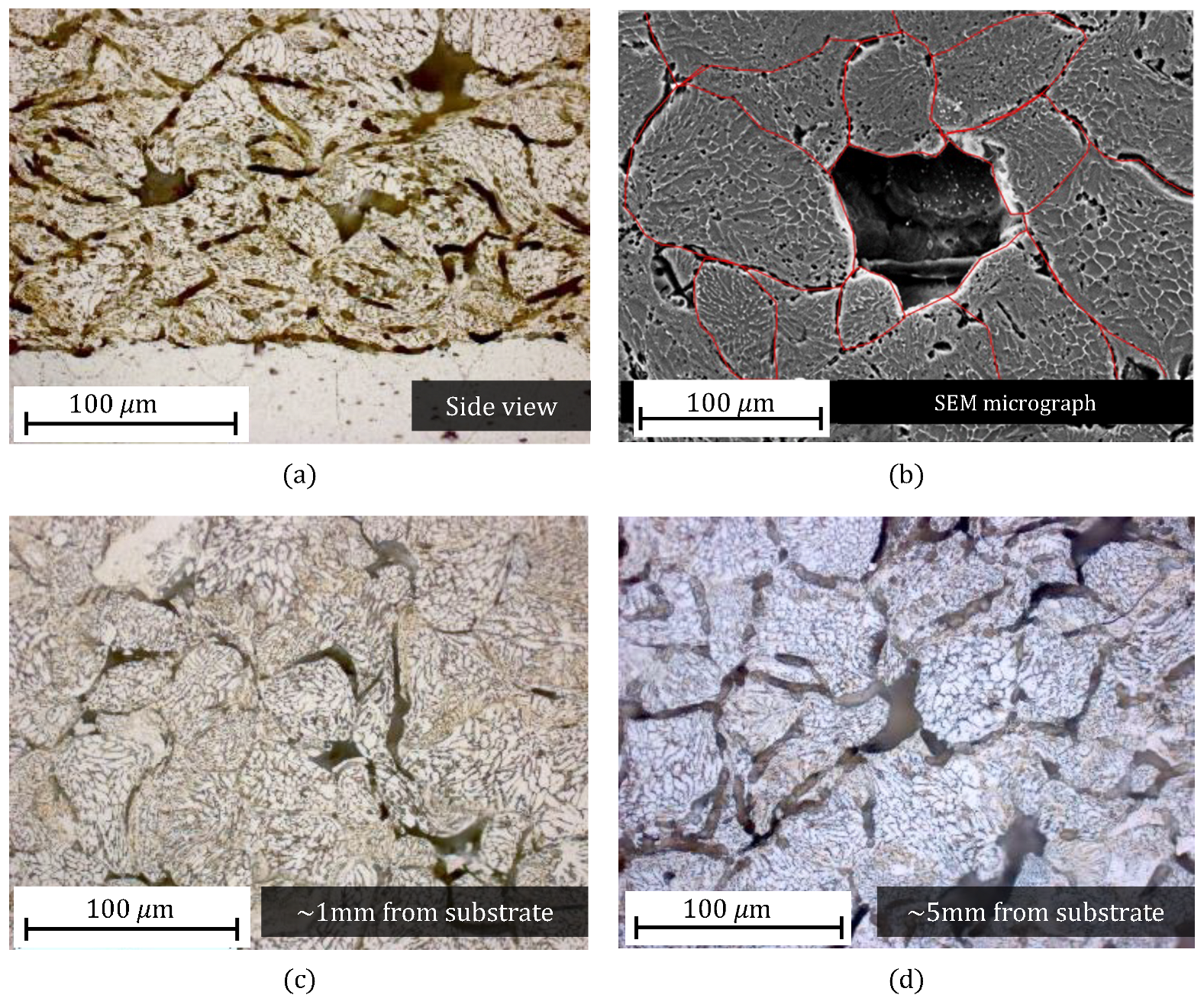
3.1. Optical Microscopy (OM)
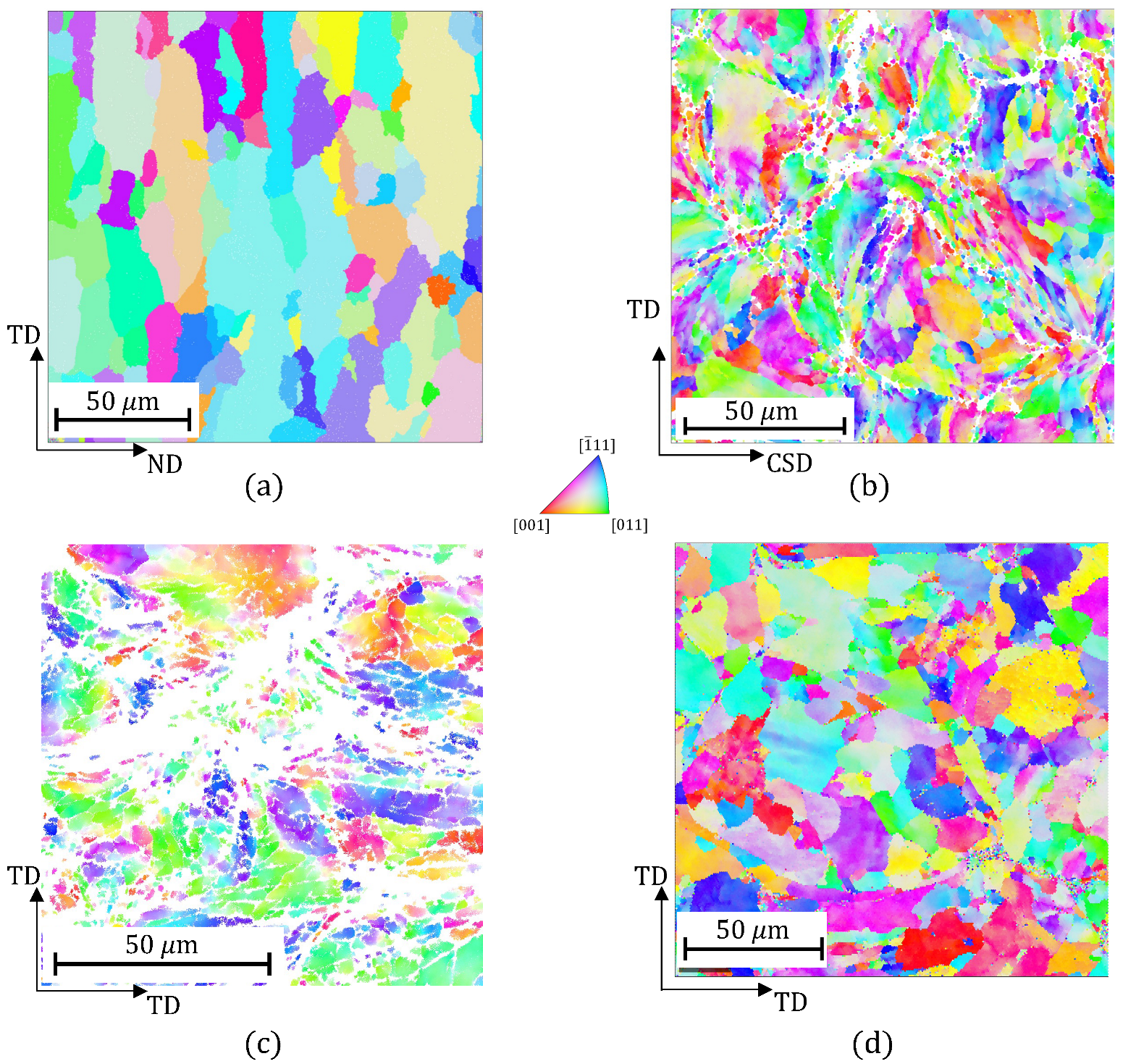
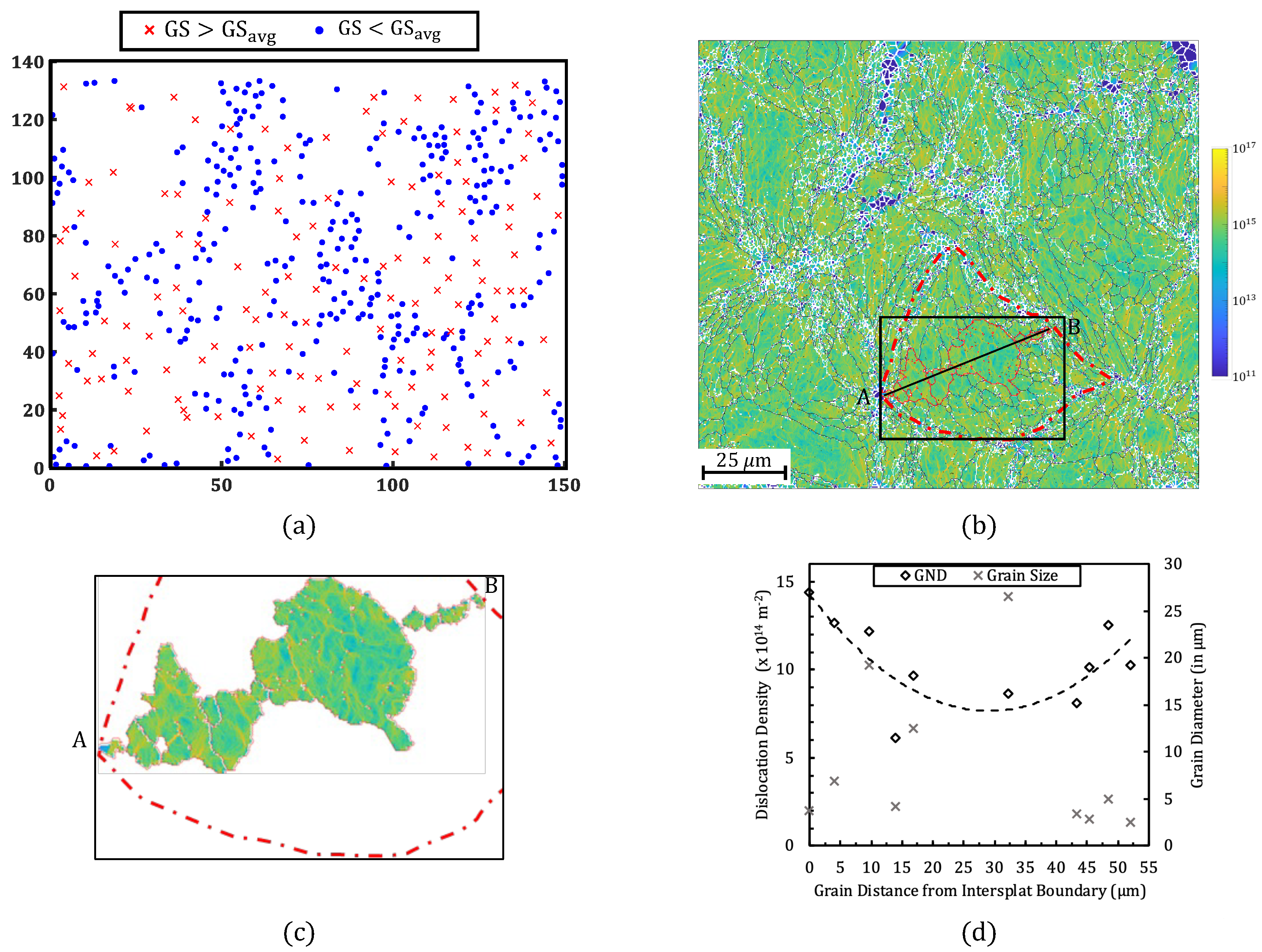
3.2. Electron Backscatter Diffraction (EBSD)
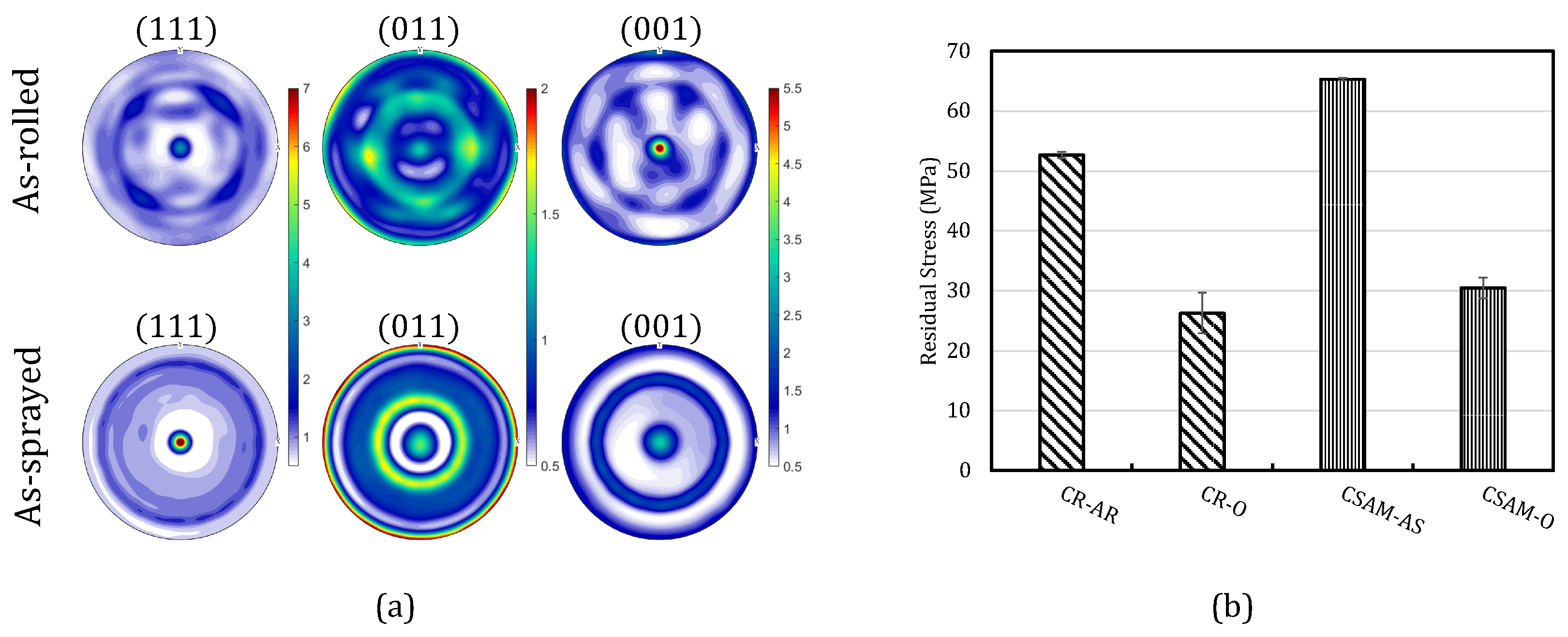
3.3. X-ray Diffraction (XRD)
3.4. Uniaxial Tension Test
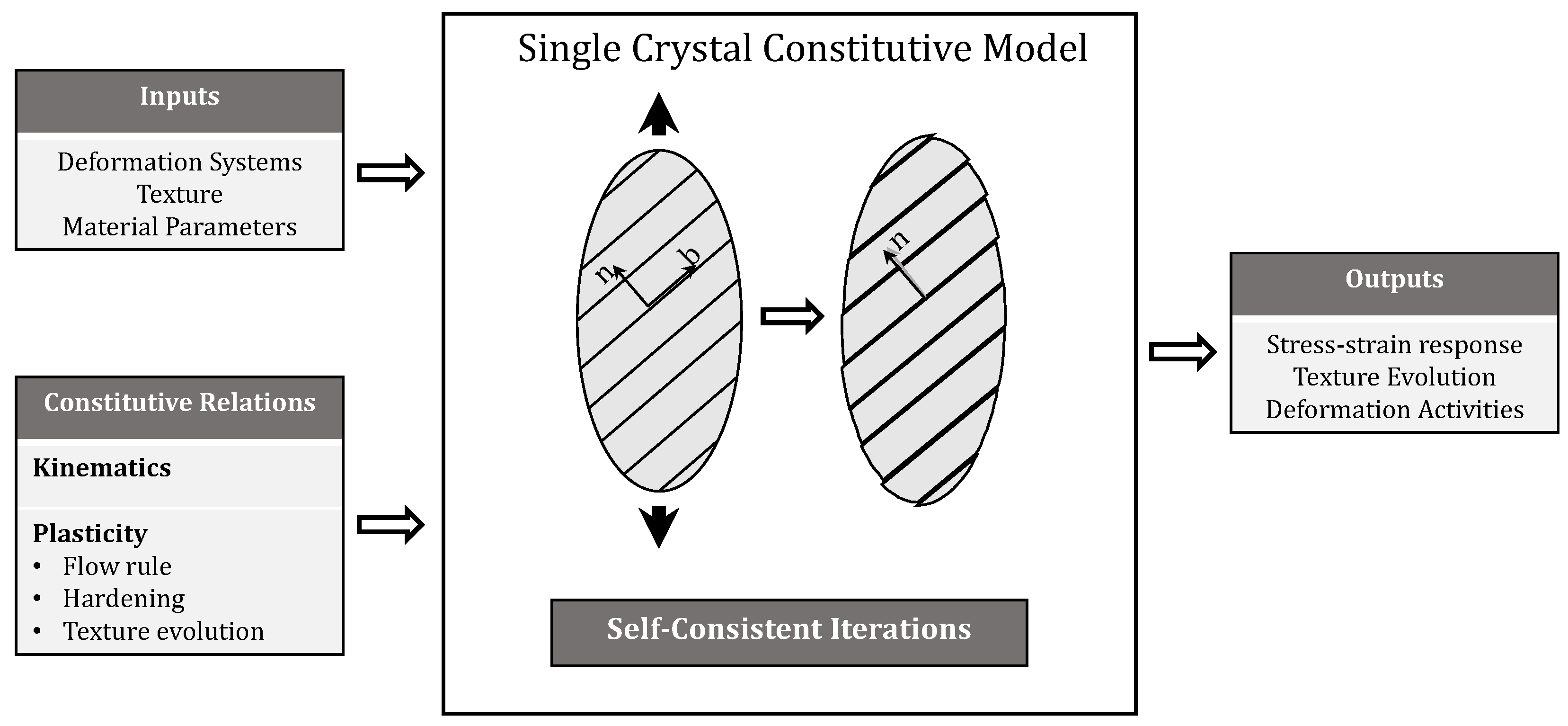
4. Simulation Method
4.1. Viscoplastic Self-Consistent (VPSC)
4.2. Hardening Rule
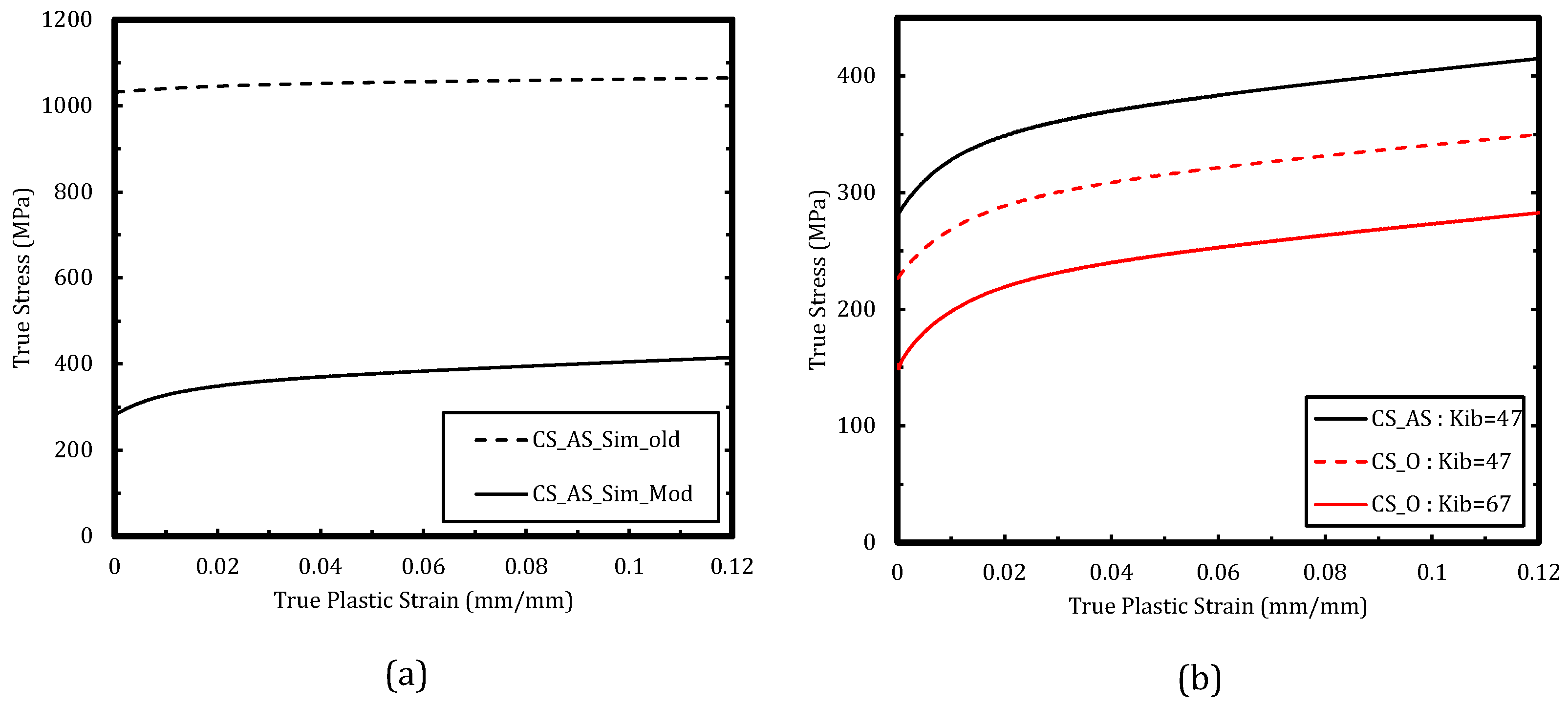
4.3. Intersplat Boundary Effects
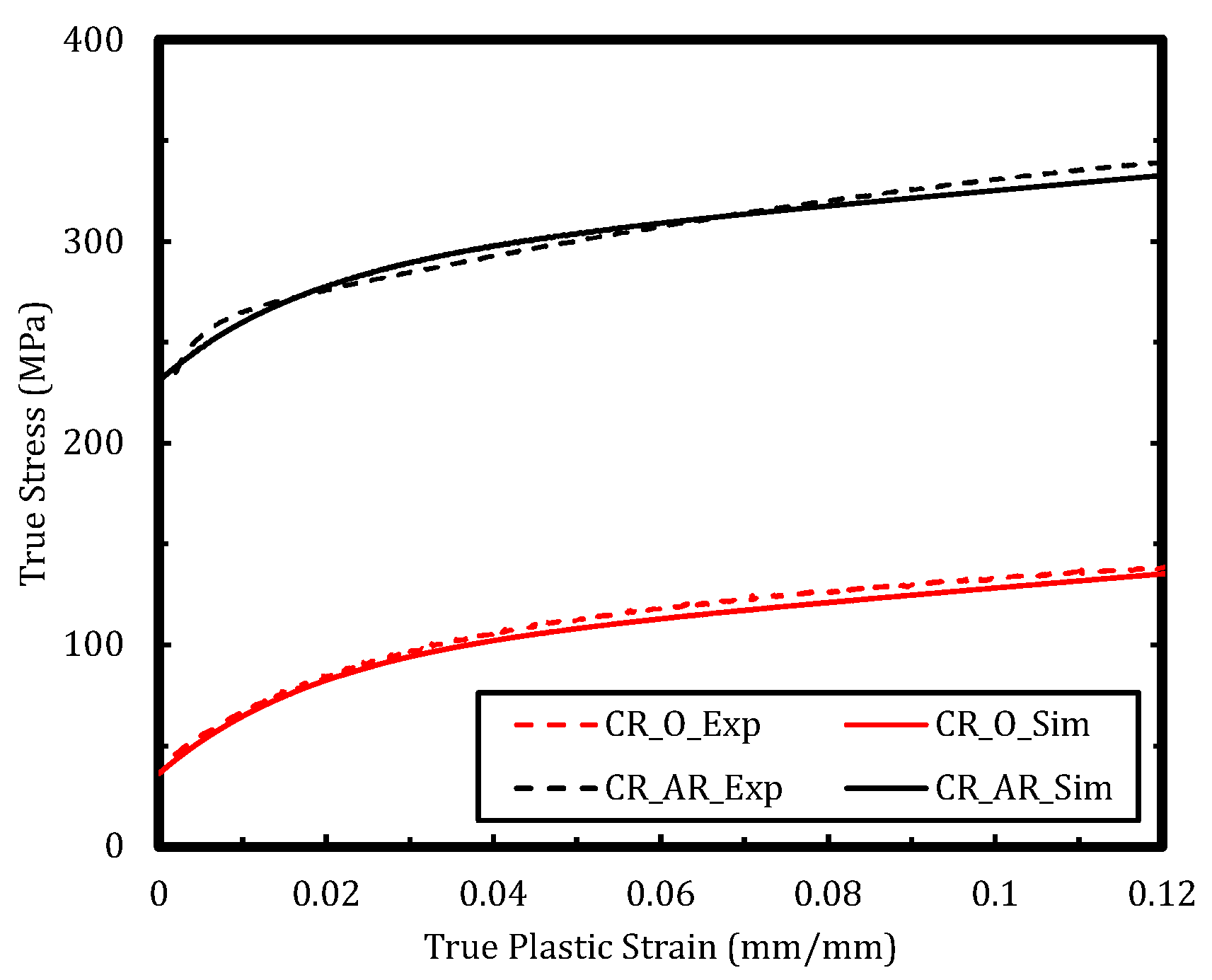
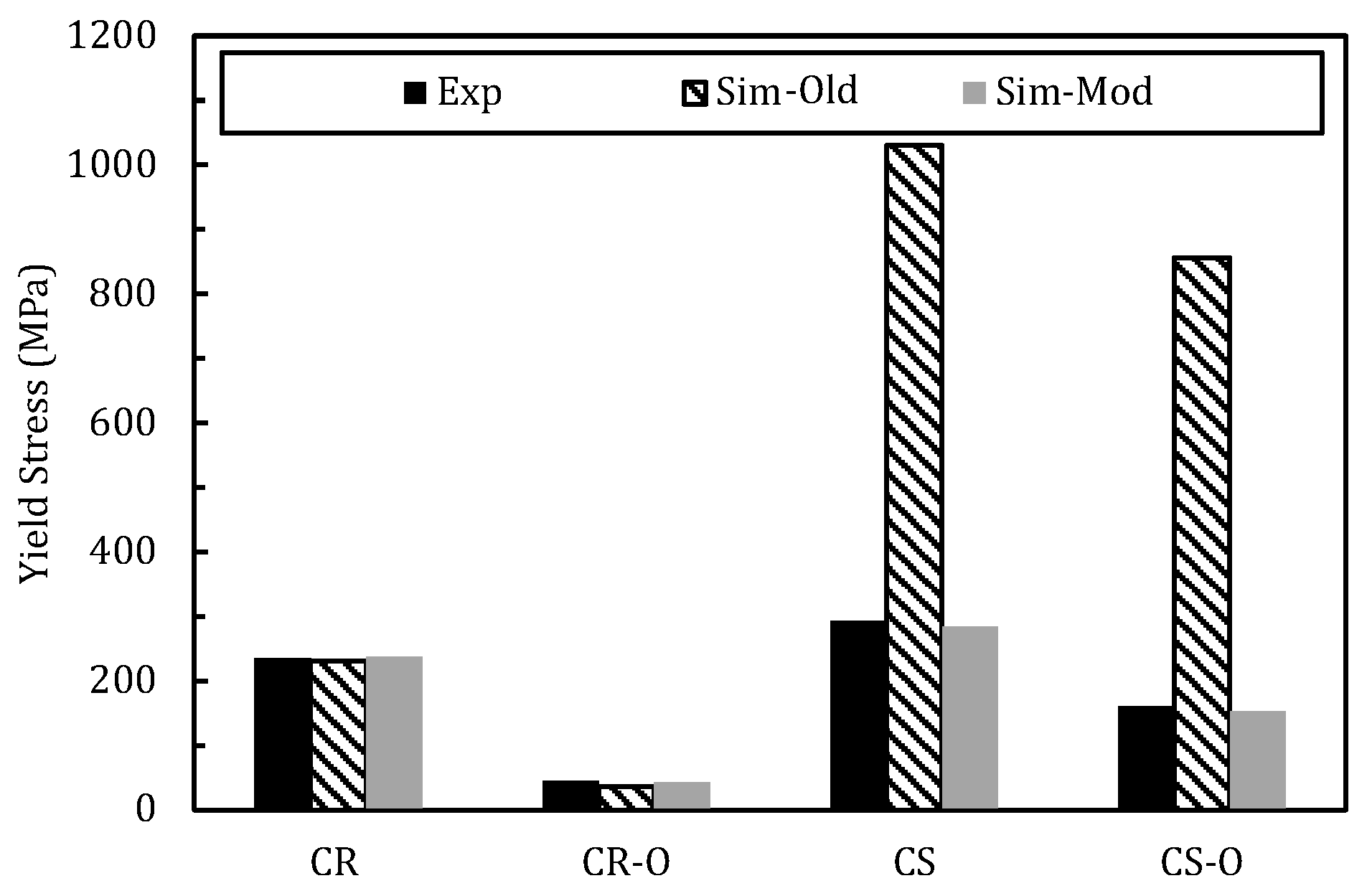
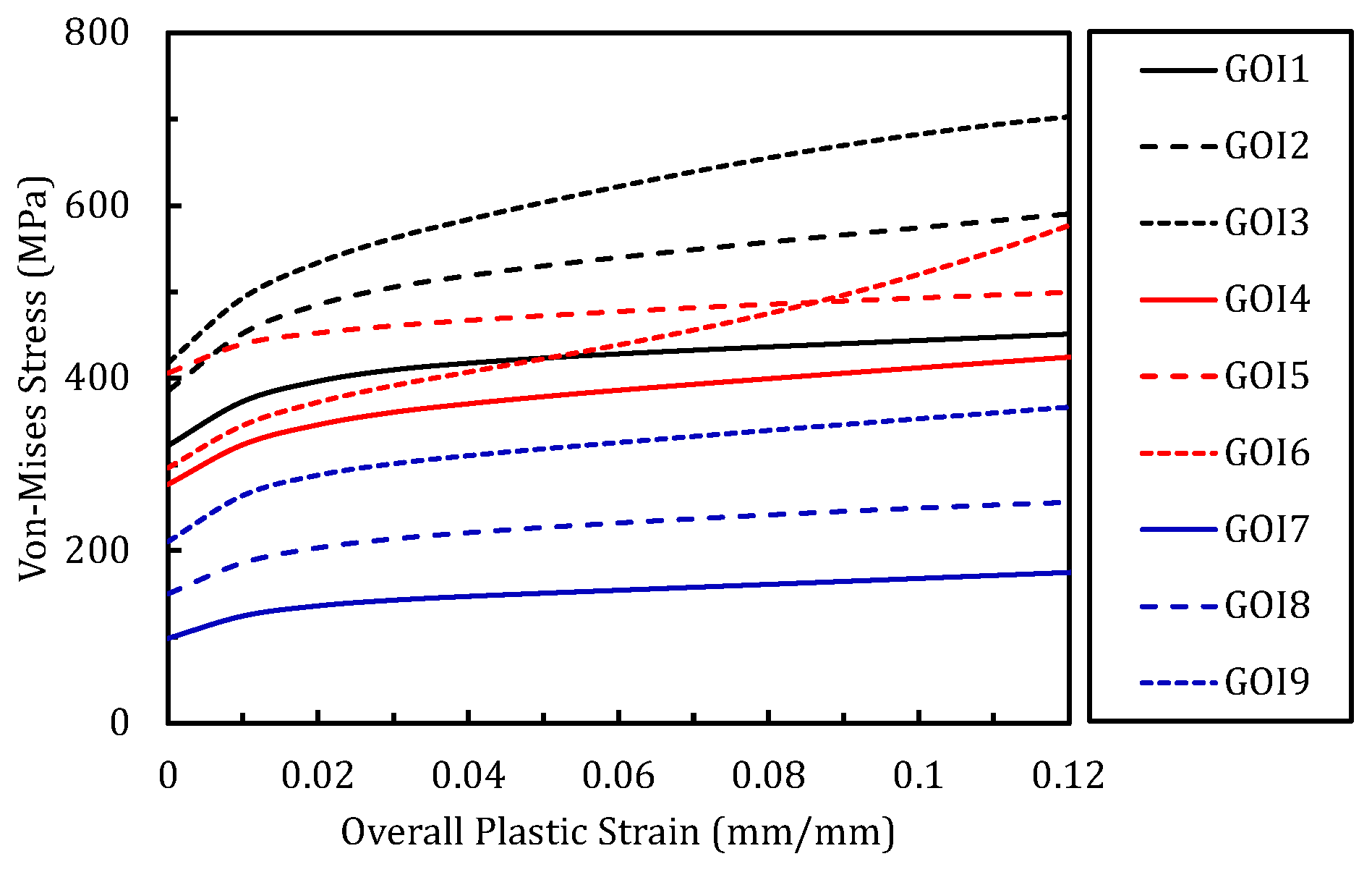
5. Simulation Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chakrabarty, R.; Song, J. Crystal plasticity finite element investigation of deformation of single crystal copper during cold spray. Addit. Manuf. 2022, 49, 1024–1084. [Google Scholar] [CrossRef]
- Yin, S.; Cavaliere, P.; Aldwell, B.; Jenkins, R.; Liao, H.; Li, W.; Lupoi, R. Cold spray additive manufacturing and repair: Fundamentals and applications. Addit. Manuf. 2018, 21, 628–650. [Google Scholar] [CrossRef]
- Rokni, M.R.; Widener, C.A.; Champagne, V.K.; Crawford, G.A.; Nutt, S.R. The effects of heat treatment on 7075 Al cold spray deposits. Surf. Coatings Technol. 2017, 310, 278–285. [Google Scholar] [CrossRef]
- Yin, S.; Lupoi, R. Cold Spray Additive Manufacturing; Springer: Berlin, Germany, 2021. [Google Scholar]
- Sabard, A.; McNutt, P.; Begg, H.; Hussain, T. Cold spray deposition of solution heat treated, artificially aged and naturally aged Al 7075 powder. Surf. Coatings Technol. 2020, 385, 125367. [Google Scholar] [CrossRef]
- Bagherifard, S.; Monti, S.; Zuccoli, M.V.; Riccio, M.; Kondás, J.; Guagliano, M. Cold spray deposition for additive manufacturing of freeform structural components compared to selective laser melting. Mater. Sci. Eng. A 2018, 721, 339–350. [Google Scholar] [CrossRef]
- Judas, J.; Zapletal, J.; Řehořek, L.; Jan, V. Effects of annealing temperature on microstructure and mechanical properties of cold sprayed AA7075. Procedia Struct. Integr. 2023, 43, 160–165. [Google Scholar] [CrossRef]
- Singh, R.; Kondás, J.; Bauer, C.; Cizek, J.; Medricky, J.; Csaki, S.; Čupera, J.; Procházka, R.; Melzer, D.; Konopik, P. Bulk-like ductility of cold spray additively manufactured copper in the as-sprayed state. Addit. Manuf. Lett. 2022, 3, 100052. [Google Scholar] [CrossRef]
- Stoltenhoff, T.; Borchers, C.; Gärtner, F.; Kreye, H. Microstructures and key properties of cold-sprayed and thermally sprayed copper coatings. Surf. Coatings Technol. 2006, 200, 4947–4960. [Google Scholar] [CrossRef]
- Rokni, M.; Widener, C.; Ozdemir, O.; Crawford, G. Microstructure and mechanical properties of cold sprayed 6061 Al in As-sprayed and heat treated condition. Surf. Coatings Technol. 2017, 309, 641–650. [Google Scholar] [CrossRef]
- Yin, S.; Cizek, J.; Chen, C.; Jenkins, R.; O’Donnell, G.; Lupoi, R. Metallurgical bonding between metal matrix and core-shelled reinforcements in cold sprayed composite coating. Scr. Mater. 2020, 177, 49–53. [Google Scholar] [CrossRef]
- Evans, W.C.; Dan, X.; Houshmand, A.; Müftü, S.; Ando, T. Microstructural Characterization of Aluminum 6061 Splats Cold Spray Deposited on Aluminum 6061-T6 Substrate. Metall. Mater. Trans. A 2019, 50, 3937–3948. [Google Scholar] [CrossRef]
- Prashar, G.; Vasudev, H. A comprehensive review on sustainable cold spray additive manufacturing: State of the art, challenges and future challenges. J. Clean. Prod. 2021, 310, 127606. [Google Scholar] [CrossRef]
- Birt, A.; Champagne, V.; Sisson, R.; Apelian, D. Microstructural analysis of cold-sprayed Ti-6Al-4V at the micro-and nano-scale. J. Therm. Spray Technol. 2015, 24, 1277–1288. [Google Scholar] [CrossRef]
- Sabard, A.; Hussain, T. Inter-particle bonding in cold spray deposition of a gas-atomised and a solution heat-treated Al 6061 powder. J. Mater. Sci. 2019, 54, 12061–12078. [Google Scholar] [CrossRef]
- Pathak, S.; Saha, G.C. Development of sustainable cold spray coatings and 3D additive manufacturing components for repair/manufacturing applications: A critical review. Coatings 2017, 7, 122. [Google Scholar] [CrossRef]
- Bedard, B.A.; Flanagan, T.J.; Ernst, A.T.; Nardi, A.; Dongare, A.M.; Brody, H.D.; Champagne, V.K.; Lee, S.W.; Aindow, M. Microstructure and Micromechanical Response in Gas-Atomized Al 6061 Alloy Powder and Cold-Sprayed Splats. J. Therm. Spray Technol. 2018, 27, 1563–1578. [Google Scholar] [CrossRef]
- Guo, D.; Kazasidis, M.; Hawkins, A.; Fan, N.; Leclerc, Z.; MacDonald, D.; Nastic, A.; Nikbakht, R.; Ortiz-Fernandez, R.; Rahmati, S.; et al. Cold spray: Over 30 years of development toward a hot future. J. Therm. Spray Technol. 2022, 31, 866–907. [Google Scholar] [CrossRef]
- Blochet, Q.; Delloro, F.; N’guyen, F.; Jeulin, D.; Borit, F.; Jeandin, M. Effect of the cold-sprayed aluminum coating-substrate interface morphology on bond strength for aircraft repair application. J. Therm. Spray Technol. 2017, 26, 671–686. [Google Scholar] [CrossRef]
- Kumar, S.; Bodapati, B.R.; Vinay, G.; Kumar, V.; Chavan, N.M.; Suresh Babu, P.; Jyothirmayi, A. Estimation of inter-splat bonding and its effect on functional properties of cold sprayed coatings. Surf. Coatings Technol. 2021, 420, 127318. [Google Scholar] [CrossRef]
- Liu, T.; Leazer, J.D.; Brewer, L.N. Particle deformation and microstructure evolution during cold spray of individual Al-Cu alloy powder particles. Acta Mater. 2019, 168, 13–23. [Google Scholar] [CrossRef]
- Chen, C.; Xie, Y.; Yin, S.; Li, W.; Luo, X.; Xie, X.; Zhao, R.; Deng, C.; Wang, J.; Liao, H.; et al. Ductile and high strength Cu fabricated by solid-state cold spray additive manufacturing. J. Mater. Sci. Technol. 2023, 134, 234–243. [Google Scholar] [CrossRef]
- Assadi, H.; Kreye, H.; Gärtner, F.; Klassen, T. Cold spraying—A materials perspective. Acta Mater. 2016, 116, 382–407. [Google Scholar] [CrossRef]
- Hutasoit, N.; Javed, M.A.; Rashid, R.A.R.; Wade, S.; Palanisamy, S. Effects of build orientation and heat treatment on microstructure, mechanical and corrosion properties of Al6061 aluminium parts built by cold spray additive manufacturing process. Int. J. Mech. Sci. 2021, 204, 106526. [Google Scholar] [CrossRef]
- Mangalarapu, T.B.; Kumar, S.; Ramakrishna, M.; Gandham, P.; Suresh, K. Precipitation behavior of cold sprayed Al6061 coatings. Materialia 2022, 24, 101510. [Google Scholar] [CrossRef]
- Rahmati, S.; Veiga, R.G.A.; Jodoin, B.; Zúñiga, A. Crystal orientation and grain boundary effects on plastic deformation of FCC particles under high velocity impacts. Materialia 2021, 15, 101004. [Google Scholar] [CrossRef]
- Zhao, P.; Zhang, Q.; Guo, Y.; Liu, H.; Deng, Z. Atomic simulation of crystal orientation effect on coating surface generation mechanisms in cold spray. Comput. Mater. Sci. 2020, 184. [Google Scholar] [CrossRef]
- Msolli, S.; Zhang, Z.Q.; Seng, D.H.L.; Zhang, Z.; Guo, J.; Reddy, C.D.; Sridhar, N.; Pan, J.; Tan, B.H.; Loi, Q. An experimentally validated dislocation density based computational framework for predicting microstructural evolution in cold spray process. Int. J. Solids Struct. 2021, 225, 111065. [Google Scholar] [CrossRef]
- Sayahlatifi, S.; Shao, C.; McDonald, A.; Hogan, J. 3D Microstructure-Based Finite Element Simulation of Cold-Sprayed Al-Al2O3 Composite Coatings Under Quasi-Static Compression and Indentation Loading. J. Therm. Spray Technol. 2022, 31, 102–118. [Google Scholar] [CrossRef]
- Rokni, M.; Widener, C.; Champagne, V.; Crawford, G. Microstructure and mechanical properties of cold sprayed 7075 deposition during non-isothermal annealing. Surf. Coatings Technol. 2015, 276, 305–315. [Google Scholar] [CrossRef]
- Rokni, M.; Nardi, A.; Champagne, V.; Nutt, S. Effects of preprocessing on multi-direction properties of aluminum alloy cold-spray deposits. J. Therm. Spray Technol. 2018, 27, 818–826. [Google Scholar] [CrossRef]
- Bagherifard, S.; Guagliano, M. Fatigue performance of cold spray deposits: Coating, repair and additive manufacturing cases. Int. J. Fatigue 2020, 139, 105744. [Google Scholar] [CrossRef]
- Herriott, C.; Li, X.; Kouraytem, N.; Tari, V.; Tan, W.; Anglin, B.; Rollett, A.D.; Spear, A.D. A multi-scale, multi-physics modeling framework to predict spatial variation of properties in additive-manufactured metals. Model. Simul. Mater. Sci. Eng. 2019, 27, 025009. [Google Scholar] [CrossRef]
- Yang, K.; Li, W.; Yang, X.; Xu, Y.; Vairis, A. Effect of heat treatment on the inherent anisotropy of cold sprayed copper deposits. Surf. Coatings Technol. 2018, 350, 519–530. [Google Scholar] [CrossRef]
- Sun, W.; Tan, A.W.Y.; Wu, K.; Yin, S.; Yang, X.; Marinescu, I.; Liu, E. Post-Process Treatments on Supersonic Cold Sprayed Coatings: A Review. Coatings 2020, 10, 123. [Google Scholar] [CrossRef]
- Lebensohn, R.; Castañeda, P.P.; Brenner, R.; Castelnau, O. Computational Methods for Microstructure-Property Relationships; Springer: Berlin, Germany, 2011. [Google Scholar]
- Patil, C.S.; Chakraborty, S.; Niezgoda, S.R. Comparison of full field predictions of crystal plasticity simulations using the Voce and the dislocation density based hardening laws. Int. J. Plast. 2021, 147, 103099. [Google Scholar] [CrossRef]
- Liu, J.; Xiong, W.; Behera, A.; Thompson, S.; To, A.C. Mean-field polycrystal plasticity modeling with grain size and shape effects for laser additive manufactured FCC metals. Sci. Direct 2017, 112, 35–42. [Google Scholar] [CrossRef]
- Christy, T.; Murugan, N.; Kumar, S. A comparative study on the microstructures and mechanical properties of Al 6061 alloy and the MMC Al 6061/TiB2/12p. J. Miner. Mater. Charact. Eng. 2010, 9, 57–65. [Google Scholar]
- McMaster-Carr. Available online: https://www.mcmaster.com/ (accessed on 1 August 2022).
- Bachmann, F.; Hielscher, R.; Schaeben, H. Texture analysis with MTEX—Free and open source software toolbox. Solid State Phenom. 2010, 160, 63–68. [Google Scholar] [CrossRef]
- The MathWorks Inc. MATLAB, version: 9.13.0 (R2022b); The MathWorks Inc.: Natick, MA, USA, 2022. [Google Scholar]
- Lutterotti, L. Total pattern fitting for the combined size–strain–stress–texture determination in thin film diffraction. Nucl. Instruments Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2010, 268, 334–340. [Google Scholar] [CrossRef]
- ASTM-E0008/E0008M-22; Standard Test Methods for Tension Testing of Metallic Materials. ASTM International: West Conshohocken, PA, USA, 2022.
- Kazasidis, M.; Verna, E.; Yin, S.; Lupoi, R. The effect of heat treatment and impact angle on the erosion behavior of nickel-tungsten carbide cold spray coating using response surface methodology. Emergent Mater. 2021, 4, 1605–1618. [Google Scholar] [CrossRef]
- Abubakar, A.A. A hybrid computational approach for modeling cold spray deposition. Eng. Sci. Technol. Int. J. 2023, 48, 101579. [Google Scholar] [CrossRef]
- Pantleon, W. Resolving the geometrically necessary dislocation content by conventional electron backscattering diffraction. Scr. Mater. 2008, 58, 994–997. [Google Scholar] [CrossRef]
- Yang, K.; Li, W.; Guo, X.; Yang, X.; Xu, Y. Characterizations and anisotropy of cold-spraying additive-manufactured copper bulk. J. Mater. Sci. Technol. 2018, 34, 1570–1579. [Google Scholar] [CrossRef]
- Champagne, V.; Helfritch, D. Critical assessment 11: Structural repairs by cold spray. Mater. Sci. Technol. 2015, 31, 627–634. [Google Scholar] [CrossRef]
- Wang, Q.; Shankar, R.M.; Liu, Z. Visco-plastic self-consistent modeling of crystallographic texture evolution related to slip systems activated during machining Ti-6AL-4V. J. Alloys Compd. 2021, 853, 157336. [Google Scholar] [CrossRef]
- Lebensohn, R.A.; Tomé, C.N. A self-consistent anisotropic approach for the simulation of plastic deformation and texture development of polycrystals: Application to zirconium alloys. Acta Metall. Mater. 1993, 41, 2611–2624. [Google Scholar] [CrossRef]
- Tomé, C.; Lebensohn, R.; VISCO-PLASTIC SELF-CONSISTENT (VPSC). Los Alamos National Laboratory. Available online: https://public.lanl.gov/lebenso/VPSC7c_manual.pdf (accessed on 1 December 2021).
- Anglin, B.; Gockel, B.; Rollett, A. Developing constitutive model parameters via a multi-scale approach. Integr. Mater. Manuf. Innov. 2016, 5, 212–231. [Google Scholar] [CrossRef]
- Bobzin, K.; Wietheger, W.; Hebing, J.; Gerdt, L. Softening Behavior of Cold-Sprayed Aluminum-Based Coatings AA1200 and AA7075 During Annealing. J. Therm. Spray Technol. 2021, 30, 358–370. [Google Scholar] [CrossRef]
- Devi, G.N.; Kumar, S.; Balaji, T.S.; Mangalarapu, T.B.; Chandrasekhar, S.B.; Gopal, A.V.; Jyothirmayi, A. Influence of inter-splat bonding state of cold sprayed IN625 and IN718 coatings on mechanical and corrosion performance. Surf. Coatings Technol. 2022, 445, 128731. [Google Scholar] [CrossRef]
- Rokni, M.; Widener, C.; Champagne, V. Microstructural stability of ultrafine grained cold sprayed 6061 aluminum alloy. Appl. Surf. Sci. 2014, 290, 482–489. [Google Scholar] [CrossRef]
- Liu, X.; Qiao, X.; Xie, W.; Pei, R.; Yuan, L.; Zheng, M. Influence of grain size on microstructure, mechanical properties and strain hardening behavior of Mg-0.3Ca (wt.%) alloy. Mater. Sci. Eng. A 2022, 839, 142847. [Google Scholar] [CrossRef]

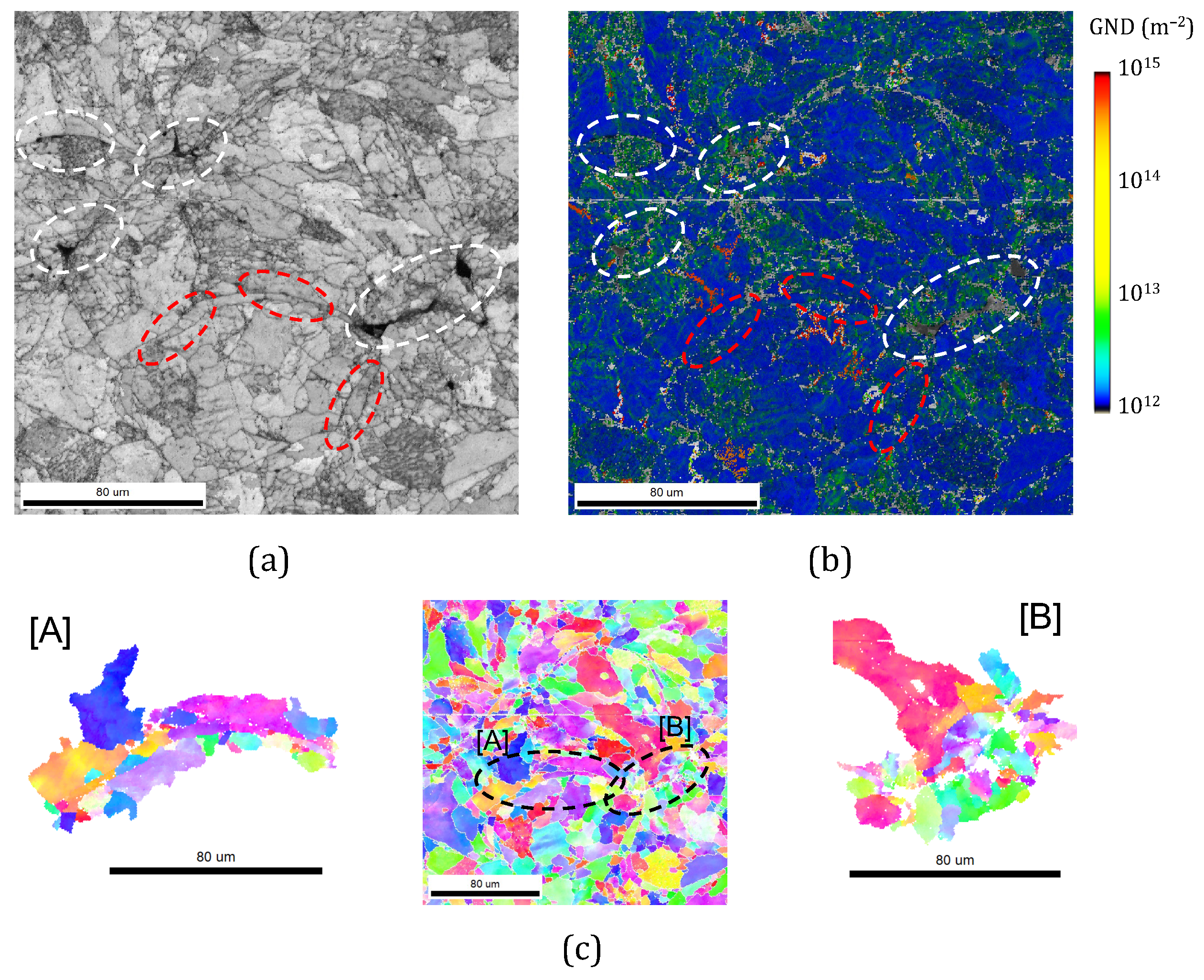
| Element | Al | Mg | Fe | Si | Cu | Mn | V | Ti |
|---|---|---|---|---|---|---|---|---|
| Weight (%) | 97.25 | 1.08 | 0.17 | 0.63 | 0.32 | 0.52 | 0.01 | 0.02 |
| Rolled | As-Sprayed CSAM | Annealed CSAM | |
|---|---|---|---|
| Average grain size | 27.8 | 10.3 | 17.9 |
| Average grain aspect ratio | 2.18 | 2.27 | 2.28 |
| Rolled | Rolled Annealed | CSAM | CSAM Annealed | |
|---|---|---|---|---|
| YS (MPa) | 243 ± 3 | 53 ± 2 | 293 | 160 |
| UTS (MPa) | 344 ± 17 | 142 ± 1 | 345 | 200 |
| % Elongation | 10.8 ± 0.5 | 17.8 ± 3.1 | 4 | 17 |
| Parameters | (Annealed) | ||||||
|---|---|---|---|---|---|---|---|
| Value | −132 | 30.0 | 750 | 65 | 1310.0 | 47.0 | 67.0 |
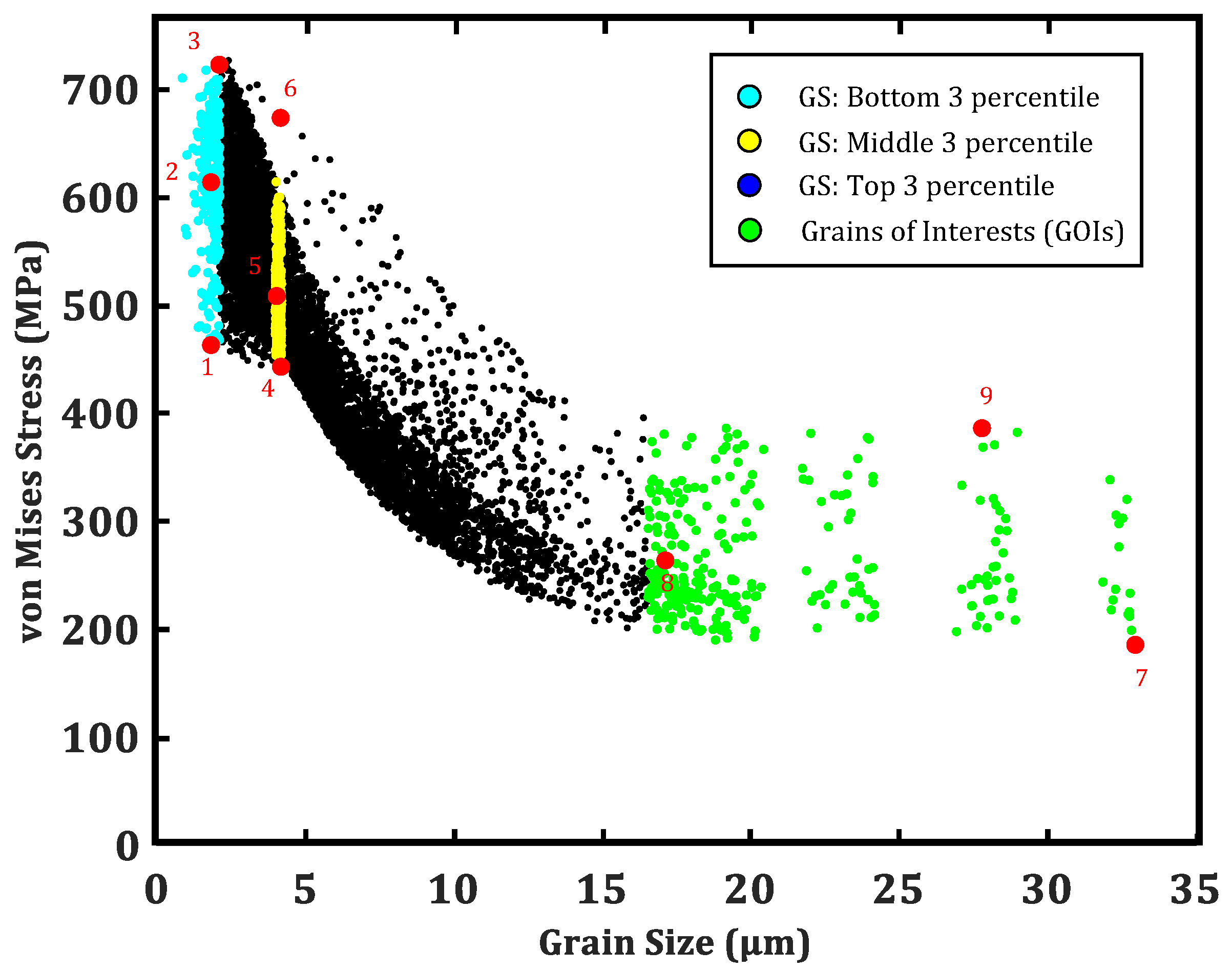
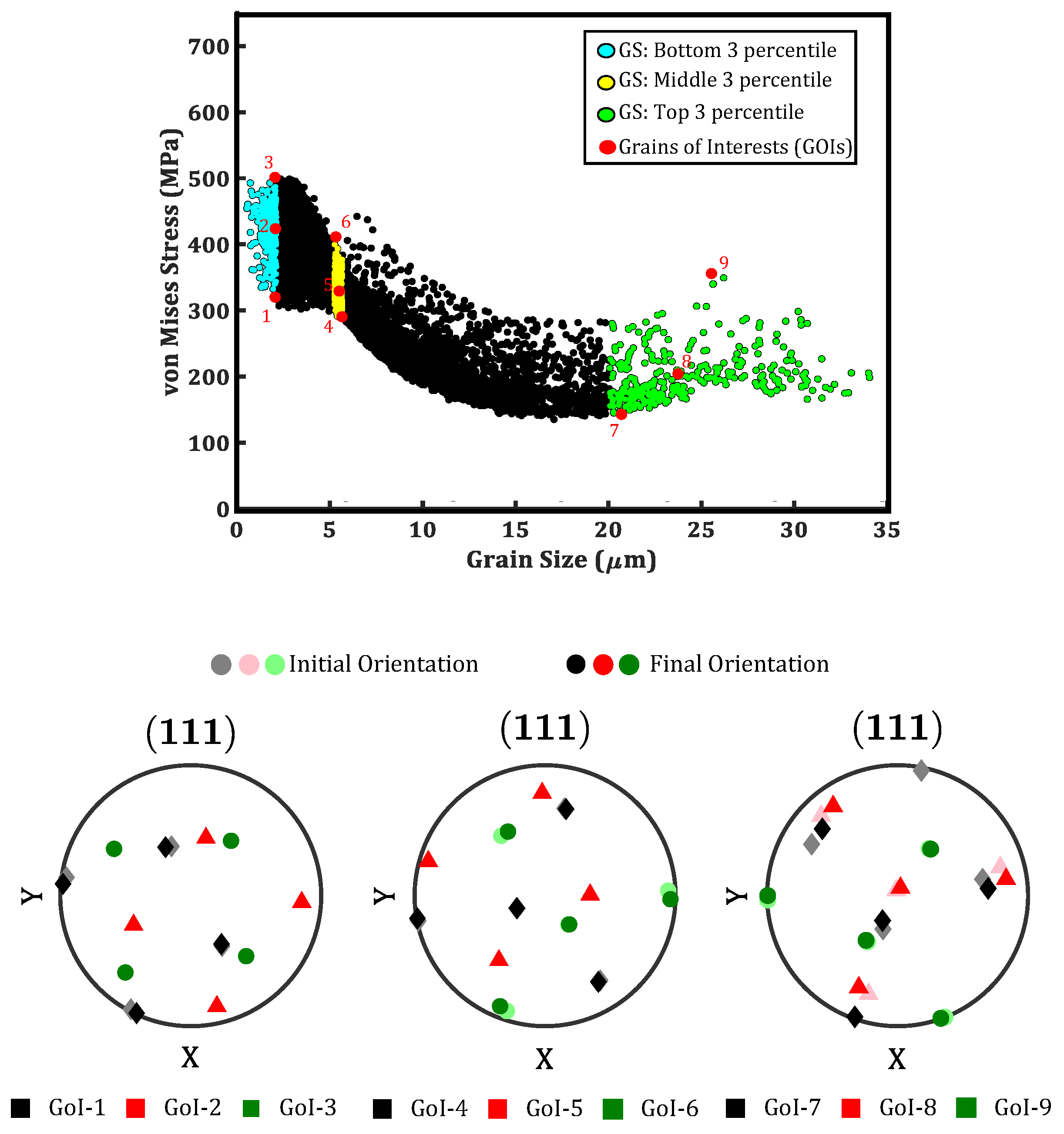
| Grain Size | ||||
|---|---|---|---|---|
| Bottom 3% | Middle 3% | Top 3% | ||
| Stress | Minimum | GoI-1 | GoI-4 | GoI-7 |
| Mean | GoI-2 | GoI-5 | GoI-8 | |
| Maximum | GoI-3 | GoI-6 | GoI-9 | |
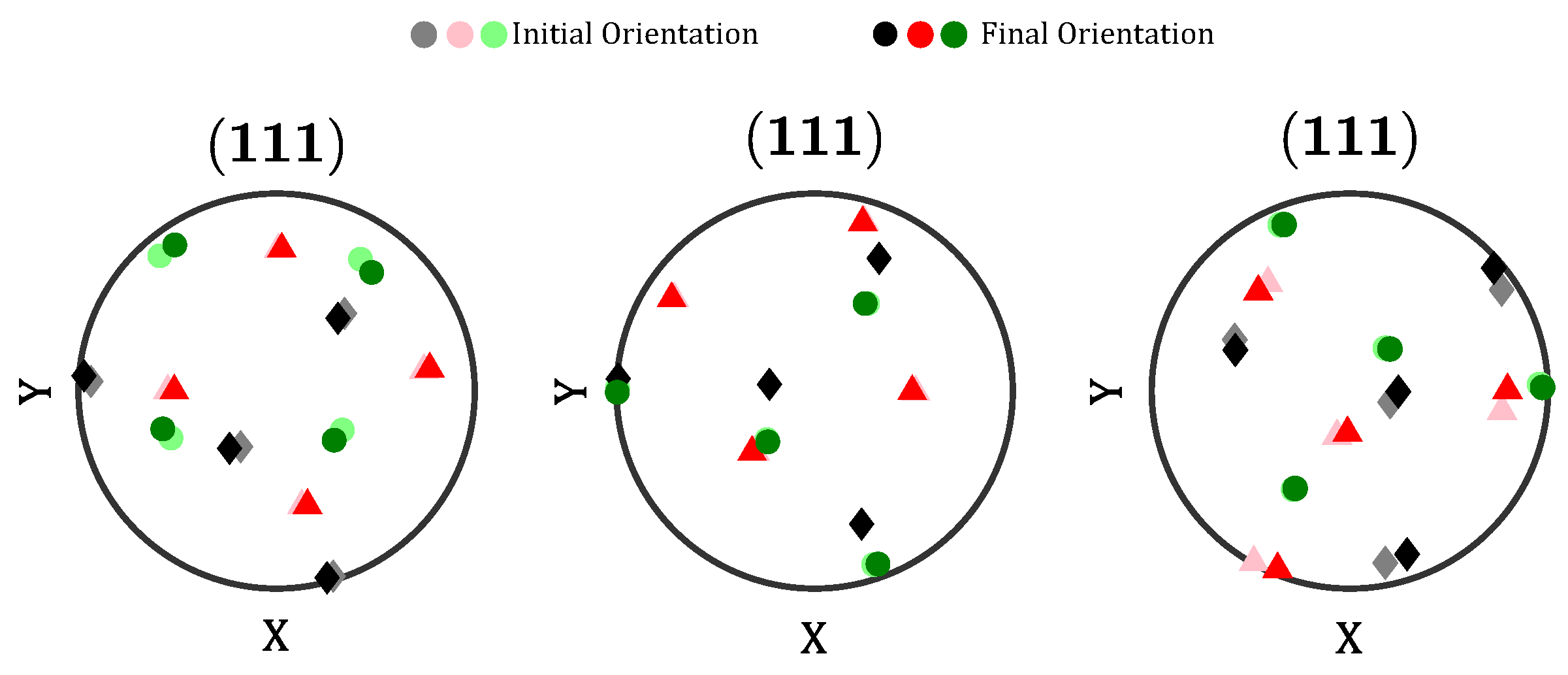
| As-Sprayed | Grain Size (µm) | Stress (MPa) | Initial Orientation | SF (Max) | Final Orientation |
|---|---|---|---|---|---|
| GoI-1 | 1.83 | 463.2 | (313.6, 137.2, 78.3) | 0.354 | (315.7, 135.1, 83.6) |
| GoI-2 | 1.83 | 613.8 | (216.2, 169.1, 166.3) | 0.462 | (210.3, 166.6, 159.9) |
| GoI-3 | 2.11 | 722.5 | (267.5, 65.7, 100.5) | 0.465 | (260.6, 67.34, 101.94) |
| GoI-4 | 4.18 | 442.9 | (124.9, 66.7, 298.4) | 0.315 | (124.9, 66.7, 298.4) |
| GoI-5 | 4.04 | 508.4 | (103.9, 27.9, 97.8) | 0.496 | (106.7, 28.3, 94.9) |
| GoI-6 | 4.17 | 674.0 | (233.5, 82.6, 139.6) | 0.286 | (234.9, 83.1, 139.4) |
| GoI-7 | 32.97 | 185.5 | (49.5, 134.3, 64.1) | 0.497 | (57.5, 137.9, 65.8) |
| GoI-8 | 17.13 | 263.6 | (191.2, 61.9, 204.0) | 0.437 | (197.7, 59.4, 205.1) |
| GoI-9 | 27.8 | 386.0 | (135.9, 130.7, 163.5) | 0.317 | (135.9, 129.5, 164.9) |
| Annealed | Grain Size (µm) | Stress (MPa) | Initial Orientation | SF (Max) | Final Orientation |
| GoI-1 | 2.05 | 320.1 | (29.2, 138.7, 273.1) | 0.373 | (30.9, 136.8, 271.2) |
| GoI-2 | 2.07 | 423.7 | (230.3, 91.6, 333.4) | 0.421 | (230.3, 91.6, 333.4) |
| GoI-3 | 2.04 | 501.3 | (276.6, 96.1, 190.1) | 0.460 | (276.6, 96.1, 190.1) |
| GoI-4 | 5.65 | 290.5 | (321.5, 118.3, 65.8) | 0.391 | (320.5, 118.2, 66.2) |
| GoI-5 | 5.50 | 329.3 | (118.8, 115.6, 346.4) | 0.410 | (118.7, 115.6, 345.9) |
| GoI-6 | 5.32 | 411.43 | (48.6, 47.1, 73.3) | 0.347 | (44.1, 49.3, 74.1) |
| GoI-7 | 20.7 | 142.88 | (207.9, 71.7, 207.8) | 0.479 | (198.3, 67.9, 210.6) |
| GoI-8 | 23.7 | 204.26 | (135.6, 128.2, 227.2) | 0.475 | (130.5, 127.9, 230.5) |
| GoI-9 | 25.54 | 355.91 | (326.1, 135.2, 89.2) | 0.295 | (323.9, 135.1, 88.8) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Williams, A.; Paudel, Y.; Mujahid, S.; Pepi, M.; Czech, P.; El Kadiri, H.; Rhee, H. Crystal Plasticity Modeling to Capture Microstructural Variations in Cold-Sprayed Materials. Crystals 2024, 14, 329. https://doi.org/10.3390/cryst14040329
Williams A, Paudel Y, Mujahid S, Pepi M, Czech P, El Kadiri H, Rhee H. Crystal Plasticity Modeling to Capture Microstructural Variations in Cold-Sprayed Materials. Crystals. 2024; 14(4):329. https://doi.org/10.3390/cryst14040329
Chicago/Turabian StyleWilliams, Aulora, YubRaj Paudel, Shiraz Mujahid, Marc Pepi, Peter Czech, Haitham El Kadiri, and Hongjoo Rhee. 2024. "Crystal Plasticity Modeling to Capture Microstructural Variations in Cold-Sprayed Materials" Crystals 14, no. 4: 329. https://doi.org/10.3390/cryst14040329
APA StyleWilliams, A., Paudel, Y., Mujahid, S., Pepi, M., Czech, P., El Kadiri, H., & Rhee, H. (2024). Crystal Plasticity Modeling to Capture Microstructural Variations in Cold-Sprayed Materials. Crystals, 14(4), 329. https://doi.org/10.3390/cryst14040329









