Ginzburg–Landau Analysis on the Physical Properties of the Kagome Superconductor CsV3Sb5
Abstract
1. Introduction
2. Two-Band Anisotropic Ginzburg–Landau Theory
3. Calculation on the Upper Critical Field of CsVSb
3.1. The Upper Critical Field Parallel to the c-Axis
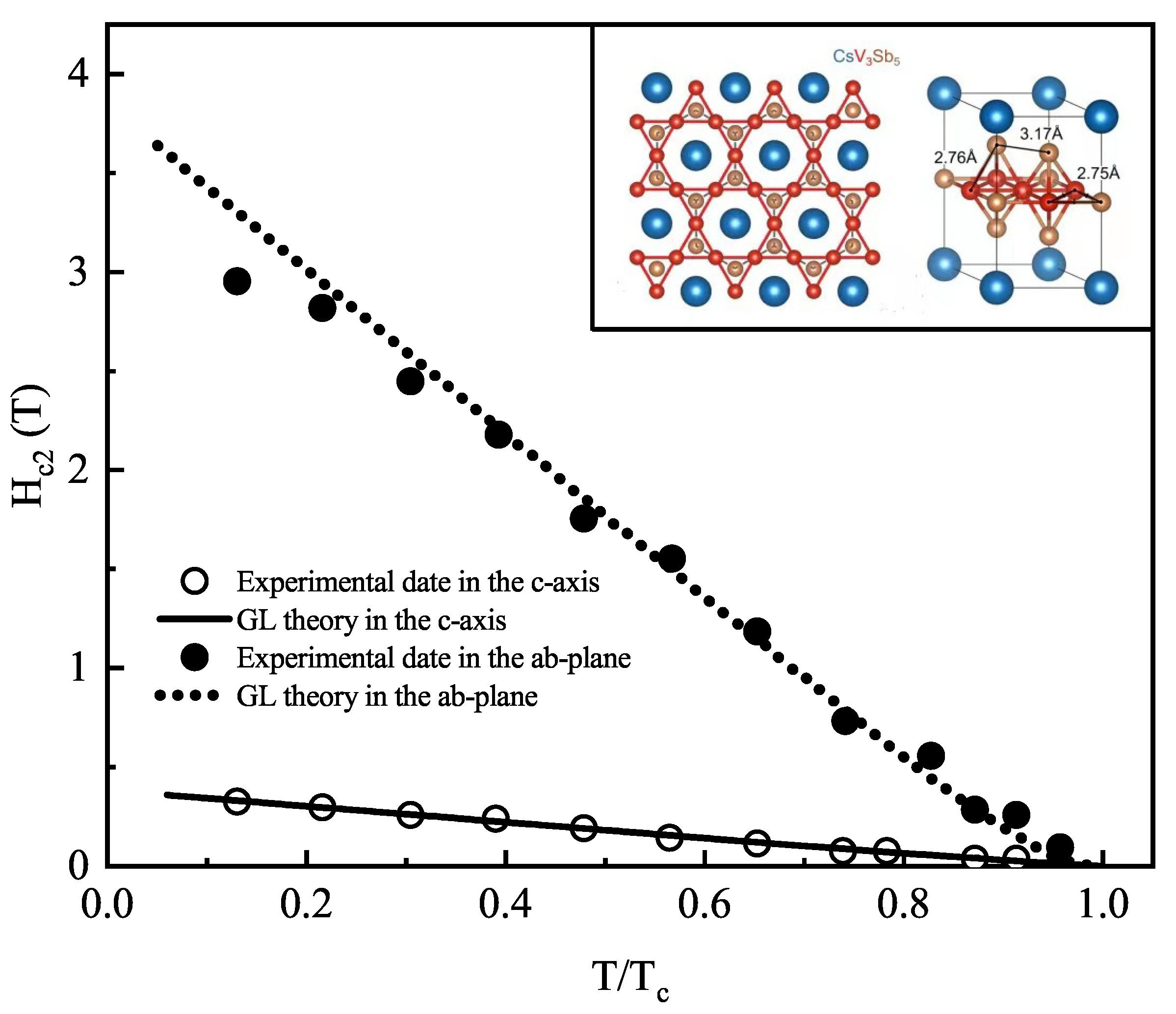
3.2. The Upper Critical Field Parallel to the -Plane
4. Calculation on the Magnetic Penetration Depth of CsVSb
5. KW Ratio and the Semi-Heavy–Fermion System
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ortiz, B.R.; Gomes, L.C.; Morey, J.R.; Winiarski, M.; Oswald, I.W.H.; Mangum, J.S.; Neilson, J.R.; Ertekin, E.; McQueen, T.M.; Toberer, E.S. New kagome prototype materials: Discovery of KV3Sb5, RbV3Sb5 and CsV3Sb5. Phys. Rev. Mater. 2019, 3, 094407. [Google Scholar] [CrossRef]
- Ortiz, B.R.; Teicher, S.M.L.; Hu, Y.; Zuo, J.L.; Sarte, P.M.; Schueller, E.C.; Abeykoon, A.M.M.; Krogstad, M.J.; Rosenkranz, S.; Osborn, R.; et al. CsV3Sb5: A Z2 topological kagome metal with a superconducting ground state. Phys. Rev. Lett. 2020, 125, 247002. [Google Scholar] [CrossRef] [PubMed]
- Ortiz, B.R.; Sarte, P.M.; Kenney, E.M.; Graf, M.J.; Teicher, S.M.L.; Seshadri, R.; Wilson, S.D. Superconductivity in the Z2 kagome metal KV3Sb5. Phys. Rev. Mater. 2021, 5, 034801. [Google Scholar] [CrossRef]
- Yin, Q.W.; Tu, Z.J.; Gong, C.S.; Fu, Y.; Yan, S.H.; Lei, H.C. Superconductivity and normal-state properties of kagome metal RbV3Sb5 single crystals. Chin. Phys. Lett. 2021, 38, 037403. [Google Scholar] [CrossRef]
- Shumiya, N.; Hossain, M.S.; Yin, J.X.; Jiang, Y.X.; Ortiz, B.R.; Liu, H.X.; Shi, Y.G.; Yin, Q.W.; Lei, H.C.; Zhang, S.T.S.; et al. Intrinsic nature of chiral charge order in the kagome superconductor RbV3Sb5. Phys. Rev. B 2021, 104, 035131. [Google Scholar] [CrossRef]
- Guguchia, Z.; Mielke, C., III; Das, D.; Gupta, R.; Yin, J.X.; Liu, H.; Yin, Q.; Christensen, M.H.; Tu, Z.; Gong, C.; et al. Tunable unconventional kagome superconductivity in charge ordered RbV3Sb5 and KV3Sb5. Nat. Commun. 2023, 14, 153. [Google Scholar] [CrossRef]
- Li, H.X.; Zhang, T.T.; Yilmaz, T.; Pai, Y.Y.; Marvinney, C.E.; Said, A.; Yin, Q.W.; Gong, C.S.; Tu, Z.J.; Vescovo, E.; et al. Observation of unconventional charge density wave without acoustic phonon anomaly in kagome superconductors AV3Sb5(A = Rb, Cs). Phys. Rev. X 2021, 11, 031050. [Google Scholar] [CrossRef]
- Liang, Z.W.; Hou, X.Y.; Zhang, F.; Ma, W.R.; Wu, P.; Zhang, Z.Y.; Yu, F.H.; Ying, J.J.; Jiang, K.; Shan, L.; et al. Three-dimensional charge density wave and surface-dependent vortex-core states in a kagome superconductor CsV3Sb5. Phys. Rev. X 2021, 11, 031026. [Google Scholar] [CrossRef]
- Zhao, H.; Li, H.; Ortiz, B.R.; Teicher, S.M.L.; Park, T.; Ye, M.X.; Wang, Z.Q.; Balents, L.; Wilson, S.D.; Zeljkovic, I. Cascade of correlated electron states in the kagome superconductor CsV3Sb5. Nature 2021, 599, 216. [Google Scholar] [CrossRef]
- Ortiz, B.R.; Teicher, S.M.L.; Kautzsch, L.; Sarte, P.M.; Ratcliff, N.; Harter, J.; Ruff, J.P.C.; Seshadri, R.; Wilson, S.D. Fermi surface mapping and the nature of charge-density-wave order in the kagome superconductor CsV3Sb5. Phys. Rev. X 2021, 11, 041030. [Google Scholar] [CrossRef]
- Mu, C.; Yin, Q.W.; Tu, Z.J.; Gong, C.S.; Lei, H.C.; Li, Z.; Luo, J.L. S-wave superconductivity in kagome metal CsV3Sb5 revealed by 121/123Sb NQR and 51V NMR measurements. Chin. Phys. Lett. 2021, 38, 077402. [Google Scholar] [CrossRef]
- Ptok, A.; Kobialka, A.; Sternik, M.; Lazewski, J.; Jochym, P.T.; Oles, M.A.; Piekarz, P. Dynamical study of the origin of the charge density wave in AV3Sb5 (A = K, Rb, Cs) compounds. Phys. Rev. B 2022, 105, 235134. [Google Scholar] [CrossRef]
- Subedi, A. Hexagonal-to-base-centered-orthorhombic 4Q charge density wave order in kagome metals KV3Sb5, RbV3Sb5, and CsV3Sb5. Phys. Rev. Mater. 2022, 6, 015001. [Google Scholar] [CrossRef]
- Wang, Z.X.; Wu, Q.; Yin, Q.W.; Gong, C.S.; Tu, Z.J.; Lin, T.; Liu, Q.M.; Shi, L.Y.; Zhang, S.J.; Wu, D.; et al. Unconventional charge density wave and photoinduced lattice symmetry change in the kagome metal CsV3Sb5 probed by time-resolved spectroscopy. Phys. Rev. B 2021, 104, 165110. [Google Scholar] [CrossRef]
- Ratcliff, N.; Hallett, L.; Ortiz, B.R.; Wilson, S.D.; Harter, J.W. Coherent phonon spectroscopy and interlayer modulation of charge density wave order in the kagome metal CsV3Sb5. Phys. Rev. Mater. 2021, 5, L111801. [Google Scholar] [CrossRef]
- Wulferding, D.; Lee, S.; Choi, Y.; Yin, Q.W.; Tu, Z.J.; Gong, C.S.; Lei, H.C.; Yousuf, S.; Song, J.; Lee, H.; et al. Emergent nematicity and intrinsic versus extrinsic electronic scattering processes in the kagome metal CsV3Sb5. Phys. Rev. Res. 2022, 4, 023215. [Google Scholar] [CrossRef]
- Ni, S.L.; Ma, S.; Zhang, Y.H.; Yuan, J.; Yang, H.T.; Lu, Z.Y.W.; Wang, N.N.; Sun, J.P.; Zhao, Z.; Li, D.; et al. Anisotropic superconducting properties of kagome metal CsV3Sb5. Chin. Phys. Lett. 2021, 38, 057403. [Google Scholar] [CrossRef]
- Tan, H.X.; Liu, Y.Z.; Wang, Z.Q.; Yan, B.H. Charge density waves and electronic properties of superconducting kagome metals. Phys. Rev. Lett. 2021, 127, 046401. [Google Scholar] [CrossRef]
- Chen, H.; Yang, H.T.; Hu, B.; Zhao, Z.; Yuan, J.; Xing, Y.Q.; Qian, G.J.; Huang, Z.H.; Li, G.; Ye, Y.H.; et al. Roton pair density wave in a strong-coupling kagome superconductor. Nature 2021, 599, 222. [Google Scholar] [CrossRef]
- Jiang, Y.X.; Yin, J.X.; Denner, M.M.; Shumiya, N.; Ortiz, B.R.; Xu, G.; Guguchia, Z.; He, Y.Y.; Hossain, M.S.; Liu, X.X.; et al. Unconventional chiral charge order in kagome superconductor KV3Sb5. Nat. Mater. 2021, 20, 1353. [Google Scholar] [CrossRef]
- Du, F.; Luo, S.S.; Ortiz, B.R.; Chen, Y.; Duan, W.Y.; Zhang, D.T.; Lu, X.; Wilson, S.D.; Song, Y.; Yuan, H.Q. Pressure-induced double superconducting domes and charge instability in the kagome metal KV3Sb5. Phys. Rev. B 2021, 103, L220504. [Google Scholar] [CrossRef]
- Uykur, E.; Ortiz, B.R.; Wilson, S.D.; Dressel, M.; Tsirlin, A.A. Optical detection of the density-wave instability in the kagome metal KV3Sb5. NPJ Quantum Mater. 2022, 7, 16. [Google Scholar] [CrossRef]
- Zhao, C.C.; Wang, L.S.; Xia, W.; Yin, Q.W.; Ni, J.M.; Huang, Y.Y.; Tu, C.P.; Tao, Z.C.; Tu, Z.J.; Gong, C.S.; et al. Nodal superconductivity and superconducting domes in the topological kagome metal CsV3Sb5. arXiv 2021, arXiv:abs/2102.08356. [Google Scholar]
- Freitas, R.R.Q.; Rivelino, R.; de Brito Mota, F.; de Castilho, C.M.C.; Kakanakova-Georgieva, A.; Gueorguiev, G.K. Topological insulating phases in two-dimensional bismuth-containing single layers preserved by hydrogenation. J. Phys. Chem. C 2015, 119, 23599. [Google Scholar] [CrossRef]
- Freitas, R.R.Q.; de Brito Mota, F.; Rivelino, R.; de Castilho, C.M.C.; Kakanakova-Georgieva, A.; Gueorguiev, G.K. Spin-orbit-induced gap modification in buckled honeycomb XBi and XBi3 (X = B, Al, Ga, In) sheets. J. Phys. Condens. Matter 2015, 27, 485306. [Google Scholar] [CrossRef] [PubMed]
- Neupert, T.; Denner, M.M.; Yin, J.X.; Thomale, R.; Hasan, M.Z. Charge order and superconductivity in kagome materials. Nat. Phys. 2022, 18, 137. [Google Scholar] [CrossRef]
- Liu, Z.H.; Zhao, N.N.; Yin, Q.W.; Gong, C.S.; Tu, Z.J.; Li, M.; Song, W.H.; Liu, Z.T.; Shen, D.W.; Huang, Y.B.; et al. Charge-density-wave-induced bands renormalization and energy gaps in a kagome superconductor RbV3Sb5. Phys. Rev. X 2021, 11, 041010. [Google Scholar] [CrossRef]
- Chen, X.; Zhan, X.H.; Wang, X.J.; Deng, J.; Liu, X.B.; Chen, X.; Guo, J.G.; Chen, X.L. Highly robust reentrant superconductivity in CsV3Sb5 under pressure. Chin. Phys. Lett. 2021, 38, 057402. [Google Scholar] [CrossRef]
- Ye, L.D.; Kang, M.G.; Liu, J.W.; Von Cube, F.; Wicker, C.R.; Suzuki, T.; Jozwiak, C.; Bostwick, A.; Rotenberg, E.; Bell, D.C.; et al. Massive Dirac fermions in a ferromagnetic kagome metal. Nature 2018, 555, 638. [Google Scholar] [CrossRef]
- Guo, H.M.; Franz, M. Topological insulator on the kagome lattice. Phys. Rev. B 2009, 80, 113102. [Google Scholar] [CrossRef]
- Mazin, I.I.; Jeschke, H.O.; Lechermann, F.; Lee, H.; Fink, M.; Thomale, R.; Valentí, R. Theoretical prediction of a strongly correlated Dirac metal. Nat. Commun. 2014, 5, 4261. [Google Scholar] [CrossRef] [PubMed]
- Duan, W.Y.; Nie, Z.Y.; Luo, S.S.; Yu, F.H.; Ortiz, B.R.; Yin, L.C.; Su, H.; Du, F.; Wang, A.; Chen, Y.; et al. Nodeless superconductivity in the kagome metal CsV3Sb5. Sci. China Phys. Mech. Astron. 2021, 64, 107462. [Google Scholar] [CrossRef]
- Doh, H.; Sigrist, M.; Cho, B.K.; Lee, S.I. Phenomenological theory of superconductivity and magnetism in Ho1−xDyxNi2B2C. Phys. Rev. Lett. 1999, 83, 5350. [Google Scholar] [CrossRef]
- Askerzade, I.N.; Gencer, A.; Güclü, N. On the Ginzburg-Landau analysis of the upper critical field Hc2 in MgB2. Supercond. Sci. Technol. 2002, 15, L13. [Google Scholar] [CrossRef]
- Zhitomirsky, M.E.; Dao, V.H. Ginzburg-Landau theory of vortices in a multigap superconductor. Phys. Rev. B 2004, 69, 054508. [Google Scholar] [CrossRef]
- Fu, Y.; Zhao, N.N.; Chen, Z.; Yin, Q.W.; Tu, Z.J.; Gong, C.S.; Xi, C.Y.; Zhu, X.D.; Sun, Y.P.; Liu, K.; et al. Quantum transport evidence of topological band structures of kagome superconductor CsV3Sb5. Phys. Rev. Lett. 2021, 12, 207002. [Google Scholar] [CrossRef] [PubMed]
- Kong, Y.; Dolgov, O.V.; Jepsen, O.; Andersen, O.K. Electron-phonon interaction in the normal and superconducting states of MgB2. Phys. Rev. B 2001, 64, 020501(R). [Google Scholar] [CrossRef]
- Kresin, V.Z. Transport properties and determination of the basic parameters of superconductors with overlapping bands. J. Low Temp. Phys. 1973, 11, 519. [Google Scholar] [CrossRef]
- Tinkham, M. Introduction to Superconductivity; McGraw-Hill Inc.: New York, NY, USA, 1996. [Google Scholar]
- de Gennes, P.G. Superconductivity of Metals and Alloys; Westview Press: New York, NY, USA, 1966. [Google Scholar]
- Yu, F.H.; Wu, T.; Wang, Z.Y.; Lei, B.; Zhuo, W.Z.; Ying, J.J.; Chen, X.H. Concurrence of anomalous Hall effect and charge density wave in a superconducting topological kagome metal. Phys. Rev. B 2021, 104, L041103. [Google Scholar] [CrossRef]
- Yang, S.Y.; Wang, Y.J.; Ortiz, B.R.; Liu, D.; Gayles, J.; Derunova, E.; Gonzalez-Hernandez, R.; Šmejkal, L.; Chen, Y.L.; Parkin, S.S.P.; et al. Giant, unconventional anomalous Hall effect in the metallic frustrated magnet candidate, KV3Sb5. Sci. Adv. 2020, 6, eabb6003. [Google Scholar] [CrossRef] [PubMed]
- Gupta, R.; Das, D.; Mielke, C.H., III; Guguchia, Z.; Shiroka, T.; Baines, C.; Bartkowiak, M.; Luetkens, H.; Khasanov, R.; Yin, Q.; et al. Microscopic evidence for anisotropic multigap superconductivity in the CsV3Sb5 kagome superconductor. NPJ Quantum Mater. 2022, 7, 49. [Google Scholar] [CrossRef]
- Steglich, F.; Aarts, J.; Bredl, C.D.; Lieke, W.; Meschede, D.; Franz, W.; Schäfer, H. Superconductivity in the presence of strong pauli paramagnetism: CeCu2Si2. Phys. Rev. Lett. 1979, 43, 1892. [Google Scholar] [CrossRef]
- Kadowaki, K.; Woods, S.B. Universal relationship of the resistivity and specific heat in heavy-fermion compounds. Solid State Commun. 1986, 58, 507. [Google Scholar] [CrossRef]
- Jacko, A.C.; Fjærestad, J.O.; Powell, B.J. A unified explanation of the Kadowaki-Woods ratio in strongly correlated metals. Nat. Phys. 2009, 5, 422. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, T.; Che, J.; Ye, C.; Huang, H. Ginzburg–Landau Analysis on the Physical Properties of the Kagome Superconductor CsV3Sb5. Crystals 2023, 13, 321. https://doi.org/10.3390/cryst13020321
Han T, Che J, Ye C, Huang H. Ginzburg–Landau Analysis on the Physical Properties of the Kagome Superconductor CsV3Sb5. Crystals. 2023; 13(2):321. https://doi.org/10.3390/cryst13020321
Chicago/Turabian StyleHan, Tianyi, Jiantao Che, Chenxiao Ye, and Hai Huang. 2023. "Ginzburg–Landau Analysis on the Physical Properties of the Kagome Superconductor CsV3Sb5" Crystals 13, no. 2: 321. https://doi.org/10.3390/cryst13020321
APA StyleHan, T., Che, J., Ye, C., & Huang, H. (2023). Ginzburg–Landau Analysis on the Physical Properties of the Kagome Superconductor CsV3Sb5. Crystals, 13(2), 321. https://doi.org/10.3390/cryst13020321





