Envelope Function Analysis of Quasicrystals
Abstract
:1. Introduction
2. Method of Envelope Function Analysis
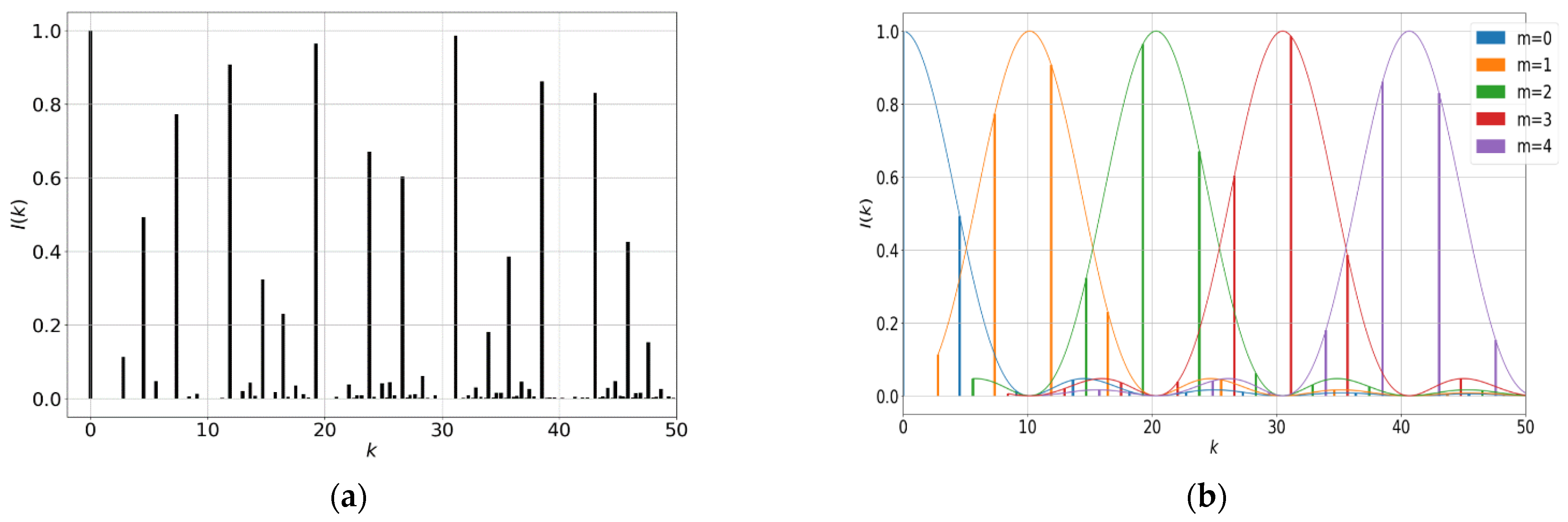
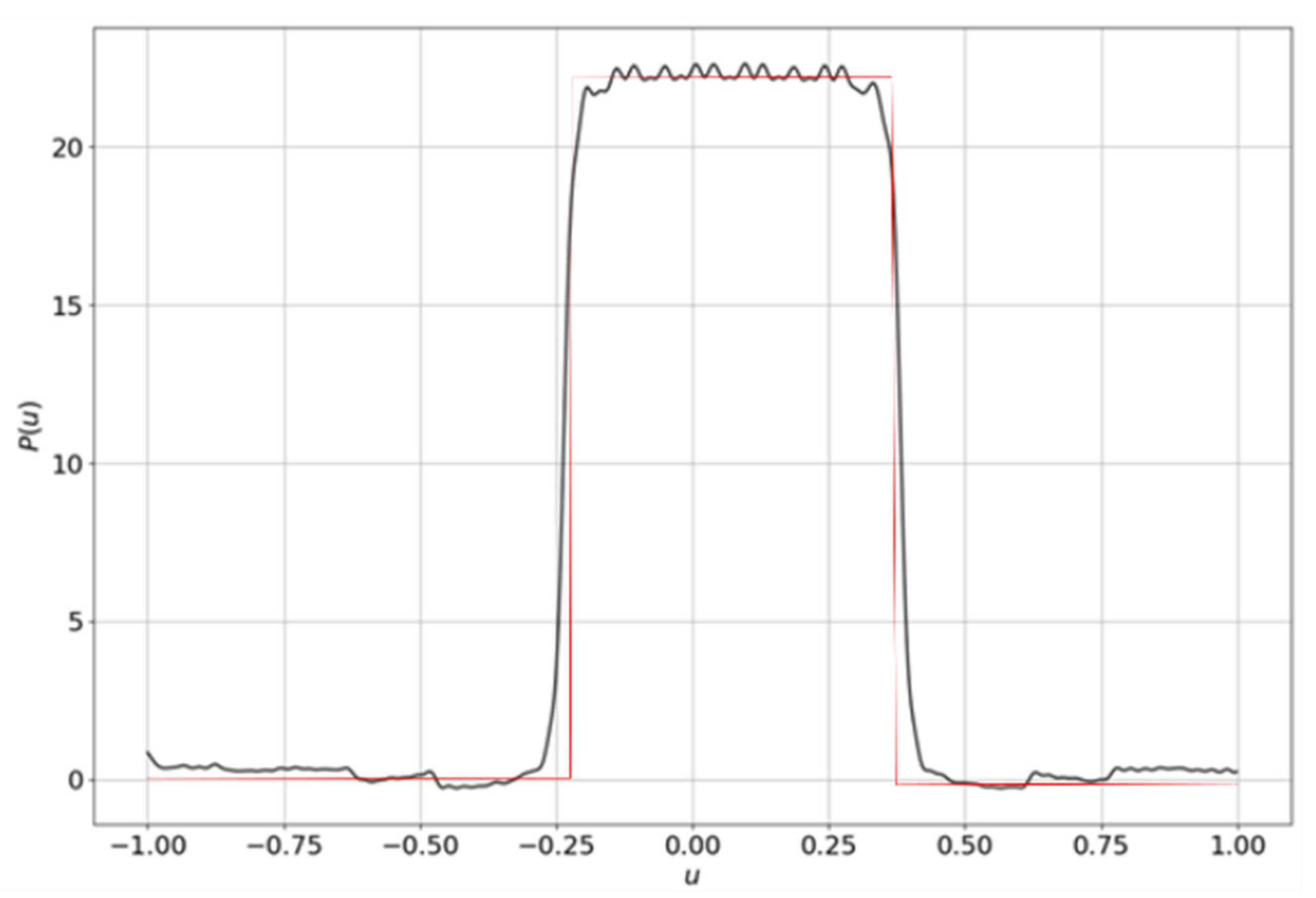
2.1. Model 1D Quasicrystal
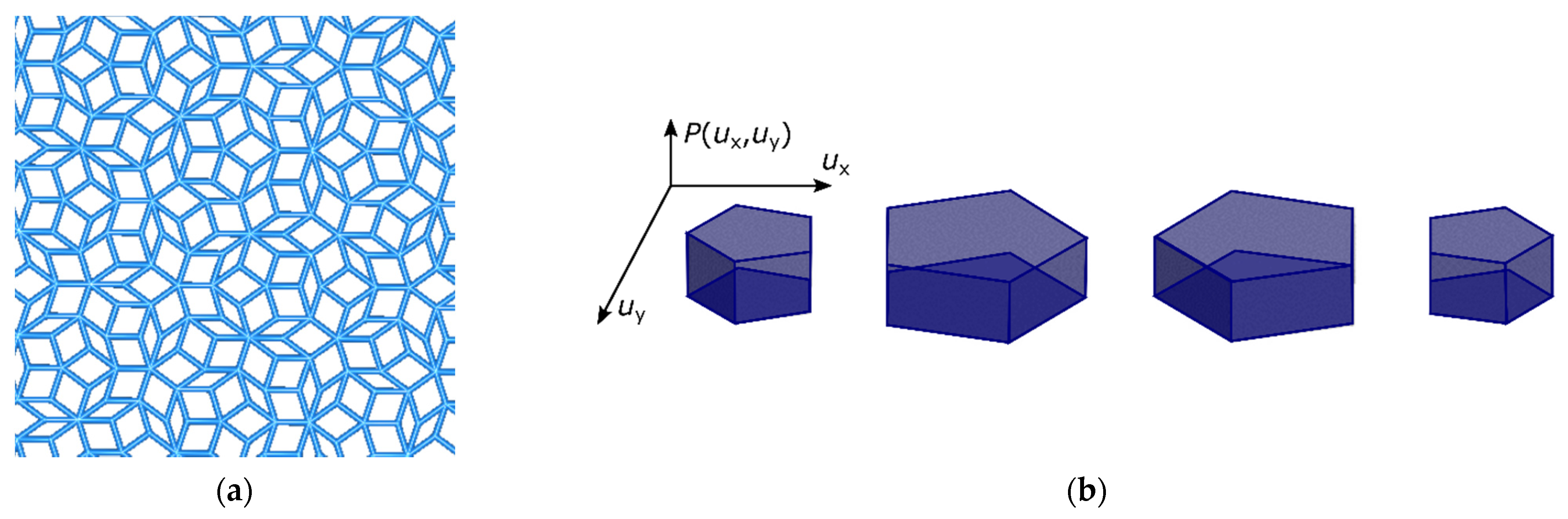
2.2. Penrose Tiling
2.3. Envelope Function Analysis of the Vertex-Decoration Rhombic Penrose Tiling
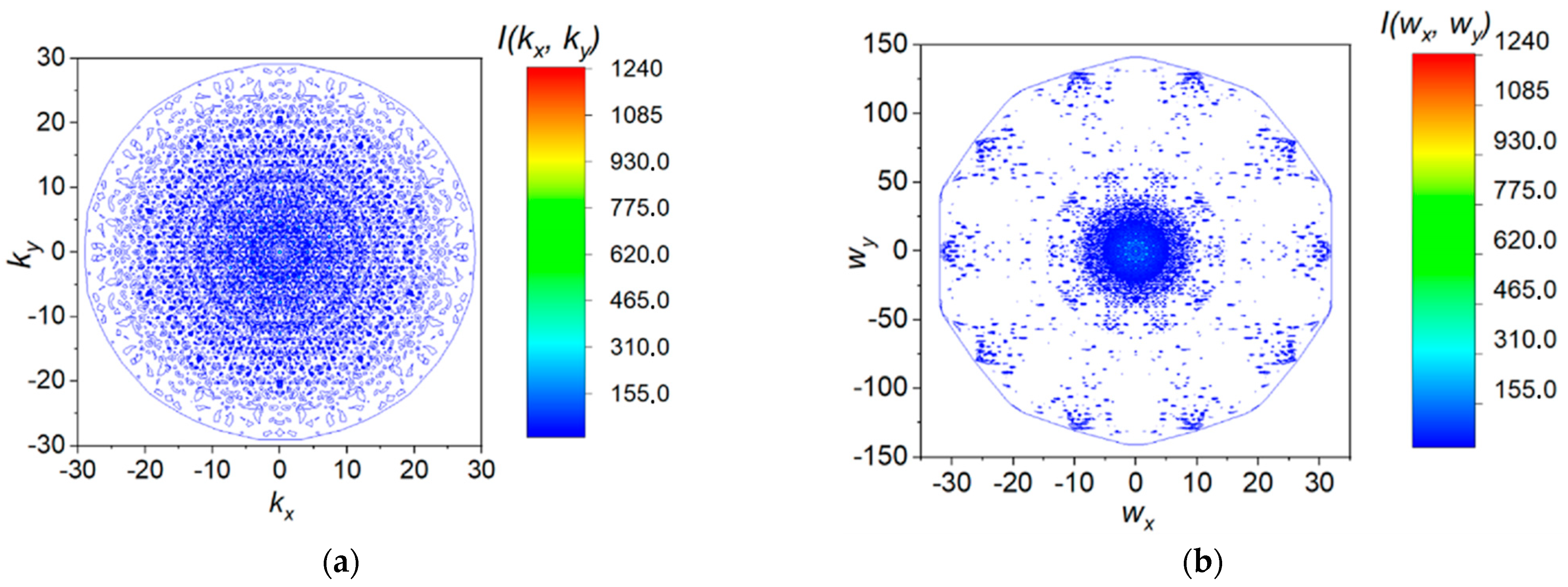
3. Application of the Envelope Function Method to Real Decagonal Quasicrystal
3.1. Decagonal Quasicrystal Al-Cu-Rh
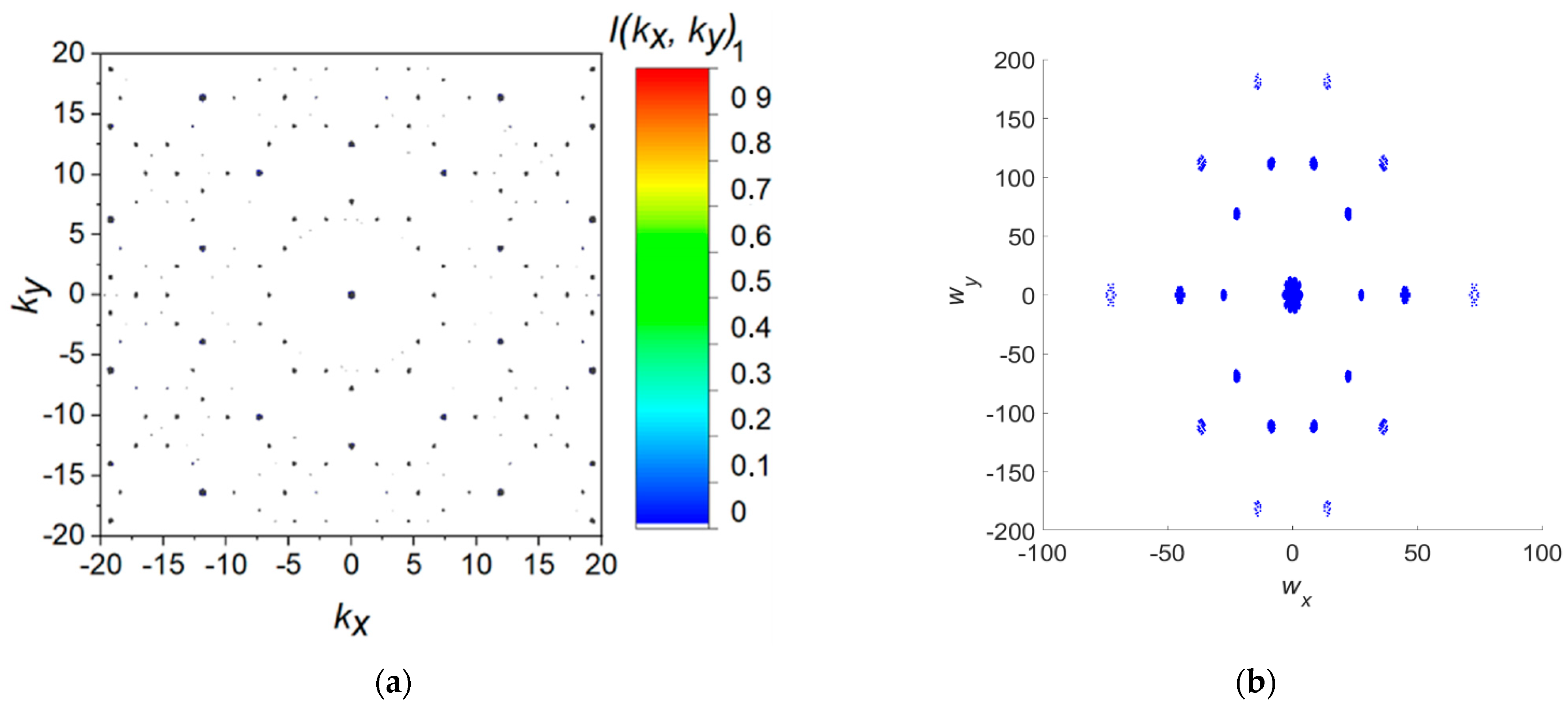
3.2. Envelope Function Analysis of Al-Cu-Rh
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Takakura, H.; Shiono, M.; Sato, T.J.; Yamamoto, A.; Tsai, A.P. Ab initio structure determination of icosahedral Zn-Mg-Ho quasicrystals by density modification method. Phys. Rev. Lett. 2001, 86, 236–239. [Google Scholar] [CrossRef] [PubMed]
- Oszlanyi, G.; Suto, A. Ab initio structure solution by charge flipping. Acta Cryst. A 2004, 60, 134–141. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Palatinus, L. Ab initio determination of incommensurately modulated structures by charge-flipping in superspace. Acta Cryst. A 2004, 60, 604–610. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, A. Software package for structure analysis of quasicrystals. Sci. Tech. Adv. Mat. 2008, 9, 013001. [Google Scholar] [CrossRef] [PubMed]
- Palatinus, L.; Chapuis, G. A computer program for the solution of crystal structures by charge flipping in arbitrary dimensions. J. Appl. Cryst. 2007, 40, 786–790. [Google Scholar] [CrossRef] [Green Version]
- Elser, V. Phase retrieval by iterated projections. J. Opt. Soc. Am. A 2003, 20, 40–55. [Google Scholar] [CrossRef] [Green Version]
- Shechtman, D.S.; Blech, I.; Gratias, D.; Cahn, J. Metallic phase with long-range orientational order and no translational symmetry. Phys. Rev. Lett. 1984, 53, 1951–1953. [Google Scholar] [CrossRef] [Green Version]
- Levine, D.; Steinhardt, P.J. Quasicrystals: A new class of ordered structures. Phys. Rev. Lett. 1984, 53, 2477–2480. [Google Scholar] [CrossRef] [Green Version]
- Socolar, J.E.S.; Steinhardt, P.J. Quasicrystals. II. Unit-cell configurations. Phys. Rev. B 1986, 34, 617–647. [Google Scholar] [CrossRef]
- Dubois, J.M. Useful Quasicrystals; World Scientific: Singapore, 2005. [Google Scholar]
- Zou, Y.; Kuczera, P.; Sologubenko, A.; Sumigawa, T.; Kitamura, T.; Steurer, W.; Spolenak, R. Superior room-temperature ductility of typically brittle quasicrystals at small sizes. Nat. Comm. 2016, 7, 12261. [Google Scholar] [CrossRef] [Green Version]
- Takasaki, A.; Gondek, L.; Czub, J.; Klimkowicz, A.; Zywczak, A.; Swierczek, K. Hydrogen Storage in Ti/Zr-Based Amorphous and Quasicrystal Alloys. In Hydrogen Storage Technologies; Sankir, M., Sankir, N.D., Eds.; Scrivener Publishing LLC: Beverly, MA, USA; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2008; pp. 117–145. [Google Scholar]
- Vardeny, Z.; Nahata, A.; Agrawal, A. Optics of photonic quasicrystals. Nat. Photon. 2014, 7, 177–187. [Google Scholar] [CrossRef]
- Wolny, J.; Kozakowski, B.; Kuczera, P.; Pytlik, L.; Strzałka, R. What periodicities can be found in diffraction patterns of quasicrystals? Acta Cryst. A 2014, 70, 181–185. [Google Scholar] [CrossRef] [PubMed]
- Wolny, J.; Kuczera, P.; Strzalka, R. Periodically distributed objects with quasicrystalline diffraction pattern. Appl. Phys. Lett. 2015, 106, 131905. [Google Scholar] [CrossRef]
- Kozakowski, B.; Wolny, J. Average Unit Cell in Fourier Space and Its Application to Decagonal Quasicrystal. In Aperiodic Crystals; Schmid, S., Withers, R.L., Lifshitz, R., Eds.; Springer Science: Dordrecht, The Netherlands; Business Media: Berlin/Heidelberg, Germany, 2013; pp. 125–132. [Google Scholar]
- Strzalka, R.; Buganski, I.; Wolny, J. Statistical Approach to Diffraction of Periodic and Non-Periodic Crystals—Review. Crystals 2016, 104, 1–19. [Google Scholar]
- Steurer, W.; Deloudi, S. Crystallography of Quasicrystals: Concepts, Methods and Structures; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Baake, M.; Grimm, U. Aperiodic Order Vol. 1: A Mathematical Invitation; Cambridge University Press: Cambridge, UK, 2013; p. 149. [Google Scholar]
- Yamamoto, A. A five-dimensional model of decagonal Al–Pd–Mn quasicrystals. Acta Cryst. A 1993, 49, C337. [Google Scholar] [CrossRef] [Green Version]
- Takakura, H.; Yamamoto, A.; Tsai, A.P. The structure of a decagonal Al72Ni20Co8 quasicrystal. Acta Cryst. A 2001, 57, 576–585. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kuczera, P.; Wolny, J.; Steurer, W. Comparative structural study of decagonal quasicrystals in the systems Al–Cu–Me (Me = Co; Rh; Ir). Acta Cryst. B 2012, 68, 578–589. [Google Scholar] [CrossRef]
- Wolny, J.; Buganski, I.; Strzalka, R. Model refinements of quasicrystals. Cryst. Rev. 2018, 24, 22–64. [Google Scholar] [CrossRef]
- de Wolff, P.M.; Janssen, T.; Janner, A. The superspace groups for incommensurate crystal structures with one-dimensional modulation. Acta Cryst. A 1981, 37, 625–636. [Google Scholar] [CrossRef]
- Duneau, M.; Katz, A. Quasiperiodic patterns. Phys. Rev. Lett. 1985, 54, 2688–2691. [Google Scholar] [CrossRef]
- Yamamoto, A. Crystallography of quasiperiodic crystals. Acta Cryst. A 1996, 52, 509–560. [Google Scholar] [CrossRef]
- Janssen, T.; Chapuis, G.; de Boissieu, M. Aperiodic Crystals: From Modulated Phases to Quasicrystals: Structure and Properties; IUCr Monographs on Crystallography, Oxford Science Publications: Oxford, UK, 2013. [Google Scholar]
- Kozakowski, B.; Wolny, J. Decorated quasicrystals and their diffraction patterns. Philos. Mag. 2005, 86, 549–555. [Google Scholar] [CrossRef]
- Steurer, W. Quasicrystals: What do we know? What do we want to know? What can we know? Acta Cryst. A 2018, 74, 1–11. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wolny, J.; Buganski, I.; Kuczera, P.; Strzalka, R. Pushing the limits of crystallography. J. Appl. Cryst. 2016, 49, 2106–2115. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Strzalka, R.; Buganski, I.; Kuczera, P.; Pytlik, L.; Wolny, J. Atomic Structure of Decagonal Al-Cu-Rh Quasicrystal–Revisited: New Correction for Phonons. Crystals 2019, 9, 78. [Google Scholar] [CrossRef] [Green Version]
- Buganski, I.; Strzalka, R.; Wolny, J. New approach to phason disorder for a decagonal quasicrystal: The moments’ series expansion of the tiling’s distribution function for AlCuRh. J. Appl. Cryst. 2020, 93, 904–913. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Strzałka, R.; Chuchra, Ł.; Wolny, J. Envelope Function Analysis of Quasicrystals. Crystals 2022, 12, 536. https://doi.org/10.3390/cryst12040536
Strzałka R, Chuchra Ł, Wolny J. Envelope Function Analysis of Quasicrystals. Crystals. 2022; 12(4):536. https://doi.org/10.3390/cryst12040536
Chicago/Turabian StyleStrzałka, Radosław, Łukasz Chuchra, and Janusz Wolny. 2022. "Envelope Function Analysis of Quasicrystals" Crystals 12, no. 4: 536. https://doi.org/10.3390/cryst12040536
APA StyleStrzałka, R., Chuchra, Ł., & Wolny, J. (2022). Envelope Function Analysis of Quasicrystals. Crystals, 12(4), 536. https://doi.org/10.3390/cryst12040536





