Phase Diagram of a Strained Ferroelectric Nanowire
Abstract
:1. Introduction
2. Materials and Methods
2.1. Functional
2.2. Phase-Field Simulations
2.3. Atomistic Simulations
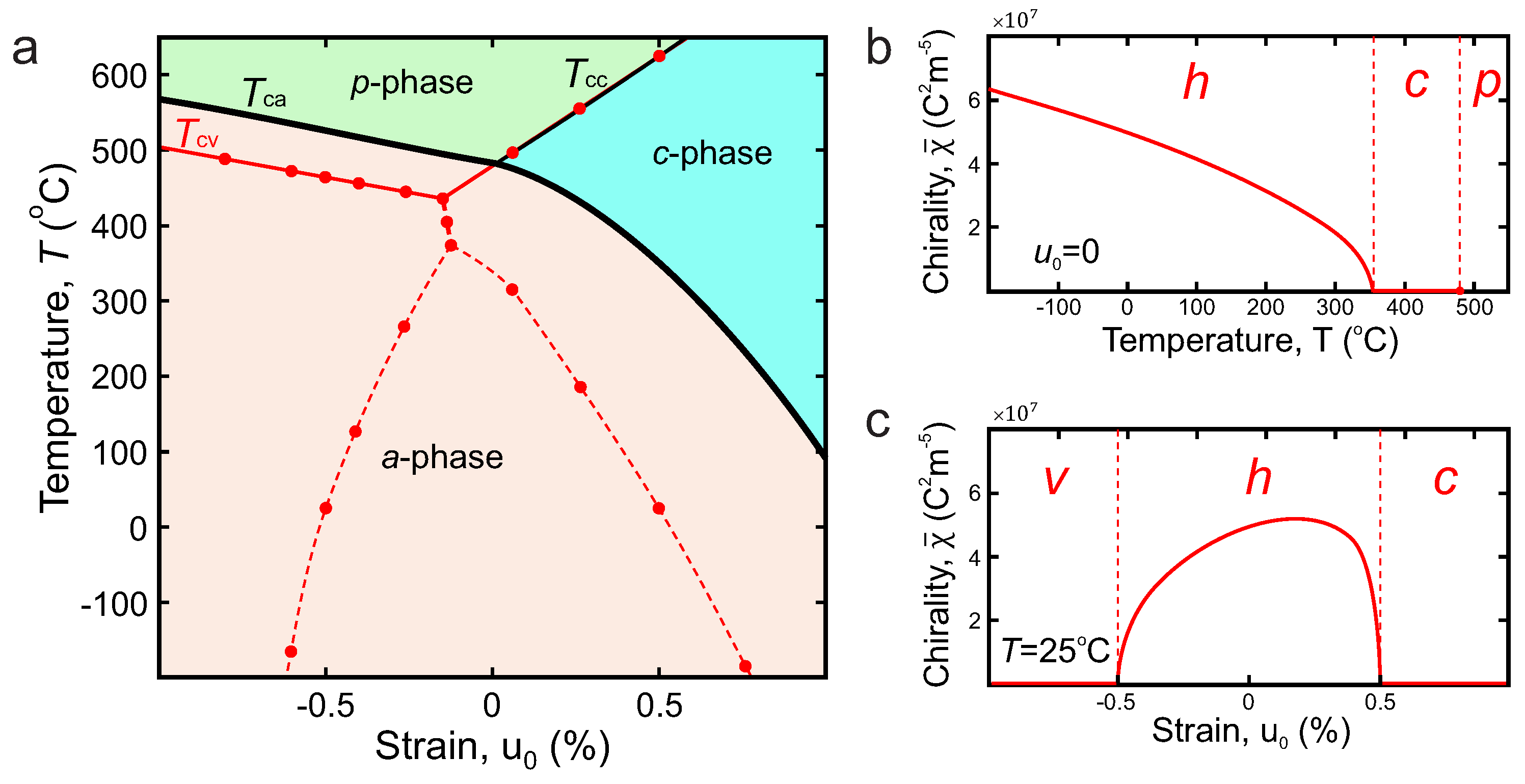
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Oh, S.; Hwang, H.; Yoo, I.K. Ferroelectric materials for neuromorphic computing. APL Mater. 2019, 7, 091109. [Google Scholar] [CrossRef] [Green Version]
- Mikolajick, T.; Schroeder, U.; Slesazeck, S. The Past, the Present, and the Future of Ferroelectric Memories. IEEE Trans. Electron. Devices 2020, 67, 1434–1443. [Google Scholar] [CrossRef]
- Das, S.; Hong, Z.; McCarter, M.; Shafer, P.; Shao, Y.T.; Muller, D.A.; Martin, L.W.; Ramesh, R. A new era in ferroelectrics. APL Mater. 2020, 8, 120902. [Google Scholar] [CrossRef]
- Chen, S.; Yuan, S.; Hou, Z.; Tang, Y.; Zhang, J.; Wang, T.; Li, K.; Zhao, W.; Liu, X.; Chen, L.; et al. Recent progress on topological structures in ferroic thin films and heterostructures. Adv. Mater. 2021, 33, 2000857. [Google Scholar] [CrossRef] [PubMed]
- Tian, G.; Yang, W.; Gao, X.; Liu, J.M. Emerging phenomena from exotic ferroelectric topological states. APL Mater. 2021, 9, 020907. [Google Scholar] [CrossRef]
- Stephanovich, V.A.; Luk’yanchuk, I.A.; Karkut, M.G. Domain-enhanced interlayer coupling in ferroelectric/paraelectric superlattices. Phys. Rev. Lett. 2005, 94, 047601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yadav, A.; Nelson, C.; Hsu, S.; Hong, Z.; Clarkson, J.; Schleputz, C.; Damodaran, A.; Shafer, P.; Arenholz, E.; Dedon, L.; et al. Observation of polar vortices in oxide superlattices. Nature 2016, 530, 198–201. [Google Scholar] [CrossRef]
- Das, S.; Tang, Y.; Hong, Z.; Gonçalves, M.; McCarter, M.; Klewe, C.; Nguyen, K.; Gómez-Ortiz, F.; Shafer, P.; Arenholz, E.; et al. Observation of room-temperature polar skyrmions. Nature 2019, 568, 368–372. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, Y.; Feng, Y.; Zhu, Y.; Tang, Y.; Yang, L.; Zou, M.; Geng, W.; Han, M.; Guo, X.; Wu, B.; et al. Polar meron lattice in strained oxide ferroelectrics. Nat. Mater. 2020, 19, 881–886. [Google Scholar] [CrossRef]
- Naumov, I.; Bratkovsky, A.M. Unusual polarization patterns in flat epitaxial ferroelectric nanoparticles. Phys. Rev. Lett. 2008, 101, 107601. [Google Scholar] [CrossRef] [Green Version]
- Stachiotti, M.G.; Sepliarsky, M. Toroidal ferroelectricity in PbTiO3 nanoparticles. Phys. Rev. Lett. 2011, 106, 137601. [Google Scholar] [CrossRef] [Green Version]
- Mangeri, J.; Espinal, Y.; Jokisaari, A.; Alpay, S.P.; Nakhmanson, S.; Heinonen, O. Topological phase transformations and intrinsic size effects in ferroelectric nanoparticles. Nanoscale 2017, 9, 1616–1624. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kondovych, S.; Pavlenko, M.; Tikhonov, Y.; Razumnaya, A.; Lukyanchuk, I. Vortex states in a ferroelectric cylinder. arXiv 2021, arXiv:2112.10129. [Google Scholar]
- Di Rino, F.; Sepliarsky, M.; Stachiotti, M.G. Topology of the polarization field in PbTiO3 nanoparticles of different shapes by atomic-level simulations. J. Appl. Phys. 2020, 127, 144101. [Google Scholar] [CrossRef]
- Nahas, Y.; Prokhorenko, S.; Louis, L.; Gui, Z.; Kornev, I.; Bellaiche, L. Discovery of stable skyrmionic state in ferroelectric nanocomposites. Nat. Commun. 2015, 6, 8542. [Google Scholar] [CrossRef] [PubMed]
- Tikhonov, Y.; Kondovych, S.; Mangeri, J.; Pavlenko, M.; Baudry, L.; Sené, A.; Galda, A.; Nakhmanson, S.; Heinonen, O.; Razumnaya, A.; et al. Controllable skyrmion chirality in ferroelectrics. Sci. Rep. 2020, 10, 8657. [Google Scholar] [CrossRef]
- Luk’yanchuk, I.; Tikhonov, Y.; Razumnaya, A.; Vinokur, V. Hopfions emerge in ferroelectrics. Nat. Commun. 2020, 11, 2433. [Google Scholar] [CrossRef]
- Alexe, M.; Hesse, D.; Schmidt, V.; Senz, S.; Fan, H.J.; Zacharias, M.; Gösele, U. Ferroelectric nanotubes fabricated using nanowires as positive templates. Appl. Phys. Lett. 2006, 89, 172907. [Google Scholar] [CrossRef]
- Rørvik, P.M.; Grande, T.; Einarsrud, M.A. One-dimensional nanostructures of ferroelectric perovskites. Adv. Mater. 2011, 23, 4007–4034. [Google Scholar] [CrossRef]
- Varghese, J.; Whatmore, R.W.; Holmes, J.D. Ferroelectric nanoparticles, wires and tubes: Synthesis, characterisation and applications. J. Mater. Chem. C 2013, 1, 2618–2638. [Google Scholar] [CrossRef] [Green Version]
- Polking, M.J.; Alivisatos, A.P.; Ramesh, R. Synthesis, physics, and applications of ferroelectric nanomaterials. MRS Commun. 2015, 5, 27–44. [Google Scholar] [CrossRef]
- Liang, L.; Kang, X.; Sang, Y.; Liu, H. One-dimensional ferroelectric nanostructures: Synthesis, properties, and applications. Adv. Sci. 2016, 3, 1500358. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xia, W.; Lu, Y.; Zhu, X. Preparation Methods of Perovskite-Type Oxide Materials. In Revolution of Perovskite; Springer: Berlin/Heidelberg, Germany, 2020; pp. 61–93. [Google Scholar] [CrossRef]
- Morozovska, A.N.; Eliseev, E.A.; Glinchuk, M.D. Ferroelectricity enhancement in confined nanorods: Direct variational method. Phys. Rev. B 2006, 73, 214106. [Google Scholar] [CrossRef]
- Morozovska, A.N.; Glinchuk, M.D.; Eliseev, E.A. Phase transitions induced by confinement of ferroic nanoparticles. Phys. Rev. B 2007, 76, 014102. [Google Scholar] [CrossRef] [Green Version]
- Ma, W. Surface tension and Curie temperature in ferroelectric nanowires and nanodots. Appl. Phys. A 2009, 96, 915–920. [Google Scholar] [CrossRef]
- Wang, J.; Tagantsev, A.K.; Setter, N. Effective-surface-energy approach for size effects in ferroics. Phys. Rev. B 2015, 91, 125432. [Google Scholar] [CrossRef]
- Naumov, I.I.; Bellaiche, L.; Fu, H. Unusual phase transitions in ferroelectric nanodisks and nanorods. Nature 2004, 432, 737. [Google Scholar] [CrossRef]
- Lahoche, L.; Luk’yanchuk, I.; Pascoli, G. Stability of vortex phases in ferroelectric easy-plane nano-cylinders. Integr. Ferroelectr. 2008, 99, 60–66. [Google Scholar] [CrossRef] [Green Version]
- Liu, Z.; Yang, B.; Cao, W.; Fohtung, E.; Lookman, T. Enhanced Energy Storage with Polar Vortices in Ferroelectric Nanocomposites. Phys. Rev. Appl. 2017, 8, 034014. [Google Scholar] [CrossRef]
- Morozovska, A.N.; Hertel, R.; Cherifi-Hertel, S.; Reshetnyak, V.Y.; Eliseev, E.A.; Evans, D.R. Chiral polarization textures induced by the flexoelectric effect in ferroelectric nanocylinders. Phys. Rev. B 2021, 104, 054118. [Google Scholar] [CrossRef]
- Haun, M.J.; Furman, E.; Jang, S.; McKinstry, H.; Cross, L. Thermodynamic theory of PbTiO3. J. Appl. Phys. 1987, 62, 3331–3338. [Google Scholar] [CrossRef]
- Baudry, L.; Lukyanchuk, I.; Vinokur, V.M. Ferroelectric symmetry-protected multibit memory cell. Sci. Rep. 2017, 7, 42196. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, J.; Shi, S.Q.; Chen, L.Q.; Li, Y.; Zhang, T.Y. Phase-field simulations of ferroelectric/ferroelastic polarization switching. Acta Mater. 2004, 52, 749–764. [Google Scholar] [CrossRef]
- Luk’yanchuk, I.A.; Lahoche, L.; Sené, A. Universal properties of ferroelectric domains. Phys. Rev. Lett. 2009, 102, 147601. [Google Scholar] [CrossRef]
- Chen, L.Q. Landau Free-Energy Coefficients. In Physics of Ferroelectrics: A Modern Perspective; Rabe, K.M., Dawber, M., Lichtensteiger, C., Ahn, C.H., Triscone, J.M., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 363–369. [Google Scholar]
- Mokrý, P.; Sluka, T. Identification of defect distribution at ferroelectric domain walls from evolution of nonlinear dielectric response during the aging process. Phys. Rev. B 2016, 93, 064114. [Google Scholar] [CrossRef] [Green Version]
- Logg, A.; Mardal, K.A.; Wells, G.N. (Eds.) Automated Solution of Differential Equations by the Finite Element Method. Volume 84: Lecture Notes in Computational Science and Engineering; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]
- Devonshire, A.F. XCVI. Theory of barium titanate: Part I. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1949, 40, 1040–1063. [Google Scholar] [CrossRef]
- Janelli, A.; Fazio, R. Adaptive stiff solvers at low accuracy and complexity. J. Comput. Appl. Math. 2006, 191, 246. [Google Scholar] [CrossRef] [Green Version]
- Balay, S.; Abhyankar, S.; Adams, M.F.; Benson, S.; Brown, J.; Brune, P.; Buschelman, K.; Constantinescu, E.; Dalcin, L.; Dener, A.; et al. PETSc Web Page. 2021. Available online: https://petsc.org/ (accessed on 3 March 2022).
- Balay, S.; Abhyankar, S.; Adams, M.F.; Benson, S.; Brown, J.; Brune, P.; Buschelman, K.; Constantinescu, E.; Dalcin, L.; Dener, A.; et al. PETSc/TAO Users Manual; Technical Report ANL-21/39; Revision 3.16; Argonne National Laboratory: Lemont, IL, USA, 2021. [Google Scholar]
- Geuzaine, C.; Remacle, J.F. Gmsh: A three-dimensional finite element mesh generator with built-in pre- and post-processing facilities. Int. J. Numer. Methods Eng. 2009, 79, 1039–1331. [Google Scholar] [CrossRef]
- Tinte, S.; Stachiotti, M.G.; Phillpot, S.R.; Sepliarsky, M.; Wolf, D.; Migoni, R.L. Ferroelectric properties of BaxSr1−xTiO3 solid solutions obtained by molecular dynamics simulation. J. Phys. Condens. Matter 2004, 16, 3495–3506. [Google Scholar] [CrossRef]
- Sepliarsky, M.; Cohen, R.E. First-principles based atomistic modeling of phase stability in PMN–xPT. J. Phys. Condens. Matter 2011, 23, 435902. [Google Scholar] [CrossRef] [Green Version]
- Machado, R.; Di Loreto, A.; Frattini, A.; Sepliarsky, M.; Stachiotti, M. Site occupancy effects of Mg impurities in BaTiO3. J. Alloys Compd. 2019, 809, 151847. [Google Scholar] [CrossRef]
- Dick, B.G.; Overhauser, A.W. Theory of the Dielectric Constants of Alkali Halide Crystals. Phys. Rev. 1958, 112, 90–103. [Google Scholar] [CrossRef]
- Sepliarsky, M.; Wu, Z.; Asthagiri, A.; Cohen, R. Atomistic model potential for PbTiO3 and PMN by fitting first principles results. Ferroelectrics 2004, 301, 55–59. [Google Scholar] [CrossRef]
- Sepliarsky, M.; Asthagiri, A.; Phillpot, S.; Stachiotti, M.; Migoni, R. Atomic-level simulation of ferroelectricity in oxide materials. Curr. Opin. Solid State Mater. Sci. 2005, 9, 107–113. [Google Scholar] [CrossRef]
- Sepliarsky, M.; Stachiotti, M.G.; Migoni, R.L. Interface Effects in Ferroelectric PbTiO3 Ultrathin Films on a Paraelectric Substrate. Phys. Rev. Lett. 2006, 96, 137603. [Google Scholar] [CrossRef] [Green Version]
- Todorov, I.T.; Smith, W.; Trachenko, K.; Dove, M.T. DL_POLY_3: New dimensions in molecular dynamics simulations via massive parallelism. J. Mater. Chem. 2006, 16, 1911–1918. [Google Scholar] [CrossRef] [Green Version]
- Pertsev, N.A.; Zembilgotov, A.G.; Tagantsev, A.K. Effect of Mechanical Boundary Conditions on Phase Diagrams of Epitaxial Ferroelectric Thin Films. Phys. Rev. Lett. 1998, 80, 1988–1991. [Google Scholar] [CrossRef]
- Tagantsev, A.K.; Cross, L.E.; Fousek, J. Domains in Ferroic Crystals and Thin Films; Springer: Berlin/Heidelberg, Germany, 2010; Volume 13. [Google Scholar]
- Hooton, J.A.; Merz, W.J. Etch Patterns and Ferroelectric Domains in BaTiO3 Single Crystals. Phys. Rev. 1955, 98, 409. [Google Scholar] [CrossRef]
- Hlinka, J.; Marton, P. Phenomenological model of a 90° domain wall in BaTiO3-type ferroelectrics. Phys. Rev. B 2006, 74, 104104. [Google Scholar] [CrossRef]
- Baudry, L.; Sené, A.; Luk’yanchuk, I.A.; Lahoche, L.; Scott, J.F. Polarization vortex domains induced by switching electric field in ferroelectric films with circular electrodes. Phys. Rev. B 2014, 90, 024102. [Google Scholar] [CrossRef] [Green Version]
- Martelli, P.W.; Mefire, S.M.; Luk’yanchuk, I.A. Multidomain switching in the ferroelectric nanodots. Europhys. Lett. 2015, 111, 50001. [Google Scholar] [CrossRef] [Green Version]
- Chen, P.; Tan, C.; Jiang, Z.; Gao, P.; Sun, Y.; Li, X.; Zhu, R.; Liao, L.; Hou, X.; Wang, L.; et al. Manipulation of polar vortex chirality in oxide superlattices. arXiv 2021, arXiv:2104.12310. [Google Scholar]
- Behera, P.; May, M.A.; Gómez-Ortiz, F.; Susarla, S.; Das, S.; Nelson, C.T.; Caretta, L.; Hsu, S.L.; McCarter, M.R.; Savitzky, B.H.; et al. Electric field control of chirality. Sci. Adv. 2022, 8, eabj8030. [Google Scholar] [CrossRef] [PubMed]

| Coefficient | Analytical | Numerical, PTO | Units |
|---|---|---|---|
| 3.8 ( C) × 10 | C m N | ||
| , | 3.25 × 10 0.047 p | C m N | |
| 11 × 10 0.004 p | C m N | ||
| , | −0.03 × 10 | C m N | |
| 0.42 × 10 | C m N | ||
| , | 0.71 × 10 | C m N | |
| 0.83 × 10 | C m N | ||
| , , | 0.26 × 10 | C m N | |
| , , | 0.61 × 10 | C m N | |
| −3.7 × 10 | C m N |
| Analytical | Numerical, PTO | |
|---|---|---|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pavlenko, M.A.; Di Rino, F.; Boron, L.; Kondovych, S.; Sené, A.; Tikhonov, Y.A.; Razumnaya, A.G.; Vinokur, V.M.; Sepliarsky, M.; Lukyanchuk, I.A. Phase Diagram of a Strained Ferroelectric Nanowire. Crystals 2022, 12, 453. https://doi.org/10.3390/cryst12040453
Pavlenko MA, Di Rino F, Boron L, Kondovych S, Sené A, Tikhonov YA, Razumnaya AG, Vinokur VM, Sepliarsky M, Lukyanchuk IA. Phase Diagram of a Strained Ferroelectric Nanowire. Crystals. 2022; 12(4):453. https://doi.org/10.3390/cryst12040453
Chicago/Turabian StylePavlenko, Maksim A., Franco Di Rino, Leo Boron, Svitlana Kondovych, Anaïs Sené, Yuri A. Tikhonov, Anna G. Razumnaya, Valerii M. Vinokur, Marcelo Sepliarsky, and Igor A. Lukyanchuk. 2022. "Phase Diagram of a Strained Ferroelectric Nanowire" Crystals 12, no. 4: 453. https://doi.org/10.3390/cryst12040453
APA StylePavlenko, M. A., Di Rino, F., Boron, L., Kondovych, S., Sené, A., Tikhonov, Y. A., Razumnaya, A. G., Vinokur, V. M., Sepliarsky, M., & Lukyanchuk, I. A. (2022). Phase Diagram of a Strained Ferroelectric Nanowire. Crystals, 12(4), 453. https://doi.org/10.3390/cryst12040453







