Crystal Plasticity Modeling of Grey Cast Irons under Tension, Compression and Fatigue Loadings
Abstract
:1. Introduction
2. Materials and Methods
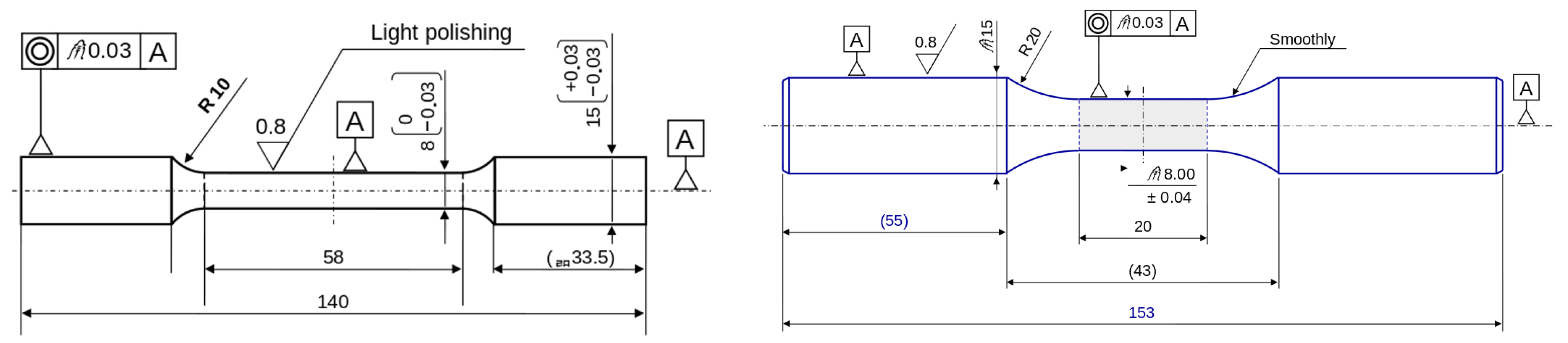
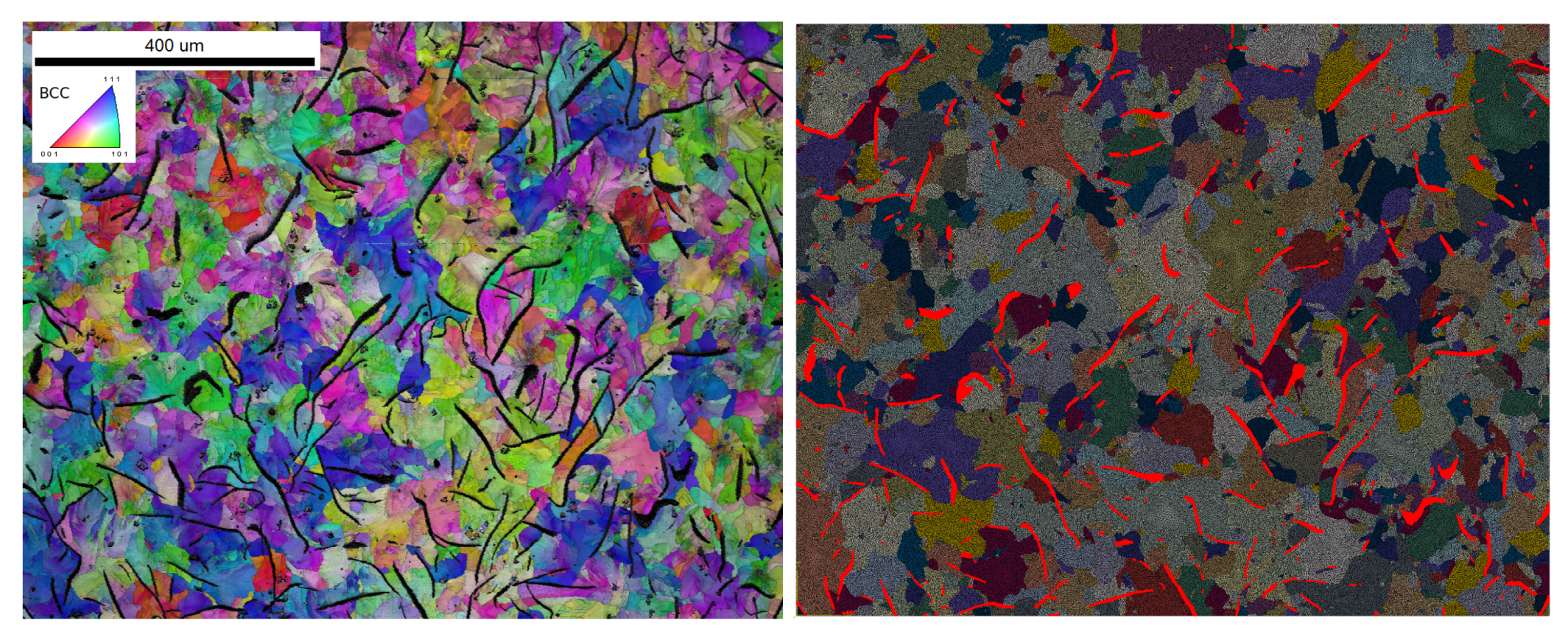
2.1. Material and Experimental Conditions
2.2. Crystal Plasticity Modeling
2.3. Damage Extension
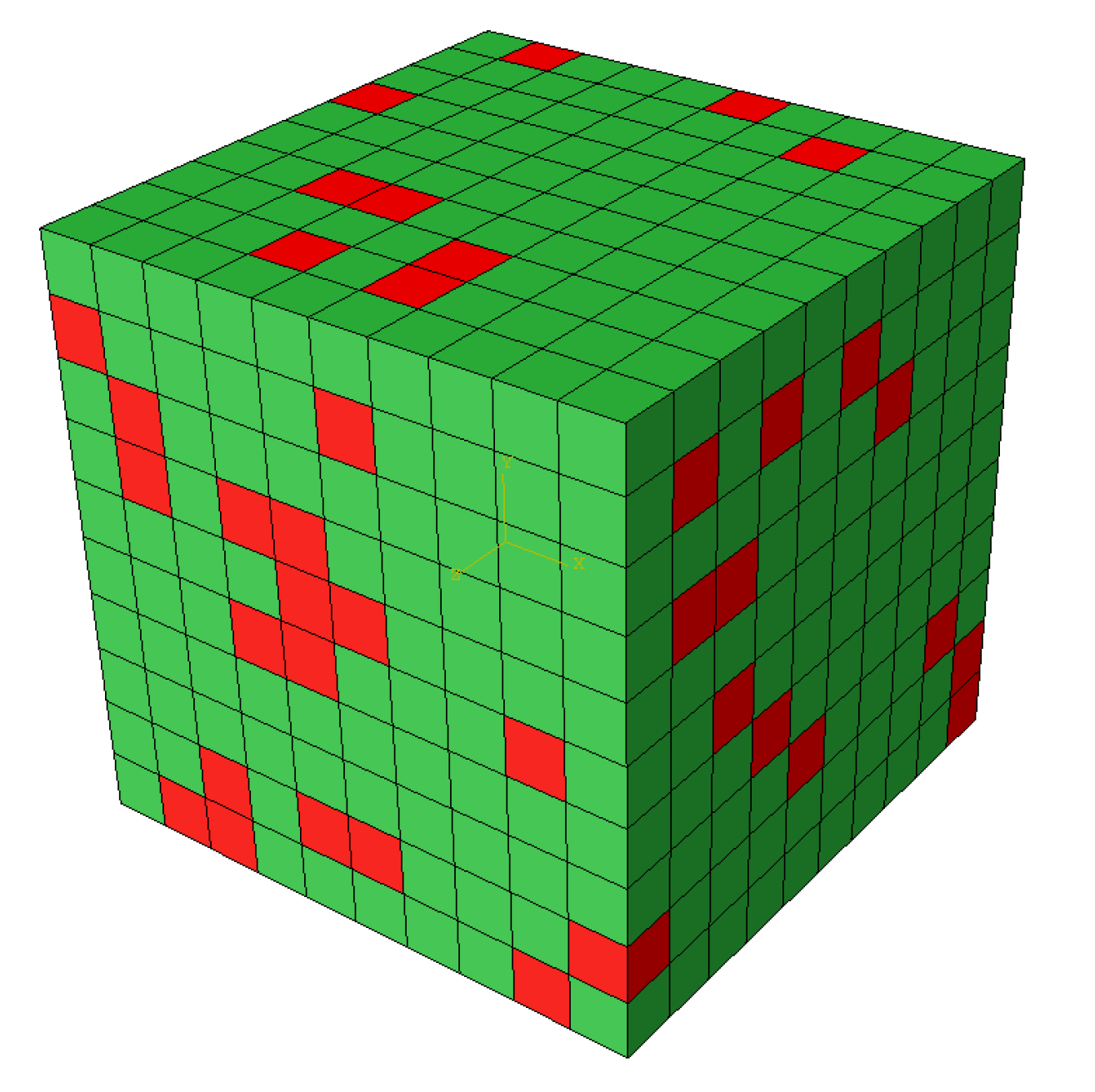
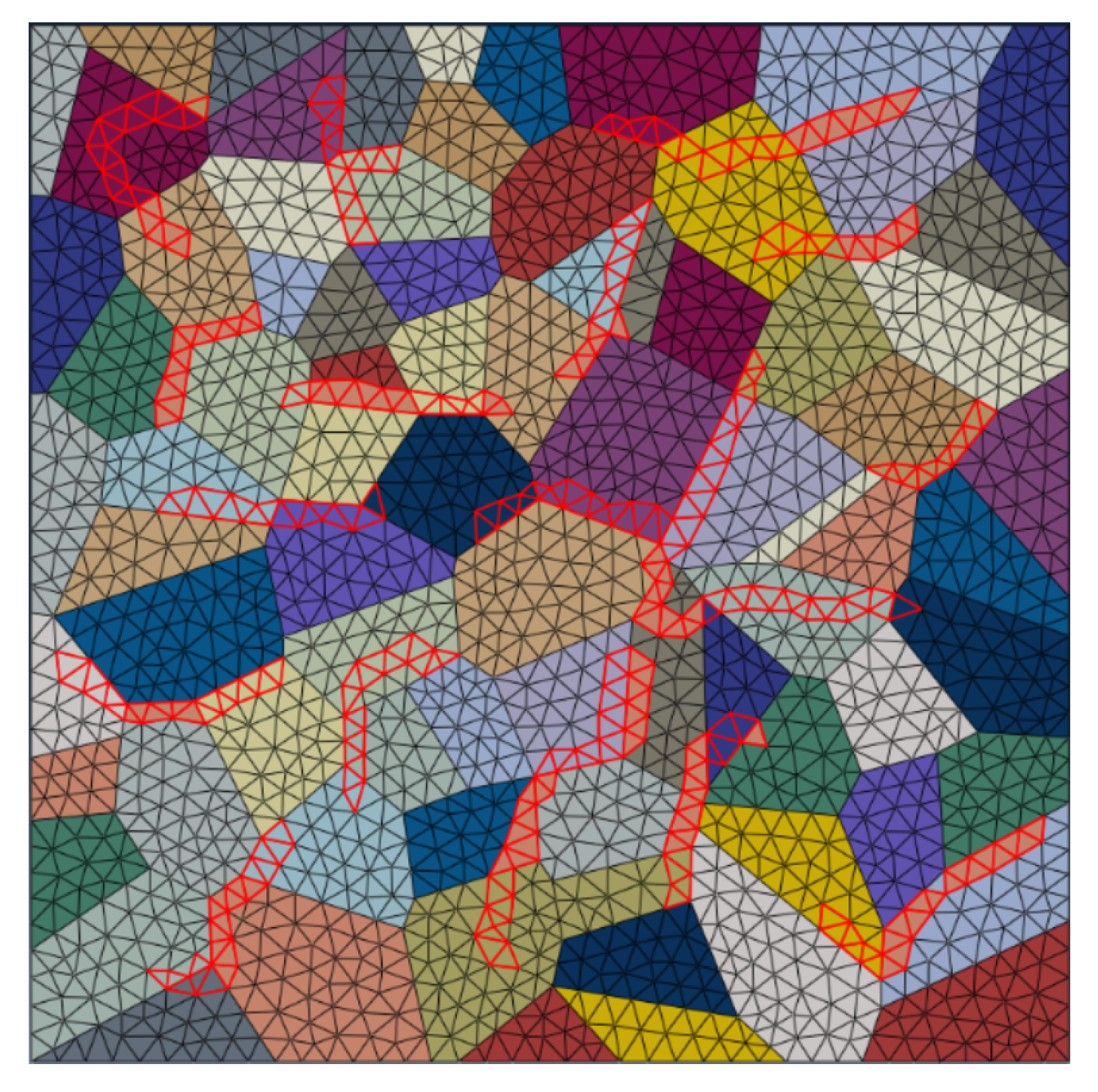
2.4. Finite-Element Models
3. Results and Discussion
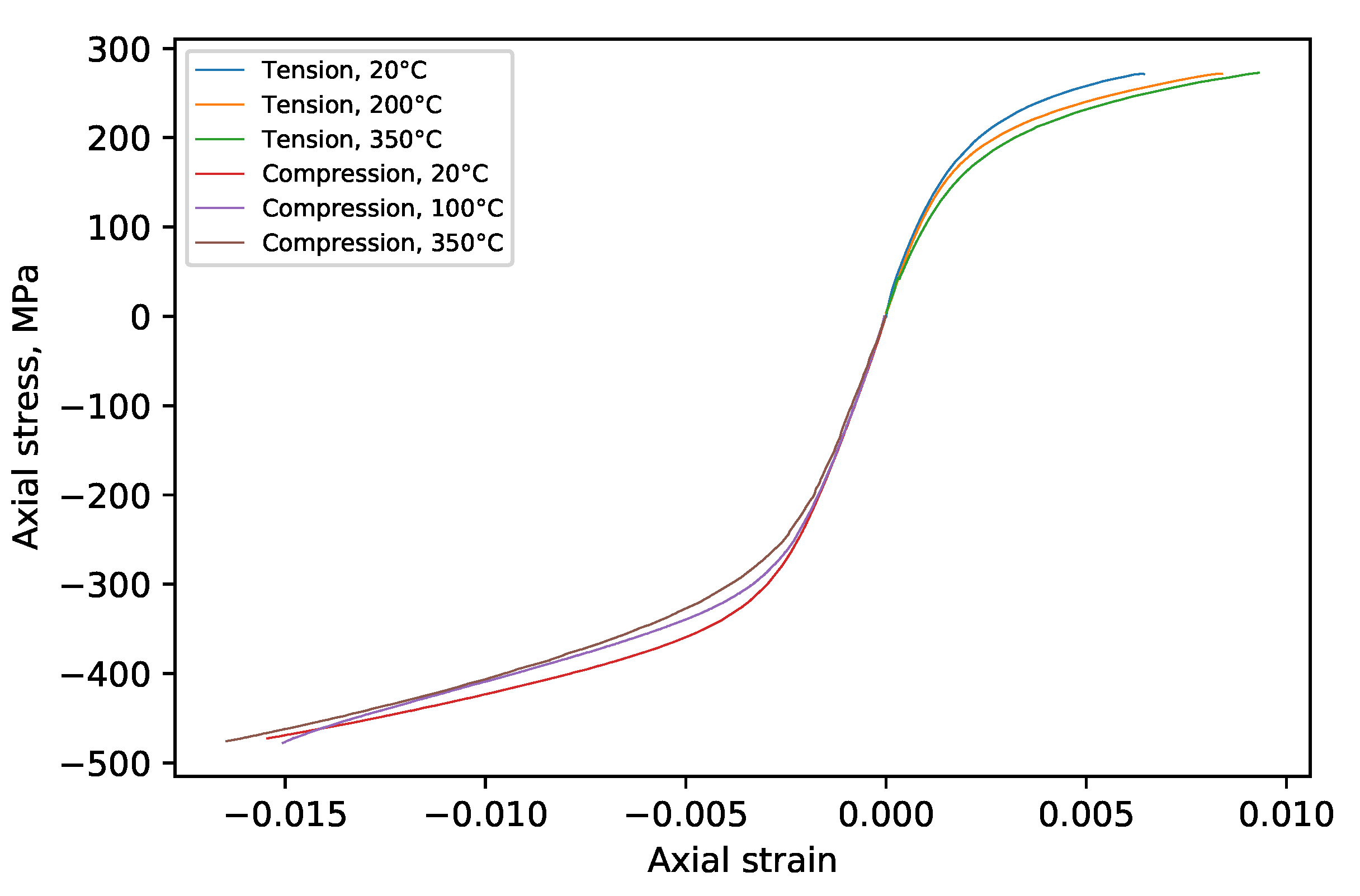
3.1. Experimental Results
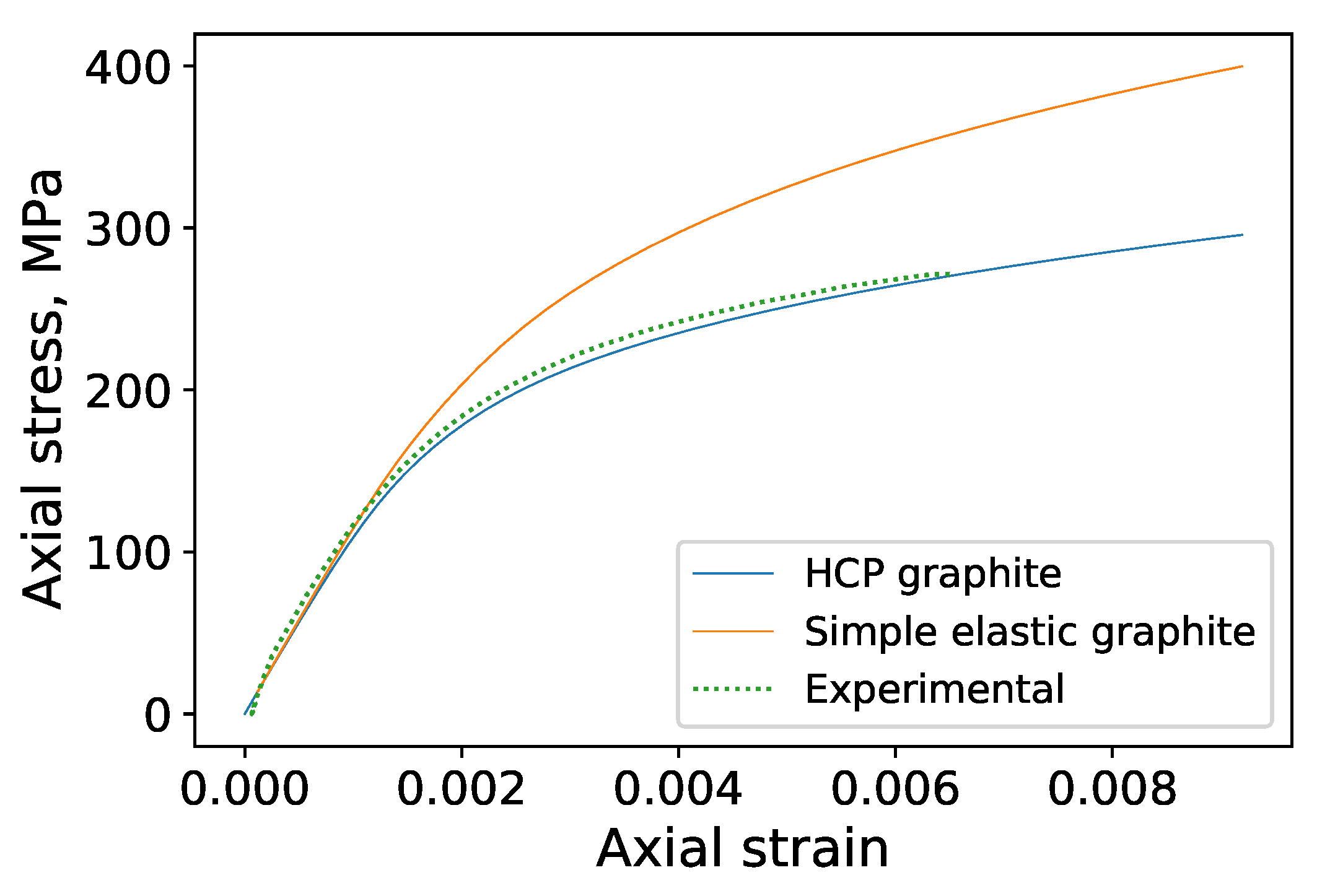
3.2. Model of Graphite
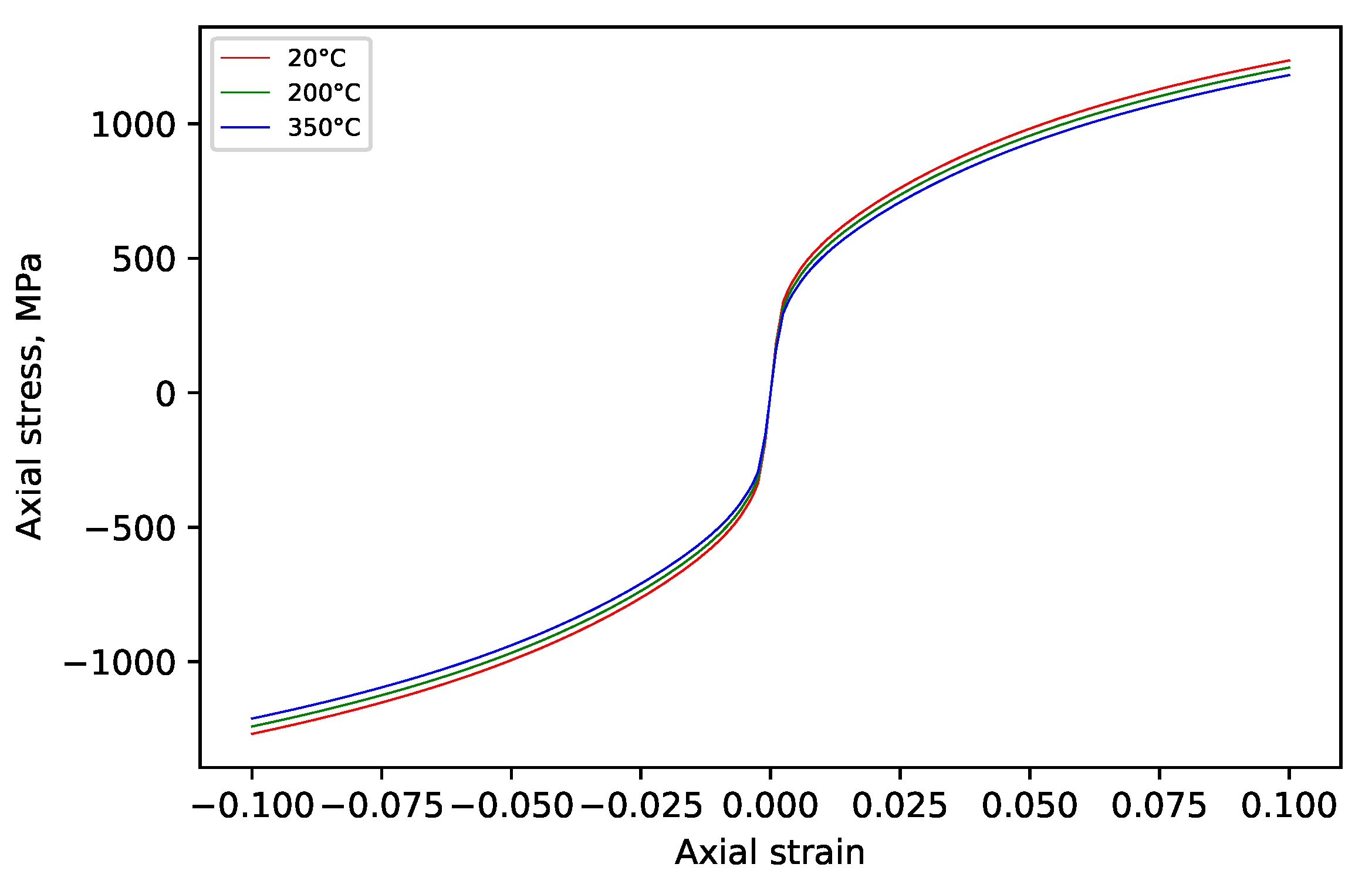
3.3. Model of Matrix Metal
3.4. Stress–Strain Behavior of the Model in Tension and Compression at Different Temperatures
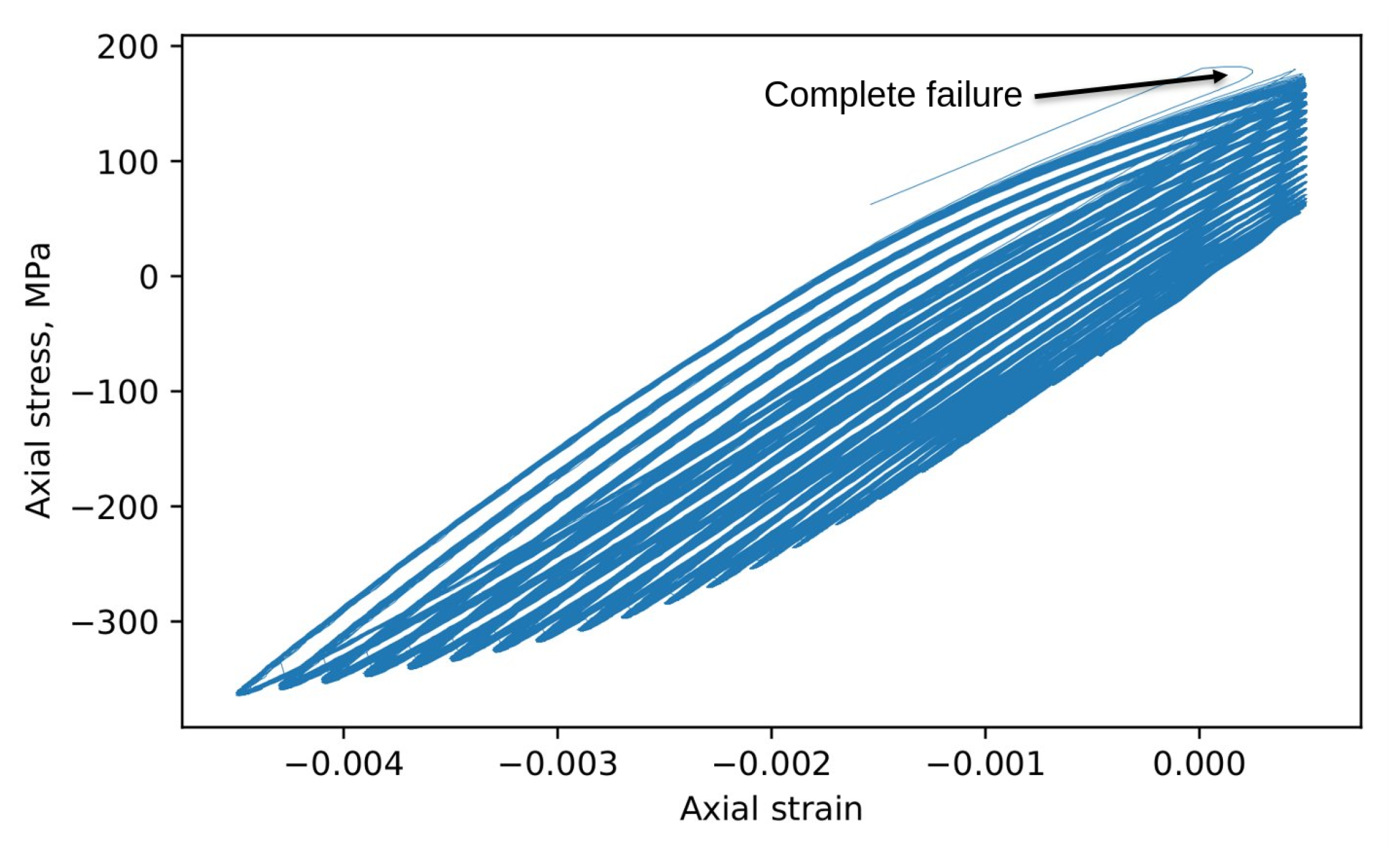
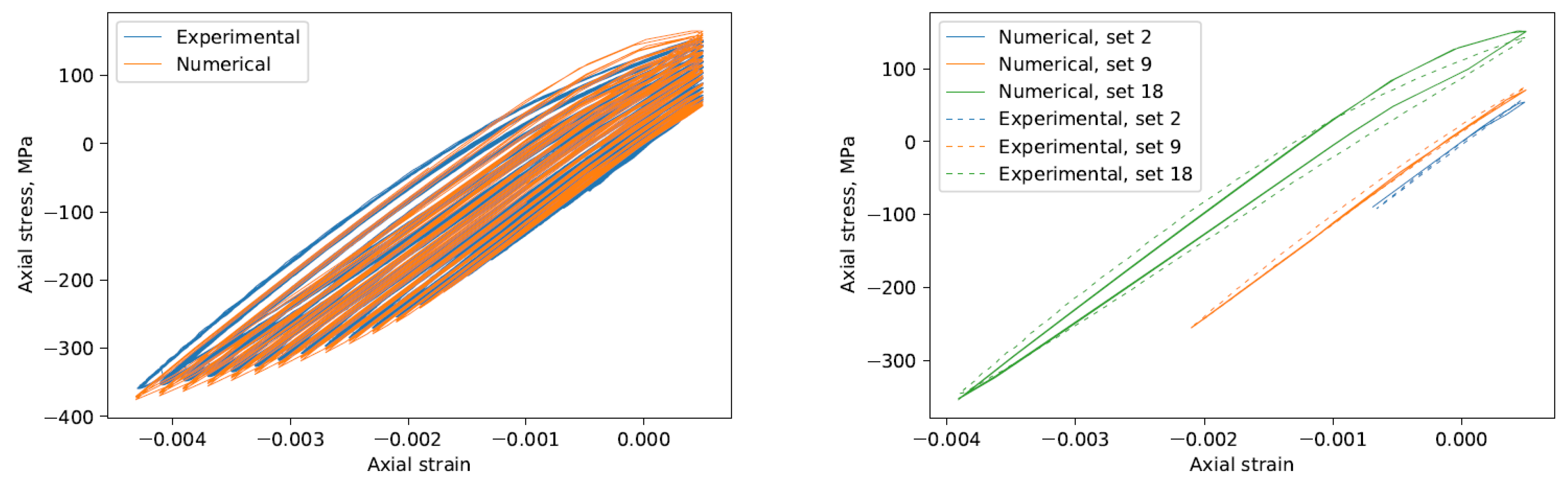
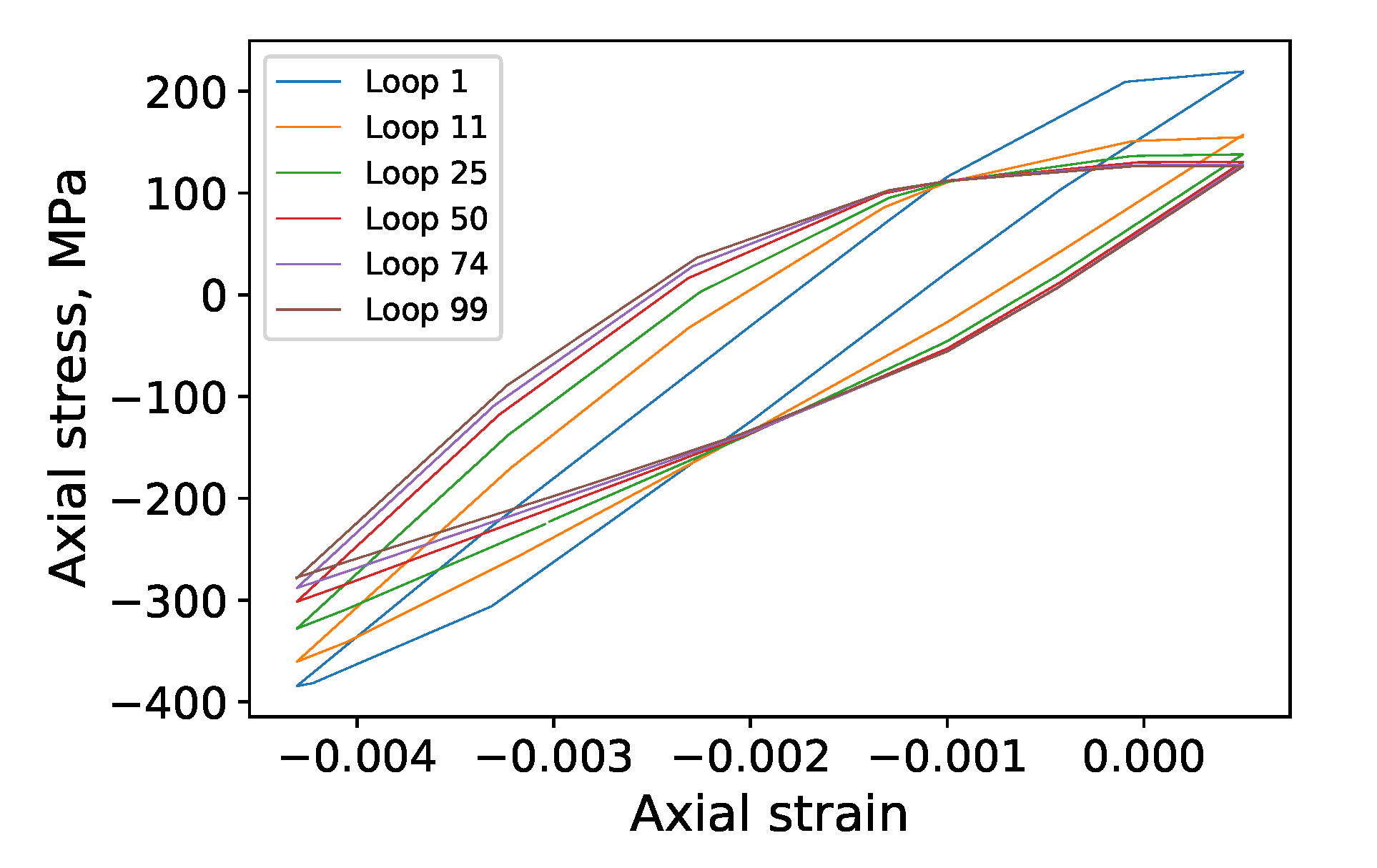
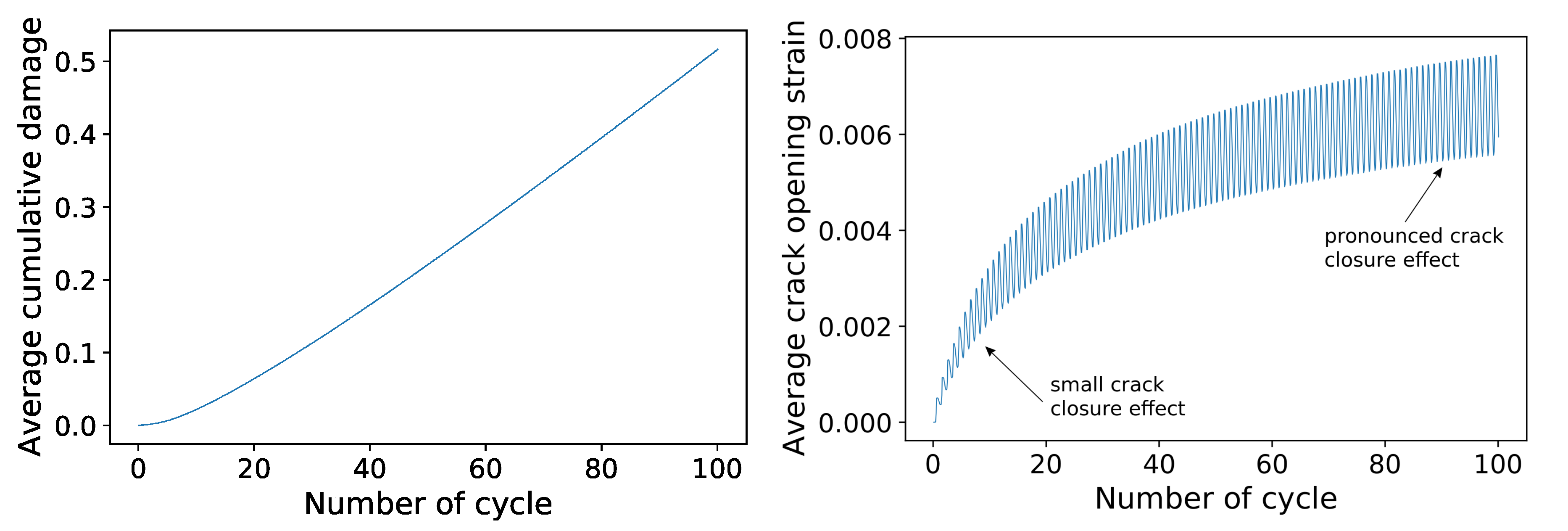
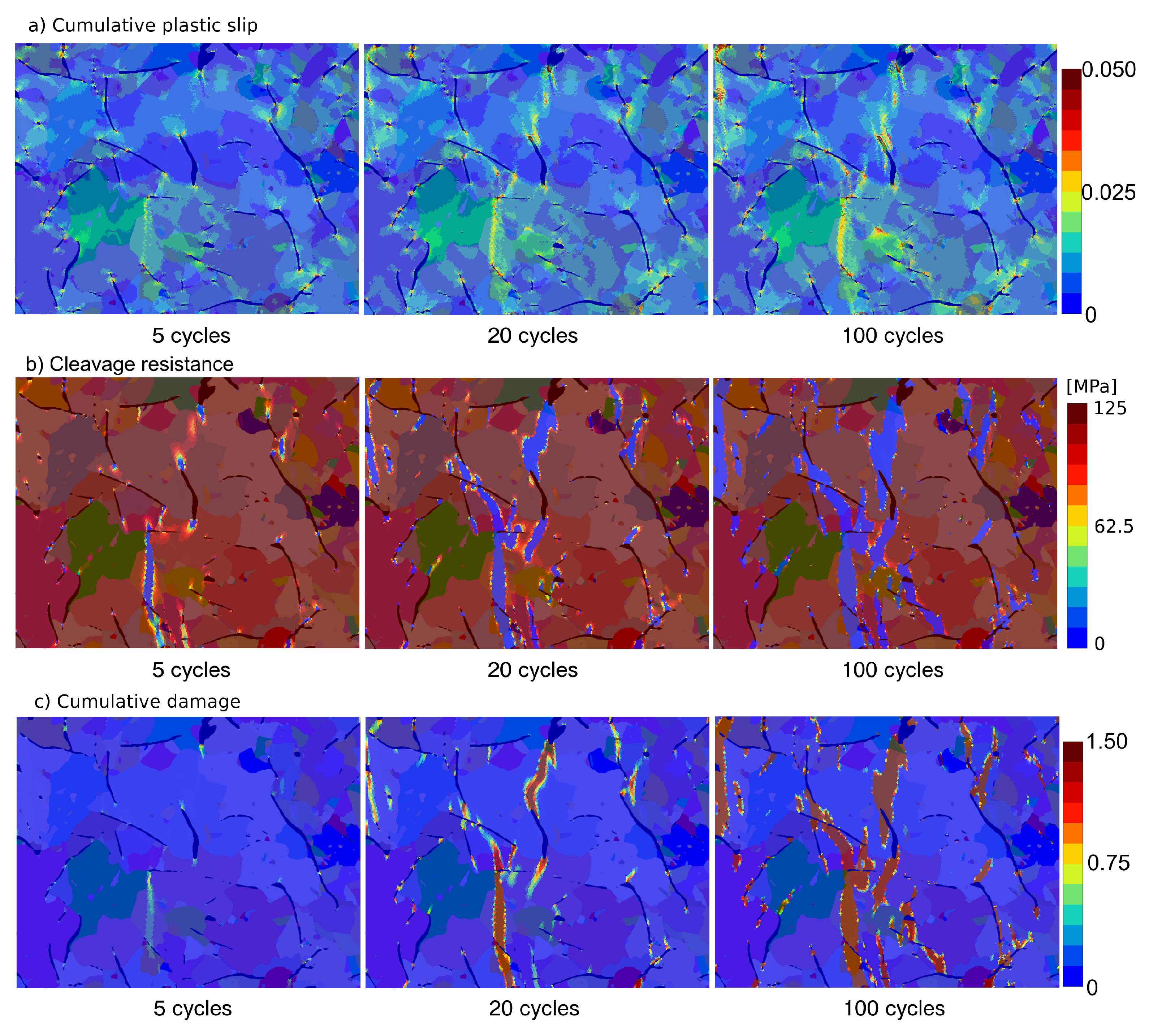
3.5. Fatigue Behavior of the Model
4. Conclusions
- Crystal plasticity microstructural models with a damage model involved are able to capture some of the main material response features observed in the experiment, such as asymmetry of the behavior in tension and compression, tensile softening in fatigue tests and temperature dependence. In addition, it is important to include plasticity properties for graphite to capture the experimental results (not only elastic, and it can be strongly argued that they cannot be modelled by voids, as can be found in the literature);
- Qualitative studies were performed to demonstrate the ability of the model to simulate experimentally observed effects. A further refinement of the model parameters can be achieved; however, this requires more extensive experimental data, as well as revealing the underlying damage phenomena and plasticity–damage couplings. This is evident in the present work, since damage was introduced into the model mainly for the proper modeling softening behavior. Thus, further work is required to gain proper confidence in fatigue modeling based on the model with micromechanical damage, especially in terms of the choice of parameters;
- Utilization of different representations of polycrystalline and phase structures at the microscale allows for the investigation of fine-scale mechanical behavior. The use of EBSD-based computational domains allows for the analysis of general microstructural characteristics and damage progression. However, a fully discretized 3D microstructure with tomography data would contribute to an understanding of the influence of graphite networks, grain structure and local defects (e.g., voids), which will ultimately allow for the tailoring of better cast iron microstructures, depending on the specific performance and cost requirements of the material.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| BCC | Body-Centered Cubic (crystal system) |
| CP | Crystal Plasticity |
| EBSD | Electron Backscatter Diffraction |
| HCP | Hexagonal Close Packed (crystal system) |
| FEM | Finite Element Method |
| FIB | Focused Ion Beam |
| SEM | Scanning Electron Microscope |
References
- Riposan, I.; Chisamera, M.; Stan, S. Special Report CHINA FOUNDRY New developments in high quality grey cast irons. China Foundry 2014, 11, 351–364. [Google Scholar]
- Downing, S.D.; Socie, D.F. Stress/strain simulation model for grey cast iron. Int. J. Fatigue 1982, 4, 143–148. [Google Scholar] [CrossRef]
- Wiese, J.W.; Dantzig, J.A. Modeling stress development during the solidification of gray iron castings. Metall. Trans. A 1990, 21, 489–497. [Google Scholar] [CrossRef]
- Josefson, B.L.; Hjelm, H.E. Modelling Elastoplastic Deformations in Grey Cast Iron. In Low Cycle Fatigue and Elasto-Plastic Behaviour of Materials—3; Rie, K.T., Grünling, H.W., König, G., Neumann, P., Nowack, H., Schwalbe, K.H., Seeger, T., Eds.; Springer: Dordrecht, The Netherlands, 1992; pp. 465–472. [Google Scholar] [CrossRef]
- Hjelm, H.E. Yield Surface for Grey Cast Iron Under Biaxial Stress. J. Eng. Mater. Technol. 1994, 116, 148–154. [Google Scholar] [CrossRef]
- Altenbach, H.; Stoychev, G.B.; Tushtev, K.N. On elastoplastic deformation of grey cast iron. Int. J. Plast. 2001, 17, 719–736. [Google Scholar] [CrossRef]
- Seifert, T.; Riedel, H. Mechanism-based thermomechanical fatigue life prediction of cast iron. Part I: Models. Int. J. Fatigue 2010, 32, 1358–1367. [Google Scholar] [CrossRef]
- Manual, A.U. Abaqus User’s Manual, Version 2020; Dassault Systèmes Simulia Corp.: Johnston, RI, USA, 2020. [Google Scholar]
- Hosseini, E.; Holdsworth, S.R.; Flueeler, U. A temperature-dependent asymmetric constitutive model for cast irons under cyclic loading conditions. J. Strain Anal. Eng. Des. 2017, 53, 106–114. [Google Scholar] [CrossRef]
- Norman, V.; Calmunger, M. On the micro- and macroscopic elastoplastic deformation behaviour of cast iron when subjected to cyclic loading. Int. J. Plast. 2019, 115, 200–215. [Google Scholar] [CrossRef]
- Oksanen, V.; Valtonen, K.; Andersson, P.; Vaajoki, A.; Laukkanen, A.; Holmberg, K.; Kuokkala, V. Comparison of laboratory rolling–Sliding wear tests with in-service wear of nodular cast iron rollers against wire ropes. Wear 2015, 340–341, 73–81, Papers from the NORDTRIB 2014 conference co-edited by Prof. Ion Marius Sivebæk and Lars Pleth Nielsen. [Google Scholar] [CrossRef]
- Metzger, M.; Seifert, T. Computational assessment of the microstructure-dependent plasticity of lamellar gray cast iron—Part I: Methods and microstructure-based models. Int. J. Solids Struct. 2015, 66, 184–193. [Google Scholar] [CrossRef]
- Metzger, M.; Seifert, T. Computational assessment of the microstructure-dependent plasticity of lamellar gray cast iron—Part II: Initial yield surfaces and directions. Int. J. Solids Struct. 2015, 66, 194–206. [Google Scholar] [CrossRef]
- Metzger, M.; Seifert, T. Computational assessment of the microstructure-dependent plasticity of lamellar gray cast iron—Part III: A new yield function derived from microstructure-based models. Int. J. Solids Struct. 2016, 87, 102–109. [Google Scholar] [CrossRef]
- Metzger, M.; Seifert, T. Computational assessment of the microstructure-dependent plasticity of lamellar gray cast iron—Part IV: Assessment of the yield surface after plastic loading. Int. J. Solids Struct. 2018, 141–142, 173–182. [Google Scholar] [CrossRef]
- Pina, J.; Kouznetsova, V.; Geers, M. Thermo-mechanical analyses of heterogeneous materials with a strongly anisotropic phase: The case of cast iron. Int. J. Solids Struct. 2015, 63, 153–166. [Google Scholar] [CrossRef]
- Pina, J.; Shafqat, S.; Kouznetsova, V.; Hoefnagels, J.; Geers, M. Microstructural study of the mechanical response of compacted graphite iron: An experimental and numerical approach. Mater. Sci. Eng. A 2016, 658, 439–449. [Google Scholar] [CrossRef]
- Besson, J.; Foerch, R. Object-oriented programming applied to the finite element method part I. general concepts. Rev. Eur. Des Eléments Finis 1998, 7, 535–566. [Google Scholar] [CrossRef]
- Méric, L.; Cailletaud, G. Single crystal modeling for structural calculations. Part 1—Model presentation. J. Eng. Mater. Technol 1991, 113, 162–170. [Google Scholar] [CrossRef]
- Zhang, X.; Qu, Y.; Li, R. Fracture Mechanism of Ferritic Ductile Iron under Instrumented Impact Load at Low Temperatures. ISIJ Int. 2014, 54, 2309–2313. [Google Scholar] [CrossRef] [Green Version]
- Aslan, O.; Cordero, N.; Gaubert, A.; Forest, S. Micromorphic approach to single crystal plasticity and damage. Int. J. Eng. Sci. 2011, 49, 1311–1325. [Google Scholar] [CrossRef]
- Aslan, O.; Quilici, S.; Forest, S. Numerical modeling of fatigue crack growth in single crystals based on microdamage theory. Int. J. Damage Mech. 2011, 20, 681–705. [Google Scholar] [CrossRef]
- Sabnis, P.; Forest, S.; Cormier, J. Microdamage modelling of crack initiation and propagation in FCC single crystals under complex loading conditions. Comput. Methods Appl. Mech. Eng. 2016, 312, 468–491. [Google Scholar] [CrossRef]
- Lindroos, M.; Laukkanen, A.; Andersson, T.; Vaara, J.; Mantyla, A.; Frondelius, T. Micromechanical modeling of short crack nucleation and growth in high cycle fatigue of martensitic microstructures. Comput. Mater. Sci. 2019, 170, 109185. [Google Scholar] [CrossRef]
- Lindroos, M.; Scherer, J.M.; Forest, S.; Laukkanen, A.; Andersson, T.; Vaara, J.; Mantyla, A.; Frondelius, T. Micromorphic crystal plasticity approach to damage regularization and size effects in martensitic steels. Int. J. Plas. 2022, 151, 103187. [Google Scholar] [CrossRef]
- Andriollo, T.; Zhang, Y.; Fæster, S.; Thorborg, J.; Hattel, J. Impact of micro-scale residual stress on in-situ tensile testing of ductile cast iron: Digital volume correlation vs. model with fully resolved microstructure vs. periodic unit cell. J. Mech. Phys. Solids 2019, 125, 714–735. [Google Scholar] [CrossRef]
- Herrmann, C.; Schmid, S.; Schneider, D.; Selzer, M.; Nestler, B.; Skobir Balantič, D. Computational Determination of Macroscopic Mechanical and Thermal Material Properties for Different Morphological Variants of Cast Iron. Metals 2021, 11, 1588. [Google Scholar] [CrossRef]
- Bonora, N.; Ruggiero, A. Micromechanical modeling of ductile cast iron incorporating damage. Part I: Ferritic ductile cast iron. Int. J. Solids Struct. 2005, 42, 1401–1424. [Google Scholar] [CrossRef]
- Andriollo, T.; Fæster, S.; Winther, G. Probing the structure and mechanical properties of the graphite nodules in ductile cast irons via nano-indentation. Mech. Mater. 2018, 122, 85–95. [Google Scholar] [CrossRef] [Green Version]
- Lindroos, M.; Pinomaa, T.; Antikainen, A.; Lagerbom, J.; Reijonen, J.; Lindroos, T.; Andersson, T.; Laukkanen, A. Micromechanical modeling approach to single track deformation, phase transformation and residual stress evolution during selective laser melting using crystal plasticity. Addit. Manuf. 2021, 38, 101819. [Google Scholar] [CrossRef]





| Model | Type of Elements | Number of Elements | Wall-Clock Time, s |
|---|---|---|---|
| Model 1 | 3D cubic | 1000 | 61 |
| Model 2 | 2D triangular | 3731 | 50 |
| Model 3 | 3D triangular prism | 1,215,858 | 36,200 |
| part of Model 3 | 3D triangular prism | 41,265 | 7502 |
| part of Model 3 | 3D triangular prism | 15,481 | 2296 |
| Parameter | Value in Tension | Value in Compression |
|---|---|---|
| [GPa] | 6 | 20 |
| 0.25 | 0.25 | |
| n | 9.7 | 9.7 |
| K [MPa/s] | 1 | 100 |
| [MPa] | 1 | 100 |
| Q [MPa] | 5 | 50 |
| b | 2 | 20 |
| c | 25,000 | 25,000 |
| d | 1200 | 600 |
| all 1.0 | all 1.0 |
| Parameter | Value |
|---|---|
| [GPa] | |
| n | 9.7 |
| K [MPa/s] | |
| [MPa] | |
| Q [MPa] | 80 |
| b | 30 |
| c | 30000 |
| d | 600 |
| all 1.0 | |
| Damage parameters | |
| 125.0 | |
| 0.1 | |
| H | −1000.0 |
| 90.0 | |
| 11.0 | |
| 10.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Balobanov, V.; Lindroos, M.; Andersson, T.; Laukkanen, A. Crystal Plasticity Modeling of Grey Cast Irons under Tension, Compression and Fatigue Loadings. Crystals 2022, 12, 238. https://doi.org/10.3390/cryst12020238
Balobanov V, Lindroos M, Andersson T, Laukkanen A. Crystal Plasticity Modeling of Grey Cast Irons under Tension, Compression and Fatigue Loadings. Crystals. 2022; 12(2):238. https://doi.org/10.3390/cryst12020238
Chicago/Turabian StyleBalobanov, Viacheslav, Matti Lindroos, Tom Andersson, and Anssi Laukkanen. 2022. "Crystal Plasticity Modeling of Grey Cast Irons under Tension, Compression and Fatigue Loadings" Crystals 12, no. 2: 238. https://doi.org/10.3390/cryst12020238
APA StyleBalobanov, V., Lindroos, M., Andersson, T., & Laukkanen, A. (2022). Crystal Plasticity Modeling of Grey Cast Irons under Tension, Compression and Fatigue Loadings. Crystals, 12(2), 238. https://doi.org/10.3390/cryst12020238







