Computational Modeling of Dislocation Slip Mechanisms in Crystal Plasticity: A Short Review
Abstract
1. Introduction
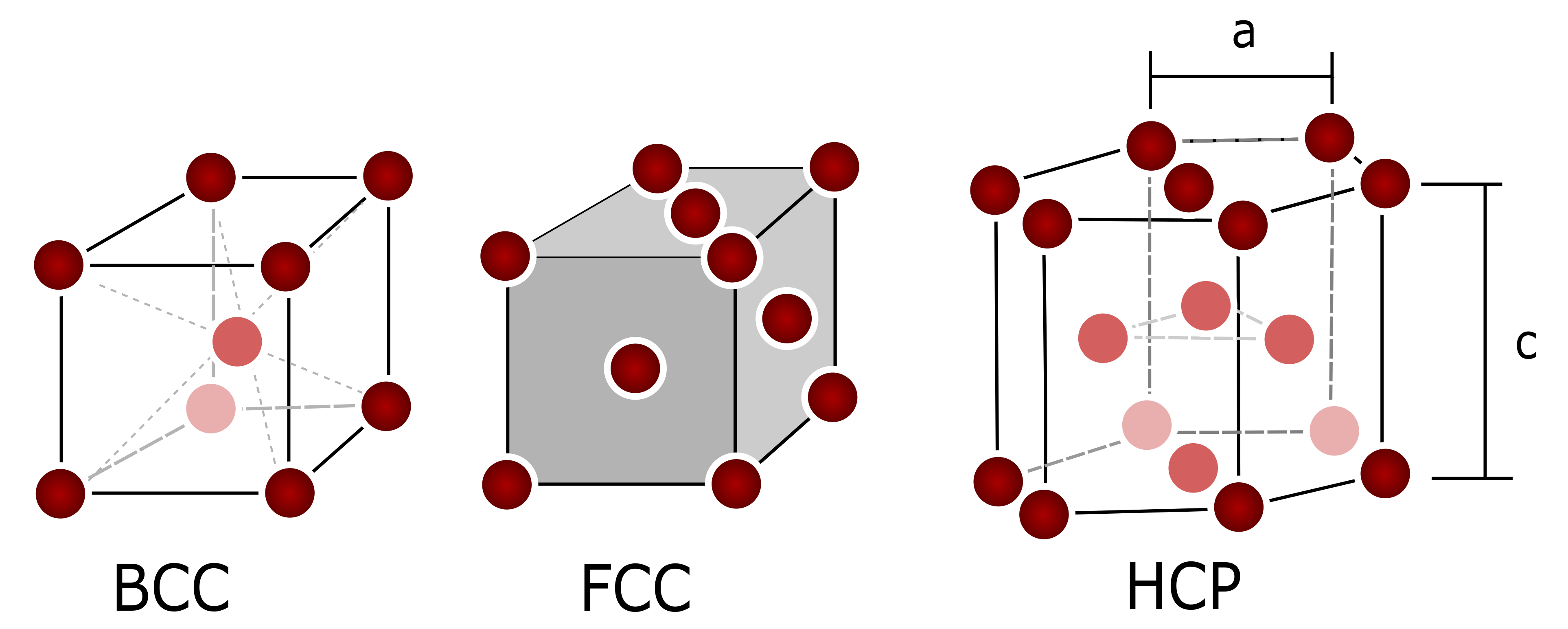
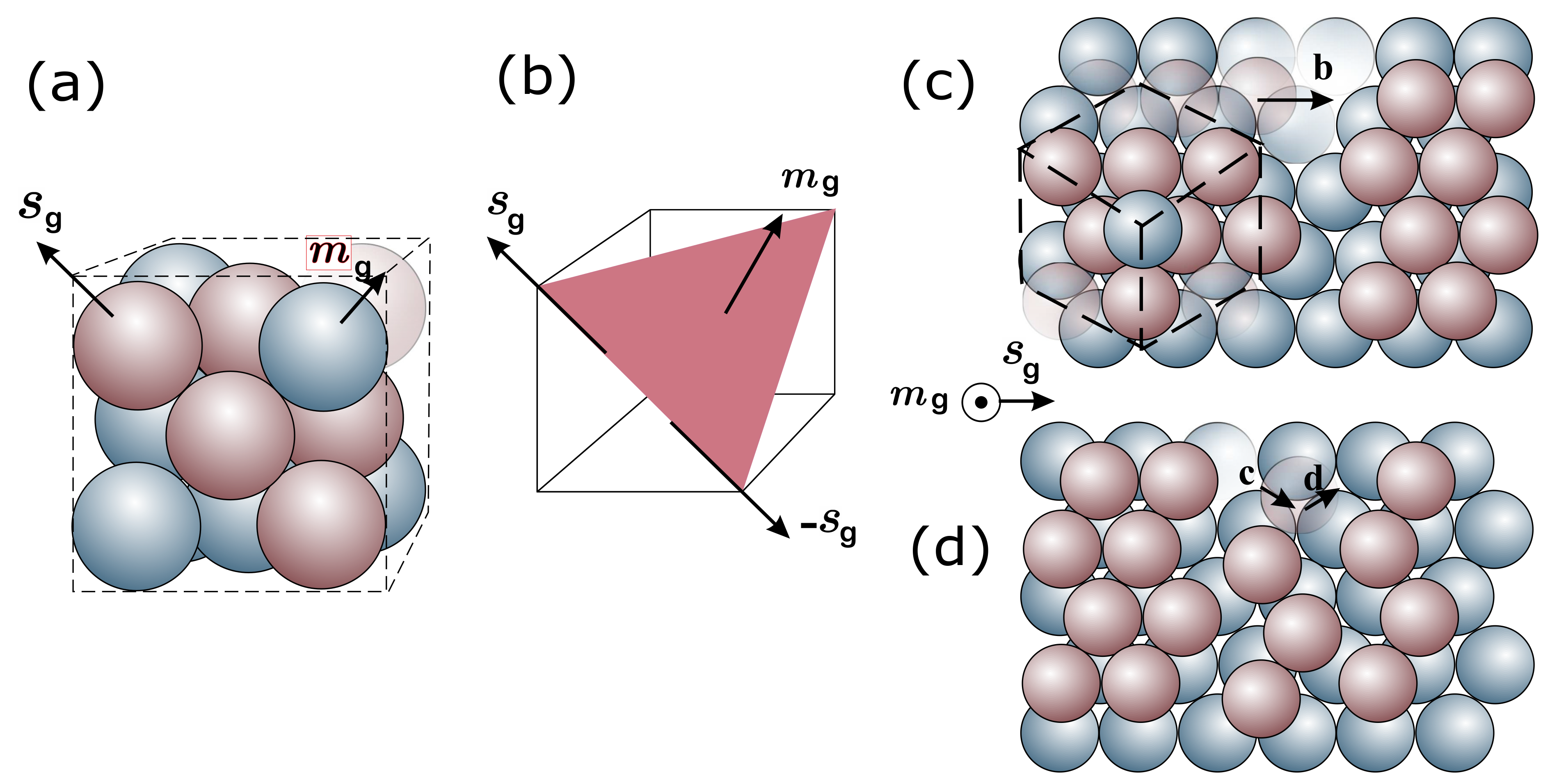
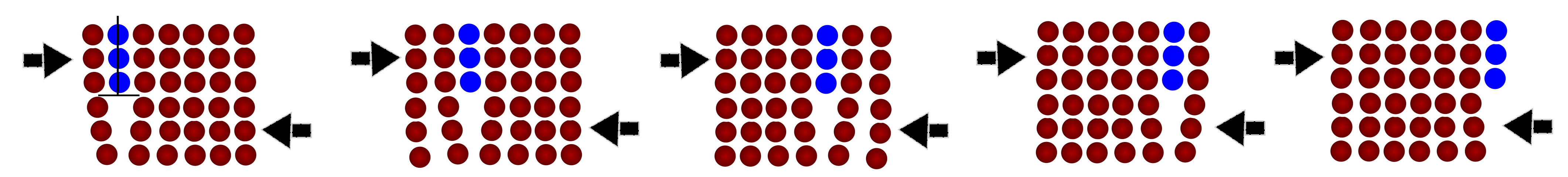
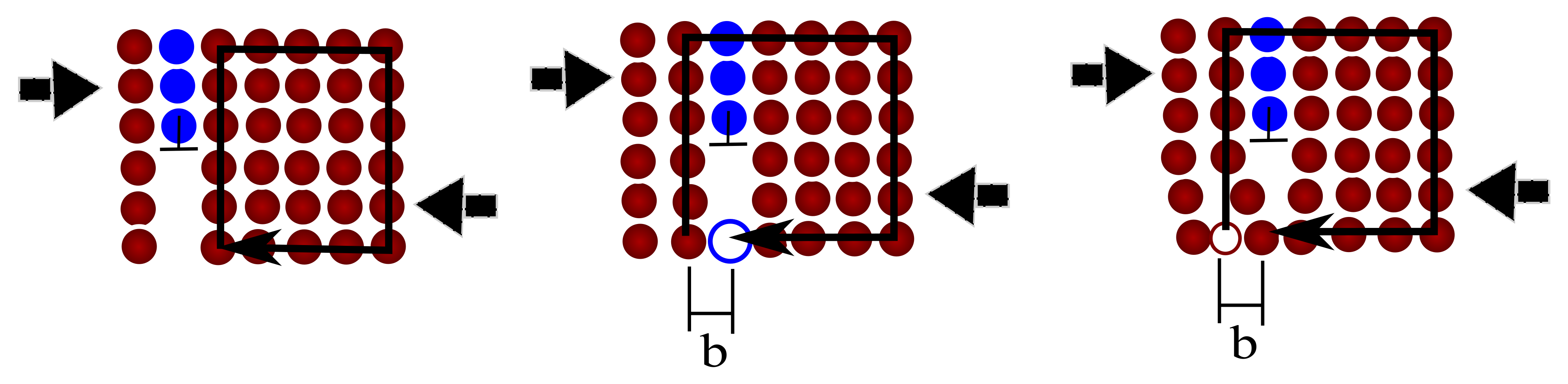
2. Mechanism of Dislocation Slip: Physical Aspects
3. Continuum Framework of Crystal Plasticity
3.1. Kinematics
3.2. Constitutive Models
3.2.1. Phenomenological Models
3.2.2. Physics-Based Models
(a) Dislocation density-based models
(b) Geometrically necessary dislocations (GND) models
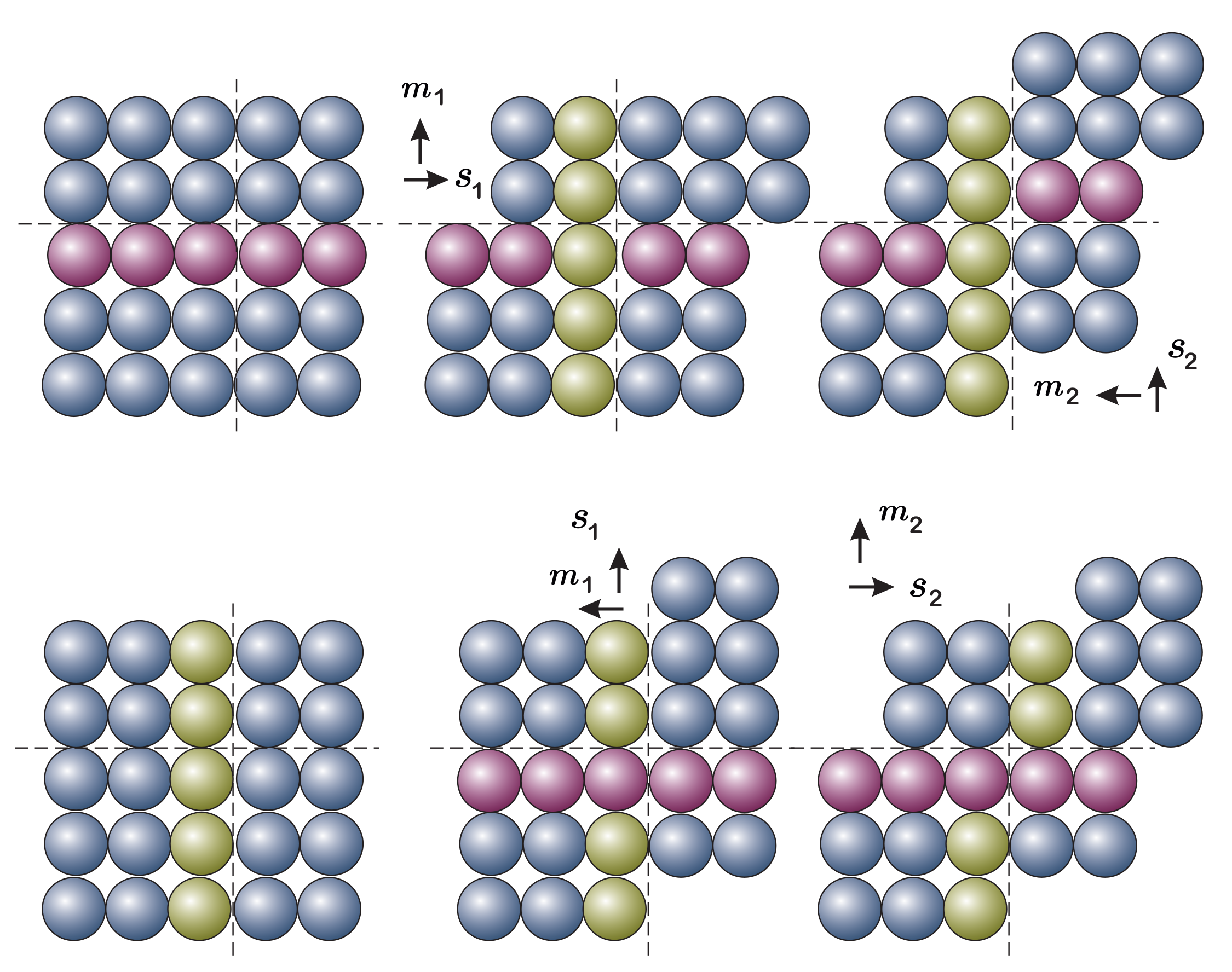
(c) Continuum Dislocation Dynamic Models
3.3. Numerical Implementation Aspects
3.3.1. Integration Algorithm
| Algorithm 1: Typical structure of an elastic-predictor/plastic-corrector return-mapping algorithm |
Require: Given the state variables at time t and the total strain at time .
|
3.3.2. Type of Elements
4. Searching the Active Set of Slip Systems
4.1. Rate-Dependent Approach
4.2. Rate-Independent Approach
5. Examples of Applications
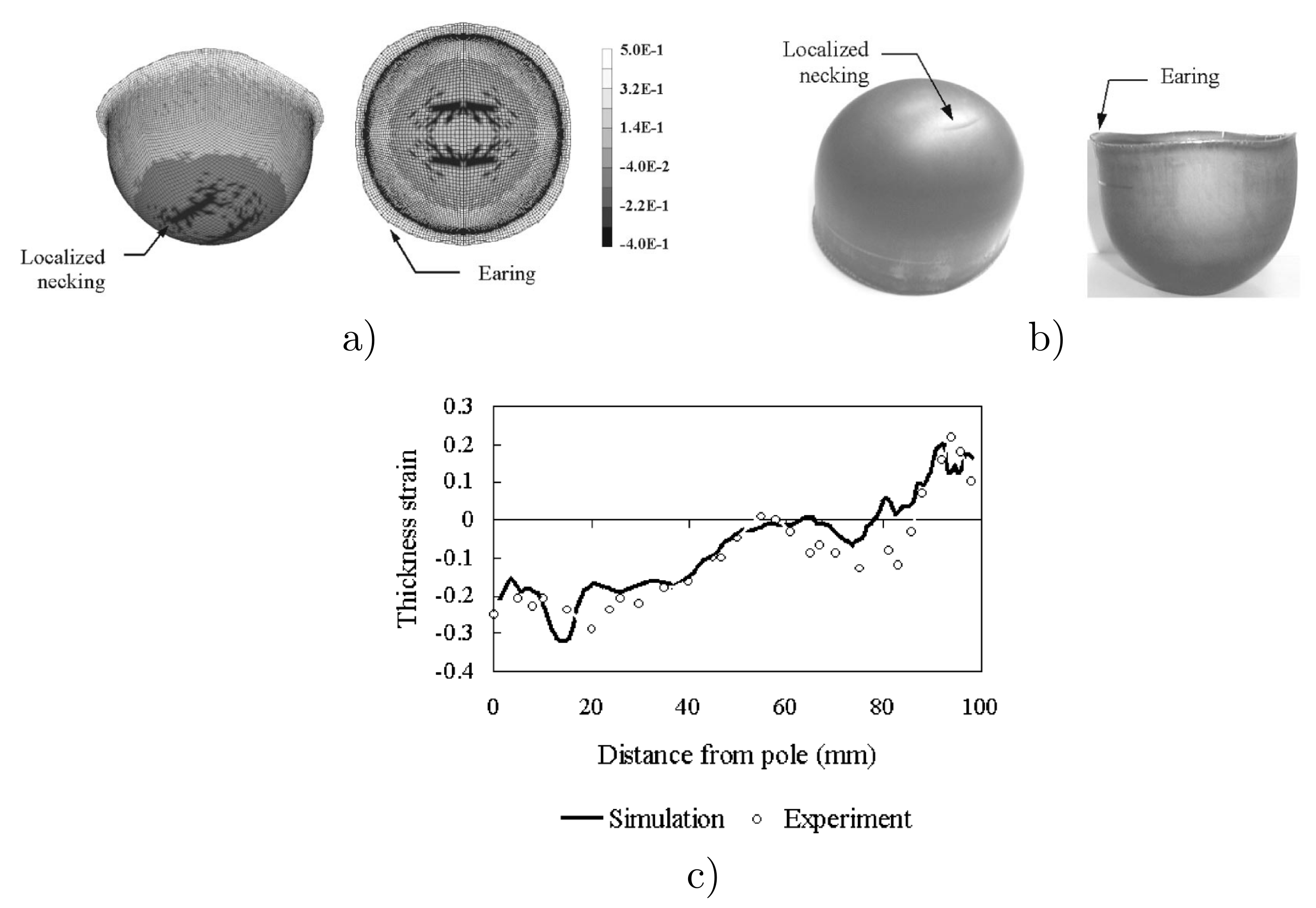
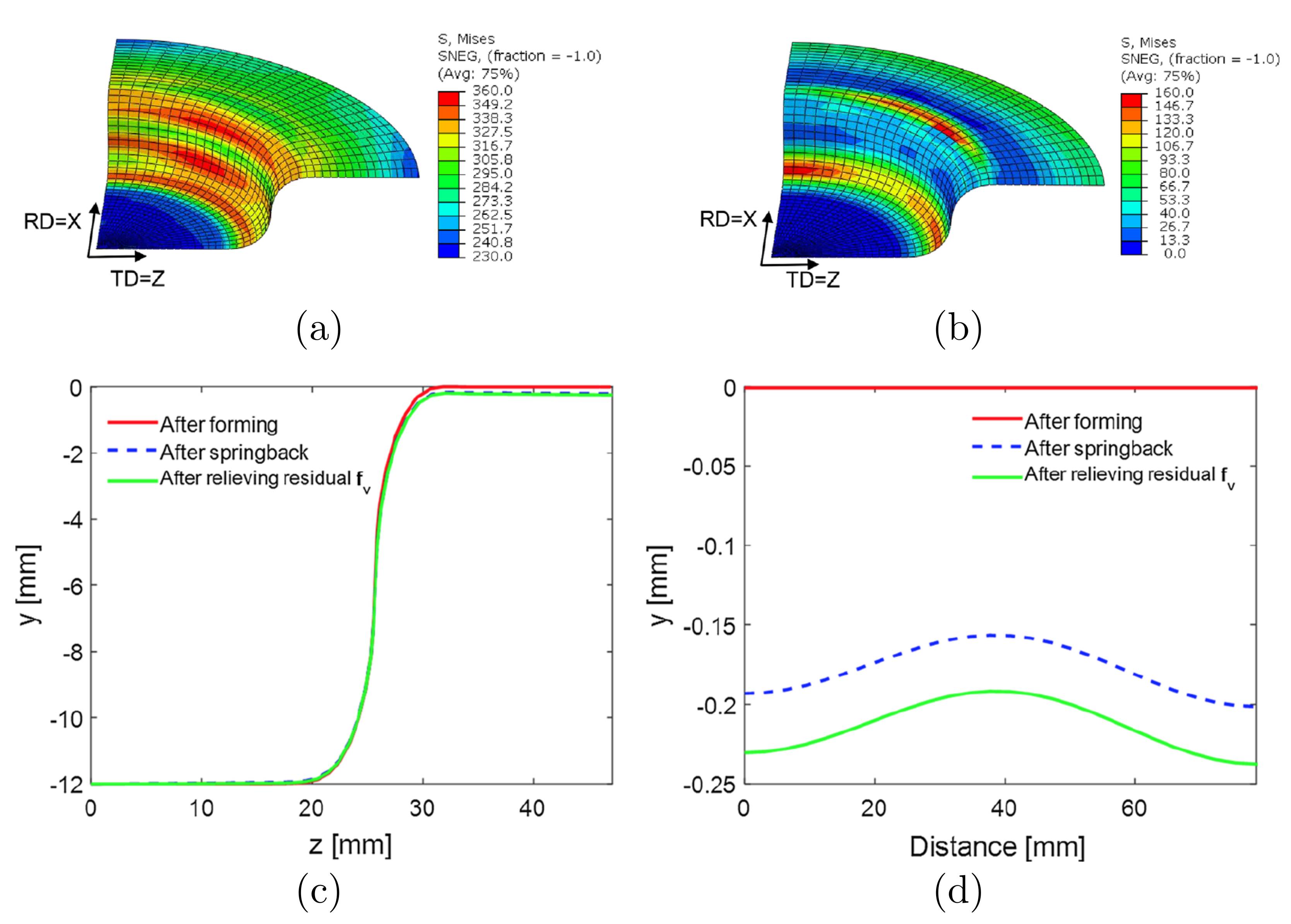
5.1. Engineering Forming Problems
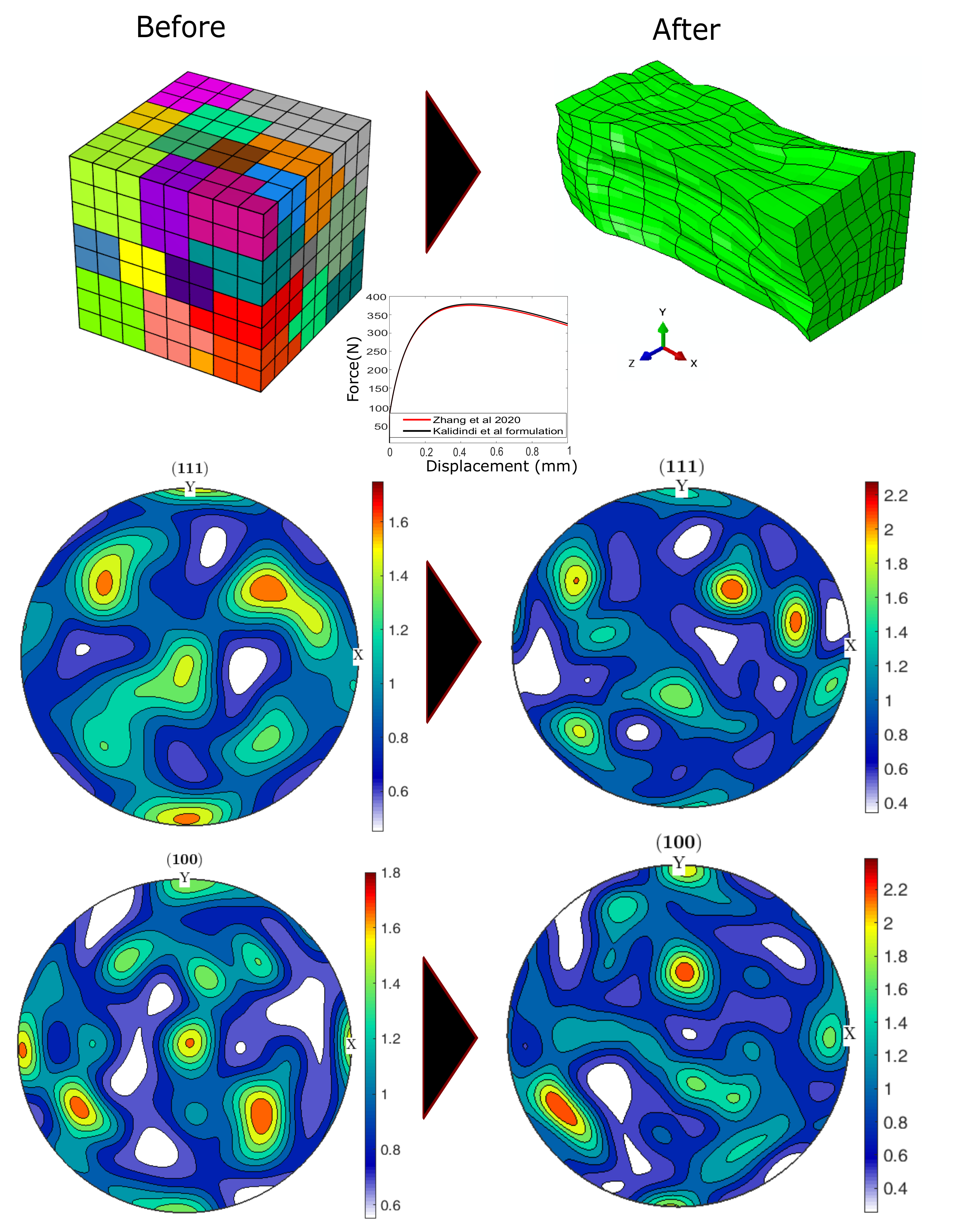
5.2. Virtual Material Testing
5.2.1. Texture Evolution
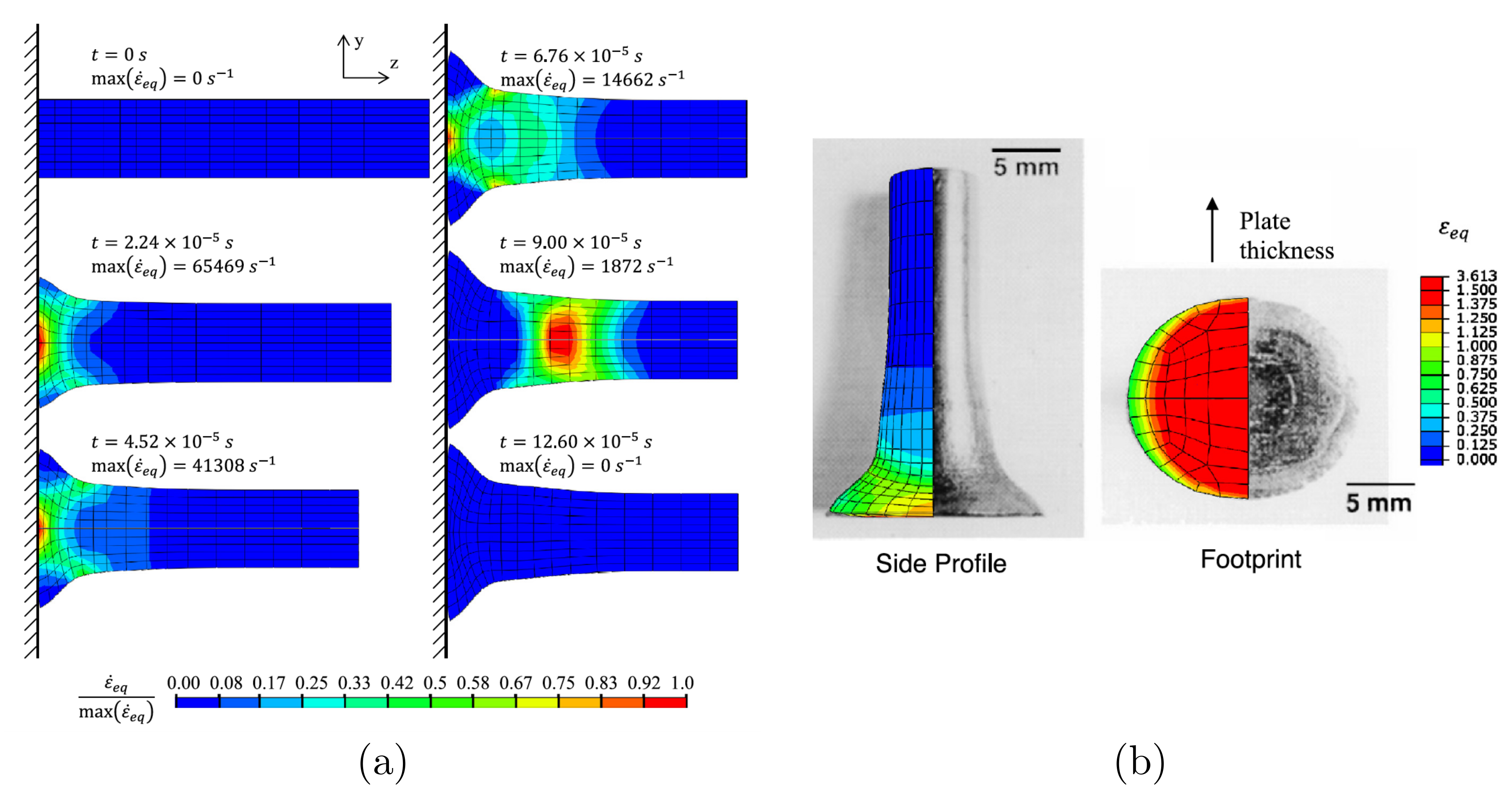
5.2.2. Impact Test
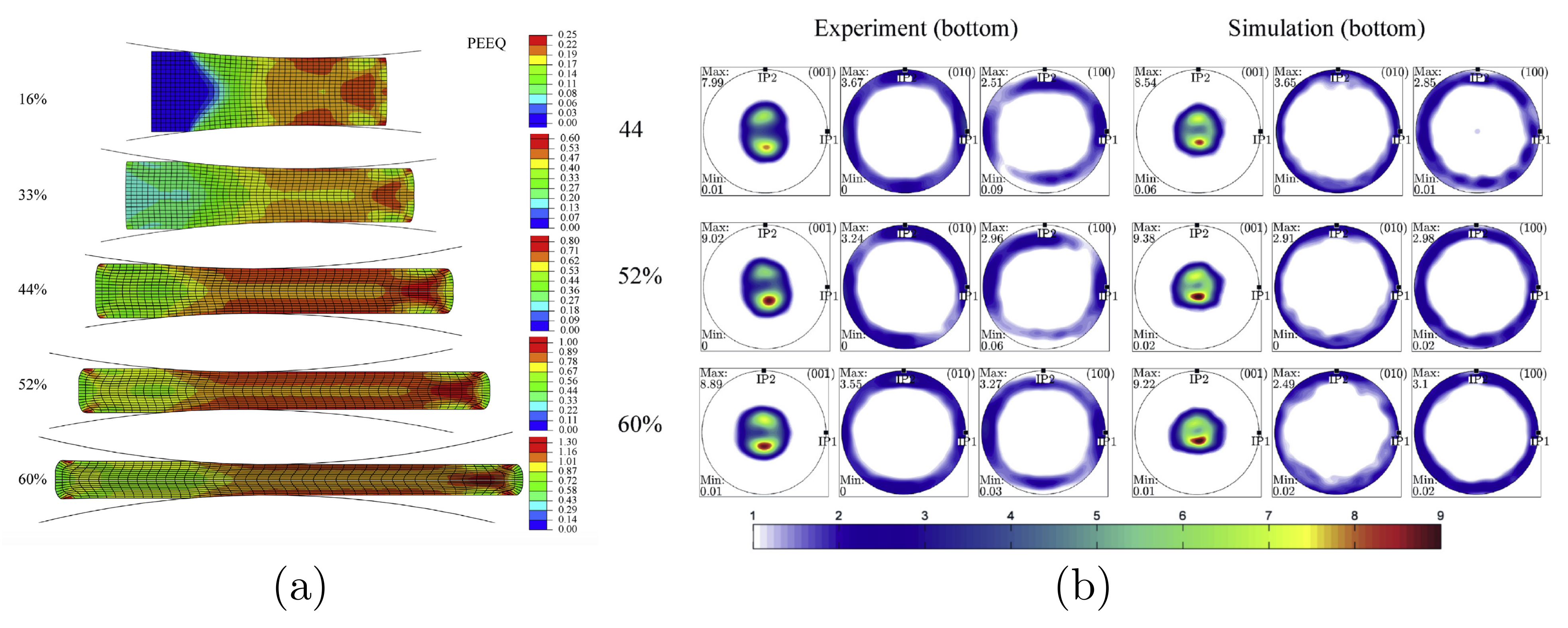
5.2.3. Rolling Test
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wagoner, R.; Chenot, J. Metal Forming Analysis; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Bathe, K.J. Finite Element Procedures, 2nd ed.; Klaus-Jürgen Bathe: Watertown, MA, USA, 2014. [Google Scholar]
- Balluffi, R. Introduction to Elasticity Theory for Crystal Deffects; World Scientific: Hackensack, NJ, USA, 2017. [Google Scholar]
- Kocks, U.; Tomé, C.; Wenk, H.R. Texture and Anisotropy: Preferred Orientations in Polycrystals and Their Effect on Paterials Properties; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Peirce, D.; Asaro, R.J.; Needleman, A. An analysis of nonuniform and localized deformation in ductile single crystals. Acta Metall. 1982, 30, 1087–1119. [Google Scholar] [CrossRef]
- Miehe, C.; Schröder, J.; Schotte, J. Computational homogenization analysis in finite plasticity Simulation of texture development in polycrystalline materials. Comput. Methods Appl. Mech. Eng. 1999, 171, 387–418. [Google Scholar] [CrossRef]
- Horstemeyer, M. Integrated Computational Materials Engineering for Metals; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Cuitiño, A.M.; Ortiz, M. Computational modelling of single crystals. Model. Simul. Mater. Sci. Eng. 1992, 1, 225–263. [Google Scholar] [CrossRef]
- Fohrmeister, V.; Díaz, G.; Mosler, J. Classic crystal plasticity theory vs crystal plasticity theory based on strong discontinuities. Theoretical and algorithmic aspects. Int. J. Numer. Methods Eng. 2019, 117, 1283–1303. [Google Scholar] [CrossRef]
- Kim, J.; Yoon, J. Necking behavior of AA 6022-T4 based on the crystal plasticity and damage models. Int. J. Plast. 2015, 73, 3–23. [Google Scholar] [CrossRef]
- Aim, U.; Siddiq, M.; Kartal, M. Representative volume element (RVE) based crystal plasticity study of void growth on phase boundary in titanium alloys. Comput. Mater. Sci. 2019, 161, 346–350. [Google Scholar]
- Noell, P.; Carroll, J.; Hattar, K.; Clark, B.; Boyce, B. Do voids nucleate at grain boundaries during ductile rupture? Acta Mater. 2017, 137, 103–114. [Google Scholar] [CrossRef]
- Miehe, C.; Welschinger, F.; Hofacker, M. Thermodynamically consistent phase-field models of fracture: Variational principles and multi-field FE implementations. Int. J. Numer. Methods Eng. 2010, 83, 1273–1311. [Google Scholar] [CrossRef]
- Grilli, N.; Koslowski, M. The effect of crystal anisotropy and plastic response on the dynamic fracture of energetic materials. J. Appl. Phys. 2019, 126. [Google Scholar] [CrossRef]
- Kohlhoff, S.; Gumbsch, P.; Fischmeister, H. Crack propagation in BCC crystals studied with a combined finite-element and atomistic model. Philos. Mag. A 1991, 64, 851–878. [Google Scholar] [CrossRef]
- Shilkrot, L.E.; Miller, R.; Curtin, W. Coupled atomistic and discrete dislocation plasticity. Phys. Rev. Lett. 2002, 89, 25501. [Google Scholar] [CrossRef] [PubMed]
- Shiari, B.; Miller, R.; Curtin, W. Coupled atomistic/discrete dislocation simulations of nanoindentation at finite temperature. J. Eng. Mater. Technol. 2005, 127, 358–368. [Google Scholar] [CrossRef]
- Kochmann, J.; Wulfinghoff, S.; Ehle, L.; Mayer, J.; Svendsen, B.; Reese, S. Efficient and accurate two-scale FE-FFT-based prediction of the effective material behavior of elasto-viscoplastic polycrystals. Comput. Mech. 2018, 61, 751–764. [Google Scholar] [CrossRef]
- Valdenaire, P.L.; Le Bouar, Y.; Appolaire, B.; Finel, A. Density-based crystal plasticity: From the discrete to the continuum. Phys. Rev. B 2016, 93, 214111. [Google Scholar] [CrossRef]
- Roters, F.; Eisenlohr, P.; Hantcherli, L.; Tjahjanto, D.; Bieler, T.; Raabe, D. Overview of constitutive laws, kinematics, homogenization and multiscale methods in crystal plasticity finite-element modeling: Theory, experiments, applications. Acta Mater. 2010, 58, 1152–1211. [Google Scholar] [CrossRef]
- Roters, F.; Eisenlohr, P.; Bieler, T.; Raabe, D. Crystal Plasticity Finite Element Methods in Materials Science and Engineering; Wiley-VHC: Weinheim, Germany, 2010. [Google Scholar]
- Zhuang, Z.; Liu, Z.; Cui, Y. Dislocation Mechanism-Based Crystal Plasticity; Academic Press: Cambridge, MA, USA, 2019. [Google Scholar]
- Abbaschian, R.; Abbaschian, L.; Reed-Hill, R. Physical Metallurgy Principles; Cengage Learning: Stamford, CT, USA, 2009. [Google Scholar]
- Borja, R.I. Plasticity Modeling and Computation; Springer: Berlin, Germany, 2013. [Google Scholar]
- Hosford, W.F. The Mechanics of Crystals and Textured Polycrystals; Oxford University Press: Oxford, UK, 1993. [Google Scholar]
- Dunne, F.; Petrinic, N. Introduction to Computational Plasticity; Oxford University Press: Oxford, UK, 2005. [Google Scholar]
- Hull, D.; Bacon, D. Introduction to Dislocations; Butterworth-Heinemann: Oxford, UK, 2011. [Google Scholar]
- Zheng, Z.; Balint, D.; Dunne, F. Rate sensitivity in discrete dislocation plasticity in hexagonal close-packed crystals. Acta Mater. 2016, 107, 17–26. [Google Scholar] [CrossRef]
- Testa, G.; Bonora, N.; Ruggiero, A.; Iannitti, G. Flow Stress of bcc Metals over a Wide Range of Temperature and Strain Rates. Metals 2020, 10, 120. [Google Scholar] [CrossRef]
- Rogne, B.R.S.; Thaulow, C. Strengthening mechanisms of iron micropillars. Philos. Mag. 2015, 95, 1814–1828. [Google Scholar] [CrossRef]
- Hall, E. The Deformation and Ageing of Mild Steel: III Discussion of Results. Proc. Phys. Soc. Sect. B 1951, 64, 747–753. [Google Scholar] [CrossRef]
- Petch, N. The Cleavage Strength of Polycrystals. J. Iron Steel Inst. 1953, 174, 25–28. [Google Scholar]
- Schmid Erich, W.B. Plasticity of Crystals with Special Reference to Metals; F.A. Hughes: London, UK, 1950. [Google Scholar]
- Montáns, F.; Bathe, K. Computational Plasticity; Chapter Towards a Model for Large Strain Anisotropic Elasto-Plasticity; Springer: Berlin, Germany, 2007. [Google Scholar]
- Park, S.J.; Han, H.N.; Oh, K.H.; Raabe, D.; Kim, J.K. Finite element simulation of grain interaction and orientation fragmentation during plastic deformation of BCC metals. Mater. Sci. Forum 2002, 408–412, 371–376. [Google Scholar] [CrossRef]
- Sachtleber, M.; Zhao, Z.; Raabe, D. Experimental investigation of plastic grain interaction. Mater. Sci. Eng. A 2002, 336, 81–87. [Google Scholar] [CrossRef]
- Ma, A.; Roters, F.; Raabe, D. On the consideration of interactions between dislocations and grain boundaries in crystal plasticity finite element modeling—Theory, experiments, and simulations. Acta Mater. 2006, 54, 2181–2194. [Google Scholar] [CrossRef]
- Kothari, M.; Anand, L. Elasto-viscoplastic constitutive equations for polycrystalline metals: Application to tantalum. J. Mech. Phys. Solids 1998, 46, 51–67. [Google Scholar] [CrossRef]
- Kalidindi, S.; Bronkhorst, C.; Anand, L. Crystallographic texture evolution in bulk deformation processing of FCC metals. J. Mech. Phys. Solids 1992, 40, 537–569. [Google Scholar] [CrossRef]
- Kalidindi, S.R. Incorporation of deformation twinning in crystal plasticity models. J. Mech. Phys. Solids 1998, 46, 267–290. [Google Scholar] [CrossRef]
- Taylor, G. The mechanism of plastic deformation of crystals. Proc. R. Soc. A 1934, 165, 362–387. [Google Scholar]
- Basinski, Z. Thermally activated glide in face-centred cubic metals and its application to the theory of strain hardening. Philos. Mag. 1959, 4, 393–432. [Google Scholar] [CrossRef]
- Kuhlmann-Wilsdorf, D. A new theory of workhardening. Trans. Metall. Soc. AIME 1962, 224, 1047–1061. [Google Scholar]
- Essmann, U.; Mughrabi, H. Annihilation of dislocations during tensile and cyclic deformation and limits of dislocation densities. Philos. Mag. A 1979, 40, 731–756. [Google Scholar] [CrossRef]
- Essmann, U.; Mughrabi, H. Multislip in FCC crystals: A theoretical approach compared with experimental-data. Acta Metall. 1982, 30, 1627–1637. [Google Scholar]
- Lavrentev, F.; Pokhil, Y. Relation of dislocation density in different slip systems to work-hardening parameters for magnesium crystals. Mater. Sci. Eng. 1975, 18, 261–270. [Google Scholar] [CrossRef]
- Bertin, N.; Tomé, C.; Beyerlein, I.; Barnett, M.; Capolungo, L. On the strength of dislocation interactions and their effect on latent hardening in pure Magnesium. Int. J. Plast. 2014, 62, 72–92. [Google Scholar] [CrossRef]
- Arturo Rubio, R.; Haouala, S.; LLorca, J. Grain boundary strengthening of FCC polycrystals. J. Mater. Res. 2019, 34, 2263–2274. [Google Scholar] [CrossRef]
- Haouala, S.; Alizadeh, R.; Bieler, T.; Segurado, J.; LLorca, J. Effect of slip transmission at grain boundaries in Al bicrystals. Int. J. Plast. 2020, 126, 102600. [Google Scholar] [CrossRef]
- Ma, A.; Hartmaier, A. On the influence of isotropic and kinematic hardening caused by strain gradients on the deformation behaviour of polycrystals. Philos. Mag. 2014, 94, 125–140. [Google Scholar] [CrossRef]
- Ma, A.; Hartmaier, A. A study of deformation and phase transformation coupling for TRIP-assisted steels. Int. J. Plast. 2015, 64, 40–55. [Google Scholar] [CrossRef]
- Asaro, R.J. Micromechanics of Crystals and Polycrystals. Adv. Appl. Mech. 1983, 23, 1–115. [Google Scholar] [CrossRef]
- Lee, E.H. Elastic-Plastic Deformation at Finite Strains. J. Appl. Mech. 1969, 36, 1–6. [Google Scholar] [CrossRef]
- Rice, J. Inelastic constitutive relations for solids: An internal-variable theory and its application to metal plasticity. J. Mech. Phys. Solids 1971, 19, 433–455. [Google Scholar] [CrossRef]
- Hill, R.; Rice, J.R. Constitutive analysis of elastic-plastic crystals at arbitrary strain. J. Mech. Phys. Solids 1972, 20, 401–413. [Google Scholar] [CrossRef]
- Simo, J. Handbook of Numerical Analysis VI; Chapter Numerical Analysis and Simulation of Plasticity; Elsevier: Amsterdam, The Netherlands, 1998; pp. 183–499. [Google Scholar]
- Hashiguchi, K. Multiplicative Hyperelastic-Based Plasticity for Finite Elastoplastic Deformation/Sliding: A Comprehensive Review. Arch. Comput. Methods Eng. 2019, 26, 597–637. [Google Scholar] [CrossRef]
- Gurtin, M.; Fried, E.; Anand, L. The Mechanics and Thermodynamics of Continua; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Shutov, A.; Pfeiffer, S.; Ihlemann, J. On the simulation of multistage forming processes: Invariance under change of the reference configuration. Mater. Sci. Eng. Technol. 2012, 43, 617–625. [Google Scholar]
- Shutov, A.; Ihlemann, J. Analysis of some basic approaches to finite strain elastoplasticity in view of reference change. Int. J. Plast. 2014, 63, 183–197. [Google Scholar] [CrossRef]
- Hartley, C.; Mishin, Y. Characterization and visualization of the lattice misfit associated with dislocation cores. Acta Mater. 2005, 53, 1313–1321. [Google Scholar] [CrossRef]
- Nye, J.F. Some geometrical relations in dislocated crystals. Acta Metall. 1953, 1, 153–162. [Google Scholar] [CrossRef]
- Kröner, E. Benefits and shortcomings of the continuous theory of dislocations. Int. J. Solids Struct. 2001, 38, 1115–1134. [Google Scholar] [CrossRef]
- Forest, S.; Barbe, F.; Cailletaud, G. Cosserat modelling of size effects in the mechanical behaviour of polycrystals and multi-phase materials. Int. J. Solids Struct. 2000, 37, 7105–7126. [Google Scholar] [CrossRef]
- Gurtin, M.E. A gradient theory of single-crystal viscoplasticity that accounts for geometrically necessary dislocations. J. Mech. Phys. Solids 2002, 50, 5–32. [Google Scholar] [CrossRef]
- Evers, L.P.; Brekelmans, W.A.; Geers, M.G. Scale dependent crystal plasticity framework with dislocation density and grain boundary effects. Int. J. Solids Struct. 2004, 41, 5209–5230. [Google Scholar] [CrossRef]
- Evers, L.P.; Brekelmans, W.A.; Geers, M.G. Non-local crystal plasticity model with intrinsic SSD and GND effects. J. Mech. Phys. Solids 2004, 52, 2379–2401. [Google Scholar] [CrossRef]
- Ma, A.; Roters, F. A constitutive model for fcc single crystals based on dislocation densities and its application to uniaxial compression of aluminium single crystals. Acta Mater. 2004, 52, 3603–3612. [Google Scholar] [CrossRef]
- Han, C.S.; Gao, H.; Huang, Y.; Nix, W.D. Mechanism-based strain gradient crystal plasticity—I. Theory. J. Mech. Phys. Solids 2005, 53, 1188–1203. [Google Scholar] [CrossRef]
- Kuroda, M.; Tvergaard, V. Studies of scale dependent crystal viscoplasticity models. J. Mech. Phys. Solids 2006, 54, 1789–1810. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Anand, L.; Lele, S.P. Gradient single-crystal plasticity with free energy dependent on dislocation densities. J. Mech. Phys. Solids 2007, 55, 1853–1878. [Google Scholar] [CrossRef]
- Gurtin, M.E. A finite-deformation, gradient theory of single-crystal plasticity with free energy dependent on densities of geometrically necessary dislocations. Int. J. Plast. 2008, 24, 702–725. [Google Scholar] [CrossRef]
- Kuroda, M.; Tvergaard, V. On the formulations of higher-order strain gradient crystal plasticity models. J. Mech. Phys. Solids 2008, 56, 1591–1608. [Google Scholar] [CrossRef]
- Grilli, N.; Koslowski, M. The effect of crystal orientation on shock loading of single crystal energetic materials. Comput. Mater. Sci. 2018, 155, 235–245. [Google Scholar] [CrossRef]
- Zhang, M.; Nguyen, K.; Segurado, J.; Montáns, F. A multiplicative finite strain crystal plasticity formulation based on additive elastic corrector rates: Theory and numerical implementation. Int. J. Plast. 2020, 137, 102899. [Google Scholar] [CrossRef]
- Latorre, M.; Montáns, F. A new class of plastic flow evolution equations for anisotropic multiplicative elastoplasticity based on the notion of a corrector elastic strain rate. Appl. Math. Model. 2018, 55, 716–740. [Google Scholar] [CrossRef]
- Zhang, M.; Montáns, F. A simple formulation for large-strain cyclic hyperelasto-plasticity using elastic correctors. Theory and algorithmic implementation. Int. J. Plast. 2019, 113, 185–217. [Google Scholar] [CrossRef]
- Nguyen, K.; Sanz, M.A.; Montáns, F. Plane-stress constrained multiplicative hyperelasto-plasticity with nonlinear kinematic hardening. Consistent theory based on elastic corrector rates and algorithmic implementation. Int. J. Plast. 2020, 128, 102592. [Google Scholar] [CrossRef]
- Caminero, M.; Montáns, F.; Bathe, K.J. Modeling large strain anisotropic elasto-plasticity with logarithmic strain and stress measures. Comput. Struct. 2011, 89, 826–843. [Google Scholar] [CrossRef]
- Sánz, M.; Montáns, F.; Latorre, M. Computational anisotropic hardening multiplicative elastoplasticity based on the corrector elastic logarithmic strain rate. Comput. Methods Appl. Mech. Eng. 2017, 320, 82–121. [Google Scholar] [CrossRef][Green Version]
- Latorre, M.; Montáns, F. Stress and strain mapping tensors and general work-conjugacy in large strain continuum mechanics. Appl. Math. Model. 2016, 40, 3938–3950. [Google Scholar] [CrossRef]
- Peirce, D.; Asaro, R.J.; Needleman, A. Material rate dependence and localized deformation in crystalline solids. Acta Metall. 1983, 31, 1951–1976. [Google Scholar] [CrossRef]
- Needleman, A.; Asaro, R.J.; Lemonds, J.; Peirce, D. Finite element analysis of crystalline solids. Comput. Methods Appl. Mech. Eng. 1985, 52, 689–708. [Google Scholar] [CrossRef]
- Fivel, M.; Tabourot, L.; Rauch, E.; Canova, G. Identification through mesoscopic simulations of macroscopic parameters of physically based constitutive equations for the plastic behavior of FCC single crystals. J. Phys. IV 1998, 8, 151–158. [Google Scholar]
- Groh, S.; Maron, E.; Horstemeyer, M.; Zbib, H. Multiscale modeling of the plasticity in an aluminum single crystal. Int. J. Plast. 2009, 25, 1456–1473. [Google Scholar] [CrossRef]
- Meric, L.; Poubanne, P.; Cailletaud, G. Single Crystal Modeling for Structural Calculations: Part 1—Model Presentation. J. Eng. Mater. Technol. 1991, 113, 162–170. [Google Scholar] [CrossRef]
- Cailletaud, G. A micromechanical approach to inelastic behaviour of metals. Int. J. Plast. 1992, 8, 55–73. [Google Scholar] [CrossRef]
- Hu, Z.; Rauch, E.F.; Teodosiu, C. Work-hardening behavior of mild steel under stress reversal at large strains. Int. J. Plast. 1992, 8, 839–856. [Google Scholar] [CrossRef]
- Hasija, V.; Ghosh, S.; Mills, M.J.; Joseph, D.S. Deformation and creep modeling in polycrystalline Ti-6Al alloys. Acta Mater. 2003, 51, 4533–4549. [Google Scholar] [CrossRef]
- Venkatramani, G.; Ghosh, S.; Mills, M. A size-dependent crystal plasticity finite-element model for creep and load shedding in polycrystalline titanium alloys. Acta Mater. 2007, 55, 3971–3986. [Google Scholar] [CrossRef]
- Cruzado, A.; LLorca, J.; Segurado, J. Modeling cyclic deformation of inconel 718 superalloy by means of crystal plasticity and computational homogenization. Int. J. Solids Struct. 2017, 122–123, 148–161. [Google Scholar] [CrossRef]
- Tome, C.; Canova, G.R.; Kocks, U.F.; Christodoulou, N.; Jonas, J.J. The relation between macroscopic and microscopic strain hardening in F.C.C. polycrystals. Acta Metall. 1984, 32, 1637–1653. [Google Scholar] [CrossRef]
- Bassani, J.L.; Wu, T.Y. Latent hardening in single crystals. II. Analytical characterization and predictions. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1991, 435, 21–41. [Google Scholar] [CrossRef]
- Polmear, I.J. From Traditional Alloys to Nanocrystals; Butterworth-Heinemann: Burlington, MA, USA, 2006. [Google Scholar]
- Estrin, Y.; Mecking, H. A unified phenomenological description of work hardening and creep based on one-parameter models. Acta Metall. 1984, 32, 57–70. [Google Scholar] [CrossRef]
- Estrin, Y. Dislocation-Density–Related Constitutive Modeling. In Unified Constitutive Laws of Plastic Deformation; Krausz, A., Krausz, K., Eds.; Academic Press: Cambridge, MA, USA, 1996; pp. 69–106. [Google Scholar] [CrossRef]
- Mecking, H.; Kocks, U.F. Kinetics of flow and strain-hardening. Acta Metall. 1981, 29, 1865–1875. [Google Scholar] [CrossRef]
- Devincre, B.; Hoc, T.; Kubin, L. Dislocation mean free paths and strain hardening of crystals. Science 2008, 320, 1745–1748. [Google Scholar] [CrossRef]
- Cheong, K.S.; Busso, E.P. Discrete dislocation density modelling of single phase FCC polycrystal aggregates. Acta Mater. 2004, 52, 5665–5675. [Google Scholar] [CrossRef]
- De Sansal, C.; Devincre, B.; Kubin, L. Grain size strengthening in microcrystalline copper: A three-dimensional dislocation dynamics simulation. Key Eng. Mater. 2010, 423, 25–32. [Google Scholar] [CrossRef]
- Estrin, Y.; Kubin, L.P. Local strain hardening and nonuniformity of plastic deformation. Acta Metall. 1986, 34, 2455–2464. [Google Scholar] [CrossRef]
- Kameda, T.; Zikry, M.A. Three dimensional dislocation-based crystalline constitutive formulation for ordered intermetallics. Scr. Mater. 1998, 38, 631–636. [Google Scholar] [CrossRef]
- Rezvanian, O.; Zikry, M.A.; Rajendran, A.M. Statistically stored, geometrically necessary and grain boundary dislocation densities: Microstructural representation and modelling. Proc. R. Soc. A Math. Phys. Eng. Sci. 2007, 463, 2833–2853. [Google Scholar] [CrossRef]
- Shi, J.; Zikry, M.A. Grain-boundary interactions and orientation effects on crack behavior in polycrystalline aggregates. Int. J. Solids Struct. 2009, 46, 3914–3925. [Google Scholar] [CrossRef]
- Shanthraj, P.; Zikry, M.A. Dislocation density evolution and interactions in crystalline materials. Acta Mater. 2011, 59, 7695–7702. [Google Scholar] [CrossRef]
- Orowan, E. Zur Kristallplastizität. I: Tieftemperaturplastizität und Beckersche Formel. Z. Phys. 1934, 89, 605–613. [Google Scholar] [CrossRef]
- Kocks, U. Thermodynamics and Kinetics of Slip, Volume 19 of Progress in Materials Science; Pergamon Press: Oxford, UK, 2003. [Google Scholar]
- Rodríguez-Galán, D.; Sabirov, I.; Segurado, J. Temperature and stain rate effect on the deformation of nanostructured pure titanium. Int. J. Plast. 2015, 70, 191–205. [Google Scholar] [CrossRef]
- Shahba, A.; Ghosh, S. Crystal plasticity FE modeling of Ti alloys for a range of strain-rates. Part I: A unified constitutive model and flow rule. Int. J. Plast. 2016, 87, 48–68. [Google Scholar] [CrossRef]
- Cailard, D.; Martin, J. Thermally Activated Mechanisms in Crystal Plasticity; Elsevier: Amsterdam, The Netherlands, 2003. [Google Scholar]
- Taylor, G.I. Plastic strain in metals. J. Inst. Met. 1938, 62, 307–324. [Google Scholar]
- Elkhodary, K.; Lee, W.; Sun, L.P.; Brenner, D.W.; Zikry, M.A. Deformation mechanisms of an Ω precipitate in a high-strength aluminum alloy subjected to high strain rates. J. Mater. Res. 2011, 26, 487–497. [Google Scholar] [CrossRef]
- Stelmashenko, N.A.; Walls, M.G.; Brown, L.M.; Milman, Y.V. Microindentations on W and Mo oriented single crystals: An STM study. Acta Metall. Mater. 1993, 41, 2855–2865. [Google Scholar] [CrossRef]
- Wang, Y.; Raabe, D.; Klüber, C.; Roters, F. Orientation dependence of nanoindentation pile-up patterns and of nanoindentation microtextures in copper single crystals. Acta Mater. 2004, 52, 2229–2238. [Google Scholar] [CrossRef]
- Zaafarani, N.; Raabe, D.; Singh, R.N.; Roters, F.; Zaefferer, S. Three-dimensional investigation of the texture and microstructure below a nanoindent in a Cu single crystal using 3D EBSD and crystal plasticity finite element simulations. Acta Mater. 2006, 54, 1863–1876. [Google Scholar] [CrossRef]
- Zaafarani, N.; Raabe, D.; Roters, F.; Zaefferer, S. On the origin of deformation-induced rotation patterns below nanoindents. Acta Mater. 2008, 56, 31–42. [Google Scholar] [CrossRef]
- Sánchez-Martín, R.; Pérez-Prado, M.T.; Segurado, J.; Bohlen, J.; Gutiérrez-Urrutia, I.; Llorca, J.; Molina-Aldareguia, J.M. Measuring the critical resolved shear stresses in Mg alloys by instrumented nanoindentation. Acta Mater. 2014, 71, 283–292. [Google Scholar] [CrossRef]
- Motz, C.; Schöberl, T.; Pippan, R. Mechanical properties of micro-sized copper bending beams machined by the focused ion beam technique. Acta Mater. 2005, 53, 4269–4279. [Google Scholar] [CrossRef]
- Weber, F.; Schestaknow, I.; Roters, F.; Raabe, D. Texture Evolution During Bending of a Single Crystal Copper Nanowire Studied by EBSD and Crystal Plasticity Finite Element Simulations. Adv. Eng. Mater. 2008, 10, 737–741. [Google Scholar] [CrossRef]
- Kiener, D.; Grosinger, W.; Dehm, G.; Pippan, R. A further step towards an understanding of size-dependent crystal plasticity: In situ tension experiments of miniaturized single-crystal copper samples. Acta Mater. 2008, 56, 580–592. [Google Scholar] [CrossRef]
- Gong, J.; Wilkinson, A.J. A microcantilever investigation of size effect, solid-solution strengthening and second-phase strengthening for a prism slip in alpha-Ti. Acta Mater. 2011, 59, 5970–5981. [Google Scholar] [CrossRef]
- Zhou, C.; Beyerlein, I.J.; Lesar, R. Plastic deformation mechanisms of fcc single crystals at small scales. Acta Mater. 2011, 59, 7673–7682. [Google Scholar] [CrossRef]
- Norton, A.D.; Falco, S.; Young, N.; Severs, J.; Todd, R.I. Microcantilever investigation of fracture toughness and subcritical crack growth on the scale of the microstructure in Al2O3. J. Eur. Ceram. Soc. 2015, 35, 4521–4533. [Google Scholar] [CrossRef]
- Raabe, D.; Ma, D.; Roters, F. Effects of initial orientation, sample geometry and friction on anisotropy and crystallographic orientation changes in single crystal microcompression deformation: A crystal plasticity finite element study. Acta Mater. 2007, 55, 4567–4583. [Google Scholar] [CrossRef]
- Ashby, M.F. The deformation of plastically non-homogeneous materials. Philos. Mag. 1970, 21, 399–424. [Google Scholar] [CrossRef]
- Evers, L.P.; Parks, D.M.; Brekelmans, W.A.; Geers, M.G. Crystal plasticity model with enhanced hardening by geometrically necessary dislocation accumulation. J. Mech. Phys. Solids 2002, 50, 2403–2424. [Google Scholar] [CrossRef]
- Gao, H.; Huang, Y. Geometrically necessary dislocation and size-dependent plasticity. Scr. Mater. 2003, 48, 113–118. [Google Scholar] [CrossRef]
- Kuroda, M. Plasticity and Beyond: Microstructures, Crystal-Plasticity and Phase Transitions; Chapter on Scale-Dependent Crystal Plasticity Models; CSIM Courses and Lectures; Springer: Berlin, Germany, 2014. [Google Scholar]
- Fleck, N.A.; Muller, G.M.; Ashby, M.F.; Hutchinson, J.W. Strain gradient plasticity: Theory and experiment. Acta Metall. Mater. 1994, 42, 475–487. [Google Scholar] [CrossRef]
- Gao, H.; Huang, Y.; Nix, W.D.; Hutchinson, J.W. Mechanism-based strain gradient plasticity—I. Theory. J. Mech. Phys. Solids 1999, 47, 1239–1263. [Google Scholar] [CrossRef]
- Cheong, K.S.; Busso, E.P.; Arsenlis, A. A study of microstructural length scale effects on the behaviour of FCC polycrystals using strain gradient concepts. Int. J. Plast. 2005, 21, 1797–1814. [Google Scholar] [CrossRef]
- Busso, E.P.; Meissonnier, F.T.; O’Dowd, N.P. Gradient-dependent deformation of two-phase single crystals. J. Mech. Phys. Solids 2000, 48, 2333–2361. [Google Scholar] [CrossRef]
- Dunne, F.P.; Rugg, D.; Walker, A. Lengthscale-dependent, elastically anisotropic, physically-based hcp crystal plasticity: Application to cold-dwell fatigue in Ti alloys. Int. J. Plast. 2007, 23, 1061–1083. [Google Scholar] [CrossRef]
- Han, C.S.; Ma, A.; Roters, F.; Raabe, D. A Finite Element approach with patch projection for strain gradient plasticity formulations. Int. J. Plast. 2007, 23, 690–710. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Needleman, A. Boundary conditions in small-deformation, single-crystal plasticity that account for the Burgers vector. J. Mech. Phys. Solids 2005, 53, 1–31. [Google Scholar] [CrossRef]
- Yefimov, S.; Van Der Giessen, E.; Groma, I. Bending of a single crystal: Discrete dislocation and nonlocal crystal plasticity simulations. Model. Simul. Mater. Sci. Eng. 2004, 12, 1069–1086. [Google Scholar] [CrossRef]
- Bardella, L. A deformation theory of strain gradient crystal plasticity that accounts for geometrically necessary dislocations. J. Mech. Phys. Solids 2006, 54, 128–160. [Google Scholar] [CrossRef]
- Bayley, C.J.; Brekelmans, W.A.; Geers, M.G. A comparison of dislocation induced back stress formulations in strain gradient crystal plasticity. Int. J. Solids Struct. 2006, 43, 7268–7286. [Google Scholar] [CrossRef]
- Borg, U.; Niordson, C.F.; Kysar, J.W. Size effects on void growth in single crystals with distributed voids. Int. J. Plast. 2008, 24, 688–701. [Google Scholar] [CrossRef]
- Bardella, L.; Segurado, J.; Panteghini, A.; Llorca, J. Latent hardening size effect in small-scale plasticity. Model. Simul. Mater. Sci. Eng. 2013, 21. [Google Scholar] [CrossRef]
- Niordson, C.F.; Kysar, J.W. Computational strain gradient crystal plasticity. J. Mech. Phys. Solids 2014, 62, 31–47. [Google Scholar] [CrossRef]
- Sandfeld, S.; Hochrainer, T.; Gumbsch, P.; Zaiser, M. Numerical implementation of a 3D continuum theory of dislocation dynamics and application to micro-bending. Philos. Mag. 2010, 90, 3697–3728. [Google Scholar] [CrossRef]
- Groma, I. Link between the microscopic and mesoscopic length-scale description of the collective behavior of dislocations. Phys. Rev. B Condens. Matter Mater. Phys. 1997, 56, 5807–5813. [Google Scholar] [CrossRef]
- El-Azab, A. Statistical mechanics treatment of the evolution of dislocation distributions in single crystals. Phys. Rev. B Condens. Matter Mater. Phys. 2000, 61, 11956–11966. [Google Scholar] [CrossRef]
- Acharya, A. A model of crystal plasticity based on the theory of continuously distributed dislocations. J. Mech. Phys. Solids 2001, 49, 761–784. [Google Scholar] [CrossRef]
- Acharya, A.; Roy, A. Size effects and idealized dislocation microstructure at small scales: Predictions of a Phenomenological model of Mesoscopic Field Dislocation Mechanics: Part I. J. Mech. Phys. Solids 2006, 54, 1687–1710. [Google Scholar] [CrossRef]
- Hochrainer, T.; Zaiser, M.; Gumbsch, P. A three-dimensional continuum theory of dislocation systems: Kinematics and mean-field formulation. Philos. Mag. 2007, 87, 1261–1282. [Google Scholar] [CrossRef]
- Hochrainer, T.; Zaiser, M.; Gumbsch, P. Dislocation transport and line length increase in averaged descriptions of dislocations. AIP Conf. Proc. 2009, 1168, 1133–1136. [Google Scholar] [CrossRef]
- Xia, S.X.; El-Azab, A. A preliminary investigation of dislocation cell structure formation in metals using continuum dislocation dynamics. IOP Conf. Ser. Mater. Sci. Eng. 2015, 89. [Google Scholar] [CrossRef]
- Hochrainer, T. Thermodynamically consistent continuum dislocation dynamics. J. Mech. Phys. Solids 2016, 88, 12–22. [Google Scholar] [CrossRef]
- Sarma, G.; Zacharia, T. Integration algorithm for modeling the elasto-viscoplastic response of polycrystalline materials. J. Mech. Phys. Solids 1999, 47, 1219–1238. [Google Scholar] [CrossRef]
- Maniatty, A.; Dawson, P.; Lee, Y. A time integration algorithm for elasto-viscoplastic cubic crystals applied to modelling polycrystalline deformation. Int. J. Numer. Methods Eng. 1992, 35, 1565–1588. [Google Scholar] [CrossRef]
- Roters, F.; Diehl, M.; Shanthraj, P.; Eisenlohr, P.; Reuber, C.; Wong, S.L.; Maiti, T.; Ebrahimi, A.; Hochrainer, T.; Fabritius, H.O.; et al. DAMASK: The Düsseldorf Advanced Material Simulation Kit for modeling multi-physics crystal plasticity, thermal, and damage phenomena from the single crystal up to the component scale. Comput. Mater. Sci. 2019, 158, 420–478. [Google Scholar] [CrossRef]
- Arsenlis, A.; Parks, D.M.; Becker, R.; Bulatov, V.V. On the evolution of crystallographic dislocation density in non-homogeneously deforming crystals. J. Mech. Phys. Solids 2004, 52, 1213–1246. [Google Scholar] [CrossRef]
- Borja, R.I.; Wren, J.R. Discrete micromechanics of elastoplastic crystals. Int. J. Numer. Methods Eng. 1993, 36, 3815–3840. [Google Scholar] [CrossRef]
- Miehe, C. Exponential map algorithm for stress updates in anisotropic multiplicative elastoplasticity for single crystals. Int. J. Numer. Methods Eng. 1996, 39, 3367–3390. [Google Scholar] [CrossRef]
- Asaro, R.; Needleman, A. Texture development and strain hardening in rate dependent polycrystals. Acta Metall. 1985, 33, 923–953. [Google Scholar] [CrossRef]
- Castañeda, P.P. The effective mechanical properties of nonlinear isotropic composites. J. Mech. Phys. Solids 1991, 39, 45–71. [Google Scholar] [CrossRef]
- Lebensohn, R.A.; Tomé, C.N. A self-consistent anisotropic approach for the simulation of plastic deformation and texture development of polycrystals: Application to zirconium alloys. Acta Metall. Mater. 1993, 41, 2611–2624. [Google Scholar] [CrossRef]
- Lebensohn, R.; Uhlenhut, H.; Hartig, C.; Mecking, H. Plastic flow of γ-TiAl-Based polysynthetically twinned crystals: Micromechanical modeling and experimental validation. Acta Mater. 1998, 46, 4701–4709. [Google Scholar] [CrossRef]
- Masson, R.; Bornert, M.; Suquet, P.; Zaoui, A. Affine formulation for the prediction of the effective properties of nonlinear composites and polycrystals. J. Mech. Phys. Solids 2000, 48, 1203–1227. [Google Scholar] [CrossRef]
- Lebensohn, R.A.; Tomé, C.N.; Castañeda, P.P. Self-consistent modelling of the mechanical behaviour of viscoplastic polycrystals incorporating intragranular field fluctuations. Philos. Mag. 2007, 87, 4287–4322. [Google Scholar] [CrossRef]
- Bellec, V.; Bøe, R.; Rise, L.; Thorsnes, T. Influence of bottom currents on the Lofoten continental margin, North Norway. Rend. Online Soc. Geol. Ital. 2009, 7, 155–157. [Google Scholar]
- Lebensohn, R.A.; Zecevic, M.; Knezevic, M.; McCabe, R.J. Average intragranular misorientation trends in polycrystalline materials predicted by a viscoplastic self-consistent approach. Acta Mater. 2016, 104, 228–236. [Google Scholar] [CrossRef]
- Segurado, J.; Lebensohn, R.A.; Llorca, J.; Tomé, C.N. Multiscale modeling of plasticity based on embedding the viscoplastic self-consistent formulation in implicit finite elements. Int. J. Plast. 2012, 28, 124–140. [Google Scholar] [CrossRef]
- Knezevic, M.; Crapps, J.; Beyerlein, I.J.; Coughlin, D.R.; Clarke, K.D.; McCabe, R.J. Anisotropic modeling of structural components using embedded crystal plasticity constructive laws within finite elements. Int. J. Mech. Sci. 2016, 105, 227–238. [Google Scholar] [CrossRef]
- Zecevic, M.; Beyerlein, I.J.; Knezevic, M. Coupling elasto-plastic self-consistent crystal plasticity and implicit finite elements: Applications to compression, cyclic tension-compression, and bending to large strains. Int. J. Plast. 2017, 93, 187–211. [Google Scholar] [CrossRef]
- Knezevic, M.; Beyerlein, I.J. Multiscale Modeling of Microstructure-Property Relationships of Polycrystalline Metals during Thermo-Mechanical Deformation. Adv. Eng. Mater. 2018, 1700956, 1–19. [Google Scholar] [CrossRef]
- Borja, R.I.; Rahmani, H. Discrete micromechanics of elastoplastic crystals in the finite deformation range. Comput. Methods Appl. Mech. Eng. 2014, 275, 234–263. [Google Scholar] [CrossRef]
- Miehe, C. Multisurface thermoplasticity for single crystals at large strains in terms of Eulerian vector updates. Int. J. Solids Struct. 1996, 33, 3103–3130. [Google Scholar] [CrossRef]
- Weber, G.; Anand, L. Finite deformation constitutive equations and a time integration procedure for isotropic, hyperelastic-viscoplastic solids. Comput. Methods Appl. Mech. Eng. 1990, 79, 173–202. [Google Scholar] [CrossRef]
- Nakamachi, E.; Xie, C.L.; Harimoto, M. Drawability assessment of BCC steel sheet by using elastic/crystalline viscoplastic finite element analyses. Int. J. Mech. Sci. 2001, 43, 631–652. [Google Scholar] [CrossRef]
- Helming, K.; Eschner, T. A new approach to texture analysis of multiphase materials using a texture component model. Cryst. Res. Technol. 1990, 25, K203–K208. [Google Scholar] [CrossRef]
- Raabe, D.; Roters, F. Using texture components in crystal plasticity finite element simulations. Int. J. Plast. 2004, 20, 339–361. [Google Scholar] [CrossRef]
- Zhao, Z.; Roters, F.; Mao, W.; Raabe, D. Introduction of a texture component crystal plasticity finite element method for anisotropy simulations. Adv. Eng. Mater. 2001, 3, 984–990. [Google Scholar] [CrossRef]
- Raabe, D.; Zhao, Z.; Roters, F. A finite element method on the basis of texture components for fast predictions of anisotropic forming operations. Steel Res. 2001, 72, 421–426. [Google Scholar] [CrossRef]
- Raabe, D.; Sachtleber, M.; Zhao, Z.; Roters, F.; Zaefferer, S. Micromechanical and macromechanical effects in grain scale polycrystal plasticity experimentation and simulation. Acta Mater. 2001, 49, 3433–3441. [Google Scholar] [CrossRef]
- Zecevic, M.; Knezevic, M. Modeling of Sheet Metal Forming Based on Implicit Embedding of the Elasto-Plastic Self-Consistent Formulation in Shell Elements: Application to Cup Drawing of AA6022-T4. JOM 2017, 69, 922–929. [Google Scholar] [CrossRef]
- Zecevic, M.; Knezevic, M. A new visco-plastic self-consistent formulation implicit in dislocation-based hardening within implicit finite elements: Application to high strain rate and impact deformation of tantalum. Comput. Methods Appl. Mech. Eng. 2018, 341, 888–916. [Google Scholar] [CrossRef]
- Maudlin, P.J.; Gray, G.T.; Cady, C.M.; Kaschner, G.C. High-rate material modelling and validation using the Taylor cylinder impact test. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1999, 357, 1707–1729. [Google Scholar] [CrossRef]
- Maudlin, P.J.; Bingert, J.F.; House, J.W.; Chen, S.R. On the modeling of the Taylor cylinder impact test for orthotropic textured materials: Experiments and simulations. Int. J. Plast. 1999, 15, 139–166. [Google Scholar] [CrossRef]
- Zecevic, M.; Knezevic, M.; Beyerlein, I.J.; McCabe, R.J. Texture formation in orthorhombic alpha-uranium under simple compression and rolling to high strains. J. Nucl. Mater. 2016, 473, 143–156. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, K.; Zhang, M.; Amores, V.J.; Sanz, M.A.; Montáns, F.J. Computational Modeling of Dislocation Slip Mechanisms in Crystal Plasticity: A Short Review. Crystals 2021, 11, 42. https://doi.org/10.3390/cryst11010042
Nguyen K, Zhang M, Amores VJ, Sanz MA, Montáns FJ. Computational Modeling of Dislocation Slip Mechanisms in Crystal Plasticity: A Short Review. Crystals. 2021; 11(1):42. https://doi.org/10.3390/cryst11010042
Chicago/Turabian StyleNguyen, Khanh, Meijuan Zhang, Víctor Jesús Amores, Miguel A. Sanz, and Francisco J. Montáns. 2021. "Computational Modeling of Dislocation Slip Mechanisms in Crystal Plasticity: A Short Review" Crystals 11, no. 1: 42. https://doi.org/10.3390/cryst11010042
APA StyleNguyen, K., Zhang, M., Amores, V. J., Sanz, M. A., & Montáns, F. J. (2021). Computational Modeling of Dislocation Slip Mechanisms in Crystal Plasticity: A Short Review. Crystals, 11(1), 42. https://doi.org/10.3390/cryst11010042





