Single-Shot Measurement of Post-Pulse-Generated Pre-Pulse in High-Power Laser Systems
Abstract
1. Introduction
2. Materials and Methods
2.1. Laser System Layout and Experimental Condition
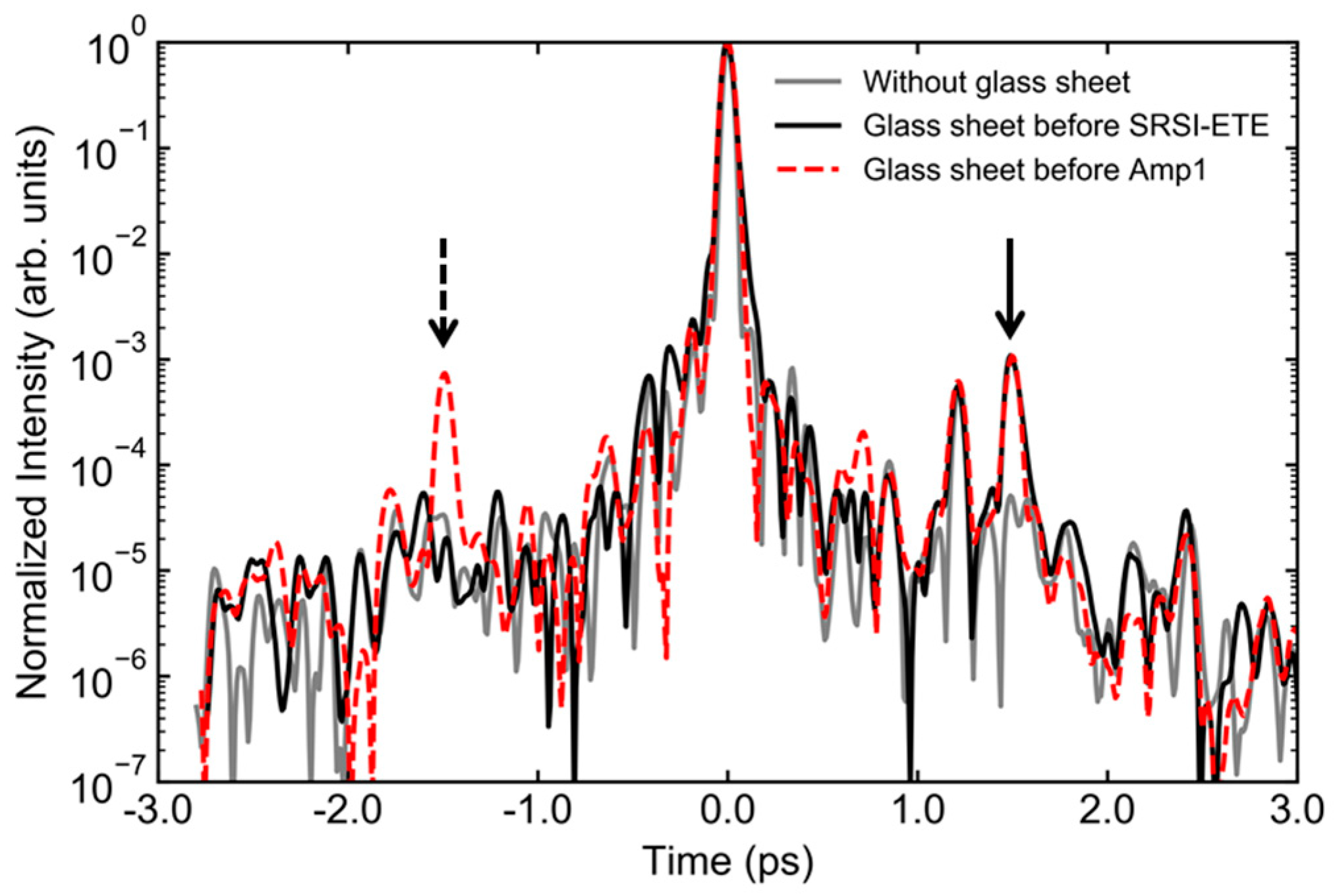
2.2. Methods for Measurement of Temporal Contrast
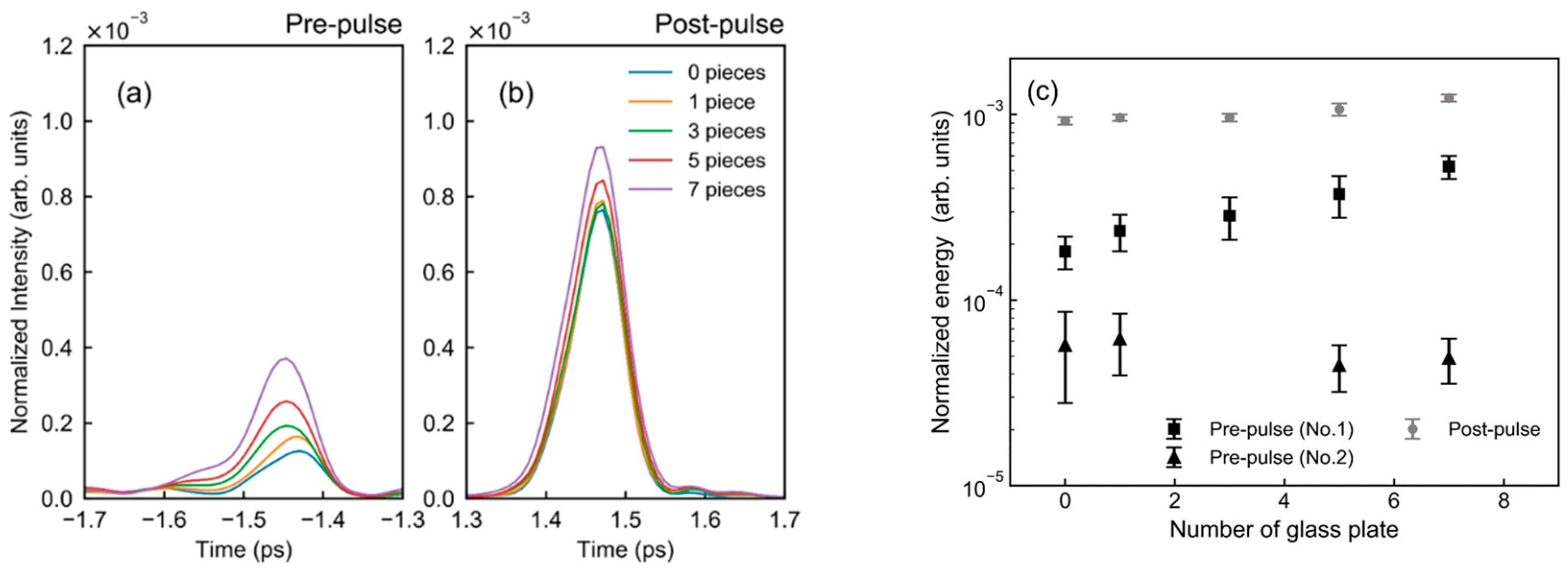
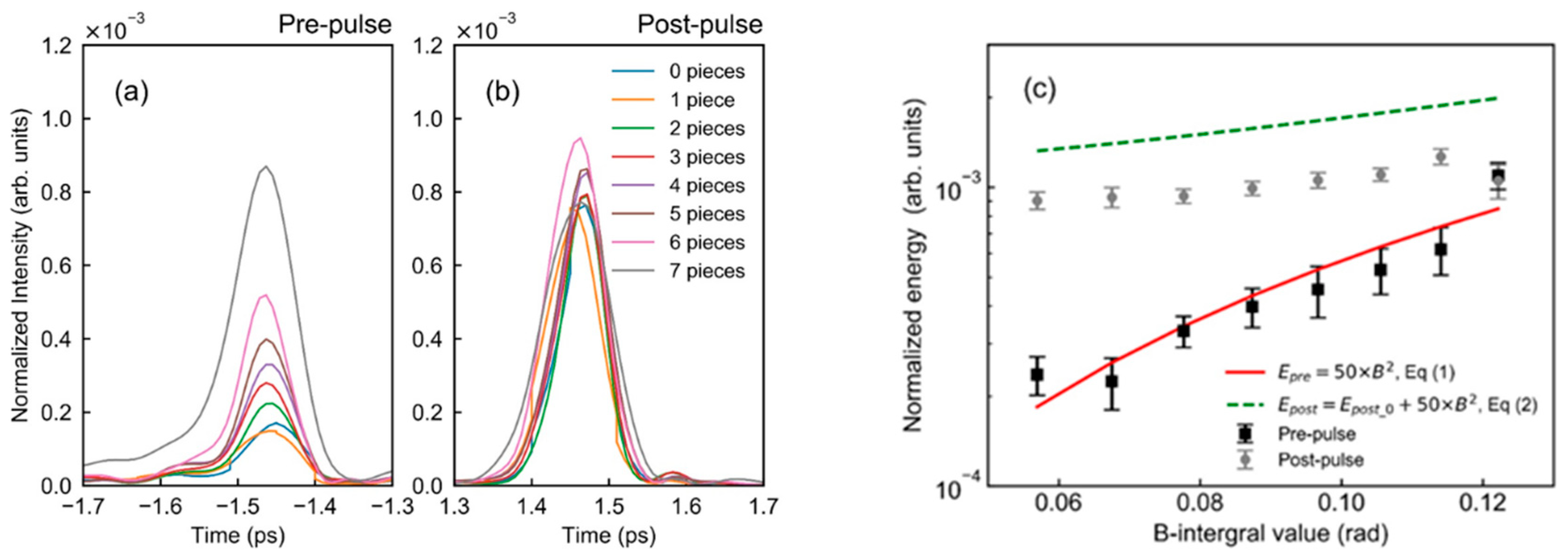
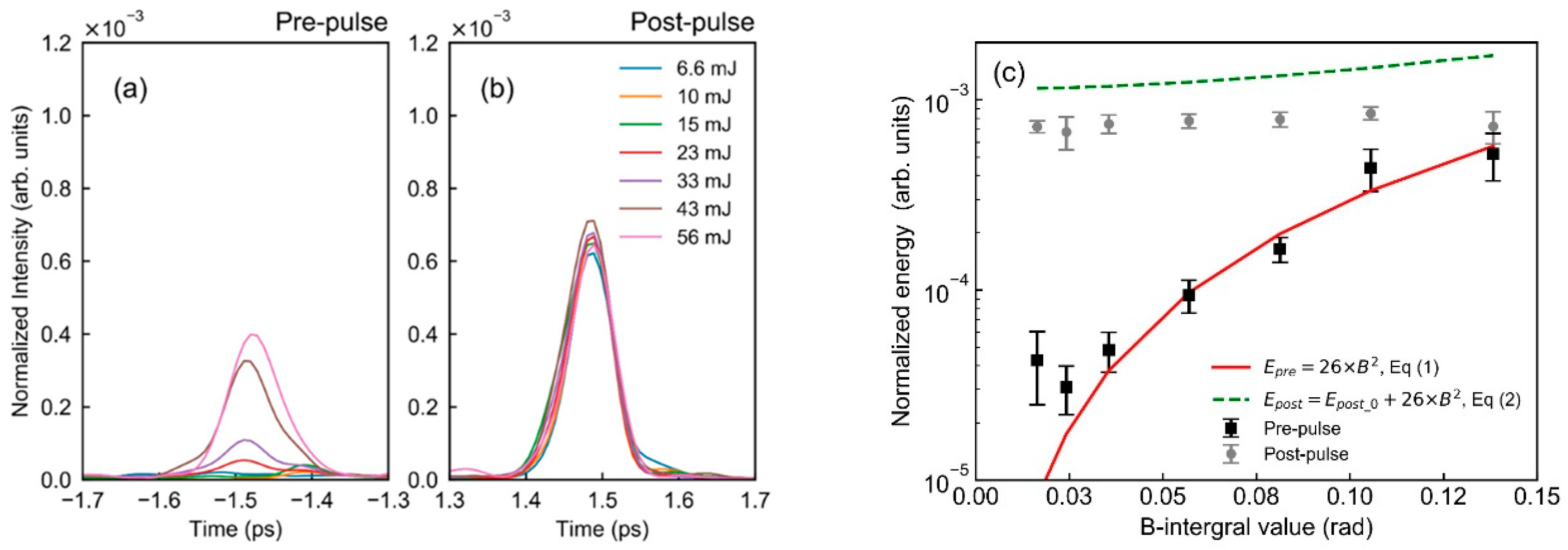
2.3. Method of Calculating the B-Integral
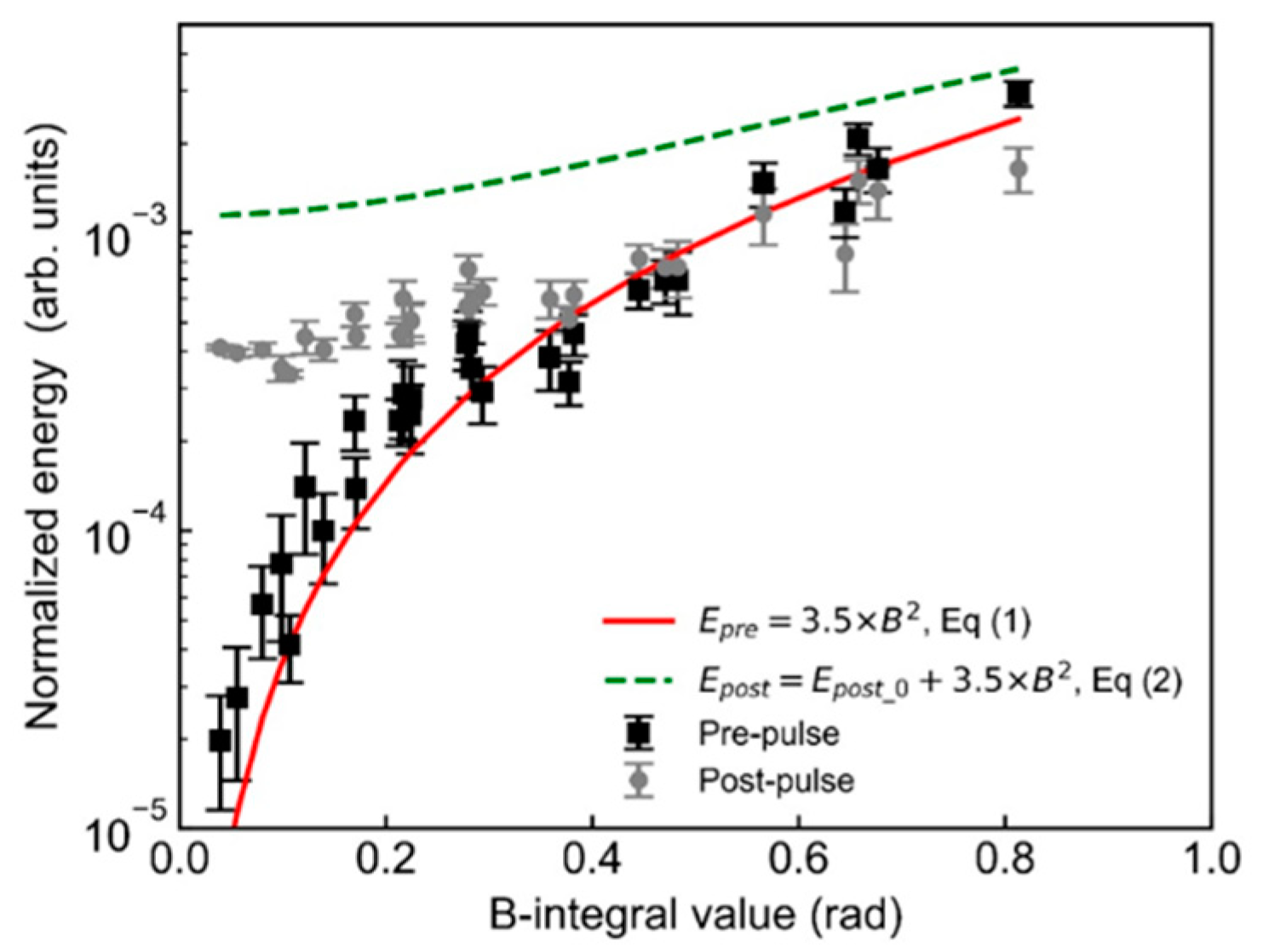
2.4. Pre-Pulse Intensity Generated by the Post-Pulse
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Strickland, D.; Mourou, G. Compression of amplified chirped optical pulses. Opt. Commun. 1985, 56, 219–221. [Google Scholar] [CrossRef]
- Extreme Light Infrastructure. Available online: https://eli-laser.eu/ (accessed on 1 June 2020).
- Danson, C.; Hillier, D.; Hopps, N.; Neely, D. Petawatt class lasers worldwide. High Power Laser Sci. Eng. 2015, 3, 3. [Google Scholar] [CrossRef]
- Kaluza, M.; Schreiber, J.; Santala, M.I.K.; Tsakiris, G.D.; Eidmann, K.; Meyer-Ter-Vehn, J.; Witte, K.J. Influence of the Laser Prepulse on Proton Acceleration in Thin-Foil Experiments. Phys. Rev. Lett. 2004, 93, 045003. [Google Scholar] [CrossRef] [PubMed]
- Metzkes-Ng, J.; Kluge, T.; Zeil, K.; Bussmann, M.; Kraft, S.; Cowan, T.E.; Schramm, U. Experimental observation of transverse modulations in laser-driven proton beams. New J. Phys. 2014, 16, 23008. [Google Scholar] [CrossRef]
- Mangles, S.P.D.; Thomas, A.; Kaluza, M.C.; Lundh, O.; Lindau, F.; Persson, A.; Najmudin, Z.; Wahlström, C.-G.; Murphy, C.D.; Kamperidis, C.; et al. Effect of laser contrast ratio on electron beam stability in laser wakefield acceleration experiments. Plasma Phys. Control. Fusion 2006, 48, B83–B90. [Google Scholar] [CrossRef]
- Ivanov, V.V.; Maksimchuk, A.; Mourou, G. Amplified spontaneous emission in a Ti:sapphire regenerative amplifier. Appl. Opt. 2003, 42, 7231–7234. [Google Scholar] [CrossRef]
- Kane, S.; Squier, J. Fourth-order-dispersion limitations of aberration-free chirped-pulse amplification systems. J. Opt. Soc. Am. B 1997, 14, 1237–1244. [Google Scholar] [CrossRef]
- Hooker, C.; Tang, Y.; Chekhlov, O.; Collier, J.; Divall, E.; Ertel, K.; Hawkes, S.; Parry, B.; Rajeev, P.P. Improving coherent contrast of petawatt laser pulses. Opt. Express 2011, 19, 2193–2203. [Google Scholar] [CrossRef]
- Didenko, N.V.; Konyashchenko, A.V.; Lutsenko, A.; Tenyakov, S.Y. Contrast degradation in a chirped-pulse amplifier due to generation of prepulses by postpulses. Opt. Express 2008, 16, 3178. [Google Scholar] [CrossRef]
- Jackson, M.K.; Boyer, G.R.; Paye, J.; Franco, M.A.; Mysyrowicz, A. Temporal diffraction by nonlinear interaction in optical fibers. Opt. Lett. 1992, 17, 1770–1772. [Google Scholar] [CrossRef]
- Liu, X.; Wagner, R.; Maksimchuk, A.; Goodman, E.; Workman, J.; Umstadter, D.; Migus, A. Nonlinear temporal diffraction and frequency shifts resulting from pulse shaping in chirped-pulse amplification systems. Opt. Lett. 1995, 20, 1163–1165. [Google Scholar] [CrossRef] [PubMed]
- Perry, M.D.; Ditmire, T.; Stuart, B.C. Self-phase modulation in chirped-pulse amplification. Opt. Lett. 1994, 19, 2149–2151. [Google Scholar] [CrossRef] [PubMed]
- Schimpf, D.N.; Seise, E.; Limpert, J.; Tünnermann, A. The impact of spectral modulations on the contrast of pulses of nonlinear chirped-pulse amplification systems. Opt. Express 2008, 16, 10664–10674. [Google Scholar] [CrossRef]
- Kalashnikov, M.; Andreev, A.; Schönnagel, H. Limits of the temporal contrast for CPA lasers with beams of high aperture. Ultrafast Nonlinear Opt. 2009, 7501, 750104. [Google Scholar] [CrossRef]
- Keppler, S.; Hornung, M.; Bödefeld, R.; Kahle, M.; Hein, J.; Kaluza, M.C. All-reflective, highly accurate polarization rotator for high-power short-pulse laser systems. Opt. Express 2012, 20, 20742–20747. [Google Scholar] [CrossRef] [PubMed]
- Khodakovskiy, N.; Kalashnikov, M.; Gontier, E.; Falcoz, F.; Paul, P.-M. Degradation of picosecond temporal contrast of Ti:sapphire lasers with coherent pedestals. Opt. Lett. 2016, 41, 4441. [Google Scholar] [CrossRef]
- Kiriyama, H.; Miyasaka, Y.; Sagisaka, A.; Ogura, K.; Nishiuchi, M.; Pirozhkov, A.S.; Fukuda, Y.; Kando, M.; Kondo, K. Experimental investigation on the temporal contrast of pre-pulses by post-pulses in a petawatt laser facility. Opt. Lett. 2020, 45, 1100–1103. [Google Scholar] [CrossRef]
- Schimpf, D.; Seise, E.; Limpert, J.; Tünnermann, A. Decrease of pulse-contrast in nonlinear chirped-pulse amplification systems due to high-frequency spectral phase ripples. Opt. Express 2008, 16, 8876. [Google Scholar] [CrossRef]
- Khodakovskiy, N. Methods of Ultra-Fast Laser Contrast Diagnostics and Optimization. Ph.D. Thesis, Free University of Berlin, Berlin, Germany, 2020. [Google Scholar]
- Luan, S.; Hutchinson, M.H.R.; Smith, R.A.; Zhou, F. High dynamic range third-order correlation measurement of picosecond laser pulse shapes. Meas. Sci. Technol. 1993, 4, 1426–1429. [Google Scholar] [CrossRef]
- Oksenhendler, T.; Coudreau, S.; Forget, N.; Crozatier, V.; Grabielle, S.; Herzog, R.; Gobert, O.; Kaplan, D. Self-referenced spectral interferometry. Appl. Phys. A 2010, 99, 7–12. [Google Scholar] [CrossRef]
- Oksenhendler, T.; Bizouard, P.; Albert, O.; Bock, S.; Schramm, U. High dynamic, high resolution and wide range single shot temporal pulse contrast measurement. Opt. Express 2017, 25, 12588. [Google Scholar] [CrossRef] [PubMed]
- Kiriyama, H.; Pirozhkov, A.S.; Nishiuchi, M.; Fukuda, Y.; Ogura, K.; Sagisaka, A.; Miyasaka, Y.; Mori, M.; Sakaki, H.; Dover, N.P.; et al. High-contrast high-intensity repetitive petawatt laser. Opt. Lett. 2018, 43, 2595–2598. [Google Scholar] [CrossRef] [PubMed]
- Obst, L.; Metzkes-Ng, J.; Bock, S.; Cochran, G.E.; Cochran, T.E.; Oksenhendler, T.; Poole, P.L.; Prencipe, I.; Rehwald, M.; Rödel, C.; et al. On-shot characterization of single plasma mirror temporal contrast improvement. Plasma Phys. Control. Fusion 2018, 60, 054007. [Google Scholar] [CrossRef]
- Jullien, A.; Canova, L.; Albert, O.; Boschetto, D.; Antonucci, L.; Cha, Y.-H.; Rousseau, J.; Chaudet, P.; Cheriaux, G.; Etchepare, J.; et al. Spectral broadening and pulse duration reduction during cross-polarized wave generation: Influence of the quadratic spectral phase. Appl. Phys. A 2007, 87, 595–601. [Google Scholar] [CrossRef]
- Heebner, J.E.; Acree, R.L., Jr.; Alessi, D.; Barnes, A.I.; Bowers, M.W.; Browning, D.F.; Budge, T.S.; Burns, S.; Chang, L.S.; Christensen, K.S.; et al. Injection laser system for Advanced Radiographic Capability using chirped pulse amplification on the National Ignition Facility. Appl. Opt. 2019, 58, 8501–8510. [Google Scholar] [CrossRef] [PubMed]

| No. | Location of Glass Sheet | Laser Energy after Amp2 (mJ) | Pumped Amplifier | Number of Glass Plates | B-Integral (rad) |
|---|---|---|---|---|---|
| 1 | After Amp1 | 12–14 | Amp1 | 0–7 | 3.6–7.6 × 10−2 |
| 2 | After beam expander | 12–14 | Amp1 | 0–7 | 2.3–2.4 × 10−2 |
| 3 | After Amp1 | 20–23 | Amp1 | 0–7 | 5.7–12 × 10−2 |
| 4 | After Amp1 | 6.6–56 | Amp1 | N/A | 1.6–14 × 10−2 |
| 5 | Before Amp1 | 6.4–640 | Amp1 and 2 | N/A | 1.0–8.0 × 10−1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kon, A.; Nishiuchi, M.; Kiriyama, H.; Kando, M.; Bock, S.; Ziegler, T.; Pueschel, T.; Zeil, K.; Schramm, U.; Kondo, K. Single-Shot Measurement of Post-Pulse-Generated Pre-Pulse in High-Power Laser Systems. Crystals 2020, 10, 657. https://doi.org/10.3390/cryst10080657
Kon A, Nishiuchi M, Kiriyama H, Kando M, Bock S, Ziegler T, Pueschel T, Zeil K, Schramm U, Kondo K. Single-Shot Measurement of Post-Pulse-Generated Pre-Pulse in High-Power Laser Systems. Crystals. 2020; 10(8):657. https://doi.org/10.3390/cryst10080657
Chicago/Turabian StyleKon, Akira, Mamiko Nishiuchi, Hiromitsu Kiriyama, Masaki Kando, Stefan Bock, Tim Ziegler, Thomas Pueschel, Karl Zeil, Ulrich Schramm, and Kiminori Kondo. 2020. "Single-Shot Measurement of Post-Pulse-Generated Pre-Pulse in High-Power Laser Systems" Crystals 10, no. 8: 657. https://doi.org/10.3390/cryst10080657
APA StyleKon, A., Nishiuchi, M., Kiriyama, H., Kando, M., Bock, S., Ziegler, T., Pueschel, T., Zeil, K., Schramm, U., & Kondo, K. (2020). Single-Shot Measurement of Post-Pulse-Generated Pre-Pulse in High-Power Laser Systems. Crystals, 10(8), 657. https://doi.org/10.3390/cryst10080657






