Complex Disorder in Type-I Clathrates: Synthesis and Structural Characterization of A8GaxSn46−x (A = Rb, Cs; 6.9 < x < 7.5)
Abstract
1. Introduction
2. Materials and Methods
2.1. Synthesis
2.2. Powder X-Ray Diffraction (PXRD)
2.3. Single-Crystal X-Ray Diffraction (SCXRD)
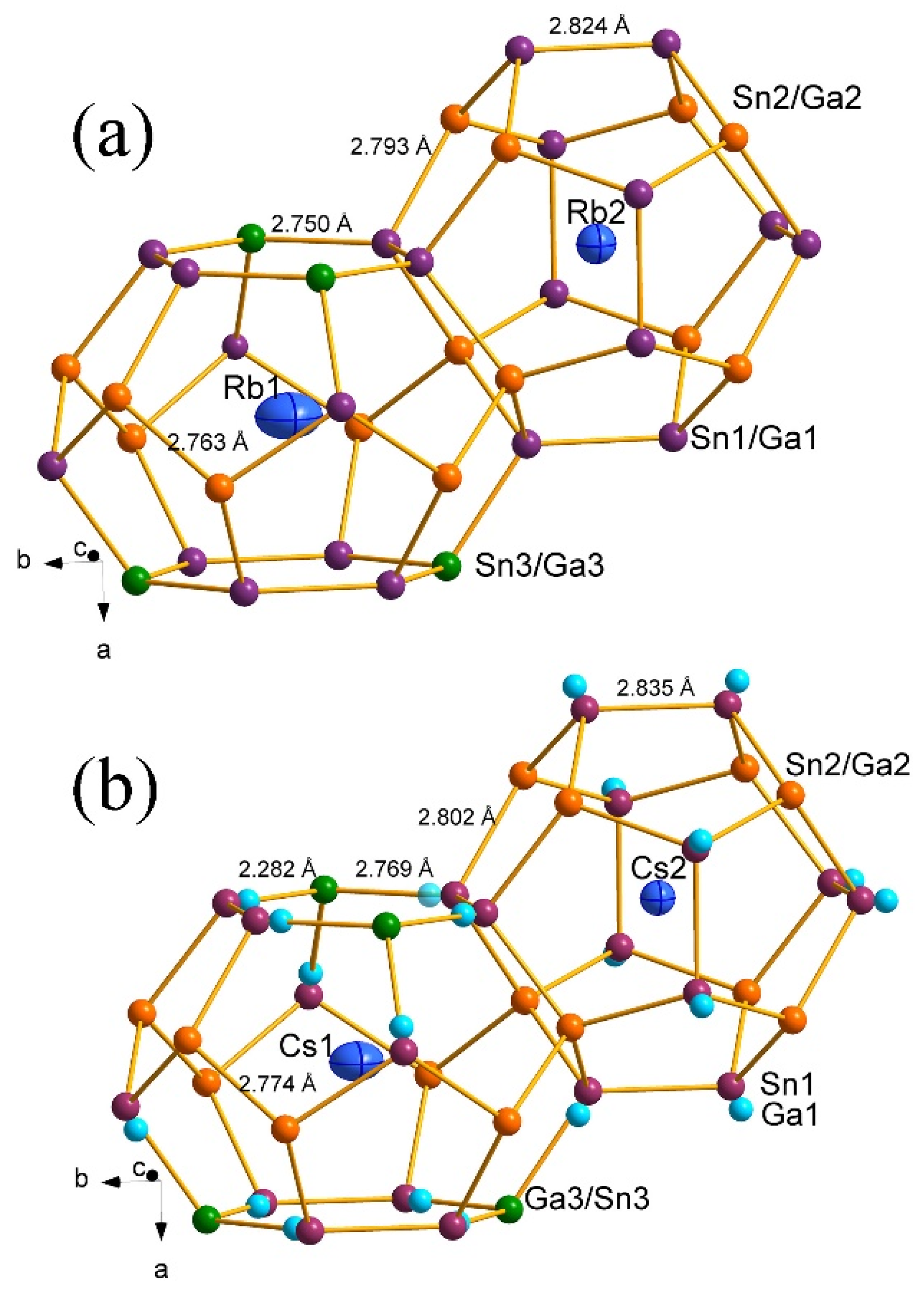
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Iversen, B.B.; Palmqvist, A.E.C.; Cox, D.E.; Nolas, G.S.; Stucky, G.D.; Blake, N.P.; Metiu, H. Why are clathrates good candidates for thermoelectric materials? J. Solid State Chem. 2000, 149, 455–458. [Google Scholar] [CrossRef]
- Shevelkov, A.V.; Kovnir, K. Zintl Clathrates; Springer: Berlin/Heidelberg, Germany, 2010; pp. 97–142. [Google Scholar]
- Christensen, M.; Johnsen, S.; Iversen, B.B. Thermoelectric clathrates of type I. Dalton Trans. 2010, 39, 978–992. [Google Scholar] [CrossRef] [PubMed]
- Kasper, J.S.; Hagenmuller, P.; Pouchard, M.; Cros, C. Clathrate Structure of Silicon Na8Si46 and NaxSi136 (x < 11). Science 1965, 150, 1713–1714. [Google Scholar] [CrossRef] [PubMed]
- Bobev, S.; Sevov, S.C. Clathrates of Group 14 with Alkali Metals: An Exploration. J. Solid State Chem. 2000, 153, 92–105. [Google Scholar] [CrossRef]
- Prokofiev, A.; Svagera, R.; Waas, M.; Weil, M.; Bernardi, J.; Paschen, S. Mechanism of Rare Earth Incorporation and Crystal Growth of Rare Earth Containing Type-I Clathrates. Cryst. Growth Des. 2016, 16, 25–33. [Google Scholar] [CrossRef]
- Paschen, S.; Budnyk, S.; Köhler, U.; Prots, Y.; Hiebl, K.; Steglich, F.; Grin, Y. New type-I clathrates with ordered Eu distribution. Phys. B Condens. Matter 2006, 383, 89–92. [Google Scholar] [CrossRef]
- Slack, G.A. New Materials and Performance Limits fro Thermoelectric Cooling. In CRC Handbook of Thermoelectrics; Rowe, D.M., Ed.; CRC Press: Boca Raton, FL, USA, 1995; pp. 407–440. ISBN 9780849301469. [Google Scholar]
- Nolas, G.S. The Physics and Chemistry of Inorganic Clathrates, 1st ed.; Nolas, G.S., Ed.; Springer: Dordrecht, The Netherlands, 2014; ISBN 978-94-017-9127-4. [Google Scholar]
- Owens-Baird, B.; Wang, J.; Grass Wang, S.; Chen, Y.-S.; Lee, S.; Donadio, D.; Kovnir, K. III–V Clathrate Semiconductors with Outstanding Hole Mobility: Cs8In27Sb19 and A8Ga27Sb19 (A = Cs, Rb). J. Am. Chem. Soc. 2020, 142, 2031–2041. [Google Scholar] [CrossRef]
- Guloy, A.M.; Ramlau, R.; Tang, Z.; Schnelle, W.; Baitinger, M.; Grin, Y. A guest-free germanium clathrate. Nature 2006, 443, 320–323. [Google Scholar] [CrossRef]
- Beekman, M.; Nolas, G.S. Inorganic clathrate-II materials of Group 14: Synthetic routes and physical properties. J. Mater. Chem. 2008, 18, 842–851. [Google Scholar] [CrossRef]
- Karttunen, A.J.; Fässler, T.F.; Linnolahti, M.; Pakkanen, T.A. Structural Principles of Semiconducting Group 14 Clathrate Frameworks. Inorg. Chem. 2011, 50, 1733–1742. [Google Scholar] [CrossRef]
- Schäfer, M.; Bobev, S. New Type-I and Type-II Clathrates in the Systems Cs–Na–Ga–Si, Rb–Na–Ga–Si, and Rb–Na–Zn–Si. Inorganics 2014, 2, 79–95. [Google Scholar] [CrossRef]
- Schäfer, M.C.; Bobev, S. Cu and Zn Substituted Silicon Clathrates with the Cubic Type-II Structure: Synthesis and Characterization of Cs8Na16Cu3.8Si132.2 and Cs8Na16Zn6.9Si129.1. Z. Anorg. Allg. Chem. 2017, 643, 1874–1880. [Google Scholar] [CrossRef]
- Schäfer, M.; Bobev, S. Synthesis and Structural Characterization of the New Clathrates K8Cd4Ge42, Rb8Cd4Ge42, and Cs8Cd4Ge42. Materials 2016, 9, 236. [Google Scholar] [CrossRef]
- Baranets, S.; Schäfer, M.; Bobev, S. Synthesis and structural characterization of the type-I clathrates K8AlxSn46−x and Rb8AlxSn46−x (x = 6.4–9.7). Acta Crystallogr. Sec. C Struct. Chem. 2019, 75, 1535–1540. [Google Scholar] [CrossRef]
- Schäfer, M.C.; Bobev, S. Copper and Zinc Substitutions in Clathrates of Tin: Synthesis, Structural Characterization, and Physical Properties of A8Cu2.67Sn43.33 and A8Zn4Sn42 (A = K, Rb, Cs) with the Type-I Structure. Chem. Mater. 2013, 25, 3737–3744. [Google Scholar] [CrossRef]
- Schäfer, H.; Eisenmann, B.; Müller, W. Zintl Phases: Transitions between Metallic and Ionic Bonding. Angew. Chemie Int. Ed. Engl. 1973, 12, 694–712. [Google Scholar] [CrossRef]
- Xu, J.; Wu, J.; Heguri, S.; Tanabe, Y.; Liu, G.Q.; Jiang, J.; Jiang, H.; Tanigaki, K. Single Crystal Structure Study of Type I Clathrate K8Zn4Sn42 and K8In8Sn38. J. Electron. Mater. 2017, 46, 2765–2769. [Google Scholar] [CrossRef]
- Wilkinson, A.P.; Lind, C.; Young, R.A.; Shastri, S.D.; Lee, P.L.; Nolas, G.S. Preparation, Transport Properties, and Structure Analysis by Resonant X-ray Scattering of the Type I Clathrate Cs8Cd4Sn42. Chem. Mater. 2002, 14, 1300–1305. [Google Scholar] [CrossRef]
- Baran, V.; Fischer, A.; Scherer, W.; Fässler, T.F. Synthesis of Large Single Crystals and Thermoelectrical Properties of the Type-I Clathrate K8Zn4Sn42. Z. Anorg. Allg. Chem. 2013, 639, 2125–2128. [Google Scholar] [CrossRef]
- Nolas, G.S.; Cohn, J.L.; Dyck, J.S.; Uher, C.; Yang, J. Transport properties of polycrystalline type-I Sn clathrates. Phys. Rev. B Condens. Matter Mater. Phys. 2002, 65, 1652011–1652016. [Google Scholar] [CrossRef]
- Schäfer, M.C.; Bobev, S. K and Ba distribution in the structures of the clathrate compounds KxBa16−x(Ga,Sn)136 (x = 0.8, 4.4, and 12.9) and KxBa8−x(Ga,Sn)46 (x = 0.3). Acta Crystallogr. Sec. C Cryst. Struct. Commun. 2013, 69, 319–323. [Google Scholar] [CrossRef] [PubMed]
- Stefanoski, S.; Dong, Y.; Nolas, G.S. Structural characterization and low-temperature physical properties of p-type single-crystal K8Ga8.5Sn37.5 grown by self-flux method. J. Solid State Chem. 2013, 204, 166–169. [Google Scholar] [CrossRef]
- Westerhaus, W.; Shuster, H.-U. Preparation and Crystal Structure of Ternary Phases with a Modified K8Ge46 Clathrat Structure. Z. Naturforsch. B 1977, 32, 1365–1367. [Google Scholar] [CrossRef]
- Tanaka, T.; Onimaru, T.; Suekuni, K.; Mano, S.; Fukuoka, H.; Yamanaka, S.; Takabatake, T. Interplay between thermoelectric and structural properties of type-I clathrate K8Ga8Sn38 single crystals. Phys. Rev. B Condens. Matter Mater. Phys. 2010, 81, 165110. [Google Scholar] [CrossRef]
- Kröner, R.; Peters, K.; Schnering, H.G.; von Nesper, R. Crystal structure of the clathrates K8Al8Ge38 and K8Al8Sn38. Z. Krist. New Cryst. Struct. 1998, 213, 675–676. [Google Scholar] [CrossRef]
- Kröner, R.; Peters, K.; Schnering, H.G.; von Nesper, R. Crystal structure of the clathrates Rb8Al8Ge38 and Rb8Al8Sn38. Z. Krist. New Cryst. Struct. 1998, 213, 669–670. [Google Scholar] [CrossRef]
- Von Schnering, H.G.; Kröner, R.; Menke, H.; Peters, K.; Nesper, R. Crystal structure of the clathrates Rb8Ga8Sn38, Rb8Ga8Ge38 and Rb8Ga8Si38. Z. Krist. New Cryst. Struct. 1998, 213, 677–678. [Google Scholar] [CrossRef]
- Kröner, R.; Peters, K.; von Schnering, H.G.; Nesper, R. Crystal structure of the clathrates Cs8Ga8Ge38 and Cs8Ga8Sn38. Z. Krist. New Cryst. Struct. 1998, 213, 671–672. [Google Scholar]
- Schäfer, M.C.; Bobev, S. On the possibility for Rb- and Eu-cation ordering in type-I clathrates: Synthesis and homogeneity range of the novel compounds Rb8−xEux(In,Ge)46 (0.6 ≤ x ≤ 1.8). Acta Crystallogr. Sec. C Cryst. Struct. Commun. 2013, 69, 1457–1461. [Google Scholar] [CrossRef]
- Kishimoto, K.; Sasaki, Y.; Koyanagi, T.; Ohoyama, K.; Akai, K. Crystal structure and thermoelectric properties of KxBa8−xZnyGe46−y clathrates. J. Appl. Phys. 2012, 111, 093716. [Google Scholar] [CrossRef]
- Von Schnering, H.G.; Kröner, R.; Baitinger, M.; Peters, K.; Nesper, R.; Grin, Y. Crystal structure of the defect clathrate Cs8Sn44□2. Zeitschrift fur Krist. New Cryst. Struct. 2000, 215, 205–206. [Google Scholar] [CrossRef]
- Kaltzoglou, A.; Fässler, T.; Christensen, M.; Johnsen, S.; Iversen, B.; Presniakov, I.; Sobolev, A.; Shevelkov, A. Effects of the order-disorder phase transition on the physical properties of A8Sn44□2 (A = Rb, Cs). J. Mater. Chem. 2008, 18, 5630–5637. [Google Scholar] [CrossRef]
- SAINT; Bruker AXS Inc.: Madison, WI, USA, 2014.
- SADABS; Bruker AXS Inc.: Madison, WI, USA, 2014.
- Sheldrick, G.M. SHELXT—Integrated space-group and crystal-structure determination. Acta Crystallogr. Sec. A Found. Adv. 2015, 71, 3–8. [Google Scholar] [CrossRef]
- Sheldrick, G.M. Crystal structure refinement with SHELXL. Acta Crystallogr. Sec. C Struct. Chem. 2015, 71, 3–8. [Google Scholar] [CrossRef] [PubMed]
- Gelato, L.M.; Parthé, E. STRUCTURE TIDY—A computer program to standardize crystal structure data. J. Appl. Crystallogr. 1987, 20, 139–143. [Google Scholar] [CrossRef]
- Baranets, S.; He, H.; Bobev, S. Niobium-bearing arsenides and germanides from elemental mixtures not involving niobium: A new twist to an old problem in solid-state synthesis. Acta Crystallogr. Sec. C Struct. Chem. 2018, 74, 623–627. [Google Scholar] [CrossRef] [PubMed]
- Baran, V.; Senyshyn, A.; Karttunen, A.J.; Fischer, A.; Scherer, W.; Raudaschl-Sieber, G.; Fässler, T.F. A Combined Metal-Halide/Metal Flux Synthetic Route towards Type-I Clathrates: Crystal Structures and Thermoelectric Properties of A8Al8Si38 (A = K, Rb, and Cs). Chem. Eur. J. 2014, 20, 15077–15088. [Google Scholar] [CrossRef] [PubMed]
- Schäfer, M.C.; Bobev, S. Tin Clathrates with the Type II Structure. J. Am. Chem. Soc. 2013, 135, 1696–1699. [Google Scholar] [CrossRef]
- Zhao, J.-T.; Corbett, J.D. Zintl Phases in Alkali-Metal-Tin Systems: K8Sn25 with Condensed Pentagonal Dodecahedra of Tin. Two A8Sn44 Phases with a Defect Clathrate Structure. Inorg. Chem. 1994, 33, 5721–5726. [Google Scholar] [CrossRef]
- Kaltzoglou, A.; Fässler, T.F.; Gold, C.; Scheidt, E.W.; Scherer, W.; Kume, T.; Shimizu, H. Investigation of substitution effects and the phase transition in type-I clathrates RbxCs8−xSn44□2 (1.3 ≤ x ≤ 2.1) using single-crystal X-ray diffraction, Raman spectroscopy, heat capacity and electrical resistivity. J. Solid State Chem. 2009, 182, 2924–2929. [Google Scholar] [CrossRef]
- Baran, V.; Fässler, T.F. Si-based Clathrates with Partial Substitution by Zn and Ga: K8Zn3.5Si42.5, Rb7.9Zn3.6Si42.4, and Cs8−xGa8−ySi38+y. Z. Anorg. Allg. Chem. 2015, 641, 1435–1443. [Google Scholar] [CrossRef]
- Nolas, G.S.; Chakoumakos, B.C.; Mahieu, B.; Long, G.J.; Weakley, T.J.R. Structural Characterization and Thermal Conductivity of Type-I Tin Clathrates. Chem. Mater. 2000, 12, 1947–1953. [Google Scholar] [CrossRef]
- Dubois, F.; Fässler, T.F. Ordering of Vacancies in Type-I Tin Clathrate: Superstructure of Rb8Sn44□2. J. Am. Chem. Soc. 2005, 127, 3264–3265. [Google Scholar] [CrossRef] [PubMed]
- Kaltzoglou, A.; Hoffmann, S.D.; Fässler, T.F. Order-Disorder Phase Transition in Type-I Clathrate Cs8Sn44□2. Eur. J. Inorg. Chem. 2007, 2007, 4162–4167. [Google Scholar] [CrossRef]
- Kirsanova, M.A.; Shevelkov, A.V. Clathrates and semiclathrates of Type-I: Crystal structure and superstructures. Z. Krist. 2013, 228, 215–227. [Google Scholar] [CrossRef]

| Compound | Rb8Ga7.1(1)Sn38.9 2 | Cs8Ga7.5(1)Sn38.5 |
|---|---|---|
| fw/g mol−1 | 5797.23 | 6156.24 |
| a/Å | 11.9691(3) | 12.023(2) |
| V/Å3 | 1714.69(13) | 1737.8(9) |
| ρcalc./g cm−3 | 5.613 | 5.882 |
| μ/cm−1 | 222.39 | 205.19 |
| R1 (I > 2σ(I)) 1 | 0.0151 | 0.0168 |
| wR2 (I > 2σ(I)) 1 | 0.0328 | 0.0334 |
| R1 (all data) 1 | 0.0183 | 0.0209 |
| wR2 (all data) 1 | 0.0334 | 0.341 |
| Δρmax&min/e−Å−3 | 0.50 & −0.59 | 0.56 & −0.56 |
| Atom | Site | x | y | z | Ueq(Å2) 1 |
|---|---|---|---|---|---|
| Rb1 | 6d | ¼ | ½ | 0 | 0.041(1) |
| Rb2 | 2a | 0 | 0 | 0 | 0.017(1) |
| Sn1/Ga1 2 | 24k | 0 | 0.3119(1) | 0.1179(1) | 0.014(1) |
| Sn2/Ga2 2 | 16i | 0.18337(2) | x | x | 0.014(1) |
| Sn3/Ga3 2 | 6c | ¼ | 0 | ½ | 0.016(1) |
| Cs1 | 6d | ¼ | ½ | 0 | 0.028(1) |
| Cs2 | 2a | 0 | 0 | 0 | 0.013(1) |
| Sn1 3 | 24k | 0 | 0.3114(1) | 0.1179(1) | 0.015(1) |
| Ga1 3 | 24k | 0 | 0.3493(12) | 0.1345(8) | 0.015(1) |
| Sn2/Ga2 4 | 16i | 0.18340(2) | x | x | 0.014(1) |
| Sn3/Ga3 4 | 6c | ¼ | 0 | ½ | 0.014(1) |
| Rb8Ga7.1(1)Sn38.9 | d/Å | Cs8Ga7.5(1)Sn38.5 | d/Å |
|---|---|---|---|
| M1–M1 | 2.8235(7) | Sn1/Ga1–Sn1 | 2.8342(19)/3.069(11) |
| M1–M2 (×2) | 2.7925(2) | Sn1/Ga1–M2 (×2) | 2.8015(8)/3.031(9) |
| M1–M3 | 2.7504(3) | Sn1/Ga1–M3 | 2.7688(15)/2.282(15) |
| M2–M1 (×3) | 2.7925(2) | M2–Sn1/Ga1 (×3) | 2.8015(8)/3.031(9) |
| M2–M2 | 2.7626(8) | M2–M2 | 2.7736(10) |
| M3–M1 (×4) | 2.7504(3) | M3–Sn1/Ga1 (×4) | 2.7688(15)/2.282(15) |
| Rb1–M1 (×8) | 4.0016(2) | Cs1–Sn1/Ga1 (×8) | 4.0234(9)/3.864(6) |
| Rb2–M1 (×12) | 3.9916(3) | Cs2–Sn1 (×12) | 4.0027(16) |
| Rb2–M2 (×8) | 3.8014(4) | Cs2–M2 (×8) | 3.8192(8) |
| Formula | a (Å) | Sn1A/Sn1B (24k) | Sn3 (6c) |
|---|---|---|---|
| Rb8Sn43.3(1) | 12.0338(4) | 0.770(3)/0.230 | 0.550(3) |
| Rb8Sn44.0(1) | 12.0493(4) | 0.681(2)/0.319 | 0.663(4) |
| Cs8Sn44.0(1) | 12.0898(18) | 0.680(4)/0.320 | 0.663(5) |
| Cs8Sn44.5(1) | 12.1357(10) | 0.831(11)/0.169 | 0.743(7) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baranets, S.A.; Childs, A.B.; He, H.; Bobev, S. Complex Disorder in Type-I Clathrates: Synthesis and Structural Characterization of A8GaxSn46−x (A = Rb, Cs; 6.9 < x < 7.5). Crystals 2020, 10, 298. https://doi.org/10.3390/cryst10040298
Baranets SA, Childs AB, He H, Bobev S. Complex Disorder in Type-I Clathrates: Synthesis and Structural Characterization of A8GaxSn46−x (A = Rb, Cs; 6.9 < x < 7.5). Crystals. 2020; 10(4):298. https://doi.org/10.3390/cryst10040298
Chicago/Turabian StyleBaranets, Sviatoslav A., Amanda B. Childs, Hua He, and Svilen Bobev. 2020. "Complex Disorder in Type-I Clathrates: Synthesis and Structural Characterization of A8GaxSn46−x (A = Rb, Cs; 6.9 < x < 7.5)" Crystals 10, no. 4: 298. https://doi.org/10.3390/cryst10040298
APA StyleBaranets, S. A., Childs, A. B., He, H., & Bobev, S. (2020). Complex Disorder in Type-I Clathrates: Synthesis and Structural Characterization of A8GaxSn46−x (A = Rb, Cs; 6.9 < x < 7.5). Crystals, 10(4), 298. https://doi.org/10.3390/cryst10040298






