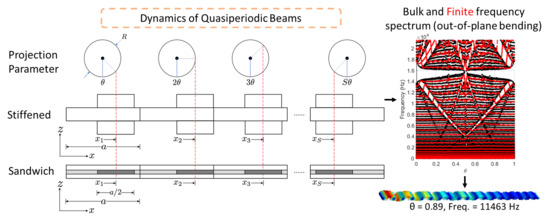
Dynamics of Quasiperiodic Beams
Abstract
1. Introduction
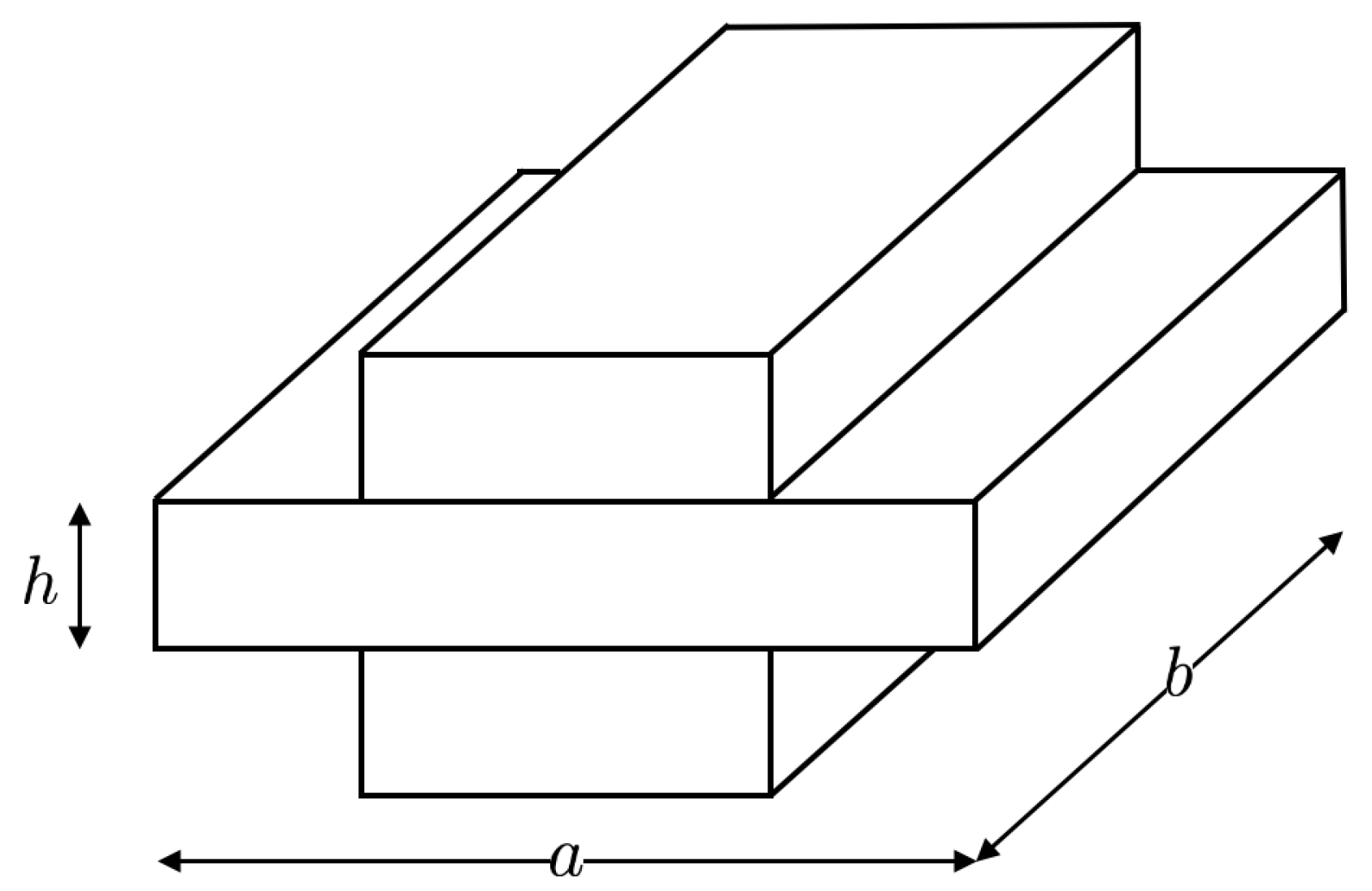
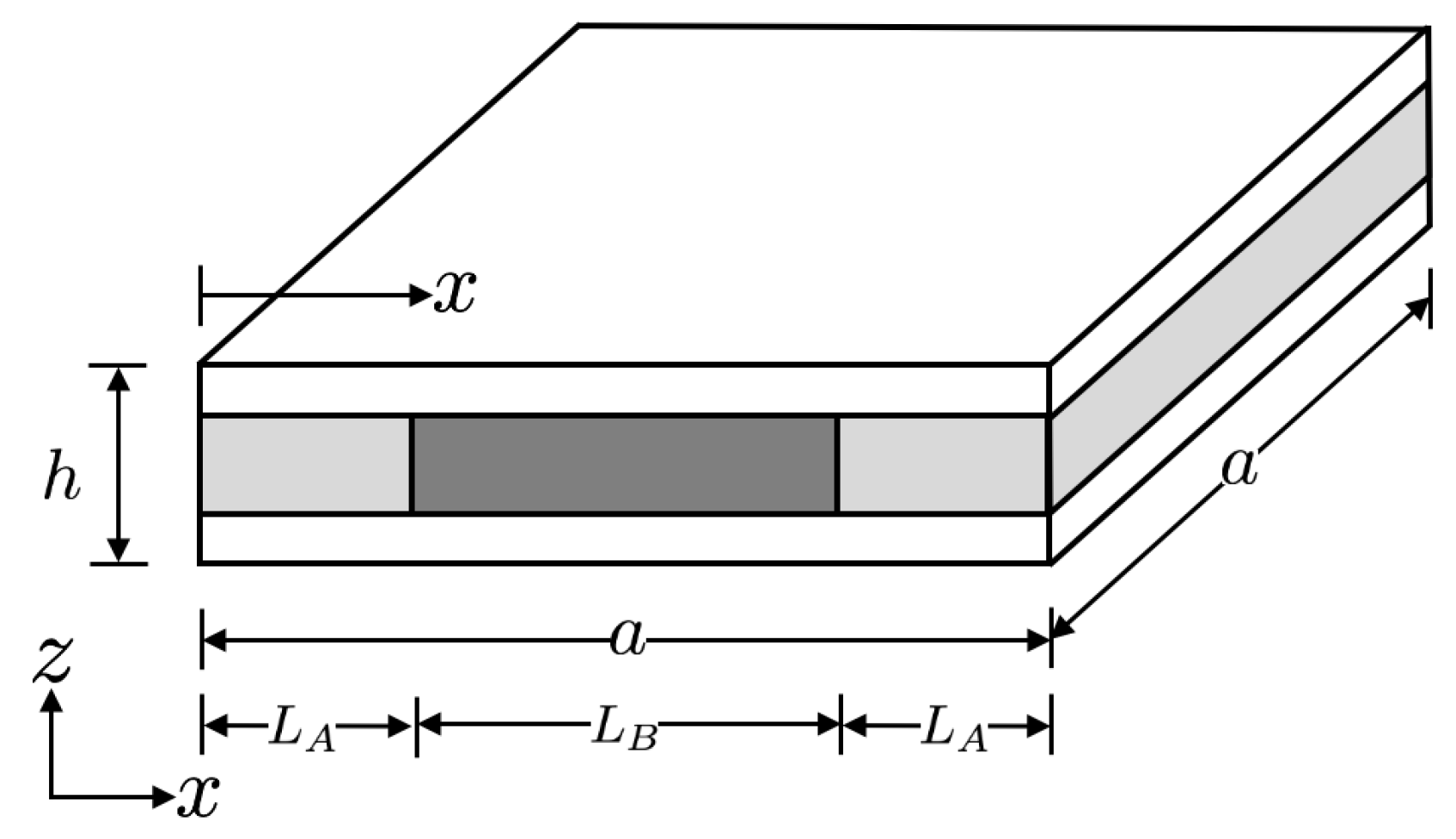
2. Continuous Beam with Stiffeners
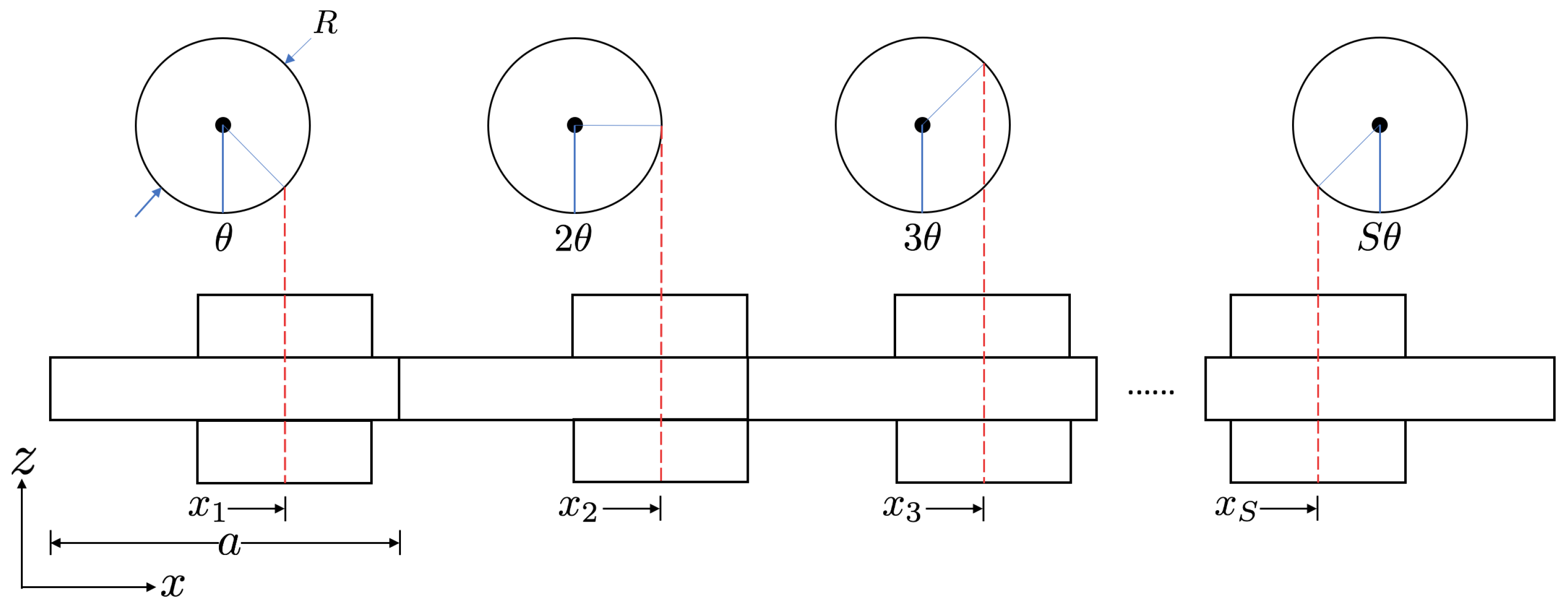
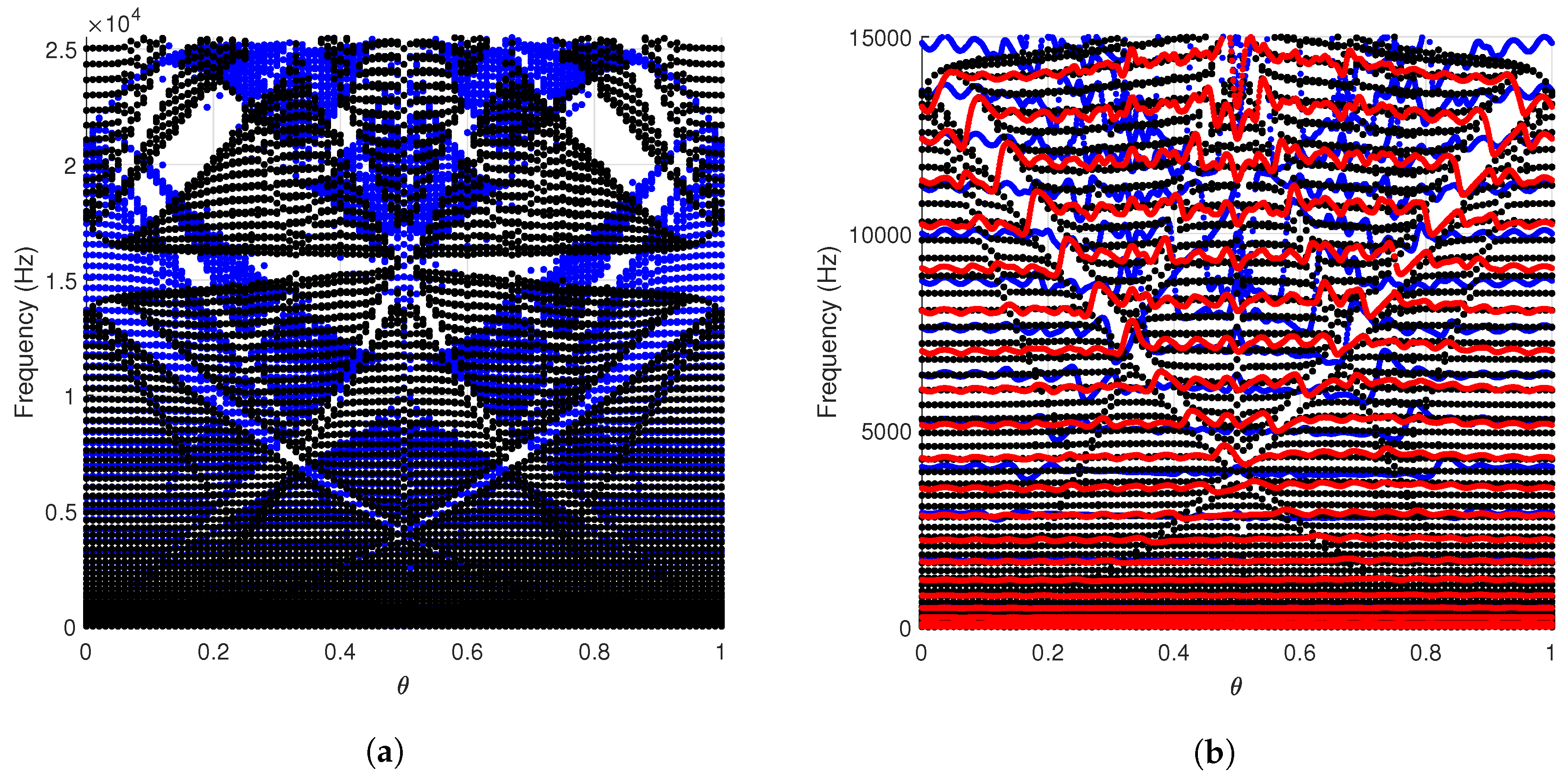
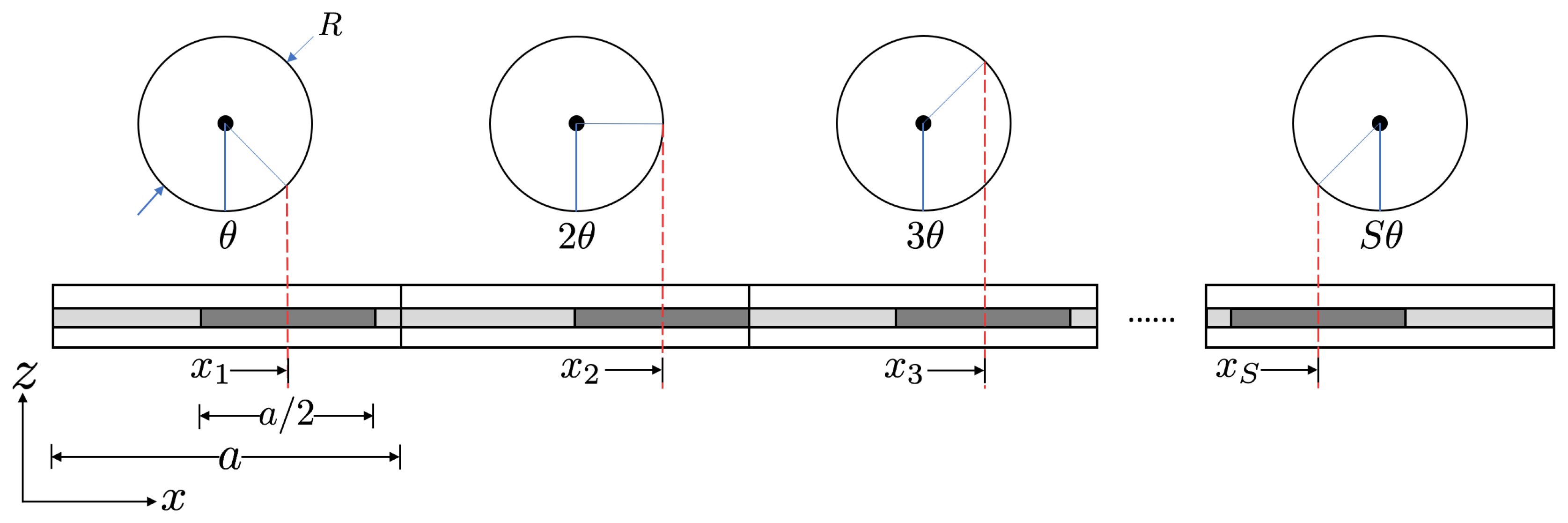
2.1. The Periodic Case: Unit Cell Analysis
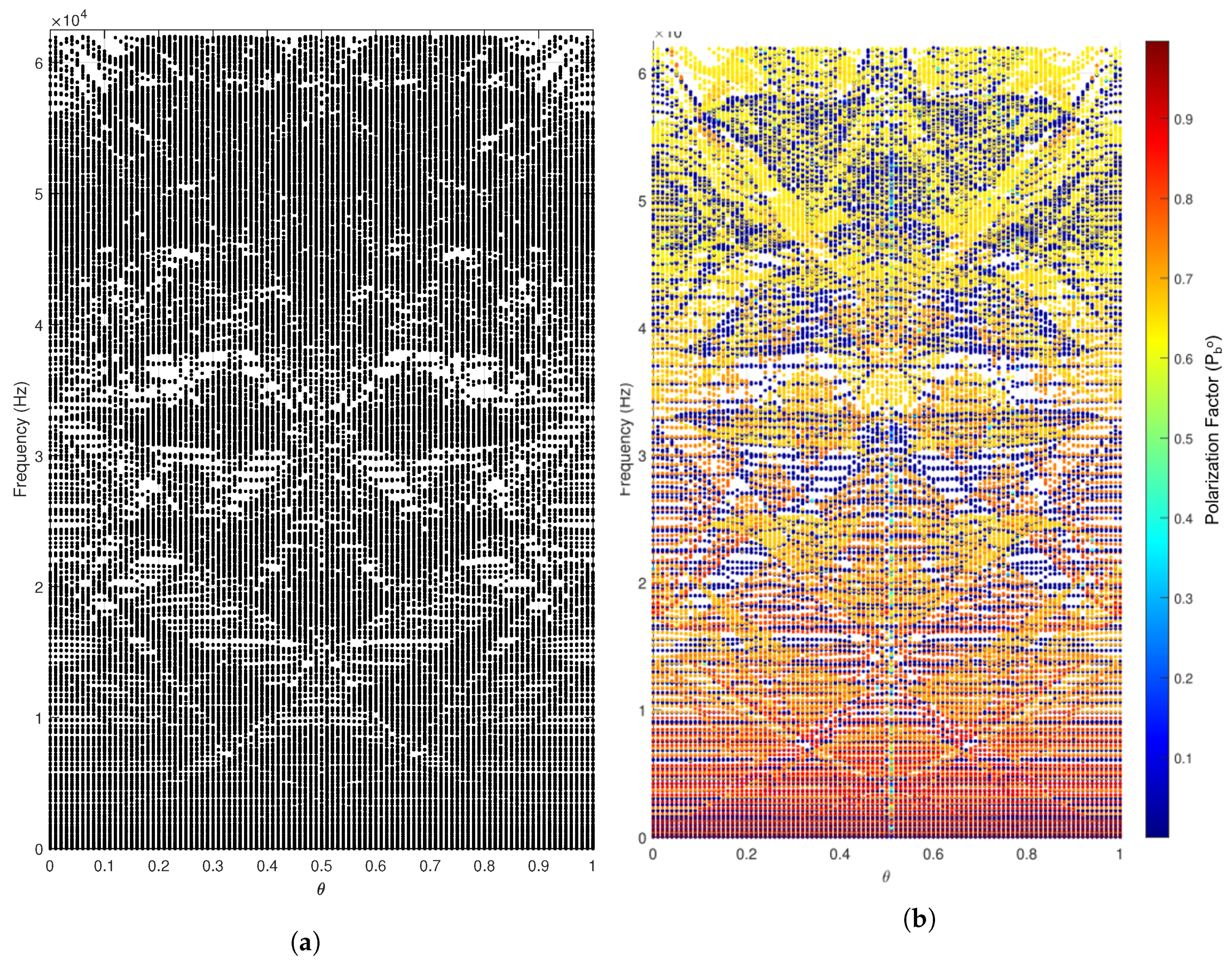
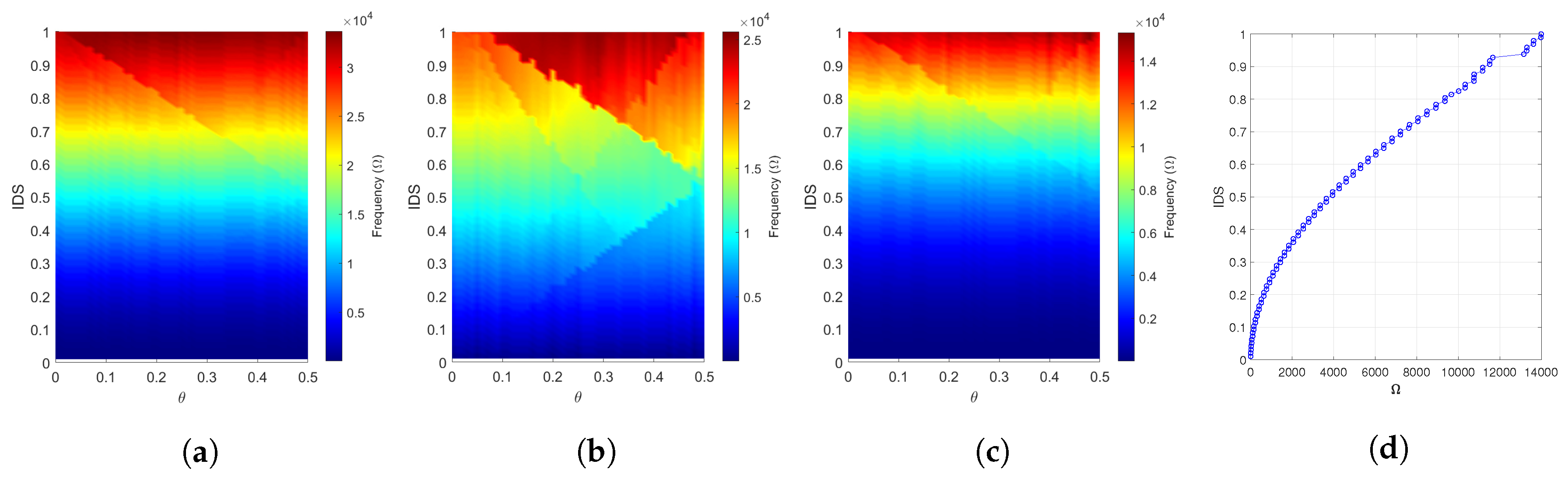
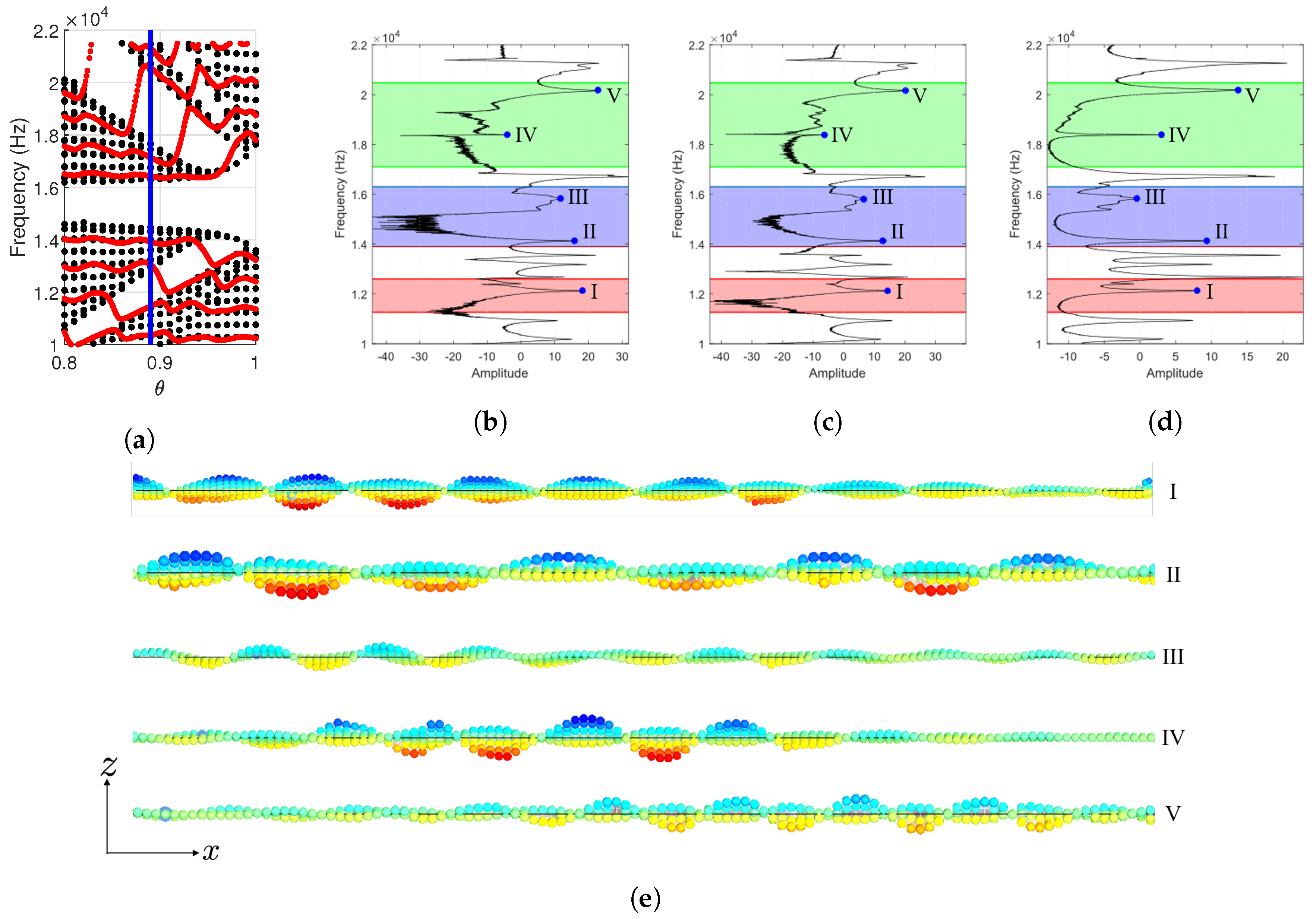
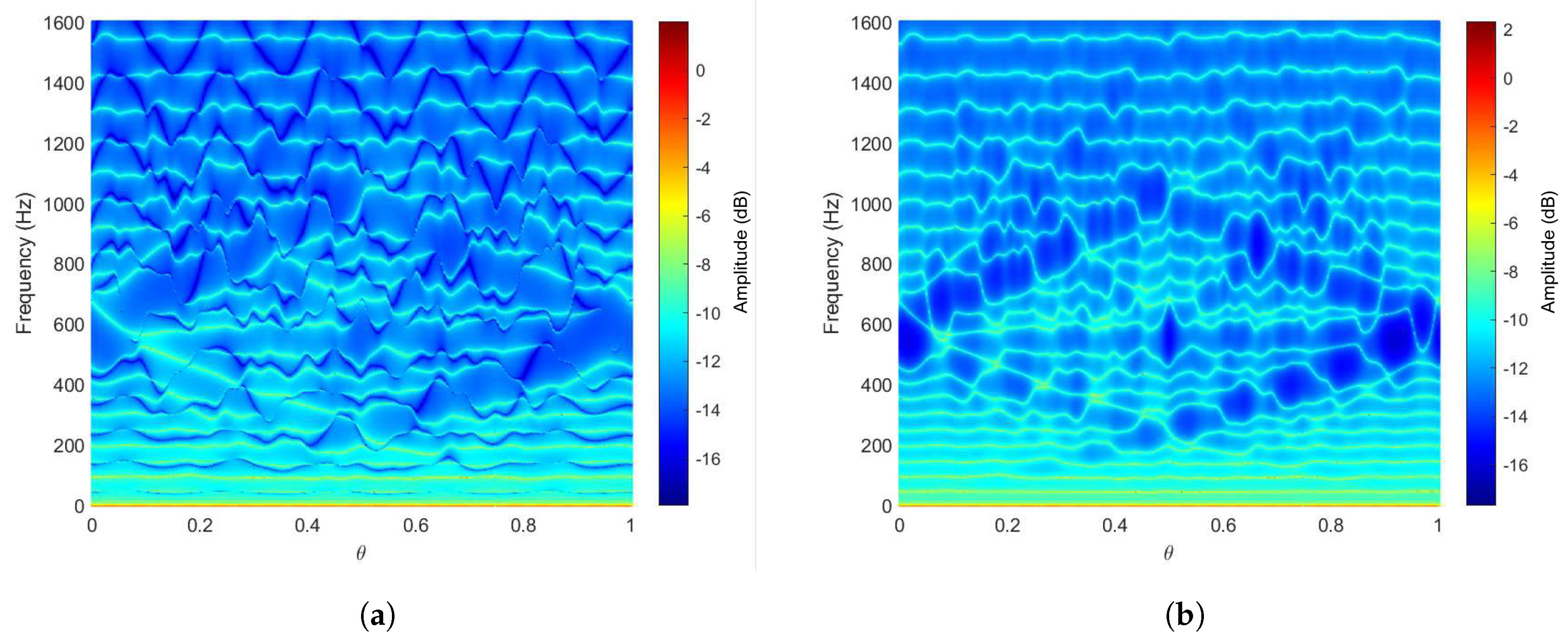
2.2. Spectral Properties—Bulk and Finite Domains
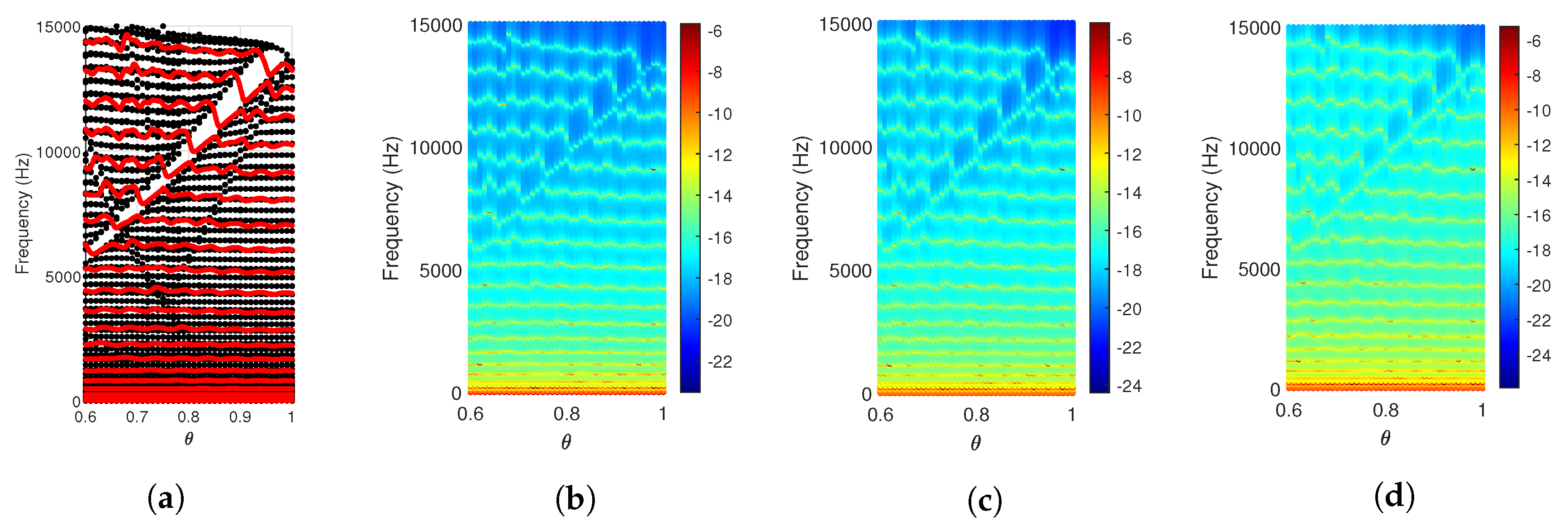
2.3. Experimental Results on a Finite Beam
3. Sandwich Quasiperiodic Beams
3.1. Dynamics of Sandwich Beams
3.1.1. Geometric and Material Properties
3.1.2. Harmonic Response
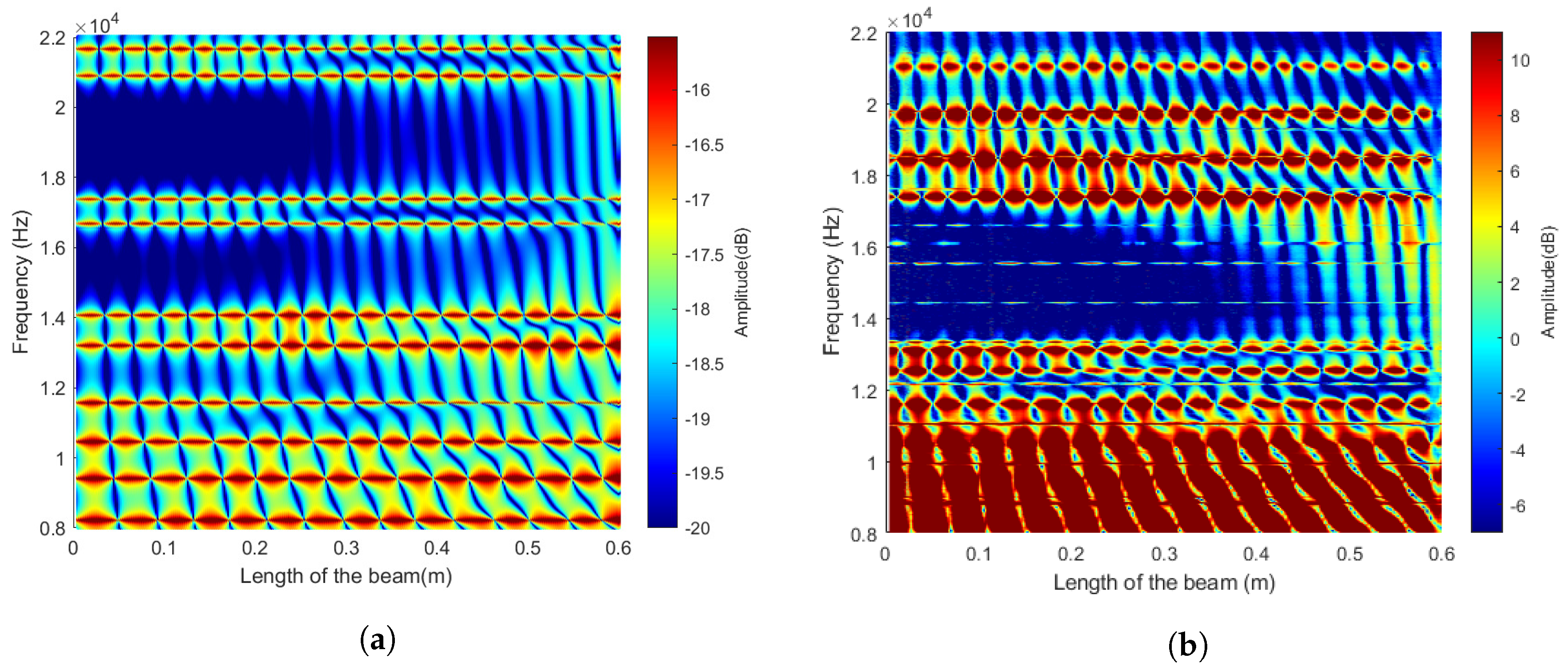
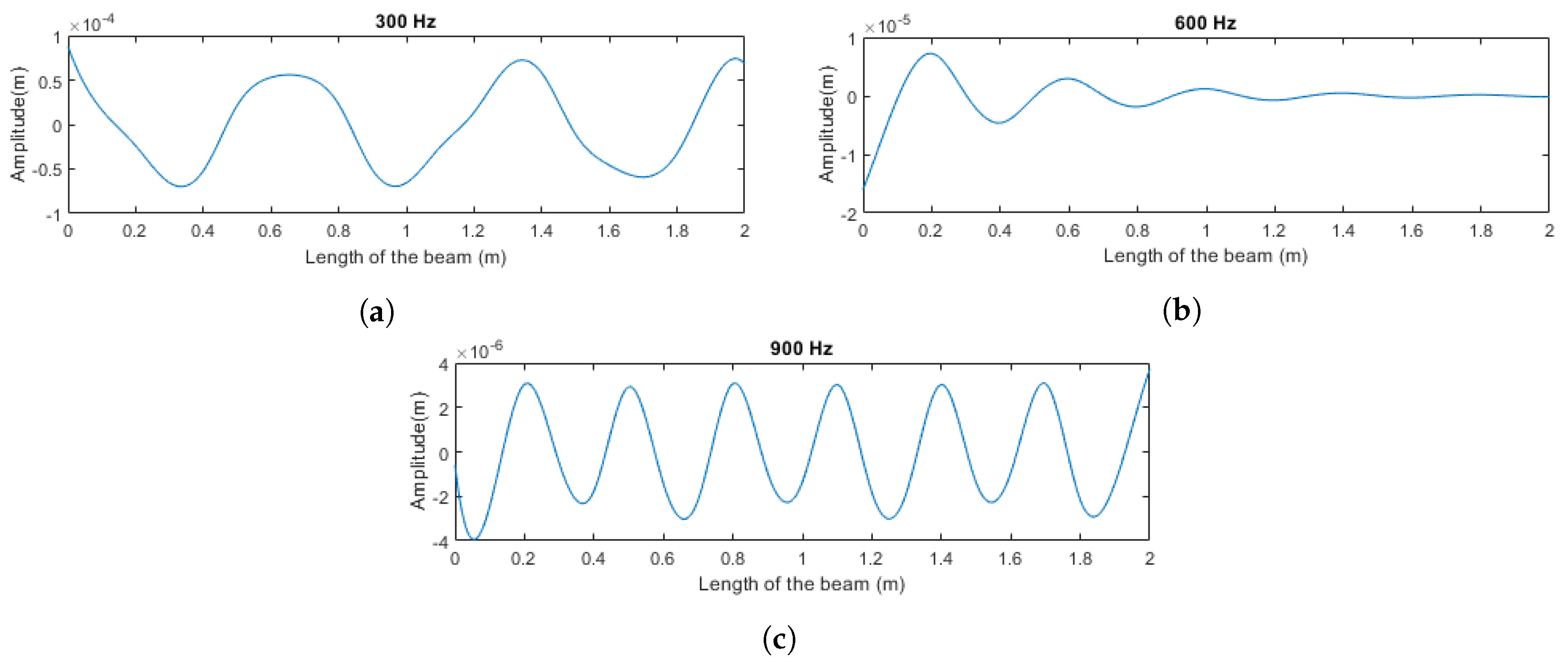
3.2. Numerical Results: Frequency Response Function
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| 3-D | Three-dimensional |
| FEM | Finite Element Method |
| FRF | Frequency Response Function |
| IDS | Integrated Density of States |
| QP | Quasi-Periodic |
References
- Süsstrunk, R.; Huber, S.D. Observation of phononic helical edge states in a mechanical topological insulator. Science 2015, 349, 47–50. [Google Scholar] [CrossRef] [PubMed]
- Mousavi, S.H.; Khanikaev, A.B.; Wang, Z. Topologically protected elastic waves in phononic metamaterials. Nat. Commun. 2015, 6, 8682. [Google Scholar] [CrossRef] [PubMed]
- Anderson, P.W. Absence of diffusion in certain random lattices. Phys. Rev. 1958, 109, 1492. [Google Scholar] [CrossRef]
- Pal, R.K.; Rosa, M.I.N.; Ruzzene, M. Topological bands and localized vibration modes in quasiperiodic beams. New J. Phys. 2019, 21, 093017. [Google Scholar] [CrossRef]
- Xia, Y.; Erturk, A.; Ruzzene, M. Topological edge states in quasiperiodic locally resonant metastructures. Phys. Rev. Appl. 2020, 13, 014023. [Google Scholar] [CrossRef]
- Chaplain, G.; Makwana, M.; Craster, R. Rayleigh–Bloch, topological edge and interface waves for structured elastic plates. Wave Motion 2019, 86, 162–174. [Google Scholar] [CrossRef]
- Hodges, C.; Woodhouse, J. Confinement of vibration by structural irregularity. J. Acoust. Soc. Am. 1981, 69, S109. [Google Scholar] [CrossRef]
- Hodges, C. Confinement of vibration by structural irregularity. J. Sound Vib. 1982, 82, 411–424. [Google Scholar] [CrossRef]
- Sievers, A.; Takeno, S. Intrinsic localized modes in anharmonic crystals. Phys. Rev. Lett. 1988, 61, 970. [Google Scholar] [CrossRef]
- Page, J. Asymptotic solutions for localized vibrational modes in strongly anharmonic periodic systems. Phys. Rev. B 1990, 41, 7835. [Google Scholar] [CrossRef]
- Sheng, P. Scattering and Localization of Classical Waves in Random Media; World Scientific: Singapore, 1990; Volume 8. [Google Scholar]
- Photiadis, D.M.; Houston, B.H. Anderson localization of vibration on a framed cylindrical shell. J. Acoust. Soc. Am. 1999, 106, 1377–1391. [Google Scholar] [CrossRef]
- Campbell, D.K.; Flach, S.; Kivshar, Y.S. Localizing energy through nonlinearity and discreteness. Phys. Today 2004, 57, 43–49. [Google Scholar] [CrossRef]
- Hu, H.; Strybulevych, A.; Page, J.; Skipetrov, S.E.; van Tiggelen, B.A. Localization of ultrasound in a three-dimensional elastic network. Nat. Phys. 2008, 4, 945–948. [Google Scholar] [CrossRef]
- Han, P.; Chan, C.; Zhang, Z. Wave localization in one-dimensional random structures composed of single-negative metamaterials. Phys. Rev. B 2008, 77, 115332. [Google Scholar] [CrossRef]
- Prodan, E.; Prodan, C. Topological phonon modes and their role in dynamic instability of microtubules. Phys. Rev. Lett. 2009, 103, 248101. [Google Scholar] [CrossRef]
- Nash, L.M.; Kleckner, D.; Read, A.; Vitelli, V.; Turner, A.M.; Irvine, W.T. Topological mechanics of gyroscopic metamaterials. Proc. Natl. Acad. Sci. USA 2015, 112, 14495–14500. [Google Scholar] [CrossRef]
- Chen, H.; Yao, L.; Nassar, H.; Huang, G. Mechanical quantum hall effect in time-modulated elastic materials. Phys. Rev. Appl. 2019, 11, 044029. [Google Scholar] [CrossRef]
- Pal, R.K.; Schaeffer, M.; Ruzzene, M. Helical edge states and topological phase transitions in phononic systems using bi-layered lattices. J. Appl. Phys. 2016, 119, 084305. [Google Scholar] [CrossRef]
- Chen, H.; Nassar, H.; Norris, A.N.; Hu, G.; Huang, G. Elastic quantum spin Hall effect in kagome lattices. Phys. Rev. B 2018, 98, 094302. [Google Scholar] [CrossRef]
- Vila, J.; Pal, R.K.; Ruzzene, M. Observation of topological valley modes in an elastic hexagonal lattice. Phys. Rev. B 2017, 96, 134307. [Google Scholar] [CrossRef]
- Liu, T.W.; Semperlotti, F. Tunable acoustic valley–hall edge states in reconfigurable phononic elastic waveguides. Phys. Rev. Appl. 2018, 9, 014001. [Google Scholar] [CrossRef]
- Ozawa, T.; Price, H.M.; Goldman, N.; Zilberberg, O.; Carusotto, I. Synthetic dimensions in integrated photonics: From optical isolation to four-dimensional quantum Hall physics. Phys. Rev. A 2016, 93, 043827. [Google Scholar] [CrossRef]
- Kraus, Y.E.; Zilberberg, O. Quasiperiodicity and topology transcend dimensions. Nat. Phys. 2016, 12, 624. [Google Scholar] [CrossRef]
- Lahini, Y.; Pugatch, R.; Pozzi, F.; Sorel, M.; Morandotti, R.; Davidson, N.; Silberberg, Y. Observation of a localization transition in quasiperiodic photonic lattices. Phys. Rev. Lett. 2009, 103, 013901. [Google Scholar] [CrossRef]
- Vyunishev, A.M.; Pankin, P.S.; Svyakhovskiy, S.E.; Timofeev, I.V.; Vetrov, S.Y. Quasiperiodic one-dimensional photonic crystals with adjustable multiple photonic bandgaps. Opt. Lett. 2017, 42, 3602–3605. [Google Scholar] [CrossRef]
- Araújo, C.; Vasconcelos, M.S.; Mauriz, P.W.; Albuquerque, E.L. Omnidirectional band gaps in quasiperiodic photonic crystals in the THz region. Opt. Mater. 2012, 35, 18–24. [Google Scholar] [CrossRef]
- Bellingeri, M.; Chiasera, A.; Kriegel, I.; Scotognella, F. Optical properties of periodic, quasi-periodic, and disordered one-dimensional photonic structures. Opt. Mater. 2017, 72, 403–421. [Google Scholar] [CrossRef]
- Jin, C.; Cheng, B.; Man, B.; Li, Z.; Zhang, D.; Ban, S.; Sun, B. Band gap and wave guiding effect in a quasiperiodic photonic crystal. Appl. Phys. Lett. 1999, 75, 1848–1850. [Google Scholar] [CrossRef]
- Biancalana, F. All-optical diode action with quasiperiodic photonic crystals. J. Appl. Phys. 2008, 104, 093113. [Google Scholar] [CrossRef]
- Kraus, Y.E.; Lahini, Y.; Ringel, Z.; Verbin, M.; Zilberberg, O. Topological states and adiabatic pumping in quasicrystals. Phys. Rev. Lett. 2012, 109, 106402. [Google Scholar] [CrossRef]
- Apigo, D.J.; Qian, K.; Prodan, C.; Prodan, E. Topological edge modes by smart patterning. Phys. Rev. Mater. 2018, 2, 124203. [Google Scholar] [CrossRef]
- Dean, C.R.; Wang, L.; Maher, P.; Forsythe, C.; Ghahari, F.; Gao, Y.; Katoch, J.; Ishigami, M.; Moon, P.; Koshino, M.; et al. Hofstadter’s butterfly and the fractal quantum Hall effect in moiré superlattices. Nature 2013, 497, 598–602. [Google Scholar] [CrossRef]
- Yu, D.; Liu, Y.; Zhao, H.; Wang, G.; Qiu, J. Flexural vibration band gaps in Euler-Bernoulli beams with locally resonant structures with two degrees of freedom. Phys. Rev. B 2006, 73, 064301. [Google Scholar] [CrossRef]
- Süsstrunk, R.; Huber, S.D. Classification of topological phonons in linear mechanical metamaterials. Proc. Natl. Acad. Sci. USA 2016, 113, E4767–E4775. [Google Scholar] [CrossRef]
- Hofstadter, D.R. Energy levels and wave functions of Bloch electrons in rational and irrational magnetic fields. Phys. Rev. B 1976, 14, 2239. [Google Scholar] [CrossRef]
- Apigo, D.J.; Cheng, W.; Dobiszewski, K.F.; Prodan, E.; Prodan, C. Observation of topological edge modes in a quasiperiodic acoustic waveguide. Phys. Rev. Lett. 2019, 122, 095501. [Google Scholar] [CrossRef]
- COMSOL Multiphysics®. Introduction to COMSOL Multiphysics®; COMSOL AB: Stockholm, Sweden, 2020; Volume 11. [Google Scholar]
- Pryor, R.W. Multiphysics Modeling Using COMSOL®: A First Principles Approach; Jones & Bartlett Learning: Burlington, MA, USA, 2009. [Google Scholar]
- Olsson III, R.H.; El-Kady, I. Microfabricated phononic crystal devices and applications. Meas. Sci. Technol. 2008, 20, 012002. [Google Scholar] [CrossRef]
- Prodan, E.; Shmalo, Y. The K-theoretic bulk-boundary principle for dynamically patterned resonators. J. Geom. Phys. 2019, 135, 135–171. [Google Scholar] [CrossRef]
- Yin, J.; Ruzzene, M.; Wen, J.; Yu, D.; Cai, L.; Yue, L. Band transition and topological interface modes in 1D elastic phononic crystals. Sci. Rep. 2018, 8, 6806. [Google Scholar] [CrossRef]
- Ruzzene, M.; Scarpa, F. Control of wave propagation in sandwich beams with auxetic core. J. Intell. Mater. Syst. Struct. 2003, 14, 443–453. [Google Scholar] [CrossRef]
- Mead, D.; Markus, S. The forced vibration of a three-layer, damped sandwich beam with arbitrary boundary conditions. J. Sound Vib. 1969, 10, 163–175. [Google Scholar] [CrossRef]
- Zhang, J.; Ashby, M. The out-of-plane properties of honeycombs. Int. J. Mech. Sci. 1992, 34, 475–489. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Langley, R. On the modal density and energy flow characteristics of periodic structures. J. Sound Vib. 1994, 172, 491–511. [Google Scholar] [CrossRef]
- Lin, Y.K.; McDaniel, T. Dynamics of beam-type periodic structures. J. Eng. Ind. 1969, 91, 1133–1141. [Google Scholar] [CrossRef]
- Roy, A.K.; Plunkett, R. Wave attenuation in periodic structures. J. Sound Vib. 1986, 104, 395–410. [Google Scholar] [CrossRef]
- Mead, D. A general theory of harmonic wave propagation in linear periodic systems with multiple coupling. J. Sound Vib. 1973, 27, 235–260. [Google Scholar] [CrossRef]
- Doyle, J.F. Wave propagation in structures. In Wave Propagation in Structures; Springer: Berlin/Heidelberg, Germany, 1989; pp. 126–156. [Google Scholar]
- Doyle, J.; Farris, T. Spectrally formulated element for wave propagation in 3-D frame structures. Int. J. Anal. Exp. Modal Anal. 1990, 5, 223–237. [Google Scholar]
- Gopalakrishnan, S.; Doyle, J. Wave propagation in connected waveguides of varying cross-section. J. Sound Vib. 1994, 175, 347–363. [Google Scholar] [CrossRef]
- Baz, A. Spectral finite-element modeling of the longitudinal wave propagation in rods treated with active constrained layer damping. Smart Mater. Struct. 2000, 9, 372. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gupta, M.; Ruzzene, M. Dynamics of Quasiperiodic Beams. Crystals 2020, 10, 1144. https://doi.org/10.3390/cryst10121144
Gupta M, Ruzzene M. Dynamics of Quasiperiodic Beams. Crystals. 2020; 10(12):1144. https://doi.org/10.3390/cryst10121144
Chicago/Turabian StyleGupta, Mohit, and Massimo Ruzzene. 2020. "Dynamics of Quasiperiodic Beams" Crystals 10, no. 12: 1144. https://doi.org/10.3390/cryst10121144
APA StyleGupta, M., & Ruzzene, M. (2020). Dynamics of Quasiperiodic Beams. Crystals, 10(12), 1144. https://doi.org/10.3390/cryst10121144