Lithium Niobate Single Crystals and Powders Reviewed—Part I
Abstract
:1. Introduction
2. Basic Notions and Early Developments
3. Crystal Structure
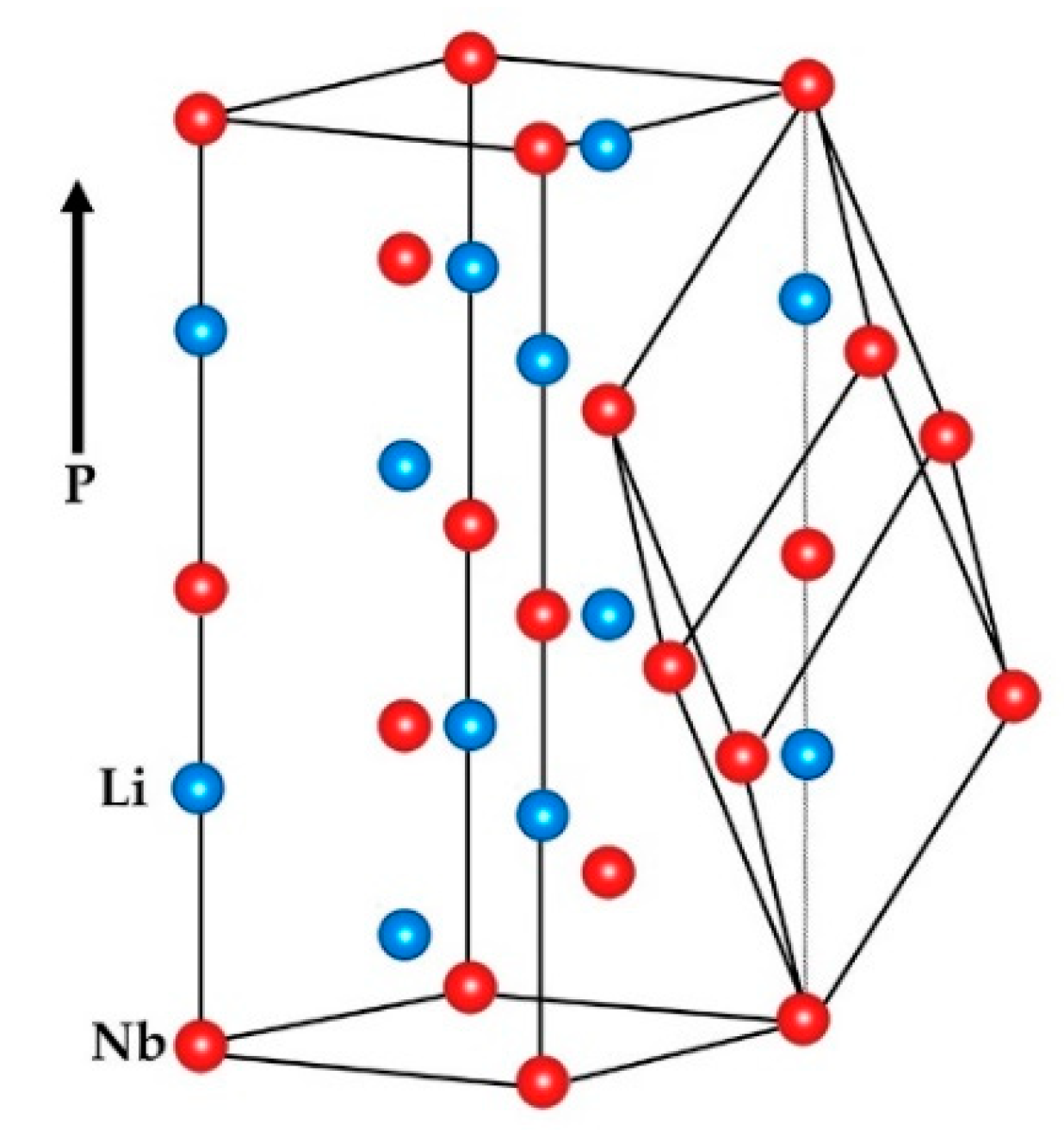
3.1. Modern/Practical Viewpoint
3.2. Earlier Thoughts: Relation to Basic Structures of Higher Symmetry
Li: (0, 0, 0.2829) ± (0, 0, 0.0023)
O: (0.0492, 0.3446, 0.0647) ± (0.0003, 0.0005, 0.0004)
4. Powders
4.1. Accurate Description of the Chemical Composition
- X-ray diffraction + structure refinement [45,47,63]. Abrahams and Marsh did earlier measurements by use of a Bond diffractometer [65,104]. Some of the available program packages for Rietveld refinement, commercial and public, and an introduction into this subject can be consulted in [104,105,106,107,108,109,110,111,112].
- Polarized raman spectroscopy [47,48,49,113,114]. The group theory elements in this field and the assignment of phonon modes in LN are discussed elsewhere [115,116,117,118,119], having a common root in the seminal paper by Schaufele and Weber (1966) [120]. The scattering geometry in polarized Raman experiments is described after Porto and Krishnan (1967) [121]. The high sensitiveness of the functional form (and intensity) of the recorded Raman spectra in LN to the experimental configuration might be observed from the educational video ‘Convenient Application of Polarized Raman Spectroscopy’, provided by the HORIBA Raman Academy [122]. The resolution of Raman bands or fitting techniques is critical for achieving great accuracy in determining the CC by this method [123,124]. Regarding this method of CC characterization, the works by Scott and Burns (1972) [125] and Balanevskaya et al. (1983) [126], are considered pioneers (both done on LNPws).
- Fundamental absorption edge [47,51,52]. One of the earliest reports on this subject was written by Redfield and Burke (1974) [127]. For practical reasons, a direct transition can be assumed regarding the intrinsic nature of the bandgap (no phonons involved for momentum conservation) [128], in which case the fundamental band gap is proportional to the square of the absorption coefficient α2 [129].
- Differential thermal analysis [43,44,45,47]. Measurement of the Curie temperature (TC) is one of the earliest calibration methods for determining the CC in LN single crystals [62]. Regarding this type of transition, as previously stated, a change in the crystal structure occurs in which the symmetry of the system decreases. The symmetry-breaking relation between the high-symmetry paraelectric structure and the ferroelectric one is consistent with a second-order transition, described by the Landau order-disorder theory [130,131]. A finite discontinuity in the heat capacity of the system having this transition has been addressed as a direct thermodynamic consequence.
4.2. Role in New Potential Applications
- ▪
- (2020) Strong and weak light scattering effects can be present simultaneously in random media (random in terms of its refractive index), such as powders. Here, accounting for optical nonlinear powders, photons can undergo multiple scattering in the sense that they undergo one SHG scattering event and single or multiple linear scattering events at the fundamental and harmonic frequencies. In contrast, ballistic photons undergo a single SHG scattering event and no linear scattering event. Depth profiling with polarization resolution of the SHG intensity from a powder stack of sLN microparticles/nanocrystals has been done in back-scattering or retro-reflection configuration. The results, supported by modeling, show that competition between multiple scattered and ballistic photons contributions holds during the focus longitudinal translation, which can be disentangled in observing polarization distortions occurring as the beam focus moves from air into powder [139]. The contribution from the multiple scattered photons dominates at all depths, whereas, at the maximum of the intensity depth profiles, the contribution of ballistic photons is at its maximum and enhanced due to collection efficiency. This work paves the way for a close and quantitative investigation of the SHG response from nonlinear optical powders and, combined with the derived conclusions in [138], the experimental configuration might also play a role in the discrimination of information: transmission experiments for the evaluation of averaged properties such as SHG efficiency (micrometer size scale of the powder) and retro-reflection experiments for overall scattering properties and local crystalline properties (nanometer size scale). The latter is a general idea somehow (not explicitly) postulated in a revision of the Kurtz and Perry method done by Aramburu et al. [140].
- ▪
- (2018) Fe-doped LNPws show, after a post-thermal treatment in a controlled reducing atmosphere, a rather strong ferromagnetic response at room temperature for a doping concentration of the order of 1 mol % [141]. This may be considered a first report of the manifestation of ferromagnetism in nanocrystalline LNPws within the regime of very low doping concentrations. Post-thermal treatment in a controlled atmosphere is a key point for inducing this behavior, which could be explained as the recombination of unpaired electrons from the donor sites (Fe impurities) to the acceptor sites (oxygen vacancies) in the surfaces of the material. A statement that needs to be further scrutinized since the opinion on the existence of this type of vacancies has changed over time; lately they are neglected, at least with respect to single crystals and within the volume (see discussion in Part II). Bulk diffusion of oxygen has been exluded from the explanation of the results recently presented by Kocksor et al. [18], where LN nanocrystals were prepared by ball-milling the crucible residues of a Czochralski grown congruent crystal. Anywise, neither the enhanced factor of surface effects nor the depletion of oxygen at the surfaces can be overruled in LNPws prepared by a mechanochemical-calcination route.
- ▪
- (2017) The same method of synthesis has been used to prepare LN micropowders whose SHG intensity is maximal at a certain λmax, in terms of the fundamental excitation wavelength. Possible tuning of λmax could be ascribed to the control of the composition and grain size of the powder [138]. Also, there is the possibility of obtaining major technical benefits by exploiting the SHG from disordered materials such as LNPws, given that neither a critical adjustment of the orientation/temperature in the material (phase-matching condition) nor the accurate engineering of a microstructure (quasi-phase matching condition), are substantially needed [142].
- ▪
- (2017) A novel fabrication process based on the powder-in-tube method to realize polarization-maintaining optical fibers has been demonstrated. It relies on the principle of “inducing an anisotropy of the refractive index in the core region by internal stress”, in which the use of powdered material with a thermal expansion coefficient (TEC) higher than that of silica (fiber core) is the key point [143]. Glass complex systems based on SiO2-Al2O3-La2O3, with a TEC around 10 times larger (5.32–6.46 × 10−6 K−1) compared to silica (0.54 × 10−6 K−1), has been used. Single crystalline LN has a TEC along the c-axis of the same order as the complex systems just mentioned (~5 × 10−6 K−1) and almost three times higher for either of the other axes (14.1 × 10−6 K−1); see Table 1. In the case of LNPws, a TEC value between these two is expected, and thus they are, in principle, good candidates for the fabrication of polarization maintaining optical fibers.
- ▪
- (2013) Cementation materials based on LN have been proposed as potential materials for an effective formation of eco-friendly end products through artificial photosynthesis; this is considered important for the global warming reduction problem [144]. Despite its wider band gap (3.8–4.1 eV) compared to that of TiO2 (3.2 eV), LN is considered a better artificial photocatalyst due to its strong remnant polarization (70 μc(cm)−2) [145]. Paraphrasing Nath et al.: “the photocatalyst LiNbO3 can be used as a construction material to emit oxygen using atmospheric CO2 and water reliably and inexpensively. The reduction of the global warming problem through the use of this photocatalyst would have a remarkably positive impact on the environment in the near future” [144]. Regarding LNPws, we emphasize that powders would not only be easier to implement than single crystals into cement-based materials but also they would enhance surface effects, perhaps improving this way the lifetime of the carriers (photo-generated electrons and holes) involved in artificial photosynthesis [145]. The importance of LNPws on these crucial matters must not be overlooked.
- ▪
- (2012) LN and LT powders of several particle sizes at the microscale and with averaged crystallite size between 30 and 300 nm, have proved to be antimicrobial agents in aqueous solutions based on cyclical thermal excitation [146]. The powders were directly obtained from the crushing of single crystals by high-energy ball milling and various solution percussion routes. The powders obtained by the latter method show smaller particle and crystallite sizes, showing improved disinfection properties due to the increased direct surface contact with the bacterium.
4.3. Methods of Synthesis
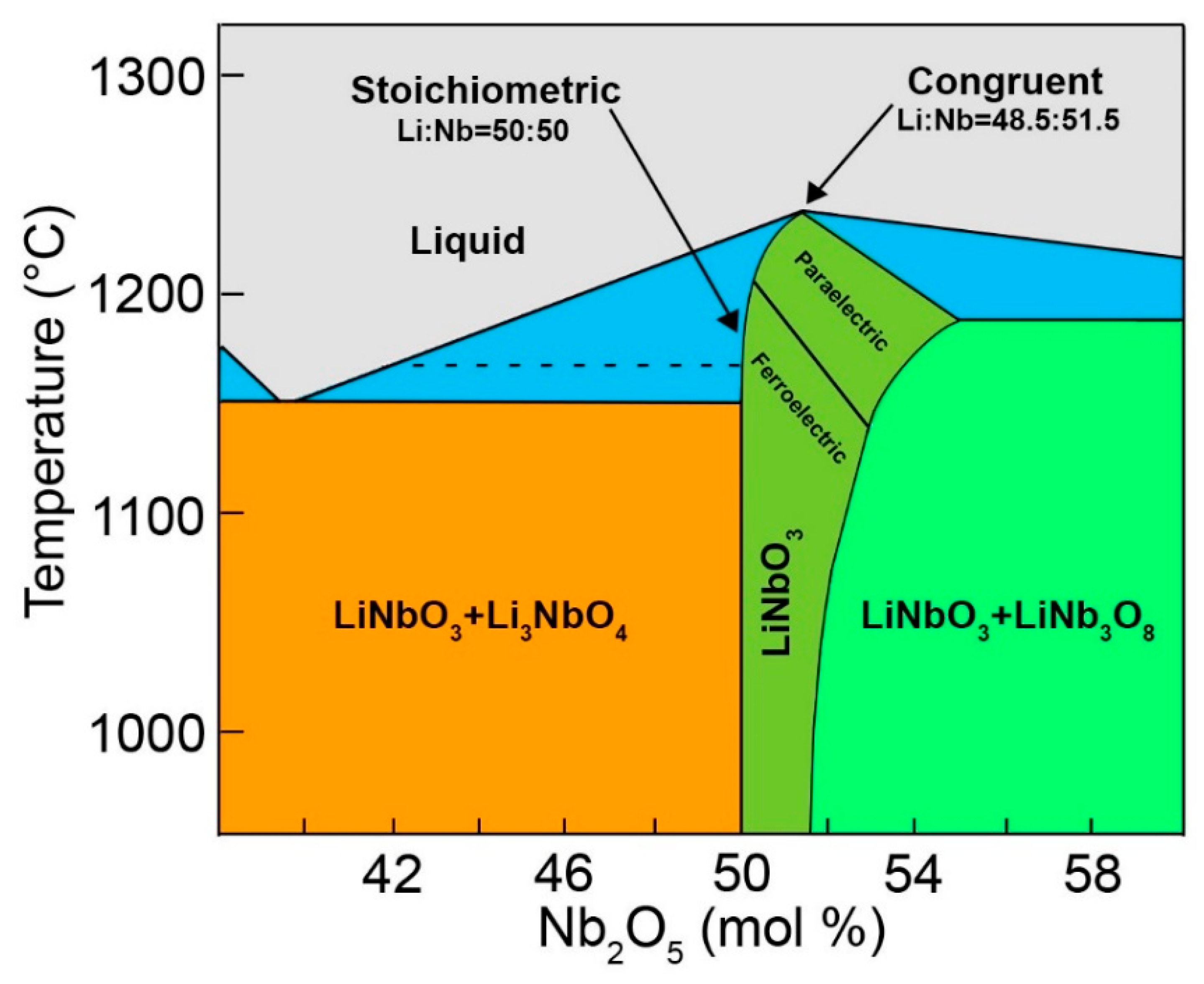
4.4. Are the Stoichiometric and Congruent Compositions Univocally Determined?
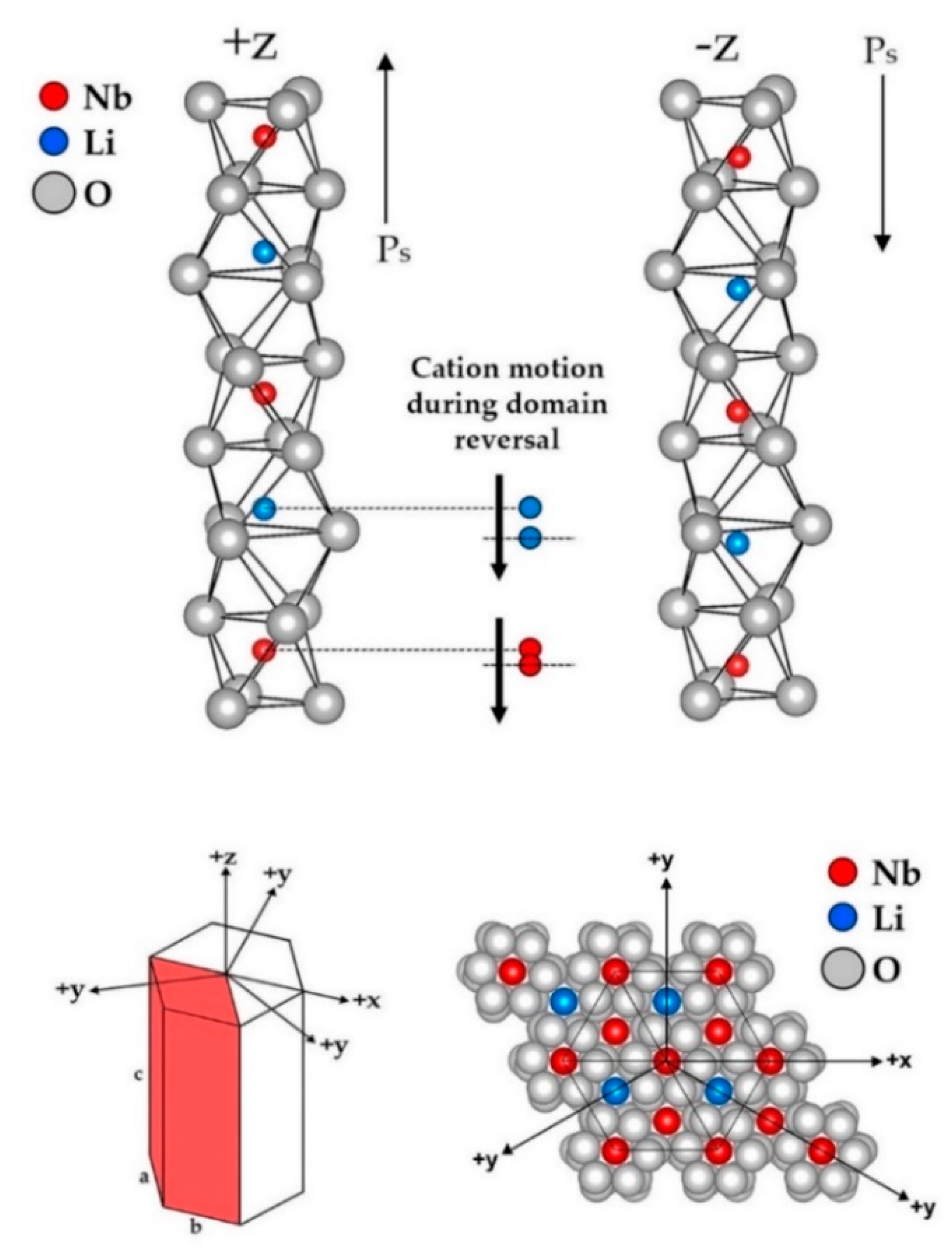
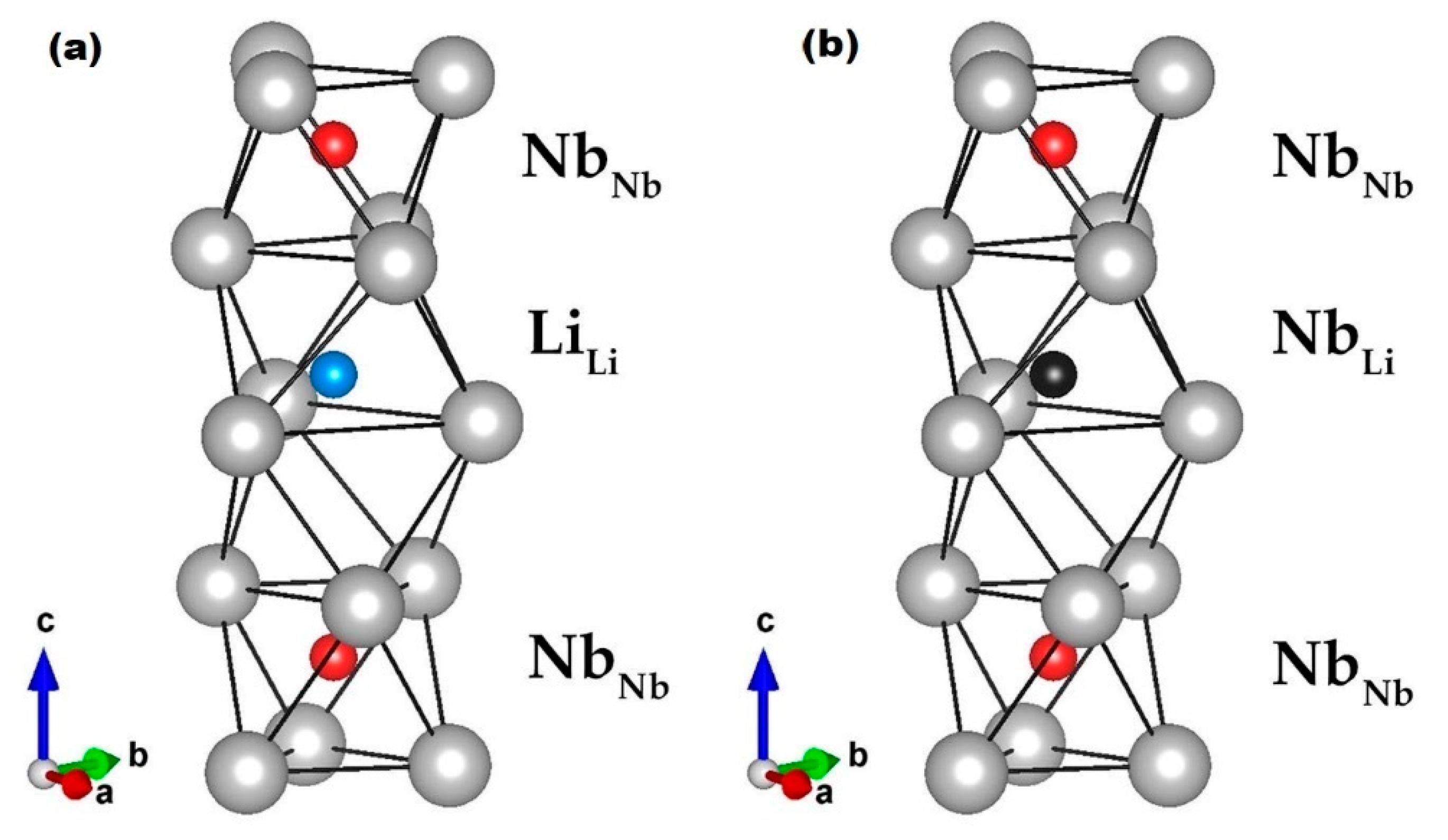
5. Ferroelectricity behind the Curtain
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Weis, R.S.; Gaylord, T.K. Lithium niobate: Summary of physical properties and crystal structure. Appl. Phys. A 1985, 37, 191–203. [Google Scholar] [CrossRef]
- Räuber, A. Chemistry and Physics of Lithium Niobate. In Current Topics in Materials Science, 1st ed.; Kaldis, E., Ed.; North-Holland: Amsterdam, The Netherlands, 1978; Volume 1, pp. 481–601. [Google Scholar]
- Bartasyte, A.; Margueron, S.; Baron, T.; Oliveri, S.; Boulet, P. Toward High-Quality Epitaxial LiNbO3 and LiTaO3 Thin Films for Acoustic and Optical Applications. Adv. Mater. Interfaces 2017, 4, 1600998. [Google Scholar] [CrossRef]
- Streque, J.; Aubert, T.; Kokanyan, N.; Bartoli, F.; Taguett, A.; Polewczyk, V.; Kokanyan, E.; Hage-Ali, S.; Boulet, P.; Elmazria, O. Stoichiometric Lithium Niobate Crystals: Towards Identifiable Wireless Surface Acoustic Wave Sensors Operable up to 600 °C. IEEE Sensors Lett. 2019, 3, 2501204. [Google Scholar] [CrossRef]
- Harvard John, A. Paulson School of Engineering and Applied Sciences, “Now Entering, Lithium Niobate Valley: Researchers Demonstrate High-Quality Optical Microstructures Using Lithium Niobate” by Leah Burrows. Available online: https://www.seas.harvard.edu/news/2017/12/now-entering-lithium-niobate-valley (accessed on 31 July 2020).
- Kösters, M.; Sturman, B.; Werheit, P.; Haertle, D.; Buse, K. Optical cleaning of congruent lithium niobate crystals. Nat. Photon 2009, 3, 510–513. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, C.; Cheng, R.; Shams-Ansari, A.; Lončar, M. Monolithic ultra-high-Q lithium niobate microring resonator. Optica 2017, 4, 1536–1537. [Google Scholar] [CrossRef]
- Desiatov, B.; Shams-Ansari, A.; Zhang, M.; Wang, C.; Loncar, M. Ultra-low-loss integrated visible photonics using thin-film lithium niobate. Optics 2019, 6, 380–384. [Google Scholar] [CrossRef] [Green Version]
- Osborne, I.S. An active platform for integrated optics. Science 2019, 364, 448. [Google Scholar] [CrossRef] [Green Version]
- Pang, C.; Li, R.; Li, Z.; Dong, N.; Cheng, C.; Nie, W.; Böttger, R.; Zhou, S.; Wang, J.; Chen, F. Lithium Niobate Crystal with Embedded Au Nanoparticles: A New Saturable Absorber for Efficient Mode-Locking of Ultrafast Laser Pulses at 1 µm. Adv. Opt. Mater. 2018, 6, 180035. [Google Scholar] [CrossRef]
- Kuhn, T.S. The Structure of Scientific Revolutions, 1st ed.; University of Chicago Press: Chicago, IL, USA, 1962; p. 172. [Google Scholar]
- Megaw, H.D. Ferroelectricity and crystal structure. II. Acta Crystallogr. 1954, 7, 187–194. [Google Scholar] [CrossRef]
- Megaw, H.D. Ferroelectricity in Crystals, 1st ed.; Methuen: London, UK, 1957. [Google Scholar]
- Megaw, H.D. A note on the structure of lithium niobate, LiNbO3. Acta Crystallogr. Sect. A Cryst. Phys. Diffr. Theor. Gen. Crystallogr. 1968, A24, 583–588. [Google Scholar] [CrossRef]
- Valasek, J. Piezo-Electric and Allied Phenomena in Rochelle Salt. Phys. Rev. 1921, 17, 475–481. [Google Scholar] [CrossRef]
- Brennecka, G.; Sherbondy, R.; Schwartz, R.; Ihlefeld, J. Ferroelectricity—A revolutionary century of discovery. Am. Cer. Soc. Bull. 2020, 99, 24–30. [Google Scholar]
- Kang, X.; Liang, L.; Song, W.; Wang, F.; Sang, Y.; Liu, H. Formation mechanism and elimination methods for anti-site defects in LiNbO3/LiTaO3 crystals. Cryst. Eng. Comm. 2016, 18, 8136–8146. [Google Scholar] [CrossRef]
- Kocsor, L.; Péter, L.; Corradi, G.; Kis, Z.; Gubicza, J.; Kovács, L. Mechanochemical Reactions of Lithium Niobate Induced by High-Energy Ball-Milling. Crystals 2019, 9, 334. [Google Scholar] [CrossRef] [Green Version]
- Sánchez-Dena, O.; Villagómez, C.J.; Fierro-Ruíz, C.D.; Padilla-Robles, A.S.; Farías, R.; Vigueras-Santiago, E.; Hernández-López, S.; Reyes-Esqueda, J.-A. Determination of the Chemical Composition of Lithium Niobate Powders. Crystals 2019, 9, 340. [Google Scholar] [CrossRef] [Green Version]
- Volk, T.; Wöhlecke, M. Introduction. In Lithium Niobate. Defects, Photorefraction and Ferroelectric Switching, 1st ed.; Springer Series in Materials Science 115; Hull, R., Osgood, R.M., Jr., Parisi, J., Warlimont, H., Eds.; Springer: Berlin/Heidelberg, Germany, 2009; Volume 115, pp. 1–8. [Google Scholar]
- Cudney, R.S.; Ríos, L.A.; Orozco-Arellanes, M.J.; Alonso, F.; Fonseca, J. Fabricación de niobato de litio periódicamente polarizado para óptica no lineal. Rev. Mex. Física 2002, 48, 548–555. [Google Scholar]
- Zachariasen, F.W.H. Standard x-ray diffraction powder patterns. [Kl.] 1 Mat. Naturv-Idensk. Kl 1928, 4, 1–8. [Google Scholar]
- Lima-de-Faria, J. Structural Mineralogy. An Introduction, 1st ed.; Springer Science+Business Media: Dordrecht, UK, 1994. [Google Scholar]
- Sumets, M.P.; Dybov, V.A.; Ievlev, V.M. LiNbO3 films: Potential application, synthesis techniques, structure, properties. Inorg. Mater. 2017, 53, 1361–1377. [Google Scholar] [CrossRef]
- Crystals: Special Issue “Recent Progress in Lithium Niobate”, Special Issue Information (Guest Editors: Robert A. Jackson and Zsuzsanna Szaller). Available online: https://www.mdpi.com/journal/crystals/special_issues/Lithium_Niobate (accessed on 31 July 2020).
- Matthias, B.T.; Remeika, J.P. Ferroelectricity in the Ilmenite Structure. Phys. Rev. 1949, 76, 1886–1887. [Google Scholar] [CrossRef]
- Nassau, K. Early History of Lithium Niobate: Personal Reminiscences. In Proceedings of the Guided Wave Optoelectronic Materials I, Los Angeles Technical Symposium, Los Angeles, CA, USA, 26 September 1984; Holman, R.L., Smyth, D.M., Eds.; SPIE Digital Library; Proc. SPIE 0460. pp. 2–5. [Google Scholar]
- Ballman, A.A. Growth of Piezoelectric and Ferroelectric Materials by the Czochralski Technique. J. Am. Ceram. Soc. 1965, 48, 112–113. [Google Scholar] [CrossRef]
- Fedulov, S.A.; Shapiro, Z.I.; Ladyzhenskii, P.B. Apparatus for Growth of Single Crystal, Single Domain LiNbO3. Kristals 1965, 10, 268–273. [Google Scholar]
- Nassau, K.; Levinstein, H.; LoIacono, G. Ferroelectric lithium niobate. 1. Growth, domain structure, dislocations and etching. J. Phys. Chem. Solids 1966, 27, 839–888. [Google Scholar] [CrossRef]
- Nassau, K.; Levinstein, H.; LoIacono, G. Ferroelectric lithium niobate. 2. Preparation of single domain crystals. J. Phys. Chem. Solids 1966, 27, 989–996. [Google Scholar] [CrossRef]
- Abrahams, S.C.; Reddy, J.M.; Bernstein, J.L. Ferroelectric Lithium Niobate. 3. Single crystal X-ray diffraction study at 24 °C. J. Phys. Chem. Solids 1966, 27, 971–1012. [Google Scholar]
- Abrahams, S.C.; Hamilton, W.C.; Reddy, J.M. Ferroelectric Lithium Niobate. 4. Single crystal neutron diffraction study at 24 °C. J. Phys. Chem. Solids 1966, 27, 1013–1018. [Google Scholar] [CrossRef]
- Abrahams, S.C.; Levinstein, H.J.; Reddy, J.M. Ferroelectric Lithium Niobate. 5. Polycrystal X-ray diffraction study between 24 °C and 1200 °C. J. Phys. Chem. Solids 1966, 27, 1019–1026. [Google Scholar]
- Bartasyte, A.; Plausinaitiene, V.; Abrutis, A.; Stanionyte, S.; Margueron, S.; Boulet, P.; Kobata, T.; Uesu, Y.; Gleize, J. Identification of LiNbO3, LiNb3O8 and Li3NbO4 phases in thin films synthesized with different deposition techniques by means of XRD and Raman spectroscopy. J. Phys. Condens. Matter 2013, 25, 205901. [Google Scholar] [CrossRef]
- Hatano, H.; Kitamura, K.; Liu, Y. Growth and Photorefractive Properties of Stoichiometric LiNbO3 and LiTaO3. In Photorefractive Materials and Their Applications 2; Springer: New York, NY, USA, 2007; Volume 114, pp. 127–164. [Google Scholar]
- Kitamura, K.; Yamamoto, J.; Iyi, N.; Kirnura, S.; Hayashi, T. Stoichiometric LiNbO3 single crystal growth by double crucible Czochralski method using automatic powder supply system. J. Cryst. Growth 1992, 116, 327–332. [Google Scholar] [CrossRef]
- Malovichko, G.I.; Grachev, V.G.; Yurchenko, L.P.; Proshko, V.Y.; Kokanyan, E.; Gabrielyan, V.T. Improvement of LiNbO3 Microstructure by Crystal Growth with Potassium. Phys. Status Solidi (a) 1992, 133, K29–K32. [Google Scholar] [CrossRef]
- Bordui, P.F.; Norwood, R.G.; Jundt, D.H.; Fejer, M.M. Preparation and characterization of off-congruent lithium niobate crystals. J. Appl. Phys. 1992, 71, 875–879. [Google Scholar] [CrossRef] [Green Version]
- Polgár, K.; Péter, Á.; Kovács, L.; Corradi, G.; Szaller, Z. Growth of stoichiometric LiNbO3 single crystals by top seeded solution growth method. J. Cryst. Growth 1997, 177, 211–216. [Google Scholar] [CrossRef]
- Polgár, K.; Péter, Á; Földvári, I. Crystal growth and stoichiometry of LiNbO3 prepared by the flux method. Opt. Mater. 2002, 19, 7–11. [Google Scholar] [CrossRef]
- Lengyel, K.; Péter, Á.; Kovács, L.; Corradi, G.; Pálfalvi, L.; Hebling, J.; Unferdorben, M.; Dravecz, G.; Hajdara, I.; Szaller, Z.; et al. Growth, defect structure, and THz application of stoichiometric lithium niobate. Appl. Phys. Rev. 2015, 2, 040601. [Google Scholar] [CrossRef] [Green Version]
- O’Bryan, H.M.; Gallagher, P.K.; Brandle, C.D. Congruent Composition and Li-Rich Phase Boundary of LiNbO3. J. Am. Ceram. Soc. 1985, 68, 493–496. [Google Scholar] [CrossRef]
- Grabmaier, B.; Otto, F. Growth and investigation of MgO-doped LiNbO3. J. Cryst. Growth 1986, 79, 682–688. [Google Scholar] [CrossRef]
- Iyi, N.; Kitamura, K.; Izumi, F.; Yamamoto, J.; Hayashi, T.; Asano, H.; Kimura, S. Comparative of defect structures in lithium niobate with different compositions. J. Solid State Chem. 1992, 101, 340–352. [Google Scholar] [CrossRef]
- Furukawa, Y.; Sato, M.; Kitamura, K.; Yajima, Y.; Minakata, M. Optical damage resistance and crystal quality of LiNbO3 single crystals with various [Li]/[Nb] ratios. J. Appl. Phys. 1992, 72, 3250–3254. [Google Scholar] [CrossRef]
- Wöhlecke, M.; Corradi, G.; Betzler, K. Optical methods to characterise the composition and homogeneity of lithium niobate single crystals. Appl. Phys. B 1996, 63, 323–330. [Google Scholar] [CrossRef]
- Schlarb, U.; Klauer, S.; Wesselmann, M.; Betzler, K. Determination of the Li/Nb ratio in lithium niobate by means of birefringence and Raman measurements. Appl. Phys. A 1993, 56, 311–315. [Google Scholar] [CrossRef]
- Malovichko, G.I.; Grachev, V.G.; Kokanyan, E.; Schirmer, O.F.; Betzler, K.; Gather, B.; Jermann, F.; Klauer, S.; Schlarb, U. Characterization of stoichiometric LiNbO3 grown from melts containing K2O. Appl. Phys. A 1993, 56, 103–108. [Google Scholar] [CrossRef]
- Vartanyan, E.S. Lecture on the All-Union Conference on Crystal Growth. Tsachkadzor-Aghveran: Armenian SSR. 1985. Available online: https://aip.scitation.org/doi/abs/10.1063/1.4929917?crawler=true&mimetype=application%2Fpdf&journalCode=are (accessed on 18 October 2020).
- Földvári, I.; Polgár, K.; Voszka, R.; Balasanyan, R.N. A simple method to determine the real composition of LiNbO3 crystals. Cryst. Res. Technol. 1984, 19, 1659–1661. [Google Scholar] [CrossRef]
- Kovacs, L.; Ruschhaupt, G.; Polgár, K.; Corradi, G.; Wöhlecke, M. Composition dependence of the ultraviolet absorption edge in lithium niobate. Appl. Phys. Lett. 1997, 70, 2801–2803. [Google Scholar] [CrossRef]
- Dravecz, G.; Kovács, L. Determination of the crystal composition from the OH- vibrational spectrum in lithium niobate. Appl. Phys. A 2007, 88, 305–307. [Google Scholar] [CrossRef]
- Dravecz, G.; Kovács, L.; Péter, Á.; Polgár, K.; Bourson, P. Raman and infrared spectroscopy characterization of LiNbO3 crystals grown from alkali metal oxide solvents. Phys. Status Solidi 2007, 4, 1313–1316. [Google Scholar]
- Schlarb, U.; Betzler, K. Refractive indices of lithium niobate as a function of temperature, wavelength, and composition: A generalized fit. Phys. Rev. B 1993, 48, 15613–15620. [Google Scholar] [CrossRef] [PubMed]
- Byer, R.L.; Young, J.F.; Feigelson, R.S. Growth of High-Quality LiNbO3 Crystals from the Congruent Melt. J. Appl. Phys. 1970, 41, 2320. [Google Scholar] [CrossRef]
- Luh, Y.; Fejer, M.; Byer, R.; Feigelson, R. Stoichiometric LiNbO3 single-crystal fibers for nonlinear optical applications. J. Cryst. Growth 1987, 85, 264–269. [Google Scholar] [CrossRef]
- Jundt, D.; Fejer, M.; Byer, R. Optical properties of lithium-rich lithium niobate fabricated by vapor transport equilibration. IEEE J. Quantum Electron. 1990, 26, 135–138. [Google Scholar] [CrossRef]
- Schmidt, N.; Betzler, K.; Grabmaier, B.C. Composition dependence of the second-harmonic phase-matching temperature in LiNbO3. Appl. Phys. Lett. 1991, 58, 34–35. [Google Scholar] [CrossRef]
- Reichert, A.; Betzler, K. Characterization of electrooptic crystals by non-collinear frequency doubling. Ferroelectrics 1992, 126, 9–14. [Google Scholar] [CrossRef] [Green Version]
- Arizmendi, L. Simple holographic method for determination of Li/Nb ratio and homogeneity of LiNbO3 crystals. J. Appl. Phys. 1988, 64, 4654–4656. [Google Scholar] [CrossRef]
- Carruthers, J.R.; Peterson, G.E.; Grasso, M.; Bridenbaugh, P.M. Nonstoichiometry and Crystal Growth of Lithium Niobate. J. Appl. Phys. 1971, 42, 1846. [Google Scholar] [CrossRef]
- Zotov, N.; Boysen, H.; Frey, F.; Metzger, T.; Born, E. Cation substitution models of congruent LiNbO3 investigated by X-ray and neutron powder diffraction. J. Phys. Chem. Solids 1994, 55, 145–152. [Google Scholar] [CrossRef]
- Lerner, P.; Legras, C.; Dumas, J.P. STOECHIOMÉTIE DES MONOCRISTAUX DE MÉTANIOBATE DE LITHIUM. J. Cryst. Growth 1968, 3, 231–235. [Google Scholar] [CrossRef]
- Abrahams, S.C.; Marsh, P. Defect structure dependence on composition in lithium niobate. Acta Crystallogr. Sect. B Struct. Sci. 1986, B42, 61–68. [Google Scholar] [CrossRef]
- Kovács, L.; Polgár, K. Density Measurements on LiNbO3 Crystals Confirming Nb Substitution for Li. Cryst. Res. Technol. 1986, 21, K101–K104. [Google Scholar] [CrossRef]
- Peterson, G.; Carruthers, J. 93Nb NMR as a sensitive and accurate probe of stoichiometry in LiNbO3 crystals. J. Solid State Chem. 1969, 1, 98–99. [Google Scholar] [CrossRef]
- Malovichko, G.; Grachev, V.; Schirmer, O. The effect of iron ions on the defect structure of lithium niobate crystals grown from K2O containing melts. Solid State Commun. 1994, 89, 195–198. [Google Scholar] [CrossRef]
- Yamada, K.; Takemura, H.; Inoue, Y.; Omi, T.; Matsumura, S. Effect of Li/Nb Ratio on the SAW Velocity of 128°Y–X LiNbO3Wafers. Jpn. J. Appl. Phys. 1987, 26, 219. [Google Scholar] [CrossRef]
- Kushibiki, J.; Takahashi, H.; Kobayashi, T.; Chubachi, N. Characterization of LiNbO3 crystals by line-focus-beam acoustic microscopy. Appl. Phys. Lett. 1991, 58, 2622–2624. [Google Scholar] [CrossRef]
- Muller, O.; Roy, R. The Major Ternary Structural Families; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1974. [Google Scholar]
- Schweinler, H.C. Ferroelectricity in the Ilmenite Structure. Phys. Rev. 1952, 87, 5–11. [Google Scholar] [CrossRef]
- Alves, A.R.; Coutinho, A.D.R. The Evolution of the Niobium Production in Brazil. Mater. Res. 2015, 18, 106–112. [Google Scholar] [CrossRef] [Green Version]
- Bailey, P. Measurement of the Proton Structure Function F2 at Very Low Q2 at HERA Doctoral Thesis. Bristol: 1952. Available online: https://www.sciencedirect.com/science/article/abs/pii/S0370269300007930 (accessed on 31 July 2020).
- Volk, T.; Wöhlecke, M. Point defects in LiNbO3. In Lithium Niobate. Defects, Photorefraction and Ferroelectric Switching, 1st ed.; Hull, R., Osgood, R.M., Jr., Parisi, J., Warlimont, H., Eds.; Springer Series in Materials Science 115; Springer: Berlin/Heidelberg, Germany, 2009; Volume 115, pp. 9–50. [Google Scholar]
- Sanna, S.; Schmidt, W.G. Lithium niobate X-cut, Y-cut, and Z-cut surfaces from ab initio theory. Phys. Rev. B 2010, 81, 214116. [Google Scholar] [CrossRef]
- Gopalan, V.; Dierolf, V.; Scrymgeour, D.A. Defect–Domain Wall Interactions in Trigonal Ferroelectrics. Annu. Rev. Mater. Res. 2007, 37, 449–489. [Google Scholar] [CrossRef] [Green Version]
- Peterson, G.E.; Carnevale, A. 93Nb NMR Linewidths in Nonstoichiometric Lithium Niobate. J. Chem. Phys. 1972, 56, 4848–4851. [Google Scholar] [CrossRef]
- Wilkinson, A.P.; Cheetham, A.K.; Jarman, R.H. The defect structure of congruently melting lithium niobate. J. Appl. Phys. 1998, 74, 3080–3083. [Google Scholar] [CrossRef]
- Peterson, G.E. Private Communication. 1965. Available online: https://aip.scitation.org/doi/abs/10.1063/1.1840478 (accessed on 18 October 2020).
- Buerger, M.J. Derivative Crystal Structures. J. Chem. Phys. 1947, 15, 1–16. [Google Scholar] [CrossRef]
- Buerger, M.J. The genesis of twin crystals. Am. Mineral. 1945, 30, 469–482. [Google Scholar]
- Bärnighausen, H. Group-Subgroup Relations between Space Groups: A Useful Tool in Crystal Chemistry. Match Commun. Math. Chem. 1980, 9, 139–175. [Google Scholar]
- Benz, K.-W.; Neumann, W. Introduction to Crystal Growth and Characterization, 1st ed.; Wiley-VCH Verlag GmbH & Co.: Weinheim, Germany, 2014. [Google Scholar]
- Megaw, H.D.; Templeton, D.H. Crystal Structures: A Working Approach. Phys. Today 1974, 27, 53. [Google Scholar] [CrossRef]
- Johnsson, M.; Lemmens, P. Crystallography and Chemistry of Perovskites. In Handbook of Magnetism and Advanced Magnetic Materials; Kronmüller, H., Parkin, S., Coey, M., Inoue, A., Kronmüller, H., Eds.; Wiley: Hoboken, NJ, USA, 2007; Volume 4, pp. 1–9. Available online: https://onlinelibrary.wiley.com/doi/abs/10.1002/9780470022184.hmm411 (accessed on 18 October 2020).
- Bhalla, A.; Guo, R.; Roy, R. The perovskite structure—A review of its role in ceramic science and technology. Mater. Res. Innov. 2000, 4, 3–26. [Google Scholar] [CrossRef]
- Howard, C.J.; Stokes, H.T. Structures and phase transitions in perovskites—A group-theoretical approach. Acta Cryst. 2005, A61, 93–111. [Google Scholar] [CrossRef] [PubMed]
- Woodward, P.M.; Mizoguchi, H.; Kim, Y.-I.; Stoltzfus, M.W. Chapter 6. The Electronic Structure of Metal Oxides. In Metal Oxides: Chemistry and Applications, 1st ed.; Fierro, J.L.G., Ed.; CRC Press Taylor & Francis Group: Boca Raton, FL, USA, 2006; pp. 133–193. [Google Scholar]
- Lufaso, M.W.; Woodward, P.M. Prediction of the crystal structures of perovskites using the software program SPuDS. Acta Crystallogr. Sect. B Struct. Sci. 2001, B57, 725–738. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rabe, K.M.; Dawber, M.; Lichtensteiger, C.; Ahn, C.H.; Triscone, J.-M. Modern Physics of Ferroelectrics: Essential Background. In Physics of Ferroelectrics: A Modern Perspective, 1st ed.; Rabe, K.M., Ahn, C.H., Triscone, J.-M., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 1–30. [Google Scholar]
- Navrotsky, A. Energetics and Crystal Chemical Systematics among Ilmenite, Lithium Niobate, and Perovskite Structures. Chem. Mater. 1998, 10, 2787–2793. [Google Scholar] [CrossRef]
- Shannon, R.D.; Prewitt, C.T. Effective Ionic Radii in Oxides and Flourides. Acta Cryst. 1969, B25, 925–946. [Google Scholar] [CrossRef]
- Takayama-Muromachi, E.; Navrotsky, A. Energetics of compounds (A2+B4+O3) with the perovskite structure. J. Solid State Chem. 1988, 72, 244–256. [Google Scholar] [CrossRef]
- Kubo, A.; Giorgi, G.; Yamashita, K. MgTaO2N Photocatalysts: Perovskite versus Ilmenite Structure. A Theoretical Investigation. J. Phys. Chem. C 2017, 121, 27813–27821. [Google Scholar] [CrossRef]
- Wilson, N.C.; Muscat, J.; Mkhonto, D.; Ngoepe, P.E.; Harrison, N.M. Structure and properties of ilmenite from first principles. Phys. Rev. B 2005, 71, 075202. [Google Scholar] [CrossRef]
- Leinenweber, K.; Utsumi, W.; Tsuchida, Y.; Yagi, T.; Kurita, K. Unquenchable high-pressure perovskite polymorphs of MnSnO3 and FeTiO3. Phys. Chem. Miner. 1991, 18, 244–250. [Google Scholar] [CrossRef]
- Mehta, A.; Leinenweber, K.; Navrotsky, A.; Akaogi, M. Calorimetric study of high pressure polymorphism in FeTiO3: Stability of the perovskite phase. Phys. Chem. Miner. 1994, 21, 207–212. [Google Scholar] [CrossRef]
- Haertling, G.H. Ferroelectric Ceramics: History and Technology. J. Am. Ceram. Soc. 1999, 82, 797–818. [Google Scholar] [CrossRef]
- Barth, T.F.W.; Posnjak, E. The Crystal Structure of Ilmenite. Z. Kristallographie Cryst. Mater. 1934, 88, 265–270. [Google Scholar] [CrossRef]
- Shiozaki, Y.; Mitsui, T. Powder neutron diffraction study of LiNbO3. J. Phys. Chem. Solids 1963, 24, 1057–1061. [Google Scholar] [CrossRef]
- Niizeki, N.; Yamada, T.; Toyoda, H. Growth Ridges, Etched Hillocks, and Crystal Structure of Lithium Niobate. J. Appl. Phys. 1967, 6, 318–327. [Google Scholar] [CrossRef]
- Heinrich, V.E.; Cox, P.A. The Surface Science of Metal Oxides, 1st ed.; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]
- Bond, W.L. Precision lattice constant determination. Acta Crystallogr. 1960, 13, 814–818. [Google Scholar] [CrossRef]
- XRD Software-DIFRAC.SUITE, TOPAS Software. Available online: https://www.bruker.com/products/x-ray-diffraction-and-elemental-analysis/x-ray-diffraction/xrd-software/topas.html (accessed on 31 July 2020).
- BGMN Home Page. Site Map. Available online: http://www.bgmn.de/ (accessed on 31 July 2020).
- Malvern Panalytical, HighScore Plus. Available online: https://www.malvernpanalytical.com/en/products/category/software/x-ray-diffraction-software/highscore-with-plus-option?creative=338893424388&keyword=x%20pert%20highscore%20plus&matchtype=e&network=g&device=c&gclid=EAIaIQobChMIwK708OSg5QIVDtvACh1vLAubEAAYASAAEgJf3PD_BwE (accessed on 31 July 2020).
- FullProf Suite. Available online: https://www.ill.eu/sites/fullprof/ (accessed on 31 July 2020).
- GSAS-II. Available online: https://subversion.xray.aps.anl.gov/trac/pyGSAS (accessed on 31 July 2020).
- Young, R.A. The Rietveld Method, 1st ed.; Oxford University Press: New York, NY, USA, 1993. [Google Scholar]
- Pecharsky, V.K.; Zavalij, P.Y. Fundamentals of Powder Diffraction and Structural Characterization of Materials, 2nd ed.; Springer: New York, NY, USA, 2009. [Google Scholar]
- Dreele, R.B. Rietveld Refinement. In Powder Diffraction: Theory and Practice, 1st ed.; Dinnebier, R.E., Billinge, S.J.L., Eds.; The Royal Society of Chemistry: Cambridge, UK, 2008; pp. 266–281. [Google Scholar]
- Bish, D.L.; Howard, S.A. Quantitative phase analysis using the Rietveld method. J. Appl. Crystallogr. 1988, 21, 86–91. [Google Scholar] [CrossRef]
- Zhang, Y.; Guilbert, L.; Bourson, P.; Polgar, K.; Fontana, M.D. Characterization of short-range heterogeneities in sub-congruent lithium niobate by micro-Raman spectroscopy. J. Phys. Condens. Matter 2006, 18, 957–963. [Google Scholar] [CrossRef]
- Fontana, M.D.; Bourson, P. Microstructure and defects probed by Raman spectroscopy in lithium niobate crystals and devices. Appl. Phys. Rev. 2015, 2, 040602. [Google Scholar] [CrossRef]
- Sanna, S.; Neufeld, S.; Rüsing, M.; Berth, G.; Zrenner, A.; Schmidt, W.G. Raman scattering efficiency in LiTaO3 and LiNbO3 crystals. Phys. Rev. B 2015, 91, 224302. [Google Scholar] [CrossRef]
- Pezzotti, G. Raman spectroscopy of piezoelectrics. J. Appl. Phys. 2013, 113, 211301. [Google Scholar] [CrossRef]
- Hermet, P.; Veithen, M.; Ghosez, P. First-principles calculations of the nonlinear optical susceptibilities and Raman scattering spectra of lithium niobate. J. Phys. Condens. Matter 2007, 19, 456202. [Google Scholar] [CrossRef]
- Margueron, S.; Bartasyte, A.; Glazer, A.M.; Simon, E.; Hlinka, J.; Gregora, I.; Gleize, J. Resolved E-symmetry zone-centre phonons in LiTaO3 and LiNbO3. J. Appl. Phys. 2012, 111, 104105. [Google Scholar] [CrossRef]
- Schaufele, R.F.; Weber, M.J. Raman Scattering by Lithium Niobate. Phys. Rev. 1966, 152, 705–708. [Google Scholar] [CrossRef]
- Porto, S.P.S.; Krishnan, R.S. Raman Effect of Corundum. J. Chem. Phys. 1967, 47, 1009–1012. [Google Scholar] [CrossRef]
- Horiba Scientific: Webinar: Applied Polarized Raman Spectroscopy Webinar (by David Tuschel). Available online: http://www.horiba.com/us/en/scientific/products/raman-spectroscopy/raman-academy/webinars/applied-polarized-raman-spectroscopy-webinar/ (accessed on 31 July 2020).
- Repelin, Y.; Husson, E.; Bennani, F.; Proust, C. Raman spectroscopy of lithium niobate and lithium tantalate. Force field calculations. J. Phys. Chem. Solids 1999, 60, 819–825. [Google Scholar] [CrossRef]
- Thermo Fisher Scientific, Application Note: Curve Fitting in Raman and IR Spectroscopy. Available online: https://www.thermofisher.com/search/results?query=Curve%20Fitting%20in%20Raman&focusarea=Search%20All (accessed on 31 July 2020).
- Scott, B.A.; Burns, G. Determination of Stoichiometry Variations in LiNbO3 and LiTaO3 by Raman Powder Spectroscopy. J. Am. Ceram. Soc. 1972, 55, 225–230. [Google Scholar] [CrossRef]
- Pyatigorskaya, L.I.; Shapiro, Z.I.; Margolin, L.N.; Bovina, E.A. Determination of the composition of LiNbO3 specimens by Raman spectroscopy. J. Appl. Spectrosc. 1983, 38, 491–493. [Google Scholar] [CrossRef]
- Redfield, D. Optical absorption edge of LiNbO3. J. Appl. Phys. 1974, 45, 4566–4571. [Google Scholar] [CrossRef]
- Thierfelder, C.; Sanna, S.; Schindlmayr, A.; Schmidt, W.G. Do we know the band gap of lithium niobate? Phys. Status Solidi 2010, 7, 362–365. [Google Scholar] [CrossRef]
- Fox, M. Optical Properties of Solids, 1st ed.; Oxford University Press: New York, NY, USA, 2001. [Google Scholar]
- Jona, F.; Shirane, G. Ferroelectric Crystals; Dover: New York, NY, USA, 1993. [Google Scholar]
- Chandra, P.; Littlewood, P.B. A Landau Primer for Ferroelectrics. In Physics of Ferroelectrics: A Modern Perspective, 1st ed.; Rabe, K.M., Ahn, C.H., Triscone, J.-M., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 69–115. [Google Scholar]
- Liu, M.; Xue, D.; Li, K. Soft-chemistry synthesis of LiNbO3 crystallites. J. Alloy. Compd. 2008, 449, 28–31. [Google Scholar] [CrossRef]
- Torrent, J.; Barrón, V. Diffuse Reflectance Spectroscopy. In Methods of Soil Analysis Part 5—Mineralogical Methods, 1st ed.; Ulery, A.L., Drees, R., Eds.; Soil Society of America: Dane County, WI, USA, 2008; pp. 367–385. [Google Scholar]
- Kubelka, P. New Contributions to the Optics of Intensely Light-Scattering Materials Part I. J. Opt. Soc. Am. 1948, 38, 448–457. [Google Scholar] [CrossRef] [PubMed]
- Kubelka, P. New Contributions to the Optics of Intensely Light-Scattering Materials Part II: Nonhomogeneous Layers. J. Opt. Soc. Am. 1954, 44, 330–335. [Google Scholar] [CrossRef]
- Kurtz, S.K.; Perry, T.T. A Powder Technique for the Evaluation of Nonlinear Optical Materials. J. Appl. Phys. 1968, 39, 3798–3813. [Google Scholar] [CrossRef]
- Chowdhury, A.U.; Zhang, S.; Simpson, G.J. Powders Analysis by Second Harmonic Generation Microscopy. Anal. Chem. 2016, 88, 3853–3863. [Google Scholar] [CrossRef]
- Dena, O.S.; García-Ramírez, E.V.; Fierro-Ruiz, C.; Vigueras-Santiago, E.; Farías, R.; Reyes-Esqueda, A. Effect of size and composition on the second harmonic generation from lithium niobate powders at different excitation wavelengths. Mater. Res. Express 2017, 4, 035022. [Google Scholar] [CrossRef]
- Sánchez-Dena, O.; Behel, Z.; Salmon, E.; Benichou, E.; Reyes-Esqueda, J.-A.; Brevet, P.-F.; Jonin, C. Polarization-resolved second harmonic generation from LiNbO3 powders. Opt. Mater. 2020, 107, 110169. [Google Scholar] [CrossRef]
- Aramburu, I.; Ortega, J.; Folcia, C.L.; Etxebarria, J. Second harmonic generation by micropowders: A revison of the Kurtz-Perry method and its practical application. Appl. Phys. B 2004, 116, 211–233. [Google Scholar] [CrossRef]
- Fierro-Ruíz, C.D.; Sánchez-Dena, O.; Cabral-Larquier, E.M.; Galindo, J.T.E.; Farías, R. Structural and Magnetic Behavior of Oxidized and Reduced Fe Doped LiNbO3 Powders. Crystals 2018, 8, 108. [Google Scholar] [CrossRef] [Green Version]
- Skipetrov, S.E. Disorder is the new order. Nat. Cell Biol. 2004, 432, 285–286. [Google Scholar] [CrossRef]
- Kudinova, M.; Humbert, G.; Auguste, J.-L.; Delaizir, G. Multimaterial polarization maintaining optical fibers fabricated with the powder-in-tube technology. Opt. Mater. Express 2017, 7, 3780–3790. [Google Scholar] [CrossRef]
- Nath, R.K.; Zain, M.F.M.; Kadhum, A.A.H. Artificial Photosynthesis using LiNbO3 as Photocatalyst for Sustainable and Environmental Friendly Construction and Reduction of Global Warming: A Review. Catal. Rev. 2013, 56, 175–186. [Google Scholar] [CrossRef]
- Yang, W.-C.; Rodriguez, B.J.; Gruverman, A.; Nemanich, R.J. Polarization-dependent electron affinity of LiNbO3 surfaces. Appl. Phys. Lett. 2004, 85, 2316–2318. [Google Scholar] [CrossRef] [Green Version]
- Gutmann, E.; Benke, A.; Gerth, K.; Böttcher, H.; Mehner, E.; Klein, C.; Krause-Buchholz, U.; Bergmann, U.; Pompe, W.; Meyer, D.C. Pyroelectrocatalytic Disinfection Using the Pyroelectric Effect of Nano- and Microcrystalline LiNbO3 and LiTaO3 Particles. J. Phys. Chem. C 2012, 116, 5383–5393. [Google Scholar] [CrossRef] [Green Version]
- Bretos, I.; Lourdes-Calzada, M. Chapter 5. Approaches Towards the Minimisation of Toxicity in Chemical Solution Deposition Processes of Lead-Based Ferroelectric Thin Films. In Multifunctional Polycrystalline Ferroelectric Materials: Processing and Properties, 1st ed.; Springer Series in Materials Science 140; Hull, R., Osgood, R.M., Jr., Parisi, J., Warlimont, H., Pardo, L., Ricote, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; Volume 140, pp. 145–216. [Google Scholar]
- Galassi, C. Chapter 1. Advances in Processing of Bulk Ferroelectric Materials. In Multifunctional Polycrystalline Ferroelectric Materials: Processing and Properties, 1st ed.; Springer Series in Materials Science 140; Hull, R., Osgood, R.M., Jr., Parisi, J., Warlimont, H., Pardo, L., Ricote, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; Volume 140, pp. 145–216. [Google Scholar]
- Saito, Y.; Takao, H.; Tani, T.; Nonoyama, T.; Takatori, K.; Homma, T.; Nagaya, T.; Nakamura, M. Lead-free piezoceramics. Nature 2004, 432, 84–87. [Google Scholar] [CrossRef]
- Shrout, T.R.; Zhang, S.J. Lead-free piezoelectric ceramics: Alternatives for PZT? J. Electroceramics 2007, 19, 113–126. [Google Scholar] [CrossRef]
- Troiler-McKinstry, S. Impact of ferroelectricity. Am. Cer. Soc. Bull. 2020, 99, 22–23. [Google Scholar]
- Reznichenko, L.A.; Verbenko, I.A.; Shilkina, L.A.; Pavlenko, A.V.; Dudkina, S.I.; Andryushina, I.N.; Andryushin, K.P.; Abubakarov, A.G.; Krasnyakova, T.V. Chapter 1. Binary, Ternary and Four-Component Systems Based on Sodium Niobate: Phase Diagrams of States, the Role of the Number of Components and Defectiveness in the Formation of the Properties. In Springer Proceedings in Physics 207: Advanced Materials: Proceedings of the International Conference on “Physics and Mechanics of New Materials and Their Applications”, PHENMA 2017, 1st ed.; Parinov, I.A., Chang, S.H., Gupta, V.K., Eds.; Springer: Cham, Switzerland, 2018; Volume 207, pp. 3–23. Available online: https://link.springer.com/chapter/10.1007/978-3-319-78919-4_1 (accessed on 31 July 2020).
- Kalinnikov, V.T.; Gromov, O.G.; Kunshina, G.B.; Kuz’Min, A.P.; Lokshin, E.P.; Ivanenko, V.I. Preparation of LiTaO3, LiNbO3, and NaNbO3 from Peroxide Solutions. Inorg. Mater. 2004, 40, 411–414. [Google Scholar] [CrossRef]
- Liu, M.; Xue, D. An efficient approach for the direct synthesis of lithium niobate powders. Solid State Ionics 2006, 177, 275–280. [Google Scholar] [CrossRef]
- Liu, M.; Xue, D.; Luo, C. Wet chemical synthesis of pure LiNbO3 powders from simple niobium oxide Nb2O5. J. Alloy. Compd. 2006, 426, 118–122. [Google Scholar] [CrossRef]
- Suryanarayana, C.; An, I.-S. Mechanical alloying and milling. Prog. Mater. Sci. 2001, 46, 1–184. [Google Scholar] [CrossRef]
- Kong, L.B.; Chang, T.S.; Ma, J.; Boey, F. Progress in synthesis of ferroelectric ceramic materials via high-energy mechanochemical technique. Prog. Mater. Sci. 2008, 53, 207–322. [Google Scholar] [CrossRef]
- Chadwick, A.V.; Pooley, M.J.; Savin, S.L.P. Lithium ion transport and microstructure in nanocrystalline lithium niobate. Phys. Status Solidi 2005, 2, 302–305. [Google Scholar] [CrossRef]
- Heitjans, P.; Masoud, M.; Feldhoff, A.; Wilkening, M. NMR and impedance studies of nanocrystalline and amorphous ion conductors: Lithium niobate as a model system. Faraday Discuss. 2007, 134, 67–82. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sugak, D.-Y.; Syvorotka, I.I.; Buryy, O.A.; Yakhnevych, U.V.; Solskii, I.M.; Martynyuk, N.V.; Suhak, Y.; Suchocki, A.; Zhydachevskii, Y.; Jakiela, R.; et al. Spatial distribution of optical coloration in single crystalline LiNbO3 after high-temperature H2/air treatments. Opt. Mater. 2017, 70, 106–115. [Google Scholar] [CrossRef]
- De Figueiredo, R.S.; Messai, A.; Hernandes, A.C.; Sombra, A.S.B. Piezoelectric lithium niobate obtained by mechanical alloying. J. Mater. Sci. Lett. 1998, 17, 449–451. [Google Scholar] [CrossRef]
- Pooley, M.J.; Chadwick, A.V. The Synthesis and Characterisation of Nanocrystalline Lithium Niobate. Radiat. Eff. Defects Solids 2003, 158, 197–201. [Google Scholar] [CrossRef]
- Diaz-Moreno, C.A.; Farías, R.; Galindo, J.T.E.; González, J.; Hurtado-Macias, A.; Bahena, D.; José-Yacamán, M.; Ramos, M. Structural Aspects LiNbO3 Nanoparticles and Their Ferromagnetic Properties. Materials 2014, 7, 7217–7225. [Google Scholar] [CrossRef] [Green Version]
- Luo, J.H. Preparation of Lithium Niobate Powders by Mechanochemical Process. Appl. Mech. Mater. 2011, 121–126, 3401–3405. [Google Scholar] [CrossRef]
- Takacs, L. Multiple combustion induced by ball milling. Appl. Phys. Lett. 1996, 69, 436–438. [Google Scholar] [CrossRef]
- Xue, J.; Wan, D.; Lee, S.-E.; Wang, J. Mechanochemical Synthesis of Lead Zirconate Titanate from Mixed Oxides. J. Am. Ceram. Soc. 1999, 82, 1687–1692. [Google Scholar] [CrossRef]
- Pitcher, M.W.; He, Y.; Bianconi, P.A. Facile in situ synthesis of oriented LiNbO3 single crystals in a polymer matrix. Mater. Chem. Phys. 2005, 90, 57–61. [Google Scholar] [CrossRef]
- Zhenxiang, C.; Kiyoshi, O.; Akimitsu, M.; Hideo, K. Formation of Niobates from Aqueous Peroxide Solution. Chem. Lett. 2004, 33, 1620–1621. [Google Scholar]
- Zeng, H.C.; Tung, S.K. Synthesis of Lithium Niobate Gels Using a Metal Alkoxide−Metal Nitrate Precursor. Chem. Mater. 1996, 8, 2667–2672. [Google Scholar] [CrossRef]
- Camargo, E.R. Low temperature synthesis of lithium niobate powders based on water-soluble niobium malato complexes. Solid State Ionics 2002, 151, 413–418. [Google Scholar] [CrossRef]
- Niederberger, M.; Pinna, N.; Polleux, J.; Antonietti, M. A General Soft-Chemistry Route to Perovskites and Related Materials: Synthesis of BaTiO3, BaZrO3, and LiNbO3 Nanoparticles. Angew. Chem. Int. Ed. 2004, 43, 2270–2273. [Google Scholar] [CrossRef] [PubMed]
- An, C.; Tang, K.; Wang, C.; Shen, G.; Jin, Y.; Qian, Y. Characterization of LiNbO3 nanocrystals prepared via a convenient hydrothermal route. Mater. Res. Bull. 2002, 37, 1791–1796. [Google Scholar] [CrossRef]
- Yoshikawa, Y.; Tsuzuki, K. Fabrication of Transparent Lead Lanthanum Zirconate Titanate Ceramics from Fine Powders by Two-Stage Sintering. J. Am. Ceram. Soc. 1992, 75, 2520–2528. [Google Scholar] [CrossRef]
- Oren, E.E.; Taspinar, E.; Tas, A.C. Preparation of Lead Zirconate by Homogeneous Precipitation and Calcination. J. Am. Ceram. Soc. 1997, 80, 2714–2716. [Google Scholar] [CrossRef]
- Camargo, E.R.; Frantti, J.; Kakihana, M. Low-temperature chemical synthesis of lead zirconate titanate (PZT) powders free from halides and organics. J. Mater. Chem. 2001, 11, 1875–1879. [Google Scholar] [CrossRef]
- Blum, J.B.; Gurkovich, S.R. Sol-gel-derived PbTiO3. J. Mater. Sci. 1985, 20, 4479–4483. [Google Scholar] [CrossRef]
- Kim, S.; Jun, M.-C.; Hwang, S.-C. Preparation of Undoped Lead Titanate Ceramics via Sol-Gel Processing. J. Am. Ceram. Soc. 2004, 82, 289–296. [Google Scholar] [CrossRef]
- Tartaj, J.; Moure, C.; Lascano, L.; Duran, P. Sintering of dense ceramics bodies of pure lead titanate obtained by seeding-assisted chemical sol-gel. Mater. Res. Bull. 2001, 36, 2301–2310. [Google Scholar] [CrossRef]
- Santos, I.; Loureiro, L.; Silva, M.; Cavaleiro, A.M.V. Studies on the hydrothermal synthesis of niobium oxides. Polyhedron 2002, 21, 2009–2015. [Google Scholar] [CrossRef]
- Sato, S.; Murakata, T.; Yanagi, H.; Miyasaka, F.; Iwaya, S. Hydrothermal synthesis of fine perovskite PbTiO3 powders with a simple mode of size distribution. J. Mater. Sci. 1994, 29, 5657–5663. [Google Scholar] [CrossRef]
- Peterson, C.R.; Slamovich, E.B. Effect of Processing Parameters on the Morphology of Hydrothermally Derived PbTiO3Powders. J. Am. Ceram. Soc. 1999, 82, 1702–1710. [Google Scholar] [CrossRef]
- Chen, D.-R.; Jiao, X.-L.; Xu, R.-R. Hydrothermal synthesis of PbZrxTi1−xO3 (x = 0.45--0.65) powders without using alkaline mineralizer. J. Mater. Sci. Lett. 1998, 17, 53–56. [Google Scholar] [CrossRef]
- Narendar, Y.; Messing, G.L. Kinetic Analysis of Combustion Synthesis of Lead Magnesium Niobate from Metal Carboxylate Gels. J. Am. Ceram. Soc. 2005, 80, 915–924. [Google Scholar] [CrossRef]
- Arendt, R.; Rosolowski, J.; Szymaszek, J. Lead zirconate titanate ceramics from molten salt solvent synthesized powders. Mater. Res. Bull. 1979, 14, 703–709. [Google Scholar] [CrossRef]
- Chiu, C.C.; Li, C.C.; Desu, S.B. Molten Salt Synthesis of a Complex Perovskite, Pb(Fe0.5Nb0.5)O3. J. Am. Ceram. Soc. 1991, 74, 38–41. [Google Scholar] [CrossRef]
- Kuz’Minov, Y.S.; Osiko, V.V. Nonstoichiometric composition of lithium niobate crystal. Ferroelectrics 1993, 142, 105–113. [Google Scholar] [CrossRef]
- Vyalikh, A.; Zschornak, M.; Köhler, T.; Nentwich, M.; Weigel, T.; Hanzig, J.; Zaripov, R.; Vavilova, E.; Gemming, S.; Brendler, E.; et al. Analysis of the defect clusters in congruent lithium tantalate. Phys. Rev. Mater. 2018, 2, 013804. [Google Scholar] [CrossRef] [Green Version]
- Spaldin, N.A. Analogies and Differences between Ferroelectrics and Ferromagnets. In Physics of Ferroelectrics: A Modern Perspective, 1st ed.; Rabe, K.M., Ahn, C.H., Triscone, J.-M., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 175–218. [Google Scholar]
- Toyoura, K.; Ohta, M.; Nakamura, A.; Matsunaga, K. First-principles study on phase transition and ferroelectricity in lithium niobate and tantalate. J. Appl. Phys. 2015, 118, 064103. [Google Scholar] [CrossRef]
- Sirenko, A.A.; Bernhard, C.; Golnik, A.; Clark, A.M.; Hao, J.; Si, W.; Xi, X.X. Soft-mode hardening in SrTiO3 thin films. Nature 2000, 404, 373–376. [Google Scholar] [CrossRef] [PubMed]
- Gehring, P.M.; Wakimoto, S.; Ye, Z.-G.; Shirane, G. Soft Mode Dynamics above and below the Burns Temperature in the Relaxor Pb(Mg1/3Nb2/3)O3. Phys. Rev. Lett. 2001, 87, 277601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cohen, R.E. Origin of ferroelectricity in perovskite oxides. Nature 1992, 358, 136–138. [Google Scholar] [CrossRef]
- Opik, U.; Pryce, M.H.L. Studies of the Jahn-Teller effect. I. A survey of the static problem. Proc. R. Soc. Lond. Ser. A, Math. Phys. Sci. 1957, 238, 425–447. [Google Scholar] [CrossRef]
- Halasyamani, P.S.; Poeppelmeier, K.R. Noncentrosymmetric Oxides. Chem. Mater. 1998, 10, 2753–2769. [Google Scholar] [CrossRef]
- Bersuker, I.B. The Jahn–Teller Effect; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Bersuker, I.B. Modern Aspects of the Jahn−Teller Effect Theory and Applications to Molecular Problems. Chem. Rev. 2001, 101, 1067–1114. [Google Scholar] [CrossRef]
- Burdett, J.K. Use of the Jahn-Teller theorem in inorganic chemistry. Inorg. Chem. 1981, 20, 1959–1962. [Google Scholar] [CrossRef]
- Bersuker, I.B. The Jahn-Teller Effect and Vibronic Interactions in Modern Chemistry; Springer: New York, NY, USA, 1984. [Google Scholar]
- Atanasov, M.; Reinen, D. Density Functional Studies on the Lone Pair Effect of the Trivalent Group (V) Elements: I. Electronic Structure, Vibronic Coupling, and Chemical Criteria for the Occurrence of Lone Pair Distortions in AX3 Molecules (A = N to Bi; X = H, and F to I). J. Phys. Chem. A 2001, 105, 5450–5467. [Google Scholar] [CrossRef]
- Troiler-McKinstry, S. Chapter 3. Crystal Chemistry of Piezoelectric Materials. In Piezoelectric and Acoustic Materials for Transducer Applications, 1st ed.; Safari, A., Koray Akdogan, E., Eds.; Springer Science+Business Media, LLC: New York, NY, USA, 2008; pp. 39–56. [Google Scholar]
- Ringwood, A.E. Mineralogical constitution of the deep mantle. J. Geophys. Res. Space Phys. 1962, 67, 4005–4010. [Google Scholar] [CrossRef]
- Mitchell, R.H. Perovskites Modern and Ancient; Almaz Press: Ontario, CA, USA, 2002. [Google Scholar]
- Extance, A. The reality behind solar power’s next star material. Nature 2019, 570, 429–432. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Snaith, H.J. A decade of perovskite photovoltaics. Nat. Energy 2019, 4, 1. Available online: https://ui.adsabs.harvard.edu/abs/2019NatEn...4....1./abstract (accessed on 31 July 2020). [CrossRef] [Green Version]
| Property | CG | nST |
|---|---|---|
| OPTICAL | ||
| Transparency region (nm) | 320–5000 | 300–5000 |
| Refractive index @ 633 nm (no, ne) | (2.286, 2.203) | (2.288, 2.190) |
| Electro-optical coefficient @ 633 nm | ||
| , , (pm V−1) | 32, 10, 6.8 | 38, 10.4, --- |
| , , (pm V−1) | 31, 8.6, 3.4 | ---, ---, 4.5 |
| Nonlinear optical coefficient @ 1060 nm | ||
| d33, d31, d22 (pm V−1) | 34, 6, 3 | 42, 5, 2.5 |
| NON-OPTICAL | ||
| Crystal structure | Trigonal | |
| Space and point group (RT, hex) | R3c, 3m | |
| Lattice constant aH (pm) | 515.0 | 514.7 |
| Lattice constant cH (pm) | 1386.4 | 1385.6 |
| Melting point (°C) | 1255 | <1200 |
| Curie temperature (°C) | 1140 | 1206 |
| Density (g cm−1) | 4.647 | 4.635 |
| Thermal expansion @ 300 K | ||
| αa 10−6 (K−1) | 14.1 | 14.1 |
| αc 10−6 (K−1) | 4.1 | 6.0 |
| Specific heat at RT (kJ kg−1 K−1) | 0.628 | 0.651 |
| Thermal conductivity at RT (W m−1 K−1) | 3.92 | 5.97 |
| Spontaneous polarization (µC cm−2) | 71 | 62 |
| Dielectric constant | ||
| , , | 84, 30, 44, 29 | 54, 42, 42, 41 |
| Method | Measured Parameter | Equation; Accuracy (mol %) | References |
|---|---|---|---|
| OPTICAL | |||
| Fundamental UV optical absorption | fundamental absorption edge | nonlinear; 0.02 | [51,52] |
| Polarized Raman spectroscopy | linewidth of Raman modes | linear; 0.05 | [47,48,49] |
| Unpolarized infrared spectroscopy | intensity ratio I3480/I3465 of peaks located at the wavenumbers in subscripts | linear; 0.01 | [53,54] |
| Sellmeier equation | refractive index (extraordinary) | nonlinear; --- | [55] |
| Dispersion of birefringence | refractive index (ordinary and extraordinary) | linear; <0.01 | [47,48,49] |
| Phase matching T for second harmonic generation (SHG) | phase matching T for SHG | linear; <0.01 | [39,56,57,58,59] |
| spontaneous noncolinear frequency doubling | cone angle | nonlinear; --- | [60] |
| Holographic scattering (photorefractive effect) | reading and writing angles with respect to the normal of the crystal surface | ---; --- | [61] |
| NON-OPTICAL | |||
| Melt composition | Li2O content of the melt | nonlinear; 0.3 | [46,47,62] |
| Differential thermal analysis | Curie temperature | nonlinear/linear; 0.1 | [39]/[43] |
| X-ray and neutron diffraction + structure refinement | cell volume | linear; 0.3 | [45,63] |
| Density measurements | Density | ---; --- | [45,64,65,66] |
| Nuclear magnetic resonance (NMR) and electron paramagnetic resonance (EPR) | linewidth of NMR and EPR signals | linear for Fe doping concentrations smaller than 0.01 mol %; --- | [49,67,68] |
| Velocity of surface acoustic waves (SAW) | velocity of SAW | ---; 0.01 | [69,70] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sánchez-Dena, O.; Fierro-Ruiz, C.D.; Villalobos-Mendoza, S.D.; Carrillo Flores, D.M.; Elizalde-Galindo, J.T.; Farías, R. Lithium Niobate Single Crystals and Powders Reviewed—Part I. Crystals 2020, 10, 973. https://doi.org/10.3390/cryst10110973
Sánchez-Dena O, Fierro-Ruiz CD, Villalobos-Mendoza SD, Carrillo Flores DM, Elizalde-Galindo JT, Farías R. Lithium Niobate Single Crystals and Powders Reviewed—Part I. Crystals. 2020; 10(11):973. https://doi.org/10.3390/cryst10110973
Chicago/Turabian StyleSánchez-Dena, Oswaldo, Cesar David Fierro-Ruiz, Sergio David Villalobos-Mendoza, Diana María Carrillo Flores, José Trinidad Elizalde-Galindo, and Rurik Farías. 2020. "Lithium Niobate Single Crystals and Powders Reviewed—Part I" Crystals 10, no. 11: 973. https://doi.org/10.3390/cryst10110973
APA StyleSánchez-Dena, O., Fierro-Ruiz, C. D., Villalobos-Mendoza, S. D., Carrillo Flores, D. M., Elizalde-Galindo, J. T., & Farías, R. (2020). Lithium Niobate Single Crystals and Powders Reviewed—Part I. Crystals, 10(11), 973. https://doi.org/10.3390/cryst10110973







