Endogenously Emerging Gender Pay Gap in an Experimental Teamwork Setting
Abstract
1. Introduction
2. The Experimental Game
2.1. Stage 1: Team Choice Stage
2.2. Stage 2: Contribution Stage
2.3. Stage 3: Punishment Stage
3. Theoretical Considerations and Hypotheses
3.1. Nash Equilibrium with Myopic and Selfish Preferences
3.2. Socially Optimal Behavior
3.3. Hypotheses on Gender Differences
4. Experimental Design and Procedures
5. Results
5.1. First Period Behavior
5.1.1. Initial Team Choices
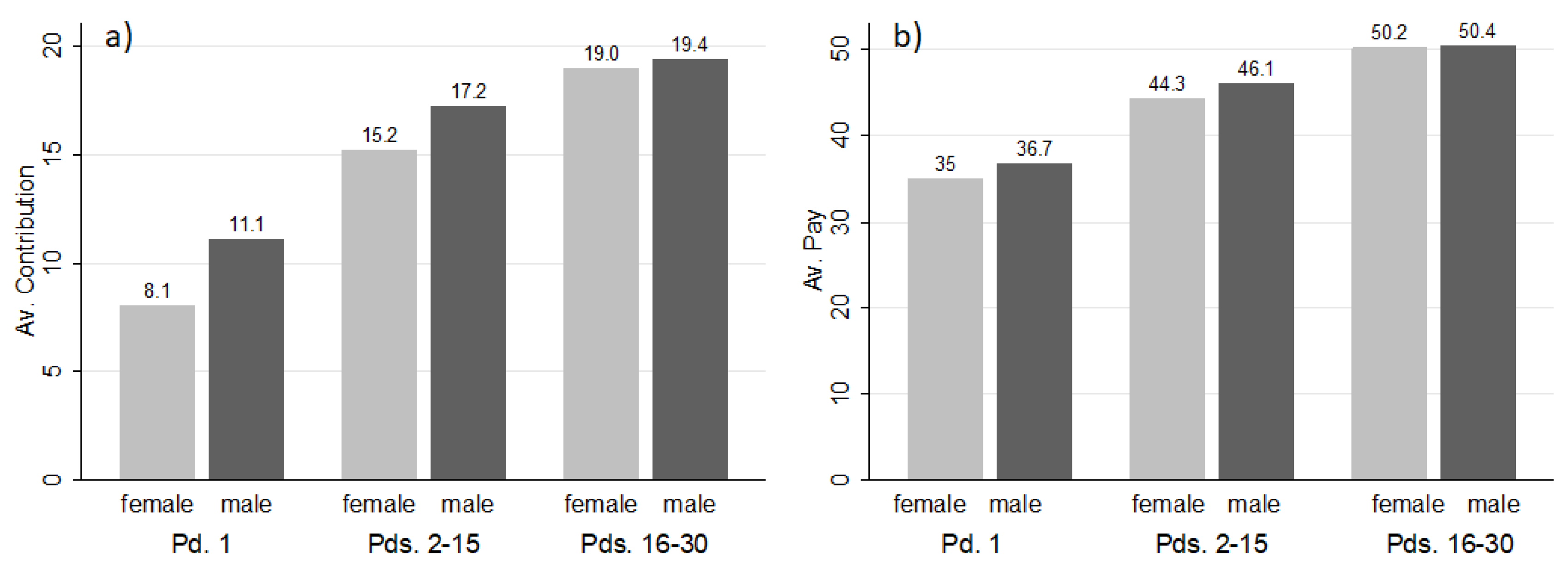
5.1.2. Initial Contributions, Punishment, and Pay
5.2. Behavior over Time
5.2.1. Team Choices over Time
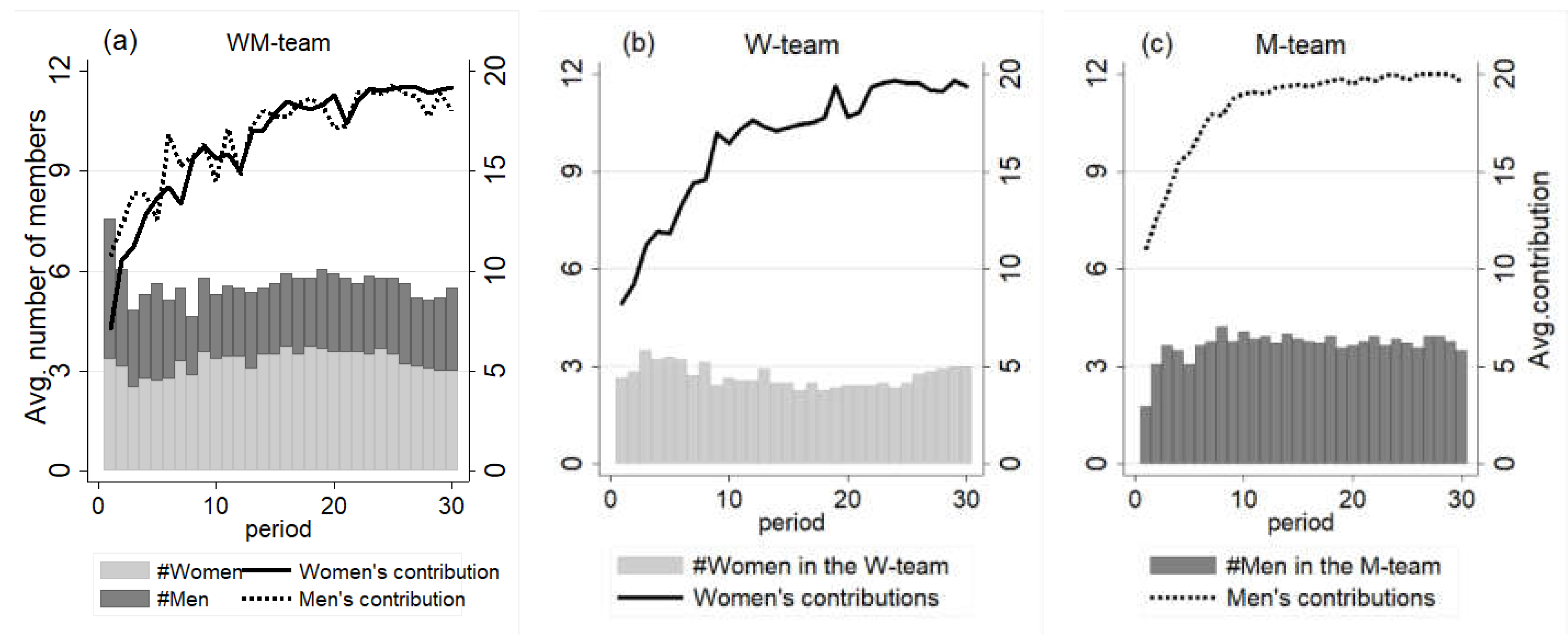
5.2.2. Contributions over Time
5.2.3. Punishment Behavior over Time
5.2.4. Pay
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| DV = Sent Punishment | (a) Tobit Regression Punisher = Man | (b) Tobit Regression Punisher = Woman |
|---|---|---|
| contribution difference btw. punisher & the punished | 0.593 (0.215) *** | −0.131 (0.111) |
| wm-team (dummy) | 0.331 (1.590) | −1.954 (0.828) ** |
| contribution difference btw. punisher and & the punished x wm-team | −0.239 (0.184) | 0.283 (0.137) ** |
| constant | −4.324 (2.277) * | 1.082 (0.698) |
| N | 228 | 162 |
| Std. err. adjusted for clusters | Yes | Yes |
| DV = Received Punishment | (a) Tobit Regression Punished = Man | (b) Tobit Regression Punished = Woman |
|---|---|---|
| other teammates’ average contributions | −0.438 (0.083) *** | −0.301 (0.073) *** |
| neg. contribution diff. between the subject and the teammates | 1.227 (0.106) *** | 1.269 (0.134) *** |
| pos. contribution diff. between the subject and the teammates | −0.147 (0.136) | −0.131 (0.132) |
| constant | 3.127 (1.245) ** | 0.158 (0.694) |
| N | 2029 | 1912 |
| Std. err. adjusted for clusters | Yes | Yes |

Appendix B
- G-Group: Women, as well as men, can enter this group.
- F-Group: Only women can enter this group.
- M-Group: Only men can enter this group.
- tokens you have kept = endowment − your contribution to the project
- earnings from the project = 1.6 × sum of the contributions of all group members/number of group members
| Your Contribution to the Project | The Sum of Others’ Contributions in Your Group | Number of Team Members in Your Group | Your Earnings from the Project | Your Earnings from Stage 1 | |
| Example 1 | 7 | 45 | 5 | 45 × 1.6/5 = 14.4 | 20 − 7 + 14.4 = 27.4 |
| Example 2 | 1 | 33 | 7 | 33 × 1.6/7 = 7.5 | 20 − 1 + 7.5 = 26.5 |
| Example 3 | 16 | 67 | 4 | 67 × 1.6/ 4= 26.8 | 20 − 16 + 26.8 = 30.8 |
- Each negative token you assign to a group member reduces her payoff by 3 tokens.
- If you assign 0 tokens to a group member, her/his payoff won’t change.

- tokens you have kept = 20 – sum of the tokens that you have assigned to the other group members
- less the threefold number of negative tokens you obtained from other group members
- Your payoff from Stage 1 20 – your contribution to the project + 1.6 × sum of the contributions of all group members/number of group members
- + Your payoff from Stage 2 20 – sum of the tokens you have assigned to other group members – 3 × (number of tokens you obtained from other group members)
- = Your round payoff

References
- What Works—Iris Bohnet | Harvard University Press. Available online: http://www.hup.harvard.edu/catalog.php?isbn=9780674089037 (accessed on 12 October 2018).
- Smale, A.; Miller, C.C. Germany Sets Gender Quota in Boardrooms. The New York Times. Available online: https://www.nytimes.com/2015/03/07/world/europe/german-law-requires-more-women-on-corporate-boards.html (accessed on 12 October 2018).
- Ahern, K.R.; Dittmar, A.K. The Changing of the Boards: The Impact on Firm Valuation of Mandated Female Board Representation. Q. J. Econ. 2012, 127, 137–197. [Google Scholar] [CrossRef]
- Hoogendoorn, S.; Oosterbeek, H.; van Praag, M. The Impact of Gender Diversity on the Performance of Business Teams: Evidence from a Field Experiment. Manag. Sci. 2013, 59, 1514–1528. [Google Scholar] [CrossRef]
- Azmat, G.; Petrongolo, B. Gender and the labor market: What have we learned from field and lab experiments? Labour Econ. 2014, 30, 32–40. [Google Scholar] [CrossRef]
- Chatman, J.A.; O’Reilly, C.A. Asymmetric Reactions to Work Group Sex Diversity among Men and Women. Acad. Manag. J. 2004, 47, 193–208. [Google Scholar] [CrossRef]
- Gürerk, Ö.; Irlenbusch, B.; Rockenbach, B. The Competitive Advantage of Sanctioning Institutions. Science 2006, 312, 108–111. [Google Scholar] [CrossRef] [PubMed]
- Gürerk, Ö.; Irlenbusch, B.; Rockenbach, B. On cooperation in open communities. J. Public Econ. 2014, 120, 220–230. [Google Scholar] [CrossRef]
- Croson, R.; Gneezy, U. Gender Differences in Preferences. J. Econ. Lit. 2009, 47, 448–474. [Google Scholar] [CrossRef]
- Balliet, D.; Li, N.P.; Macfarlan, S.J.; Van Vugt, M. Sex differences in cooperation: A meta-analytic review of social dilemmas. Psychol. Bull. 2011, 137, 881–909. [Google Scholar] [CrossRef] [PubMed]
- Women Dislike Competing against men_DIOGO GERALDES.pdf. Available online: https://drive.google.com/file/d/0B_nE1lM3ktKNdksyYU5pQzJBODQ/view?usp=drive_open&usp=embed_facebook (accessed on 12 October 2018).
- Dufwenberg, M.; Muren, A. Generosity, anonymity, gender. J. Econ. Behav. Organ. 2006, 61, 42–49. [Google Scholar] [CrossRef]
- Apesteguia, J.; Azmat, G.; Iriberri, N. The Impact of Gender Composition on Team Performance and Decision Making: Evidence from the Field. Manag. Sci. 2011, 58, 78–93. [Google Scholar] [CrossRef]
- Charness, G.; Rustichini, A. Gender differences in cooperation with group membership. Games Econ. Behav. 2011, 72, 77–85. [Google Scholar] [CrossRef]
- Nowell, C.; Tinkler, S. The influence of gender on the provision of a public good. J. Econ. Behav. Organ. 1994, 25, 25–36. [Google Scholar] [CrossRef]
- Brown-Kruse, J.; Hummels, D. Gender effects in laboratory public goods contribution: Do individuals put their money where their mouth is? J. Econ. Behav. Organ. 1993, 22, 255–267. [Google Scholar] [CrossRef]
- Mason, C.F.; Phillips, O.R.; Redington, D.B. The role of gender in a non-cooperative game. J. Econ. Behav. Organ. 1991, 15, 215–235. [Google Scholar] [CrossRef]
- Fischbacher, U. z-Tree: Zurich toolbox for ready-made economic experiments. Exp. Econ. 2007, 10, 171–178. [Google Scholar] [CrossRef]
- Greiner, B. Subject pool recruitment procedures: organizing experiments with ORSEE. J. Econ. Sci. Assoc. 2015, 1, 114–125. [Google Scholar] [CrossRef]
- Ortmann, A.; Tichy, L.K. Gender differences in the laboratory: Evidence from prisoner’s dilemma games. J. Econ. Behav. Organ. 1999, 39, 327–339. [Google Scholar] [CrossRef]
- Rapoport, A.; Chammah, A.M. Sex differences in factors contributing to the level of cooperation in the Prisoner’s Dilemma game. J. Pers. Soc. Psychol. 1965, 2, 831–838. [Google Scholar] [CrossRef]
- Halladay, B. Gender, Emotions, and Tournament Performance in the Laboratory. Games 2017, 8, 26. [Google Scholar] [CrossRef]
- Fehr, E.; Gächter, S. Cooperation and Punishment in Public Goods Experiments. Am. Econ. Rev. 2000, 90, 980–994. [Google Scholar] [CrossRef]
- Charness, G.; Villeval, M.-C. Cooperation and Competition in Intergenerational Experiments in the Field and the Laboratory. Am. Econ. Rev. 2009, 99, 956–978. [Google Scholar] [CrossRef]
- Bolton, G.E.; Katok, E. An experimental test for gender differences in beneficent behavior. Econ. Lett. 1995, 48, 287–292. [Google Scholar] [CrossRef]
- Eckel, C.C.; Grossman, P.J. Chapter 113 Men, Women and Risk Aversion: Experimental Evidence. In Handbook of Experimental Economics Results; Plott, C.R., Smith, V.L., Eds.; Elsevier: Amsterdam, The Netherlands, 2008; Volume 1, pp. 1061–1073. [Google Scholar]
- Gilligan, C. In a Different Voice; Harvard University Press: Cambridge, MA, USA, 1993; ISBN 978-0-674-44544-4. [Google Scholar]
- Simpson, B.; Van Vugt, M. Sex differences in cooperation: Integrating the evolutionary and social psychology literatures. Adv. Group Process. 2008, 26, 81–103. [Google Scholar]
| 1 | As reported in a New York Times article [2], in 2015, Germany passed a law that requires the 100 plus biggest companies of the country to give at least 30 percent of the seats in their corporate boards to women. |
| 2 | Endogenously formed teams have rarely been studied with regard to gender diversity. In this respect, we are only aware of an attitude survey conducted by Chatman and O’Reilly [6] who report that women express a greater likelihood of leaving homogeneous groups than do men in the clothing and retail industries. |
| 3 | |
| 4 | From previous experimental studies, we know that even in finitely repeated games players show a behavior that is more in line with predictions from an infinitely repeated game. Thus, the threats of no cooperation in the future, punishment, or leaving the team might lead to more cooperative behavior. There are, however, no apparent differences for our three teams concerning these patterns of behavior. |
| 5 | All tests in the result section report two-tailed significance levels. Because of the interdependence of the decisions, within and across teams, all following within and across team comparisons are tested with Wilcoxon matched-pairs test, using group (team) averages as independent observations. To save space, we abbreviate the Wilcoxon matched-pairs test to WMPT. |
| 6 | Average pay is the net payoff after the punishment stage, including the lump-sum payment, also including possible costs for allocating punishment points and deductions due received punishment from others. |
| 7 | This finding is in contrast to Ortmann and Tichy [20], who report that women cooperate significantly more than men do in the first round of a repeated, binary choice prisoner’s dilemma game in exogenously formed two-player teams. This difference disappears by the last period. |
| 8 | See Table A1 in the Appendix A. |
| 9 | This is in line with Rapoport and Chammah [21], who report that male-male interactions are more cooperative than female-female interactions. |
| 10 | Of all the possible groups, 8.9 percent consisted of only one subject in a given period. If there is only one subject in a group, then the single player’s endowment is added automatically to their private account. |
| 11 | Halladay [22] finds women increase performance when experiencing negative emotions, while male performance remains unaffected. |
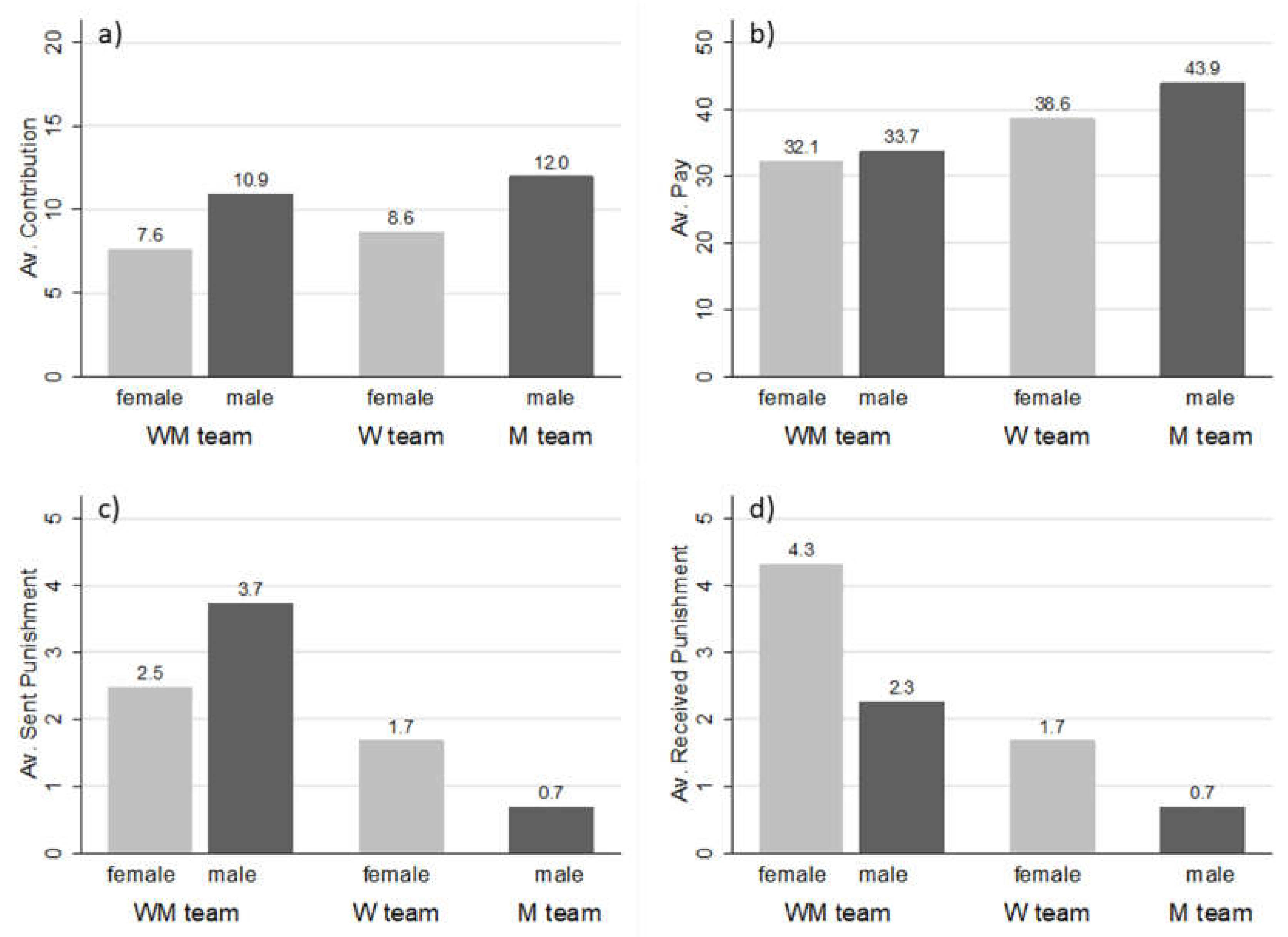
| 12 | For a visual comparison of average contributions, pay, sent punishment points, and received punishment points, averaged over all periods for each of the teams, respectively, see Figure A1 in the Appendix A. |
| 13 | If we control for the contribution difference between the punisher and the receiver, neither men nor women discriminate between genders when punishing. In other words, women do not punish other women more than men punish women. Men do not punish other men not more severely than women punish men. |
| 14 | The question of whether women or men contribute more in a public goods game provided inconclusive answers. While some studies find—as we do—men to be more cooperative than women (e.g., Brown-Kruse and Hummels [16]) others find the opposite (e.g., Charness and Villeval [24]) or report no differences (e.g., Bolton and Katok [25], Eckel and Grossman [26]). See Croson and Gneezy [9] for an extensive discussion on this issue. |
| (a) DV = Change Teams | (b) DV = Contribution | (c) DV = Received Punishment | (d) DV = Contribution Difference | |
|---|---|---|---|---|
| punished | 1.030 (0.100) *** | |||
| other teammates’ average contributions | −0.355 (0.077) *** | |||
| other team’s contributions higher | 0.210 (0.135) | |||
| man | −0.264 (0.124) ** | 1.102 (2.411) | −0.243 (0.347) | |
| punished x man | −0.102 (0.085) | |||
| other team’s average contributions higher x man | 0.341 (0.204) * | |||
| others’ average contributions in the own team | 1.272 (0.108) *** | |||
| others’ average contributions in the own team x man | −0.026 (0.137) | |||
| neg. contribution diff. between the subject and the teammates | 1.257 (0.098) *** | |||
| pos. contribution diff. between the subject and the teammates | −0.116 (0.897) | |||
| received punishment (previous period) | 0.321 (0.054) *** | |||
| received punishment (previous period) x man | 0.104 (0.146) | |||
| constant | −1.501 (0.136) *** | −0.592 (1.125) | 1.252 (0.931) | 0.576 (0.189) *** |
| N | 4763 | 4749 | 4928 | 931 |
| Std. err. adjusted for 14 groups | Yes | Yes | Yes | Yes |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gürerk, Ö.; Irlenbusch, B.; Rockenbach, B. Endogenously Emerging Gender Pay Gap in an Experimental Teamwork Setting. Games 2018, 9, 98. https://doi.org/10.3390/g9040098
Gürerk Ö, Irlenbusch B, Rockenbach B. Endogenously Emerging Gender Pay Gap in an Experimental Teamwork Setting. Games. 2018; 9(4):98. https://doi.org/10.3390/g9040098
Chicago/Turabian StyleGürerk, Özgür, Bernd Irlenbusch, and Bettina Rockenbach. 2018. "Endogenously Emerging Gender Pay Gap in an Experimental Teamwork Setting" Games 9, no. 4: 98. https://doi.org/10.3390/g9040098
APA StyleGürerk, Ö., Irlenbusch, B., & Rockenbach, B. (2018). Endogenously Emerging Gender Pay Gap in an Experimental Teamwork Setting. Games, 9(4), 98. https://doi.org/10.3390/g9040098





