How to Split Gains and Losses? Experimental Evidence of Dictator and Ultimatum Games
Abstract
:1. Introduction
2. Related Literature
3. Design, Procedure, and Descriptive Statistics
3.1. Experimental Procedure
3.2. Treatments (Gains vs. Losses)
3.2.1. Gains Treatments
3.2.2. Loss Treatments
3.3. Personality Traits and Socio-Demographics
4. Results
4.1. Analyses of Players’ Demands in Dictator and Ultimatum Games
4.2. Analyses of Personality Traits and Various Socio-Demographics
5. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Histograms
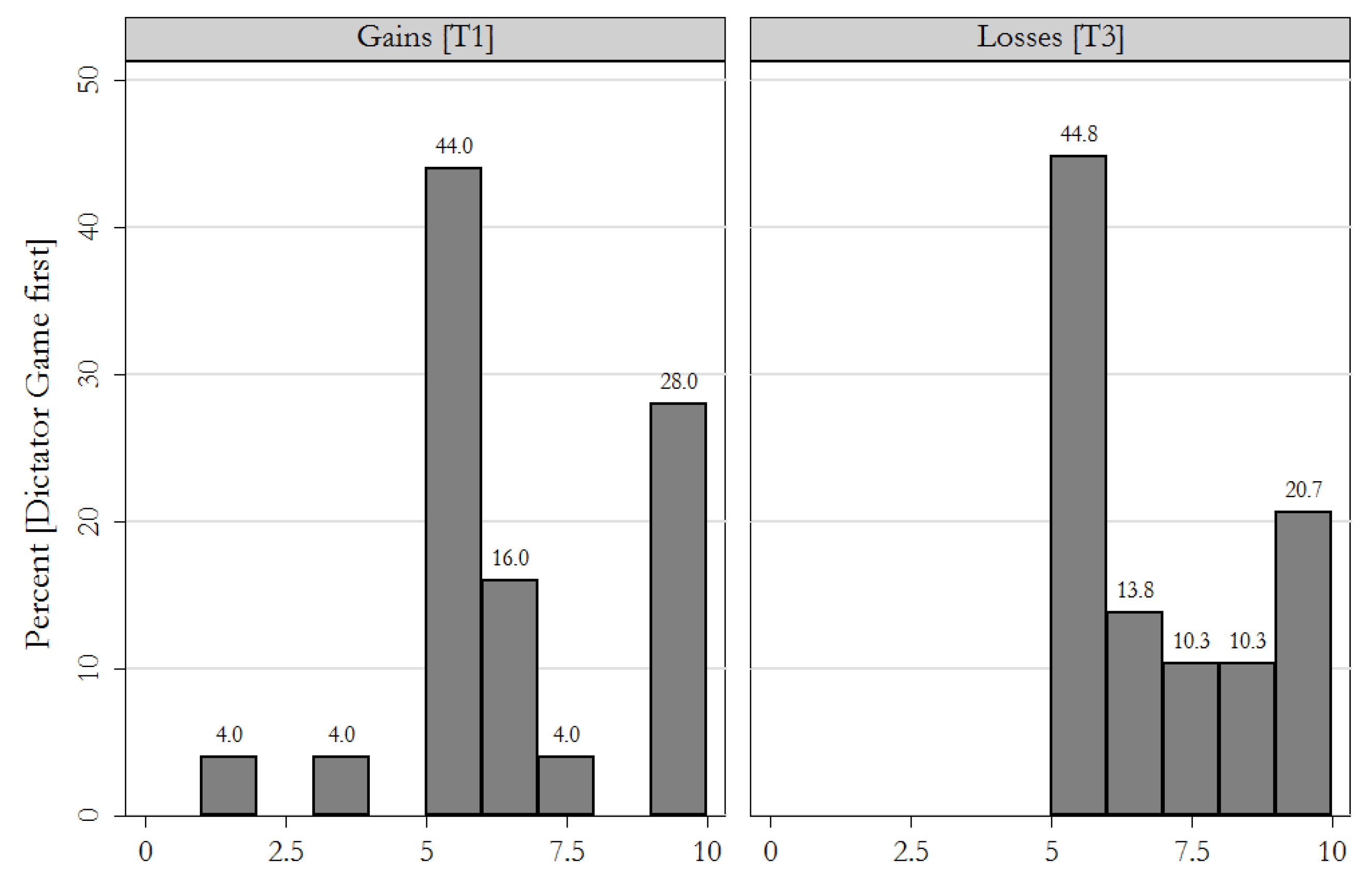
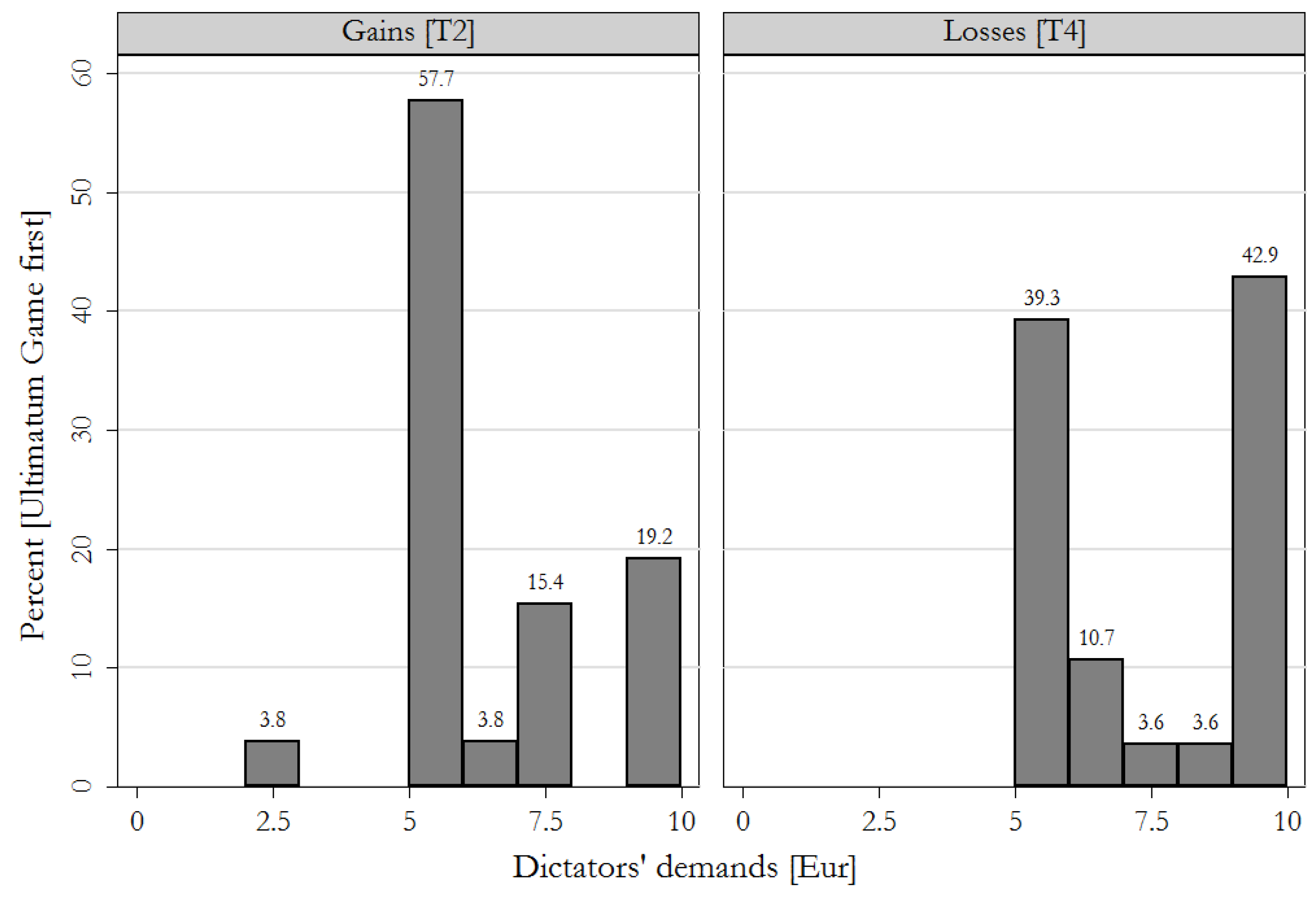

Appendix B. Experimental Instructions—First Sub-Session Loss Treatment
Questionnaire—Personality Traits (Big5)
Appendix C. Experimental Instructions—Second Sub-Session Loss Treatment
General instruction
Instruction Dictator Game
Game Description

Instruction Ultimatum Game
Game Description

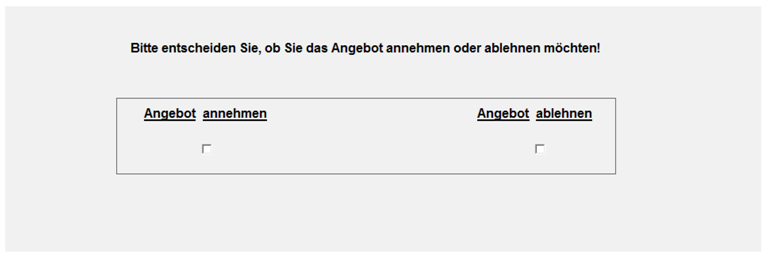
Appendix D. Experimental Instructions—Gains Treatment
General Instructions
Instructions for the Dictator Game
Game Description

Instructions for the Ultimatum Game
Game Description
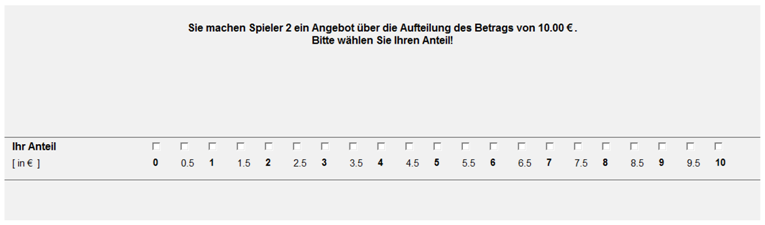
Questionnaire—Personal Traits (Big5)
References
- Blanco, M.; Engelmann, D.; Normann, H.T. A within-subject analysis of other-regarding preferences. Games Econ. Behav. 2011, 72, 321–338. [Google Scholar] [CrossRef] [Green Version]
- Zhao, K.; Kashima, Y.; Smillie, L. From windfall sharing to property ownership: Prosocial personality traits in giving and taking dictator games. Games 2018, 9, 30. [Google Scholar] [CrossRef]
- Kahneman, D.; Knetsch, J.L.; Thaler, R.H. Fairness and the assumptions of economics. J. Bus. 1986, 59, S285–S300. [Google Scholar] [CrossRef]
- Forsythe, R.; Horowitz, J.L.; Savin, N.E.; Sefton, M. Fairness in simple bargaining experiments. Games Econ. Behav. 1994, 6, 347–369. [Google Scholar] [CrossRef]
- Güth, W.; Schmittberger, R.; Schwarze, B. An experimental analysis of ultimatum bargaining. J. Econ. Behav. Organ. 1982, 3, 367–388. [Google Scholar] [CrossRef]
- Engel, C. Dictator games: A meta study. Exp. Econ. 2011, 14, 583–610. [Google Scholar] [CrossRef]
- Güth, W.; Kocher, M.G. More than thirty years of ultimatum bargaining experiments: Motives, variations, and a survey of the recent literature. J. Econ. Behav. Organ. 2014, 108, 396–409. [Google Scholar] [CrossRef] [Green Version]
- Rosenboim, M.; Shavit, T. Whose money is it anyway? Using prepaid incentives in experimental economics to create a natural environment. Exp. Econ. 2012, 15, 145–157. [Google Scholar] [CrossRef]
- Brandts, J.; Charness, G. The strategy versus the direct-response method: A first survey of experimental comparisons. Exp. Econ. 2011, 14, 375–398. [Google Scholar] [CrossRef] [Green Version]
- Neumann, T.; Schosser, S.; Vogt, B. Ultimatum bargaining over losses and gains—An experimental comparison. Soc. Sci. Res. 2017, 67, 49–58. [Google Scholar] [CrossRef] [PubMed]
- Güth, W.; Tietz, R. Ultimatum bargaining behavior: A survey and comparison of experimental results. J. Econ. Psychol. 1990, 11, 417–449. [Google Scholar] [CrossRef]
- Kahneman, D.; Tversky, A. Prospect Theory: An Analysis of Decision under Risk. Econometrica 1979, 47, 263. [Google Scholar] [CrossRef] [Green Version]
- Tversky, A.; Kahneman, D. Advances in prospect theory: Cumulative representation of uncertainty. J. Risk Uncertain. 1992, 5, 297–323. [Google Scholar] [CrossRef] [Green Version]
- List, J.A. On the Interpretation of Giving in Dictator Games. J. Polit. Econ. 2007, 115, 482–493. [Google Scholar] [CrossRef]
- Bardsley, N. Dictator game giving: Altruism or artefact? Exp. Econ. 2008, 11, 122–133. [Google Scholar] [CrossRef]
- Korenok, O.; Millner, E.L.; Razzolini, L. Taking, giving, and impure altruism in dictator games. Exp. Econ. 2014, 17, 488–500. [Google Scholar] [CrossRef]
- Korenok, O.; Millner, E.L.; Razzolini, L. Impure altruism in dictators’ giving. J. Public Econ. 2013, 97, 1–8. [Google Scholar] [CrossRef]
- Cox, J.; List, J.; Price, M.; Sadiraj, V.; Samek, A. Moral Costs and Rational Choice: Theory and Experimental Evidence; NBER Working Paper No. 22234. Cambridge, MA, USA, 2016. Available online: http://www.nber.org/papers/w22234.pdf (accessed on 28 June 2018).
- Camerer, C.F.; Johnson, E.J.; Rymon, T.; Sen, S. Cognition and framing in sequential bargaining for gains and losses. In Frontiers of Game Theory; Binmore, K.G., Kirman, A.P., Tani, P., Eds.; MIT Press: Cambridge, MA, USA, 1993; pp. 27–47. [Google Scholar]
- Buchan, N.; Croson, R.; Johnson, E.; Wu, G. Gain and loss ultimatums. In Experimental and Behavorial Economics (Advances in Applied Microeconomics); Morgan, J., Ed.; Emerald Group Publishing Limited: Bingley, UK, 2005; pp. 1–23. [Google Scholar]
- Lusk, J.L.; Hudson, M.D. Bargaining over losses. Int. Game Theory Rev. 2010, 12, 83–91. [Google Scholar] [CrossRef]
- Berger, R.; Rauhut, H.; Prade, S.; Helbing, D. Bargaining over waiting time in ultimatum game experiments. Soc. Sci. Res. 2012, 41, 372–379. [Google Scholar] [CrossRef] [PubMed]
- Kroll, E.B.; Morgenstern, R.; Neumann, T.; Schosser, S.; Vogt, B. Bargaining power does not matter when sharing losses—Experimental evidence of equal split in the Nash bargaining game. J. Econ. Behav. Organ. 2014, 108, 261–272. [Google Scholar] [CrossRef]
- Noussair, C.N.; Stoop, J. Time as a medium of reward in three social preference experiments. Exp. Econ. 2015, 18, 442–456. [Google Scholar] [CrossRef]
- Okada, E.M.; Hoch, S.J. Spending Time versus Spending Money. J. Consum. Res. 2004, 31, 313–323. [Google Scholar] [CrossRef] [Green Version]
- Ellingsen, T.; Johannesson, M. Time is not money. J. Econ. Behav. Organ. 2009, 72, 96–102. [Google Scholar] [CrossRef] [Green Version]
- Leclerc, F.; Schmitt, B.H.; Dube, L. Waiting Time and Decision Making: Is Time like Money? J. Consum. Res. 1995, 22, 110. [Google Scholar] [CrossRef]
- Schosser, S.; Trarbach, J.N.; Vogt, B. How does the perception of pain determine the selection between different treatments? J. Econ. Behav. Organ. 2016, 131, 174–182. [Google Scholar] [CrossRef]
- Lang, H.; DeAngelo, G.; Bongard, M. Theory of Mind and General Intelligence in Dictator and Ultimatum Games. Games 2018, 9, 16. [Google Scholar] [CrossRef]
- Ben-Ner, A.; Kong, F.; Putterman, L. Share and share alike? Gender-pairing, personality, and cognitive ability as determinants of giving. J. Econ. Psychol. 2004, 25, 581–589. [Google Scholar] [CrossRef]
- Gong, X.; Xia, L.-X.; Sun, Y.; Guo, L.; Carpenter, V.C.; Fang, Y.; Chen, Y. Proposal allocation ratio as a moderator of interpersonal responsibility effects on hostile decision-making in the ultimatum game. Front. Psychol. 2017, 8, 1959. [Google Scholar] [CrossRef] [PubMed]
- Hilbig, B.E.; Zettler, I.; Leist, F.; Heydasch, T. It takes two: Honesty–Humility and Agreeableness differentially predict active versus reactive cooperation. Pers. Individ. Dif. 2013, 54, 598–603. [Google Scholar] [CrossRef]
- Nguyen, C.M.; Koenigs, M.; Yamada, T.H.; Teo, S.H.; Cavanaugh, J.E.; Tranel, D.; Denburg, N.L. Trustworthiness and negative affect predict economic decision-making. J. Cogn. Psychol. 2011, 23, 748–759. [Google Scholar] [CrossRef] [PubMed]
- Neumann, T.; Schosser, S.; Vogt, B. Non-acceptance of Losses—An Experimental Study on the Importance of the Sign of Final Outcomes in Ultimatum Bargaining. In Operations Research Proceedings 2017: Selected Papers of the Annual International Conference of the German Operations Research Society (GOR), Freie Universität Berlin, Germany, 6–8 September 2017; Kliewer, N., Ehmke, J.F., Borndörfer, R., Eds.; Springer: Cham, Switzerland, 2018; pp. 287–292. [Google Scholar]
- Hennig-Schmidt, H.; Irlenbusch, B.; Rilke, R.M.; Walkowitz, G. Asymmetric outside options in ultimatum bargaining: A systematic analysis. Int. Game Theory Rev. 2018, 47, 301–329. [Google Scholar] [CrossRef]
- Rauhut, H.; Winter, F. A sociological perspective on measuring social norms by means of strategy method experiments. Soc. Sci. Res. 2010, 39, 1181–1194. [Google Scholar] [CrossRef] [Green Version]
- Baquero, G.; Smit, W.; Wathieu, L. The Generosity Effect: Fairness in Sharing Gains and Losses; ESMT-13-08. Berlin, Germany, 2013. Available online: https://www.econstor.eu/bitstream/10419/96540/1/ESMT-13-08.pdf (accessed on 22 September 2018).
- Bock, O.; Baetge, I.; Nicklisch, A. Hroot: Hamburg registration and organization online tool. Eur. Econ. Rev. 2014, 71, 117–120. [Google Scholar] [CrossRef]
- Fischbacher, U. z-Tree: Zurich toolbox for ready-made economic experiments. Exp. Econ. 2007, 10, 171–178. [Google Scholar] [CrossRef] [Green Version]
- Takezawa, M.; Gummerum, M.; Keller, M. A stage for the rational tail of the emotional dog: Roles of moral reasoning in group decision making. J. Econ. Psychol. 2006, 27, 117–139. [Google Scholar] [CrossRef]
- Rammstedt, B.; Kemper, C.J.; Klein, M.C.; Beierlein, C.; Kovaleva, A. A short scale for assessing the big five dimensions of personality: 10 Item Big Five Inventory (BFI-10). Methoden Daten Anal. 2013, 7, 233–249. [Google Scholar] [CrossRef]
- John, O.P.; Donahue, E.M.; Kentle, R.L. The Big Five Inventory—Versions 4a and 54; Berkeley, Institute of Personality and Social Research, University of California: Berkeley, CA, USA, 1991. [Google Scholar]
- Cohen, J. Statistical Power Analysis for the Behavioral Sciences, 2nd ed.; Taylor and Francis: Hoboken, NJ, USA, 2013. [Google Scholar]
- Fehr, E.; Schmidt, K.M. A Theory of Fairness, Competition, and Cooperation. Q. J. Econ. 1999, 114, 817–868. [Google Scholar] [CrossRef] [Green Version]
- Bolton, G.E.; Ockenfels, A. ERC: A Theory of Equity, Reciprocity, and Competition. Am. Econ. Rev. 2000, 90, 166–193. [Google Scholar] [CrossRef] [Green Version]
- Bolton, G.E.; Zwick, R. Anonymity versus Punishment in Ultimatum Bargaining. Games Econ. Behav. 1995, 10, 95–121. [Google Scholar] [CrossRef]
- Güth, W.; Huck, S.; Müller, W. The Relevance of Equal Splits in Ultimatum Games. Games Econ. Behav. 2001, 37, 161–169. [Google Scholar] [CrossRef]
| 1 | According to Cohen [43], the values used to classify effect sizes as “no effect”, “small”, “medium” and “large” are: (no effect), (small effect), (medium effect) and (large effect). |

| Payoffs | |||
|---|---|---|---|
| Dictator | Receiver | ||
| Domain | Gains | ||
| Loss | |||
| Payoffs, If Responder | |||||
|---|---|---|---|---|---|
| Accepts the Offer | Rejects the Offer | ||||
| Proposer | Responder | Proposer | Responder | ||
| Domain | Gains | ||||
| Loss | |||||
| Task Order | |||
|---|---|---|---|
| DG First | UG First | ||
| Domain | Gains | T1 | T2 |
| Loss | T3 | T4 | |
| No. of Dictators Who Demand | Classified As | T1 | T2 |
|---|---|---|---|
| (100%) | Egoist | 6 | 4 |
| (99%; 80%) | Rather egoistic | 1 | 1 |
| (79%; 70%) | (Empirical) Average | 1 | 4 |
| (69%; 51%) | Rather altruistic | 7 | 2 |
| (50%) | Equal split | 8 | 14 |
| (49%; 0%) | Altruist | 2 | 1 |
| Average demand (in EUR) | 6.44 | 6.23 | |
| Standard deviation (in EUR) | 2.46 | 2.10 | |
| No. of Proposers Who Demand | T1 | T2 |
|---|---|---|
| More than 50% | 11 | 7 |
| Exact 50% | 12 | 19 |
| Less than 50% | 1 | 0 |
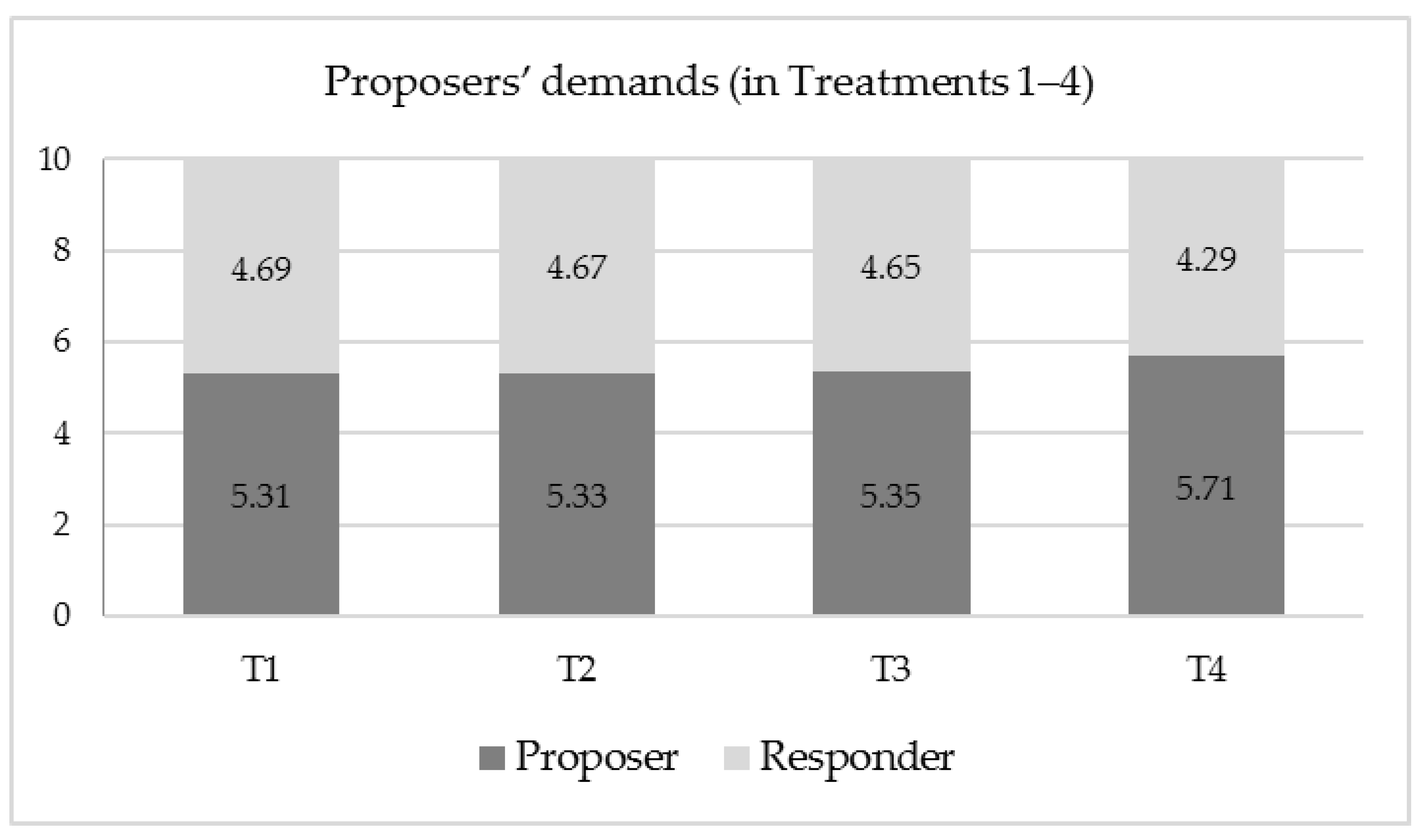
| Average demand (in EUR) | 5.31 | 5.33 |
| Standard deviation (in EUR) | 1.11 | 0.64 |
| No. of Dictators Who Demand | Classified As | T3 | T4 |
|---|---|---|---|
| (100%) | Egoist | 6 | 10 |
| (99%; 80%) | Rather egoistic | 3 | 3 |
| (79%; 70%) | (Empirical) Average | 3 | 1 |
| (69%; 51%) | Rather altruistic | 5 | 4 |
| (50%) | Equal split | 12 | 10 |
| (49%; 0%) | Altruist | 0 | 0 |
| Average demand | 6.72 | 7.40 | |
| Standard deviation | 1.98 | 2.28 | |
| No. of Proposers Who Demand | T3 | T4 |
|---|---|---|
| More than 50% | 8 | 8 |
| Exactly 50% | 18 | 20 |
| Less than 50% | 1 | 0 |
| Average demand | 5.35 | 5.71 |
| Standard deviation | 0.74 | 1.37 |
| Big Five Dimension | N | Mean | Standard Deviation | Min–Max (1–5) |
|---|---|---|---|---|
| Extraversion | 216 | 3.0879 | 0.9483 | 1–5 |
| Agreeableness | 216 | 3.0278 | 0.7228 | 1–4.5 |
| Conscientiousness | 216 | 3.3657 | 0.8869 | 1–5 |
| Neuroticism | 216 | 3.0671 | 0.8621 | 1–5 |
| Openness | 216 | 3.6898 | 0.9480 | 1–5 |
| Treatment 1: Dictator game first, ultimatum game second in the gains domain | ||||||
| Role | No. | Average earnings | Role | No. | Average earnings from game 1 | Average earnings from game 2 |
| Dictator | 24 | 6.44 | Proposer | 10 | 6.00 | 4.95 |
| Responder | 14 | 6.79 | 4.43 | |||
| Receiver | 24 | 3.56 | Proposer | 14 | 3.39 | 5.57 |
| Responder | 10 | 3.75 | 5.05 | |||
| Treatment 2: Ultimatum game first, dictator game second in the gains domain | ||||||
| Proposer | 26 | 5.33 | Dictator | 14 | 5.25 | 6.14 |
| Receiver | 12 | 5.42 | 3.63 | |||
| Responder | 26 | 4.67 | Dictator | 12 | 4.96 | 6.33 |
| Receiver | 14 | 4.43 | 3.89 | |||
| Treatment 3: Dictator game first, ultimatum game second in the loss domain | ||||||
| Dictator | 27 | 6.72 | Proposer | 14 | 6.43 | 5.39 |
| Responder | 13 | 7.31 | 4.69 | |||
| Receiver | 27 | 3.28 | Proposer | 13 | 5.31 | 3.23 |
| Responder | 14 | 4.61 | 3.03 | |||
| Treatment 4: Ultimatum game first, dictator game second in the loss domain | ||||||
| Proposer | 28 | 5.71 | Dictator | 14 | 5.79 | 7.18 |
| Receiver | 14 | 5.64 | 2.36 | |||
| Responder | 28 | 4.29 | Dictator | 14 | 4.18 | 7.68 |
| Receiver | 14 | 4.39 | 2.79 | |||
| Dictators’ Demands (T2 and T4) | Proposers’ Demands (T1 and T3) | |
|---|---|---|
| Variable | Model 1 | Model 2 |
| Treatment () | 8.6476 | −0.1373 |
| Prior experience (earnings from game 1) | 0.5126 ** | 0.2226 ** |
| Constant | 38.9779 ** | 31.3067 ** |
| 54 | 51 | |
| 0.1419 | 0.2797 |
| Dictators’ Demands | Proposers’ Demands | |||||
|---|---|---|---|---|---|---|
| Variable | Model 1 (T1 & T3) | Model 2 (T2 & T4) | Model 3 (Full Sample) | Model 4 (T1 & T3) | Model 5 (T2 & T4) | Model 6 (Full Sample) |
| Treatment (loss) | –2.4045 | 10.651 * | 4.0092 | –0.8623 | 2.8092 | 1.8081 |
| Female | –23.405 *** | 13.299 * | –6.3274 | –4.5162 | –3.3929 | –2.8007 |
| Openness | –7.2574 ** | –1.85 | –2.9452 | –0.8459 | 1.5705 | 0.4078 |
| Conscientiousness | –1.1840 | –0.0233 | 0. 6275 | 1.8452 | –1.1249 | 0.4678 |
| Extraversion | 2.5093 | –1.5724 | 1.1362 | 1.0018 | –1.2262 | –0.1293 |
| Agreeableness | –3.7358 | –11.727 *** | –9.3528 *** | 0.8639 | –5.183 *** | –2.721 ** |
| Neuroticism | 5.2635 | –3.8702 | 2.6916 | 2.3878 | –0.0557 | 1.3967 |
| Constant | 101.29 *** | 114.72 *** | 93.70 *** | 40.8 1*** | 73.28 *** | 56.15 *** |
| 54 | 54 | 108 | 51 | 54 | 105 | |
| 0.3681 | 0.2879 | 0.1535 | 0.0967 | 0.2292 | 0.0860 | |
| 0.2719 | 0.1796 | 0.0942 | –0.0503 | 0.1119 | 0.02 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Neumann, T.; Kierspel, S.; Windrich, I.; Berger, R.; Vogt, B. How to Split Gains and Losses? Experimental Evidence of Dictator and Ultimatum Games. Games 2018, 9, 78. https://doi.org/10.3390/g9040078
Neumann T, Kierspel S, Windrich I, Berger R, Vogt B. How to Split Gains and Losses? Experimental Evidence of Dictator and Ultimatum Games. Games. 2018; 9(4):78. https://doi.org/10.3390/g9040078
Chicago/Turabian StyleNeumann, Thomas, Sabrina Kierspel, Ivo Windrich, Roger Berger, and Bodo Vogt. 2018. "How to Split Gains and Losses? Experimental Evidence of Dictator and Ultimatum Games" Games 9, no. 4: 78. https://doi.org/10.3390/g9040078
APA StyleNeumann, T., Kierspel, S., Windrich, I., Berger, R., & Vogt, B. (2018). How to Split Gains and Losses? Experimental Evidence of Dictator and Ultimatum Games. Games, 9(4), 78. https://doi.org/10.3390/g9040078





