Contribution-Based Grouping under Noise
Abstract
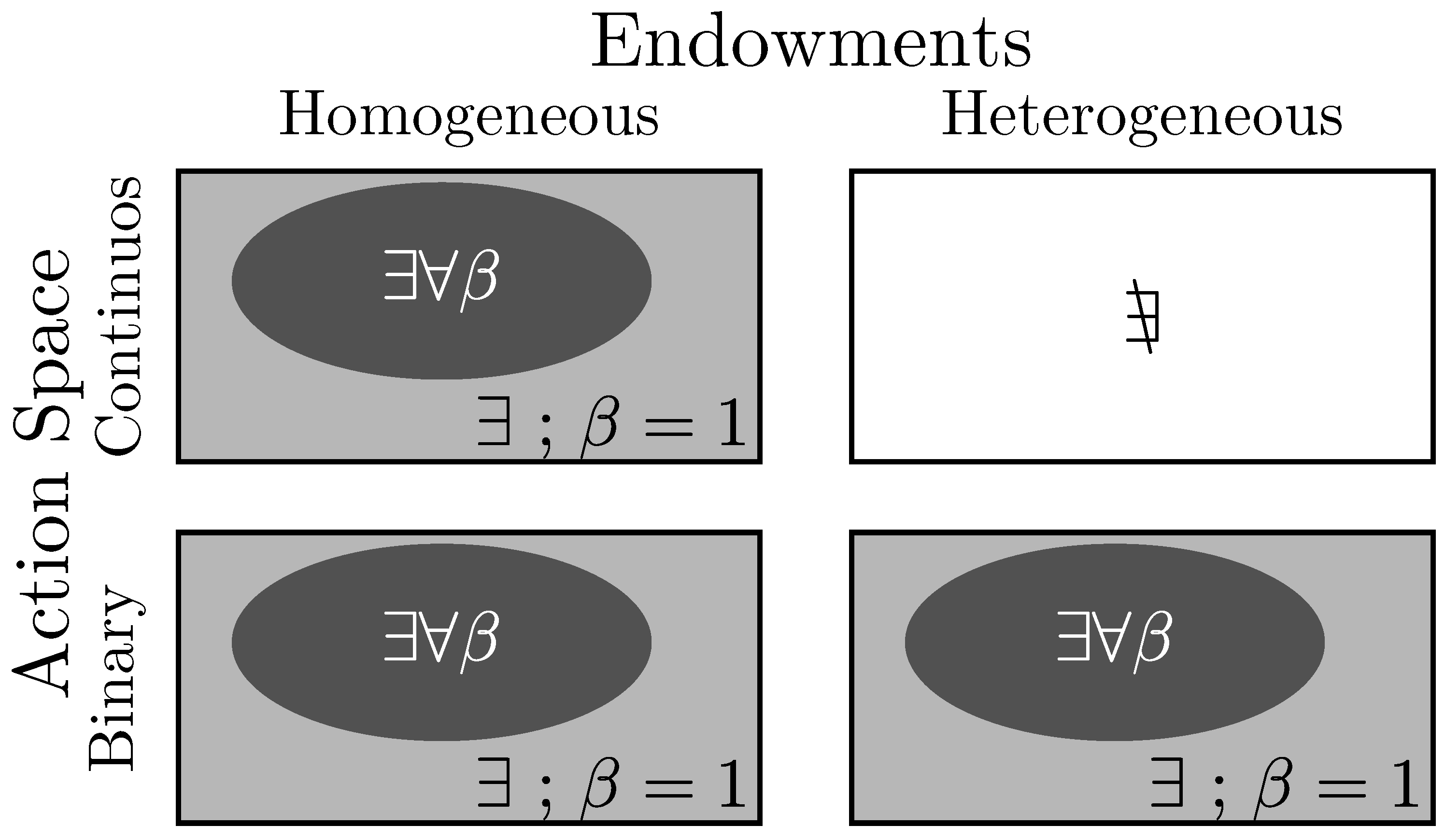
:1. Motivation
2. The Mechanism
2.1. The Model
3. Nash Equilibria
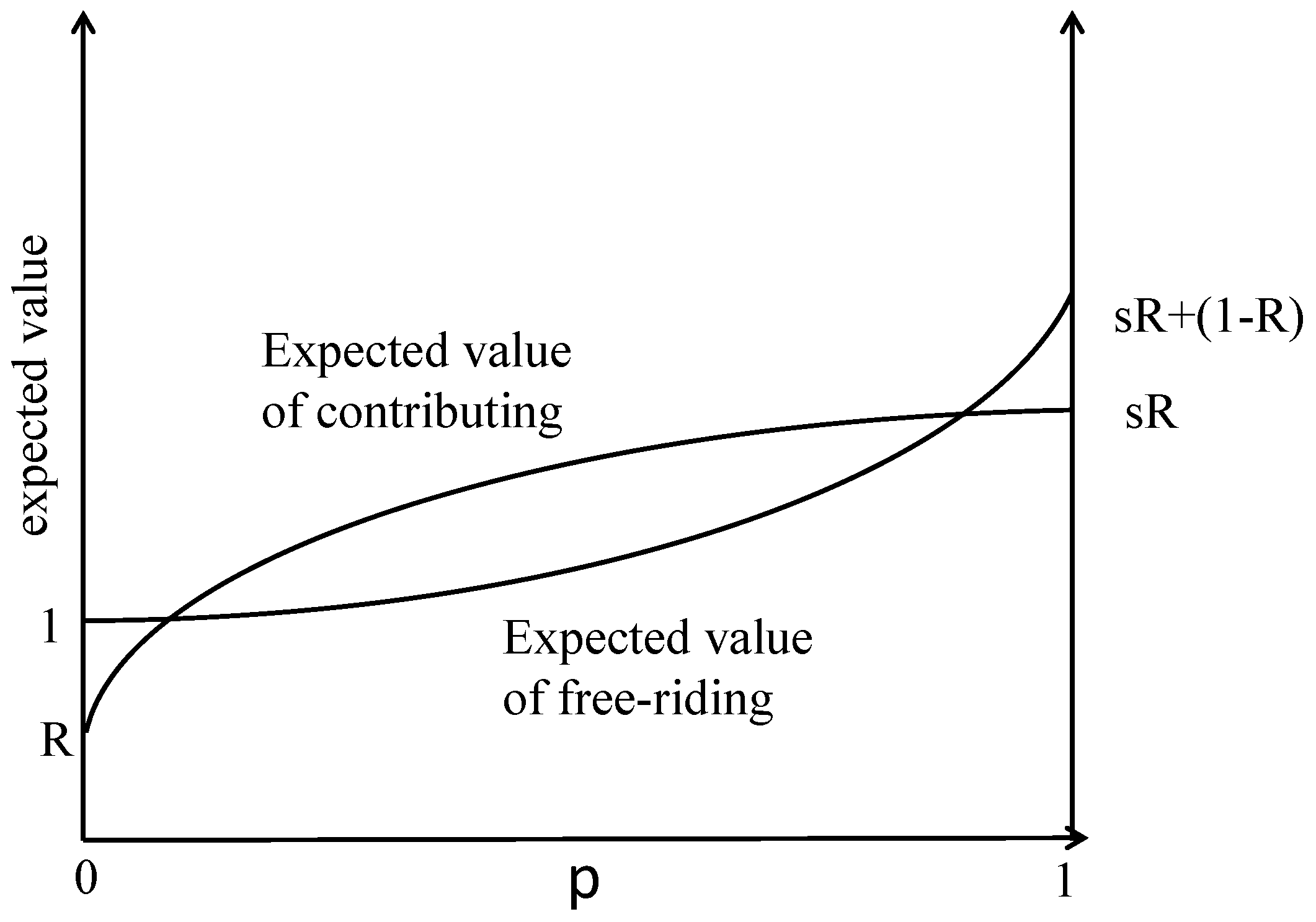
3.1. Game 1: ‘Baseline’
3.2. Game 2: ‘Heterogeneity Extension’ (Extension 1)
3.2.1. Heterogeneity and Continuous Action Space
3.2.2. Binary Action Space
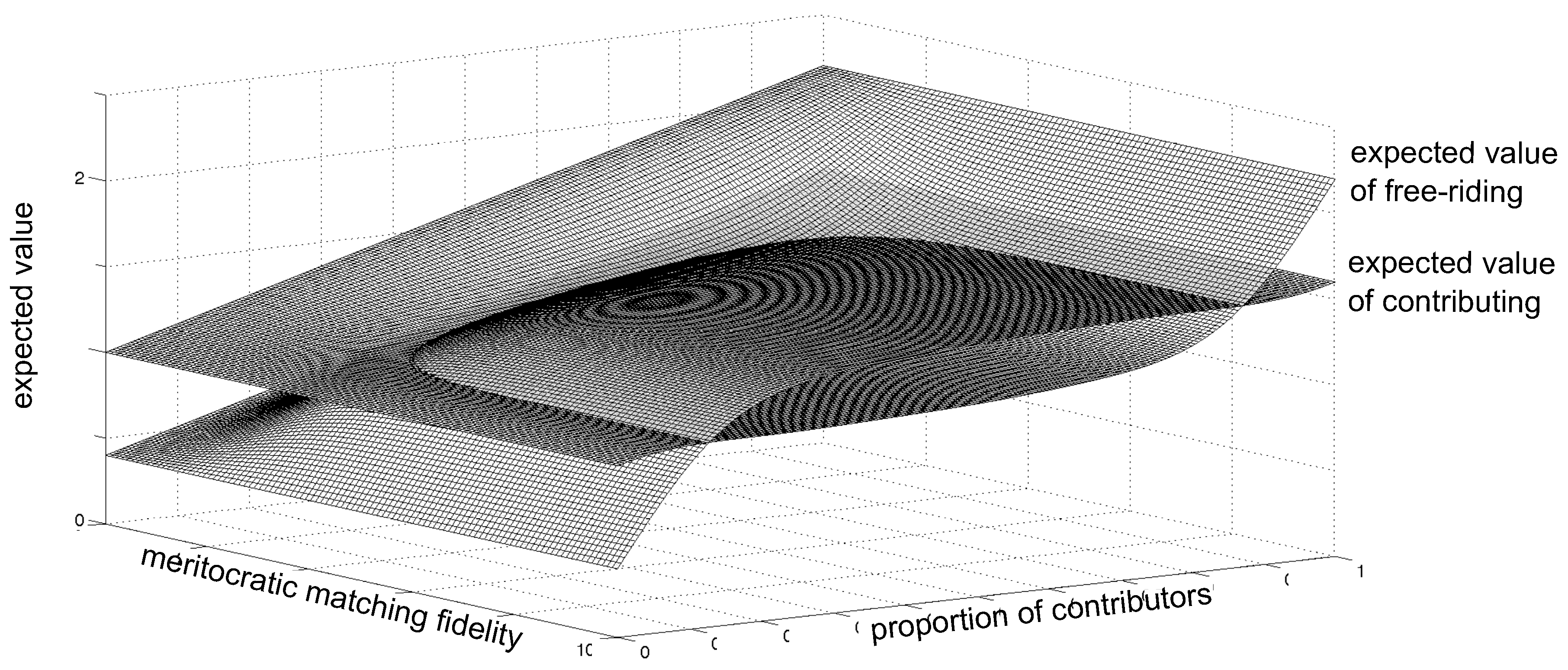
3.3. Game 3: ‘Noise Extension’ (Extension 2)
3.4. Remark: Mixed-Strategy Nash Equilibria
4. Welfare Comparison
You can’t have your cake and eat it, too, is a good candidate for the fundamental theorem of economic analysis. We can’t have our cake of market efficiency and share it equally.[24], p. 1.
4.1. Homogeneous Endowments
4.2. Heterogeneous Endowments
5. Logit Dynamics
| Algorithm 1: Logit choice dynamics. | |
| 1 | Initialization: Assign the initial endowments to each player sampling them from a Gaussian distribution with mean and standard deviation . If some endowments are zero or negative, the sampling is repeated.a |
| 2 | Initialization: Set initial strategy to 0 (start the simulation in the fully-defective stateb), and set time t to zero. |
| repeat | |
 | |
| until ; | |
- a
- The standard deviation is chosen for this to be an unlikely event.
- b
- Different initial starting conditions have been explored, and they have been observed to have no effect on the final outcome of the simulation.
- c
- Inertia was added to ensure the convergence to the pure-strategy Nash equilibrium (if existing). Without inertia, the best-response dynamics could oscillate around such an equilibrium.
- d
- For a definition, see, e.g., [34].
6. Summary
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Pure Strategy Nash Equilibria
Appendix A.1. Perfect Meritocracy
- We have a population of n players with .
- We have g groups, , of size s.
- is the payoff of agent i with .
Appendix A.2. Fuzzy Mechanism
Appendix B. Mixed-Strategy Nash Equilibria
Appendix C. Properties of the Fuzzy Ranking
- For , the contributions are perfectly observable, and the ranking follows a perfect ordering.
- For , contributions do not matter, and the ordering of the players is determined completely at random. This follows from the property of the normal distribution for ().
- For , the expected ranking for every player has the following properties:
Proofs
References
- Abreu, D.; Pearce, D.; Stacchetti, E. Toward a theory of discounted repeated games with imperfect monitoring. Econom. J. Econom. Soc. 1990, 58, 1041–1063. [Google Scholar] [CrossRef]
- Fudenberg, D.; Levine, D.; Maskin, E. The folk theorem with imperfect public information. Econom. J. Econom. Soc. 1994, 62, 997–1039. [Google Scholar] [CrossRef]
- Wu, J.; Axelrod, R. How to cope with noise in the iterated prisoner’s dilemma. J. Confl. Resolut. 1995, 39, 183–189. [Google Scholar] [CrossRef]
- Alchian, A.A.; Demsetz, H. Production, information costs, and economic organization. Am. Econ. Rev. 1972, 62, 777–795. [Google Scholar]
- Gunnthorsdottir, A.; Vragov, R.; Seifert, S.; McCabe, K. Near-efficient equilibria in contribution-based competitive grouping. J. Public Econ. 2010, 94, 987–994. [Google Scholar] [CrossRef]
- Andreoni, J. Why free ride? Strategies and learning in public goods experiments. J. Public Econ. 1988, 37, 291–304. [Google Scholar] [CrossRef]
- Andreoni, J. An experimental test of the public goods crowding-out hypothesis. Am. Econ. Rev. 1993, 83, 1317–1327. [Google Scholar]
- Andreoni, J. Cooperation in public-goods experiments: kindness or confusion? Am. Econ. Rev. 1995, 85, 891–904. [Google Scholar]
- Palfrey, T.R.; Prisbrey, J.E. Altruism, reputation and noise in linear public goods experiments. J. Public Econ. 1996, 61, 409–427. [Google Scholar] [CrossRef]
- Palfrey, T.R.; Prisbrey, J.E. Anomalous behavior in public goods experiments: How much and why? Am. Econ. Rev. 1997, 87, 829–846. [Google Scholar]
- Goeree, J.K.; Holt, C.A.; Laury, S.K. Private costs and public benefits: Unraveling the effects of altruism and noisy behavior. J. Public Econ. 2002, 83, 255–276. [Google Scholar] [CrossRef]
- Ferraro, P.J.; Vossler, C.A. The source and significance of confusion in public goods experiments. BE J. Econ. Anal. Policy 2010, 10, 53. [Google Scholar] [CrossRef]
- Fischbacher, U.; Gaechter, S. Social preferences, beliefs, and the dynamics of free riding in public good experiments. Am. Econ. Rev. 2010, 100, 541–556. [Google Scholar] [CrossRef]
- Bayer, R.C.; Renner, E.; Sausgruber, R. Confusion and learning in the voluntary contributions game. Exp. Econ. 2013, 16, 478–496. [Google Scholar] [CrossRef]
- Nax, H.H.; Burton-Chellew, M.N.; West, S.A.; Young, H.P. Learning in a Black Box. J. Econ. Behav. Organ. 2016, 127, 1–15. [Google Scholar]
- Burton-Chellew, M.N.; Nax, H.H.; West, S.A. Payoff-based learning explains the decline in cooperation in public goods games. Proc. R. Soc. Lond. B Biol. Sci. 2015, 282, 20142678. [Google Scholar] [CrossRef] [PubMed]
- Chaudhuri, A. Sustaining cooperation in laboratory public goods experiments: A selective survey of the literature. Exp. Econ. 2011, 14, 47–83. [Google Scholar] [CrossRef]
- Ledyard, J.O. Public Goods: A Survey of Experimental Research. In Handbook of Experimental Economics; Kagel, J.H., Roth, A.E., Eds.; California Institute of Technology: Pasadena, CA, USA, 1995; Volume 37, pp. 111–194. [Google Scholar]
- Gunnthorsdottir, A.; Thorsteinsson, P. Tacit Coordination and Equilibrium Selection in a Merit-Based Grouping Mechanism: A Cross-Cultural Validation Study. In Proceedings of the 19th International Congress on Modelling and Simulation (MODSIM2011), Perth, Australia, 12–16 December 2011. [Google Scholar]
- Rabanal, J.P.; Rabanal, O.A. Efficient Investment via Assortative Matching: A Laboratory Experiment; Hamilton College: Clinton, NY, USA, Unpublished work; 2014. [Google Scholar]
- Ones, U.; Putterman, L. The ecology of collective action: A public goods and sanctions experiment with controlled group formation. J. Econ. Behav. Organ. 2007, 62, 495–521. [Google Scholar] [CrossRef]
- Duca, S.; Helbing, D.; Nax, H.H. Assortative Matching with Inequality in Voluntary Contribution Games. Comput. Econ. 2017, in press. [Google Scholar] [CrossRef]
- Gunnthorsdottir, A.; Vragov, R.; Shen, J. TACIT Coordinationin Contribution-Based Grouping with Two Endowment Levels. Res. Exp. Econ. 2010, 13, 13–75. [Google Scholar]
- Okun, A.M. Equality and Efficiency—The Big Tradeoff; The Brookings Institution: Washington, DC, USA, 1975. [Google Scholar]
- Harsanyi, J. Cardinal Utility in Welfare Economics and in the Theory of Risk-Taking. J. Polit. Econ. 1953, 61, 434–435. [Google Scholar] [CrossRef]
- Binmore, K. Natural Justice; Oxford University Press: Oxford, UK, 2005. [Google Scholar]
- Harsanyi, J.C.; Selten, R. A General Theory of Equilibrium Selection in Games; MIT Press: Cambridge, MA, USA, 1988. [Google Scholar]
- Atkinson, A.B. On the measurement of inequality. J. Econ. Theory 1970, 2, 244–263. [Google Scholar] [CrossRef]
- Jones-Lee, M.W.; Loomes, G. Discounting and Safety. Oxf. Econ. Pap. 1995, 47, 501–512. [Google Scholar] [CrossRef]
- Dorfman, R. A formula for the Gini coefficient. Rev. Econ. Stat. 1979, 146–149. [Google Scholar] [CrossRef]
- Gini, C. Concentration and dependency ratios. Riv. Polit. Econ. 1997, 87, 769–792. [Google Scholar]
- Blume, L.E. The statistical mechanics of strategic interaction. Games Econ. Behav. 1993, 5, 387–424. [Google Scholar] [CrossRef]
- Mäs, M.; Nax, H.H. A behavioral study of “noise” in coordination games. J. Econ. Theory. 2016, 162, 195–208. [Google Scholar] [CrossRef] [Green Version]
- McKelvey, R.D.; Palfrey, T.R. Quantal Response Equilibria for Normal Form Games. Games Econ. Behav. 1995, 10, 6–38. [Google Scholar] [CrossRef]
- Cinyabuguma, M.; Page, T.; Putterman, L. Cooperation under the threat of expulsion in a public goods experiment. J. Public Econ. 2005, 89, 1421–1435. [Google Scholar] [CrossRef]
- Charness, G.B.; Yang, C.L. Endogenous Group Formation and Public Goods Provision: Exclusion, Exit, Mergers, and Redemption; University of California at Santa Barbara: Santa Barbara, CA, USA, Unpublished work; 2008. [Google Scholar]
- Ahn, T.; Isaac, R.M.; Salmon, T.C. Endogenous group formation. J. Public Econ. Theory 2008, 10, 171–194. [Google Scholar] [CrossRef]
- Ehrhart, K.; Keser, C. Mobility and Cooperation: On the Run; Centre interuniversitaire de recherche en analyse des organisations (CIRANO): Montréal, QC, Canada, Unpublished work; 1999. [Google Scholar]
- Coricelli, G.; Fehr, D.; Fellner, G. Partner Selection in Public Goods Experiments. J. Confl. Resolut. 2004, 48, 356–378. [Google Scholar] [CrossRef]
- Page, T.; Putterman, L.; Unel, B. Voluntary association in public goods experiments: Reciprocity, mimicryand efficiency. Econ. J. 2005, 115, 1032–1053. [Google Scholar] [CrossRef]
- Brekke, K.; Nyborg, K.; Rege, M. The fear of exclusion: Individual effort when group formation is endogenous. Scand. J. Econ. 2007, 109, 531–550. [Google Scholar] [CrossRef]
- Brekke, K.; Hauge, K.; Lind, J.T.; Nyborg, K. Playing with the good guys. A public good game with endogenous group formation. J. Public Econ. 2011, 95, 1111–1118. [Google Scholar] [CrossRef]
- Simon, H.A. A mechanism for social selection and successful altruism. Science 1990, 250, 1665–1668. [Google Scholar] [CrossRef] [PubMed]
- Bowles, S.; Gintis, H. A Cooperative Species—Human Reciprocity and Its Evolution; Princeton University Press: Princeton, NJ, USA, 2011. [Google Scholar]
- Fehr, E.; Camerer, C. Social neuroeconomics: The neural circuitry of social preferences. Trends Cognit. Sci. 2007, 11, 419–427. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Allchin, D. The Evolution of Morality; Evolution: Education and Outreach; Springer: Berlin, Germany, 2009. [Google Scholar]
- Hamilton, W.D. The Genetical Evolution of Social Behaviour I. J. Theor. Biol. 1964, 7, 1–16. [Google Scholar] [CrossRef]
- Hamilton, W.D. The Genetical Evolution of Social Behaviour II. J. Theor. Biol. 1964, 7, 17–52. [Google Scholar] [CrossRef]
- Nowak, M.A. Five rules for the evolution of cooperation. Science 2006, 314, 1560–1563. [Google Scholar] [CrossRef] [PubMed]
- Alger, I.; Weibull, J. Homo Moralis—Preference Evolution Under Incomplete Information and Assortative Matching. Econometrica 2013, 81, 2269–2302. [Google Scholar]
- Grund, T.; Waloszek, C.; Helbing, D. How Natural Selection Can Create Both Self- and Other-Regarding Preferences, and Networked Minds. Sci. Rep. 2013, 3, 1480. [Google Scholar] [CrossRef] [PubMed]
- Newton, J. The preferences of Homo Moralis are unstable under evolving assortativity. Int. J. Game Theory 2017, 46, 583–589. [Google Scholar] [CrossRef]
- Nax, H.H.; Rigos, A. Assortativity evolving from social dilemmas. J. Theor. Biology 2016, 395, 194–203. [Google Scholar] [CrossRef] [PubMed]
- Newton, J. Shared intentions: the evolution of collaboration. Games Econ. Behav. 2017, 104, 517–534. [Google Scholar] [CrossRef]
- Cabral, L.M.B. Asymmetric Equilibria in Symmetric Games with Many Players. Econ. Lett. 1988, 27, 205–208. [Google Scholar] [CrossRef]
- Gardiner, C.W. Stochastic Methods; Springer: Berlin, Germany, 2009. [Google Scholar]
| 1 | |
| 2 | With a slight abuse of notation, from now on, we will write and to indicate the “no meritocracy” case where , and the ranking, and consequentially, the matching, of the players is (uniformly) randomly selected. |
| 3 | Meritocracy guides smoothly from (i) no meritocracy (grouping is random as in [6]) to (ii) full meritocracy (the case of perfect contribution-based grouping as in [5]). Note that many public goods experiments use variants of Andreoni’s random (re-)matching implementation (e.g., [6,7,8,9,10,11,12,13,14,15,16]); see [17,18] for reviews. |
| 4 | Thus, full-contribution is collectively efficient, and zero-contribution, despite collectively inefficient, is the unique Nash equilibrium under no meritocracy. |
| 5 | See [5], Theorem 1. |
| 6 | In fact, recent evidence suggests that players may endogenously implement variants of contribution-based competitive grouping over time and then converge to the high-contribution equilibria [21]. |
| 7 | For a marginal per capita rate of return , the game is not a social dilemma anymore: “cooperate” becomes a dominant strategy, and the only existing equilibrium is for everybody to contribute everything. |
| 8 | Note that, whenever pure strategy highly-efficient equilibria exist, there could also exist several asymmetric Nash equilibria whose characterization is not easily obtained. |
| 9 | |
| 10 | The work in [27] defines that outcome payoff-dominates if for all i, and there exists a j such that . |
| 11 | See, for example, [29] for a discussion of this generalization. |
| 12 | With , requires efficiency gains of more than twice the amount lost by any player to compensate for the additional inequality. |
| 13 | For continuous action space, the only equilibrium is non-contribution by all, and therefore, there is no social welfare analysis to be made. |
| 14 | |
| 15 | |
| 16 | Using the terminology of [46], our paper therefore studies a ‘system’ rather than moral ‘acts’ or ‘intentions’. In our mechanism, the system assorts contributions, i.e., actions, as other evolutionary biology mechanisms that lead to cooperation as, for example, kin selection (e.g., [47,48,49]), local interaction and/or assortative matching of preferences [50,51,52,53,54]. |
| 17 | It is easy to check that . |
| 18 | See Appendix C. |
| 19 | Details concerning the use of the law of large numbers can be followed based on the proof in [55]. |
| 20 | See, e.g., [56] |


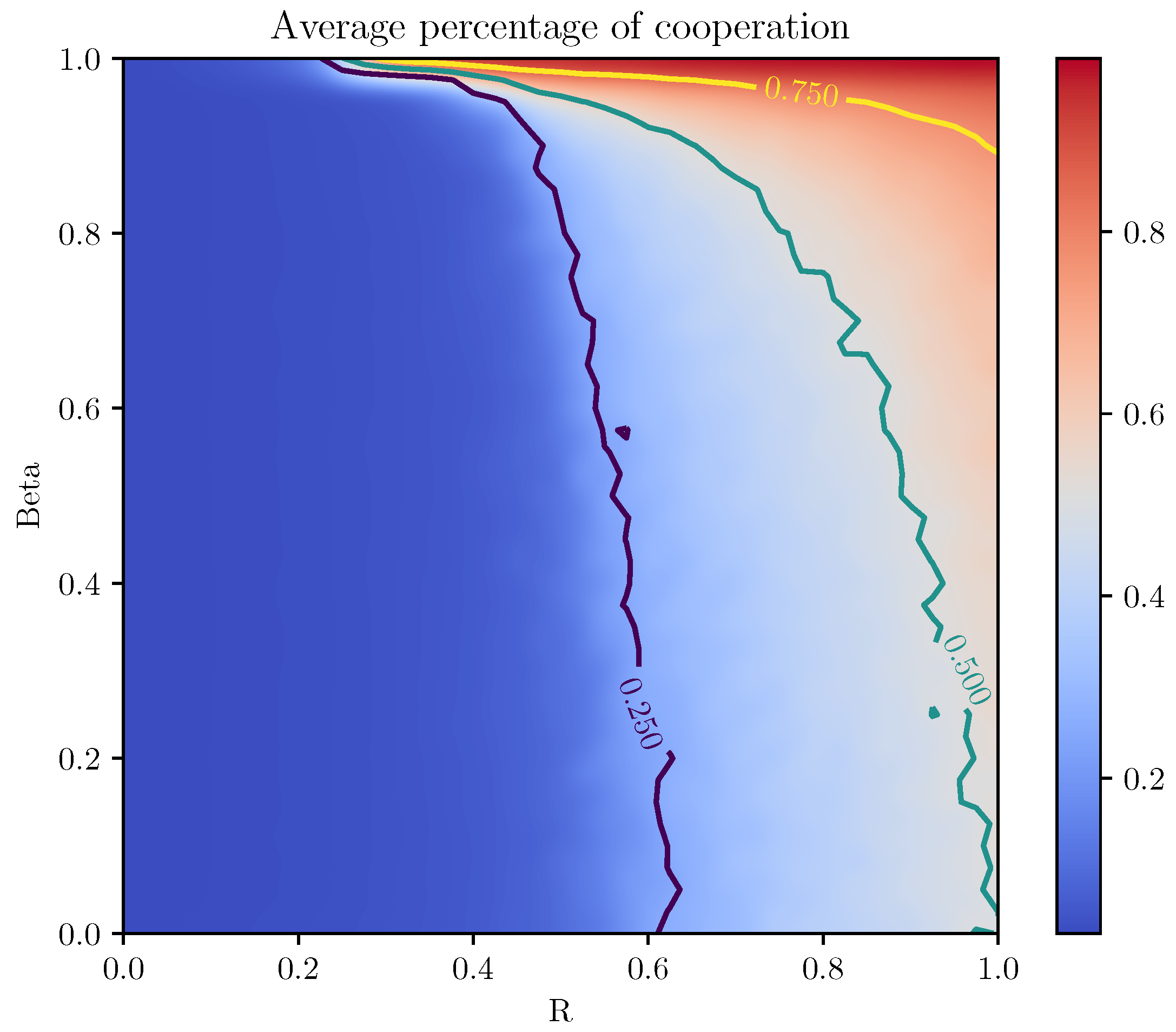
| High-Contribution Equilibrium | Payoff | Non-Contribution Equilibrium |
|---|---|---|
| When | When | |
| 0 | 0.0 | 0 |
| 0 | 0.2 | 0 |
| 0 | 0.4 | 0 |
| 0 | 0.6 | 0 |
| 13 14 () 2 | 0.8 | 0 |
| 0 | 1.0 | 16 () 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 |
| 0 | 1.2 | 0 |
| 0 | 1.4 | 0 |
| 1 2 3 4 5 6 7 8 9 10 11 12 () 12 | 1.6 | 0 |
| 15 16 () 2 | 1.8 | 0 |
| 24.4 | efficiency | 16 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nax, H.H.; Murphy, R.O.; Duca, S.; Helbing, D. Contribution-Based Grouping under Noise. Games 2017, 8, 50. https://doi.org/10.3390/g8040050
Nax HH, Murphy RO, Duca S, Helbing D. Contribution-Based Grouping under Noise. Games. 2017; 8(4):50. https://doi.org/10.3390/g8040050
Chicago/Turabian StyleNax, Heinrich H., Ryan O. Murphy, Stefano Duca, and Dirk Helbing. 2017. "Contribution-Based Grouping under Noise" Games 8, no. 4: 50. https://doi.org/10.3390/g8040050
APA StyleNax, H. H., Murphy, R. O., Duca, S., & Helbing, D. (2017). Contribution-Based Grouping under Noise. Games, 8(4), 50. https://doi.org/10.3390/g8040050





