Sustainability of Intertwined Supply Networks: A Game-Theoretic Approach
Abstract
:1. Introduction
- (1)
- to present an active system representation of ISNs, and to describe the functioning of various types of ISNs;
- (2)
- to give a game-theoretic formalization of ISNs with consideration of their structure;
- (3)
- to specify the notions of the system’s sustainability and sustainable management in game-theoretic terms;
- (4)
- to study a dynamic game-theoretic model of Social and Private Interests Coordination Engines (SPICE-model) for ISNs with application to marketing.
- a well-grounded active system interpretation of ISNs is presented, and the role of control in the functioning of various types of ISNs is described;
- a game-theoretic formalization of ISNs as active systems is proposed, taking into account their structure. Special cases of the general model are highlighted;
- the notions of the system’s sustainability and sustainable management capability are specified within the deterministic ISN model;
- a dynamic game-theoretic model of Social and Private Interests Coordination Engines (SPICE-model) for ISNs is constructed, and application to marketing is considered. This model is studied for the linear functions of the private interests of influence agents.
2. Literature Review
3. Intertwined Supply Networks: Control and Formalization
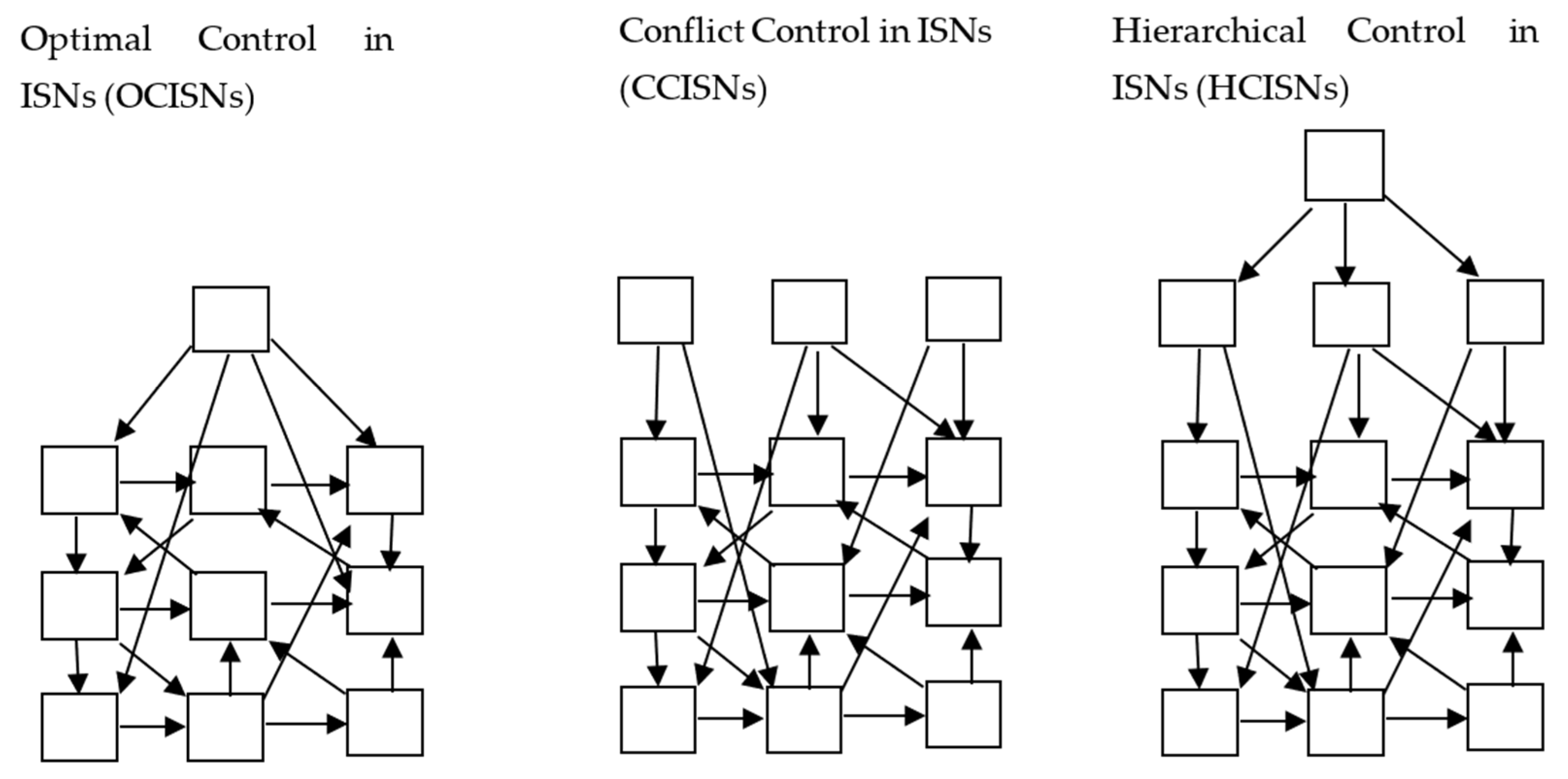
3.1. Optimal Control in ISNs
3.2. Conflict Control in ISNs
3.3. Hierarchical Control in ISNs
4. Notion of Sustainability
4.1. General Case
- , which globally maximizes the payoff functional of the Principal;
- , which ensures the compatibility of the agents’ interests with the Principal’s interests, albeit neglecting viability;
- , which ensures viability with due consideration of the Principal’s interests, albeit neglecting the agents’ interests;
- , which ensures viability with due consideration of the agents’ interests, albeit neglecting the Principal’s interests;
- , which ensures viability with the perfect compatibility of all interests.
- open-loop strategies (Stackelberg games) or feedback strategies (inverse Stackelberg games); see [69];
- compulsion or impulsion ;
- the deterministic or stochastic model.
- The Principal shows indifference, , being interested only in the viability condition (11). Then, the set becomes needless, and it remains to verify the condition
- The Principal maximizes the social welfare
- in the case of compulsion,
- in the case of impulsion,
4.2. Sustainability of Intertwined Supply Networks
5. SPICE-Models of Intertwined Supply Networks in Marketing
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- World Commission on Environment and Development (WCED). Our Common Future; Oxford University Press: Oxford, UK, 1987. [Google Scholar]
- Aubin, J.P. Viability Theory; Boston: Birkhäuser, Switzerland, 1991. [Google Scholar]
- Govindan, K.; Hasanagic, M. A Systematic Review on Drivers, Barriers, and Practices Towards Circular Economy: A Supply Chain Perspective. Int. J. Prod. Res. 2018, 56, 278–311. [Google Scholar] [CrossRef]
- Howard, M.; Hopkinson, P.; Miemczyk, J. The Regenerative Supply Chain: A Framework for Developing Circular Economy Indicators. Int. J. Prod. Res. 2018, 57, 7300–7318. [Google Scholar] [CrossRef] [Green Version]
- Laffont, J.-J.; Martimort, D. The Theory of Incentives: The Principal-Agent Model; Princeton University Press: Princeton, NJ, USA, 2002. [Google Scholar]
- Myerson, R. Incentive Compatibility and the Bargaining Problem. Econometrica 1979, 47, 61–73. [Google Scholar] [CrossRef] [Green Version]
- Myerson, R. Optimal Coordination Mechanisms in Generalized Principal-Agent Models. J. Math. Econ. 1982, 10, 67–81. [Google Scholar] [CrossRef]
- Myerson, R. Mechanism Design by an Informed Principal. Econometrica 1983, 51, 1767–1798. [Google Scholar] [CrossRef]
- Burkov, V.N.; Gubko, M.V.; Kondrat’ev, V.V.; Korgin, N.A.; Novikov, D.A. Mechanism Design and Management: Mathematical Methods for Smart Organizations; Novikov, D., Ed.; Nova Science Publishers: Hauppauge, NY, USA, 2013. [Google Scholar]
- Novikov, D. Theory of Control in Organizations; Nova Science Publishers: Hauppauge, NY, USA, 2013. [Google Scholar]
- Gorelov, M.A.; Kononenko, F.A. Dynamic Models of Conflicts. III. Hierarchical Games. Autom. Remote Control 2015, 76, 264–277. [Google Scholar] [CrossRef]
- Ivanov, D.; Dolgui, A. Viability of Intertwined Supply Networks: Extending the Supply Chain Resilience Angles towards Survivability. A Position Paper Motivated by COVID-19 Outbreak. Int. J. Prod. Res. 2020, 58, 2904–2915. [Google Scholar] [CrossRef] [Green Version]
- Ougolnitsky, G. Sustainable Management; Nova Science Publishers: Hauppauge, NY, USA, 2011. [Google Scholar]
- Ougolnitsky, G. Game Theoretic Formalization of the Concept of Sustainable Development in the Hierarchical Control Systems. Ann. Oper. Res. 2014, 220, 69–86. [Google Scholar] [CrossRef]
- Ougolnitsky, G.A. Sustainable Management as a Key to Sustainable Development. In Sustainable Development: Processes, Challenges and Prospects; Reyes, D., Ed.; Nova Science Publishers: Hauppauge, NY, USA, 2015; pp. 87–128. [Google Scholar]
- Ougolnitsky, G.A. A System Approach to the Regional Sustainable Management. Adv. Syst. Sci. Appl. 2017, 17, 52–62. [Google Scholar]
- Ugol’nitskii, G.A.; Usov, A.B. Equilibria in Models of Hierarchically Organized Dynamic Systems with Regard to Sustainable Development Conditions. Autom. Remote Control 2014, 75, 1055–1068. [Google Scholar] [CrossRef]
- Kornienko, S.A.; Ougolnitsky, G.A. Dynamic Stackelberg Games with Requirements to the Controlled System as a Model of Sustainable Environmental Management. Adv. Syst. Sci. Appl. 2014, 14, 325–345. [Google Scholar]
- Kates, R.W.; Clark, W.C.; Corell, R.; Hall, J.M.; Jaeger, C.C.; Lowe, I.; Mccarthy, J.J.; Schellnhuber, H.J.; Bolin, B.; Dickson, N.M.; et al. Sustainability Science. Science 2001, 292, 641–642. [Google Scholar] [CrossRef] [PubMed]
- Pinter, L.; Hardi, P.; Martinucci, A.; Hall, J. Bellagio STAMP: Principles for Sustainability Assessment and Measurement. Ecol. Indic. 2012, 17, 20–28. [Google Scholar] [CrossRef]
- Zaccai, E. Over Two Decades in Pursuit of Sustainable Development: Influence, Transformations, Limits. Environ. Dev. 2012, 1, 79–90. [Google Scholar] [CrossRef]
- Bonneuil, N.; Saint-Pierre, P. Population Viability in Three Trophic-Level Food Chains. Appl. Math. Comput. 2005, 169, 1086–1105. [Google Scholar] [CrossRef] [Green Version]
- Bonneuil, N.; Boucekkine, R. Viable Nash Equilibria in the Problem of Common Pollution. Pure Appl. Funct. Anal. 2017, 2, 427–440. [Google Scholar]
- Martinet, V.; Doyen, L. Sustainability of an Economy with an Exhaustible Resource: A Viable Control Approach. Resour. Energy Econ. 2007, 29, 17–39. [Google Scholar] [CrossRef]
- Martinet, V. A Characterization of Sustainability with Indicators. J. Environ. Econ. Manag. 2011, 61, 183–197. [Google Scholar] [CrossRef]
- Cairns, R.D.; Long, N.V. Maximin: A Direct Approach to Sustainability. Environ. Dev. Econ. 2006, 11, 275–300. [Google Scholar] [CrossRef]
- Cairns, R.D.; Martinet, V. An Environmental-Economic Measure of Sustainable Development. Eur. Econ. Rev. 2014, 69, 4–17. [Google Scholar] [CrossRef] [Green Version]
- Doyen, L.; Martinet, V. Maximin, Viability and Sustainability. J. Econ. Dyn. Control 2012, 36, 1414–1430. [Google Scholar] [CrossRef] [Green Version]
- Basar, T.; Olsder, G. Dynamic Noncooperative Game Theory; SIAM: Philadelphia, PA, USA, 1999. [Google Scholar]
- Dockner, E.; Jorgensen, S.; Long, N.V.; Sorger, G. Differential Games in Economics and Management Science; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Yeung, D.W.K.; Petrosyan, L. Subgame Consistent Economic Optimization; Birkhauser: Basel, Switzerland, 2012. [Google Scholar]
- Ougolnitsky, G.A.; Usov, A.B. Computer Simulations as a Solution Method for Differential Games. In Computer Simulations: Advances in Research and Applications; Pfeffer, M.D., Bachmaier, E., Eds.; Nova Science Publishers: Hauppauge, NY, USA, 2018; pp. 63–106. [Google Scholar]
- Long, N.V. A Survey of Dynamic Games in Economics; World Scientific Publishing Company: Singapore, 2010. [Google Scholar]
- Gorbaneva, O.I.; Ougolnitsky, G.A. System Compatibility, Price of Anarchy and Control Mechanisms in the Models of Concordance of Private and Public Interests. Adv. Syst. Sci. Appl. 2015, 15, 45–59. [Google Scholar]
- Gorbaneva, O.I.; Ougolnitsky, G. Static Models of Coordination of Social and Private Interests in Resource Allocation. Autom. Remote Control 2018, 79, 1319–1341. [Google Scholar] [CrossRef]
- Sukhinov, A.I.; Ougolnitsky, G.A.; Usov, A.B. Methods of Solving the Theoretic Game Models for Coordinating Interests in Regulating the Fishery Industry. Math. Models Comput. Simul. 2020, 12, 176–184. [Google Scholar] [CrossRef]
- Ugol’nitskii, G.A.; Usov, A.B. Dynamic Models for Coordinating Private and Public Interests in Economic Corruption. J. Comput. Syst. Sci. Int. 2020, 59, 39–48. [Google Scholar] [CrossRef]
- Chkhartishvili, A.; Gubanov, D.; Novikov, D. Social Networks: Models of Information Influence, Control, and Confrontation; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
- Jackson, M. Social and Economic Networks; Princeton University Press: Princeton, NJ, USA, 2008. [Google Scholar]
- Proskurnikov, A.; Tempo, R. A Tutorial on Modeling and Analysis of Dynamic Social Networks. Part I. Annu. Rev. Control 2017, 43, 65–79. [Google Scholar] [CrossRef] [Green Version]
- Proskurnikov, A.; Tempo, R. A Tutorial on Modeling and Analysis of Dynamic Social Networks. Part II. Annu. Rev. Control 2018, 45, 166–190. [Google Scholar] [CrossRef] [Green Version]
- Novikov, D.A. Games and Networks. Autom. Remote Control 2014, 75, 1145–1154. [Google Scholar] [CrossRef]
- Sedakov, A.; Zhen, M. Opinion Dynamics Game in a Social Network with Two Influence Nodes. Vestn. SPb Gos. Univ. Ser. Appl. Math. Inform. Sci. Contr. Proc. 2019, 15, 118–125. [Google Scholar] [CrossRef] [Green Version]
- Agieva, M.T.; Korolev, A.V.; Ougolnitsk, G.A. Modeling and Simulation of Impact and Control in Social Networks, The First International EURO Mini-Conference on Modelling and Simulation of Social-Behavioural Phenomena in Creative Societies (MSBC 2019), Vilnius, Lithuania, 18–20 September 2019. In Communications in Computer and Information Science; Agarwal, N., Sakalauskas, L., Weber, G.-W., Eds.; Springer: Berlin/Heidelberg, Germany, 2019; Volume 1079, pp. 29–40. [Google Scholar]
- Korolev, A.V.; Ougolnitsky, G.A. Optimal Resource Allocation in the Difference and Differential Stackelberg Games on Marketing Networks. J. Dyn. Games 2020, 7, 141–162. [Google Scholar] [CrossRef] [Green Version]
- Agieva, M.; Gorbaneva, O. Dynamic SPICE-model of Resource Allocation in Marketing Networks. In Contributions to Game Theory and Management; Springer: Berlin/Heidelberg, Germany, 2020; Volume 13, pp. 8–23. [Google Scholar]
- Agieva, M.; Gorbaneva, O.; Ougolnitsky, G. Dynamic SPICE-model of Resource Allocation in Marketing Networks with Co-directed Interests. In Proceedings of the 2nd International Conference on Control Systems, Mathematical Modeling, Automation and Energy Efficiency (SUMMA2020), Lipetsk, Russia, 12–14 November 2020. [Google Scholar]
- Agieva, M.; Korolev, A.V.; Ougolnitsky, G.A. Game Theoretic Models of Sustainable Management in Marketing Networks. In Contributions to Game Theory and Management; Springer: Berlin/Heidelberg, Germany, 2020; Volume 13, pp. 24–56. [Google Scholar]
- Tur, A.; Petrosyan, L. Strong Time-Consistent Solution for Cooperative Differential Games with Network Structure. Mathematics 2021, 9, 755. [Google Scholar] [CrossRef]
- Petrosyan, L.A.; Yeung, D.W.K. Shapley value for differential network games: Theory and application. J. Dyn. Games 2021, 8, 151–166. [Google Scholar] [CrossRef]
- Petrosyan, L.; Bulgakova, M.; Sedakov, A. Time-Consistent Solutions for Two-Stage Network Games with Pairwise Interactions. Mob. Netw. Appl. 2021, 26, 491–500. [Google Scholar] [CrossRef]
- Rentizelas, A.; Trivyza, N.; Oswald, S.; Siegl, S. Reverse supply network design for circular economy pathways of wind turbine blades in Europe. Int. J. Prod. Res. 2022, 60, 1795–1814. [Google Scholar] [CrossRef]
- Zhao, H.; Chen, J.; Ai, X. Contract strategy in the presence of chain to chain competition. Int. J. Prod. Res. 2022, 60, 1913–1931. [Google Scholar] [CrossRef]
- Kamalahmadi, M.; Shekarian, M.; Parast, M.M. The impact of flexibility and redundancy on improving supply chain resilience to disruptions. Int. J. Prod. Res. 2022, 60, 1992–2020. [Google Scholar] [CrossRef]
- Dolgui, A.; Ivanov, D.; Sokolov, B. Ripple Effect in the Supply Chain: An Analysis and Recent Literature. Int. J. Prod. Res. 2018, 56, 414–430. [Google Scholar] [CrossRef] [Green Version]
- Ivanov, D. Predicting the Impact of Epidemic Outbreaks on the Global Supply Chains: A Simulation-Based Analysis on the Example of Coronavirus (COVID-19/SARS-CoV-2) Case. Transp. Res. Part E 2020, 136, 101922. [Google Scholar] [CrossRef]
- Ivanov, D.; Das, A. Coronavirus (COVID-19/SARS-CoV-2) and Supply Chain Resilience: A Research Note. Int. J. Integr. Supply Manag. 2020, 13, 90–102. [Google Scholar] [CrossRef]
- Dubey, R.; Gunasekarann, A.; Papadopoulos, T. Disaster Relief Operations: Past, Present and Future. Ann. Oper. Res. 2019, 283, 1–8. [Google Scholar] [CrossRef]
- Fraccascia, L.; Giannoccaro, I.; Albino, V. Rethinking Resilience in Industrial Symbiosis: Conceptualization and Measurements. Ecol. Econ. 2017, 137, 148–162. [Google Scholar] [CrossRef]
- Hosseini, S.; Ivanov, D.; Dolgui, A. Review of Quantitative Methods for Supply Chain Resilience Analysis. Transp. Res. Part. E 2019, 125, 285–307. [Google Scholar] [CrossRef]
- Wang, J.; Dou, R.; Muddada, R.R.; Zhang, W. Management of a Holistic Supply Chain Network for Proactive Resilience: Theory and Case Study. Comput. Ind. Eng. 2018, 125, 668–677. [Google Scholar] [CrossRef]
- Yoon, J.; Talluri, S.; Yildiz, H.; Ho, W. Models for Supplier Selection and Risk Mitigation: A Holistic Approach. Int. J. Prod. Res. 2018, 56, 3636–3661. [Google Scholar] [CrossRef]
- Basole, R.C.; Bellamy, M.A. Supply Network Structure, Visibility, and Risk Diffusion: A Computational Approach. Decis. Sci. 2014, 45, 753–789. [Google Scholar] [CrossRef]
- Choi, T.-M.; Taleizadeh, A.A.; Yue, X. Game Theory Applications in Production Research in the Sharing and Circular Economy Era. Int. J. Prod. Res. 2020, 58, 118–127. [Google Scholar] [CrossRef] [Green Version]
- Zhao, K.; Zuo, Z.; Blackhurst, J.V. Modelling Supply Chain Adaptation for Disruptions: An Empirically Grounded Complex Adaptive Systems Approach. J. Oper. Manag. 2019, 65, 190–212. [Google Scholar] [CrossRef]
- Bressanelli, G.; Perona, M.; Saccani, N. Challenges in Supply Chain Redesign for the Circular Economy: A Literature Review and a Multiple Case Study. Int. J. Prod. Res. 2018, 57, 7395–7422. [Google Scholar] [CrossRef] [Green Version]
- Harsanyi, J.C. Games with Incomplete Information Played by Bayesian Players. Manag. Sci. 1967, 14, 159–182. [Google Scholar] [CrossRef]
- De Groot, M.H. Reaching a Consensus. J. Am. Stat. Assoc. 1974, 69, 118–121. [Google Scholar] [CrossRef]
- Olsder, G.J. Phenomena in Inverse Stackelberg Games. Part 2: Dynamic Problems. J. Optim. Theory Appl. 2009, 143, 601–618. [Google Scholar] [CrossRef] [Green Version]
- Papadimitriou, C.H. Algorithms, Games, and the Internet. In Proceedings of the 33rd Symposium on Theory of Computing, Crete, Greece, 6–8 July 2001; pp. 749–753. [Google Scholar]

| Reference Number | Brief Content |
|---|---|
| [12] | A position paper introducing the notion of intertwined supply networks |
| [8,9,10,11,12,13,17,18,19] | Analysis of supply chains (resilience, ripple effect, circular economy, and so on) and development of the notion of ISN, including some game-theoretic models |
| [2,14,15,16] | Differential game-theoretic models with networked structure |
| [3,4,5,6,7] | Authors’ approach to the differential game-theoretic modeling of network systems with applications in marketing |
| With Principal | Without Principal | |
|---|---|---|
| Independent agents | Differential (difference) normal-form game | Hierarchical (Stackelberg differential (difference) game of players with state-space viability constraints |
| Grand coalition of agents (cooperation) | Continuous- or discrete-time optimal control problem | Hierarchical (Stackelberg) differential (difference) game of 2 players with state-space viability constraints |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gorbaneva, O.; Ougolnitsky, G. Sustainability of Intertwined Supply Networks: A Game-Theoretic Approach. Games 2022, 13, 35. https://doi.org/10.3390/g13030035
Gorbaneva O, Ougolnitsky G. Sustainability of Intertwined Supply Networks: A Game-Theoretic Approach. Games. 2022; 13(3):35. https://doi.org/10.3390/g13030035
Chicago/Turabian StyleGorbaneva, Olga, and Guennady Ougolnitsky. 2022. "Sustainability of Intertwined Supply Networks: A Game-Theoretic Approach" Games 13, no. 3: 35. https://doi.org/10.3390/g13030035
APA StyleGorbaneva, O., & Ougolnitsky, G. (2022). Sustainability of Intertwined Supply Networks: A Game-Theoretic Approach. Games, 13(3), 35. https://doi.org/10.3390/g13030035







