Economic Harmony—A Rational Theory of Fairness and Cooperation in Strategic Interactions
Abstract
1. Introduction
2. Theory
3. Predicting Behavior in Strategic Games
3.1. The Ultimatum Game
3.2. Bargaining Games with Alternating Offers
3.2.1. Comparison with Experimental Results
3.2.2. Equal Discount Factors
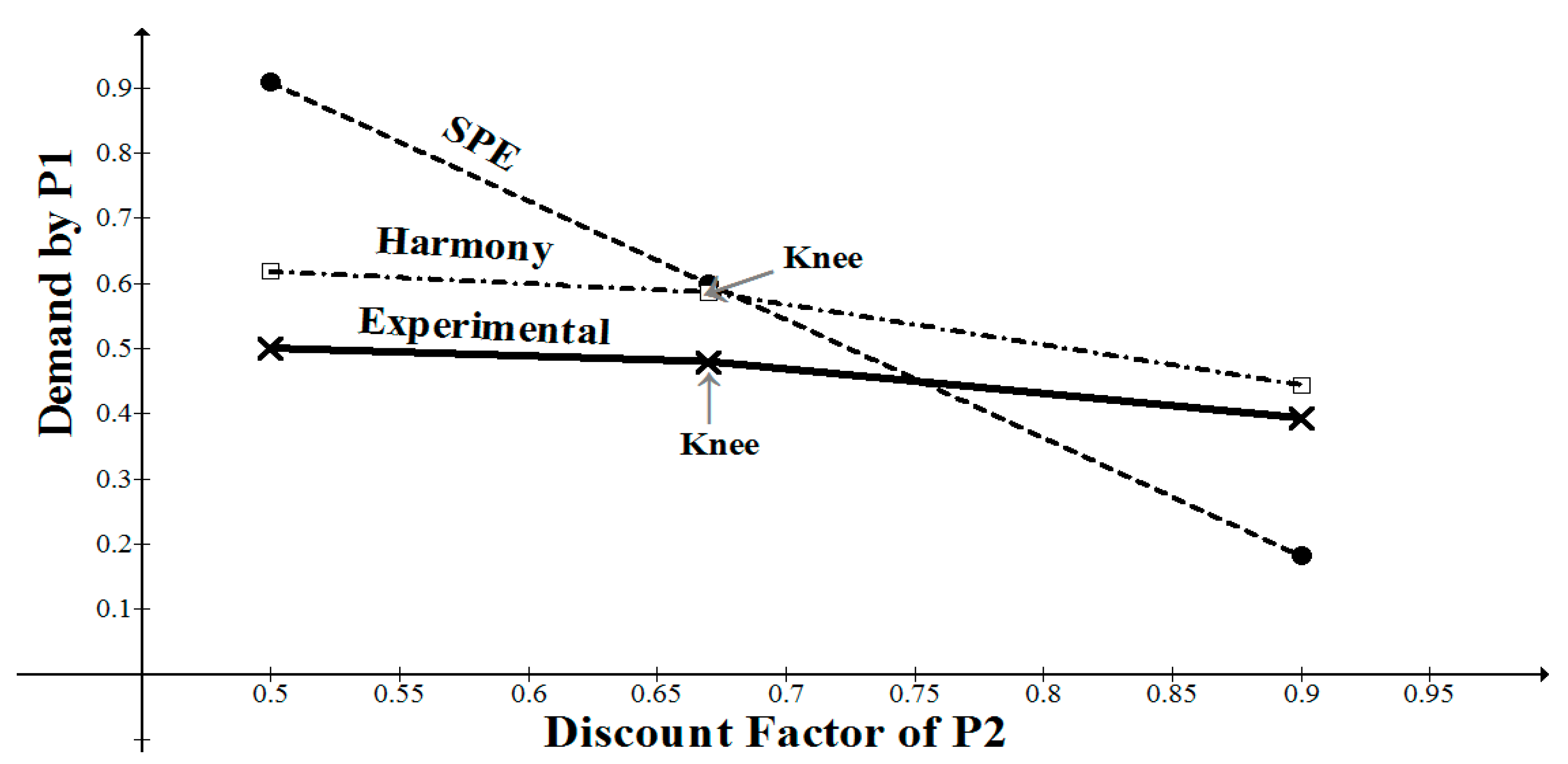
3.2.3. Unequal Discount Factors
3.3. Three Social Dilemmas
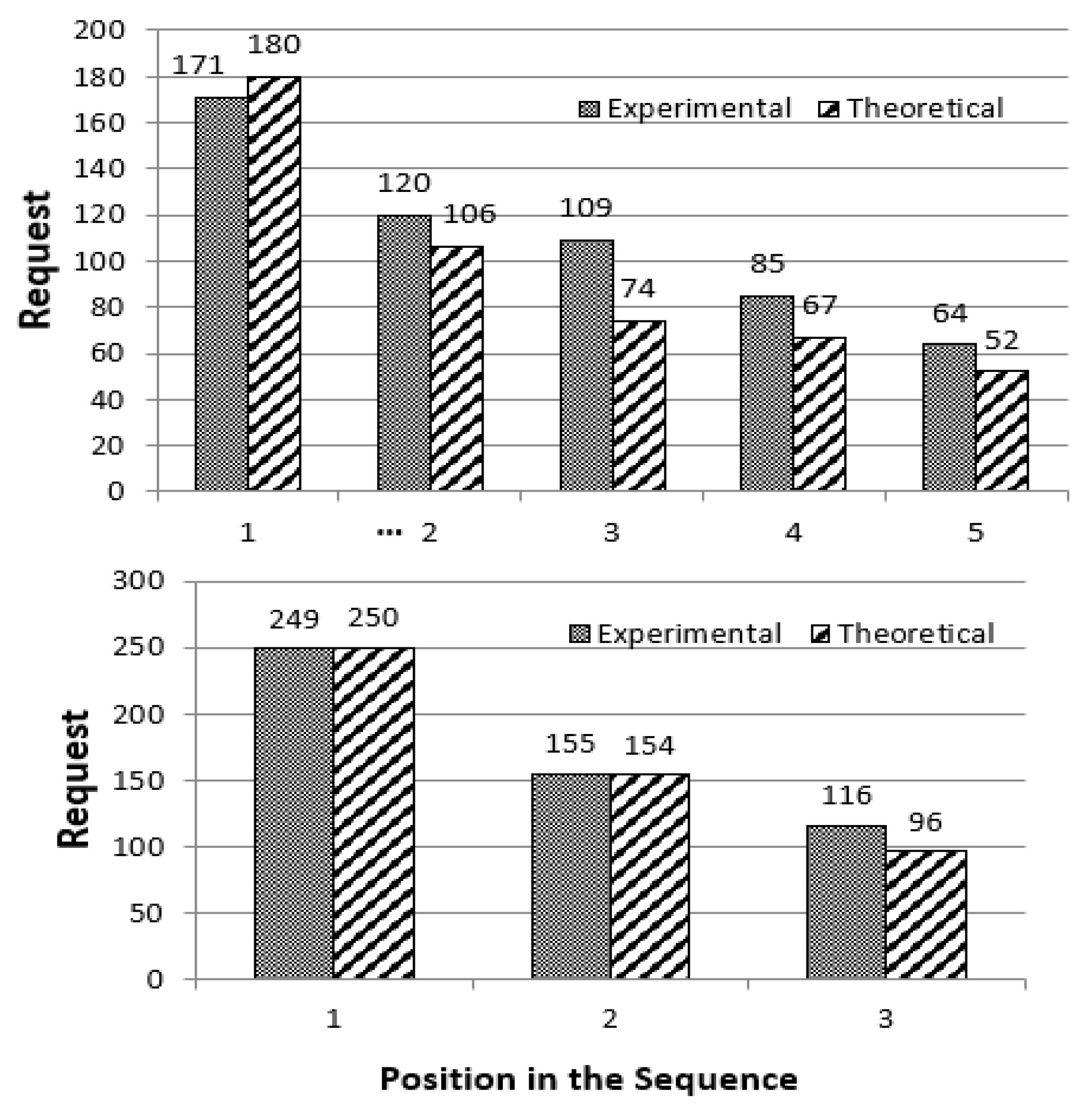
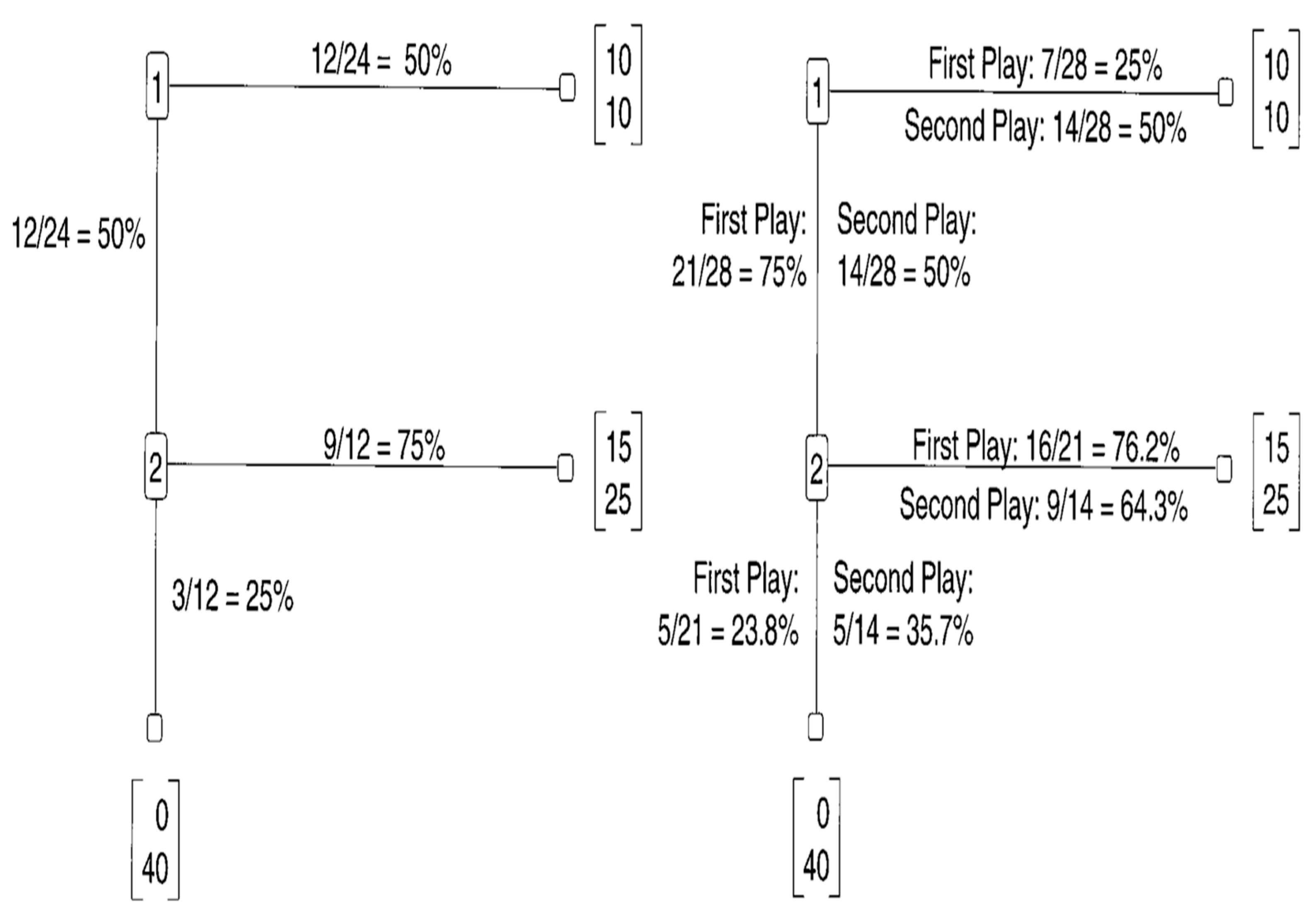
3.3.1. The Common-Pool Resource Dilemma Game
3.3.2. Comparison with Experimental Results
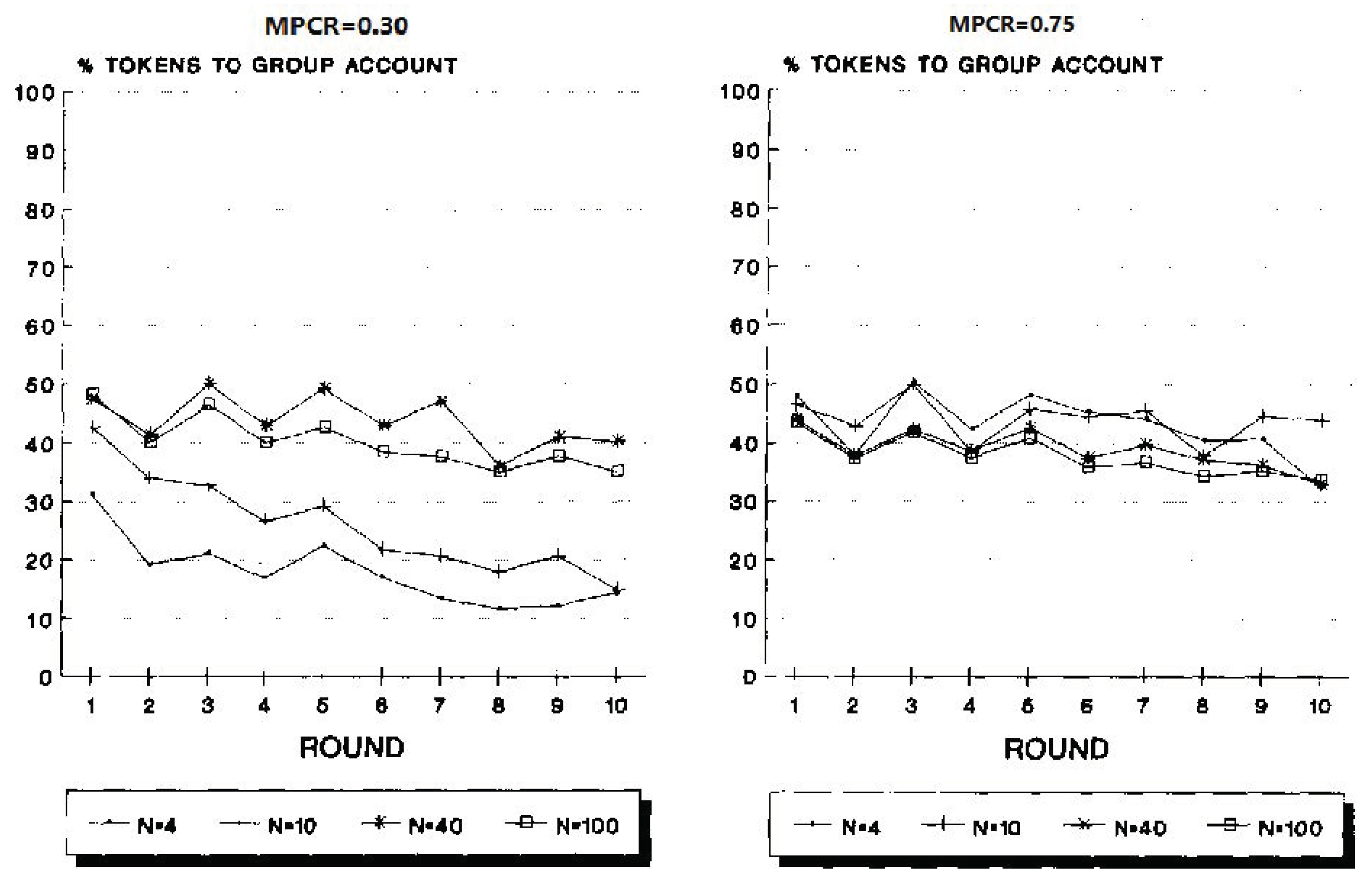
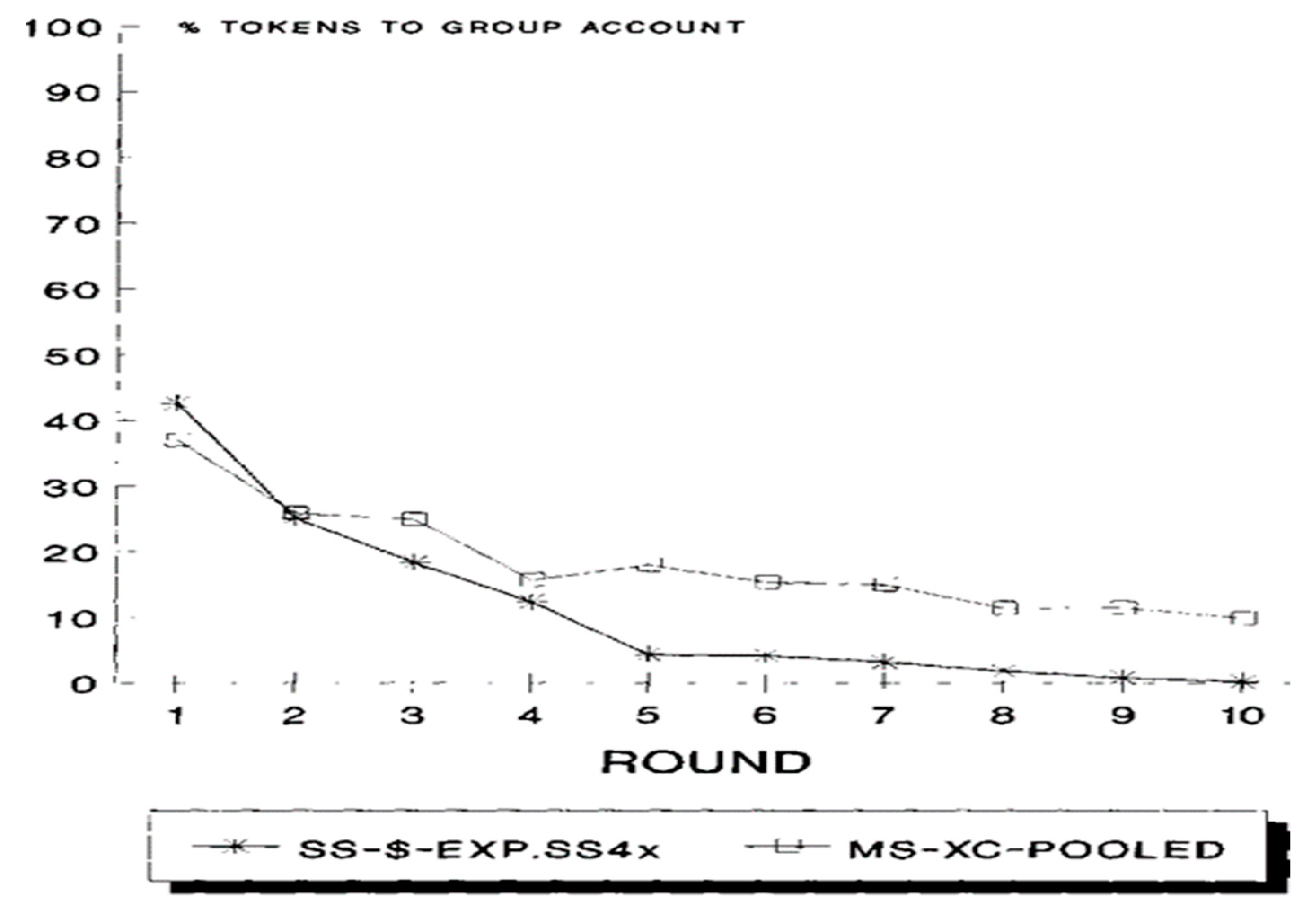
3.4. The Public Goods Game
Comparison with Experimental Results
3.5. Trust Game
Comparison with Experimental Results
4. Discussion
4.1. The Golden Ratio as a Point of Harmony
4.2. Concluding Remarks
Funding
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Nash, J. Equilibrium points in n-person games. Proc. Natl. Acad. Sci. USA 1950, 36, 48–49. [Google Scholar] [CrossRef] [PubMed]
- Nash, J. Non-Cooperative Games. Ann. Math. 1951, 54, 286–295. [Google Scholar] [CrossRef]
- Osborne, M.J. An Introduction to Game Theory; Oxford University Press: Oxford, UK, 2004. [Google Scholar]
- Selten, R. Reexamination of the perfectness concept for equilibrium points in extensive games. Int. J. Game Theory 1975, 4, 25–55. [Google Scholar] [CrossRef]
- Van Damm, E. Strategic Equilibrium. Handb. Game Theory Econ. Appl. 2002, 3, 1521–1596. [Google Scholar]
- Samuelson, P.A. The pure theory of public expenditure. Rev. Econ. Stat. 1954, 36, 387–389. [Google Scholar] [CrossRef]
- Barry, B.; Hardin, R. (Eds.) Rational Man and Irrational Society; Sage: Beverly Hills, CA, USA, 1982. [Google Scholar]
- Ledyard, J. Public goods: A survey of experimental research. In Handbook of Experimental Economics; Kagel, J., Roth, A., Eds.; Princeton University Press: Princeton, NJ, USA, 1995; pp. 253–279. [Google Scholar]
- Fehr, E.; Fischbacher, U. The nature of human altruism. Nature 2003, 425, 785–791. [Google Scholar] [CrossRef] [PubMed]
- Gardner, R.; Elinor, O.; Walker, J.M. The Nature of Common-Pool Resource Problems. Ration. Soc. 1990, 2, 335–358. [Google Scholar] [CrossRef]
- Keser, C.; Gardner, R. Strategic behavior of experienced subjects in a common pool resource game. Int. J. Game Theory 1999, 28, 241–252. [Google Scholar] [CrossRef]
- Berg, J.; Dickhaut, J.; McCabe, K. Trust, reciprocity, and social history. Games Econ. Behav. 1995, 10, 122–142. [Google Scholar] [CrossRef]
- Ortmann, A.; Fitzgerald, J.; Boeing, C. Trust, reciprocity, and social history: A re-examination. Exp. Econ. 2000, 3, 81–100. [Google Scholar] [CrossRef]
- McCabe, K.A.; Smith, V.L. A Comparison of naïve and sophisticated subject behavior with game theoretic predictions. Proc. Natl. Acad. Sci. USA 2000, 97, 3777–3781. [Google Scholar] [CrossRef]
- Güth, W.; Schmittberger, R.; Schwartze, B. An experimental analysis of Ultimatum games. J. Econ. Behav. Organ. 1982, 3, 367–388. [Google Scholar] [CrossRef]
- Rubinstein, A. Perfect equilibrium in a bargaining model. Econometrica 1982, 50, 97–109. [Google Scholar] [CrossRef]
- Stahl, I. Bargaining Theory; Stockholm School of Economics: Stockholm, Sweden, 1972. [Google Scholar]
- Fehr, E.; Schmidt, K.M. A theory of fairness, competition, and cooperation. Q. J. Econ. 1999, 114, 817–868. [Google Scholar] [CrossRef]
- Bolton, G.E.; Ockenfels, A. ERC: A theory of equity, reciprocity, and competition. Am. Econ. Rev. 2000, 90, 166–193. [Google Scholar] [CrossRef]
- Capraro, V. A model of human cooperation in social dilemmas. PLoS ONE 2013, 8, e72427. [Google Scholar] [CrossRef] [PubMed]
- Kirchsteiger, G. The role of envy in ultimatum games. J. Econ. Behav. Org. 1994, 25, 373–389. [Google Scholar] [CrossRef]
- Page, K.M.; Nowak, M.A. Empathy leads to fairness. Bull. Math. Biol. 2002, 64, 1101. [Google Scholar] [CrossRef]
- Nowak, M.A.; Page, K.M.; Sigmund, K. Fairness versus reason in the ultimatum game. Science 2000, 289, 1773–1775. [Google Scholar] [CrossRef]
- Suleiman, R. Economic harmony: An epistemic theory of economic interactions. Games 2017, 8, 2. [Google Scholar] [CrossRef]
- Suleiman, R. On gamesmen and fairmen: Explaining fairness in noncooperative bargaining games. R. Soc. Open Sci. 2018, 5, 171709. [Google Scholar] [CrossRef] [PubMed]
- Kahneman, D.; Knetsch, J.L.; Thaler, R.H. Fairness and the assumptions of economics. J. Bus. 1986, 59, 285–300. [Google Scholar] [CrossRef]
- Suleiman, R. Expectations and fairness in a modified ultimatum game. J. Econ. Psychol. 1996, 17, 531–554. [Google Scholar] [CrossRef]
- Oosterbeek, H.; Sloof, R.; Van de Kuilen, G. Cultural differences in ultimatum game experiments: Evidence from a meta-analysis. J. Exp. Econ. 2004, 7, 171–188. [Google Scholar] [CrossRef]
- Henrich, J.; Boyd, R.; Bowles, S.; Camerer, C.; Fehr, E.; Gintis, H.; McElreath, R.; Alvard, M.; Barr, A.; Ensminger, J.; et al. “Economic man” in cross-cultural perspective: Behavioral experiments in 15 small-scale societies. Behav. Brain Sci. 2005, 28, 795–855. [Google Scholar] [CrossRef] [PubMed]
- Dunlap, R.A. The Golden Ratio and Fibonacci Numbers; World Scientific: Singapore, 1997. [Google Scholar]
- Livio, M. The Golden Ratio: The Story of Phi, the World’s Most Astonishing Number; Broadway Books: New York, NY, USA, 2002. [Google Scholar]
- Liua, J.P.; Chow, S.C. A two one-sided tests procedure for assessment of individual bioequivalence. J. Biopharm. Stat. 1997, 7, 49–61. [Google Scholar] [CrossRef] [PubMed]
- Fehr, E.; Gächter, S. Altruistic punishment in humans. Nature 2002, 415, 137–140. [Google Scholar] [CrossRef]
- O’Gorman, R.; Henrich, J.; Van Vugt, M. Constraining free riding in public goods games: Designated solitary punishers can sustain human cooperation. Proc. R. Soc. B 2009, 276, 323–329. [Google Scholar] [CrossRef]
- Samid, Y.; Suleiman, R. Effectiveness of coercive and voluntary institutional solutions to social dilemmas. In New Issues and Paradigms in Research on Social Dilemmas; Biel, A., Eek, D., Garling, T., Gustafsson, M., Eds.; Springer: Berlin, Germany, 2008; pp. 124–141. [Google Scholar]
- Ostrom, E. Building Trust to Solve Commons Dilemmas: Taking Small Steps to Test an Evolving Theory of Collective Action. In Games, Groups, and the Global Good; Levin, S., Ed.; Springer Series in Game Theory (The Official Series of the Game Theory Society); Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Brandt, H.; Hauert, C.; Sigmund, K. Punishment and reputation in spatial public goods games. Proc. R. Soc. Lond. B 2003, 270, 1099–1104. [Google Scholar] [CrossRef]
- Cong, R.; Wu, B.; Qiu, Y.; Wang, L. Evolution of Cooperation Driven by Reputation-Based Migration. PLoS ONE 2012, 7, e35776. [Google Scholar] [CrossRef]
- Schuster, S. A new solution concept for the ultimatum game leading to the golden ratio. Sci. Rep. 2017, 7, 5642. [Google Scholar] [CrossRef] [PubMed]
- Neelin, J.; Sonnenschein, H.; Spiegel, M. A further test of noncooperative bargaining theory. Am. Econ. Rev. 1988, 78, 824–836. [Google Scholar]
- Binmore, K.; McCarthy, J.; Pontic, G.; Samuelson, L.; Shaked, A. A backward induction experiment. J. Econ. Theory 2002, 104, 48–88. [Google Scholar] [CrossRef]
- Ochs, J.; Roth, A.E. An experimental study of sequential bargaining. Am. Econ. Rev. 1989, 79, 355–384. [Google Scholar]
- Weg, E.; Rapoport, A.; Felsenthal, D.S. Two-person bargaining behavior in fixed discounting factors games with infinite horizon. Games Econ. Behav. 1990, 2, 76–95. [Google Scholar] [CrossRef]
- Camerer, C.F.; Johnson, E.J.; Rymon, T.; Sen, S. Cognition and framing in sequential bargaining for gains and losses. In Frontiers of Game Theory; Binmore, K., Kirman, A., Tani, P., Eds.; MIT Press: Cambridge, MA, USA, 1993; pp. 27–48. [Google Scholar]
- Johnson, E.J.; Camerer, C.; Sen, S.; Rymon, T. Detecting failures of backward induction: Monitoring information search in sequential bargaining. J. Econ. Theory 2002, 104, 16–47. [Google Scholar] [CrossRef]
- Weg, E.; Zwick, R. Toward the settlement of the fairness issues in ultimatum games: A bargaining approach. JEBO 1994, 24, 19–34. [Google Scholar]
- Spiegel, M.; Currie, J.; Sonnenschein, H.; Sen, A. Understanding when agents are fairmen or gamesmen. Games Econ. Behav. 1994, 7, 104–115. [Google Scholar] [CrossRef]
- Binmore, K.; Shaked, A.; Sutton, J. Testing noncooperative bargaining theory: A preliminary study. Am. Econ. Rev. 1985, 75, 1178–1780. [Google Scholar]
- Dawes, R.M. Social Dilemmas. Annu. Rev. Psychol. 1980, 31, 69–193. [Google Scholar] [CrossRef]
- Hardin, G.R. The tragedy of the commons. Science 1968, 162, 1243–1248. [Google Scholar] [CrossRef] [PubMed]
- Harrison, G.; Hirshleifer, J. An experimental evaluation of weakest-link/best-shot models of public goods. J. Public Econ. 1989, 97, 201–225. [Google Scholar] [CrossRef]
- Rapoport, A.; Budescu, D.V.; Suleiman, R. Sequential requests from randomly distributed shared resources. J. Math. Psychol. 1993, 37, 241–265. [Google Scholar] [CrossRef]
- Budescu, D.V.; Au, W.; Chen, X. Effects of protocol of play and social orientation in resource dilemmas. J. Organ. Behav. Hum. Dec. 1997, 69, 179–193. [Google Scholar] [CrossRef]
- Budescu, D.V.; Au, W. A model of sequential effects in common pool resource dilemmas. J. Behav. Decis. Mak. 2002, 15, 37–63. [Google Scholar] [CrossRef]
- Suleiman, R.; Budescu, D.V. Common pool resource (CPR) dilemmas with incomplete information. In Games and Human Behavior; Budescu, D.V., Erev, I., Zwig, R., Eds.; Lawrence Erlbaum Associates, Inc.: Hillsdale, NJ, USA, 1999; pp. 387–410. [Google Scholar]
- Budescu, D.V.; Rapoport, A.; Suleiman, R. Common pool resource dilemmas under uncertainty: Qualitative tests of equilibrium solutions. Games Econ. Behav. 1995, 10, 171–201. [Google Scholar] [CrossRef]
- Budescu, D.V.; Suleiman, R.; Rapoport, A. Positional order and group size effects in resource dilemmas with uncertain resources. J. Organ. Behav. Hum. Dec. 1995, 61, 225–238. [Google Scholar] [CrossRef]
- Rapoport, A. Public goods and the MCS experimental paradigm. Am. Political Sci. Rev. 1985, 79, 48–155. [Google Scholar] [CrossRef]
- Van de Kragt, A.J.C.; Orbell, J.M.; Dawes, R.M. The minimal contributing set as a solution to public goods problems. Am. Political Sci. Rev. 1983, 77, 112–122. [Google Scholar] [CrossRef]
- Isaac, R.M.; Walker, J.M.; Williams, A.W. Group size and the voluntary provision of public goods: Experimental evidence utilizing large groups. J. Public Econ. 1994, 54, 1–36. [Google Scholar] [CrossRef]
- Isaac, M.; Walker, J. Group size effects in public goods provision: The voluntary contribution mechanism. Q. J. Econ. 1988, 103, 179–200. [Google Scholar] [CrossRef]
- Cox, J.C. How to identify trust and reciprocity. Games Econ. Behav. 2004, 46, 260–281. [Google Scholar] [CrossRef]
- Pillutla, M.M.; Malhotra, D.; Murnigham, J.K. Attributions of trust and the calculus of reciprocity. J. Exp. Soc. Psychol. 2003, 39, 448–455. [Google Scholar] [CrossRef]
- Bchir, M.A.; Rozan, A.; Willinger, M. Does higher trust lead to higher performance? An experimental investigation. Econ. Bull. 2012, 32, 1873–1877. [Google Scholar]
- Johnson, N.D.; Mislin, A.A. Trust games: A meta-analysis. J. Econ. Psychol. 2011, 32, 865–889. [Google Scholar] [CrossRef]
- Smith, V.L.; Wilson, B.J. HUMANOMICS: Moral Sentiments and the Wealth of Nations for the Twenty-First Century; Cambridge University Press: Cambridge, MA, USA, 2019. [Google Scholar]
- Smith, V.L. Trust, Reciprocity, and Social History: New Pathways of Learning When Max U (Own Reward) Fails Decisively; ESI Working Paper; Chapman University, Economic Science Institute: Orange, CA, USA, 2020; pp. 20–28. [Google Scholar]
- Smith, A. (1759; 1853): Or, an Essay towards an Analysis of the Principles by Which Men Naturally Judge the Conduct and Character, First of Their Neighbors and Then of Themselves. With a Biographical Critical Memoir of the Author, by Dugald Stewart. London: Henry, G. Bohm, Second Edition. Available online: http:/Ioll.libertyfund.org/titles/2620 (accessed on 15 February 2022).
- Harsanyi, J.C.; Selten, R.A. General Theory of Equilibrium Selection in Games; MIT-Press: Cambridge, MA, USA, 1988. [Google Scholar]
- Hoftman, E.; McCabe, K.; Shachat, K.; Smith, V. Preferences, property rights and anonymity in bargaining games. Games Econ. Behav. 1994, 7, 346–380. [Google Scholar] [CrossRef]
- Forsythe, R.; Horowitz, J.L.; Savin, N.E.; Sefton, M. Fairness in simple bargaining experiments. Games Econ. Behav. 1994, 6, 347–369. [Google Scholar] [CrossRef]
- Harrison, G.W.; McCabe, K.A. Expectations and fairness in a simple bargaining experiment. Int. J. Game Theory 1996, 25, 303–327. [Google Scholar] [CrossRef]
- Jasso, G. Homans and the study of justice. In History, Theory, and Method; Treviño, A.J., Homans, G.C., Eds.; Paradigm Press: Boulder, CO, USA, 2006; pp. 203–227. [Google Scholar]
- Jasso, G. Theoretical unification in justice and beyond. Soc. Justice Res. 2007, 20, 336–371. [Google Scholar] [CrossRef]
- Klar, A.J.S. Fibonacci’s flowers. Nature 2000, 417, 595. [Google Scholar] [CrossRef]
- Mitchison, G.J. Phyllotaxis and the Fibonacci series. Science 1977, 196, 270–275. [Google Scholar] [CrossRef] [PubMed]
- Shechtman, D.; Blech, I.; Gratias, D.; Cahn, J.W. Metallic phase with long-range orientational order and no translational symmetry. Phys. Rev. Lett. 1984, 53, 1951–1954. [Google Scholar] [CrossRef]
- Coldea, R.; Tennant, D.A.; Wheeler, E.M.; Wawrzynska, E.; Prabhakaran, D.; Telling, M.; Habicht, K.; Smeibidl, P.; Kiefer, K. Quantum criticality in an Ising chain: Experimental evidence for emergent E8 symmetry. Science 2010, 327, 177–180. [Google Scholar] [CrossRef] [PubMed]
- Weiss, H.; Weiss, V. The golden mean as clock cycle of brain waves. Chaos Solitons Fract. 2003, 18, 643–652. [Google Scholar] [CrossRef]
- Roopun, A.K.; Kramer, M.A.; Carracedo, L.M.; Kaiser, M.; Davies, C.H.; Traub, R.D.; Kopell, N.J.; Whittington, M.A. Temporal interactions between cortical rhythms. Front. Neurosci. 2008, 2, 145–154. [Google Scholar] [CrossRef]
- Pittard, N.; Ewing, M.; Jevons, C. Aesthetic theory and logo design: Examining consumer response to proportion across cultures. Int. Mark. Rev. 2007, 24, 457–473. [Google Scholar] [CrossRef]
- Suleiman, R. The dark side revealed: A complete relativity theory predicts the content of the universe. Prog. Phys. 2013, 4, 34–40. [Google Scholar]
- Suleiman, R. A model of dark matter and dark energy based on relativizing Newton’s physics. World J. Condens. Matter Phys. 2018, 8, 130–155. [Google Scholar] [CrossRef][Green Version]
- Suleiman, R. Relativizing Newton; Nova Science Publishers: New York, NY, USA, 2019. [Google Scholar]
- Hardy, L. Nonlocality of a single photon revisited. Phys. Rev. Lett. 1994, 73, 2279–2283. [Google Scholar] [CrossRef]
- Otto, H.H. Reciprocity relation between the mass constituents of the universe and Hardy’s quantum entanglement probability. World J. Condens. Matter Phys. 2018, 8, 30–35. [Google Scholar] [CrossRef][Green Version]
- Scarry, E. On Beauty and Being Just; Princeton University Press: Princeton, NJ, USA, 1999. [Google Scholar]
- Wang, T.; Mo, L.; Mo, C.; Tan, L.H.; Cant, J.S.; Zhong, L.; Cupchik, G. Is moral beauty different from facial beauty? Evidence from an fMRI study. Soc. Cogn. Affect. Neurosci. 2015, 10, 814–823. [Google Scholar] [CrossRef] [PubMed]
- Weibull, J.W. Evolutionary Game Theory; MIT Press: Cambridge, MA, USA, 1995. [Google Scholar]
- Newton, J. Evolutionary game theory: A renaissance. Games 2018, 9, 31. [Google Scholar] [CrossRef]
- Smith, V.L. An experimental study of competitive market behavior source. J. Political Econ. 1962, 70, 111–137. [Google Scholar] [CrossRef]
- Smith, V.L. Foreword. In Relativizing Newton; Suleiman, R., Ed.; Nova Science Publishers: New York, NY, USA, 2019. [Google Scholar]
| Study | Pie Size | Discount Factors Equal (δ) | Horizon (T) |
|---|---|---|---|
| 1. Binmore et al. (1985) | 100 pennies. | 0.25 | 2 |
| 2. Neelin et al. (1988) | $5 & $10 | 0.25, 0.5, 0.34 | 2, 3, 5 |
| 3. Binmore et al. (2002) | 100 points payoffs determined by a roulette | 0.2, 0.3, 0.4, 0.5, 0.6, 0.7, 0.8, 0.9 | 2 |
| Unequal,) | |||
| 4. Ochs and Roth (1989) | $30 | (0.4, 0.4), (0.6, 0.4), (0.6, 0.6), (0.4, 0.6) | 2, 3 |
| 5. Weg et al. (1992) | 60 NIS | (0.9, 0.5), (0.67, 0.67), (0.5, 0.9) | Infinite |
| Discount Factor (δ) | ||||||||
|---|---|---|---|---|---|---|---|---|
| Demand | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 |
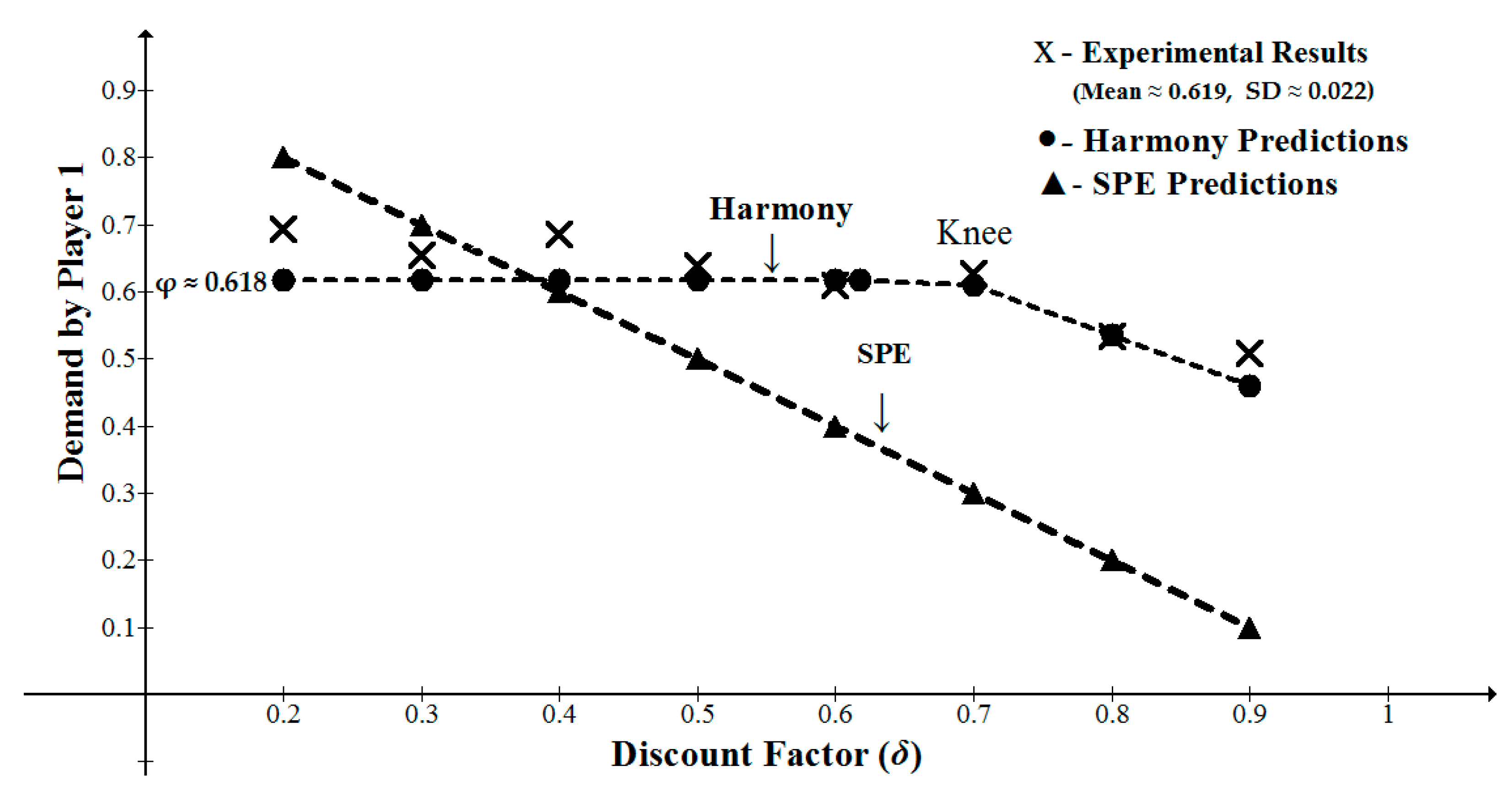
| Experimental | 0.693 | 0.655 | 0.686 | 0.639 | 0.610 | 0.628 | 0.534 | 0.509 |
| Harmony Prediction | 0.618 | 0.618 | 0.618 | 0.618 | 0.618 | 0.567 | 0.506 | 0.444 |
| SPE Prediction | 0.80 | 0.70 | 0.60 | 0.50 | 0.40 | 0.30 | 0.20 | 0.10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suleiman, R. Economic Harmony—A Rational Theory of Fairness and Cooperation in Strategic Interactions. Games 2022, 13, 34. https://doi.org/10.3390/g13030034
Suleiman R. Economic Harmony—A Rational Theory of Fairness and Cooperation in Strategic Interactions. Games. 2022; 13(3):34. https://doi.org/10.3390/g13030034
Chicago/Turabian StyleSuleiman, Ramzi. 2022. "Economic Harmony—A Rational Theory of Fairness and Cooperation in Strategic Interactions" Games 13, no. 3: 34. https://doi.org/10.3390/g13030034
APA StyleSuleiman, R. (2022). Economic Harmony—A Rational Theory of Fairness and Cooperation in Strategic Interactions. Games, 13(3), 34. https://doi.org/10.3390/g13030034





