Private Monitoring and Communication in the Repeated Prisoner’s Dilemma
Abstract
1. Introduction
2. Preliminaries
2.1. Repeated Game
 where . It is clear that each element is smaller than 1. Later I will present an assumption that guarantees each element of the figure is strictly positive when . The time interval is assumed to be small enough so that the probability that a signal is observed more than once within is negligible. Finally, Figure 1 is stated in terms of expected payoffs. One interpretation is that the game ends with probability r and players get payoffs only after it ends.
where . It is clear that each element is smaller than 1. Later I will present an assumption that guarantees each element of the figure is strictly positive when . The time interval is assumed to be small enough so that the probability that a signal is observed more than once within is negligible. Finally, Figure 1 is stated in terms of expected payoffs. One interpretation is that the game ends with probability r and players get payoffs only after it ends.2.2. Repeated Game with Communication
3. The Result
4. Proofs
4.1. Without Communication
4.2. With Communication
4.2.1. Fictitious “Public Monitoring” Game
4.2.2. Truth Telling
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abreu, D.; Milgrom, P.; Pearce, D. Information and timing in repeated partnerships. Econometrica 1991, 59, 1713–1733. [Google Scholar] [CrossRef]
- Aoyagi, M. Collusion in dynamic Bertrand oligopoly with correlated private signals and communication. J. Econom. Theory 2002, 102, 229–248. [Google Scholar] [CrossRef][Green Version]
- Zheng, B. Approximate efficiency in repeated games with correlated private signals. Games Econom. Beh. 2008, 63, 406–416. [Google Scholar] [CrossRef]
- Compte, O. Communication in repeated games with imperfect private monitoring. Econometrica 1998, 66, 597–626. [Google Scholar] [CrossRef]
- Kandori, M.; Matsushima, H. Private observation, communication and collusion. Econometrica 1998, 66, 627–652. [Google Scholar] [CrossRef]
- Awaya, Y.; Krishna, V. On communication and collusion. Am. Econom. Rev. 2016, 106, 285–315. [Google Scholar] [CrossRef]
- Awaya, Y.; Krishna, V. Communication and cooperation in repeated games. Theor. Econom. 2019, 14, 513–553. [Google Scholar] [CrossRef]
- Spector, D. Cheap talk, monitoring and collusion. Mimeo 2017. [Google Scholar]
- Stigler, G.J. A theory of oligopoly. J. Polit. Econom. 1964, 72, 44–61. [Google Scholar] [CrossRef]
- Sugaya, T. Folk Theorem in Repeated Games with Private Monitoring. Mimeo 2021. [Google Scholar] [CrossRef]
- Szolnoki, A.; Perc, M. Reentrant phase transitions and defensive alliances in social dilemmas with informed strategies. EPL Europhys.Lett. 2015, 110, 38003. [Google Scholar] [CrossRef]
- Danku, Z.; Perc, M.; Szolnoki, A. Knowing the past improves cooperation in the future. Sci. Rep. 2019, 9, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Fleckinger, P. Correlation and relative performance evaluation. J. Econom. Theory 2012, 147, 93–117. [Google Scholar] [CrossRef]
- Awaya, Y.; Do, J. Incentives under equal-pay constraint and subjective peer evaluation. Mimeo 2021. [Google Scholar]
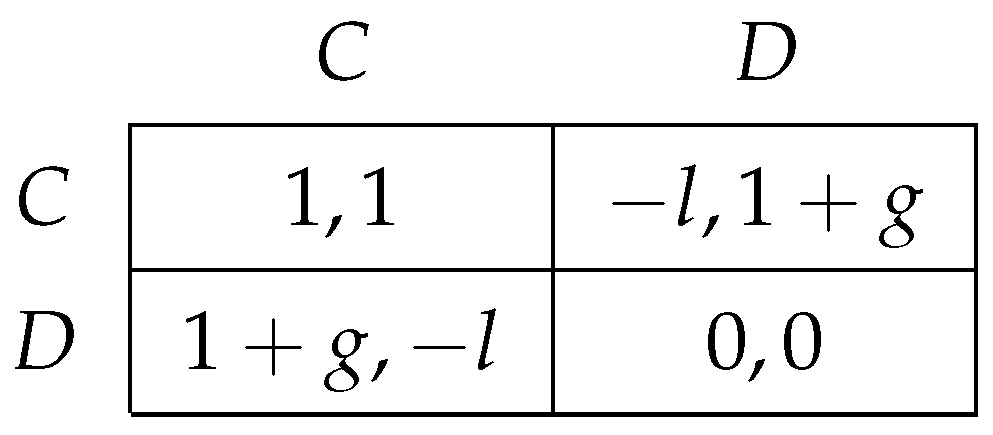
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Awaya, Y. Private Monitoring and Communication in the Repeated Prisoner’s Dilemma. Games 2021, 12, 80. https://doi.org/10.3390/g12040080
Awaya Y. Private Monitoring and Communication in the Repeated Prisoner’s Dilemma. Games. 2021; 12(4):80. https://doi.org/10.3390/g12040080
Chicago/Turabian StyleAwaya, Yu. 2021. "Private Monitoring and Communication in the Repeated Prisoner’s Dilemma" Games 12, no. 4: 80. https://doi.org/10.3390/g12040080
APA StyleAwaya, Y. (2021). Private Monitoring and Communication in the Repeated Prisoner’s Dilemma. Games, 12(4), 80. https://doi.org/10.3390/g12040080




