Simple Voting Games and Cartel Damage Proportioning
Abstract
1. Introduction
2. Illustration
3. Basic Notation and Setup
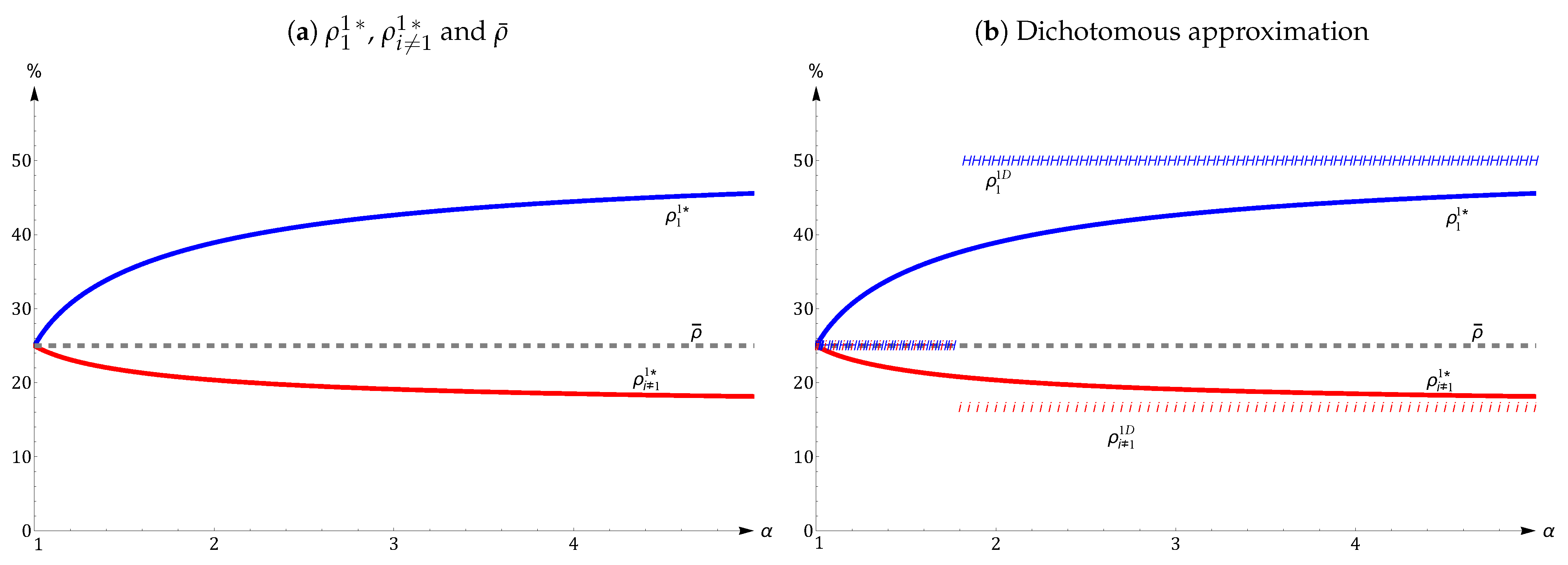
4. Dichotomous Approximation
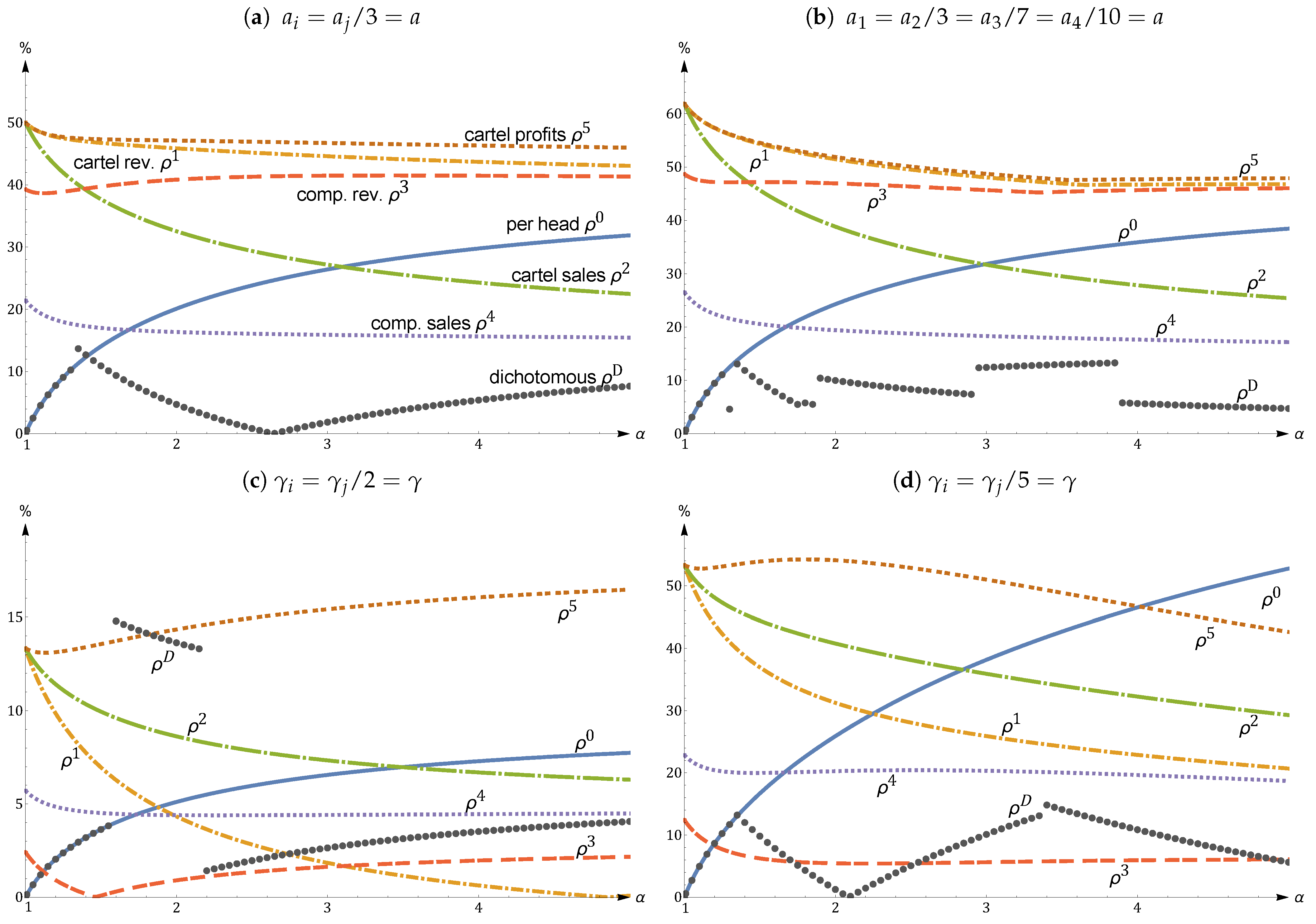
5. Comparisons to Other Heuristics in Linear Market Environments
5.1. Linear Market Model
5.2. Symmetric Firms
5.3. Asymmetric Firms
6. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. All Dichotomous Damage Scenarios with n = 5 Firms
| 1.–19. see Table 4 on p. 8 | 71. | AB, AC, ADE, BCDE | |||
| 20. | AB, AC, AD, AE | 72. | AB, AC, ADE, BDE, CDE | ||
| 21. | AB, AC, AD, AE, BC | 73. | AB, AC, BC, ADE | ||
| 22. | AB, AC, AD, AE, BC, BD | 74. | AB, AC, BC, ADE, BDE | ||
| 23. | AB, AC, AD, AE, BC, BD, BE | 75. | AB, AC, BC, ADE, BDE, CDE | ||
| 24. | AB, AC, AD, AE, BC, BD, BE, CD | 76. | AB, AC, BC, DE | ||
| 25. | AB, AC, AD, AE, BC, BD, BE, CD, CE | 77. | AB, AC, BCD, BCE | ||
| 26. | AB,AC,AD,AE,BC,BD,BE,CD,CE,DE | 78. | AB, AC, BCD, BCE, BDE | ||
| 27. | AB, AC, AD, AE, BC, BD, BE, CDE | 79. | AB, AC, BCD, BCE, BDE, CDE | ||
| 28. | AB, AC, AD, AE, BC, BD, CD | 80. | AB, AC, BCD, BDE | ||
| 29. | AB, AC, AD, AE, BC, BD, CE | 81. | AB, AC, BCD, BDE, CDE | ||
| 30. | AB, AC, AD, AE, BC, BD, CE, DE | 82. | AB, AC, BCDE | ||
| 31. | AB, AC, AD, AE, BC, BD, CDE | 83. | AB, AC, BD, ADE | ||
| 32. | AB, AC, AD, AE, BC, BDE | 84. | AB, AC, BD, ADE, BCE | ||
| 33. | AB, AC, AD, AE, BC, BDE, CDE | 85. | AB, AC, BD, ADE, BCE, CDE | ||
| 34. | AB, AC, AD, AE, BC, DE | 86. | AB, AC, BD, ADE, CDE | ||
| 35. | AB, AC, AD, AE, BCD | 87. | AB, AC, BD, CD, ADE | ||
| 36. | AB, AC, AD, AE, BCD, BCE | 88. | AB, AC, BD, CD, ADE, BCE | ||
| 37. | AB, AC, AD, AE, BCD, BCE, BDE | 89. | AB, AC, BD, CDE | ||
| 38. | AB,AC,AD,AE,BCD,BCE,BDE,CDE | 90. | AB, AC, BD, CE | ||
| 39. | AB, AC, AD, AE, BCDE | 91. | AB, AC, BD, CE, ADE | ||
| 40. | AB, AC, AD, BC, BD, CDE | 92. | AB, AC, BD, CE, DE | ||
| 41. | AB, AC, AD, BC, BD, CE | 93. | AB, AC, BDE | ||
| 42. | AB, AC, AD, BC, BD, CE, DE | 94. | AB, AC, BDE, CDE | ||
| 43. | AB, AC, AD, BC, BDE | 95. | AB, AC, DE | ||
| 44. | AB, AC, AD, BC, BDE, CDE | 96. | AB, AC, DE, BCD | ||
| 45. | AB, AC, AD, BC, BE | 97. | AB, AC, DE, BCD, BCE | ||
| 46. | AB, AC, AD, BC, BE, CDE | 98. | AB, ACD, ACE | ||
| 47. | AB, AC, AD, BC, BE, DE | 99. | AB, ACD, ACE, ADE | ||
| 48. | AB, AC, AD, BC, DE | 100. | AB, ACD, ACE, ADE, BCD | ||
| 49. | AB, AC, AD, BCD, BCE | 101. | AB, ACD, ACE, ADE, BCD, BCE | ||
| 50. | AB, AC, AD, BCD, BCE, BDE | 102. | AB, ACD, ACE, ADE, BCD, BCE, BDE | ||
| 51. | AB, AC, AD, BCD, BCE, BDE, CDE | 103. | AB,ACD,ACE,ADE,BCD,BCE,BDE,CDE | ||
| 52. | AB, AC, AD, BCDE | 104. | AB, ACD, ACE, ADE, BCD, BCE, CDE | ||
| 53. | AB, AC, AD, BCE | 105. | AB, ACD, ACE, ADE, BCD, CDE | ||
| 54. | AB, AC, AD, BCE, BDE | 106. | AB, ACD, ACE, ADE, BCDE | ||
| 55. | AB, AC, AD, BCE, BDE, CDE | 107. | AB, ACD, ACE, ADE, CDE | ||
| 56. | AB, AC, AD, BE | 108. | AB, ACD, ACE, BCD | ||
| 57. | AB, AC, AD, BE, BCD | 109. | AB, ACD, ACE, BCD, BCE | ||
| 58. | AB, AC, AD, BE, BCD, CDE | 110. | AB, ACD, ACE, BCD, BCE, CDE | ||
| 59. | AB, AC, AD, BE, CDE | 111. | AB, ACD, ACE, BCD, BDE | ||
| 60. | AB, AC, AD, BE, CE | 112. | AB, ACD, ACE, BCD, BDE, CDE | ||
| 61. | AB, AC, AD, BE, CE, BCD | 113. | AB, ACD, ACE, BCD, CDE | ||
| 62. | AB, AC, AD, BE, CE, DE | 114. | AB, ACD, ACE, BCDE | ||
| 63. | AB, AC, AD, BE, CE, DE, BCD | 115. | AB, ACD, ACE, BDE | ||
| 64. | AB, AC, ADE | 116. | AB, ACD, ACE, BDE, CDE | ||
| 65. | AB, AC, ADE, BCD | 117. | AB, ACD, ACE, CDE | ||
| 66. | AB, AC, ADE, BCD, BCE | 118. | AB, ACD, BCD, CDE | ||
| 67. | AB, AC, ADE, BCD, BCE, BDE | 119. | AB, ACD, BCDE | ||
| 68. | AB, AC, ADE, BCD, BCE, BDE, CDE | 120. | AB, ACD, BCE | ||
| 69. | AB, AC, ADE, BCD, BDE | 121. | AB, ACD, BCE, CDE | ||
| 70. | AB, AC, ADE, BCD, BDE, CDE | 122. | AB, ACD, CDE | ||
| 123. | AB, ACDE | |
| 124. | AB, AC, ADE, BDE | |
| 125. | AB, ACDE, BCDE | |
| 126. | AB, CD, ACE | |
| 127. | AB, CD, ACE, ADE | |
| 128. | AB, CD, ACE, ADE, BCE | |
| 129. | AB, CD, ACE, ADE, BCE, BDE | |
| 130. | AB, CE, ACE, BDE | |
| 131. | AB, CDE | |
| 132. | ABC, ABD, ABE | |
| 133. | ABC, ABD, ABE, ACD | |
| 134. | ABC, ABD, ABE, ACD, ACE | |
| 135. | ABC, ABD, ABE, ACD, ACE, ADE | |
| 136. | ABC, ABD, ABE, ACD, ACE, ADE, BCD | |
| 137. | ABC, ABD, ABE, ACD, ACE, ADE, BCD, BCE | |
| 138. | ABC, ABD, ABE, ACD, ACE, ADE, BCD, BCE, BDE | |
| 139. | ABC, ABD, ABE, ACD, ACE, ADE, BCD, BCE, BDE, CDE | |
| 140. | ABC, ABD, ABE, ACD, ACE, ADE, BCDE | |
| 141. | ABC, ABD, ABE, ACD, ACE, BCD | |
| 142. | ABC, ABD, ABE, ACD, ACE, BCD, BCE | |
| 143. | ABC, ABD, ABE, ACD, ACE, BCD, BDE | |
| 144. | ABC, ABD, ABE, ACD, ACE, BCD, BDE, CDE | |
| 145. | ABC, ABD, ABE, ACD, ACE, BCDE | |
| 146. | ABC, ABD, ABE, ACD, ACE, BDE | |
| 147. | ABC, ABD, ABE, ACD, ACE, BDE, CDE | |
| 148. | ABC, ABD, ABE, ACD, BCD | |
| 149. | ABC, ABD, ABE, ACD, BCD, CDE | |
| 150. | ABC, ABD, ABE, ACD, BCDE | |
| 151. | ABC, ABD, ABE, ACD, BCE | |
| 152. | ABC, ABD, ABE, ACD, BCE, CDE | |
| 153. | ABC, ABD, ABE, ACD, CDE | |
| 154. | ABC, ABD, ABE, ACDE | |
| 155. | ABC, ABD, ABE, ACDE, BCDE | |
| 156. | ABC, ABD, BCE | |
| 157. | ABC, ABD, ABE, CDE | |
| 158. | ABC, ABD, ACD, BCE | |
| 159. | ABC, ABD, ACD, BCE, BDE | |
| 160. | ABC, ABD, ACD, BCE, BDE, CDE | |
| 161. | ABC, ABD, ACD, BCDE | |
| 162. | ABC, ABD, ACE, ADE | |
| 163. | ABC, ABD, ACE, ADE, BCDE | |
| 164. | ABC, ABD, ACE, BCDE | |
| 165. | ABC, ABD, ACE, BDE | |
| 166. | ABC, ABD, ACE, BDE, CDE | |
| 167. | ABC, ABD, ACDE | |
| 168. | ABC, ABD, ACDE, BCDE | |
| 169. | ABC, ABD, CDE | |
| 170. | ABC, ABDE | |
| 171. | ABC, ABDE, ACDE | |
| 172. | ABC, ABDE, ACDE, BCDE | |
| 173. | ABC, ADE | |
| 174. | ABC, ADE, BCDE | |
| 175. | ABCD, ABCE | |
| 176. | ABCD, ABCE, ABDE | |
| 177. | ABCD, ABCE, ABDE, ACDE | |
| 178. | ABCD, ABCE, ABDE, ACDE, BCDE | |
| 179. | ABCDE |
| 1 | |
| 2 | Another application of simple games and voting power indices outside voting contexts has recently been studied by Kovacic and Zoli [11]. They show that the Penrose-Banzhaf index can improve the prediction of violent conflict in ethnically polarized societies. |
| 3 | Litigants can also strive to find out-of-court settlements, or settle with some firms and take the remaining ones to court. After a settlement “…the [remaining] claim of the injured party should be reduced by the settling infringer’s share of the harm caused …” (Directive 2014/104/EU, recital 51). |
| 4 | Other values fail to satisfy at least one property. For instance, the Banzhaf value and its restriction to simple voting games, the Penrose-Banzhaf index, do not satisfy efficiency; their normalized variants are efficient but violate linearity. They are hence unsuitable for the purpose at hand. [17,18,19] invoke similar reasoning for liability shares in successive torts. |
| 5 | Additional harm stems from deadweight losses: customers who would have made (additional) purchases, and thus would have enjoyed surplus had prices only been , failed to do so. We are unaware of cases in which compensation for this has successfully been claimed and disregard these losses in what follows. |
| 6 | Cartel benefits () reflect normalized relative profit increases of the cartel members. Yet more heuristics are conceivable: for instance, proportioning based on product-specific total overcharge damages would yield shares of which are very similar to heuristic . |
| 7 | It is easiest to think of each firm producing a single good but it is possible to let the set of products be distinct from the set of cartel members N. This can reflect multi-product firms as well as goods produced by non-cartel members. The latter’s price may have increased due to the passing on of cartel margins along a vertical value chain or due to ‘umbrella effects’ that derive from best response behavior of cartel outsiders. |
| 8 | We will assume that once a cartel has formed, other firms become at least implicitly aware of its existence. The cartel outsiders will adapt optimally to the new market environment, as is already anticipated by the cartel members. This and that the latter maximize joint profits are standard assumptions in industrial organization and seem reasonable defaults for the analysis of cartel counterfactuals. However, if there is sufficient evidence that firms pursued alternative objectives in a given cartel case then computations of could be based on these other objectives. |
| 9 | For example, in the counterfactual scenario , the price of product 1 increases to 72.90. Hence the damage caused by coalition is . If either member left S then prices would become competitive, i.e., for . |
| 10 | The analogous table for , i.e., overcharges on product 2, is very similar to Table 2. Respective Shapley shares are . |
| 11 | To fix ideas, think of a crooked architect A who is remunerated in fixed proportion to contract volumes and can define specifications so as to steer procurement for customers towards any building companies B, C, … that are willing to inflate prices. |
| 12 | The easiest way to compute the Shapley value in this scenario is to use Equation (5) of [15].–A qualitatively different scenario could be that all player pairs with cause similar incremental damages, independently of each other. The corresponding mapping with if and otherwise, is not a simple game. Still, it and the resulting Shapley value with and for may constitute a straightforward multi-level rather than dichotomous approximation of causal links and responsibilities when a structural model is difficult to estimate. Simple game approximations may sometimes be refined easily. |
| 13 | The median number of firms in price fixing US cartels is 4 according to analysis by [26], which reflects 329 cases. |
| 14 | Consider, e.g., the European plasterboard cartel. When detected, four companies (BPB PLC, Gebrüder Knauf Westdeutsche Gipswerke KG, Société Lafarge SA and Gyproc Benelux NV) were active in the cartel. They all operated in several countries but their abilities to influence prices differed locally. For instance, the first three firms were large players in Germany and France while Gyproc in France held a market share below . According to the European Commission, “[i]t is clear that the three operators considered it necessary to make Gyproc take part in the exchange as far as the German market was concerned, where that undertaking, which overall was much smaller than the three others, had a significant market share” (see [27] (recital 268)). To do justice to this case would require much deeper analysis, but this description already hints that in the German market all four firms were necessary to cause significant damage (scenario 6), whereas Gyproc’s contribution to harm was rather negligible in France (scenario 4). |
| 15 | See [28] for and [29] for . Our list comprises fewer games because requires in a cartel context but our Appendix A corrects several typos hidden in Baldan’s list. Some games in the list, such as scenario 9, would be considered as improper in the context of voting: they involve disjoint winning coalitions. If we think of A and B as two producers and of C and D as their retailers, damage may plausibly arise already if the producers or the retailers cooperate. If there is little scope for additional marginalization by vertical collusion, makes good sense. |
| 16 | For a duopoly with , cartel participation of either firm is essential for raising prices. Relative responsibilities then are irrespective of cost or demand asymmetries. |
| 17 | and , , can be shown to apply to symmetric firms also for non-linear demand and costs, both under price and quantity competition (cf. Proposition 2 in [15]). |
| 18 | For very high degrees of differentiation, a qualitative assessment might diagnose significant scope to increase already if firm 1 colludes with one, not two other firms, or if all three competitors of 1 collude. The resulting MWC are then with, again, . Extreme differentiation could conceivably lead to a bad approximation by with . This would be incompatible, however, with linear costs and demand for symmetric firms since these imply . |
| 19 | Inefficient firms 3 and 4 each pay 3.1 too much; so each efficient firm, 1 and 2, pays 3.1 too little. |
| 20 | Although the baseline parameters considered in panels (a–f) differ from the ones used in the simulations in [15], the asymmetry-dependent ‘best’ market share heuristics happen to stay unchanged. This suggests that precise parameters are less important for how heuristics perform than the economic asymmetry at hand. |
References
- Maschler, M.; Solan, E.; Zamir, S. Game Theory; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Von Neumann, J.; Morgenstern, O. Theory of Games and Economic Behavior, 3rd ed.; Princeton University Press: Princeton, NJ, USA, 1953. [Google Scholar]
- Taylor, A.D.; Zwicker, W.S. Simple Games; Princeton University Press: Princeton, NJ, USA, 1999. [Google Scholar]
- Shapley, L.S. A value for n-person games. In Contributions to the Theory of Games; Kuhn, H., Tucker, A., Eds.; Princton University Press: Princeton, NJ, USA, 1953; Volume II, pp. 307–317. [Google Scholar]
- Kurz, S.; Napel, S. Dimension of the Lisbon voting rules in the EU Council: A challenge and new world record. Optim. Lett. 2016, 10, 1245–1256. [Google Scholar] [CrossRef]
- Kober, S.; Weltge, S. Improved lower bounds on the dimension of the EU Council’s voting rules. Optim. Lett. 2021, 15, 1293–1302. [Google Scholar] [CrossRef]
- Woeginger, G. The trouble with the second quantifier. 4OR 2021, 19, 157–181. [Google Scholar] [CrossRef]
- Felsenthal, D.S.; Machover, M. The Measurement of Voting Power–Theory and Practice, Problems and Paradoxes; Edward Elgar: Cheltenham, UK, 1998. [Google Scholar]
- Laruelle, A.; Valenciano, F. Voting and Collective Decision-Making; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Napel, S. Voting power. In Oxford Handbook of Public Choice; Congleton, R., Grofman, B., Voigt, S., Eds.; Oxford University Press: Oxford, UK, 2019; Volume 1, Chapter 6; pp. 103–126. [Google Scholar]
- Kovacic, M.; Zoli, C. Ethnic distribution, effective power and conflict. Soc. Choice Welf. 2021, 57, 257–299. [Google Scholar] [CrossRef]
- European Commission. On Certain Rules Governing Actions for Damages Under National Law for Infringements of the Competition Law Provisions of the Member States and of the European Union. Directive 104/EU. 2014. Available online: http://data.europa.eu/eli/dir/2014/1 (accessed on 28 September 2021).
- Schwalbe, U. Haftungsquotierung bei Kartellschäden–Ein Ansatz aus der Theorie Kooperativer Spiele; Working Paper; University of Hohenheim: Stuttgart, Germany, 2013. [Google Scholar]
- Napel, S.; Oldehaver, G. Kartellschadensersatz und Gesamtschuldnerausgleich–Ökonomisch faire Schadensaufteilung mit dem Shapley-Wert. Neue Z. Kartellr. 2015, 3, 135–140. [Google Scholar]
- Napel, S.; Welter, D. Responsibility-Based Allocation of Cartel Damages; Working Paper; University of Bayreuth: Bayreuth, Germany, 2021. [Google Scholar]
- Young, H.P. Monotonic solutions of cooperative games. Int. J. Game Theory 1985, 14, 65–72. [Google Scholar] [CrossRef]
- Dehez, P.; Ferey, S. How to share joint liability: A cooperative game approach. Math. Soc. Sci. 2013, 66, 44–50. [Google Scholar] [CrossRef]
- Dehez, P.; Ferey, S. Multiple causation, apportionment, and the Shapley value. J. Leg. Stud. 2016, 45, 143–171. [Google Scholar]
- Huettner, F.; Karos, D. Liability Situations with Joint Tortfeasors; Working Paper; ESMT Berlin and Maastricht University: Maastricht, The Netherlands, 2017. [Google Scholar]
- Bornemann, B. Cartel Damages: Liability and Settlement. 2018. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3208840 (accessed on 26 October 2020).
- De Roos, N. Examining models of collusion: The market for lysine. Int. J. Ind. Organ. 2006, 24, 1083–1107. [Google Scholar] [CrossRef]
- Budzinski, O.; Ruhmer, I. Merger simulation in competition policy: A survey. J. Compet. Law Econ. 2010, 6, 277–319. [Google Scholar] [CrossRef]
- Baker, D.I. Revisiting history: What have we learned about private antitrust enforcement that we would recommend to others? Loyola Consum. Law Rev. 2004, 16, 379–408. [Google Scholar]
- Vives, X. Oligopoly Pricing: Old Ideas and New Tools; MIT Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Shapley, L.S.; Shubik, M. A method for evaluating the distribution of power in a committee system. Am. Political Sci. Rev. 1954, 48, 787–792. [Google Scholar] [CrossRef]
- Levenstein, M.C.; Suslow, V.Y. Price fixing hits home: An empirical study of US price-fixing conspiracies. Rev. Ind. Organ. 2016, 48, 361–379. [Google Scholar] [CrossRef]
- European Commission. Commission Decision of 27.11.02 Relating to a Proceeding under Article 81 of the EC Treaty. Case COMP/E-1/37.152–Plasterboard. C(2002)4570 Final. 2002. Available online: https://ec.europa.eu/competition/antitrust/cases/dec_docs/37152/37152_72_1.pdf (accessed on 28 September 2021).
- Straffin, P.D. Power indices in politics. In Political and Related Models; Brams, S.J., Lucas, W.F., Straffin, J., Philip, D., Eds.; Springer: New York, NY, USA, 1983; pp. 256–321. [Google Scholar]
- Baldan, J. Properties of Simple Games; Working Paper; Drew University: Madison, NJ, USA, 1992. [Google Scholar]
- Kurz, S. On the inverse power index problem. Optimization 2012, 61, 989–1011. [Google Scholar] [CrossRef][Green Version]
- Federgruen, A.; Pierson, M. The Impact of Mergers and Acquisitions in Price Competition Models; Working Paper; Columbia Business School: New York, NY, USA, 2011. [Google Scholar]
- Davis, P.; Garcés, E. Quantitative Techniques for Competition and Antitrust Analysis; Princeton University Press: Princeton, NJ, USA, 2009. [Google Scholar]
- Freixas, J.; Zwicker, W.S. Weighted voting, abstention, and multiple levels of approval. Soc. Choice Welf. 2003, 21, 399–431. [Google Scholar] [CrossRef]
- Penrose, L.S. The elementary statistics of majority voting. J. R. Stat. Soc. 1946, 109, 53–57. [Google Scholar] [CrossRef]
- Banzhaf, J.F. Weighted voting doesn’t work: A mathematical analysis. Rutgers Law Rev. 1965, 19, 317–343. [Google Scholar]
- Kalai, E.; Samet, D. On weighted Shapley values. Int. J. Game Theory 1987, 16, 205–222. [Google Scholar] [CrossRef]

| Dividing Compensation … | Firms’ Shares of |
|---|---|
| …equally per head () | |
| …by cartel revenue () | |
| …by cartel sales () | |
| …by competitive revenue () | |
| …by competitive sales ( | |
| …by cartel profits ( | |
| …by competitive profits () | |
| …by cartel benefits () |
| S | ||||
|---|---|---|---|---|
| 0 | 0 | 0 | 0 | |
| 28.20 | 28.20 | 28.20 | 0 | |
| 1.67 | 1.67 | 0 | 1.67 | |
| 0.73 | 0 | 0.73 | 0.73 | |
| 37.49 | 36.76 | 35.82 | 9.29 |
| S | ||||
|---|---|---|---|---|
| 0 | 0 | 0 | 0 | |
| 3.67 | 3.67 | 3.67 | 0 | |
| 1.25 | 1.25 | 0 | 1.25 | |
| 1.68 | 0 | 1.68 | 1.68 | |
| 12.20 | 10.52 | 10.95 | 8.53 |
| 1. | AB | 11. | AB, ACD, BCD | ||
| 2. | AB, AC | 12. | AB, AC, AD, BC, BD | ||
| 3. | AB, AC, BC | 13. | AB, BC, CD | ||
| 4. | ABC | 14. | AB, AC, AD, BC | ||
| 5. | ABC, ABD | 15. | ABC, ABD, ACD, BCD | ||
| 6. | ABCD | 16. | AB, AC, AD, BCD | ||
| 7. | AB, AC, BCD | 17. | AB, AC, AD, BC, BD, CD | ||
| 8. | AB, AC, AD | 18. | AC, AD, BC, BD | ||
| 9. | AB, CD | 19. | ABC, ABD, ACD | ||
| 10. | AB, ACD | continued for in Appendix A | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Napel, S.; Welter, D. Simple Voting Games and Cartel Damage Proportioning. Games 2021, 12, 74. https://doi.org/10.3390/g12040074
Napel S, Welter D. Simple Voting Games and Cartel Damage Proportioning. Games. 2021; 12(4):74. https://doi.org/10.3390/g12040074
Chicago/Turabian StyleNapel, Stefan, and Dominik Welter. 2021. "Simple Voting Games and Cartel Damage Proportioning" Games 12, no. 4: 74. https://doi.org/10.3390/g12040074
APA StyleNapel, S., & Welter, D. (2021). Simple Voting Games and Cartel Damage Proportioning. Games, 12(4), 74. https://doi.org/10.3390/g12040074






