1. Introduction
When voters have to decide over issues of common interest, they often find themselves influenced by visible events of debatable direct relevance. Before winning the Democratic nomination in 2008, Obama was endorsed by Oprah Winfrey in Iowa, and the endorsement was one of the most widely covered developments of the campaign—Reference [
1] estimated that Oprah’s endorsement was responsible for approximately 1 million additional votes for Obama. During the 2016 EU referendum in England, Roger Daltrey (lead singer of the iconic rock band The Who) explained in an interview by The Mirror why he thought Brexit was the right thing to do.
1 These examples share the attribute that the information provided is public and
salient, particularly noticeable. In the case of the endorsement to Obama, voters might have seen it as an informative public signal, thinking that Oprah had precise information about the candidate, or as a coordination device, believing that everyone else observed it. Alternatively, they could have paid attention to the endorsement because it was extremely noticeable, as Oprah is a famous celebrity and the endorsement happened right before the election.
This paper uses a laboratory experiment to study salient public information and voters’ decision: the main finding of the experiment is that recency bias largely affects collective decision making.
There are three main explanations for why salient public information could be influential. First, if the information provided is more accurate than voters’ private information, then voters may trust the public source more. Second, public information can serve as a coordination mechanism for voters, who may then rationally choose to disregard their private information and follow the public source even when the latter is not accurate. Finally, salient public information can be influential because of biases or heuristics affecting voters’ decision. This paper analyzes each of these explanations.
Salience of information affects how people focus their limited cognitive resources. Salience bias (or perceptual salience) refers to the fact that individuals focus more on information that is striking and perceptible and ignore information that is less so.
2 One attribute of salience that is particularly effective in politics is recency. According to the recency bias (or availability heuristic), people tend to heavily weight their judgments towards information received more recently, making new opinions biased toward latest news.
3 Public information delivered close to the vote (as Oprah’s endorsement) can be overweighted by voters, who have it readily available in their short-term memory. In real-world situations it is hard to tell why voters respond to salient public information. In particular, it is difficult to separate the importance of the way information is framed from its content, as typically the two come together.
4 This experiment is designed precisely to overcome this challenge.
I begin with the canonical majority rule committee setting, where voting aggregates members’ independent signals about the state of the world [
9,
10]. When in addition a public signal is observed by everyone, voters could use the public information as an information device (depending on accuracy) or as a coordination device (regardless of accuracy). In a recent paper, Reference [
11]—henceforth KV—noticed that if the public signal is more precise than each private signal, then majority rule no longer leads to an equilibrium in which every voter always votes according to the private signal. In this setting, there exist a
responsive equilibrium where voters change their vote as a function of their own private signals with positive probability, for values of the public signal’s accuracy below a certain threshold. Moreover, for any relative accuracy of the two signals, (non-responsive)
conformist equilibria exist where voters coordinate on the basis of the public information without considering their private signals.
To these two possible roles of the public signal—information and coordination—a laboratory experiment superimposes a third element: salience. Subjects face structurally equivalent games which differ in the salience of the information provided. One salience treatment is designed to explicitly capture subjects’ attention, by emphasizing the information with graphics and music. Another treatment changes the relative timing of private and public signals. If subjects behaved according to the equilibrium predictions, their behavior would not change substantially across different salience treatments. If, on the other hand, subjects were to process information according to salience bias, we would expect more votes for the public signal when this is salient.
The experimental results show that subjects’ behavior is responsive to signals’ precision: when the public signal is more accurate than the private, subjects follow it more than when it is less accurate than the private one. Yet, the behavior observed is far from the responsive equilibrium predictions. Subjects’ behavior also contradicts the coordination mechanism: although the conformist equilibrium is not responsive to signals’ relative precision, subjects’ behavior is. Results, instead, point towards the role of salience of information. In particular, the order of message delivery matters: subjects tend to follow the public signal more when it is the most recent signal observed before voting. Recency has a substantive and statistically significant impact on subjects’ behavior: in all the experimental sessions subjects follow the most recent signal (the last signal observed before voting) of the time, regardless of the signal’s precision. Moreover, recency has a striking homogeneous effect: the proportion of votes with the public signal under the recency treatment is greater than the proportion of votes with the public signal when this is displayed before the private one, for almost every subject in the experiment and regardless of signals’ relative accuracy. Interestingly, this result is robust to additional sessions where subjects do not vote in committees over issues of common interest, making individual choices instead. Finding the same behavior in the individual sessions suggests that coordination on public information does not explain subjects’ behavior.
This paper relates to the literature studying salience bias in voters’ decisions. In particular, recency effects have been studied in the context of electoral campaigns. Reference [
12] analyze a sender-receiver game connecting the timing of information release with voter beliefs prior to elections. They formally derive an equilibrium in which fabricated scandals are only released close to the election date, and confirm their equilibrium prediction using data on the release of US presidential scandals. Timing of message delivery in electoral campaigns has also been the subject of field experiments [
13,
14]. Reference [
13] studies the effect of phone calls by volunteers on voter turnout. The experiment shows how calls made during the final days prior to the election are most effective in mobilizing voters, and that the specific content of the conversation is less important than the timing of the call. This paper’s contribution is to provide a controlled experimental test of the role of salient public information on voting. While in field experiments it is hard to isolate the importance of salience from the informational content a message provides, this experimental design overcomes this challenge.
The paper also relates to the literature on committee decision making in voting experiments. Specifically, KV focus on the information and coordination mechanisms with private and public signals and show with a laboratory experiment that voters might be drawn to inefficient conformist equilibria where private information is ignored and voters conform to the public signal. While the main focus of this experiment is the role of salience of the public signals, some of the findings replicate the general result and other specific findings in KV: one treatment considers a public signal that is less precise than voters’ private signals, and another one presents the same public information as a common asymmetric prior instead of a public signal.
The fundamental contribution of this paper is its focus on the role of salience. The treatments are designed to raise the salience of the public message by changing when it is displayed (recency) and by using entertaining music and animations—which makes this experimental design unique. Interestingly, results show that salience treatment effects decayed over time. One interpretation of this result is that salience represents novelty, and as such it should decline with repeated treatments. Furthermore, it is noteworthy that the same salience effects hold when subjects face an individual-decision making problem, without involving groups or pivotality calculations.
Finally, the experiment shows that recency bias is a persistent presence in voters’ decision-making. When making important decisions such as voting, people may focus on features that are easy to process and vivid because available in short-term memory, rather than more informative but less salient ones. This bias in information processing can lead to suboptimal decisions. Knowing this, politicians and media can shift voters’ attention to events that take place at crucial times. As Oprah’s endorsement illustrated, endorsements are strategically timed to be maximally effective. The fact that such signals may be more effective the closer they are to voters’ decision is important, and a novel fining for empirical research on endorsements.
The remainder of the paper is organized as follows. The next section describes the theoretical model and equilibrium predictions. The following sections present experimental design and results. The last section concludes. Proofs, additional data and a copy of the experimental instructions are reported in
Appendix A.
2. The Model
This section formally derives the theoretical predictions that are tested in the laboratory experiment. The same results have been derived in KV and Reference [
15], and have been recently extended in Reference [
16].
Consider a committee that consists of n members, where n is odd. Agents make a collective decision over two alternatives. The state of the world is ∈. Both events are ex ante equally likely: , where is the common prior.
Each agent casts a vote for one of the two alternatives
:
5 we define the individual vote
if the agent votes in favor of alternative
A, and
otherwise. The committee decides by majority voting. Committee members have identical preferences, and payoffs are normalized without loss of generality to 0 or 1. Specifically, I denote by
the utility to voter
i of decision
d in state
and assume
and
for each member of the committee. This means each agent wants the collective decision to match the state of the world.
Agents receive two pieces of information before voting: a private and a public signal. The private signal is denoted by . The probability of the signal matching the state is symmetric across the two states and given by . The public signal is denoted by , with . Private signals are conditionally independent across voters, and the public signal is conditionally independent from the private signals.
Notice that, without public signals, there exists an equilibrium where each voter follows her private signal and—as the number of committee members grows—the probability that the majority takes the correct decision tends to one [
9,
10].
The timing of the game is as follows:
Nature determines the state of the world .
Each voter observes a private signal and the public signal (observed by everyone).
Agents cast their votes and the collective decision d is determined according to the majority of votes.
The true state is revealed and agents receive their payoffs.
A (mixed) voting strategy defines the probability that each agent votes for the public signal , given the realization of both private and public signals. For instance, indicates the probability that i votes for B given that and .
The next section considers how voting behavior changes depending on the signals agents receive. Agent i’s vote depends on the content of the two signals observed, and whether they agree () or disagree (). Notice that the case is equivalent to , because the model is symmetric with respect to the two states A and B. Similarly, is equivalent to .
Equilibrium Analysis
Define by the probability that a voter votes according to the public signal when the two signals agree, and the probability that she votes according to the public signal when private and public signals disagree.
Definition 1. A voting strategy is symmetric if and for every i.
Definition 2. A voting strategy is responsive if for every .
Definition 3. A profile of strategies is more informative than if That is, an equilibrium is more informative than another if it uses more information and gets to the right decision more often. I will first restrict the analysis to the most informative symmetric equilibrium, which can be (but not necessarily is) responsive to private information. In a symmetric responsive equilibrium, voters’ strategies are sensitive to private signals (and all individuals use the same strategy).
The first result shows that, when the public signal’s precision is low enough, in the most informative equilibrium voters always follow the private signal.
Lemma 1. When , the most informative symmetric equilibrium is the unique responsive equilibrium where agents always vote with their private signal.
Proof. All proofs are in the
Appendix A, unless otherwise noted. □
To get the intuition for Lemma 1, consider the decision of a voter. Under the event of pivotality, half of the other voters vote for A and half for B. The voter observes the public and private signals, which differ. Given that the other votes are collectively uninformative, the voter follows the most precise signal between the private and the public. Hence, if the private signal is more precise than the public one, following the public signal is strictly dominated.
In what follows, consider the case in which the public signal is more precise than the private, that is, . The next result shows that there exist a mixed strategy equilibrium in which voters follow the public signal with positive probability (smaller than one), provided that the precision of the public signal is lower than a threshold, , defined below.
Proposition 1 (Symmetric Responsive Equilibrium).
When the most informative symmetric equilibrium is the unique responsive equilibrium where when private and public signals agree; when private and public signals disagree, agents vote according to the public signal with probabilitywhere . The threshold is given by Notice that the voting profile described by Proposition 1 prescribes to vote according to the public signal with positive probability only if . When , Lemma lemma 1 shows that the voter’s dominant strategy is to follow the private signal. The last case to consider is when the public signal precision is above .
Corollary 1. When there exists no symmetric responsive equilibrium, and in the most informative symmetric equilibrium agents always vote with the public signal.
We can summarize the predictions for the most informative symmetric equilibrium as follows:
Besides the most informative symmetric equilibrium, which can be responsive or not, there exist other symmetric equilibria where voters conform to the public signal. Notice that these equilibria are not responsive, that is, agents do not change their vote as a function of their own signal with positive probability.
Proposition 2 (Conformist Equilibrium). There exists a symmetric Bayesian Nash equilibrium in which every agent votes according to (against) the public signal.
Proof. Consider the choice of an individual i. If every other agent votes according to (against) the public signal, agent i is not pivotal and therefore she is indifferent about which alternative to vote for. Thus every agent voting according to (against) the public signal is an equilibrium. □
Conformist equilibria can be very inefficient, especially when the public signal is below the threshold determined above. That is, introducing a public signal may be deleterious because the public signal might be seen as a focal point which makes coordination easier for committee members, thus hindering information aggregation.
In addition to the two symmetric equilibria in Proposition 2, there exist several asymmetric ones where voters conform to the public signal.
6 However, these equilibria do not seem to be very plausible. In particular, as described in the experimental setting below, groups are randomly matched in each period: because it is difficult to coordinate on asymmetric equilibria with random matching, these asymmetric equilibria are very unlikely to emerge.
3. Experimental Design
The experiment is designed to answer two questions. The first asks to what extent subjects’ behavior responds to signals’ precision. In particular, the experiment tests whether subjects vote according to the public signal when they know that it is less precise than their private ones. The first treatment of interest changes the relative precision of the private and public signals. Since there is no evidence of learning in the data (see
Appendix A), I report the results below aggregating over all rounds of the same treatment. The second question asks how salience of public information affects voting behavior. To understand the impact of salience, I create five structurally equivalent games (corresponding to different treatments), in which public information is provided in different ways.
The experiment was organized in ten separate sessions, all held at the Columbia Experimental Laboratory (CELSS). Subjects were registered students, recruited through the laboratory web site. No subject participated in more than one session. Overall, 157 subjects participated in the experiment. The experiment was conducted using the software Z-Tree [
17], and a copy of the instructions is presented in
Appendix A. Each session lasted about one hour, and earnings ranged from
to
, with an average of
(including a
show-up fee).
Each session was comprised of 70 rounds. In every round, participants were randomly matched with each other to form a committee of 5 members.
7 Subjects were told that their group’s task was to find a prize (worth 70 experimental dollars) which was hidden in one of two boxes, one red, one blue. The computer placed the prize in the red box with probability
, and each subject received two pieces of information: a private message and a public message. To make clear that the public message was common knowledge, all public messages were displayed on the two central screens of the laboratory. The private messages were displayed on each subjects’ monitor. After receiving the information, subjects voted for either the red box or the blue box.
8 The alternative that received the majority of votes was selected. After every round, subjects received feedback about the number of votes cast by their group for the red and blue box, whether the group decision was correct or not, and their earnings for the round. Individual payoffs were based on whether the group decision was correct or incorrect: 70 experimental dollars for each correct decision, 10 experimental dollars for a wrong decision. Subjects were paid the sum of their round earnings.
Table 1 shows which sessions had high public signal accuracy (
), and low (
). The accuracy of the private signal was set to
throughout all sessions. The committee size was set to
for the entire experiment. For these parameters, the equilibrium predictions for the symmetric responsive equilibrium are to follow the public signal
of the time when this is more precise than the private (
), and never follow it when
. According to the equilibrium in which subjects coordinate on (against) the public signal, everybody (nobody) follows the public signal when the two signals disagree, even when the public signal is less accurate than the private.
In addition to testing how subject behavior changes with signal relative accuracy, this experiment studies how salience affects voting decision. Salience is defined along two dimensions. The first is recency, or timing relative to voting. I hypothesize that subjects follow more the message that is closer to the vote, because they have the information more readily available in their short-term memory [
18,
19,
20]. The second dimension refers to how information is presented. Information that is visibly stunning is salient, because individuals focus more on items that are striking and perceptible [
21]: as a consequence, subjects should overemphasize the information on which their minds focus.
9 The experimental treatments varying the two dimensions of recency and emphasis are described below.
Recency. This treatment varied whether the public signal was displayed before or after the private signal.
Asymmetric Prior. The least salient way to convey the public message is not to show it at all. This treatment corresponds to the last 10 rounds of each session. In these rounds, an asymmetric prior was provided instead of a symmetric ( prior, and no public signal was displayed. Subjects were told that the computer placed the prize in the blue box with probability (or , depending on the session), and in each round each subject received only a private signal. From a Bayesian standpoint, these ten rounds conveyed the same information as the previous ones: having a symmetric prior and a public signal with accuracy is identical to having an asymmetric prior and no public signal. After receiving the private message, subjects were asked to vote for one of the two boxes, as in the first part of the experiment.
Jingle. This treatment varied the way the public signal was projected on the central screens. In the absence of this treatment, the public signal was displayed with the picture of a blue or red box (as for the private signals projected on subjects’ monitors). With the
jingle treatment, the public message was projected on the central screen with a video displaying a star jumping within an empty, white box, which then became either red or blue. The video was accompanied by a striking soundtrack, and to make the jingle treatment less repetitive, the music theme varied. I used famous music pieces such as Also sprach Zarathustra by Strauss, Eye of the tiger, The final Countdown, Thrift Shop and the Game of Thrones’ soundtrack.
10 I hypothesize that salience of the public signal is increasing in both recency and emphasis.
Subjects in different sessions were presented with the same five treatments.
11 Each subject played thirty rounds with the public signal displayed before the private, and thirty with the private displayed before the public. Among each of these thirty rounds, eight displayed the public message with the jingle, so that it is possible to evaluate the interaction between jingle and recency treatments. I decided to keep the number of jingle rounds small to ensure novelty and subjects’ interest. These conditions (recency, jingle) were randomly selected in every round, a feature designed to keep subjects engaged in the task. The only condition that was not randomly assigned is the asymmetric prior treatment, which consisted in the last ten rounds that every subject played. This design feature was chosen for feasibility, as it would not be possible to switch priors and signal structure in every round.
Table 2 shows the number of rounds for each of the treatments.
4. Results
The first treatment of interest is designed to test whether subjects in committees follow more the public signal when it is more accurate than the private. I begin by aggregating the data across the salience treatments, given that salience is irrelevant according to the theory.
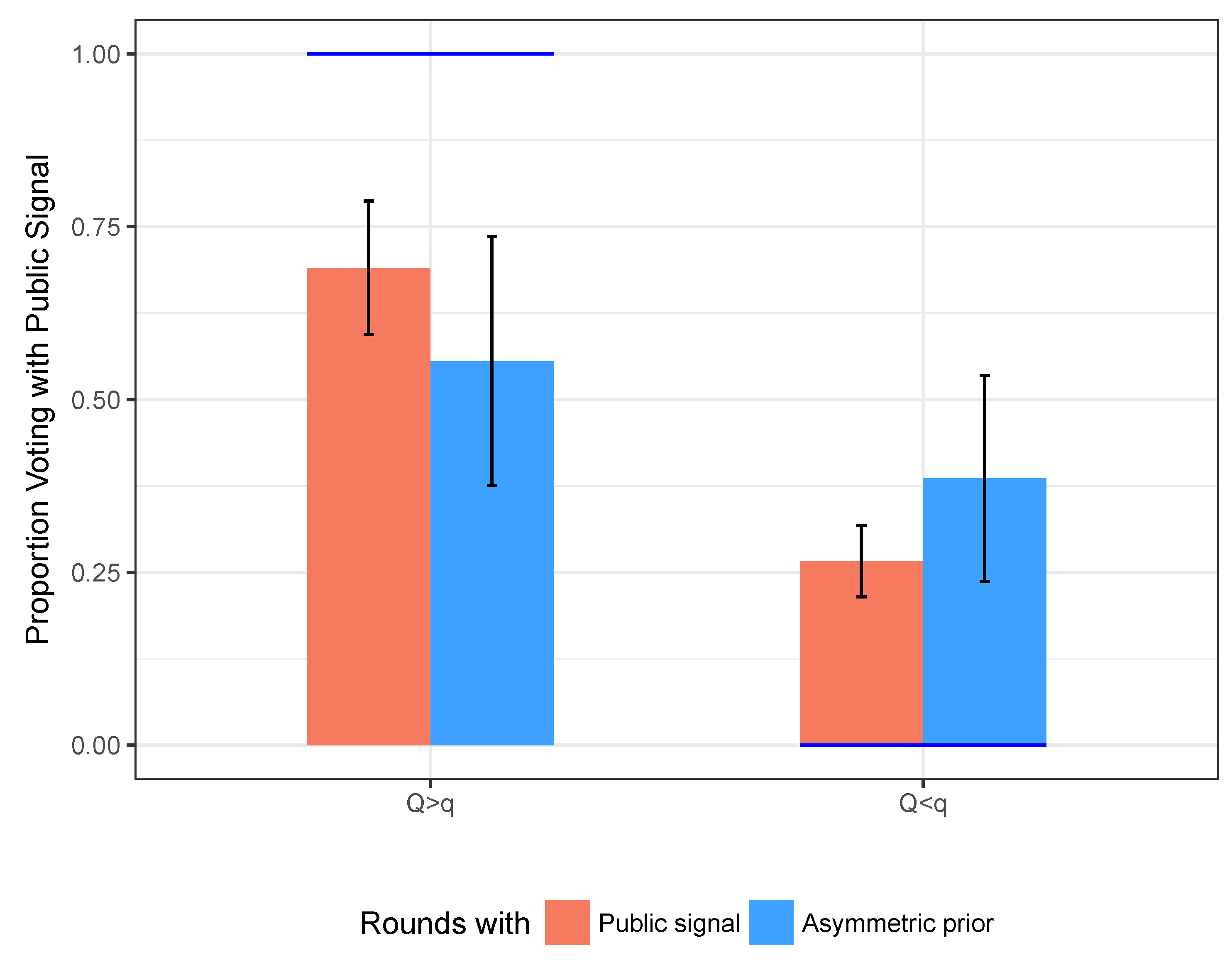
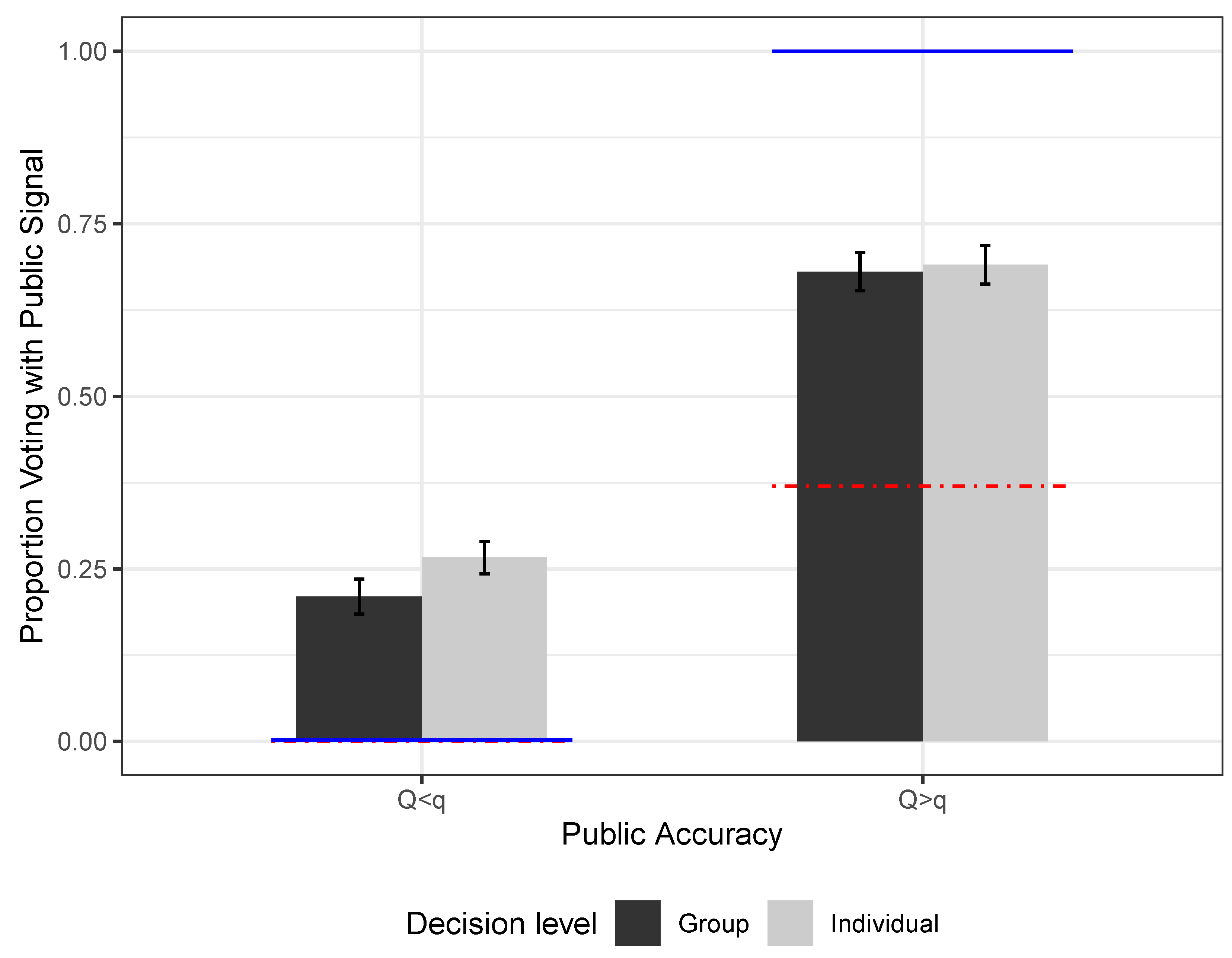
Figure 1 displays the fraction of votes cast according to the public signal when the private and public signals disagree (estimated
), as well as when the two signals agree. The second panel gives us a measure of the extent of pure noise in the experiment.
The first thing to notice is that the treatment effect goes in the expected direction: subjects vote more with the public signal when this is more precise than the private one (
vs.
, statistically significant at any conventional level). However, the observed behavior is far from the symmetric responsive equilibrium predictions (red line in
Figure 1), as well as from the conformist equilibria. In particular, it is worth noting that
of the subjects vote according to the public signal even when this is less precise than the private one, when the two disagree (left column, left plot). When presented with the trivial choice of voting after receiving two identical signals, subjects tend to vote according to both. Nevertheless, even in this case subjects commit mistakes, quantified in the right plot by the distance between the bars and the blue lines (less than
).
Given this result, the mechanism according to which voters follow public signals because of their informativeness seems to lack explanatory power.
12 It might be that subjects are influenced by public information because of conformity, or because of bias in information processing. We know there exist two symmetric conformist equilibria with coordination on (or against) the public signal, and several asymmetric equilibria. However, all of these equilibria are not responsive to the relative precision of the two signals. Since we clearly see that the behavior of voters responds to signals’ relative precision (treatment effect in
Figure 1), all the non-responsive equilibria do not reflect subjects’ behavior.
The results in
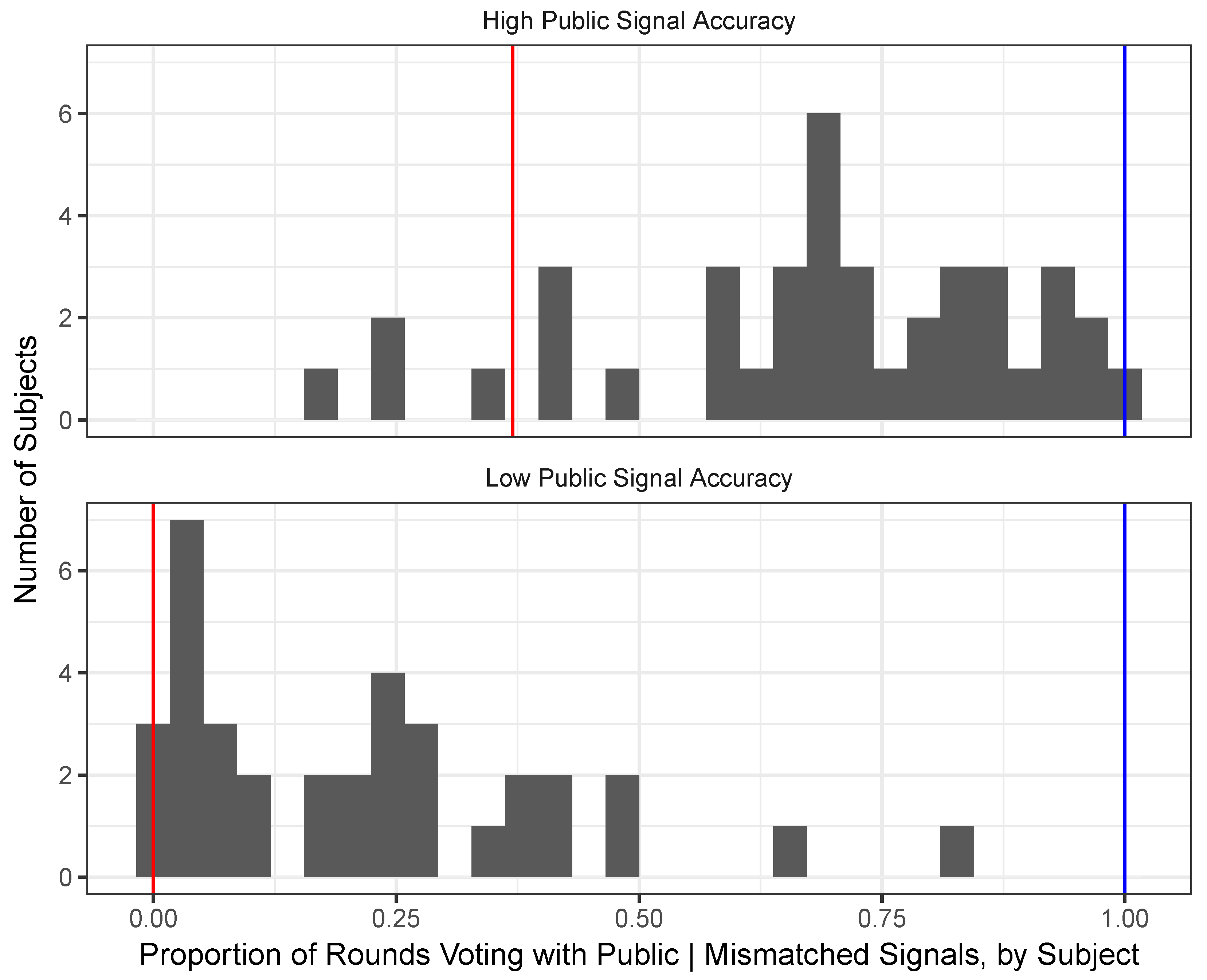
Figure 1 can be disaggregated to shed light on individual behavior.
13 Figure 2 plots the proportion of times each subject votes according to the public signal. We know that in the symmetric responsive equilibrium this proportion should be
when
and zero when
(when the public and private signals disagree). On the other hand, were people playing the conformist equilibrium, the proportion would be close to one for both values of signal precision.
Figure 2 show these equilibrium predictions and subjects’ deviations from them, which are bigger as the distance between the equilibrium predictions and the bars increase. This result again contradicts the coordination motive of players, as more subjects vote following the public signal when its precision is higher (upper graph) than when it is lower than the private one (lower graph).
One concern that could arise is that subjects’ behavior changed over time, approaching the theoretical predictions of the equilibria analyzed.
Figure A9 in
Appendix A analyzes the dynamics of subjects’ behavior over time, showing that there is no convergence to equilibrium predictions as the final rounds approach.
The next section describes how subjects responded to salience of public signals. According to the salience mechanism, framing leads subjects to select a strategy based on the frame itself, even if it is strategically irrelevant.
4.1. Subjects’ Response to Salience
This section reports separately the results for each salience treatment, starting from the asymmetric prior, which is the treatment where the public message is least salient (as it is not displayed at all), and continuing with the more salient treatments (recency and jingle).
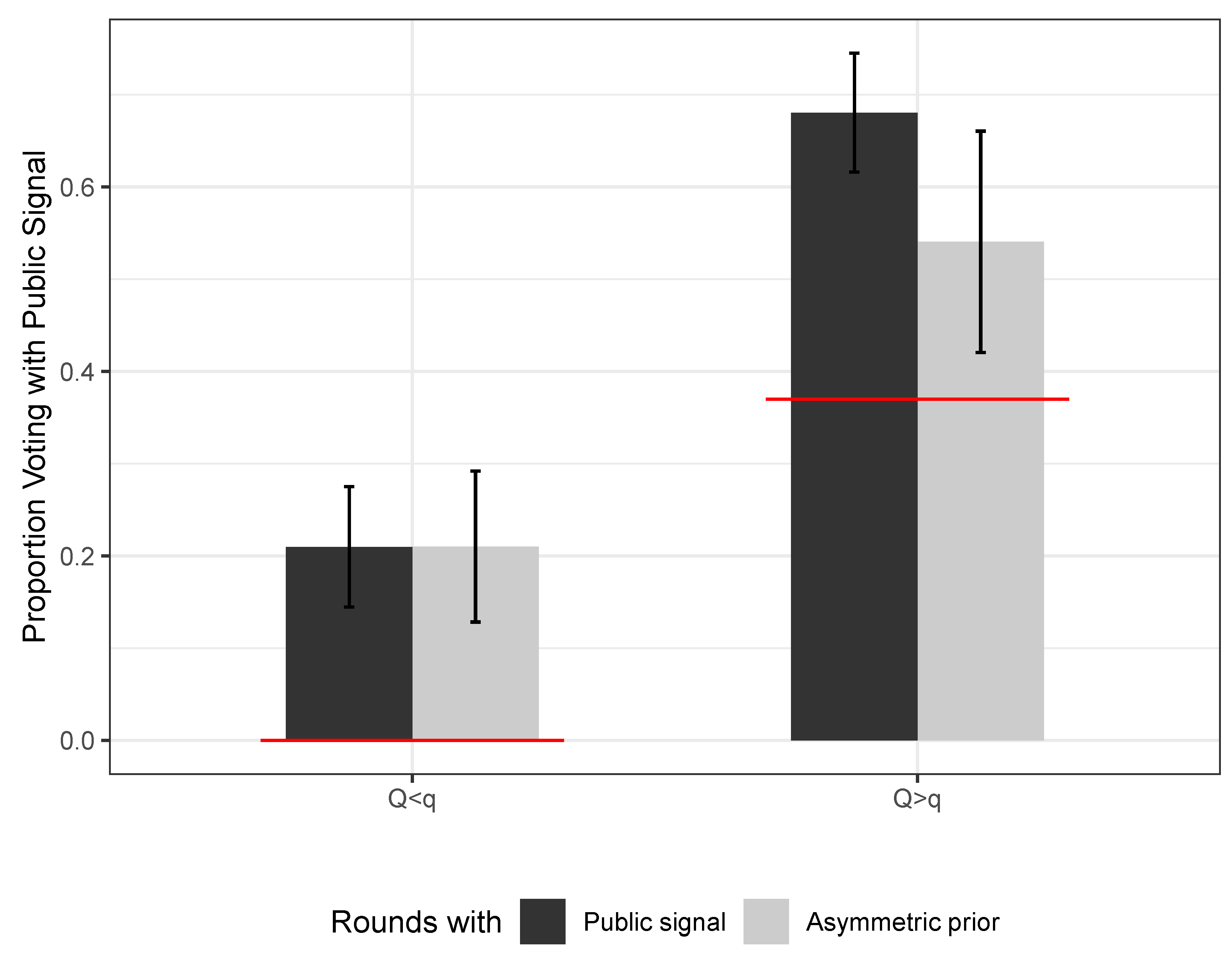
Asymmetric Prior.Figure 3 shows the proportion of votes with the public signal (under mismatch) in the treatment with public signal calculated by aggregating over the other salience treatments vs. the treatment with asymmetric prior.
The left bars correspond to the public signal being less accurate than the private (
), and we see no difference. When
instead, subjects follow the public signal more (as we saw earlier), and the treatment effect of showing the public signal is high and significant: showing the public signal correlates with subjects voting for it
of the times more than when the same signal is conflated in the prior. This difference is significant at the ten percent level. This treatment effect has the same direction of what found in KV,
14 although the magnitude is much smaller. One concern might be that any effect of the asymmetric prior treatment is driven by it being administered during the last ten rounds of each session. I performed the same comparison as in
Figure 3 considering only the last ten rounds of the first part of the experiment, when the public signal was displayed. Even with this reduced sample, there is no difference when the public signal is less precise. When the public signal is more precise, this difference is reduced to 11 percentage points, significant at the ten percent level.
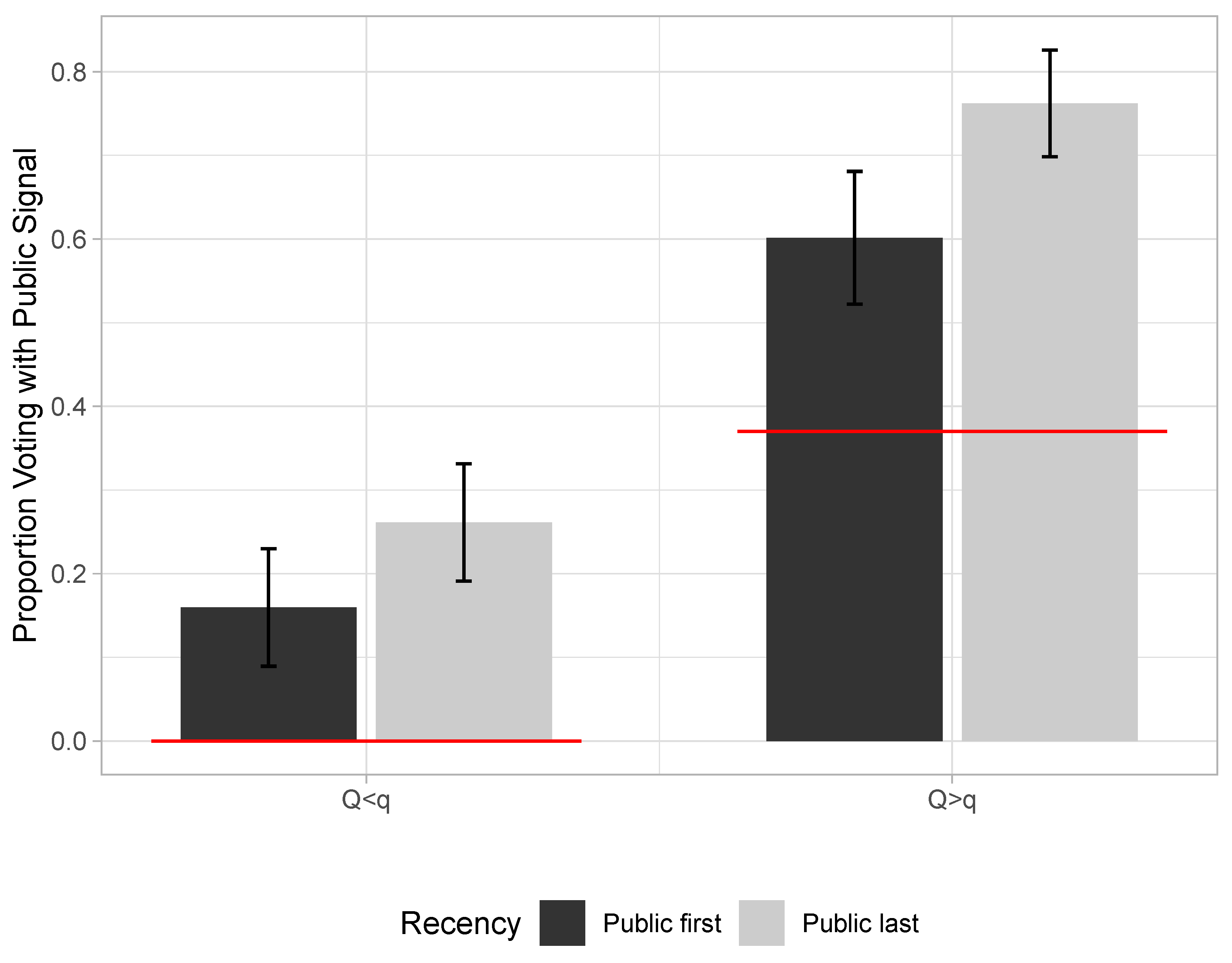
Recency. The recency treatment varied whether the public signal was projected on the central screens of the laboratory before or after the private signals were displayed on the subjects’ monitors. Recency effects were substantively and statistically significant. In particular, when the public signal accuracy is higher (right columns in
Figure 4), there is a
difference between the fraction of times subjects followed the public signal when it was displayed before the private (
) as opposed to after the private (
). When the public signal accuracy is lower than the private (left columns), there is a
difference. Both differences are significant at any conventional level.
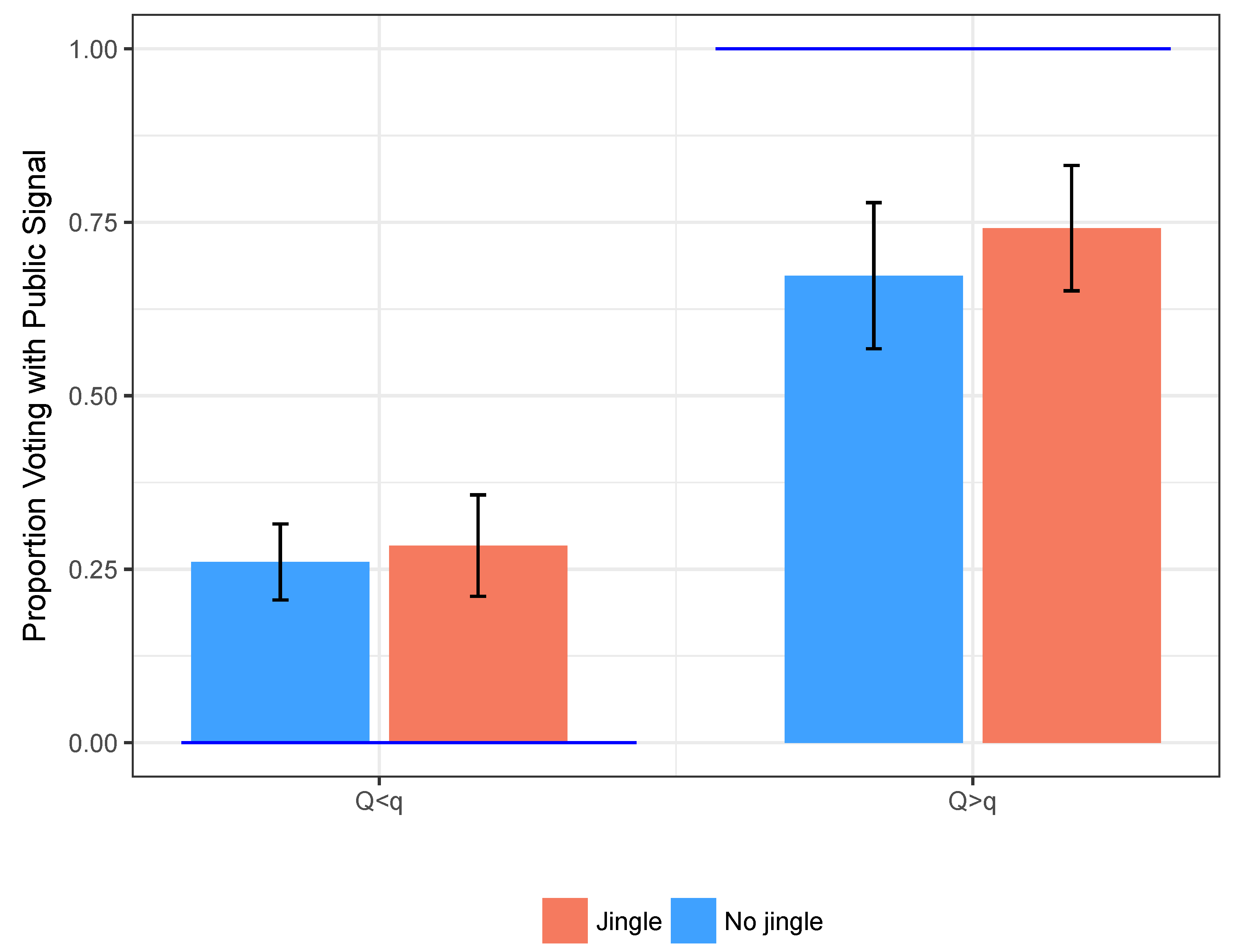
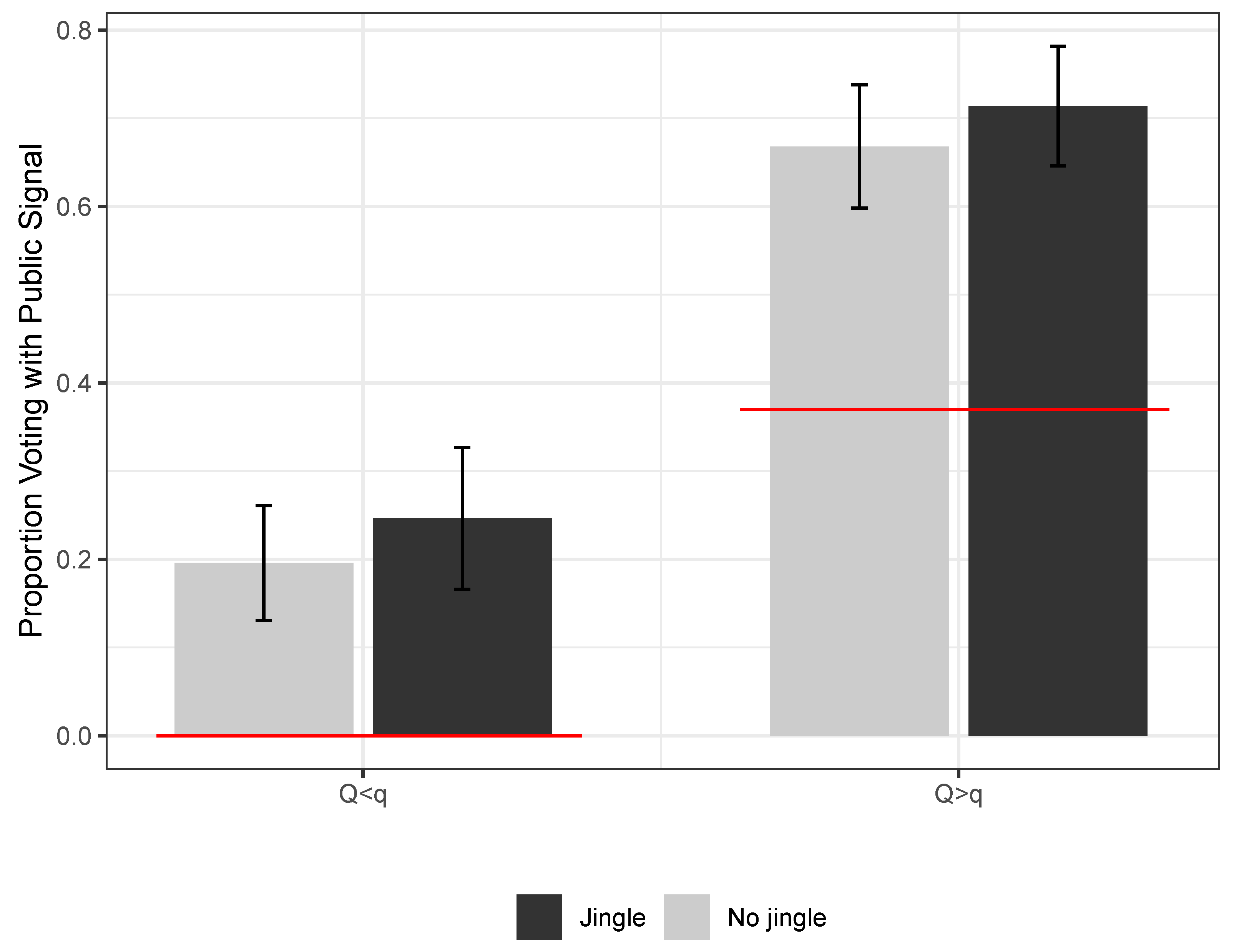
Jingle. For what concerns the jingle treatment effect, the direction is the one expected and it is in line with the recency treatment effect. The magnitude is smaller, as
Figure 5 shows. When the public signal accuracy is higher (right columns in
Figure 5), there is a
difference between the fraction of times subjects followed the public signal when it was displayed before the private (
) as opposed to closer to the vote (
). When the public signal accuracy is lower than the private (left columns), there is a
difference.
Table 3 shows an OLS regression of the probability of following the public signal under mismatch, regressed on the jingle treatment and the recency treatment. When the public signal is presented as a flashy video, subjects vote for it
of the time more. When we interact the jingle with recency, the effect increases to
. Even though the aggregate effect of the jingle is not significant, there is a pattern of response to it: subjects react more to the initial jingles. In particular, if the jingles are shown within the first 15 periods, subjects follow the public signal more than public signal displayed in later periods (
difference,
).
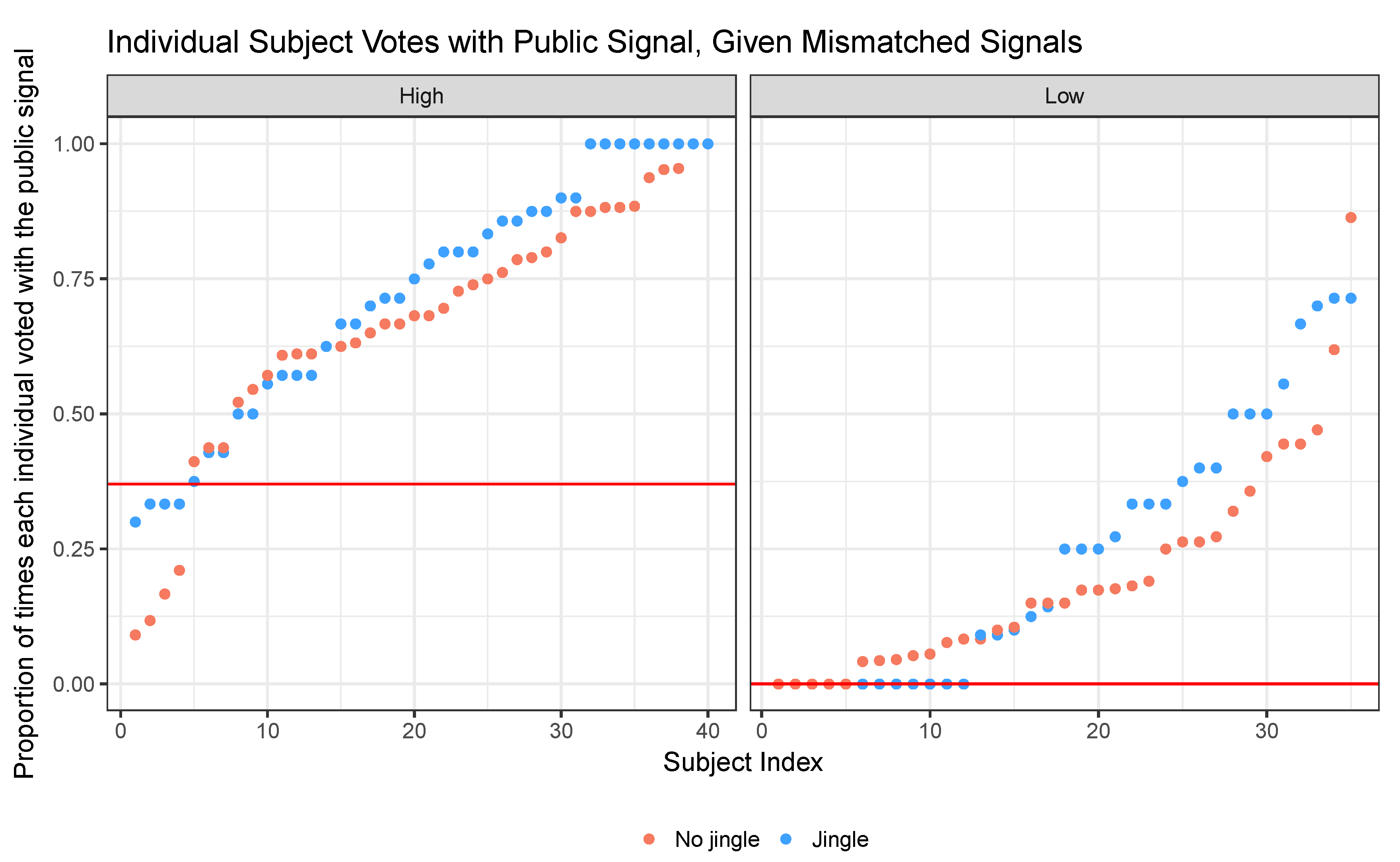
Given the magnitude and significance of the recency treatment effect, the question that arises is whether this effect is homogeneous across subjects.
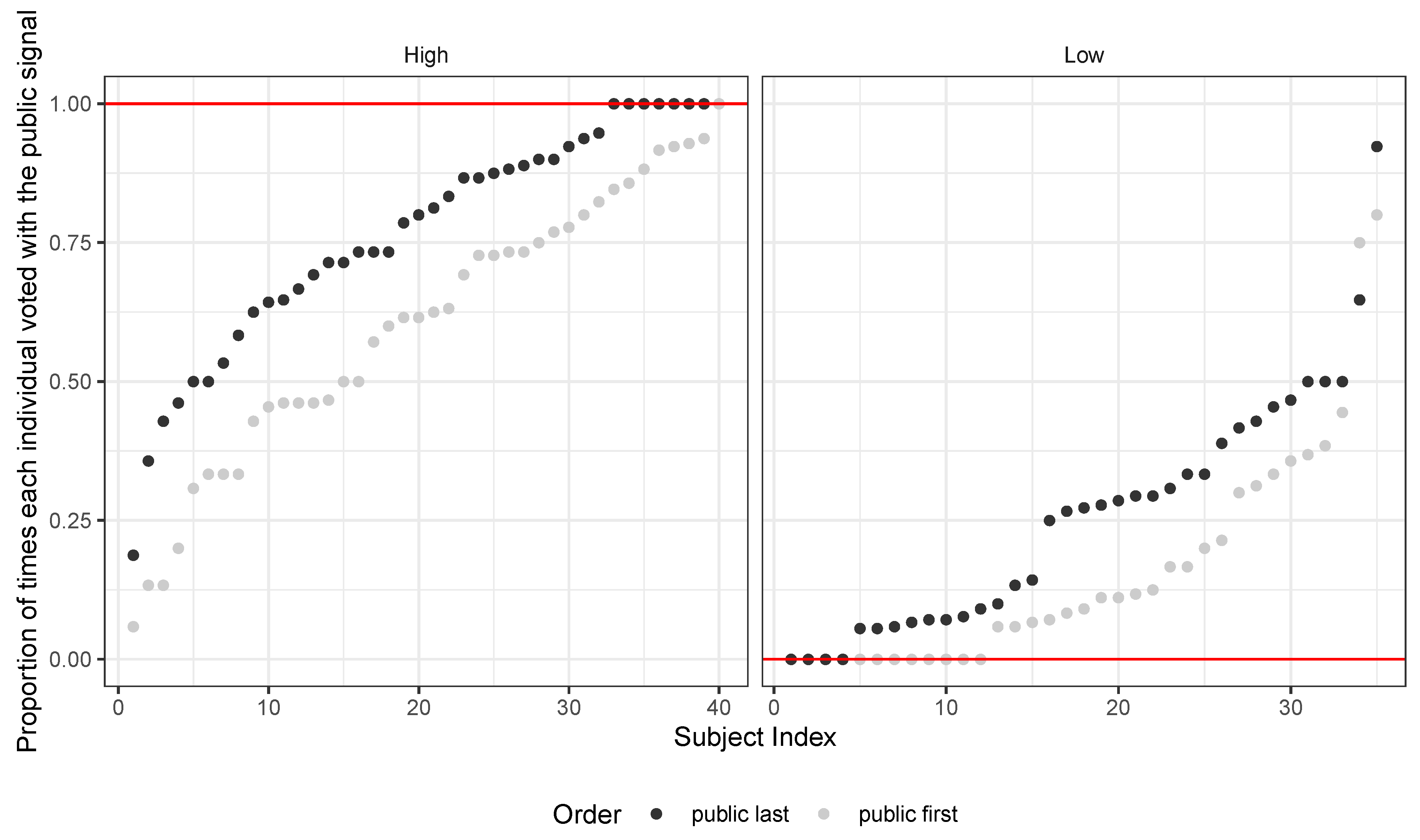
Figure 6 shows the individual treatment effect of recency of the public signal. Each dot represents the proportion of times each subject voted with the public signal. The vertical distance between red and blue dots is the individual average treatment effect of providing a public signal before vs. after the private one. The left panel corresponds to a more precise public signal. Recency of the public signal has a striking homogeneous, positive effect on the proportion of time each individual votes with the public signal.
We can see this effect from the proportion of times each individual voted with the public signal when it is displayed closer to the vote (dark dots), which is always above the same proportion when the public signal is displayed before the private signal (light dots): the “Public Last” (recency) treatment first order stochastically dominates the “Pubic First” treatment.
15Overall, there is a substantial fraction of subjects who always vote according to the most recent signal. In particular, votes match the most recent signal in
of individual decision, which is a remarkable result given that from a theoretical standpoint behavior should not be affected by the time a message is released. Moreover, subjects’ behavior is homogeneous across different sessions.
16 4.2. Individual Treatment Sessions
In the previous section, I show that the way subjects responded to the signals’ relative precision rules out the coordination mechanism, which is a possibility that might arise (see conformity equilibrium in the theory section). Sessions 5–10 were designed to fully control for this coordination mechanism. The structure of these sessions was identical to the previous four, except that subjects were paid for their individual decisions, and were not part of any group. Instructions were given to subjects in the same way as in the first sessions, with the only difference that no groups were mentioned. The absence of groups made the decision much easier, being absent any calculus of pivotality or coordination problem. The decision problem was straightforward, as it only required to compare the relative precision of the signals received: the expected payoff maximizing decision was to always follow the information contained in the more accurate message.
If less subjects followed the public signal in these individual sessions, we would have evidence that subjects used the public signal as a coordination device: voters would conform to the public signal’s content as long as they were in groups, but would stop to conform when the task was individual. If, on the other hand, the fractions of people voting for the public signal were similar in the individual and group treatments, it would be evidence that subjects did not use the public signal as a coordination device.
Aggregate data for these individual sessions show that there is no substantive difference between the fraction of votes cast according to the public signal in the individual task treatment versus the treatment with committees and group decisions (see
Figure 7).
The aggregate data show that, when the public signal is less precise than the private, subjects tend to over-follow the public (the optimal decision is to never vote with the public in both group and individual treatments). The treatment effect of relative signal precision remains in the correct direction even in the individual sessions. The similar results that we observe in the group task and individual task sessions provide evidence against the coordination mechanism in the group treatment. Yet, the fact that individuals vote too little with the public signal when it is more informative than the private is surprising, given the very simple task they are given. This result might be due to the way subjects were exposed to the messages during the experiment. Although preserving the same experimental design used in the first sessions with the group task was the most linear way to compare the two treatment conditions, some subjects might have been confused by receiving a public signal common to everyone in the room, when their payoff was determined uniquely by their decision. Hence, despite very clear instructions, the individual task might have confused some subjects. This confusion presumably did not arise in the group sessions, where the presence of a public signal observed by all subjects was intuitively related to group decision making.
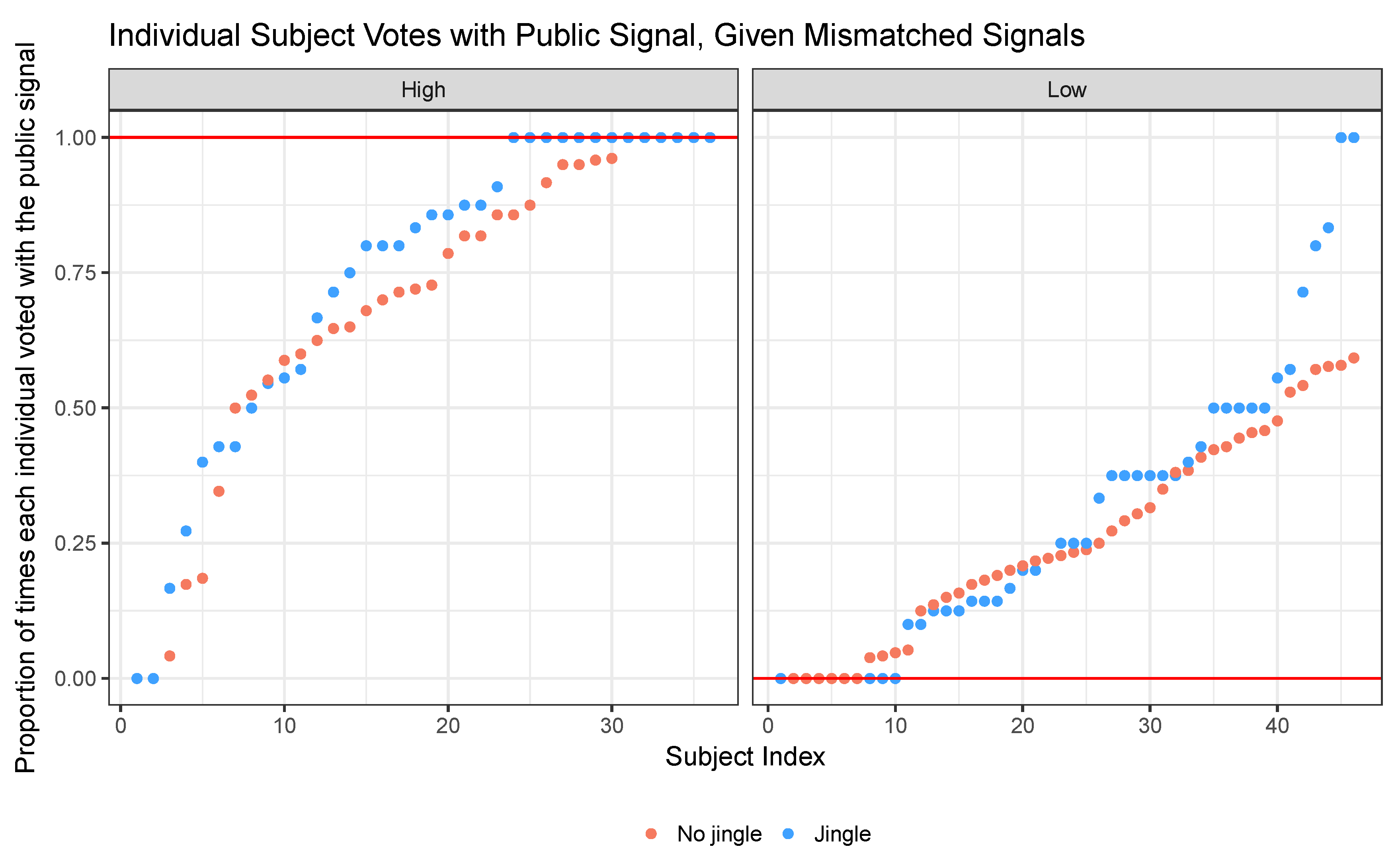
As
Figure 8 shows, individual data for the individual sessions show the same stochastic dominance that we saw in group sessions for the recency treatment. Even for these subjects, recency of the public signal has a homogeneous and positive effect on the proportion of time each individual votes with the public signal. We can see this effect from the distribution of individual votes when the public signal is displayed closer to the vote (dark dots), which stochastically dominates the votes when the public signal is displayed before the private (light dots). Even the jingle has an effect that is similar to the group sessions: when the public signal is presented as a flashy video with salient music, the probability that subjects vote for it is
higher (
p-value lower than
), although when interacted with recency, this effect is not significant anymore (
Table A1 in
Appendix A shows the OLS regression coefficients for the individual sessions). Overall, the individual sessions show that salience treatment effects (particularly in the form of recency) are robust to the nature of the task: whether subjects vote in groups or individually, they are clearly affected by the way the message is presented.
17 5. Conclusions
This paper studies the effect of salient public signals on voting behavior in a majoritarian voting game with common interest. When (in addition to private independent signals) a public signal is observed by everyone, there exist two main equilibria of interest: a symmetric responsive equilibrium, where subjects follow their private signals with positive probability, and a Bayesian equilibrium where subjects coordinate on the information provided by the public signal. Theoretically, subjects’ behavior should not be affected by signals’ salience, as long as the informational content of the signals is the same.
A laboratory experiment tests these theoretical predictions, suggesting several conclusions. First, subjects tend to follow the public signal more than what is predicted by the symmetric responsive equilibrium. If subjects treated public signals as information devices, we would expect this result only for the treatment with high public signal accuracy (
), as in Reference [
11]. Yet, subjects tend to over follow the public signal even when it is less accurate than the private one, as
Figure 1 shows. One might hypothesize that public signals are focal points acting as coordination devices when decisions are taken in groups. As shown in
Figure 2, this mechanism is contradicted by the data. Moreover, results from the individual sessions disregard the coordination mechanism, as subjects vote very similarly whether they are in groups or not.
The second conclusion is that salience of information affects voter behavior. Different treatments investigate whether subjects follow the public signal because it is displayed in a salient manner. In particular, experimental results show that the order of message delivery matters, as subjects tend to follow the public signal more when it is the most recent signal observed before voting. Interestingly, this finding is robust to sessions where subjects do not vote in committees over issues of common interest. This result of recency effect mirrors what observed in field experiments on political message effectiveness during electoral campaigns [
13].
The effect of recency of public information can have important political implications. Consider for instance the timing of political scandals’ breaking: if the timing of message delivery matters, then it is more likely that voters take into account a scandal involving a politician when voting if the scandal happens close to the election date. A recent illustration of what is known as an “October surprise” in American Politics was Comey’s announcement about reopening the email investigation of Hillary Clinton’s emails. The announcement came on 28 October 2016, ten days before the Presidential election won by Donald Trump. Although it is hard to assess the effect of this announcement on the election’s outcome, it is reasonable to believe that this affected voters more than had it been announced six months before. The fact that voters overreact to salient, recent information, can explain the strategic choice of when to drop a bombshell.