Computational Optimization of Irradiance and Fluence for Interstitial Photodynamic Therapy Treatment of Patients with Malignant Central Airway Obstruction
Abstract
Simple Summary
Abstract
1. Introduction
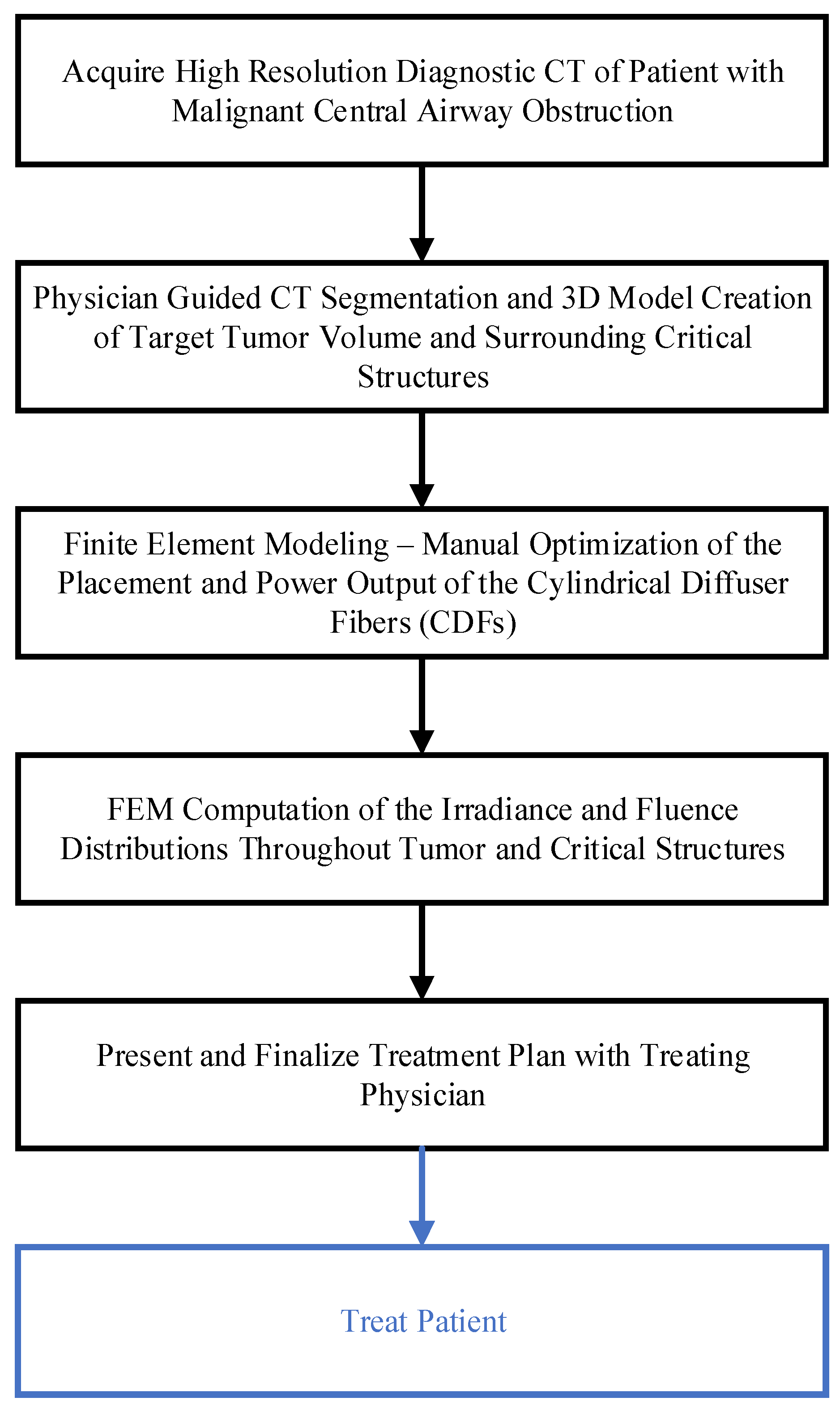
2. Materials and Methods
2.1. FEM Light Propagation Simulations in I-PDT
2.2. Image-Based FEM for I-PDT of MCAO
2.3. Comsol Optimization of I-PDT Light Delivery in the Treatment of MCAO
2.4. Dosie Optimization of Power Outputs and Treatment Times with a Novel Domination Sub-Maps Method
3. Results
3.1. Validation of the FEM Solvers in an Optical Phantom
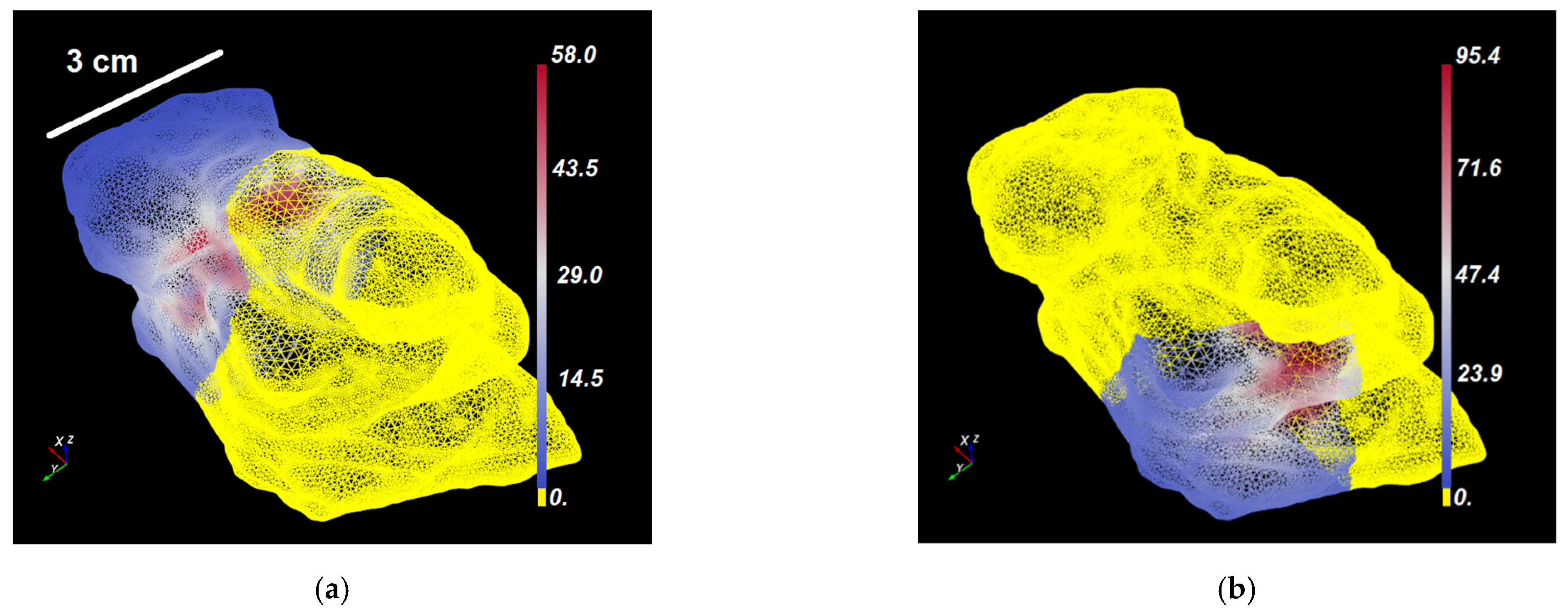
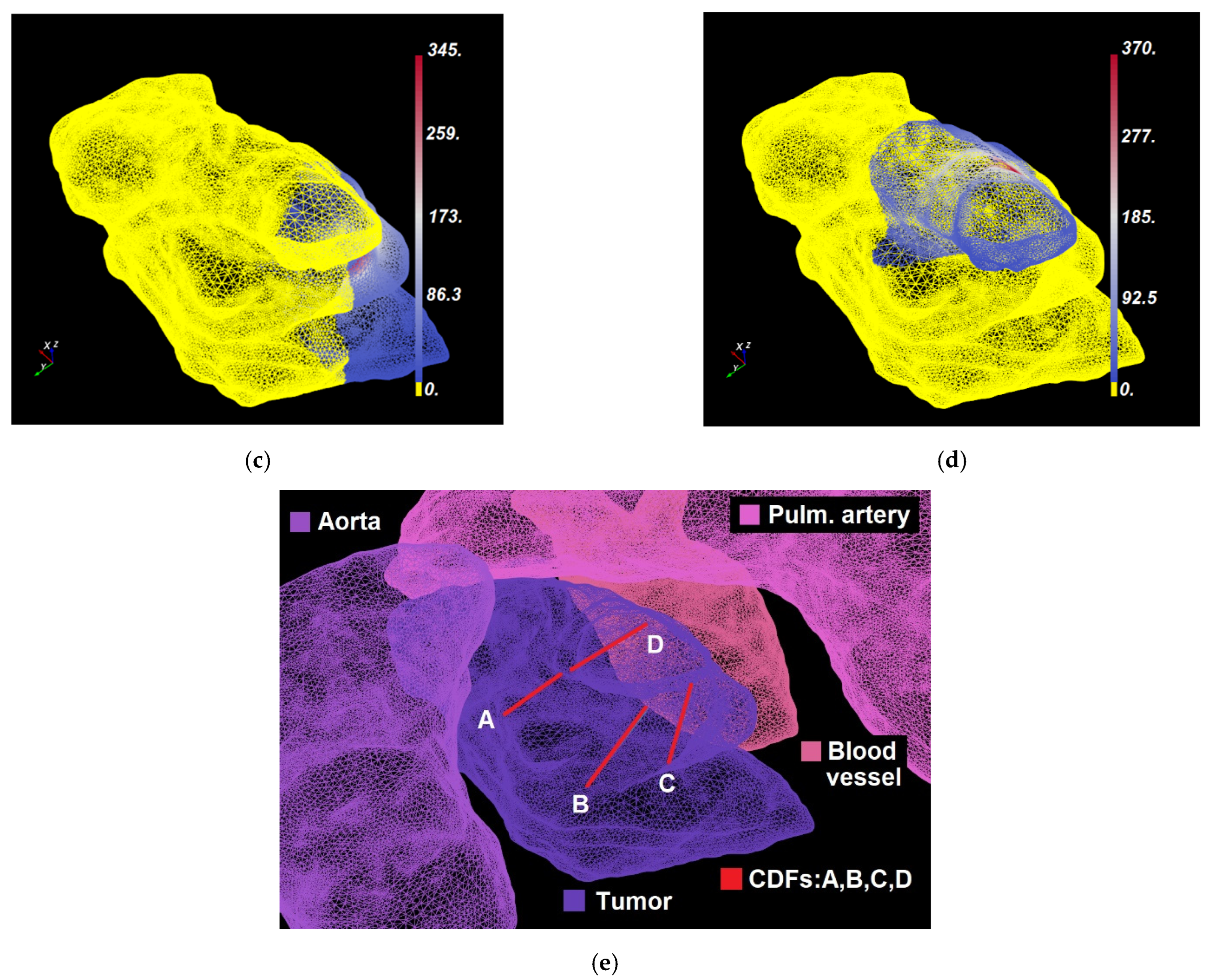
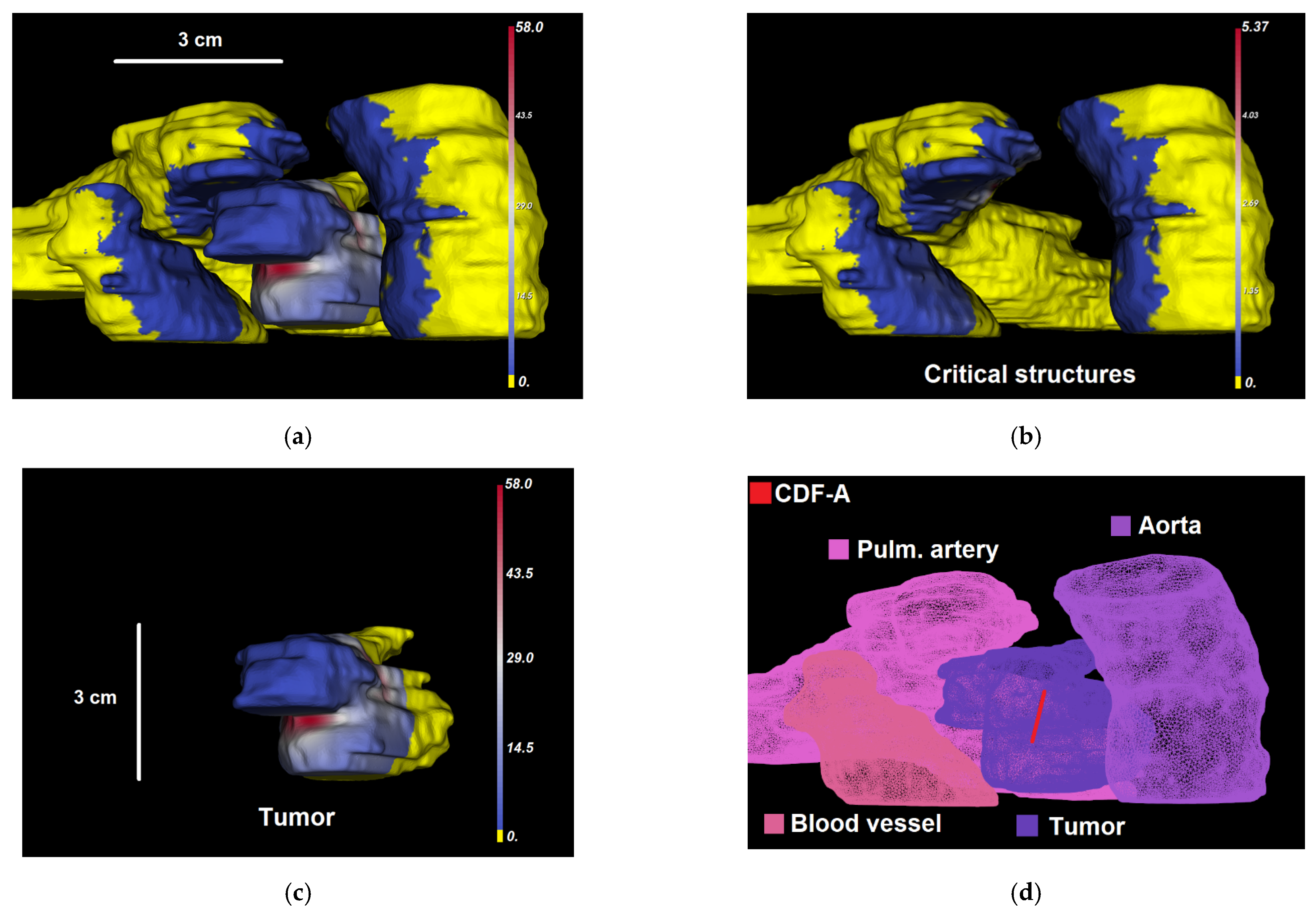
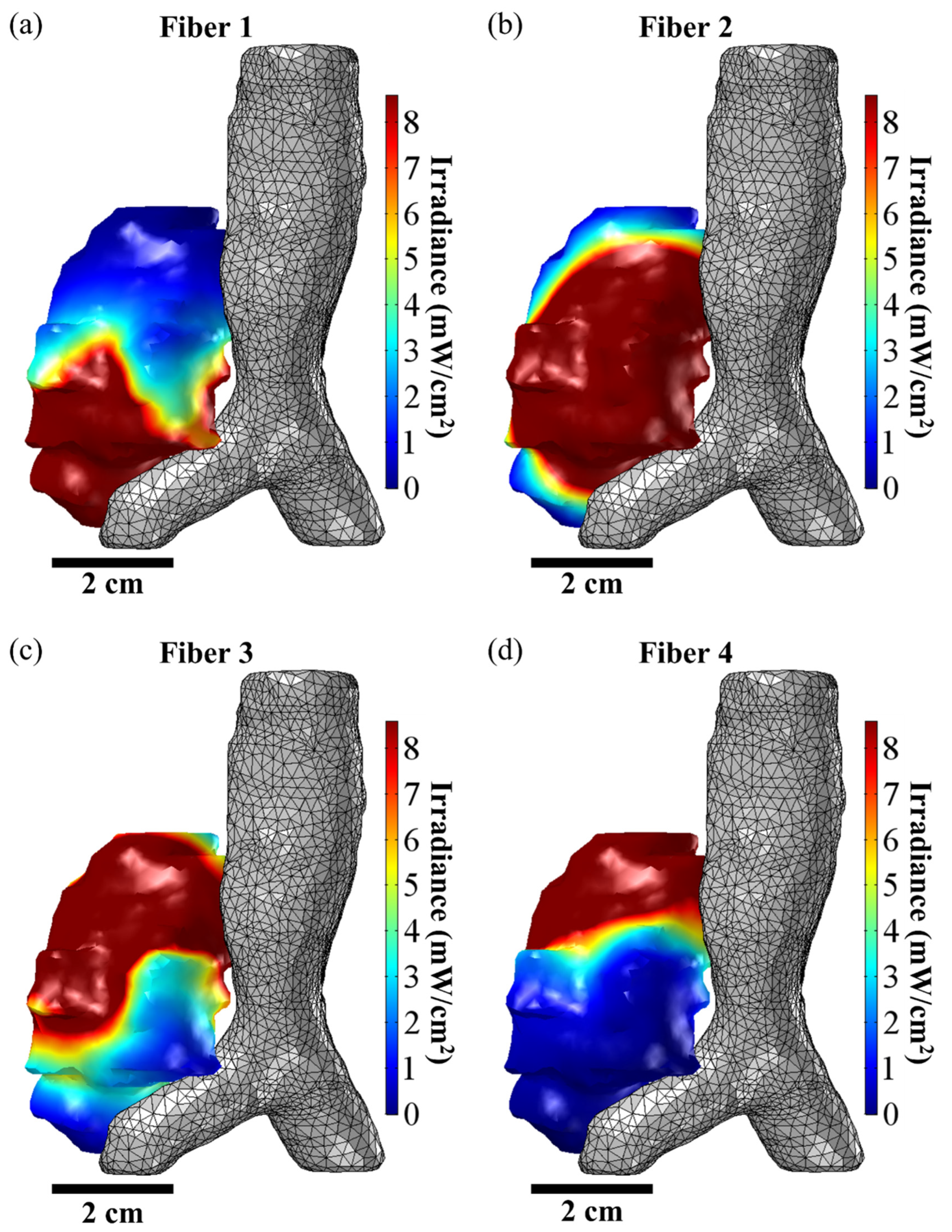
3.2. Treatment Planning for I-PDT of MCAO Using Comsol
3.3. Dosie Is in Agreement with Comsol FEM Simulations for I-PDT of MCAO
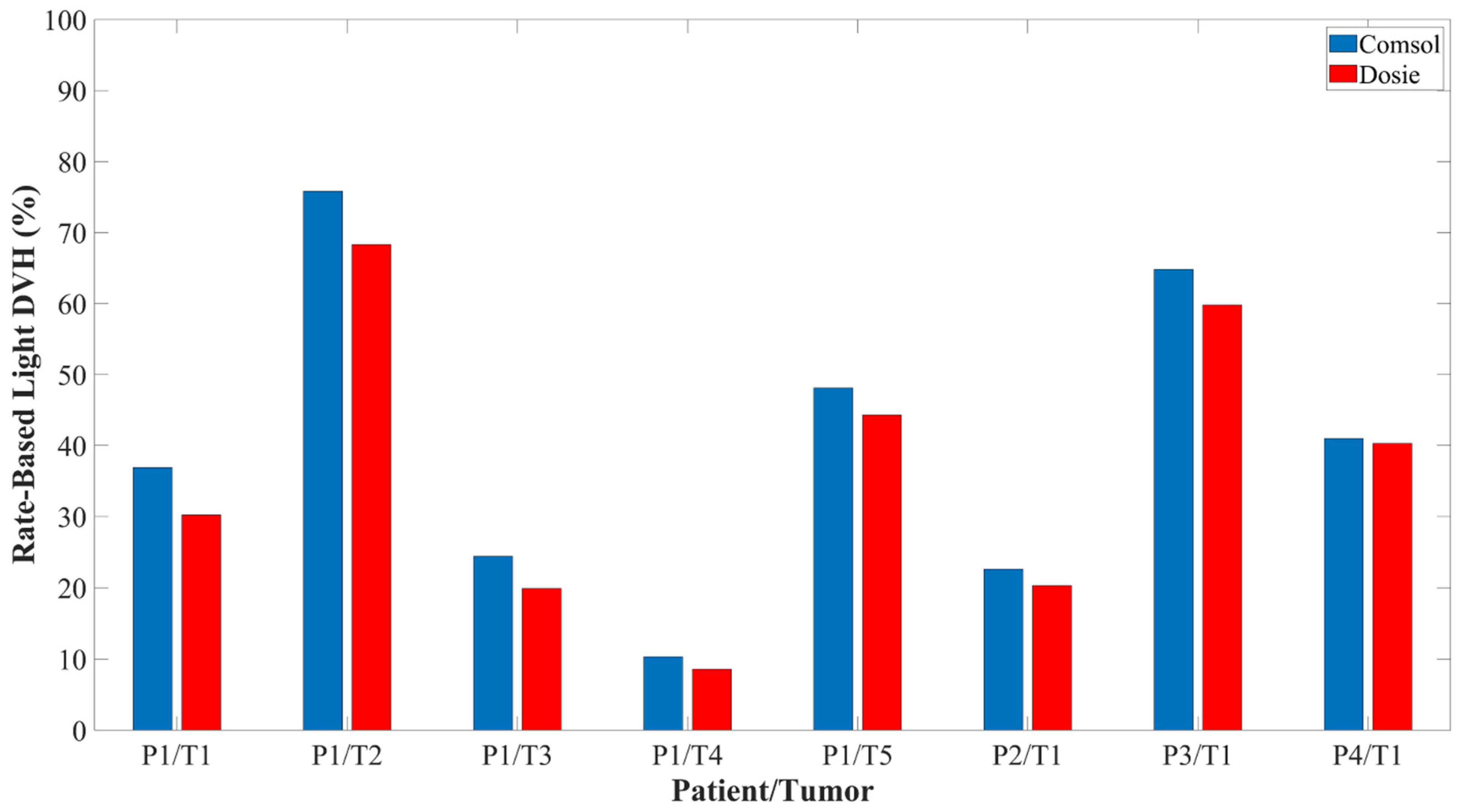
3.4. Dosie Has the Potential to Improve the Administration of Rate-Based Light Dose
4. Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Agostinis, P.; Berg, K.; Cengel, K.A.; Foster, T.H.; Girotti, A.W.; Gollnick, S.O.; Hahn, S.M.; Hamblin, M.R.; Juzeniene, A.; Kessel, D.; et al. Photodynamic therapy of cancer: An update. CA Cancer J. Clin. 2011, 61, 250–281. [Google Scholar] [CrossRef] [PubMed]
- Dougherty, T.J.; Gomer, C.J.; Henderson, B.W.; Jori, G.; Kessel, D.; Korbelik, M.; Moan, J.; Peng, Q. Photodynamic therapy. J. Natl. Cancer Inst. 1998, 90, 889–905. [Google Scholar] [CrossRef] [PubMed]
- Henderson, B.W.; Dougherty, T.J. How does photodynamic therapy work? Photochem. Photobiol. 1992, 55, 145–157. [Google Scholar] [CrossRef] [PubMed]
- Falk-Mahapatra, R.; Gollnick, S.O. Photodynamic Therapy and Immunity: An Update. Photochem. Photobiol. 2020, 96, 550–559. [Google Scholar] [CrossRef] [PubMed]
- Shafirstein, G.; Bellnier, D.; Oakley, E.; Hamilton, S.; Potasek, M.; Beeson, K.; Parilov, E. Interstitial Photodynamic Therapy-A Focused Review. Cancers 2017, 9, 12. [Google Scholar] [CrossRef] [PubMed]
- Davidson, S.R.; Weersink, R.A.; Haider, M.A.; Gertner, M.R.; Bogaards, A.; Giewercer, D.; Scherz, A.; Sherar, M.D.; Elhilali, M.; Chin, J.L.; et al. Treatment planning and dose analysis for interstitial photodynamic therapy of prostate cancer. Phys. Med. Biol. 2009, 54, 2293–2313. [Google Scholar] [CrossRef] [PubMed]
- Swartling, J.; Hoglund, O.V.; Hansson, K.; Sodersten, F.; Axelsson, J.; Lagerstedt, A.S. Online dosimetry for temoporfin-mediated interstitial photodynamic therapy using the canine prostate as model. J. Biomed. Opt. 2016, 21, 28002. [Google Scholar] [CrossRef]
- Swartling, J.; Axelsson, J.; Ahlgren, G.; Kalkner, K.M.; Nilsson, S.; Svanberg, S.; Svanberg, K.; Andersson-Engels, S. System for interstitial photodynamic therapy with online dosimetry: First clinical experiences of prostate cancer. J. Biomed. Opt. 2010, 15, 058003. [Google Scholar] [CrossRef]
- Ivanick, N.M.; Oakley, E.R.; Kunadharaju, R.; Brackett, C.; Bellnier, D.A.; Tworek, L.M.; Kurenov, S.N.; Gollnick, S.O.; Hutson, A.D.; Busch, T.M.; et al. First-In-Human Computer-Optimized Endobronchial Ultrasound-Guided Interstitial Photodynamic Therapy for Patients With Extrabronchial or Endobronchial Obstructing Malignancies. JTO Clin. Res. Rep. 2022, 3, 100372. [Google Scholar] [CrossRef]
- Shafirstein, G.; Bellnier, D.A.; Oakley, E.; Hamilton, S.; Habitzruther, M.; Tworek, L.; Hutson, A.; Spernyak, J.A.; Sexton, S.; Curtin, L.; et al. Irradiance controls photodynamic efficacy and tissue heating in experimental tumours: Implication for interstitial PDT of locally advanced cancer. Br. J. Cancer. 2018, 119, 1191–1199. [Google Scholar] [CrossRef]
- Wilson, B.C.; Patterson, M.S. The physics, biophysics and technology of photodynamic therapy. Phys. Med. Biol. 2008, 53, R61–R109. [Google Scholar] [CrossRef] [PubMed]
- Yassine, A.A.; Kingsford, W.; Xu, Y.; Cassidy, J.; Lilge, L.; Betz, V. Automatic interstitial photodynamic therapy planning via convex optimization. Biomed. Opt. Express. 2018, 9, 898–920. [Google Scholar] [CrossRef] [PubMed]
- Zhu, T.C.; Finlay, J.C. Prostate PDT dosimetry. Photodiagnosis Photodyn. Ther. 2006, 3, 234–246. [Google Scholar] [CrossRef]
- Kim, M.M.; Darafsheh, A. Light Sources and Dosimetry Techniques for Photodynamic Therapy. Photochem. Photobiol. 2020, 96, 280–294. [Google Scholar] [CrossRef] [PubMed]
- Gregor, A.; Sase, S.; Wagnieres, G. Optimization of the Distance between Cylindrical Light Distributors Used for Interstitial Light Delivery in Biological Tissues. Photonics 2022, 9, 597. [Google Scholar] [CrossRef]
- Oakley, E.; Bellnier, D.; Hutson, A.; Cooper, H.; Habitzruther, M.; Sexton, S.; Curtin, L.; Tworek, L.; Mallory, M.; Henderson, B.; et al. Irradiance, Photofrin((R)) Dose and Initial Tumor Volume are Key Predictors of Response to Interstitial Photodynamic Therapy of Locally Advanced Cancers in Translational Models. Photochem. Photobiol. 2020, 96, 397–404. [Google Scholar] [CrossRef]
- Henderson, B.W.; Busch, T.M.; Vaughan, L.A.; Frawley, N.P.; Babich, D.; Sosa, T.A.; Zollo, J.D.; Dee, A.S.; Cooper, M.T.; Bellnier, D.A.; et al. Photofrin photodynamic therapy can significantly deplete or preserve oxygenation in human basal cell carcinomas during treatment, depending on fluence rate. Cancer Res. 2000, 60, 525–529. [Google Scholar]
- Henderson, B.W.; Busch, T.M.; Snyder, J.W. Fluence rate as a modulator of PDT mechanisms. Lasers Surg. Med. 2006, 38, 489–493. [Google Scholar] [CrossRef]
- Lindberg, K.; Grozman, V.; Karlsson, K.; Lindberg, S.; Lax, I.; Wersall, P.; Persson, G.F.; Josipovic, M.; Khalil, A.A.; Moeller, D.S.; et al. The HILUS-Trial-a Prospective Nordic Multicenter Phase 2 Study of Ultracentral Lung Tumors Treated With Stereotactic Body Radiotherapy. J. Thorac. Oncol. 2021, 16, 1200–1210. [Google Scholar] [CrossRef]
- Farrugia, M.; Ma, S.J.; Hennon, M.; Nwogu, C.; Dexter, E.; Picone, A.; Demmy, T.; Yendamuri, S.; Yu, H.; Fung-Kee-Fung, S.; et al. Exceeding Radiation Dose to Volume Parameters for the Proximal Airways with Stereotactic Body Radiation Therapy Is More Likely for Ultracentral Lung Tumors and Associated with Worse Outcome. Cancers 2021, 13, 3463. [Google Scholar] [CrossRef]
- Lester, J.F.; Macbeth, F.R.; Toy, E.; Coles, B. Palliative radiotherapy regimens for non-small cell lung cancer. In Cochrane Database of Systematic Reviews; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2006; p. CD002143. [Google Scholar] [CrossRef]
- Mallow, C.; Hayes, M.; Semaan, R.; Smith, T.; Hales, R.; Brower, R.; Yarmus, L. Minimally invasive palliative interventions in advanced lung cancer. Expert. Rev. Respir. Med. 2018, 12, 605–614. [Google Scholar] [CrossRef] [PubMed]
- Oakley, E.; Wrazen, B.; Bellnier, D.A.; Syed, Y.; Arshad, H.; Shafirstein, G. A new finite element approach for near real-time simulation of light propagation in locally advanced head and neck tumors. Lasers Surg. Med. 2015, 47, 60–67. [Google Scholar] [CrossRef] [PubMed]
- Oakley, E.; Bellnier, D.A.; Hutson, A.; Wrazen, B.; Arshad, H.; Quon, H.; Shafirstein, G. Surface markers for guiding cylindrical diffuser fiber insertion in interstitial photodynamic therapy of head and neck cancer. Lasers Surg. Med. 2017, 49, 599–608. [Google Scholar] [CrossRef] [PubMed]
- Beeson, K.W., Parilov, E., Potasek, M.J., Eds.; Validation of Dosie combined Monte Carlo and photokinetic simulations for the analysis of HPPH-mediated photodynamic therapy on mice. In Proceedings of the Optical Methods for Tumor Treatment and Detection: Mechanisms and Techniques in Photodynamic Therapy XXVIII, San Francisco, CA, USA, 2–7 February 2019; Volume 10860. [Google Scholar] [CrossRef]
- Potasek, M.; Beeson, K.; Parilov, E.; Zhu, T.C.; Sun, H.; Sourvanos, D. Photodynamic therapy in a pleural cavity using Monte Carlo simulations with 2D/3D Graphical Visualization. Glob. J. Cancer Ther. 2022, 8, 34–35. [Google Scholar] [CrossRef]
- Beeson, K.W.; Parilov, E.; Potasek, M.J.; Kim, M.M.; Zhu, T.C. Validation of combined Monte Carlo and photokinetic Simulations for the outcome correlation analysis of benzoporphyrin derivative-mediated photodynamic therapy on mice. J. Biomed. Opt. 2019, 24, 035006. [Google Scholar] [CrossRef] [PubMed]
- Zhu, T.C.; Kim, M.M.; Padawer, J.; Dimofte, A.; Potasek, M.; Beeson, K.; Parilov, E. Light Fluence Dosimetry in Lung-simulating Cavities. Proc. SPIE Int. Soc. Opt. Eng. 2018, 10476, 33–39. [Google Scholar] [CrossRef]
- Ribés, A., Bruneton, A., Eds.; Visualizing results in the SALOME platform for large numerical simulations: An integration of ParaView. In Proceedings of the 2014 IEEE 4th Symposium on Large Data Analysis and Visualization (LDAV), Paris, France, 9–10 November 2014. [Google Scholar]
- Robinson, D.J.; Karakullukcu, B.M.; Kruijt, B.; Kanick, C.S.; van Veen, R.P.L.; Amelink, A.; Sterenborg, H.J.C.M.; Witjes, M.J.; Tan, B.I. Optical Spectroscopy to Guide Photodynamic Therapy of Head and Neck Tumors. IEEE J. Sel. Top. Quantum Electron. 2010, 16, 854–862. [Google Scholar] [CrossRef]
- Van Germert, M.J.C.; Welch, A.J.; Pickkering, J.W.; Tan, O.T. Laser Treatment of Port Wine Stains. In Optical-Thermal Response of Laser-Irradiated Tissue; Welch, A.J., Van Germert, M.J.C., Eds.; Lasers, Photonics, and Electro-Optics; Springer: Boston, MA, USA, 1995; pp. 789–829. [Google Scholar] [CrossRef]
- Lin, L.I. A concordance correlation coefficient to evaluate reproducibility. Biometrics 1989, 45, 255–268. [Google Scholar] [CrossRef]


| Title 1 | Input Data | Description |
|---|---|---|
| Tumor Tissue and Surrounding Normal Tissue | μa = 0.2 (1/cm) μs′ = 5.0 (1/cm) n = 1.37 | Tissue linear absorption coefficient Tissue reduced scattering coefficient Tissue refractive index |
| Pulmonary Artery, Descending Aorta, and Other Major Blood Vessels | μa = 50.0 (1/cm) μs′ = 2.32 (1/cm) n = 1.33 | Blood linear absorption coefficient Blood reduced scattering coefficient Blood refractive index |
| Distance from CDF (mm) | Average Measured Irradiance (mW/cm2) | Comsol Computed Irradiance (mW/cm2) | Dosie Computed Irradiance (mW/cm2) |
|---|---|---|---|
| 5 | 46.7 ± 3.4 | 47.0 | 50.5 |
| 10 | 15.1 ± 0.8 | 13.6 | 12.7 |
| 15 | 4.7 ± 0.2 | 4.4 | 4.0 |
| 20 | 1.4 ± 0.09 | 1.5 | 1.3 |
| 25 | 0.4 ± 0.03 | 0.5 | 0.5 |
| Patient | CDF Power Output Comsol → Dosie | Time Comsol → Dosie | Irradiance DRVH Comsol → Dosie | Effective Rate-Based Light Dose DVH Comsol → Dosie |
|---|---|---|---|---|
| P1/T1 | 120 mW → 200 mW | 500 s → 520 s | 99.5% → 100% | 36.9% → 46.7% |
| P1/T2 | 100 mW → 120 mW | 500 s → 500 s | 100% → 100% | 75.8% → 82.3% |
| P1/T3 | 120 mW → 100 mW | 500 s → 560 s | 91.2% → 90.5% | 24.4% → 27.5% |
| P1/T4 | 120 mW → 120 mW 120 mW → 160 mW | 500 s → 520 s 500 s → 540 s | 38.1% → 40.5% | 10.3% → 10.3% |
| P1/T5 | 80 mW → 100 mW | 500 s → 500 s | 100% → 100% | 48.1% → 63.1% |
| P2 | 120 mW → 100 mW | 750 s → 900 s | 72.7% → 69.3% | 22.6% → 20.3% |
| P3 | 300 mW → 400 mW 300 mW → 600 mW 300 mW → 600 mW 300 mW → 160 mW | 750 s → 740 s 750 s → 900 s 750 s → 840 s 750 s → 760 s | 92.8% → 93.4% | 64.8% → 74.4% |
| P4 | 120 mW → 100 mW 120 mW → 100 mW | 750 s → 720 s 750 s → 900 s | 70.4% → 76.0% | 41.0% → 51.7% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oakley, E.; Parilov, E.; Beeson, K.; Potasek, M.; Ivanick, N.; Tworek, L.; Hutson, A.; Shafirstein, G. Computational Optimization of Irradiance and Fluence for Interstitial Photodynamic Therapy Treatment of Patients with Malignant Central Airway Obstruction. Cancers 2023, 15, 2636. https://doi.org/10.3390/cancers15092636
Oakley E, Parilov E, Beeson K, Potasek M, Ivanick N, Tworek L, Hutson A, Shafirstein G. Computational Optimization of Irradiance and Fluence for Interstitial Photodynamic Therapy Treatment of Patients with Malignant Central Airway Obstruction. Cancers. 2023; 15(9):2636. https://doi.org/10.3390/cancers15092636
Chicago/Turabian StyleOakley, Emily, Evgueni Parilov, Karl Beeson, Mary Potasek, Nathaniel Ivanick, Lawrence Tworek, Alan Hutson, and Gal Shafirstein. 2023. "Computational Optimization of Irradiance and Fluence for Interstitial Photodynamic Therapy Treatment of Patients with Malignant Central Airway Obstruction" Cancers 15, no. 9: 2636. https://doi.org/10.3390/cancers15092636
APA StyleOakley, E., Parilov, E., Beeson, K., Potasek, M., Ivanick, N., Tworek, L., Hutson, A., & Shafirstein, G. (2023). Computational Optimization of Irradiance and Fluence for Interstitial Photodynamic Therapy Treatment of Patients with Malignant Central Airway Obstruction. Cancers, 15(9), 2636. https://doi.org/10.3390/cancers15092636








