An Integrated Monte Carlo Model for Heterogeneous Glioblastoma Treated with Boron Neutron Capture Therapy
Abstract
Simple Summary
Abstract
1. Introduction
- Heterogeneous radiosensitivity;
- Anisotropic infiltration distributions;
- Comparison of BNCT treatment to the non-uniform oxygen distribution of EBRT;
- Updated results for homogeneous GBM with isotropic infiltration distribution.
2. Materials and Methods
2.1. Cell-Based Dosimetry
2.2. Survival Fraction Calculation
3. Results
3.1. Assessment of Survival Fractions for a 2.0 cm Clinical Target Volume Margin
3.1.1. Survival Fractions in Various Regions—2.0 cm CTV Margin
3.1.2. Differential Survival Fraction—2.0 cm CTV Margin
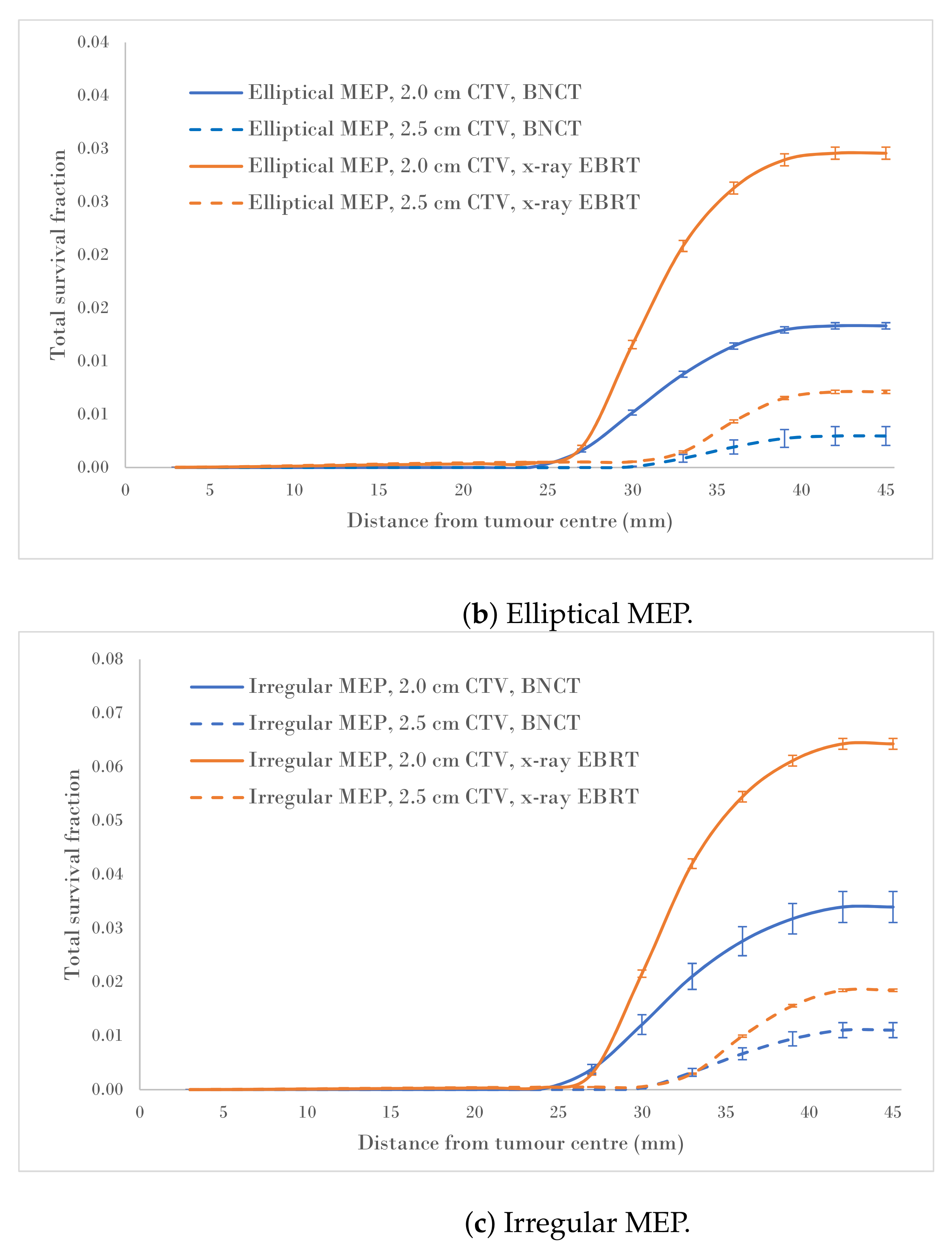
3.2. Quantification of Survival Fraction Reduction following Clinical Target Volume Margin Extension
4. Discussion
5. Conclusions
- The holy grail of radiotherapy is to maximise the tumour control while the toxicity to the surrounding normal tissue is kept at a minimum. It is evident that increasing the CTV margin improves tumour control, however, it is useful to estimate the gain when two competing goals are sought after. A quantification tool was developed to estimate the reduction in survival fraction due to the extension of the CTV for three MEP distributions. It was concluded that the reduction in SF, which was lower than that for X-ray therapy for all MEP models, may not justify the increased normal brain exposure to high LET radiation.
- It was demonstrated that BNCT is efficacious for GBM within the beam in terms of cell kill efficacy. Any increase in the dose and the CTV margin extension should be subject to further improvements in the boron micro-distribution and the neutron beam spectrum, and should be evaluated with care.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AB-BNCT | accelerator-based BNCT |
| BNCT | boron neutron capture therapy |
| CNS | central nervous system |
| CTV | clinical target volume |
| DSB | double-strand break |
| EBRT | External beam radiotherapy |
| GBM | glioblastoma |
| GTV | gross tumour volume |
| LET | linear energy transfer |
| LQ | linear quadratic |
| MEP | microscopic extension probability |
| MC | Monte Carlo |
| OER | oxygen enhancement ratio |
| PTV | planning target volume |
| RBE | radiobiological dose |
| SF | survival fraction |
| TME | tumour microenvironment |
| TLK | two-lesion kinetics |
Appendix A. An Overview of the Progression of GBM Treatment Modelling
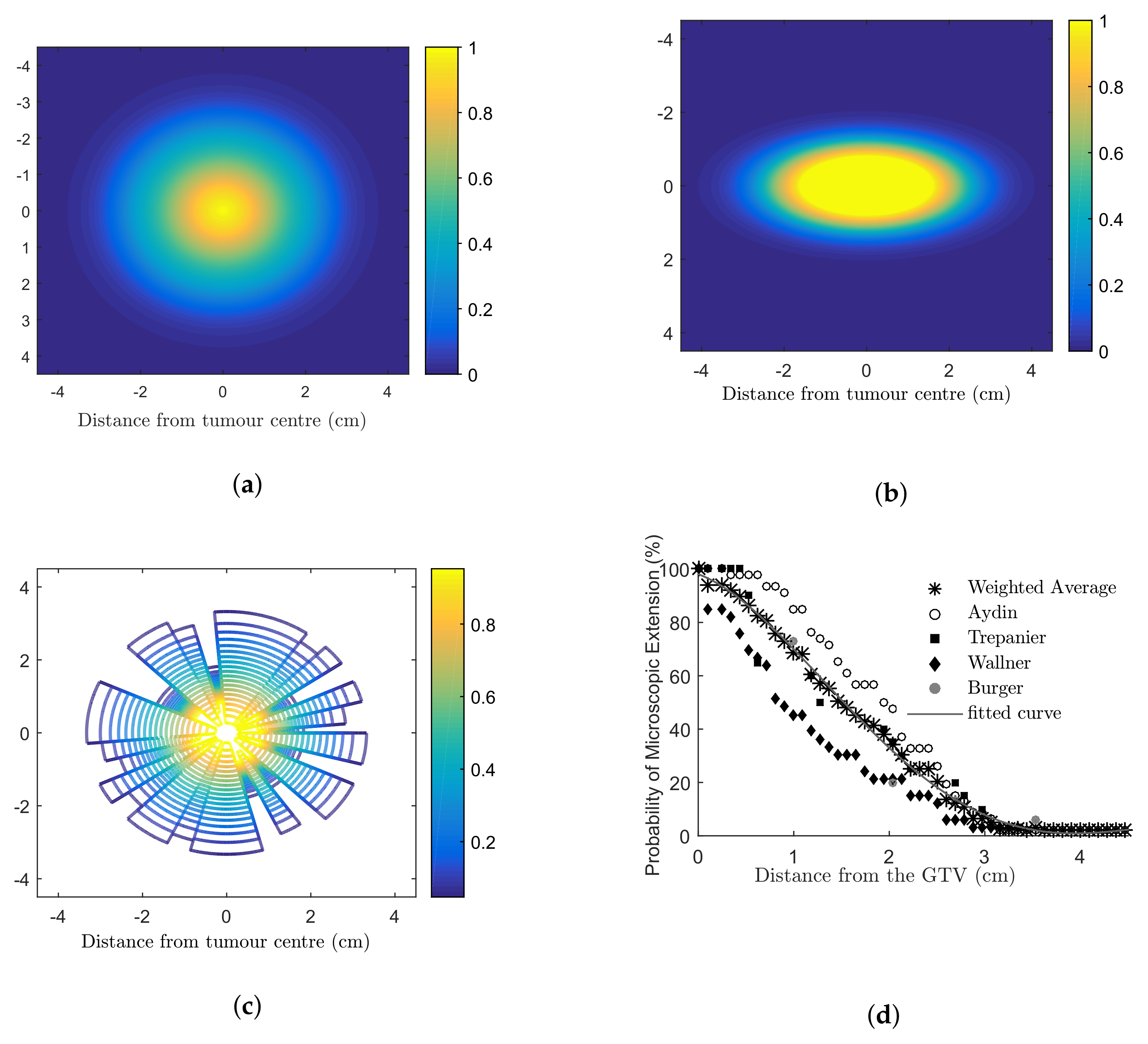
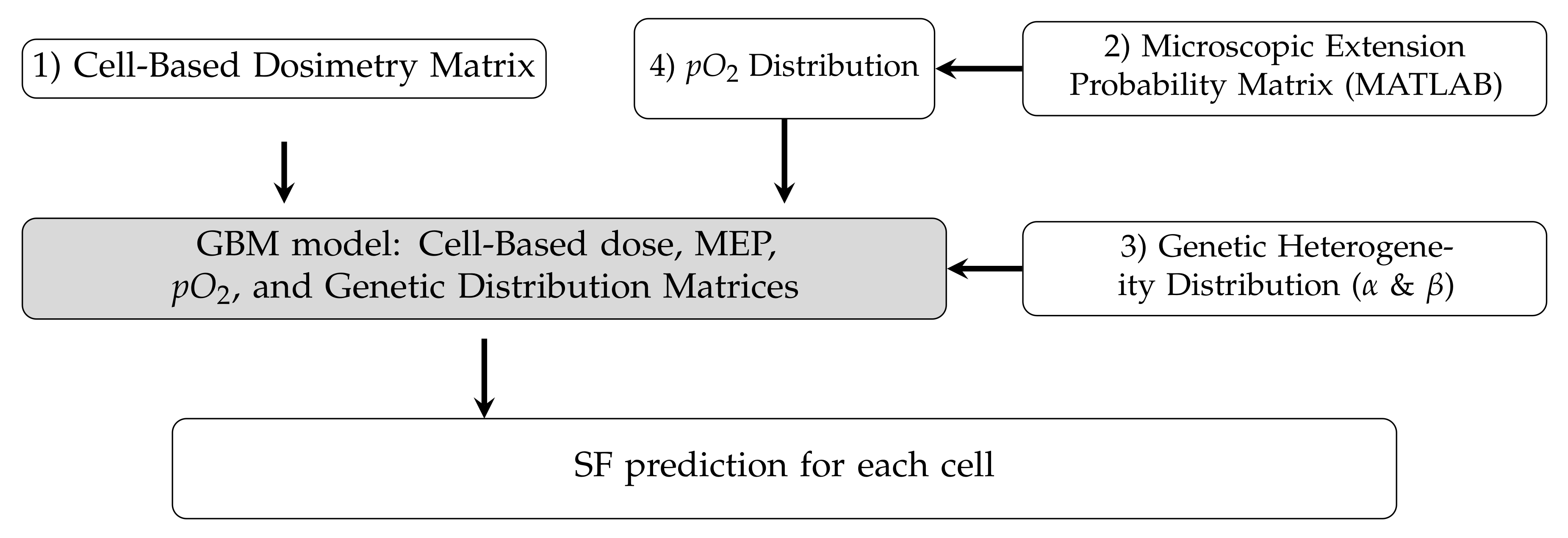
References
- Purves, D.; Augustine, G.; Fitzpatrick, D.; Hall, W.; LaMantia, A.; McNamara, J.; White, L. Neuroscience; Sinaur Associates: Sunderland, MA, USA, 2008. [Google Scholar]
- Louis, D.N.; Perry, A.; Wesseling, P.; Brat, D.J.; Cree, I.A.; Figarella-Branger, D.; Hawkins, C.; Ng, H.; Pfister, S.M.; Reifenberger, G.; et al. The 2021 WHO classification of tumors of the central nervous system: A summary. Neuro-Oncology 2021, 23, 1231–1251. [Google Scholar] [CrossRef]
- Kole, A.; Park, H.; Yeboa, D.; Rutter, C.; Corso, C.; Aneja, S.; Lester-Coll, N.; Mancini, B.; Knisely, J.; Yu, J. Concurrent chemoradiotherapy versus radiotherapy alone for “biopsy-only” glioblastoma multiforme. Cancer 2016, 22, 2364–2370. [Google Scholar] [CrossRef] [PubMed]
- Jayamanne, D.; Wheeler, H.; Cook, R.; Teo, C.; Brazier, D.; Schembri, G.; Kastelan, M.; Guo, L.; Back, M.F. Survival improvements with adjuvant therapy in patients with glioblastoma. ANZ J. Surg. 2017, 88, 196–201. [Google Scholar] [CrossRef] [PubMed]
- Jung, Y.; Ahn, S.H.; Park, S.H.; Choi, Y.H. Effect of glucose level on chemical hypoxia-and hydrogen peroxide-induced chemokine expression in human glioblastoma cell lines. Korean J. Physiol. Pharmacol. 2017, 21, 509–518. [Google Scholar] [CrossRef] [PubMed]
- Sottoriva, A.; Spiteri, I.; Piccirillo, S.G.; Touloumis, A.; Collins, V.P.; Marioni, J.C.; Curtis, C.; Watts, C.; Tavaré, S. Intratumor heterogeneity in human glioblastoma reflects cancer evolutionary dynamics. Proc. Natl. Acad. Sci. USA 2013, 110, 4009–4014. [Google Scholar] [CrossRef] [PubMed]
- Kayabolen, A.; Yilmaz, E.; Bagci-Onder, T. IDH mutations in glioma: Double-Edged sword in clinical applications? Biomedicines 2021, 9, 799. [Google Scholar] [CrossRef] [PubMed]
- Tan, A.C.; Ashley, D.M.; López, G.Y.; Malinzak, M.; Friedman, H.S.; Khasraw, M. Management of glioblastoma: State of the art and future directions. CA Cancer J. Clin. 2020, 70, 299–312. [Google Scholar] [CrossRef]
- Louis, D.N.; Perry, A.; Reifenberger, G.; Von Deimling, A.; Figarella-Branger, D.; Cavenee, W.K.; Ohgaki, H.; Wiestler, O.D.; Kleihues, P.; Ellison, D.W. The 2016 World Health Organization classification of tumors of the central nervous system: A summary. Acta Neuropathol. 2016, 131, 803–820. [Google Scholar] [CrossRef]
- Li, W.; Graeber, M.B. The molecular profile of microglia under the influence of glioma. Neuro-Oncology 2012, 14, 958–978. [Google Scholar] [CrossRef]
- Soomro, S.H.; Ting, L.R.; Qing, Y.Y.; Ren, M. Molecular biology of glioblastoma: Classification and mutational locations. J. Pak. Med. Assoc. 2017, 67, 1410–1414. [Google Scholar]
- Gunjur, A.; Bressel, M.; Ryan, G. The addition of temozolomide does not change the pattern of progression of glioblastoma multiforme post-radiotherapy. J. Med Imaging Radiat. Oncol. 2012, 56, 567–573. [Google Scholar] [CrossRef] [PubMed]
- Chan, J.L.; Lee, S.W.; Fraass, B.A.; Normolle, D.P.; Greenberg, H.S.; Junck, L.R.; Gebarski, S.S.; Sandler, H.M. Survival and failure patterns of high-grade gliomas after three-dimensional conformal radiotherapy. J. Clin. Oncol. 2002, 20, 1635–1642. [Google Scholar] [CrossRef]
- Oppitz, U.; Maessen, D.; Zunterer, H.; Richter, S.; Flentje, M. 3D-recurrence-patterns of gliobastomas after CT-planned postoperative irradiation. Radiother. Oncol. 1999, 53, 53–57. [Google Scholar] [CrossRef]
- Veliz, I.; Loo, Y.; Castillo, O.; Karachaliou, N.; Nigro, O.; Rosell, R. Advances and challenges in the molecular biology and treatment of glioblastoma—Is there any hope for the future? Ann. Transl. Med. 2015, 3, 7. [Google Scholar] [PubMed]
- Colombo, M.C.; Giverso, C.; Faggiano, E.; Boffano, C.; Acerbi, F.; Ciarletta, P. Towards the personalized treatment of glioblastoma: Integrating patient-specific clinical data in a continuous mechanical model. PLoS ONE 2015, 10, e0132887. [Google Scholar]
- Van Dyk, J. The Modern Technology of Radiation Oncology: A Compendium for Medical Physicists and Radiation Oncologists; Medical Physics Pub Corp: Madison, WI, USA, 1999. [Google Scholar]
- Barth, R.F.; Grecula, J.C.; Yang, W.; Rotaru, J.H.; Nawrocky, M.; Gupta, N.; Albertson, B.J.; Ferketich, A.K.; Moeschberger, M.L.; Coderre, J.A.; et al. Combination of boron neutron capture therapy and external beam radiotherapy for brain tumors. Int. J. Radiat. Oncol. Biol. Phys. 2004, 58, 267–277. [Google Scholar] [CrossRef]
- Moghaddasi, L.; Bezak, E.; Harriss-Phillips, W. Evaluation of current clinical target volume definitions for glioblastoma using cell-based dosimetry stochastic methods. Br. J. Radiol. 2015, 88, 20150155. [Google Scholar] [CrossRef] [PubMed]
- Moghaddasi, L.; Bezak, E.; Harriss-Phillips, W. Monte-Carlo model development for evaluation of current clinical target volume definition for heterogeneous and hypoxic glioblastoma. Phys. Med. Biol. 2016, 61, 3407. [Google Scholar] [CrossRef] [PubMed]
- Moghaddasi, L.; Bezak, E. Geant4 beam model for boron neutron capture therapy: Investigation of neutron dose components. Australas. Phys. Eng. Sci. Med. 2018, 41, 129–141. [Google Scholar] [CrossRef] [PubMed]
- Moghaddasi, L.; Bezak, E. Development of an integrated Monte Carlo model for glioblastoma multiforme treated with boron neutron capture therapy. Sci. Rep. 2017, 7, 7069. [Google Scholar] [CrossRef]
- Marek, M.; Vins, M.; Lahodova, Z.; Viererbl, L.; Koleska, M. Extended set of activation monitors for NCT beam characterization and spectral conditions of the beam after reactor fuel conversion. Appl. Radiat. Isot. 2014, 88, 157–161. [Google Scholar] [CrossRef] [PubMed]
- Kawabata, S.; Hiramatsu, R.; Kuroiwa, T.; Ono, K.; Miyatake, S.I. Boron neutron capture therapy for recurrent high-grade meningiomas: Clinical article. J. Neurosurg. 2013, 119, 837–844. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, T.; Nakai, K.; Kageji, T.; Kumada, H.; Endo, K.; Matsuda, M.; Shibata, Y.; Matsumura, A. Boron neutron capture therapy for newly diagnosed glioblastoma. Radiother. Oncol. 2009, 91, 80–84. [Google Scholar] [CrossRef] [PubMed]
- Kageji, T.; Mizobuchi, Y.; Nagahiro, S.; Nakagawa, Y.; Kumada, H. Correlation between radiation dose and histopathological findings in patients with gliblastoma treated with boron neutron capture therapy (BNCT). Appl. Radiat. Isot. 2014, 88, 20–22. [Google Scholar] [CrossRef]
- Morris, G.; Coderre, J.; Micca, P.; Fisher, C.; Capala, J.; Hopewell, J. Central nervous system tolerance to boron neutron capture therapy with p-boronophenylalanine. Br. J. Cancer 1997, 76, 1623. [Google Scholar] [CrossRef]
- Coderre, J.; Diaz, A.Z.; Ma, R. Current Status of Neutron Capture Therapy; Technical report, IAEA-TECDOC-1223; International Atomic Energy Agency Vienna International Centre: Vienna, Austria, 2001; Annex 6; ISSN 1011–4289. [Google Scholar]
- Taghian, A.; Suit, H.; Pardo, F.; Gioioso, D.; Tomkinson, K.; DuBois, W.; Gerweck, L. In vitro intrinsic radiation sensitivity of glioblastoma multiforme. Int. J. Radiat. Oncol. Biol. Phys. 1992, 23, 55–62. [Google Scholar] [CrossRef]
- Barendsen, G.; Koot, C.; Van Kersen, G.; Bewley, D.; Field, S.; Parnell, C. The effect of oxygen on impairment of the proliferative capacity of human cells in culture by ionizing radiations of different LET. Int. J. Radiat. Biol. Relat. Stud. Phys. Chem. Med. 1966, 10, 317–327. [Google Scholar] [CrossRef]
- Aydın, H.; Sillenberg, I.; von Lieven, H. Patterns of failure following CT-based 3-D irradiation for malignant glioma. Strahlentherapie Onkologie 2001, 177, 424–431. [Google Scholar] [CrossRef]
- Lee, I.H.; Piert, M.; Gomez-Hassan, D.; Junck, L.; Rogers, L.; Hayman, J.; Ten Haken, R.K.; Lawrence, T.S.; Cao, Y.; Tsien, C. Association of 11 C-Methionine PET uptake with site of failure after concurrent temozolomide and radiation for primary glioblastoma multiforme. Int. J. Radiat. Oncol. Biol. Phys. 2009, 73, 479–485. [Google Scholar] [CrossRef]
- Matsuda, M.; Yamamoto, T.; Kumada, H.; Nakai, K.; Shirakawa, M.; Tsurubuchi, T.; Matsumura, A. Dose distribution and clinical response of glioblastoma treated with boron neutron capture therapy. Appl. Radiat. Isot. 2009, 67, S19–S21. [Google Scholar] [CrossRef]
- Miyatake, S.I.; Kuwabara, H.; Kajimoto, Y.; Kawabata, S.; Yokoyama, K.; Doi, A.; Tsuji, M.; Mori, H.; Ono, K.; Kuroiwa, T. Preferential recurrence of a sarcomatous component of a gliosarcoma after boron neutron capture therapy: Case report. J. Neuro-Oncol. 2006, 76, 143–147. [Google Scholar] [CrossRef] [PubMed]
- Forton, E.; Stichelbaut, F.; Cambriani, A.; Kleeven, W.; Ahlback, J.; Jongen, Y. Overview of the IBA accelerator-based BNCT system. Appl. Radiat. Isot. 2009, 67, S262–S265. [Google Scholar] [CrossRef] [PubMed]
- Barth, R.F.; Grecula, J.C. Boron neutron capture therapy at the crossroads-Where do we go from here? Appl. Radiat. Isot. 2020, 160, 109029. [Google Scholar] [CrossRef]
- Moghaddasi, L. The Monte Carlo Method as a Design Tool in Boron Neutron Capture Therapy; IOP Publishing: Bristol, UK, 2022; pp. 17-1–17-29. [Google Scholar] [CrossRef]
- Suzuki, M. Boron neutron capture therapy (BNCT): A unique role in radiotherapy with a view to entering the accelerator-based BNCT era. Int. J. Clin. Oncol. 2020, 25, 43–50. [Google Scholar] [CrossRef]
- Tanaka, H.; Sakurai, Y.; Suzuki, M.; Masunaga, S.; Kinashi, Y.; Kashino, G.; Liu, Y.; Mitsumoto, T.; Yajima, S.; Tsutsui, H.; et al. Characteristics comparison between a cyclotron-based neutron source and KUR-HWNIF for boron neutron capture therapy. Nucl. Instruments Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2009, 267, 1970–1977. [Google Scholar] [CrossRef]
- Cartelli, D.; Capoulat, M.; Baldo, M.; Sandín, J.S.; Igarzabal, M.; Del Grosso, M.; Valda, A.; Canepa, N.; Gun, M.; Minsky, D.; et al. Status of low-energy accelerator-based BNCT worldwide and in Argentina. Appl. Radiat. Isot. 2020, 166, 109315. [Google Scholar] [CrossRef]
- Kiyanagi, Y.; Sakurai, Y.; Kumada, H.; Tanaka, H. Status of accelerator-based BNCT projects worldwide. AIP Conf. Proc. 2019, 050012. [Google Scholar] [CrossRef]
- Kumada, H.; Takada, K.; Naito, F.; Kurihara, T.; Sugimura, T.; Matsumoto, Y.; Sakurai, H.; Matsumura, A.; Sakae, T. Beam performance of the iBNCT as a compact linac-based BNCT neutron source developed by University of tsukuba. AIP Conf. Proc. 2019, 2160, 050013. [Google Scholar]
- Kononov, O.; Kononov, V.; Bokhovko, M.; Korobeynikov, V.; Soloviev, A.; Sysoev, A.; Gulidov, I.; Chu, W.; Nigg, D. Optimization of an accelerator-based epithermal neutron source for neutron capture therapy. Appl. Radiat. Isot. 2004, 61, 1009–1013. [Google Scholar] [CrossRef]
- Porra, L.; Seppälä, T.; Wendland, L.; Revitzer, H.; Joensuu, H.; Eide, P.; Koivunoro, H.; Smick, N.; Smick, T.; Tenhunen, M. Accelerator-based boron neutron capture therapy facility at the Helsinki University Hospital. Acta Oncol. 2022, 61, 269–273. [Google Scholar] [CrossRef]
- Halfon, S.; Paul, M.; Arenshtam, A.; Berkovits, D.; Bisyakoev, M.; Eliyahu, I.; Feinberg, G.; Hazenshprung, N.; Kijel, D.; Nagler, A.; et al. High-power liquid-lithium target prototype for accelerator-based boron neutron capture therapy. Appl. Radiat. Isot. 2011, 69, 1654–1656. [Google Scholar] [CrossRef] [PubMed]
- Hu, K.; Yang, Z.; Zhang, L.; Xie, L.; Wang, L.; Xu, H.; Josephson, L.; Liang, S.H.; Zhang, M.R. Boron agents for neutron capture therapy. Coord. Chem. Rev. 2020, 405, 213139. [Google Scholar] [CrossRef]
- Zaider, M. There is no mechanistic basis for the use of the linear-quadratic expression in cellular survival analysis. Med Phys. 1998, 25, 791–792. [Google Scholar] [CrossRef] [PubMed]
- Hanin, L.; Zaider, M. Cell-survival probability at large doses: An alternative to the linear-quadratic model. Phys. Med. Biol. 2010, 55, 4687. [Google Scholar] [CrossRef]
- Curtis, S.B. Lethal and potentially lethal lesions induced by radiation—A unified repair model. Radiat. Res. 1986, 106, 252–270. [Google Scholar] [CrossRef] [PubMed]
- Stewart, R.D. Two-lesion kinetic model of double-strand break rejoining and cell killing. Radiat. Res. 2001, 156, 365–378. [Google Scholar] [CrossRef]
- Douglass, M.; Bezak, E.; Penfold, S. Development of a radiation track structure clustering algorithm for the prediction of DNA DSB yields and radiation induced cell death in Eukaryotic cells. Phys. Med. Biol. 2015, 60, 3217. [Google Scholar] [CrossRef]
- Incerti, S.; Baldacchino, G.; Bernal, M.; Capra, R.; Champion, C.; Francis, Z.; Guèye, P.; Mantero, A.; Mascialino, B.; Moretto, P.; et al. The Geant4-DNA project. Int. J. Model. Simulation Sci. 2010, 1, 157–178. [Google Scholar] [CrossRef]
- Plante, I.; Cucinotta, F.A. Monte Carlo simulation of ionizing radiation tracks. In Application of Monte Carlo Methods in Biology, Medicine and Other Fields of Science; InTech: Rijeka, Croatia, 2011; pp. 315–356. [Google Scholar]
- Unkelbach, J.; Menze, B.H.; Konukoglu, E.; Dittmann, F.; Ayache, N.; Shih, H.A. Radiotherapy planning for glioblastoma based on a tumor growth model: Implications for spatial dose redistribution. Phys. Med. Biol. 2014, 59, 771. [Google Scholar] [CrossRef]
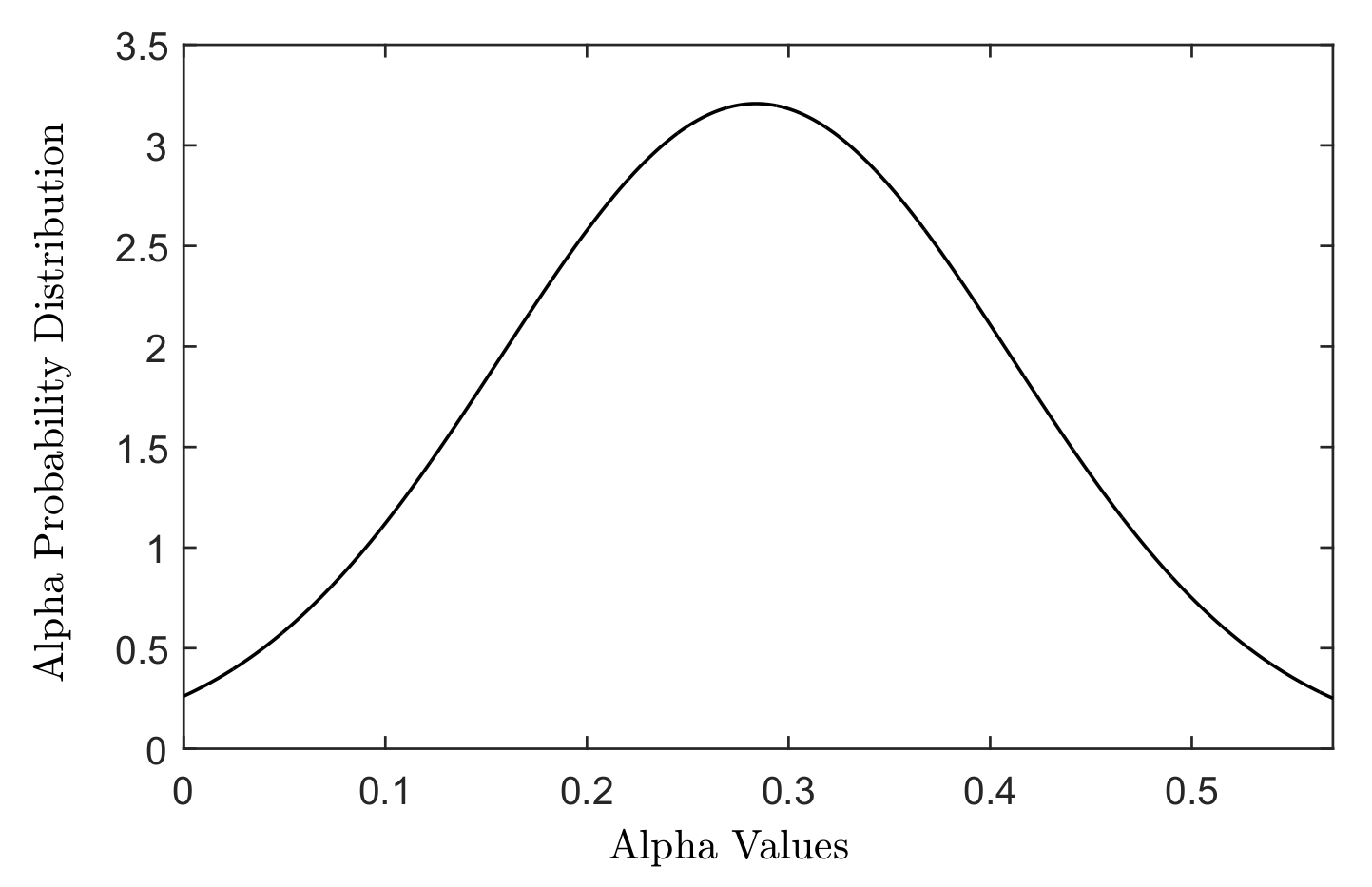

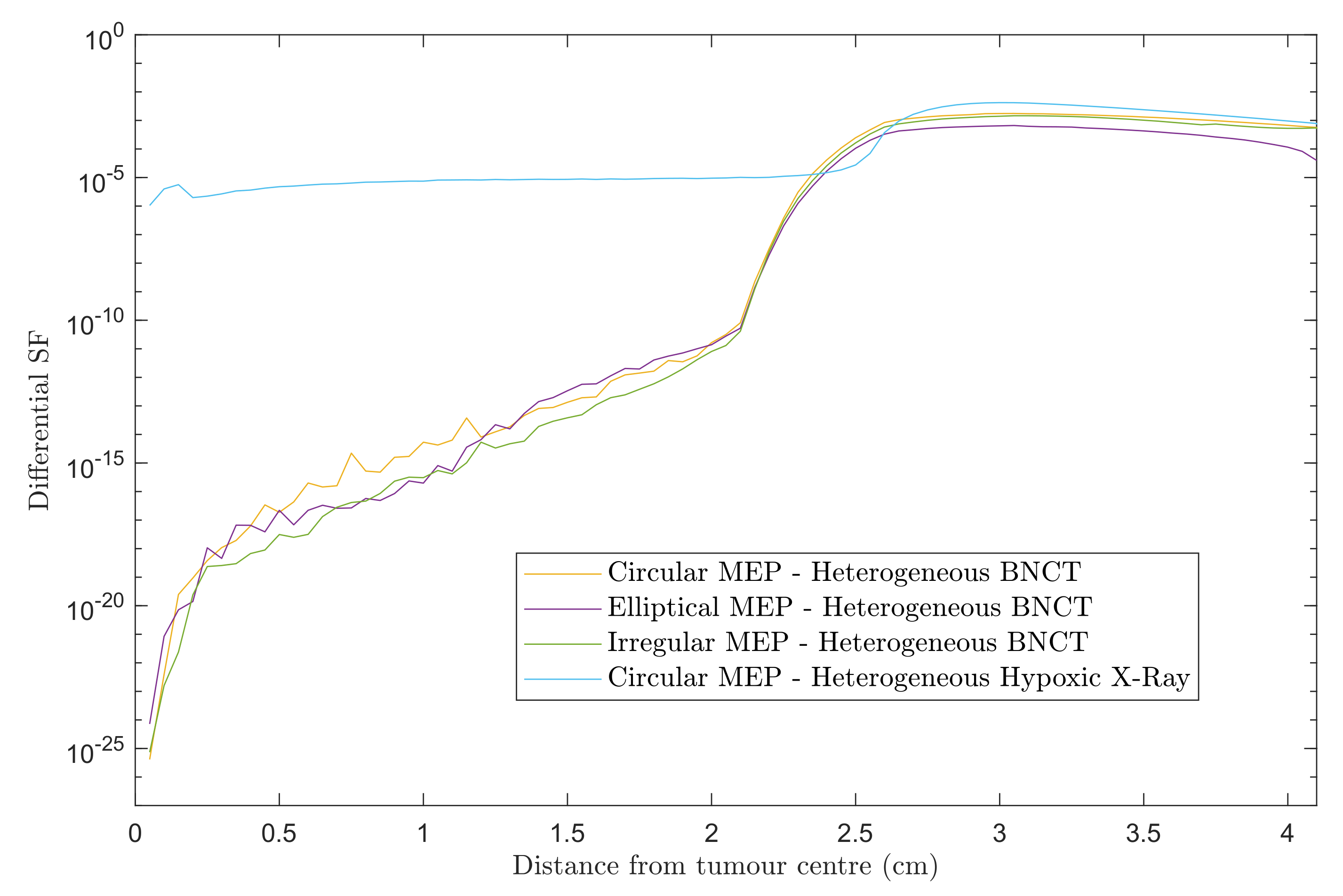

| Modality | BNCT | ||
|---|---|---|---|
| MEP | SF within the Beam (%) | SF within the Penumbra Region (%) | Total SF (%) |
| Circular | |||
| Elliptical | |||
| Irregular | |||
| Conventional X-ray EBRT | |||
| Circular | |||
| Elliptical | |||
| Irregular | |||
| MEP Model | Circular | Elliptical | Irregular |
|---|---|---|---|
| BNCT | |||
| SF with 2.0 cm CTV (%) | |||
| SF with 2.5 cm CTV (%) | |||
| Conventional X-ray Therapy | |||
| SF with 2.0 cm CTV (%) | |||
| SF with 2.5 cm CTV (%) | |||
| p-value | 0.29 | 0.41 | 0.0001 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moghaddasi, L.; Bezak, E. An Integrated Monte Carlo Model for Heterogeneous Glioblastoma Treated with Boron Neutron Capture Therapy. Cancers 2023, 15, 1550. https://doi.org/10.3390/cancers15051550
Moghaddasi L, Bezak E. An Integrated Monte Carlo Model for Heterogeneous Glioblastoma Treated with Boron Neutron Capture Therapy. Cancers. 2023; 15(5):1550. https://doi.org/10.3390/cancers15051550
Chicago/Turabian StyleMoghaddasi, Leyla, and Eva Bezak. 2023. "An Integrated Monte Carlo Model for Heterogeneous Glioblastoma Treated with Boron Neutron Capture Therapy" Cancers 15, no. 5: 1550. https://doi.org/10.3390/cancers15051550
APA StyleMoghaddasi, L., & Bezak, E. (2023). An Integrated Monte Carlo Model for Heterogeneous Glioblastoma Treated with Boron Neutron Capture Therapy. Cancers, 15(5), 1550. https://doi.org/10.3390/cancers15051550






