Orthovoltage X-ray Minibeam Radiation Therapy for the Treatment of Ocular Tumours—An In Silico Evaluation
Abstract
Simple Summary
Abstract
1. Introduction
2. Materials and Methods
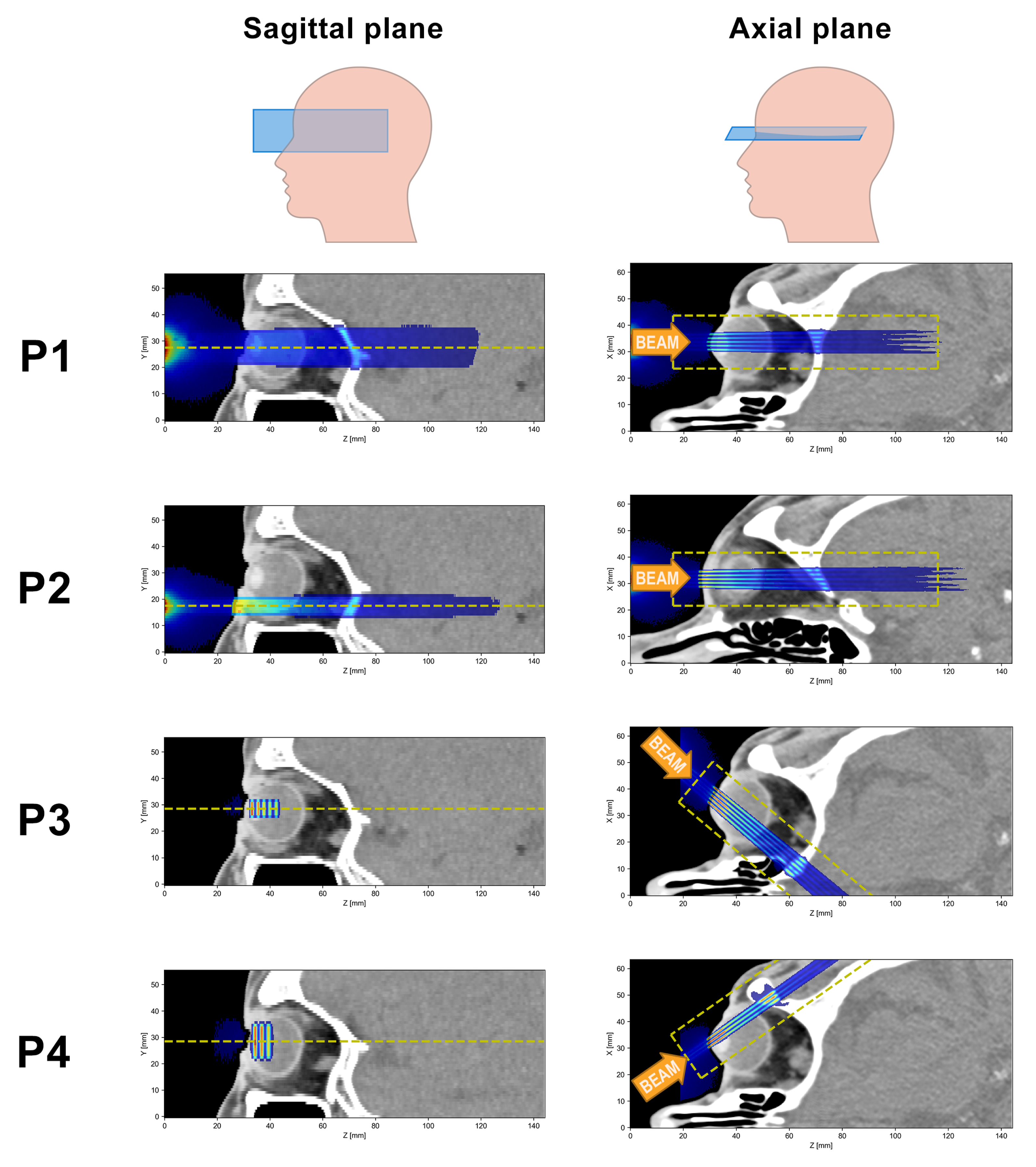
2.1. Irradiation Configurations
| Label | No. of Slits | Slit Height [mm] | Slit Width [m] | Divergence Angle [deg] | Spacing at Exit [m] | ||||
|---|---|---|---|---|---|---|---|---|---|
| colli A | 5 | 10 | 400 | 400 | 425 | 0.595 | 0.632 | 1150 | 1275 |
| colli Ah | 5 | 5 | 400 | 400 | 425 | 0.595 | 0.632 | 1150 | 1275 |
| colli A3s | 3 | 10 | 400 | 400 | - | 0.595 | - | 1150 | - |
| colli B | 5 | 10 | 400 | 409 | 500 | 0.611 | 0.744 | 1900 | 1875 |
| colli C | 3 | 10 | 400 | 425 | - | 0.632 | - | 2425 | - |
2.2. Dose Scoring and Analysis
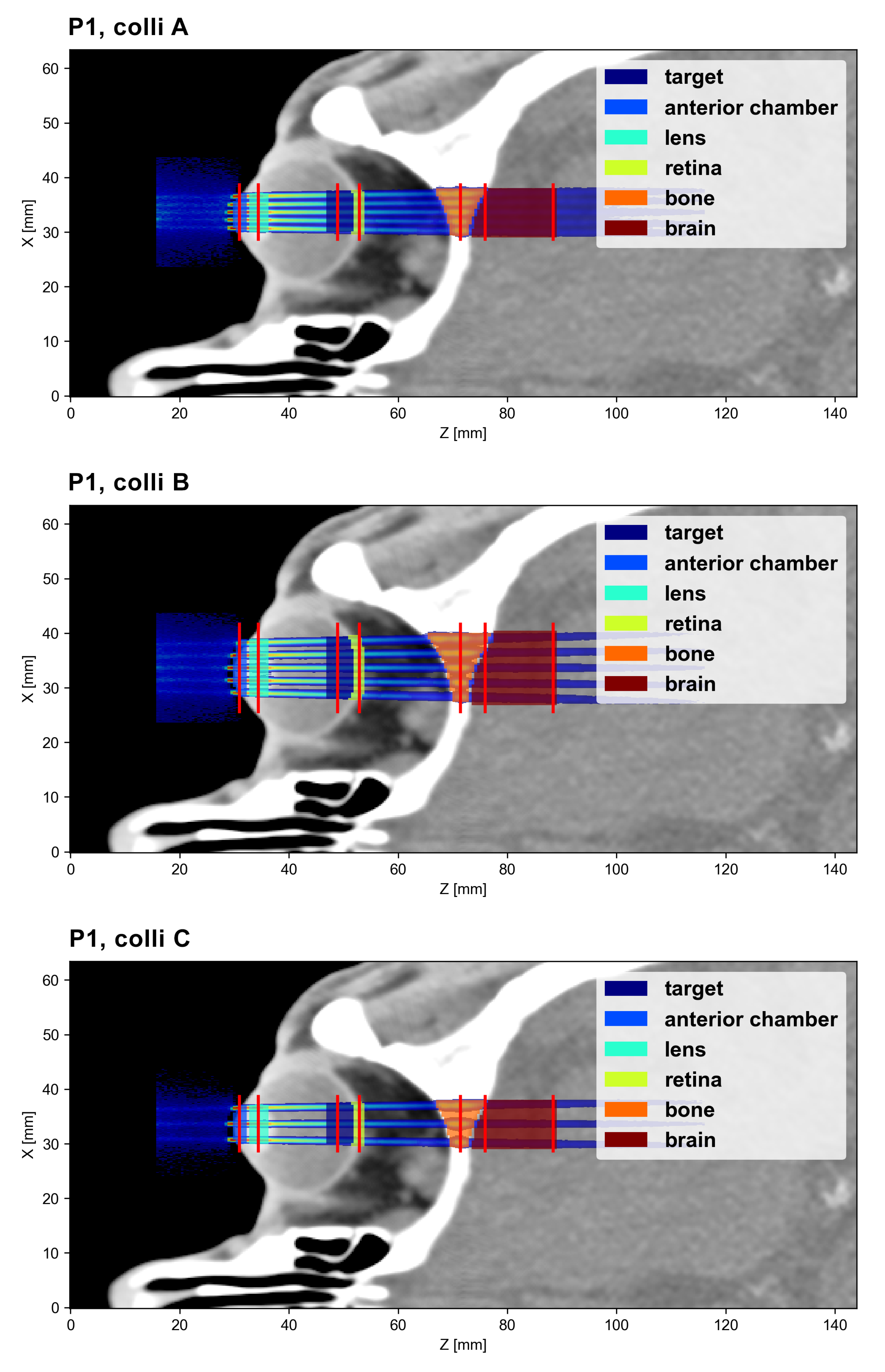
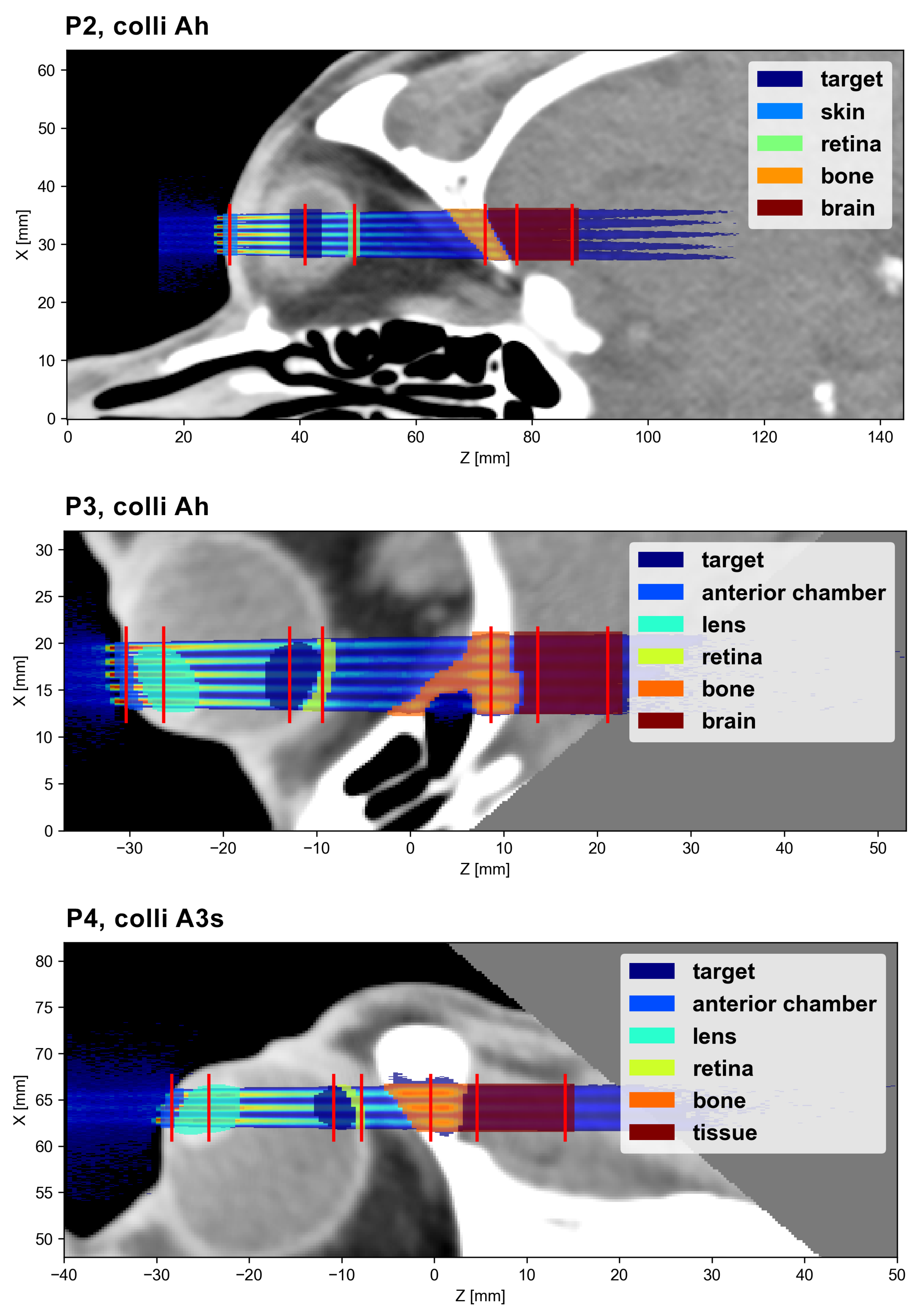
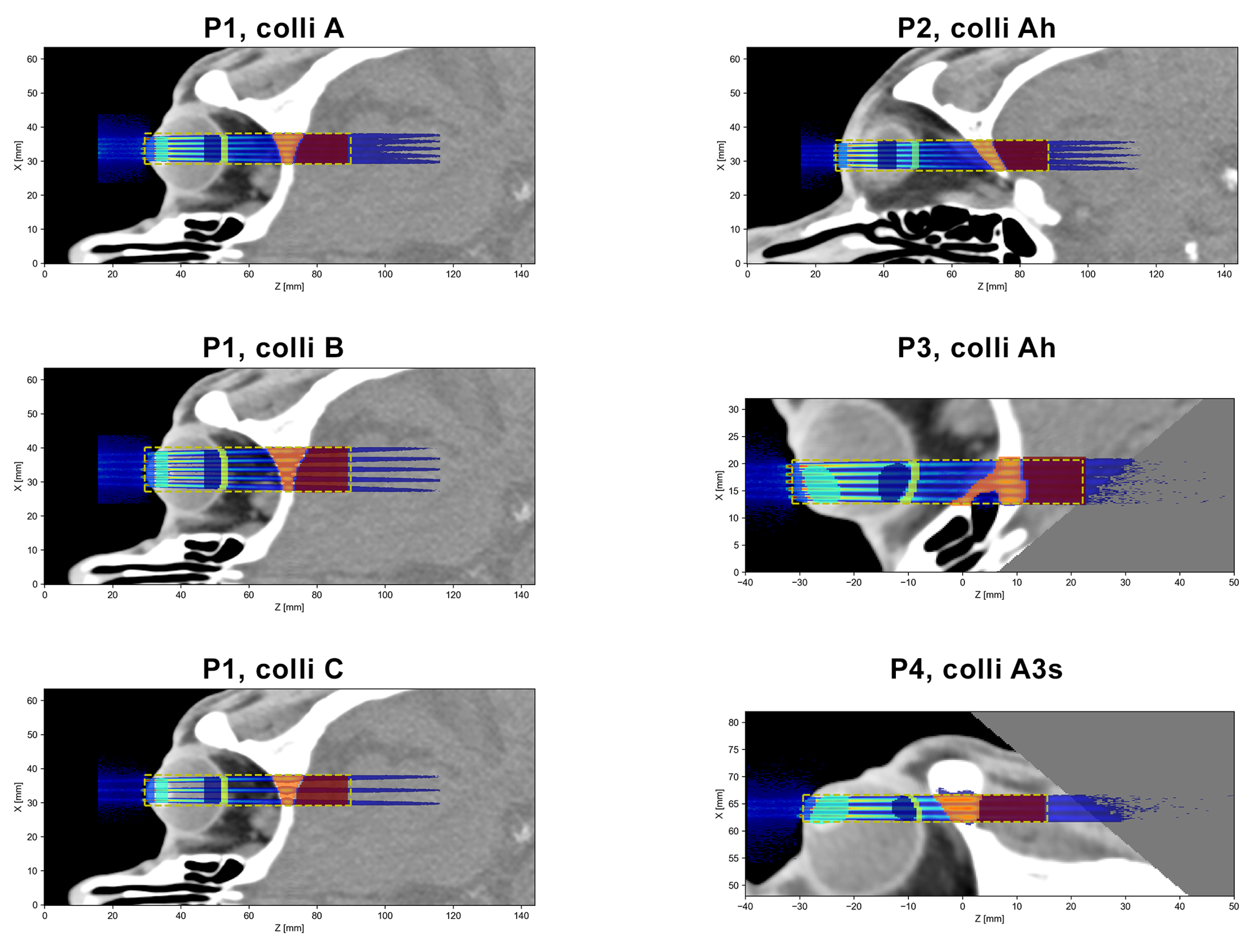
3. Results
4. Discussion
| Study | Model | Configuration | FWHM/ctc [mm] | Mean/Peak/Valley Dose [Gy] | Results/Observations |
|---|---|---|---|---|---|
| Bazyar et al., 2017 [9] | normal mouse skin | single array | 0.25/0.93 | n.r./150/∼6.5 | no radiation side effects |
| mouse melanoma model | single array | 0.25/0.93 | n.r./150/∼6.5 | MBRT more effective than conv. RT (slower growth rate, longer mean survival) | |
| Bertho et al., 2022 [20] | glioma-bearing rat brain | single array | 0.7/1.4 | 30/83/4.5 | 33% long-term survival; no skin toxicity (immunocompetent rats) |
| Dilmanian et al., 2006 [28] | normal rat spinal cord | single array | 0.68/4 | n.r./400/n.r. | irradiation tolerated long-term by 3/4 rats; lag in weight gain with respect to unirradiated controls |
| Deman et al., 2012 [8] | normal rat brain | single array | 0.62/1.22 | n.r./123/∼4.1 | no clinical alteration or MRI images abnormalities |
| glioma-bearing rat brain | two arrays, interleaved | 0.62/1.22 | 54 (homog. in target) | significantly increased survival with respect to untreated controls | |
| Prezado et al., 2012 [29] | glioma-bearing rat brain | single array | 0.64/1.12 | n.r./180/16 | no benefit with respect to untreated controls |
| glioma-bearing rat brain | two arrays, interleaved | 0.64/1.12 | 70–100 (homog. in target) | significantly increased survival with respect to untreated controls | |
| Prezado et al., 2015 [7] | normal rat brain | single array | 0.6/1.2 | n.r./100/6.6 | alive 560 days after irradiation, normal behaviour; signs of haemorrhage, small vascular damage; microcalcifications in histological analysis |
| Prezado et al., 2017 [12] | normal rat brain | single array | 0.97/1.61 | 20/58/4.7 | no brain damage in whole-brain irradiation |
| Sotiropoulos et al., 2021 [17] | glioma-bearing rat brain | single array | 0.70/1.47 | 28/81/7.2 | significantly increased survival with respect to untreated controls |
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CT | computed tomography |
| FWHM | full width at half maximum |
| MBRT | minibeam radiation therapy |
| OAR | organ at risk |
| PVDR | peak-to-valley dose ratio |
| SARRP | Small Animal Radiation Research Platform |
| ROI | region of interest |
| RT | radiation therapy |
References
- Stannard, C.; Sauerwein, W.; Maree, G.; Lecuona, K. Radiotherapy for ocular tumours. Eye 2013, 27, 119–127. [Google Scholar] [CrossRef]
- Reichstein, D.A.; Brock, A.L. Radiation therapy for uveal melanoma: A review of treatment methods available in 2021. Curr. Opin. Ophthalmol. 2021, 32, 183–190. [Google Scholar] [CrossRef] [PubMed]
- Jager, M.J.; Shields, C.L.; Cebulla, C.M.; Abdel-Rahman, M.H.; Grossniklaus, H.E.; Stern, M.H.; Carvajal, R.D.; Belfort, R.N.; Jia, R.; Shields, J.A.; et al. Uveal melanoma. Nat. Rev. Dis. Prim. 2020, 6, 24. [Google Scholar] [CrossRef]
- Dendale, R.; Lumbroso-Le Rouic, L.; Noel, G.; Feuvret, L.; Levy, C.; Delacroix, S.; Meyer, A.; Nauraye, C.; Mazal, A.; Mammar, H.; et al. Proton beam radiotherapy for uveal melanoma: Results of Curie Institut-Orsay proton therapy center (ICPO). Int. J. Radiat. Oncol. Biol. Phys. 2006, 65, 780–787. [Google Scholar] [CrossRef]
- Toutée, A.; Angi, M.; Dureau, S.; Lévy-Gabriel, C.; Rouic, L.L.L.; Dendale, R.; Desjardins, L.; Cassoux, N. Long-Term Visual Outcomes for Small Uveal Melanoma Staged T1 Treated by Proton Beam Radiotherapy. Cancers 2019, 11, 1047. [Google Scholar] [CrossRef] [PubMed]
- Prezado, Y. Divide and conquer: Spatially fractionated radiation therapy. Expert Rev. Mol. Med. 2022, 24, e3. [Google Scholar] [CrossRef]
- Prezado, Y.; Deman, P.; Varlet, P.; Jouvion, G.; Gil, S.; Le Clec’H, C.; Bernard, H.; Le Duc, G.; Sarun, S. Tolerance to Dose Escalation in Minibeam Radiation Therapy Applied to Normal Rat Brain: Long-Term Clinical, Radiological and Histopathological Analysis. Radiat. Res. 2015, 184, 314–321. [Google Scholar] [CrossRef] [PubMed]
- Deman, P.; Vautrin, M.; Edouard, M.; Stupar, V.; Bobyk, L.; Farion, R.; Elleaume, H.; Rémy, C.; Barbier, E.L.; Estève, F.; et al. Monochromatic minibeams radiotherapy: From healthy tissue-sparing effect studies toward first experimental glioma bearing rats therapy. Int. J. Radiat. Oncol. Biol. Phys. 2012, 82, e693–e700. [Google Scholar] [CrossRef]
- Bazyar, S.; Inscoe, C.R.; O’Brian, E.T.; Zhou, O.; Lee, Y.Z. Minibeam radiotherapy with small animal irradiators; in vitro and in vivo feasibility studies. Phys. Med. Biol. 2017, 62, 8924–8942. [Google Scholar] [CrossRef]
- Meyer, J.; Eley, J.; Schmid, T.E.; Combs, S.E.; Dendale, R.; Prezado, Y. Spatially fractionated proton minibeams. Br. J. Radiol. 2019, 92, 20180466. [Google Scholar] [CrossRef]
- Prezado, Y.; Thengumpallil, S.; Renier, M.; Bravin, A. X-ray energy optimization in minibeam radiation therapy. Med. Phys. 2009, 36, 4897–4902. [Google Scholar] [CrossRef] [PubMed]
- Prezado, Y.; Dos Santos, M.; Gonzalez, W.; Jouvion, G.; Guardiola, C.; Heinrich, S.; Labiod, D.; Juchaux, M.; Jourdain, L.; Sebrie, C.; et al. Transfer of Minibeam Radiation Therapy into a cost-effective equipment for radiobiological studies: A proof of concept. Sci. Rep. 2017, 7, 17295. [Google Scholar] [CrossRef] [PubMed]
- Wong, J.; Armour, E.; Kazanzides, P.; Iordachita, I.; Tryggestad, E.; Deng, H.; Matinfar, M.; Kennedy, C.; Liu, Z.; Chan, T.; et al. High-resolution, small animal radiation research platform with x-ray tomographic guidance capabilities. Int. J. Radiat. Oncol. Biol. Phys. 2008, 71, 1591–1599. [Google Scholar] [CrossRef] [PubMed]
- Perl, J.; Shin, J.; Schumann, J.; Faddegon, B.; Paganetti, H. TOPAS: An innovative proton Monte Carlo platform for research and clinical applications. Med. Phys. 2012, 39, 6818–6837. [Google Scholar] [CrossRef]
- Faddegon, B.; Ramos-Méndez, J.; Schuemann, J.; McNamara, A.; Shin, J.; Perl, J.; Paganetti, H. The TOPAS tool for particle simulation, a Monte Carlo simulation tool for physics, biology and clinical research. Phys. Med. 2020, 72, 114–121. [Google Scholar] [CrossRef]
- González, W.; Dos Santos, M.; Guardiola, C.; Delorme, R.; Lamirault, C.; Juchaux, M.; Le Dudal, M.; Jouvion, G.; Prezado, Y. Minibeam radiation therapy at a conventional irradiator: Dose-calculation engine and first tumor-bearing animals irradiation. Phys. Med. 2020, 69, 256–261. [Google Scholar] [CrossRef]
- Sotiropoulos, M.; Brisebard, E.; Le Dudal, M.; Jouvion, G.; Juchaux, M.; Crépin, D.; Sebrie, C.; Jourdain, L.; Labiod, D.; Lamirault, C.; et al. X-rays minibeam radiation therapy at a conventional irradiator: Pilot evaluation in F98-glioma bearing rats and dose calculations in a human phantom. Clin. Transl. Radiat. Oncol. 2021, 27, 44–49. [Google Scholar] [CrossRef]
- Arce, P.; Lagares, J.I.; Azcona, J.D.; Aguilar-Redondo, P.B. A proposal for a Geant4 physics list for radiotherapy optimized in physics performance and CPU time. Nucl. Instrum. Methods Phys. Res. Sect. Accel. Spectrometers Detect. Assoc. Equip. 2020, 964, 163755. [Google Scholar] [CrossRef]
- Arce, P.; Bolst, D.; Bordage, M.C.; Brown, J.M.C.; Cirrone, P.; Cortés-Giraldo, M.A.; Cutajar, D.; Cuttone, G.; Desorgher, L.; Dondero, P.; et al. Report on G4-Med, a Geant4 benchmarking system for medical physics applications developed by the Geant4 Medical Simulation Benchmarking Group. Med. Phys. 2021, 48, 19–56. [Google Scholar] [CrossRef]
- Bertho, A.; Iturri, L.; Brisebard, E.; Juchaux, M.; Gilbert, C.; Ortiz, R.; Sebrie, C.; Jourdain, L.; Lamirault, C.; Ramasamy, G.; et al. Evaluation of the Role of the Immune System Response After Minibeam Radiation Therapy. Int. J. Radiat. Oncol. Biol. Phys. 2023, 115, 426–439. [Google Scholar] [CrossRef]
- Desjardins, L.; Lumbroso-Le Rouic, L.; Levy-Gabriel, C.; Cassoux, N.; Dendale, R.; Mazal, A.; Delacroix, S.; Sastre, X.; Plancher, C.; Asselain, B. Treatment of uveal melanoma by accelerated proton beam. Dev. Ophthalmol. 2012, 49, 41–57. [Google Scholar] [CrossRef] [PubMed]
- Sikuade, M.J.; Salvi, S.; Rundle, P.A.; Errington, D.G.; Kacperek, A.; Rennie, I.G. Outcomes of treatment with stereotactic radiosurgery or proton beam therapy for choroidal melanoma. Eye 2015, 29, 1194–1198. [Google Scholar] [CrossRef]
- Akbaba, S.; Foerster, R.; Nicolay, N.H.; Arians, N.; Bostel, T.; Debus, J.; Hauswald, H. Linear accelerator-based stereotactic fractionated photon radiotherapy as an eye-conserving treatment for uveal melanoma. Radiat. Oncol. 2018, 13, 140. [Google Scholar] [CrossRef]
- Fernandez-Palomo, C.; Chang, S.; Prezado, Y. Should Peak Dose Be Used to Prescribe Spatially Fractionated Radiation Therapy?—A Review of Preclinical Studies. Cancers 2022, 14, 3625. [Google Scholar] [CrossRef] [PubMed]
- Dilmanian, F.A.; Button, T.M.; Le Duc, G.; Zhong, N.; Peña, L.A.; Smith, J.A.L.; Martinez, S.R.; Bacarian, T.; Tammam, J.; Ren, B.; et al. Response of rat intracranial 9L gliosarcoma to microbeam radiation therapy. Neuro Oncol. 2002, 4, 26–38. [Google Scholar] [CrossRef]
- Bazyar, S.; Inscoe, C.R.; Benefield, T.; Zhang, L.; Lu, J.; Zhou, O.; Lee, Y.Z. Neurocognitive sparing of desktop microbeam irradiation. Radiat. Oncol. 2017, 12, 127. [Google Scholar] [CrossRef]
- Finger, P.T. Radiation therapy for orbital tumors: Concepts, current use, and ophthalmic radiation side effects. Surv. Ophthalmol. 2009, 54, 545–568. [Google Scholar] [CrossRef] [PubMed]
- Dilmanian, F.A.; Zhong, Z.; Bacarian, T.; Benveniste, H.; Romanelli, P.; Wang, R.; Welwart, J.; Yuasa, T.; Rosen, E.M.; Anschel, D.J. Interlaced X-ray microplanar beams: A radiosurgery approach with clinical potential. Proc. Natl. Acad. Sci. USA 2006, 103, 9709–9714. [Google Scholar] [CrossRef]
- Prezado, Y.; Sarun, S.; Gil, S.; Deman, P.; Bouchet, A.; Le Duc, G. Increase of lifespan for glioma-bearing rats by using minibeam radiation therapy. J. Synchrotron. Radiat. 2012, 19, 60–65. [Google Scholar] [CrossRef]
- Parsons, J.T.; Bova, F.J.; Fitzgerald, C.R.; Mendenhall, W.M.; Million, R.R. Radiation optic neuropathy after megavoltage external-beam irradiation: Analysis of time-dose factors. Int. J. Radiat. Oncol. Biol. Phys. 1994, 30, 755–763. [Google Scholar] [CrossRef]
- Morales, S.A.; Lamond, J.P.; Lally, S.; Asbell, S.O.; Yang, J.; Lanciano, R.; Brady, L.W. Experience using frameless fractionated radiosurgery for the treatment of orbital and ocular tumors. J. Radiat. Oncol. 2012, 1, 49–55. [Google Scholar] [CrossRef]
- Guardiola, C.; Prezado, Y.; Roulin, C.; Bergs, J.W.J. Effect of X-ray minibeam radiation therapy on clonogenic survival of glioma cells. Clin. Transl. Radiat. Oncol. 2018, 13, 7–13. [Google Scholar] [CrossRef] [PubMed]


| Case | Global Relative Mean Dose Uncertainty |
|---|---|
| P1, colli A | 2.4% |
| P1, colli B | 2.7% |
| P1, colli C | 3.8% |
| P2, colli Ah | 2.6% |
| P3, colli Ah | 2.3% |
| P4, colli A3s | 1.9% |
| Position | Depth [cm] | FWHM/ctc [mm] | ||
|---|---|---|---|---|
| Colli A | Colli B | Colli C | ||
| Surface | 0 | 0.69/1.3 | 0.64/2.2 | 0.64/2.9 |
| Target | 2 | 0.90/1.5 | 0.86/2.4 | 0.85/3.1 |
| Case | Volume | [%] | [%] | [%] | PVDR |
|---|---|---|---|---|---|
| P1, colli A | target | 100 | 164 | 37 | 4.5 |
| anterior chamber | 163 | 327 | 26 | 12.8 | |
| lens | 179 | 338 | 35 | 9.8 | |
| retina | 83 | 138 | 36 | 3.8 | |
| bone | 107 | 193 | 71 | 2.7 | |
| brain | 37 | 61–44 | 26–22 | 2.3–2.0 | |
| P1, colli B | target | 100 | 227 | 18 | 12.6 |
| anterior chamber | 157 | 468 | 16 | 30.2 | |
| lens | 176 | 475 | 24 | 20.0 | |
| retina | 86 | 194 | 15 | 13.4 | |
| bone | 108 | 265 | 33 | 7.9 | |
| brain | 38 | 83–62 | 11–10 | 7.8–6.4 | |
| P1, colli C | target | 100 | 251 | 13 | 19.3 |
| anterior chamber | 154 | 527 | 12 | 44.6 | |
| lens | 180 | 533 | 18 | 29.0 | |
| retina | 85 | 215 | 11 | 19.8 | |
| bone | 108 | 288 | 25 | 11.6 | |
| brain | 38 | 90–67 | 6–6 | 14.7–10.4 | |
| P2, colli Ah | target | 100 | 196 | 29 | 6.9 |
| skin | 162 | 341 | 21 | 16.6 | |
| retina | 77 | 129 | 27 | 4.9 | |
| bone | 86 | 157 | 47 | 3.4 | |
| brain | 33 | 50–39 | 22–19 | 2.3–2.0 | |
| P3, colli Ah | target | 100 | 174 | 29 | 6.0 |
| anterior chamber | 162 | 363 | 19 | 19.6 | |
| lens | 165 | 357 | 27 | 13.2 | |
| retina | 87 | 154 | 28 | 5.4 | |
| bone | 101 | 222 | 64 | 3.5 | |
| brain | 40 | 60–47 | 22–20 | 2.7–2.4 | |
| P4, colli A3s | target | 100 | 175 | 29 | 6.0 |
| anterior chamber | 155 | 336 | 17 | 19.9 | |
| lens | 141 | 358 | 24 | 15.1 | |
| retina | 88 | 157 | 30 | 5.2 | |
| bone | 141 | 276 | 67 | 4.1 | |
| tissue | 48 | 90–66 | 28–25 | 3.2–2.6 |
| Case | Volume | [Gy] | [Gy] | [Gy] | PVDR |
|---|---|---|---|---|---|
| P1, colli A | target | 30.0 | 49.1 | 11.0 | 4.5 |
| anterior chamber | 48.8 | 98.2 | 7.7 | 12.8 | |
| lens | 53.5 | 101.4 | 10.4 | 9.8 | |
| retina | 24.8 | 41.4 | 10.8 | 3.8 | |
| bone | 32.0 | 57.8 | 21.3 | 2.7 | |
| brain | 11.2 | 18.2–13.2 | 7.8–6.5 | 2.3–2.0 | |
| P1, colli B | target | 30.0 | 68.2 | 5.4 | 12.6 |
| anterior chamber | 47.1 | 140.4 | 4.7 | 30.2 | |
| lens | 52.6 | 142.5 | 7.1 | 20.0 | |
| retina | 25.7 | 58.1 | 4.3 | 13.4 | |
| bone | 32.4 | 79.5 | 10.0 | 7.9 | |
| brain | 11.3 | 24.9–18.5 | 3.2–2.9 | 7.8–6.4 | |
| P1, colli C | target | 30.0 | 75.3 | 3.9 | 19.3 |
| anterior chamber | 46.3 | 158.0 | 3.5 | 44.6 | |
| lens | 54.0 | 159.8 | 5.5 | 29.0 | |
| retina | 25.4 | 64.6 | 3.3 | 19.8 | |
| bone | 32.5 | 86.4 | 7.5 | 11.6 | |
| brain | 11.3 | 27.0–20.0 | 1.8–1.9 | 14.7–10.4 | |
| P2, colli Ah | target | 30.0 | 58.9 | 8.5 | 6.9 |
| skin | 48.7 | 102.3 | 6.2 | 16.6 | |
| retina | 23.2 | 38.8 | 7.9 | 4.9 | |
| bone | 25.7 | 47.2 | 14.0 | 3.4 | |
| brain | 9.8 | 15.1–11.7 | 6.5–5.8 | 2.3-2.0 | |
| P3, colli Ah | target | 30.0 | 52.1 | 8.7 | 6.0 |
| anterior chamber | 48.5 | 108.9 | 5.6 | 19.6 | |
| lens | 49.6 | 107.0 | 8.1 | 13.2 | |
| retina | 25.9 | 46.2 | 8.5 | 5.4 | |
| bone | 30.3 | 66.6 | 19.3 | 3.5 | |
| brain | 11.9 | 18.1–14.2 | 6.6-6.0 | 2.7–2.4 | |
| P4, colli A3s | target | 30.0 | 52.4 | 8.7 | 6.0 |
| anterior chamber | 46.6 | 100.8 | 5.1 | 19.9 | |
| lens | 42.4 | 107.5 | 7.1 | 15.1 | |
| retina | 26.3 | 47.0 | 9.0 | 5.2 | |
| bone | 42.2 | 82.8 | 20.0 | 4.1 | |
| tissue | 14.3 | 26.9–19.8 | 8.3–7.6 | 3.2–2.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schneider, T.; Malaise, D.; Pouzoulet, F.; Prezado, Y. Orthovoltage X-ray Minibeam Radiation Therapy for the Treatment of Ocular Tumours—An In Silico Evaluation. Cancers 2023, 15, 679. https://doi.org/10.3390/cancers15030679
Schneider T, Malaise D, Pouzoulet F, Prezado Y. Orthovoltage X-ray Minibeam Radiation Therapy for the Treatment of Ocular Tumours—An In Silico Evaluation. Cancers. 2023; 15(3):679. https://doi.org/10.3390/cancers15030679
Chicago/Turabian StyleSchneider, Tim, Denis Malaise, Frédéric Pouzoulet, and Yolanda Prezado. 2023. "Orthovoltage X-ray Minibeam Radiation Therapy for the Treatment of Ocular Tumours—An In Silico Evaluation" Cancers 15, no. 3: 679. https://doi.org/10.3390/cancers15030679
APA StyleSchneider, T., Malaise, D., Pouzoulet, F., & Prezado, Y. (2023). Orthovoltage X-ray Minibeam Radiation Therapy for the Treatment of Ocular Tumours—An In Silico Evaluation. Cancers, 15(3), 679. https://doi.org/10.3390/cancers15030679









