MEMS Device for Quantitative In Situ Mechanical Testing in Electron Microscope
Abstract
:1. Introduction
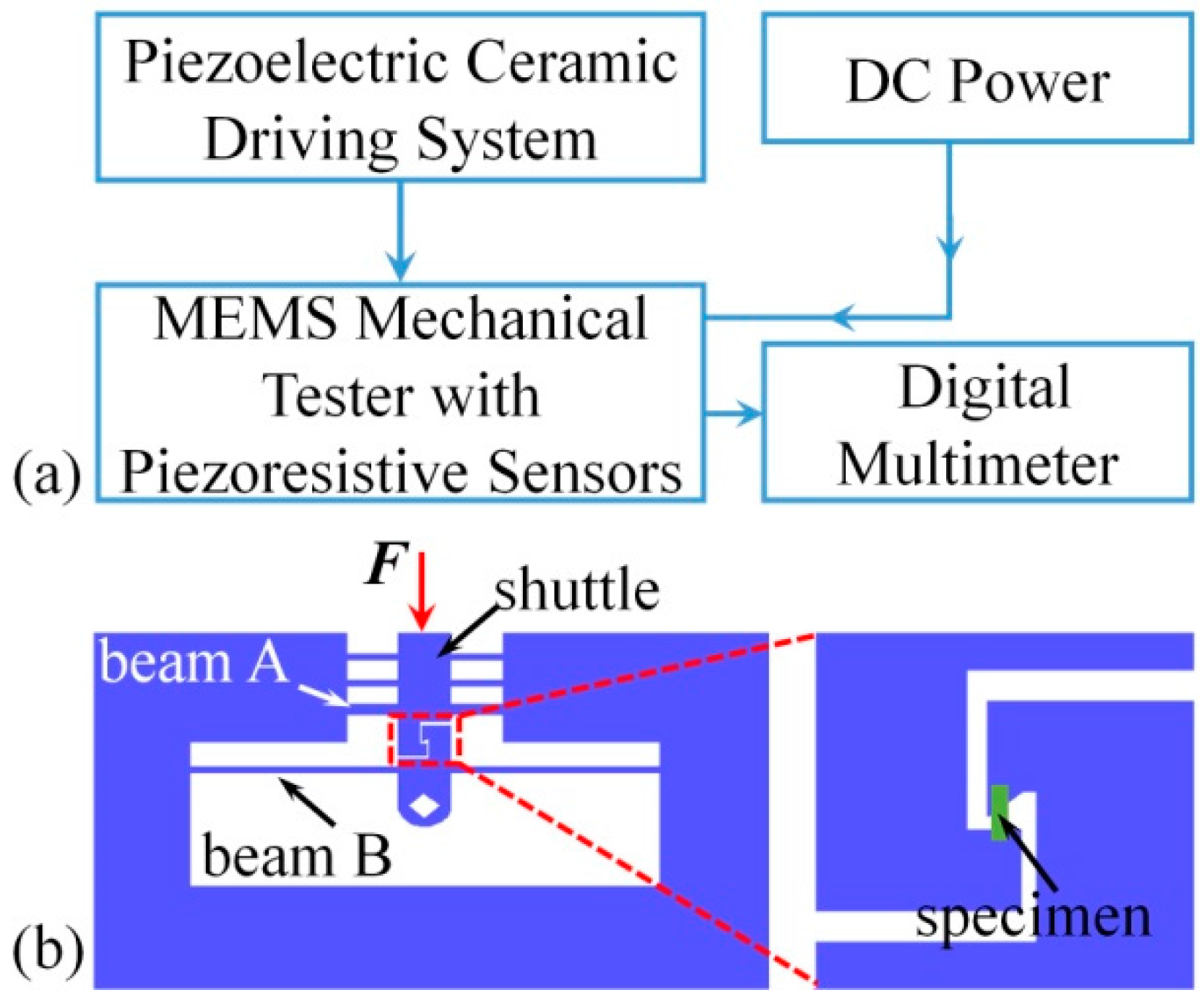
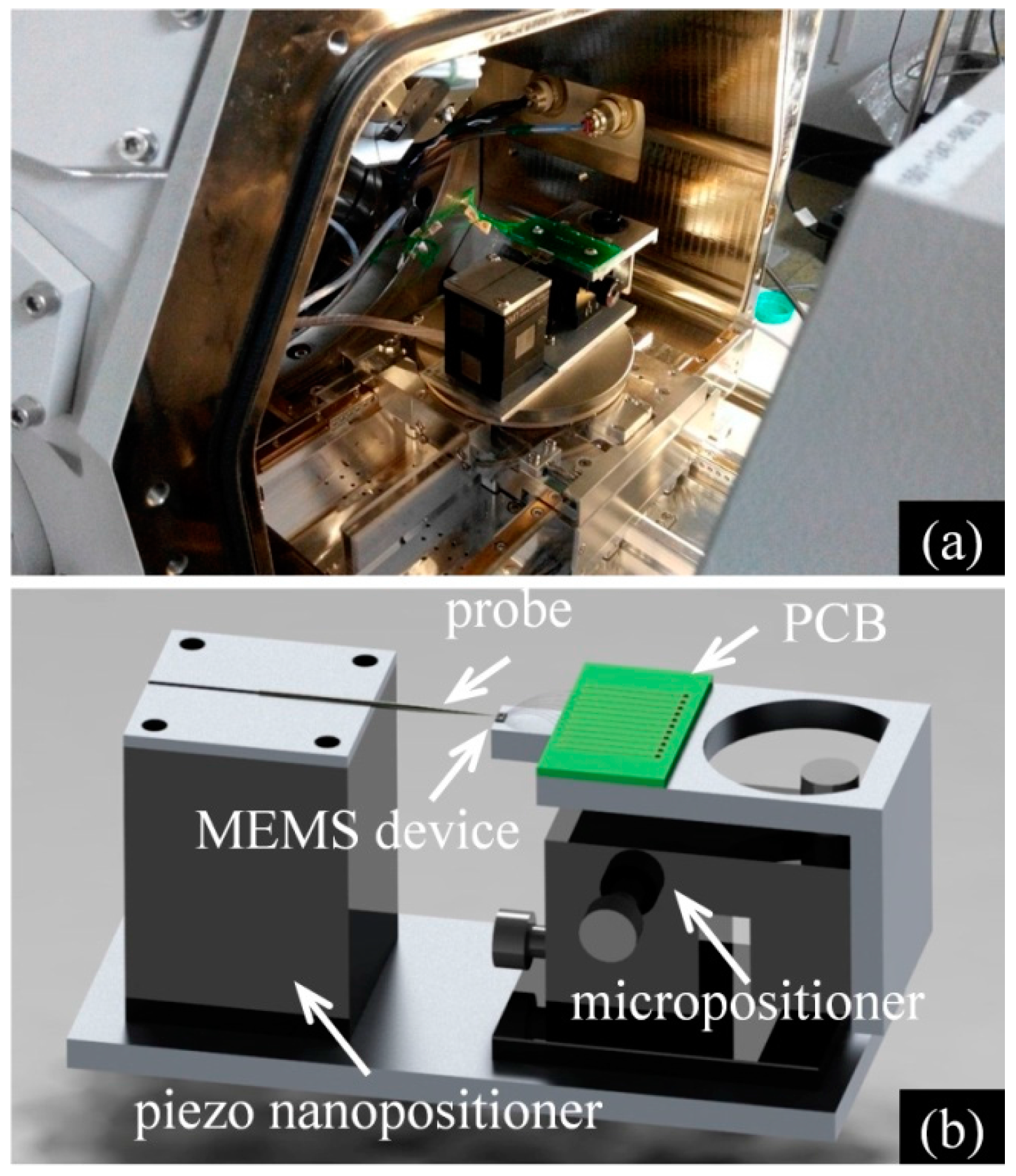
2. Mechanical Testing System
2.1. Description of the System
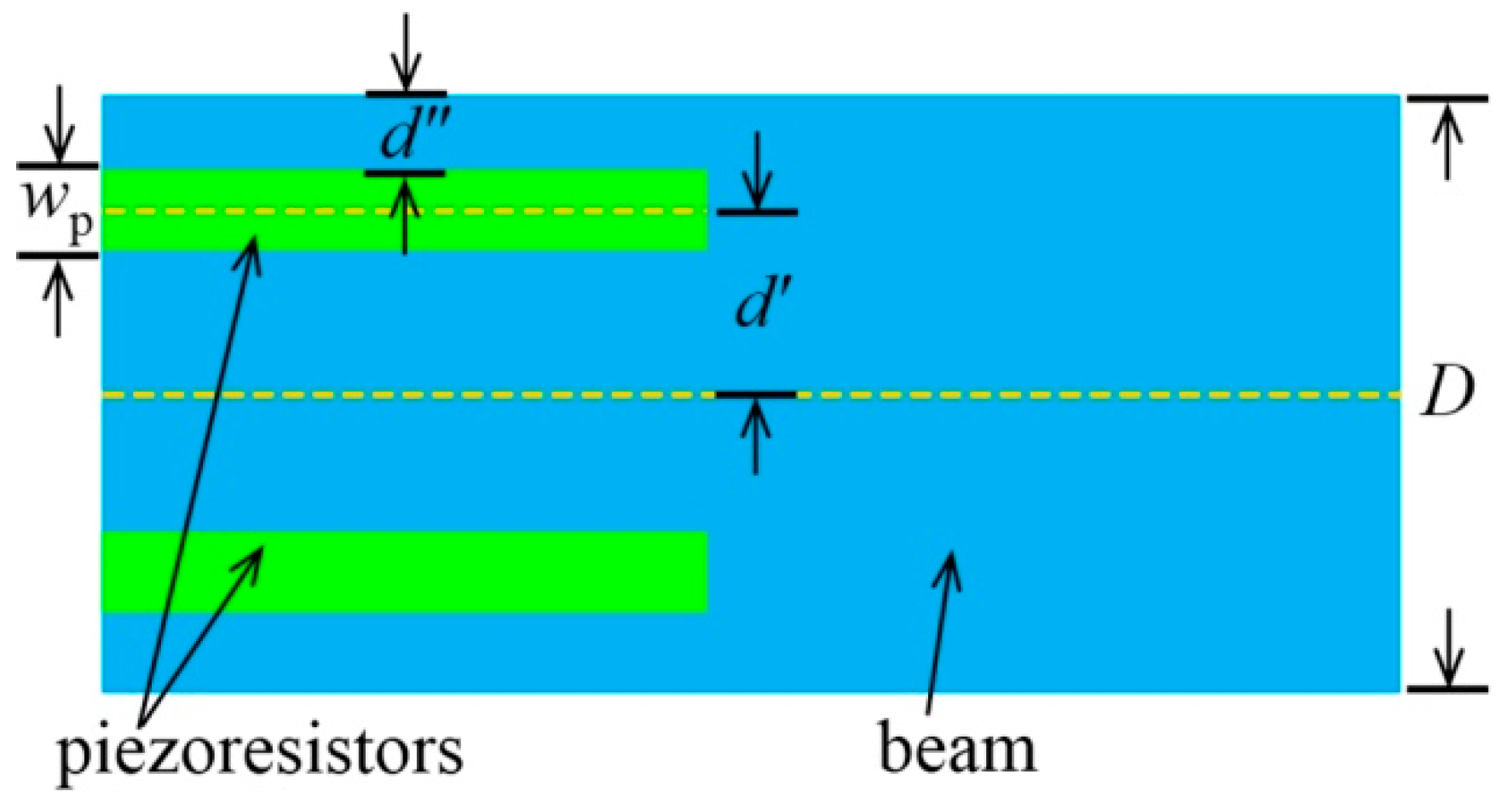
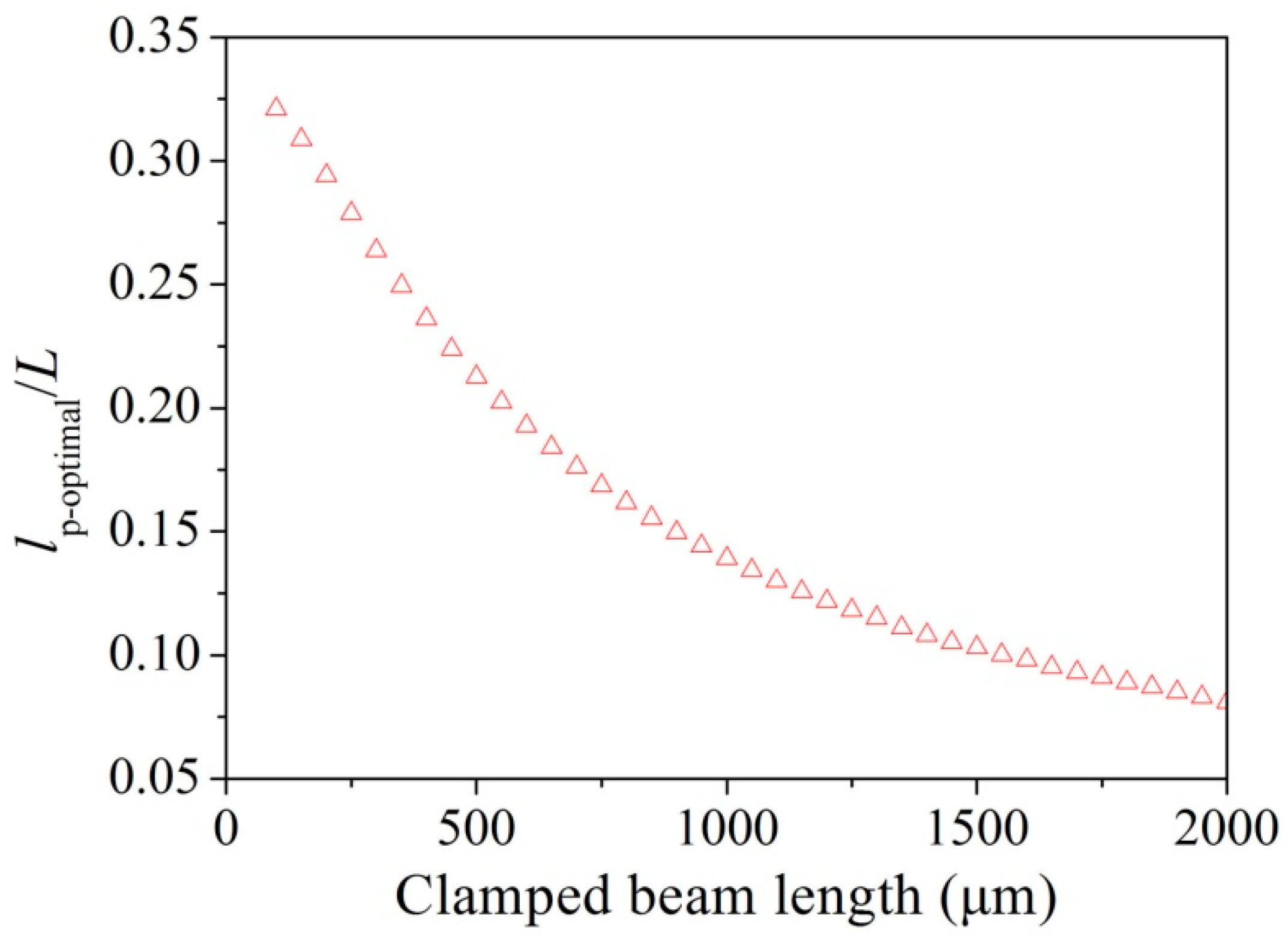
2.2. Sensor Design
3. Experiment
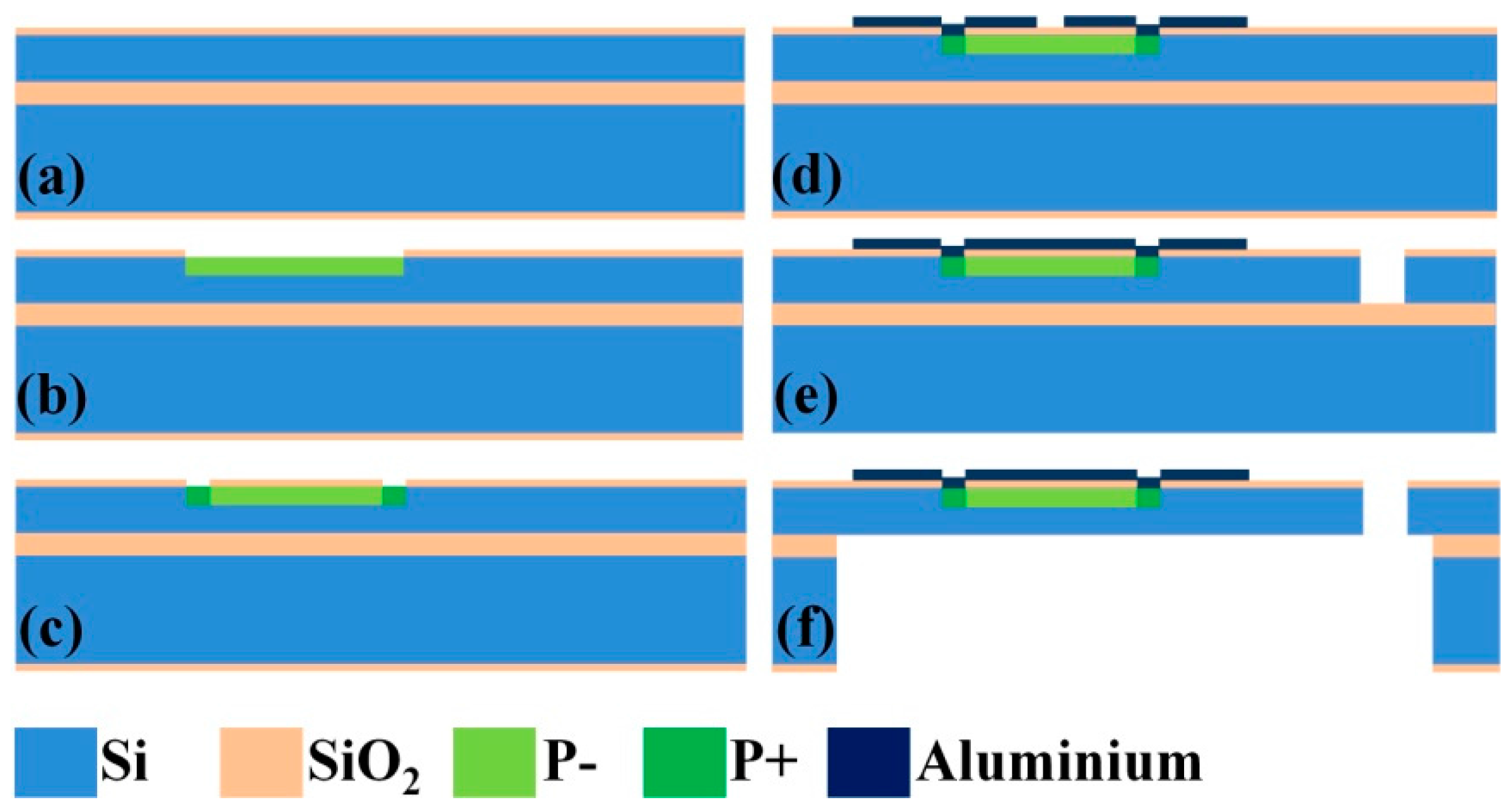
3.1. Fabrication Process
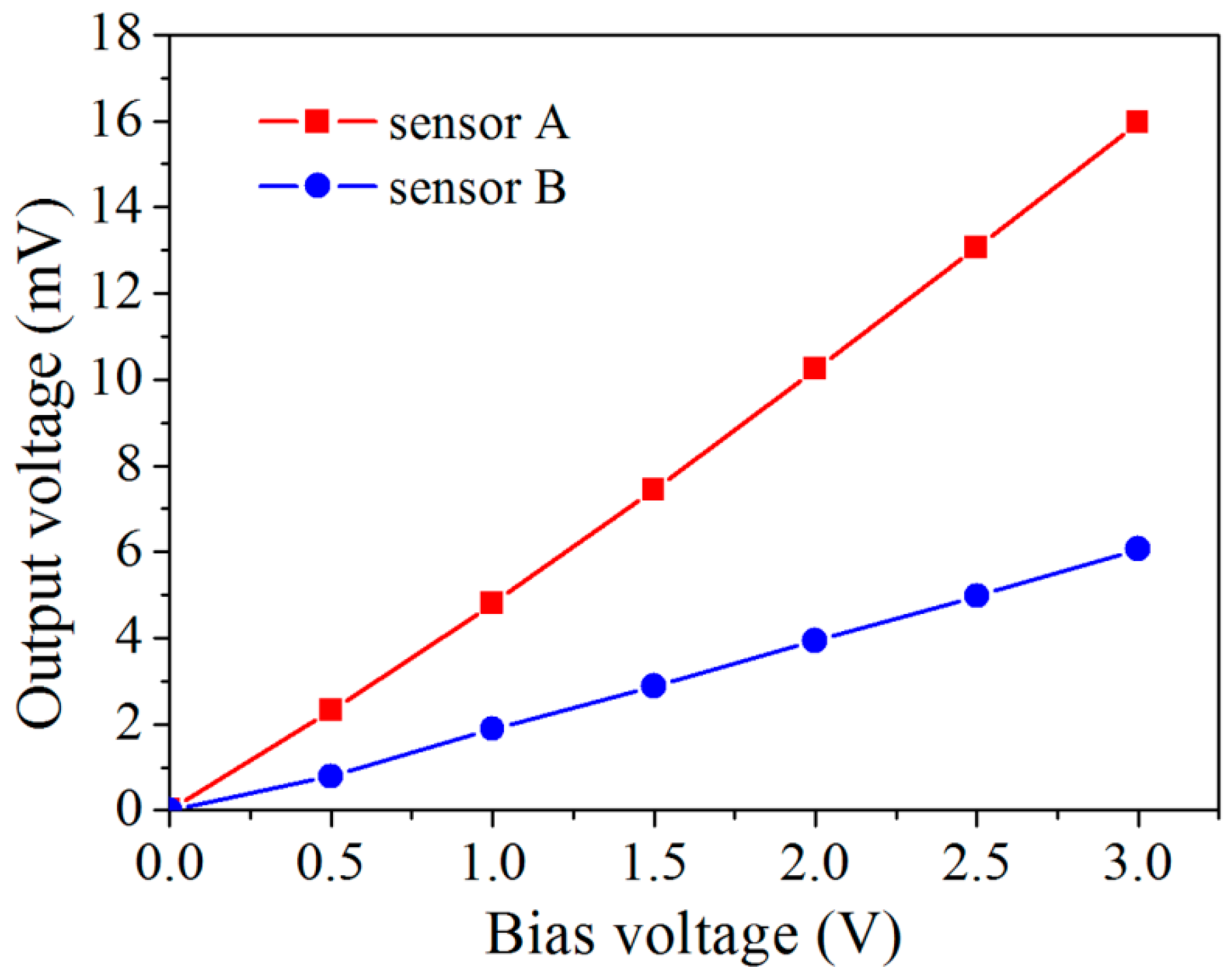
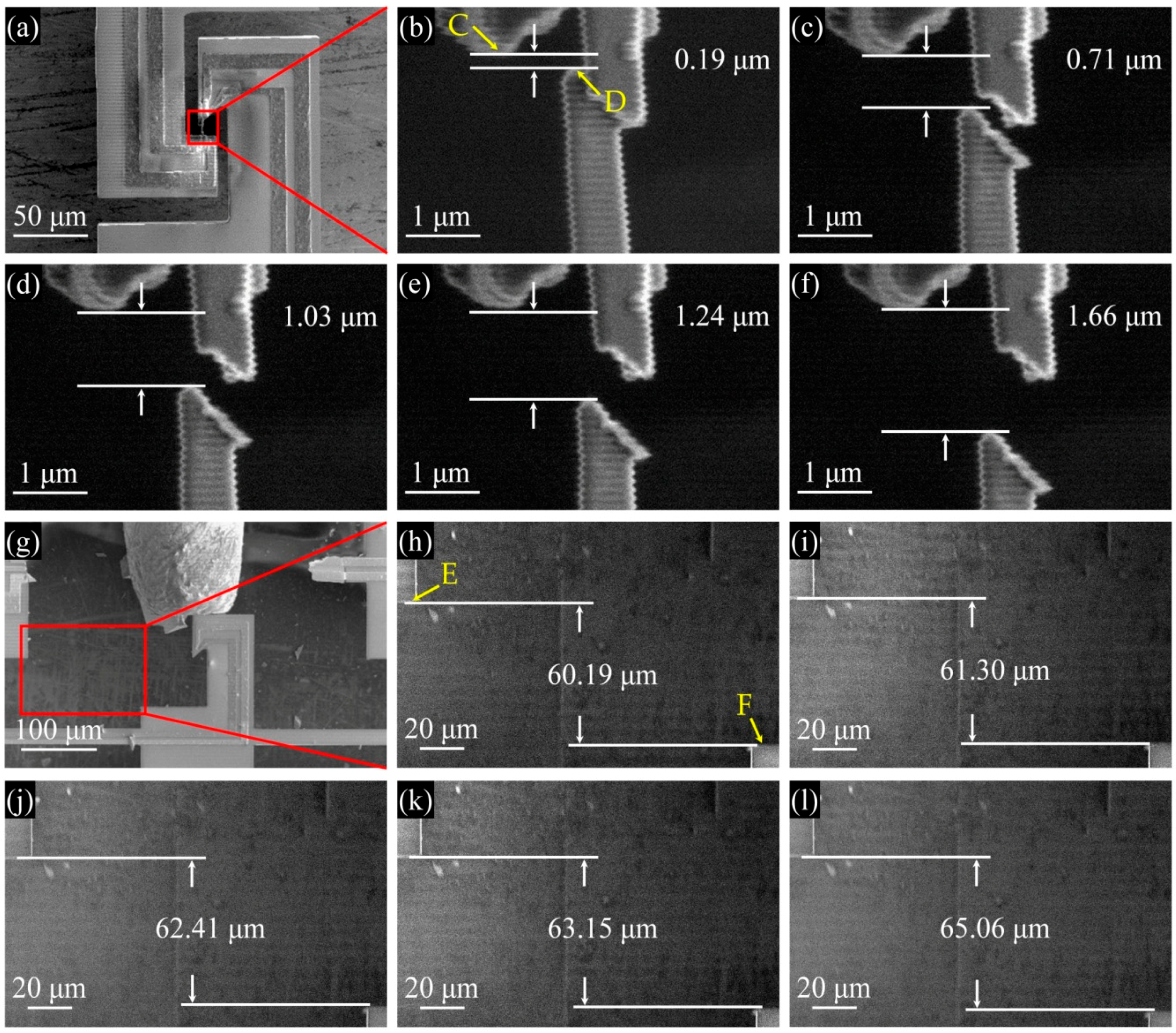
3.2. Device Calibration and Quantitative Tensile Testing
3.3. Specimen Preparation
4. Results and Discussion
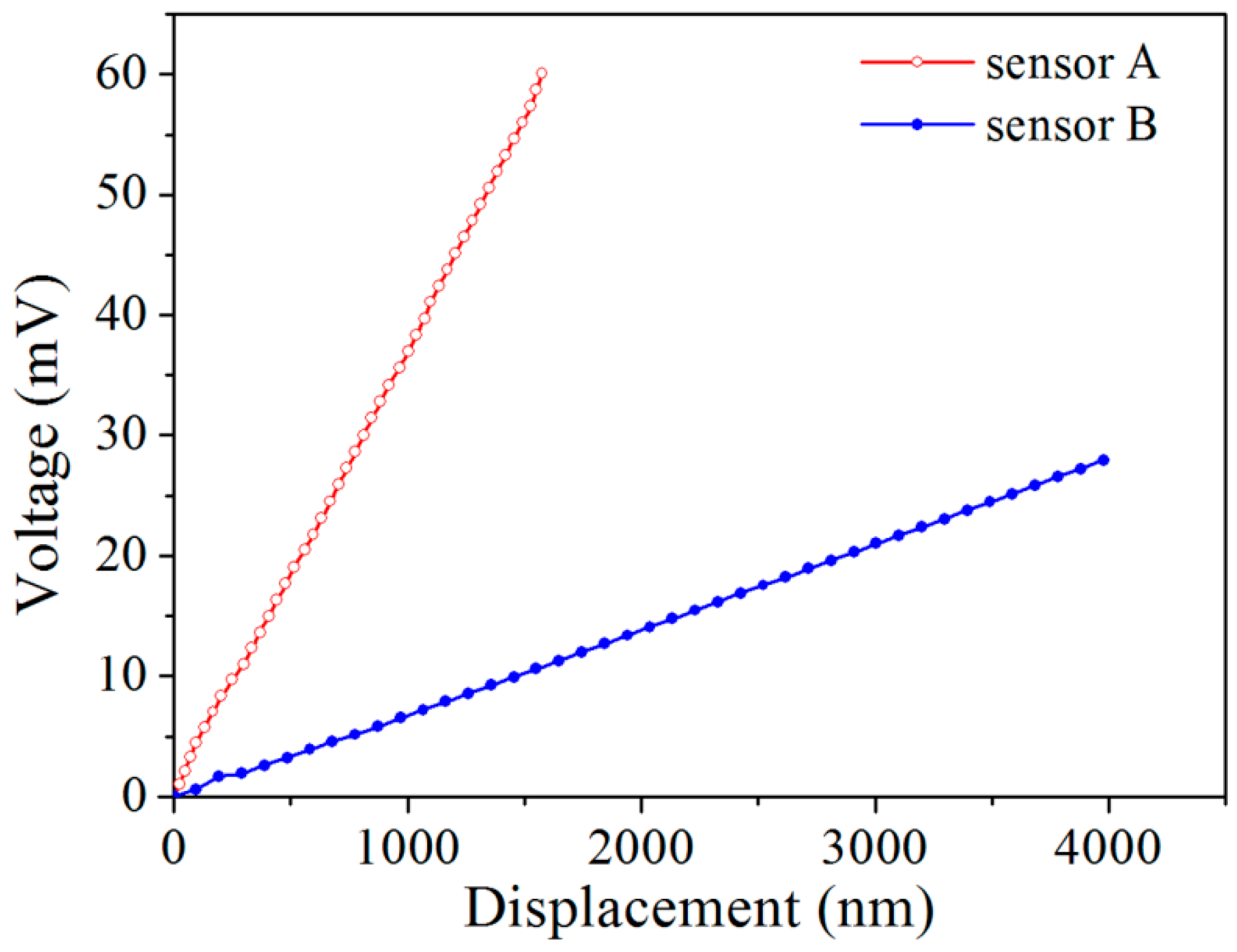
4.1. Sensors Performance
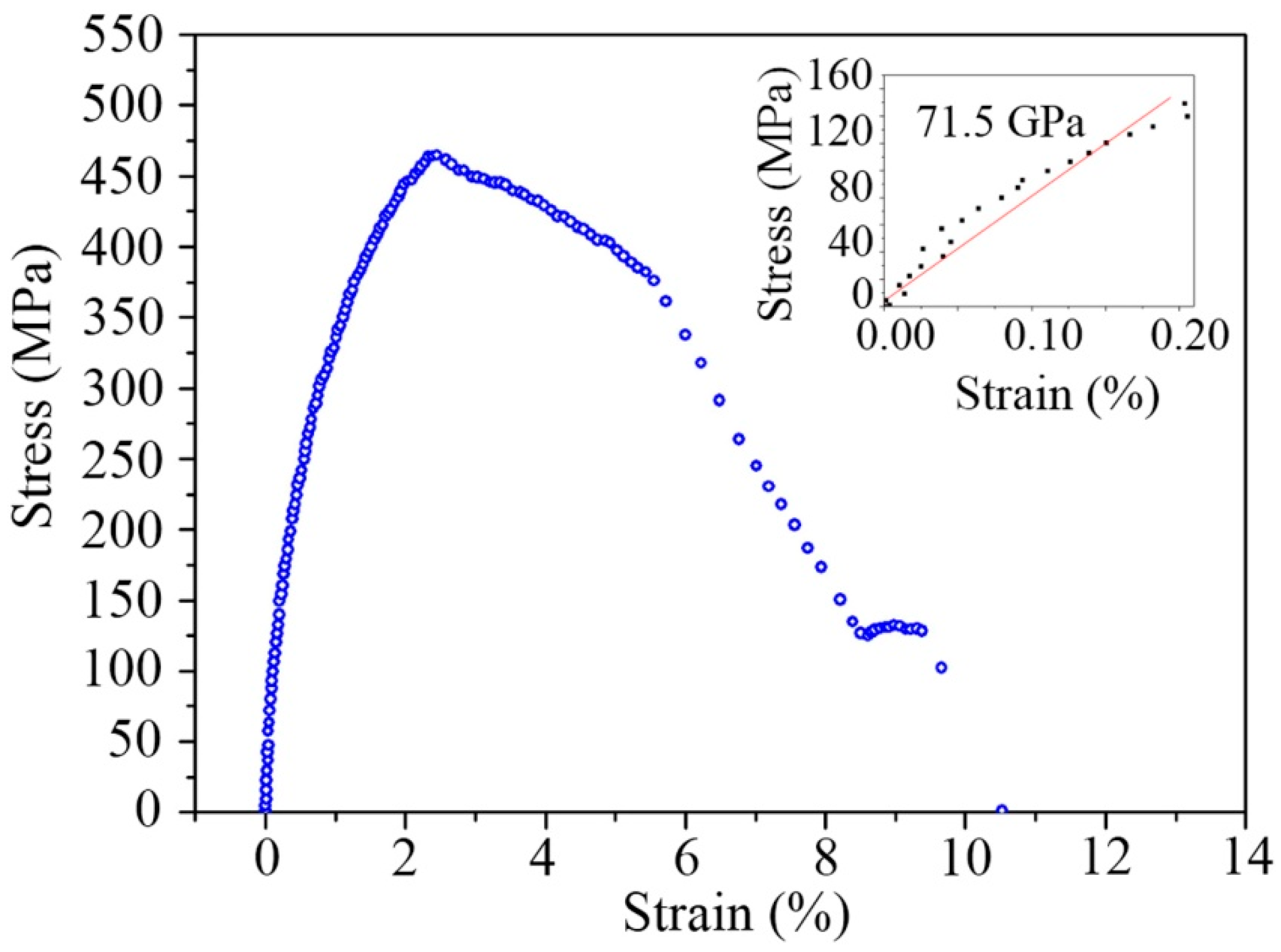
4.2. Stress–Strain Curve
5. Conclusions
- (1)
- Sensors A and B have displacement sensitivities of 37.4 μV/nm and 4.8 μV/nm.
- (2)
- Sensor A has a theoretical displacement resolution of 0.19 nm and sensor B has a force resolution of 2.1 μN.
- (3)
- The MEMS device has a displacement range limit of 5.47 μm and a theoretical load range limit of 55.0 mN.
- (4)
- Measurement of the Young’s modulus of the Al film by the device verifies the reliability of the sensors.
- (5)
- The device has a dimension small enough to be integrated on the TEM holder to study the property–structure correlation at the atomic scale.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Arzt, E. Size effects in materials due to microstructural and dimensional constraints: A comparative review. Acta Mater. 1998, 46, 5611–5626. [Google Scholar] [CrossRef]
- Tsuchiya, T.; Tabata, O.; Sakata, J.; Taga, Y. Specimen size effect on tensile strength of surface-micromachined polycrystalline silicon thin films. J. Microelectromech. Syst. 1998, 7, 106–113. [Google Scholar] [CrossRef]
- Namazu, T.; Isono, Y.; Tanaka, T. Evaluation of size effect on mechanical properties of single crystal silicon by nanoscale bending test using AFM. J. Microelectromech. Syst. 2000, 9, 450–459. [Google Scholar] [CrossRef]
- Sharpe, W.N.; Jackson, K.M.; Hemker, K.J.; Xie, Z. Effect of specimen size on Young’s modulus and fracture strength of polysilicon. J. Microelectromech. Syst. 2001, 10, 317–326. [Google Scholar] [CrossRef]
- Uchic, M.D.; Dimiduk, D.M.; Florando, J.N.; Nix, W.D. Sample dimensions influence strength and crystal plasticity. Science 2004, 305, 986–989. [Google Scholar] [CrossRef] [PubMed]
- Greer, J.R.; Oliver, W.C.; Nix, W.D. Size dependence of mechanical properties of gold at the micron scale in the absence of strain gradients. Acta Mater. 2005, 53, 1821–1830. [Google Scholar] [CrossRef]
- Parthasarathy, T.A.; Rao, S.I.; Dimiduk, D.M.; Uchic, M.D.; Trinkle, D.R. Contribution to size effect of yield strength from the stochastics of dislocation source lengths in finite samples. Scr. Mater. 2007, 56, 313–316. [Google Scholar] [CrossRef]
- Greer, J.R.; De Hosson, J.T.M. Plasticity in small-sized metallic systems: Intrinsic versus extrinsic size effect. Prog. Mater. Sci. 2011, 56, 654–724. [Google Scholar] [CrossRef]
- Han, X.D.; Zhang, Y.F.; Zheng, K.; Zhang, X.N.; Zhang, Z.; Hao, Y.J.; Guo, X.Y.; Yuan, J.; Wang, Z.L. Low-temperature in situ large strain plasticity of ceramic SiC nanowires and its atomic-scale mechanism. Nano Lett. 2007, 7, 452–457. [Google Scholar] [CrossRef] [PubMed]
- Han, X.D.; Zheng, K.; Zhang, Y.F.; Zhang, X.N.; Zhang, Z.; Wang, Z.L. Low-temperature in situ large-strain plasticity of silicon nanowires. Adv. Mater. 2007, 19, 2112–2118. [Google Scholar] [CrossRef]
- Zhang, Y.F.; Han, X.D.; Zheng, K.; Zhang, Z.; Zhang, X.N.; Fu, J.Y.; Ji, Y.; Hao, Y.J.; Guo, X.Y.; Wang, Z.L. Direct observation of super-plasticity of beta-SiC nanowires at low temperature. Adv. Funct. Mater. 2007, 17, 3435–3440. [Google Scholar] [CrossRef]
- Zheng, K.; Han, X.D.; Wang, L.H.; Zhang, Y.F.; Yue, Y.H.; Qin, Y.; Zhang, X.N.; Zhang, Z. Atomic mechanisms governing the elastic limit and the incipient plasticity of bending Si nanowires. Nano Lett. 2009, 9, 2471–2476. [Google Scholar] [CrossRef] [PubMed]
- Zheng, K.; Wang, C.C.; Cheng, Y.Q.; Yue, Y.H.; Han, X.D.; Zhang, Z.; Shan, Z.W.; Mao, S.X.; Ye, M.M.; Yin, Y.D.; et al. Electron-beam-assisted superplastic shaping of nanoscale amorphous silica. Nat. Commun. 2010, 1, 24. [Google Scholar] [CrossRef] [PubMed]
- Yue, Y.H.; Liu, P.; Zhang, Z.; Han, X.D.; Ma, E. Approaching the theoretical elastic strain limit in copper nanowires. Nano Lett. 2011, 11, 3151–3155. [Google Scholar] [CrossRef] [PubMed]
- Li, H.X.; Mao, S.C.; Zang, K.T.; Liu, Y.; Guo, Z.X.; Wang, S.B.; Zhang, Y.F.; Yin, X.Q. An in situ TEM study of the size effect on the thermally induced martensitic transformation in nanoscale NiTi shape memory alloy. J. Alloy. Compd. 2014, 588, 337–342. [Google Scholar] [CrossRef]
- Mao, S.C.; Li, H.X.; Liu, Y.; Deng, Q.S.; Wang, L.H.; Zhang, Y.F.; Zhang, Z.; Han, X.D. Stress-induced martensitic transformation in nanometric NiTi shape memory alloy strips: An in situ TEM study of the thickness/size effect. J. Alloy. Compd. 2013, 579, 100–111. [Google Scholar] [CrossRef]
- Han, X.D.; Wang, L.H.; Yue, Y.H.; Zhang, Z. In situ atomic scale mechanical microscopy discovering the atomistic mechanisms of plasticity in nano-single crystals and grain rotation in polycrystalline metals. Ultramicroscopy 2015, 151, 94–100. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Teng, J.; Liu, P.; Hirata, A.; Ma, E.; Zhang, Z.; Chen, M.; Han, X. Grain rotation mediated by grain boundary dislocations in nanocrystalline platinum. Nat. Commun. 2014, 5, 4402. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.H.; Zheng, K.; Zhang, Z.; Han, X.D. Direct Atomic-scale imaging about the mechanisms of ultralarge bent straining in Si nanowires. Nano Lett. 2011, 11, 2382–2385. [Google Scholar] [CrossRef] [PubMed]
- Yue, Y.H.; Liu, P.; Deng, Q.S.; Ma, E.; Zhang, Z.; Han, X.D. Quantitative evidence of crossover toward partial dislocation mediated plasticity in copper single crystalline nanowires. Nano Lett. 2012, 12, 4045–4049. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.H.; Zhang, Z.; Han, X.D. In situ experimental mechanics of nanomaterials at the atomic scale. NPG Asia Mater. 2013, 5, e40. [Google Scholar] [CrossRef]
- Wang, L.H.; Han, X.D.; Liu, P.; Yue, Y.H.; Zhang, Z.; Ma, E. In situ observation of dislocation behavior in nanometer grains. Phys. Rev. Lett. 2010, 105, 478–481. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Q.K.; Liu, P.; Ma, Y.; Cao, Q.P.; Wang, X.D.; Zhang, D.X.; Han, X.D.; Zhang, Z.; Jiang, J.Z. Super elastic strain limit in metallic glass films. Sci. Rep. UK 2012, 2, 852. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Wang, X.D.; Mao, S.C.; Wu, H.; Guo, X.; Ji, Y.; Han, X.D. Strongly enhanced ultraviolet emission of an Au@SiO2/ZnO plasmonic hybrid nanostructure. Nanoscale 2016, 8, 4030–4036. [Google Scholar] [CrossRef] [PubMed]
- Kong, D.L.; Sun, S.D.; Xin, T.J.; Xiao, L.R.; Sha, X.C.; Lu, Y.; Mao, S.C.; Zou, J.; Wang, L.H.; Han, X.D. Reveal the size effect on the plasticity of ultra-small sized Ag nanowires with in situ atomic-scale microscopy. J. Alloy. Compd. 2016, 676, 377–382. [Google Scholar] [CrossRef]
- Zang, K.T.; Mao, S.C.; Cai, J.X.; Liu, Y.N.; Li, H.X.; Hao, S.J.; Jiang, D.Q.; Cui, L.S. Revealing ultralarge and localized elastic lattice strains in Nb nanowires embedded in NiTi matrix. Sci. Rep. UK 2015, 5, 17530. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Ke, C.; Espinosa, H.D. Experimental techniques for the mechanical characterization of one-dimensional nanostructures. Exp. Mech. 2007, 47, 7–24. [Google Scholar] [CrossRef]
- Wang, B.; Tomar, V.; Haque, A. In-situ TEM mechanical testing of nanocrystalline zirconium thin films. Mater. Lett. 2015, 152, 105–108. [Google Scholar] [CrossRef]
- Huang, L.; Li, Q.-J.; Shan, Z.-W.; Li, J.; Sun, J.; Ma, E. A new regime for mechanical annealing and strong sample-size strengthening in body centred cubic molybdenum. Nat. Commun. 2011, 2, 547. [Google Scholar] [CrossRef] [PubMed]
- Edwards, R.L.; Coles, G.; Sharpe, W.N. Comparison of tensile and bulge tests for thin-film silicon nitride. Exp. Mech. 2004, 44, 49–54. [Google Scholar] [CrossRef]
- Majjad, H.; Basrour, S.; Delobelle, P.; Schmidt, M. Dynamic determination of Young’s modulus of electroplated nickel used in LIGA technique. Sens. Actuators A Phys. 1999, 74, 148–151. [Google Scholar] [CrossRef]
- Dzung Viet, D.; Koichi, N.; Tung Thanh, B.; Susumu, S. Micro/nano-mechanical sensors and actuators based on SOI-MEMS technology. Adv. Nat. Sci. Nanosci. Nanotechnol. 2010, 1, 013001. [Google Scholar]
- Rui, L.; Hong, W.; Xueping, L.; Guifu, D.; Chunsheng, Y. A micro-tensile method for measuring mechanical properties of MEMS materials. J. Micromech. Microeng. 2008, 18, 065002. [Google Scholar]
- Warren, O.L.; Shan, Z.; Asif, S.A.S.; Stach, E.A.; Morris, J.W., Jr.; Minor, A.M. In situ nanoindentation in the TEM. Mater. Today 2007, 10, 59–60. [Google Scholar] [CrossRef]
- Bobji, M.S.; Ramanujan, C.S.; Pethica, J.B.; Inkson, B.J. A miniaturized TEM nanoindenter for studying material deformation in situ. Meas. Sci. Technol. 2006, 17, 1324. [Google Scholar] [CrossRef]
- Pharr, G.M. Measurement of mechanical properties by ultra-low load indentation. Mater. Sci. Eng. A 1998, 253, 151–159. [Google Scholar] [CrossRef]
- Wei, Y.; Hutchinson, J.W. Hardness trends in micron scale indentation. J. Mech. Phys. Solids 2003, 51, 2037–2056. [Google Scholar] [CrossRef]
- Bucaille, J.L.; Stauss, S.; Felder, E.; Michler, J. Determination of plastic properties of metals by instrumented indentation using different sharp indenters. Acta Mater. 2003, 51, 1663–1678. [Google Scholar] [CrossRef]
- Alkorta, J.; Martínez-Esnaola, J.M.; Gil Sevillano, J. Detailed assessment of indentation size-effect in recrystallized and highly deformed niobium. Acta Mater. 2006, 54, 3445–3452. [Google Scholar] [CrossRef]
- Arsenlis, A.; Parks, D.M. Crystallographic aspects of geometrically-necessary and statistically-stored dislocation density. Acta Mater. 1999, 47, 1597–1611. [Google Scholar] [CrossRef]
- Busso, E.P.; Meissonnier, F.T.; O’Dowd, N.P. Gradient-dependent deformation of two-phase single crystals. J. Mech. Phys. Solids 2000, 48, 2333–2361. [Google Scholar] [CrossRef]
- Pantano, M.F.; Espinosa, H.D.; Pagnotta, L. Mechanical characterization of materials at small length scales. J. Mech. Sci. Technol. 2012, 26, 545–561. [Google Scholar] [CrossRef]
- Kumar, S.; Alam, T.; Haque, A. Quantitative in-situ TEM study of stress-assisted grain growth. MRS Commun. 2013, 3, 101–105. [Google Scholar] [CrossRef]
- Haque, M.A.; Espinosa, H.D.; Lee, H.J. MEMS for in situ testing—Handling, actuation, loading, and displacement measurements. MRS Bull. 2010, 35, 375–381. [Google Scholar] [CrossRef]
- Han, J.H.; Saif, M.T.A. In situ microtensile stage for electromechanical characterization of nanoscale freestanding films. Rev. Sci. Instrum. 2006, 77, 045102. [Google Scholar] [CrossRef]
- Jin, Q.H.; Li, T.; Wang, Y.L.; Li, X.X.; Zhou, P.; Xu, F.F. In-situ TEM tensile test of 90nm-thick SCS beam using MEMS chip. Sens. IEEE 2008. [Google Scholar] [CrossRef]
- Yu, S.; Bradley, J.N. MEMS capacitive force sensors for cellular and flight biomechanics. Biomed. Mater. 2007, 2, S16. [Google Scholar]
- Dongfeng, Z.; Breguet, J.M.; Clavel, R.; Sivakov, V.; Christiansen, S.; Michler, J. In situ electron microscopy mechanical testing of silicon nanowires using electrostatically actuated tensile stages. J. Microelectromech. Syst. 2010, 19, 663–674. [Google Scholar] [CrossRef]
- Barlian, A.A.; Woo-Tae, P.; Mallon, J.R.; Rastegar, A.J.; Pruitt, B.L. Review: Semiconductor piezoresistance for microsystems. Proc. IEEE 2009, 97, 513–552. [Google Scholar] [CrossRef] [PubMed]
- Tortonese, M.; Barrett, R.C.; Quate, C.F. Atomic resolution with an atomic force microscope using piezoresistive detection. Appl. Phys. Lett. 1993, 62, 834–836. [Google Scholar] [CrossRef]
- Duc, T.C.; Creemer, J.F.; Sarro, P.M. Lateral nano-Newton force-sensing piezoresistive cantilever for microparticle handling. J. Micromech. Microeng. 2006, 16, S102. [Google Scholar] [CrossRef]
- Chui, B.W.; Aeschimann, L.; Akiyama, T.; Staufer, U.; de Rooij, N.F.; Lee, J.; Goericke, F.; King, W.P.; Vettiger, P. Advanced temperature compensation for piezoresistive sensors based on crystallographic orientation. Rev. Sci. Instrum. 2007, 78, 043706. [Google Scholar] [CrossRef] [PubMed]
- Smith, C.S. Piezoresistance effect in germanium and silicon. Phys. Rev. 1954, 94, 42–49. [Google Scholar] [CrossRef]
- Kanda, Y. Piezoresistance effect of silicon. Sens. Actuators A Phys. 1991, 28, 83–91. [Google Scholar] [CrossRef]
- Taechung, Y.; Chang-Jin, K. Measurement of mechanical properties for MEMS materials. Meas. Sci. Technol. 1999, 10, 706. [Google Scholar]
- Harkey, J.A.; Kenny, T.W. 1/f noise considerations for the design and process optimization of piezoresistive cantilevers. J. Microelectromech. Syst. 2000, 9, 226–235. [Google Scholar] [CrossRef]
- Nash, W.A. Schaum’s Outline of Theory and Problems of Strength of Materials, 4th ed.; McGraw-Hill: New York, NY, USA, 1998. [Google Scholar]
- Nakladal, A.; Sager, K.; Gerlach, G. Influences of humidity and moisture on the long-term stability of piezoresistive pressure sensors. Measurement 1995, 16, 21–29. [Google Scholar] [CrossRef]
- Hoa, P.L.P.; Suchaneck, G.; Gerlach, G. Influence of polycrystalline silicon as electrical shield on reliability and stability of piezoresistive sensors. Sens. Actuators A Phys. 2005, 120, 567–572. [Google Scholar] [CrossRef]
- Park, S.J.; Doll, J.C.; Pruitt, B.L. Piezoresistive cantilever performance-part I: Analytical model for sensitivity. J. Microelectromech. Syst. 2010, 19, 137–148. [Google Scholar] [CrossRef] [PubMed]
- Rob, L.; Groeneveld, A.W.; Elwenspoek, M. Comb-drive actuators for large displacements. J. Micromech. Microeng. 1996, 6, 320. [Google Scholar]
- Haque, M.A.; Saif, M.T.A. Application of MEMS force sensors for in situ mechanical characterization of nano-scale thin films in SEM and TEM. Sens. Actuators A Phys. 2002, 97–98, 239–245. [Google Scholar] [CrossRef]




| Parameters | Value | Unit | |
|---|---|---|---|
| Sensor A | Sensor B | ||
| Α | 10−5 | - | |
| T | 300 | K | |
| μp | 0.934 × 10−2 | cm2·V−1·s−1 | |
| Bias voltage | 3.0 | V | |
| fmax | 1000 | Hz | |
| fmin | 10 | Hz | |
| Clamped beam length | 150 | 600 | Μm |
| Clamped beam width | 60 | 60 | Μm |
| Clamped beam thickness | 30 | 15 | Μm |
| Piezoresistor length | 46 | 107 | Μm |
| Piezoresistor width | 8 | 3 | Μm |
| Piezoresistor thickness | 1.1 | 1.1 | Μm |
| Theoretical displacement sensitivity | 77.1 | 7.1 | μV/nm |
| Theoretical displacement resolution | 0.19 | 4.6 | nm |
| Theoretical displacement range limit | 5.47 | 175.14 | μm |
| Theoretical load range limit | 888 | 55.0 | mN |
| Length (nm) | Width (nm) | Depth (nm) |
|---|---|---|
| 4880 | 620 | 510 |
| Sensors | Magnification | ||
|---|---|---|---|
| 2000× | 8000× | 16,000× | |
| Sensor A | 37.5 μV/nm | 37.2 μV/nm | 37.4 μV/nm |
| Sensor B | 4.6 μV/nm | 4.9 μV/nm | 4.8 μV/nm |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Mao, S.; Zhang, J.; Li, Z.; Deng, Q.; Ning, J.; Yang, X.; Wang, L.; Ji, Y.; Li, X.; et al. MEMS Device for Quantitative In Situ Mechanical Testing in Electron Microscope. Micromachines 2017, 8, 31. https://doi.org/10.3390/mi8020031
Wang X, Mao S, Zhang J, Li Z, Deng Q, Ning J, Yang X, Wang L, Ji Y, Li X, et al. MEMS Device for Quantitative In Situ Mechanical Testing in Electron Microscope. Micromachines. 2017; 8(2):31. https://doi.org/10.3390/mi8020031
Chicago/Turabian StyleWang, Xiaodong, Shengcheng Mao, Jianfei Zhang, Zhipeng Li, Qingsong Deng, Jin Ning, Xudong Yang, Li Wang, Yuan Ji, Xiaochen Li, and et al. 2017. "MEMS Device for Quantitative In Situ Mechanical Testing in Electron Microscope" Micromachines 8, no. 2: 31. https://doi.org/10.3390/mi8020031
APA StyleWang, X., Mao, S., Zhang, J., Li, Z., Deng, Q., Ning, J., Yang, X., Wang, L., Ji, Y., Li, X., Liu, Y., Zhang, Z., & Han, X. (2017). MEMS Device for Quantitative In Situ Mechanical Testing in Electron Microscope. Micromachines, 8(2), 31. https://doi.org/10.3390/mi8020031





