1. Introduction
The increasing interest in real-time measurement methods has heightened the need for structural health monitoring (SHM) systems in the modern engineering field [
1,
2]. SHM is considered an interdisciplinary procedure, including structural sensing systems, immediate data collection and processing, and real-time information feedback to control systems about the global or local structural state. The main purpose of SHM is to detect unusual structural behaviors to pinpoint failures or unhealthy structural conditions [
3], thereby increasing the safety of humans and the environment while also reducing maintenance costs. However, the real-time measurement of structural three-dimensional displacement fields, which obtains the stress and strain within a given structure, is an instrumental technology for the SHM system of engineering structures.
Existing measurement methodologies from the past decade can largely be grouped as either noncontact means based on the principle of optical imaging or contact-forming, based on strain sensors bonded to or embedded in the structural surface [
4]. Compared to contact measurement, noncontact methods are less accurate for the application of large equipment due to interference from the external environment, such as the circumstances of measurement and the surrounding environment. On the contrary, the contact method, employing different types of sensors [
5,
6,
7], such as strain gauge sensors, acceleration sensors, and fiber Bragg grating (FBG) sensors, can obtain the physical information on objective structures and reconstruct the full-field deformation field using the differential and integral relationships and the physical information on objective structures. Moreover, benefiting from the significant advantages of FBG, such as being lightweight and small in size, corrosion-resistant, and resistant to electromagnetic interference [
8,
9], much research has been devoted to the analytical evaluation and experimental testing of FBG sensors. Additionally, a great deal of previous research based on FBG sensors focuses on displacement measurement; Chen and Hu et al. [
10] conducted large-range curvature measurements based on FBGs in two-core fiber with a protective coating, which proved to be a good candidate for curvature sensing in the engineering field. Xu et al. [
11] presented an online measurement and calibration method for estimating the deformation of the sub-reflector support structure based on FBG sensors, which eliminates the effects of temperature variations on strain measurements using a temperature-compensating device. Rakotondrabe et al. [
12] employed embedded FBG sensors to detect and monitor damage to laminated composite structures, thereby developing a more cost-effective aircraft maintenance approach.
Furthermore, the measurement of structural three-dimensional displacement based on FBG sensors, known as shape sensing, has emerged as a powerful platform for SHM systems. However, shape sensing is related to an inverse problem from a mathematical perspective [
13], so the solving process results in inevitable problems, such as the uniqueness of given cases and instability arising from small disturbances. Furthermore, most of the inverse methods do not sufficiently consider the boundary conditions or structural topology, and these methods are limited to prior knowledge of precise structural loading or material, leading to a lack of potential for real-time SHM applications.
In Gherlone’s analysis of the shape sensing of structural deformation [
14], Ko’s displacement method (Ko) [
15], Modal Transformation Theory (MTT) [
16], and the inverse finite element method (iFEM), [
17] are currently the most popular methods for the real-time reconstruction of three-dimensional displacement by installing FBG sensors. In recent years, an increasing amount of literature has been published on iFEM [
18]. This methodology can provide robust, stable, and accurate displacement results and is sufficiently fast for real-time monitoring applications, meeting the requirements of an SHM system for developing the next generation of aerospace vehicles. Comparatively, the iFEM is the innovative and seminal work of Tessler and Spangler et al. [
17], who present the relationship between displacement/rotation degree of freedom and experimentally measured strain data in the inverse element, and the whole stiffness matrix can be sequentially assembled by the single inverse element matrix. Additionally, the geometrically complex structure is topologically divided into conventional plate, shell, and solid inverse elements.
The three-node inverse shell element (iMIN3) based on lowest-order anisoparametric C0 continuous and first-order shear deformation theory (FSDT) was thoroughly presented by Tessler et al. [
17]. Afterward, the robust inverse-frame element was proposed by Gherlone et al. based on the assumptions of Timoshenko beam theory [
18]. This method is tailored toward one-dimensional structures [
19], such as trusses, beams, and frames subjected to stretching, bending, transverse-shear, and torsion-deformation. In the work of Roy et al. [
20], the iFEM-beams analysis of the generic cross-section airfoil structure was significantly improved, and the reconstruction of wing test article structural deformations and loads was computationally conducted. Following the research of You and Ren et al. on inverse beam elements [
21], iEBT2 was developed for the shape-sensing analysis of civil infrastructures, and the novel inclinometer using the iFEM theoretical framework was proposed for the deformation estimation of soil structures [
22]. A remarkable effort has been made by Bao and co-workers to develop the enhanced inverse beam element. Zhao [
23] coupled the isogeometric analysis approach to eliminate the multiple singularities problem due to discontinuities for beam structures, and Chen [
24] presented a unified method for the reconstruction of Euler–Bernoulli and Timoshenko beams. To expand the practical usefulness of iFEM large-scale structures, Kefal proposed the new quadrilateral inverse-shell element (iQS4) [
25], the eight-node curved inverse-shell element (iCS8) [
26], and non-uniform rational B-spline (NURBS) technology [
27]. Additionally, considerable research based on the iFEM family has been devoted to the possible applications of SHM for ships and marine platforms [
26,
28]. In addition, Niu presented the iMITC3+ based on the mixed interpolation of tensorial components technology for the reconstruction of thin plate structures [
29], and Shang presents the iQAC using the quadrilateral area coordinates shape function to effectively deal with the element distortion [
30]. Ji [
31] proposed the deformation perception system by coupling the intelligent flexible sensing film (iFlexSense) and iFEM for the displacement reconstruction of a cantilever morphing aircraft compound.
Over the past decade, SHM systems have focused on the composite structure engineering field; however, the existing iFEM algorithms using FSDT cannot inadequately predict structures due to a high degree of anisotropy and heterogeneity. To solve this problem, Cerracchio [
32] originally proposed the three-node iFEM/RZT for the reconstruction of composite sandwich plate structures based on the kinematic assumptions of the refined zigzag theory (RZT). Subsequently, Kefal [
33] enhanced the reconstructed formulation by accurately redefining the first and second-order transverse shear strains, which conducted significantly accurate displacement predictions for thick multilayered composite and sandwich plate structures. Additionally, Sorrenti [
34] developed the robust four-node quadrilateral iFEM/RZT free with shear locking for ultra-thin laminated composite plates, and the present method has been experimentally applied for the reconstruction of moderately thick wing-shaped sandwich structures by strain sensors [
35].
Collectively, these studies outline the critical role iFEM plays in the displacement reconstruction of various types of plate structures; however, a small number of studies have focused on the transverse shear effect for the three-dimensional deformation field on thick plate structures. In addition, the main limitation of the existing iFEM, which is not available for thick plate structures, is that the shear displacement formulation for thick plate is not explicitly expressed, and the RZT requires the measurement strain information on the interface using embedded strain sensors. To address the above questions, research [
36] has presented the enhanced iFEM, employing the scaled boundary finite element theory for reconstructing the thin plate structure using single strain information. The novel three-variable shear deformation plate formulation can effectively capture a shear deformable effect for thick plates [
37].
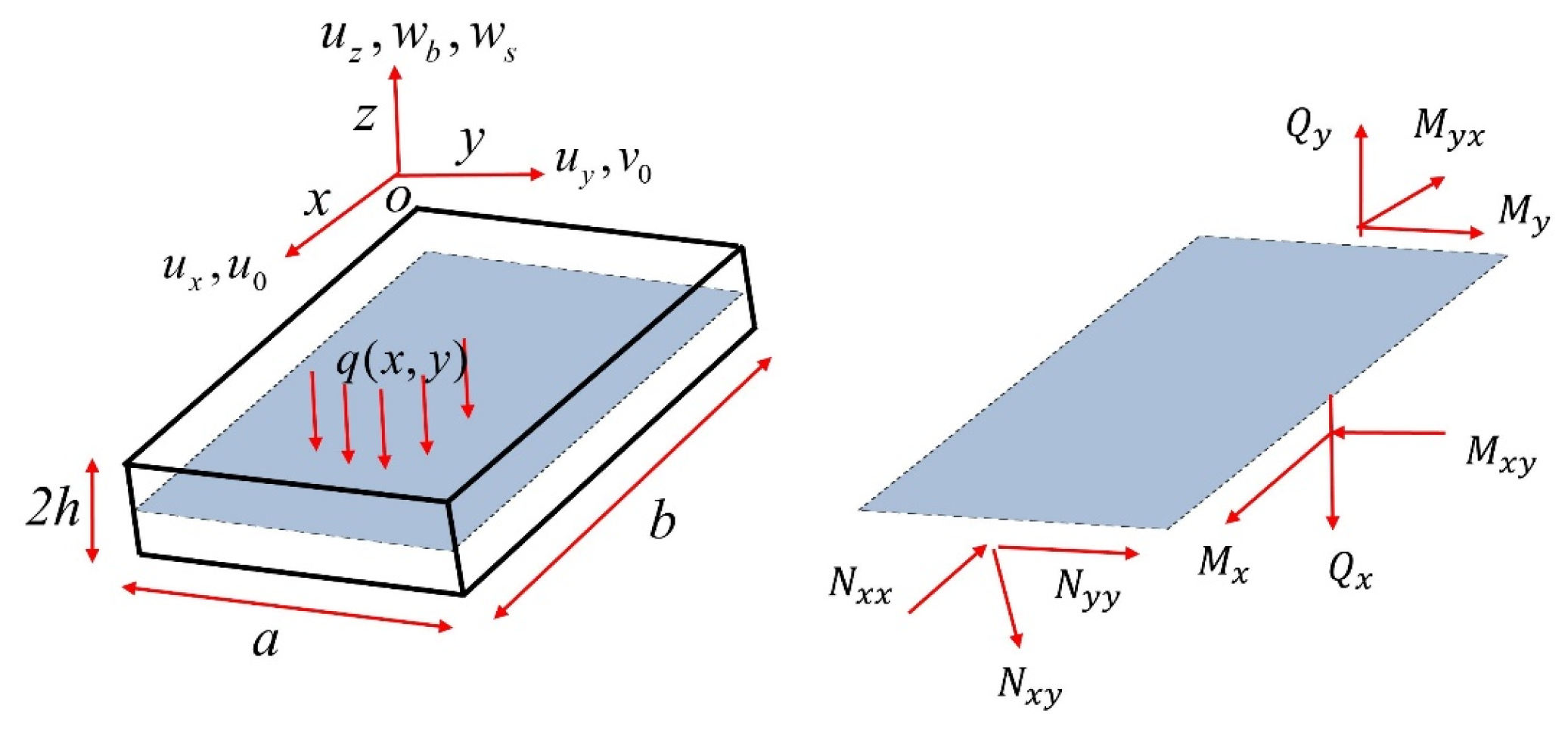
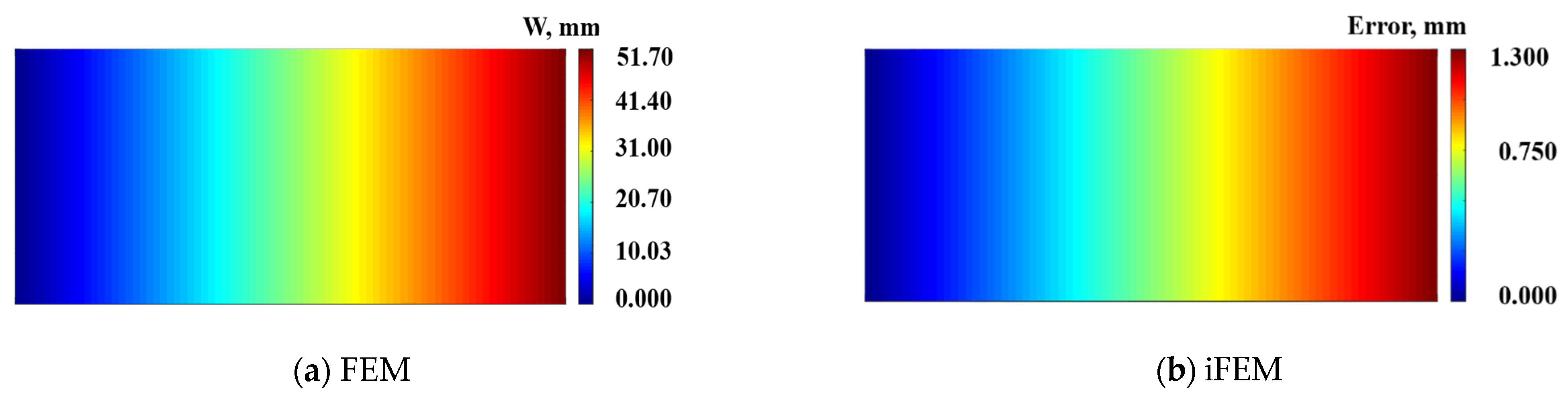
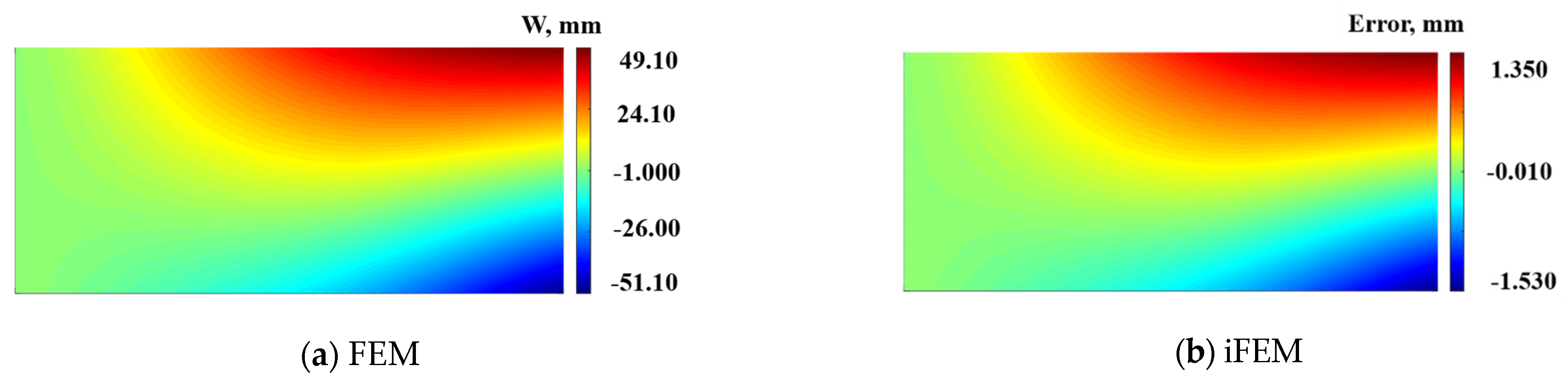
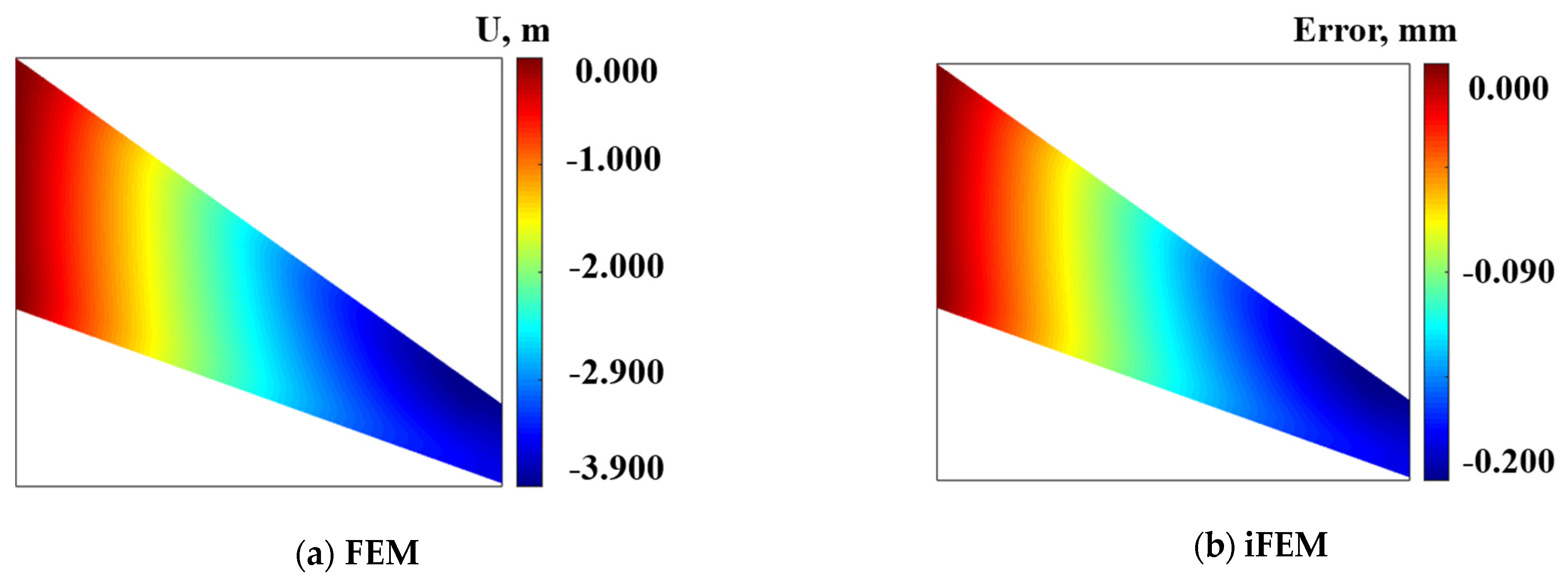
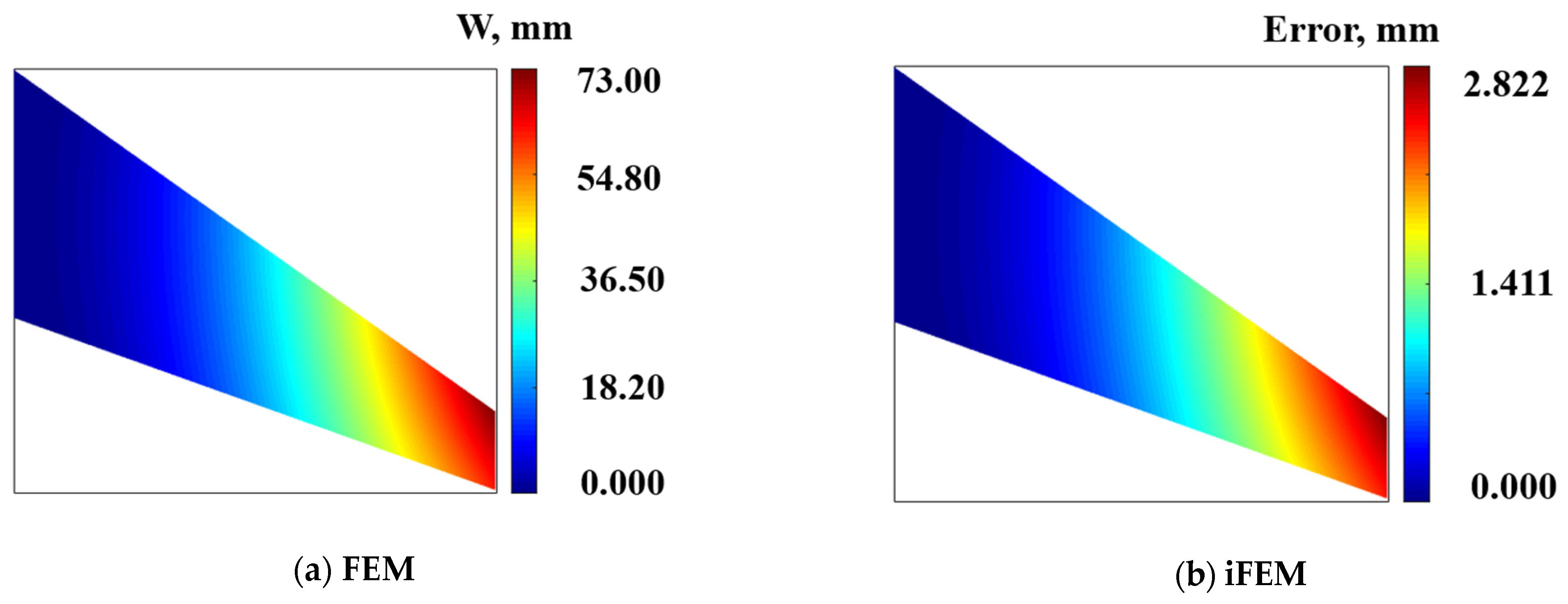
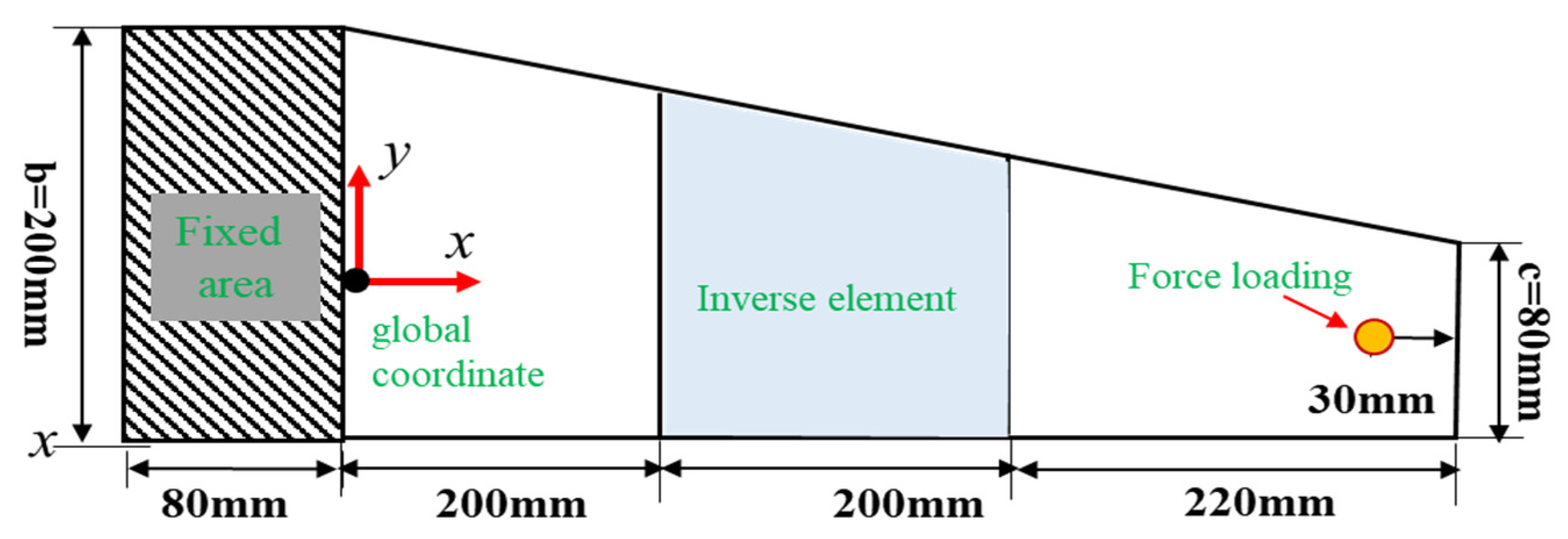
To expand the library of existing iFEM for the SHM of practical engineering structures, this study aimed to develop a refined measurement method coupling the iFEM theoretical framework and analytical form for the shape analysis of thick plates. Furthermore, this work presents the reconstructed algorithm for the reconstruction of boundary surface displacement by employing strain information, and the methodology thoroughly shows the solution technology of the sixth-order differential equation to develop the deflection function.
This paper is organized as follows:
Section 2 presents the theoretical framework of the refined iFEM formulation.
Section 3 presents the different analysis models for thick plates and estimates the results from numerical and experimental analyses. Finally, the paper concludes with
Section 4, which highlights the advantages of the present method.