Investigation on the Bending Mechanism of Single-Crystal Copper Under High Bending Rates via Molecular Dynamics
Abstract
1. Introduction
- (I)
- The material’s plastic deformation and microstructural evolution during the bending process of the flexible hinges;
- (II)
- The effect of the bending rate on the deformation behavior of the flexible hinges.
2. Materials and Methods
3. Results
3.1. Plastic Deformation Process
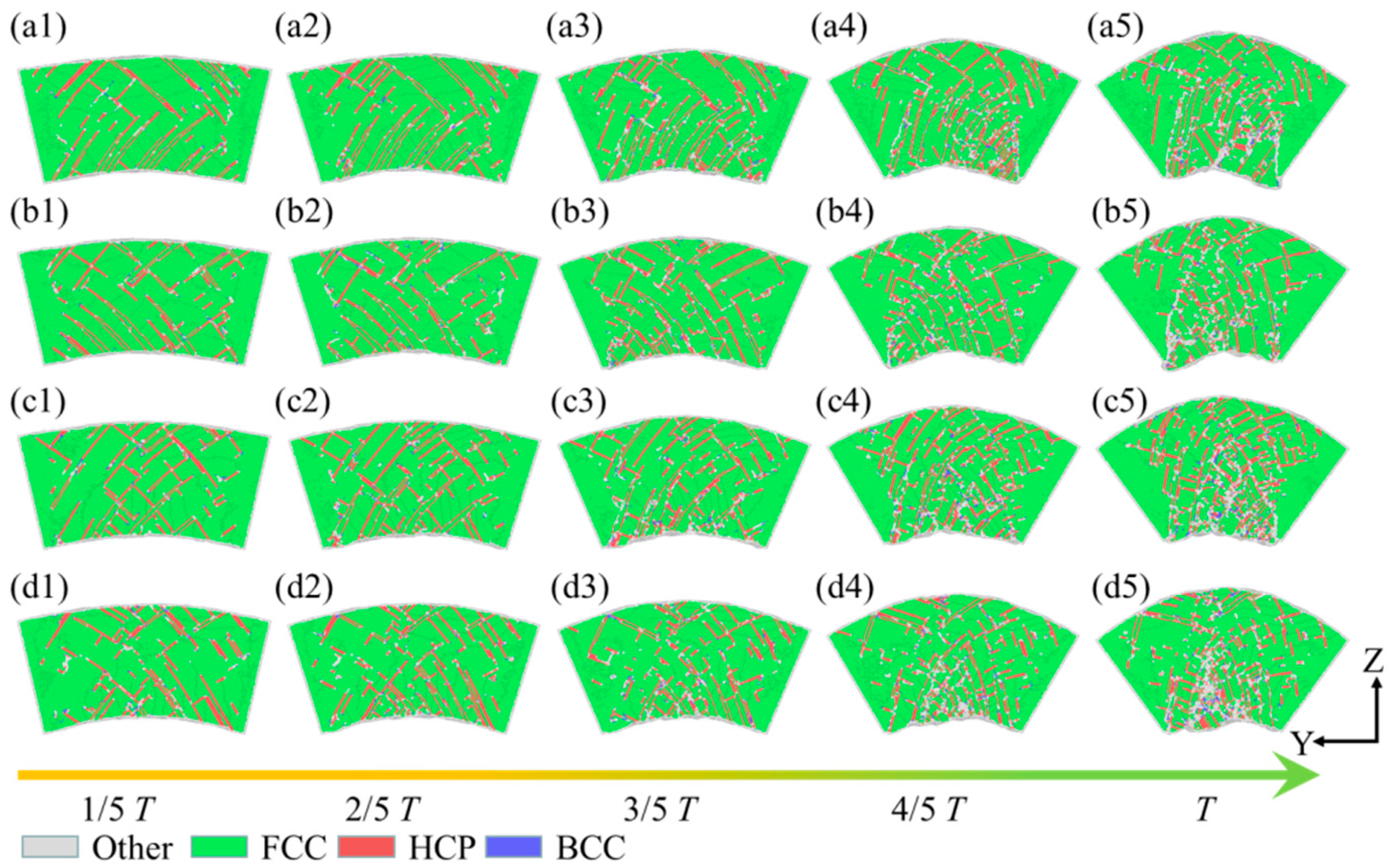
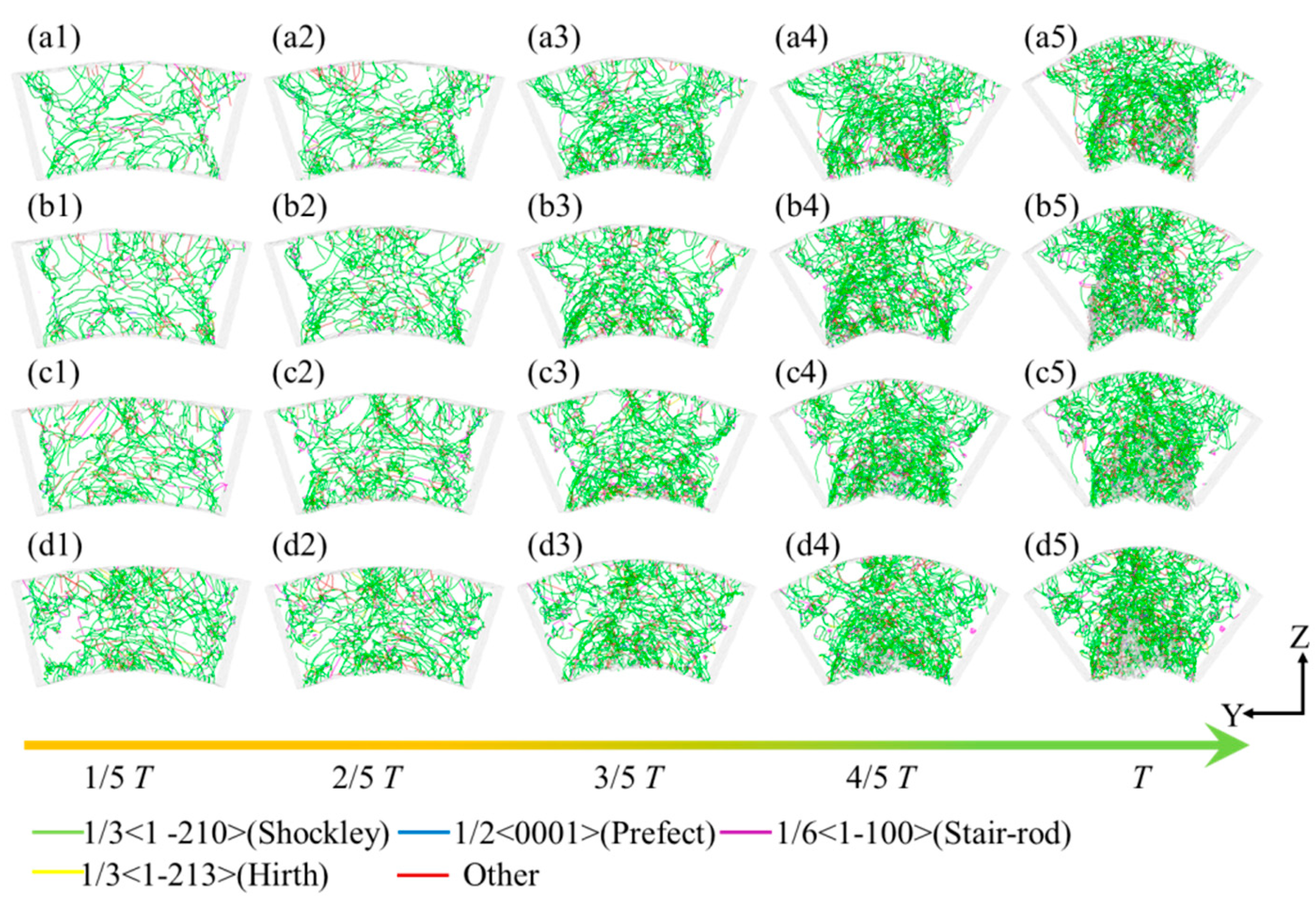
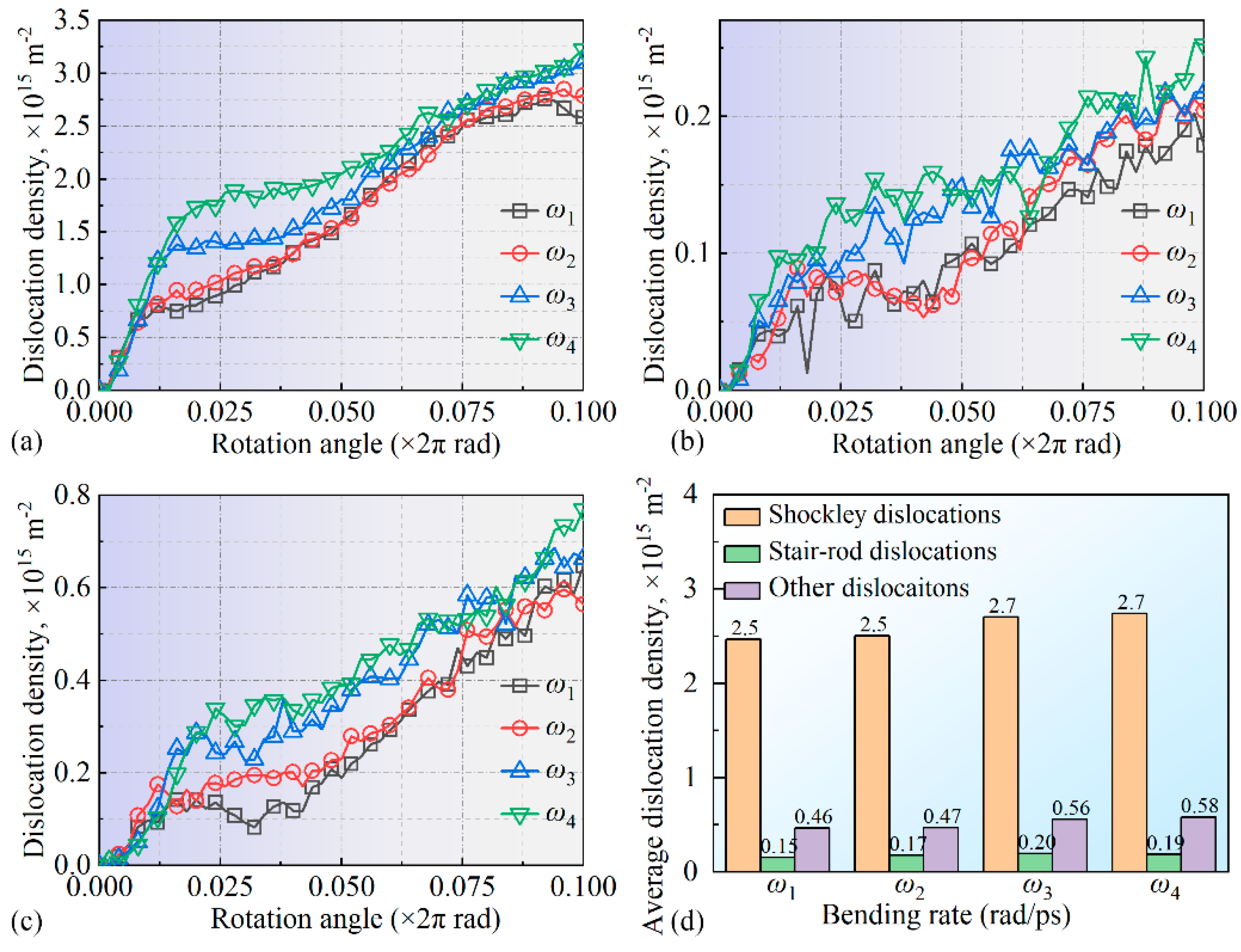
3.2. Microstructure Evolution
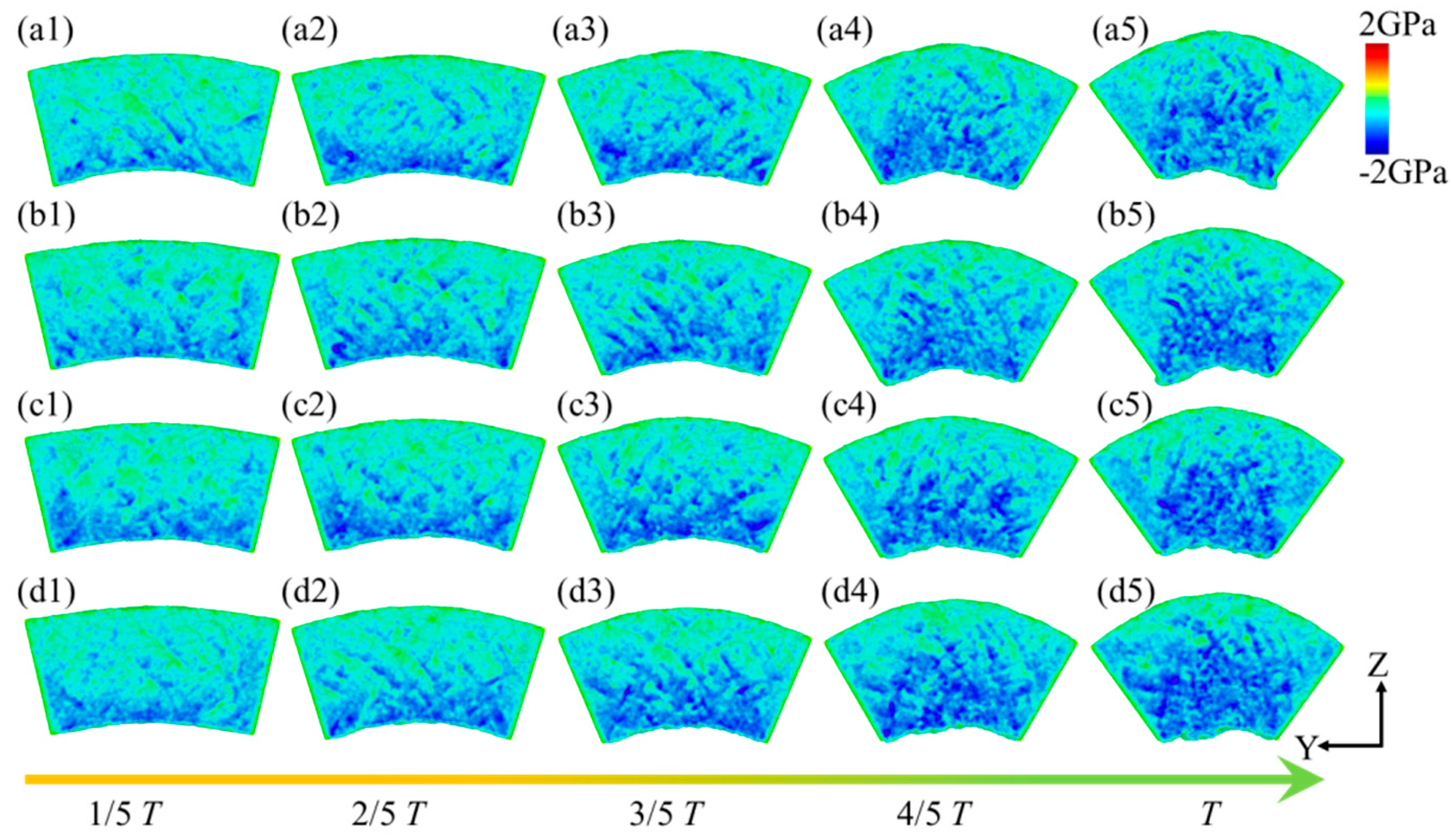
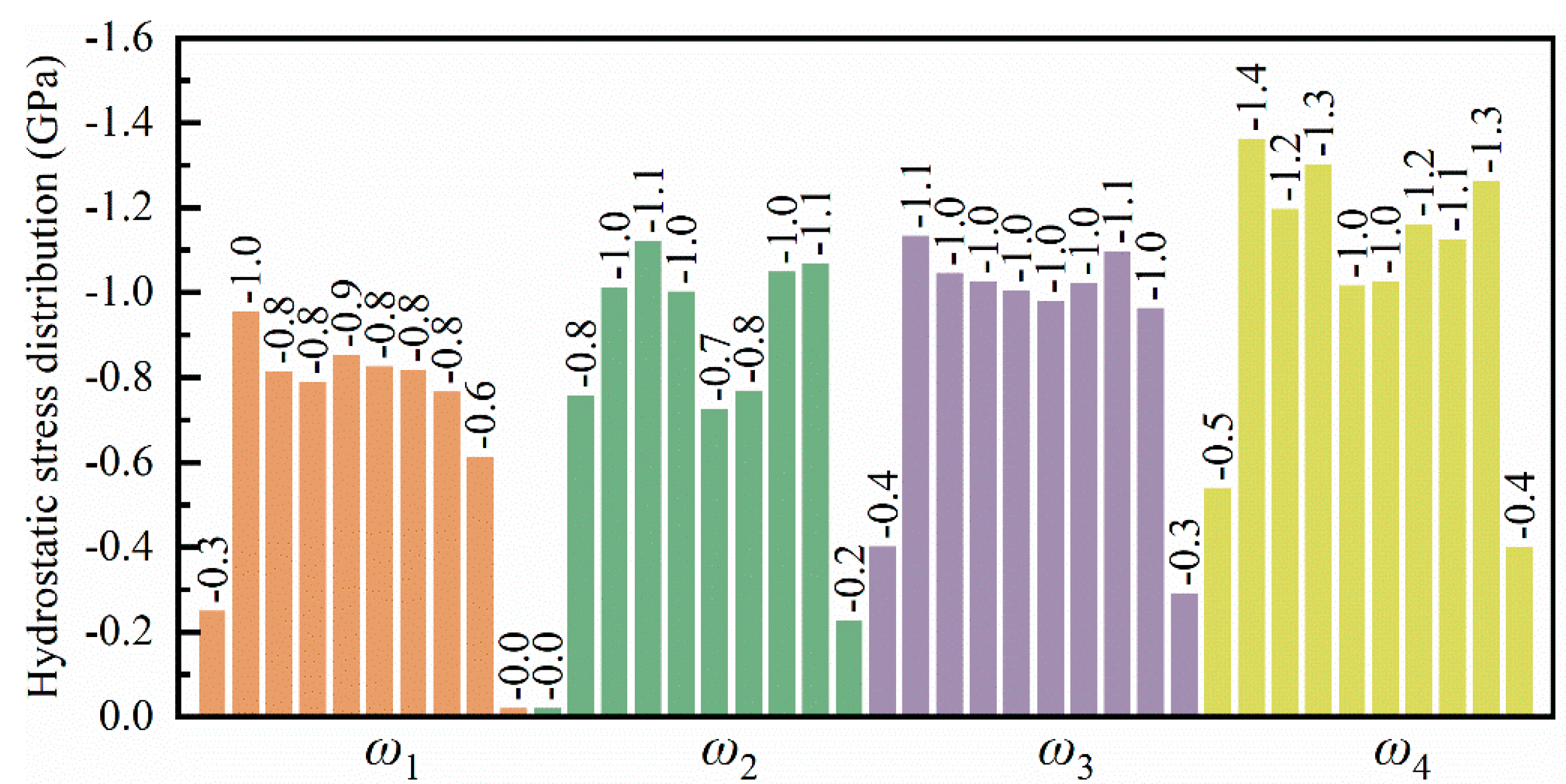
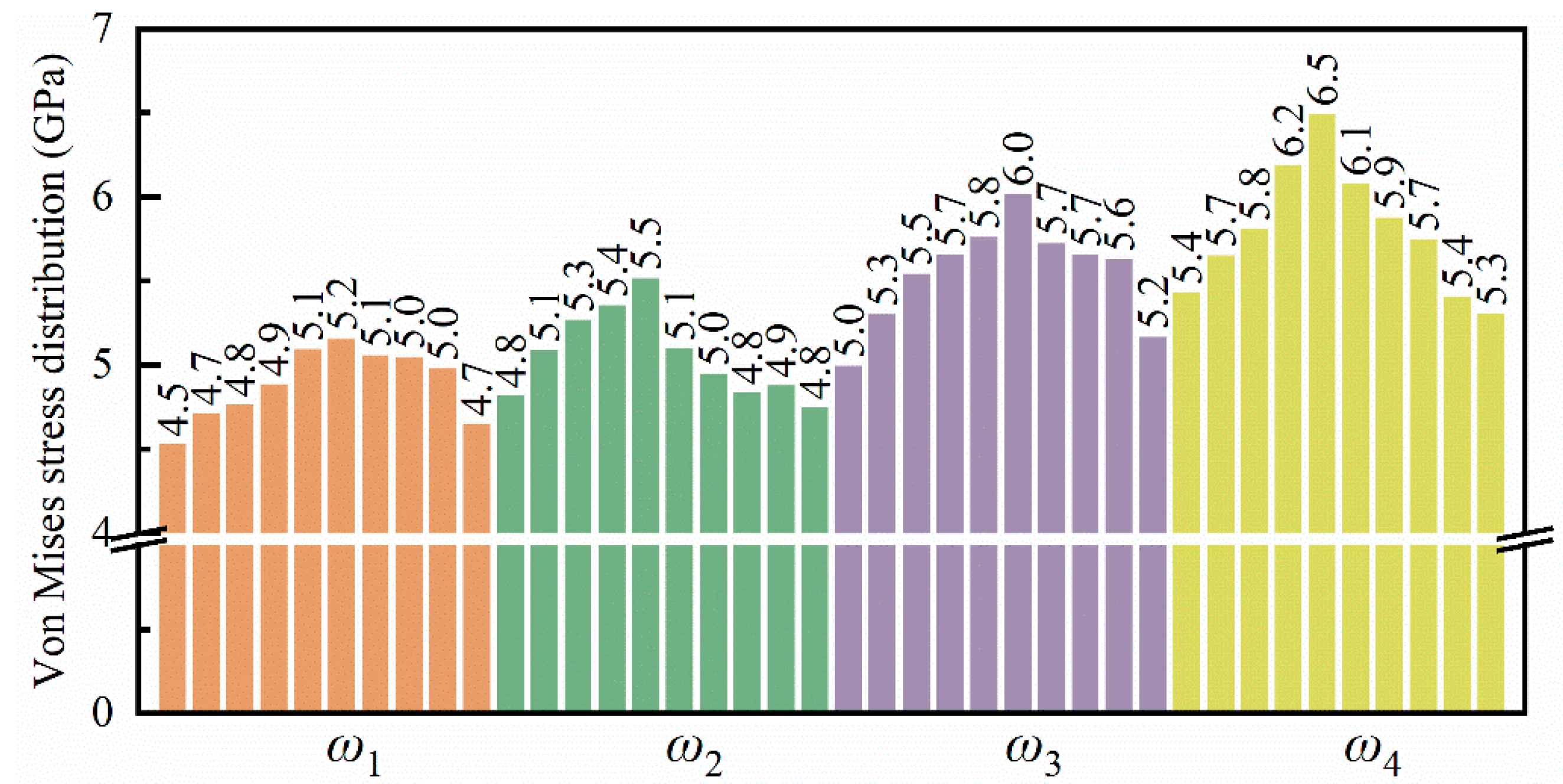
3.3. Internal Stress
3.4. Temperature
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Xu, J.; Zhang, Z.; Li, Z.; Bai, Y.; Wang, G.; Li, S.; Zeng, T.; Li, C.; Lu, Y.; Han, B.; et al. A determination of the Planck constant by the generalized joule balance method with a permanent-magnet system at NIM. Metrologia 2016, 53, 86. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Z.; Lu, Y.; Hu, P.; Liu, Y.; Xu, J.; Bai, Y.; Zeng, T.; Wang, G.; You, Q.; et al. The first determination of the Planck constant with the joule balance NIM-2. Metrologia 2017, 54, 763. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Z.; Lu, Y.; Hu, P.; Liu, Y.; Xu, J. The design and construction of the joule balance NIM-2. IEEE Trans. Instrum. Meas. 2017, 66, 1329–1336. [Google Scholar] [CrossRef]
- Hu, W.; Li, W.; Liu, Y.; Wang, Y.; Yang, X. Quasi-static analysis of a compliant tripod stage with plane compliant lever mechanism. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2017, 231, 1639–1650. [Google Scholar]
- Lobontiu, N.; Paine, J.S.N.; Garcia, E.; Goldfarb, M. Design of symmetric conic-section flexure hinges based on closed-form compliance equations. Mech. Mach. Theory 2002, 37, 477–498. [Google Scholar] [CrossRef]
- Gomez, J.F.; Booker, J.D.; Mellor, P.H. 2D shape optimization of leaf-type crossed flexure pivot springs for minimum stress. Precis. Eng. 2015, 42, 6–21. [Google Scholar] [CrossRef]
- Qiu, L.; Dai, S.; Li, Y.; Li, C. Design and analysis of a contact-aided leaf hinge (CALH) with continuous variable stiffness. Mech. Mach. Theory 2022, 169, 104653. [Google Scholar] [CrossRef]
- Oberst, S.; Tuttle, S.L.; Griffin, D.; Lambert, A.; Boyce, R.R. Experimental validation of tape springs to be used as thin-walled space structures. J. Sound Vib. 2018, 419, 558–570. [Google Scholar] [CrossRef]
- Thaker, M.; Joshi, S.J.; Arora, H.; Shah, D.B. Tape Spring for Deployable Space Structures: A Review. Adv. Space Res. 2024, 73, 5188–5219. [Google Scholar] [CrossRef]
- Chen, Z.; Chen, G.; Zhang, X. Leaf Flexure Hinge with Damping Layers: Theoretical Model and Experiments. In Proceedings of the 2014 International Conference on Manipulation, Manufacturing and Measurement on the Nanoscale (3M-NANO), Taipei, Taiwan, 27–31 October 2014. [Google Scholar]
- Meijaard, J.P.; Brouwer, D.M.; Jonker, J.B. Analytical and experimental investigation of a parallel leaf spring guidance. Multibody Syst. Dyn. 2010, 23, 77–97. [Google Scholar] [CrossRef]
- Guduru, R.K.; Shaik, S.H.; Tuniki, H.P.; Domeika, A. Development of mono leaf spring with composite material and investigating its mechanical properties. Mater. Today Proc. 2021, 45, 556–561. [Google Scholar] [CrossRef]
- Saelem, S.; Chantranuwathana, S.; Panichanun, K.; Prempreeda, P.; Wichienprakarn, P.; Kruo-ongarjnukool, P. Experimental Verification of Leaf Spring Model by Using a Leaf Spring Test Rig. In Proceedings of the 23rd Conference of the Mechanical Engineering Network of Thailand, Chiang Mai, Thailand, 4–7 November 2009. [Google Scholar]
- Ghuku, S.; Saha, K.N. Design development and performance analysis of leaf spring testing set up in elastic domain. J. Assoc. Eng. 2016, 86, 23. [Google Scholar] [CrossRef]
- Kang, D.; Gweon, D. Analysis and design of a cartwheel-type flexure hinge. Precis. Eng. 2013, 37, 33–43. [Google Scholar] [CrossRef]
- Borysiuk, V.N.; Mochalin, V.N.; Gogotsi, Y. Bending rigidity of two-dimensional titanium carbide (MXene) nanoribbons: A molecular dynamics study. Comput. Mater. Sci. 2018, 143, 418–424. [Google Scholar] [CrossRef]
- Cao, G.; Chen, X. Buckling of single-walled carbon nanotubes upon bending: Molecular dynamics simulations and finite element method. Phys. Rev. B 2006, 73, 155435. [Google Scholar] [CrossRef]
- Ru, C.Q. Effective bending stiffness of carbon nanotubes. Phys. Rev. B 2000, 62, 9973. [Google Scholar] [CrossRef]
- Zepeda-Ruiz, L.A.; Stukowski, A.; Oppelstrup, T.; Bulatov, V.V. Probing the limits of metal plasticity with molecular dynamics simulations. Nature 2017, 550, 492–495. [Google Scholar] [CrossRef]
- Chen, Q.; Zhang, Z.; Huang, Y.; Zhao, H.; Chen, Z.; Gao, K.; Yue, T.; Zhang, L.; Liu, J. Structure–mechanics relation of natural rubber: Insights from molecular dynamics simulations. ACS Appl. Polym. Mater. 2022, 4, 3575–3586. [Google Scholar] [CrossRef]
- Reddy, K.V.; Pal, S. Influence of dislocations, twins, and stacking faults on the fracture behavior of nanocrystalline Ni nanowire under constant bending load: A molecular dynamics study. J. Mol. Model. 2018, 24, 277. [Google Scholar] [CrossRef]
- Nath, S.K.D. Elastic, elastic–plastic properties of Ag, Cu and Ni nanowires by the bending test using molecular dynamics simulations. Comput. Mater. Sci. 2014, 87, 138–144. [Google Scholar] [CrossRef]
- Wu, H.A. Molecular dynamics simulation of loading rate and surface effects on the elastic bending behavior of metal nanorod. Comput. Mater. Sci. 2004, 31, 287–291. [Google Scholar] [CrossRef]
- Shen, Y.K.; Wu, H.A. Interlayer shear effect on multilayer graphene subjected to bending. Appl. Phys. Lett. 2012, 100, 101909. [Google Scholar] [CrossRef]
- Wei, Y.; Wang, B.; Wu, J.; Yang, R.; Dunn, M.L. Bending rigidity and Gaussian bending stiffness of single-layered graphene. Nano Lett. 2013, 13, 26–30. [Google Scholar] [CrossRef] [PubMed]
- Fan, H.; El-Awady, J.A. Molecular dynamics simulations of orientation effects during tension, compression, and bending deformations of magnesium nanocrystals. J. Appl. Mech. 2015, 82, 101006. [Google Scholar] [CrossRef]
- Tian, X.; Cui, J.; Zhang, C.; Ma, Z.; Wan, R.; Zhang, Q. Investigations on the deformation mechanisms of single-crystalline Cu nanowires under bending and torsion. Comput. Mater. Sci. 2014, 83, 250–254. [Google Scholar] [CrossRef]
- Horx, P.; Geyer, A. Defining the mobility range of a hinge-type connection using molecular dynamics and metadynamics. PLoS ONE 2020, 15, e0230962. [Google Scholar] [CrossRef]
- Lu, L.; Guo, Y.; Li, X.; Zhang, T. The atomic structure, magnetic properties and bending ductility of a novel Fe-PCB-Si amorphous alloy investigated by experiments and ab initio molecular dynamics. J. Alloys Compd. 2022, 904, 164101. [Google Scholar] [CrossRef]
- Van Gunsteren, W.F.; Berendsen, H.J.C. Algorithms for macromolecular dynamics and constraint dynamics. Mol. Phys. 1977, 34, 1311–1327. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.; Postma, J.P.M.; van Gunsteren, W.F.; DiNola, A.; Haak, J.R. Molecular dynamics with coupling to an external bath. J. Chem. Phys. 1984, 81, 3684–3690. [Google Scholar] [CrossRef]
- Štich, I.; Car, R.; Parrinello, M.; Baroni, S. Conjugate gradient minimization of the energy functional: A new method for electronic structure calculation. Phys. Rev. B 1989, 39, 4997. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Cioni, M.; Polino, D.; Rapetti, D.; Pesce, L.; Delle Piane, M.; Pavan, G.M. Innate dynamics and identity crisis of a metal surface unveiled by machine learning of atomic environments. J. Chem. Phys. 2023, 158, 124701. [Google Scholar] [CrossRef] [PubMed]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Modell. Simul. Mater. Sci. Eng. 2009, 18, 015012. [Google Scholar] [CrossRef]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Brown, W.M.; Crozier, P.S.; In’t Veld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS-a flexible simulation tool for particle-based materials modeling at the atomic, meso, and continuum scales. Comput. Phys. Commun. 2022, 271, 108171. [Google Scholar] [CrossRef]
- Guo, Y.B.; Xu, T.; Li, M. Atomistic calculation of internal stress in nanoscale polycrystalline materials. Philos. Mag. 2012, 92, 3064–3083. [Google Scholar] [CrossRef]
- Zhou, P.; Xu, S.; Wang, Z.; Yan, Y.; Kang, R.; Guo, D. A load identification method for the grinding damage induced stress (GDIS) distribution in silicon wafers. Int. J. Mach. Tools Manuf. 2016, 107, 1–7. [Google Scholar] [CrossRef]
- Puligheddu, M.; Xia, Y.; Chan, M.; Galli, G. Computational prediction of lattice thermal conductivity: A comparison of molecular dynamics and Boltzmann transport approaches. Phys. Rev. Mater. 2019, 3, 085401. [Google Scholar] [CrossRef]
- Horstemeyer, M.F.; Lim, J.; Lu, W.Y.; Mosher, D.A.; Baskes, M.I.; Prantil, V.C.; Plimpton, S.J. Torsion/simple shear of single crystal copper. J. Eng. Mater. Technol. 2002, 124, 322–328. [Google Scholar] [CrossRef]
- Zheng, Q.; Zhou, Z.; Ding, C.; Li, Y.; Lin, E.; Ye, S.; Piao, Z. Mechanical response of single-crystal copper under vibration excitation based on molecular dynamics simulation. J. Manuf. Process. 2022, 75, 605–616. [Google Scholar]
- Xu, W.; Dávila, L.P. Tensile nanomechanics and the Hall-Petch effect in nanocrystalline aluminium. Mater. Sci. Eng. A 2018, 710, 413–418. [Google Scholar] [CrossRef]
- Sharma, A.; Datta, D.; Balasubramaniam, R. Molecular dynamics simulation to investigate the orientation effects on nanoscale cutting of single crystal copper. Comput. Mater. Sci. 2018, 153, 241–250. [Google Scholar] [CrossRef]
- Zheng, G.P.; Li, M. Crystal instability in nanocrystalline materials. Acta Mater. 2007, 55, 5464–5472. [Google Scholar] [CrossRef]
- Rajgarhia, R.K.; Spearot, D.E.; Saxena, A. Molecular dynamics simulations of dislocation activity in single-crystal and nanocrystalline copper doped with antimony. Metall. Mater. Trans. A 2010, 41, 854–860. [Google Scholar] [CrossRef]
- Guo, Y.B.; Xu, T.; Li, M. Generalized type III internal stress from interfaces, triple junctions and other microstructural components in nanocrystalline materials. Acta Mater. 2013, 61, 4974–4983. [Google Scholar] [CrossRef]
- Zhao, K.J.; Chen, C.Q.; Shen, Y.P.; Lu, T.J. Molecular dynamics study on the nano-void growth in face-centered cubic single crystal copper. Comput. Mater. Sci. 2009, 46, 749–754. [Google Scholar] [CrossRef]
- Cao, H.; Rui, Z.; Yang, F. Mechanical properties of Cu nanowires: Effects of cross-sectional area and temperature. Mater. Sci. Eng. A 2020, 791, 139644. [Google Scholar] [CrossRef]
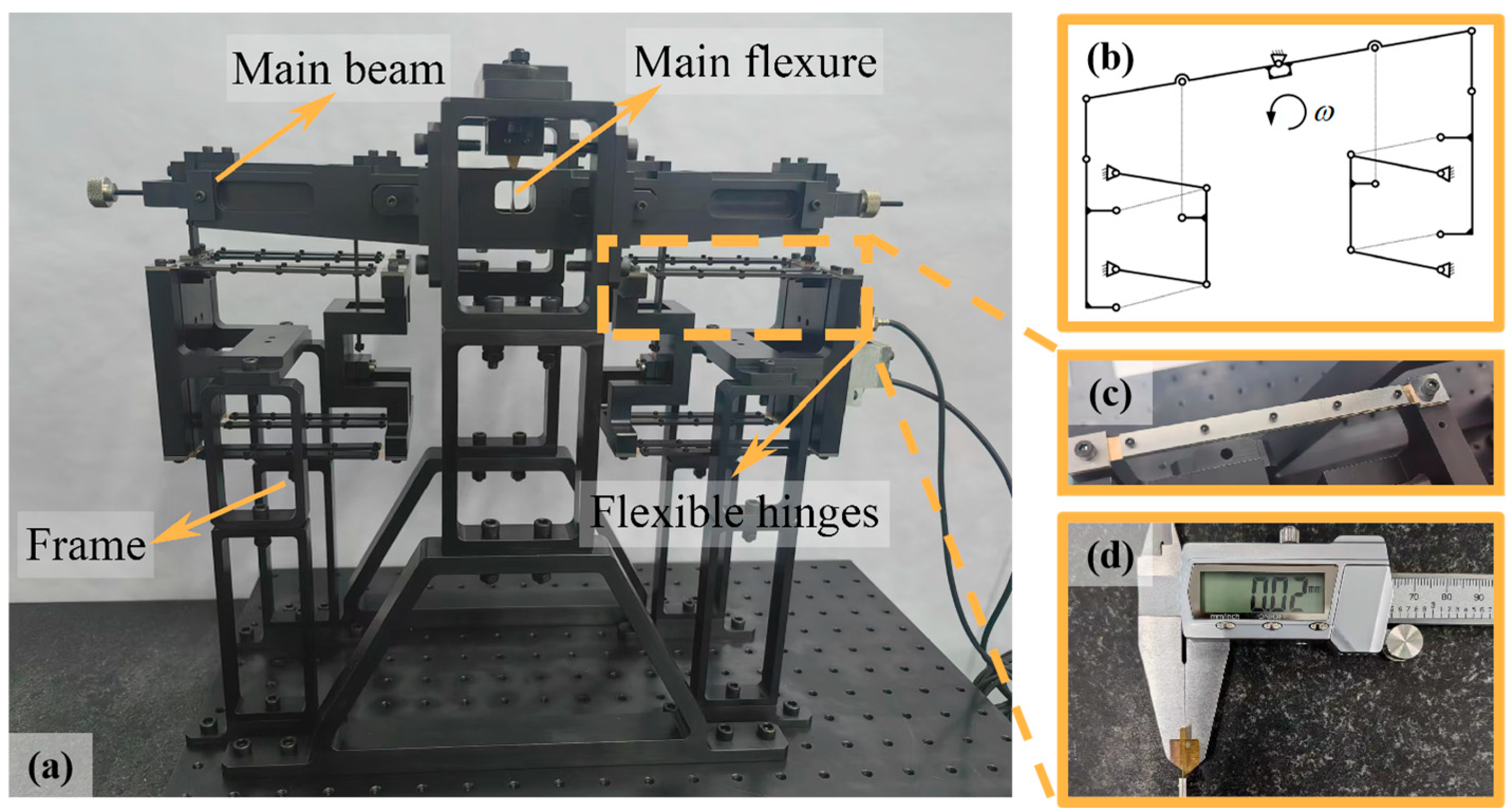

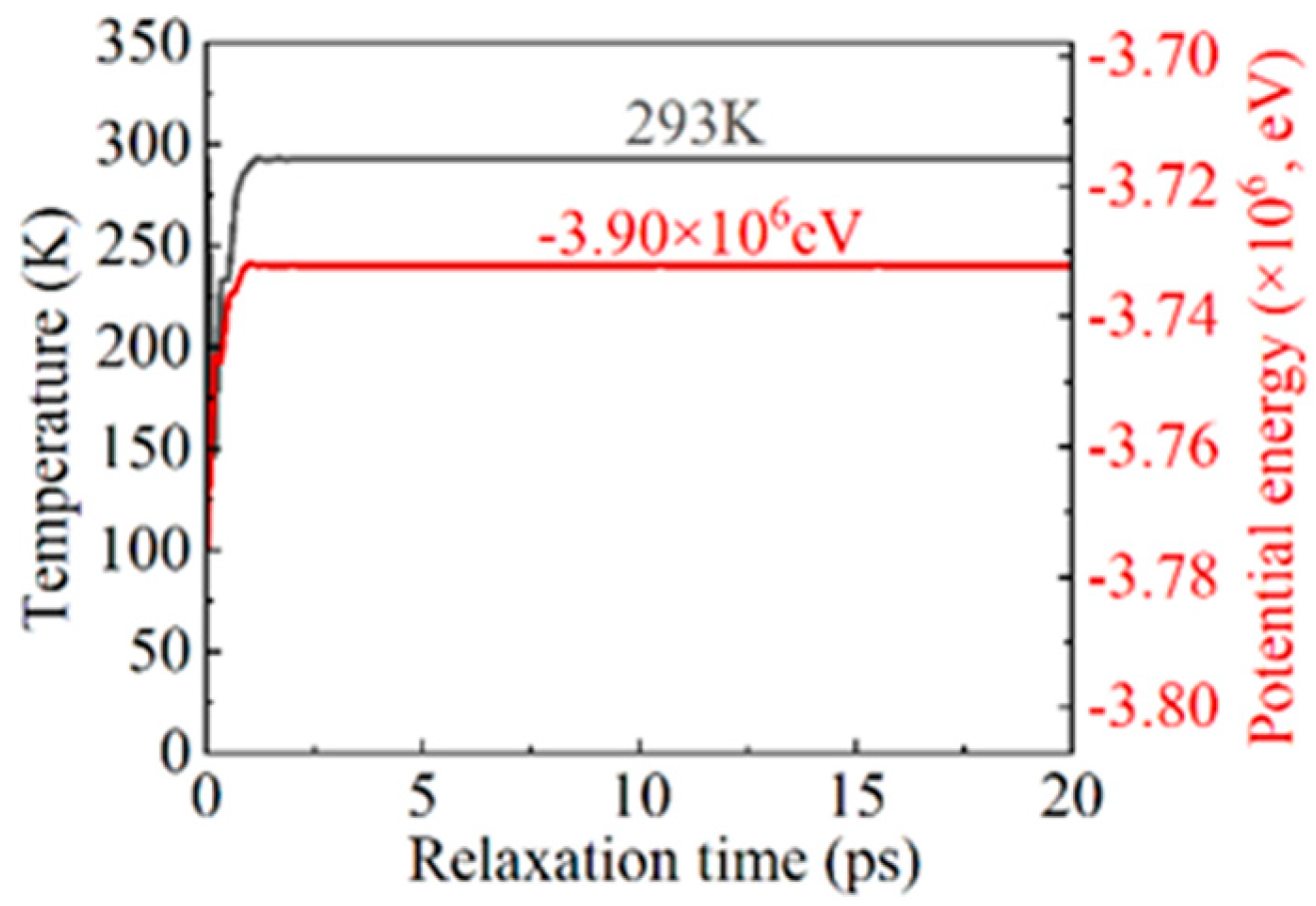
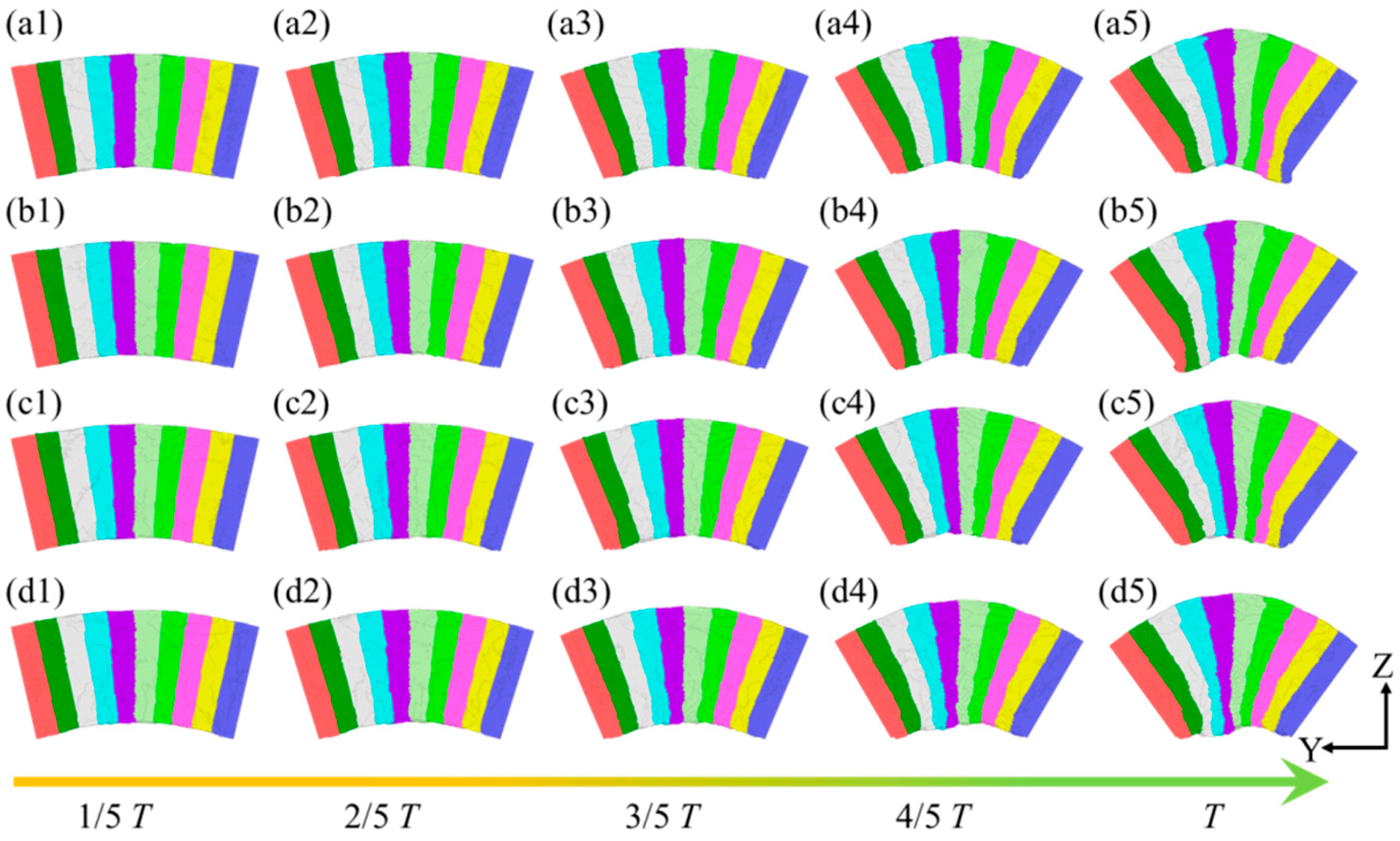
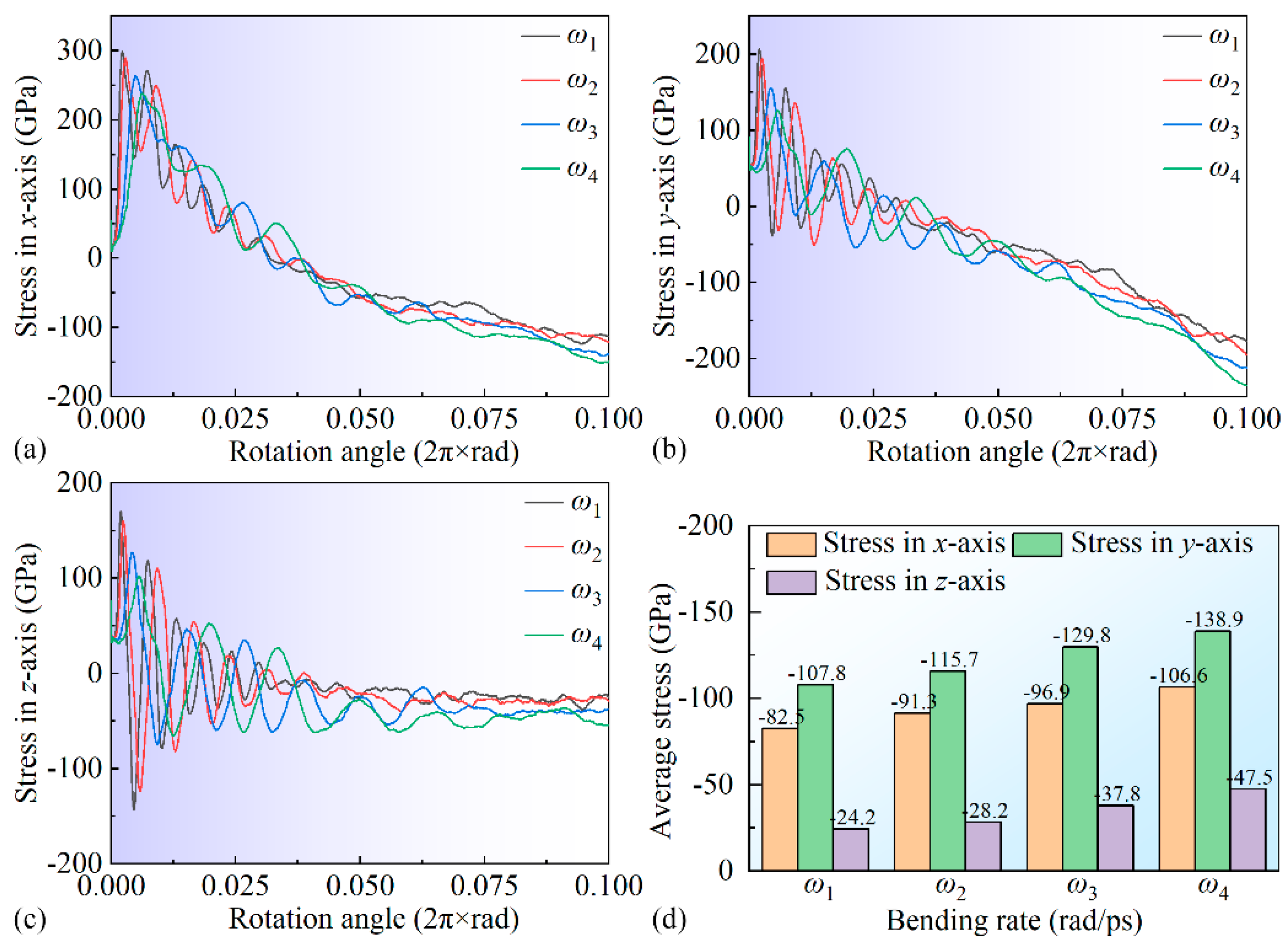





| Parameters | Value |
|---|---|
| Material | Single-crystal Cu |
| Dimensions | 100 Å × 500 Å × 250 Å |
| Number of atoms | 1,066,464 |
| Boundary conditions | Periodic boundary condition |
| Simulation ensemble | NPT, NVE |
| Potential function | EAM |
| Bending rate | ±2π/2000 rad/ps~±2π/800 rad/ps |
| Timestep | 1 fs |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, P.; Zhao, P.; Li, Z.; Wu, J.; Tan, J. Investigation on the Bending Mechanism of Single-Crystal Copper Under High Bending Rates via Molecular Dynamics. Micromachines 2025, 16, 314. https://doi.org/10.3390/mi16030314
Wu P, Zhao P, Li Z, Wu J, Tan J. Investigation on the Bending Mechanism of Single-Crystal Copper Under High Bending Rates via Molecular Dynamics. Micromachines. 2025; 16(3):314. https://doi.org/10.3390/mi16030314
Chicago/Turabian StyleWu, Peng, Pengyue Zhao, Zhengkun Li, Jianwei Wu, and Jiubin Tan. 2025. "Investigation on the Bending Mechanism of Single-Crystal Copper Under High Bending Rates via Molecular Dynamics" Micromachines 16, no. 3: 314. https://doi.org/10.3390/mi16030314
APA StyleWu, P., Zhao, P., Li, Z., Wu, J., & Tan, J. (2025). Investigation on the Bending Mechanism of Single-Crystal Copper Under High Bending Rates via Molecular Dynamics. Micromachines, 16(3), 314. https://doi.org/10.3390/mi16030314









