A Review of Pressure Drop and Mixing Characteristics in Passive Mixers Involving Miscible Liquids
Abstract
1. Introduction
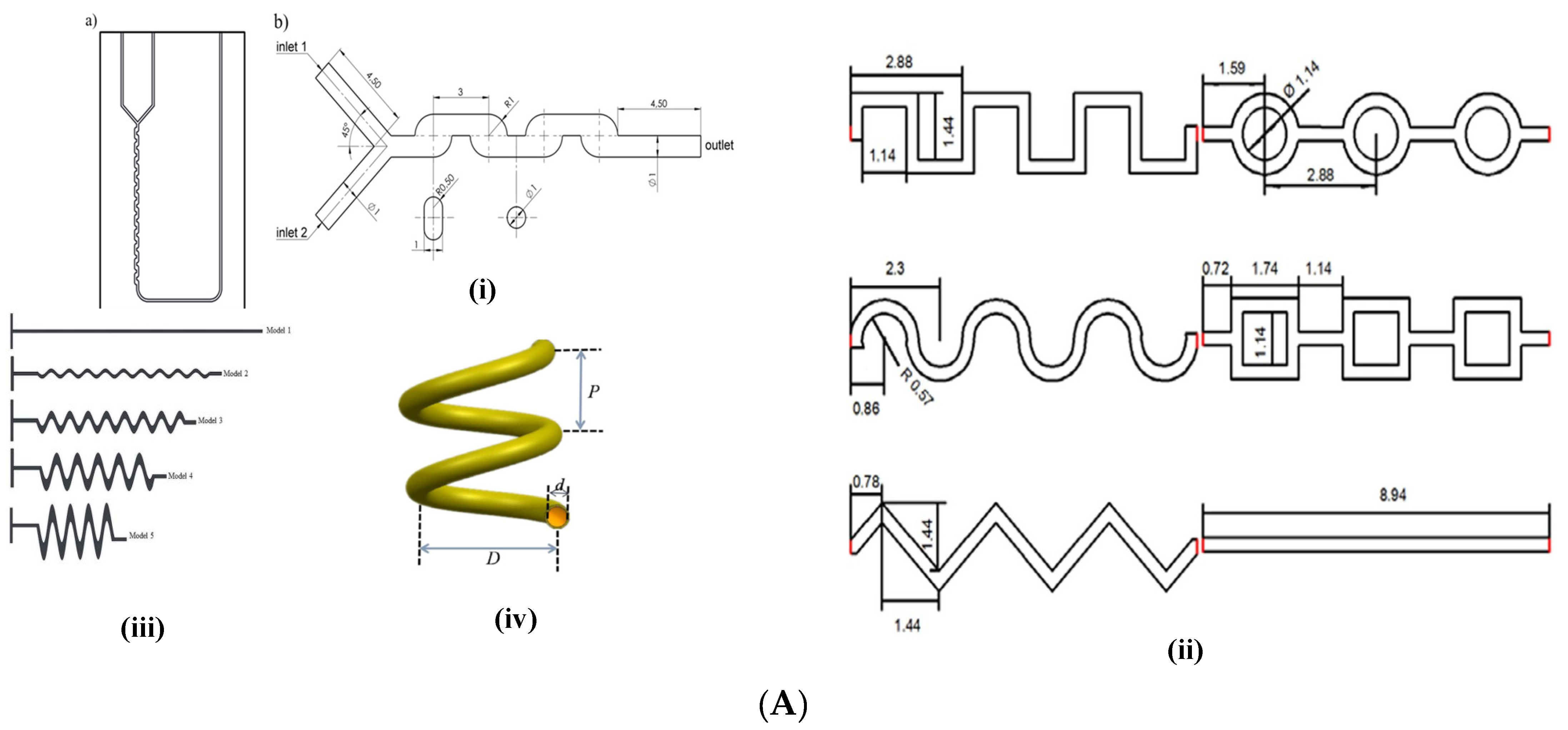
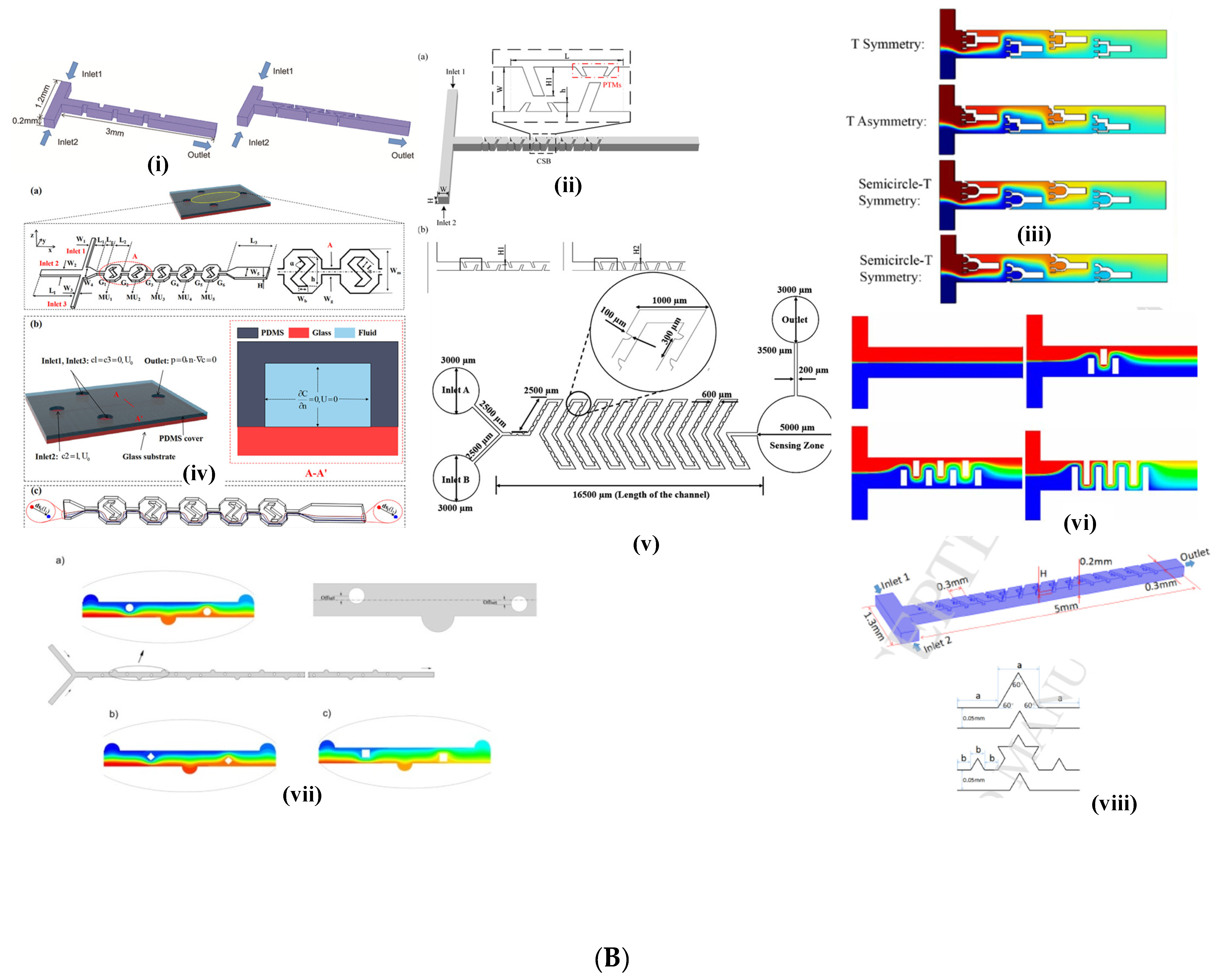
2. Categorization of Passive Micromixers
2.1. Category 1: Simple Passive Micromixers
2.2. Category 2: Passive Micromixers with Flow Direction Variation
2.3. Category 3: Passive Micromixers with Flow Obstructions
2.4. Category 4: Complex Passive Micromixers
3. Mixing Index (MI) of Passive Micromixers
3.1. Empirical/Analytical Expressions Used for Calculation of Mixing Index in Passive Micromixers
3.2. Effect of Operating, Geometric Parameters, and Fluid Properties on Mixing Index in Passive Micromixers
4. Pressure Drop in of Passive Micromixers
4.1. Analytical and Empirical Expressions for Pressure Drop in Passive Micromixers
4.2. Effect of Geometric and Operating Parameters on Pressure Drop in Passive Micromixers
5. Discussion
6. Empirical Expression for Friction Factor
7. Conclusions
- The categorization and appropriate abbreviation of micromixers introduced in the present work will contribute to the understanding flow, transport, and performance characteristics of micromixers. The categorization has been based on the size, shape, layout, whether or not it is curved or having obstructions, or a combination of shapes and complex shapes, inlet and outlet configuration, and the flow rate.
- Although MI has largely been considered as having a linear relationship with flow rate, at a certain Re, Category 1 mixers might undergo an S-shaped structure or non-uniform profile, inducing rapid mixing and increasing the MI values.
- The Peclet number is an important parameter for understanding the mixing. Diffusion coefficient influences mixing speed, with higher values promoting rapid mixing. Concentration disparities and mixing ratios influence MI while the mixing protocol, including injection sequence and timing, and the duration of the mixing process also affect mixing efficiency.
- Flow regimes over a wide range of Reynolds numbers depend on two aspects, the category of the micromixers and the Reynolds number.
- In Categories 1 and 2, the MI values are restricted to 0.1 < MI < 0.6 for Re range, 40 < Re < 300, while certain designs in Category 2 like helical coil give high MI (~upto 0.8) values due to the dean vortices and lower pressure drops. Categories 3 and 4 give MI values between 0.7 and 1 for a wide range of Re from 0.1 to 100. An interesting trend in Mi is observed with MI undergoing minima for lower Re in the range considered and then increasing with increases in Re.
- The MI can be distinctly divided into three zones with low MI values from 0.1 to 0.4 for Category 1 micromixers where the mixing is diffusion dominated, Category 2 and 3 mixed convection diffusion in the MI range 0.4 to 0.7, and Category 2 (helical mixers), but mostly Category 3 and 4, which have chaotic or stochastic motion for the MI range 0.7 to 1.
- A comprehensive representation of pressure drop versus MI is presented for the optimum operable range for micromixers. This is expected to help the design engineer to select an optimum design of micromixer for a smooth and economically viable operation. The figure also recommends the non-operable region, the operable region for low Re flows, and the operable region for special flows.
- A comparison of pressure drop in micromixers with those of microchannels shows some micromixers having an increase in pressure drop by two to three orders of magnitude. Hence, the design and selection of micromixer should be made with caution, preferably within the design limits of the optimum range proposed in the present work.
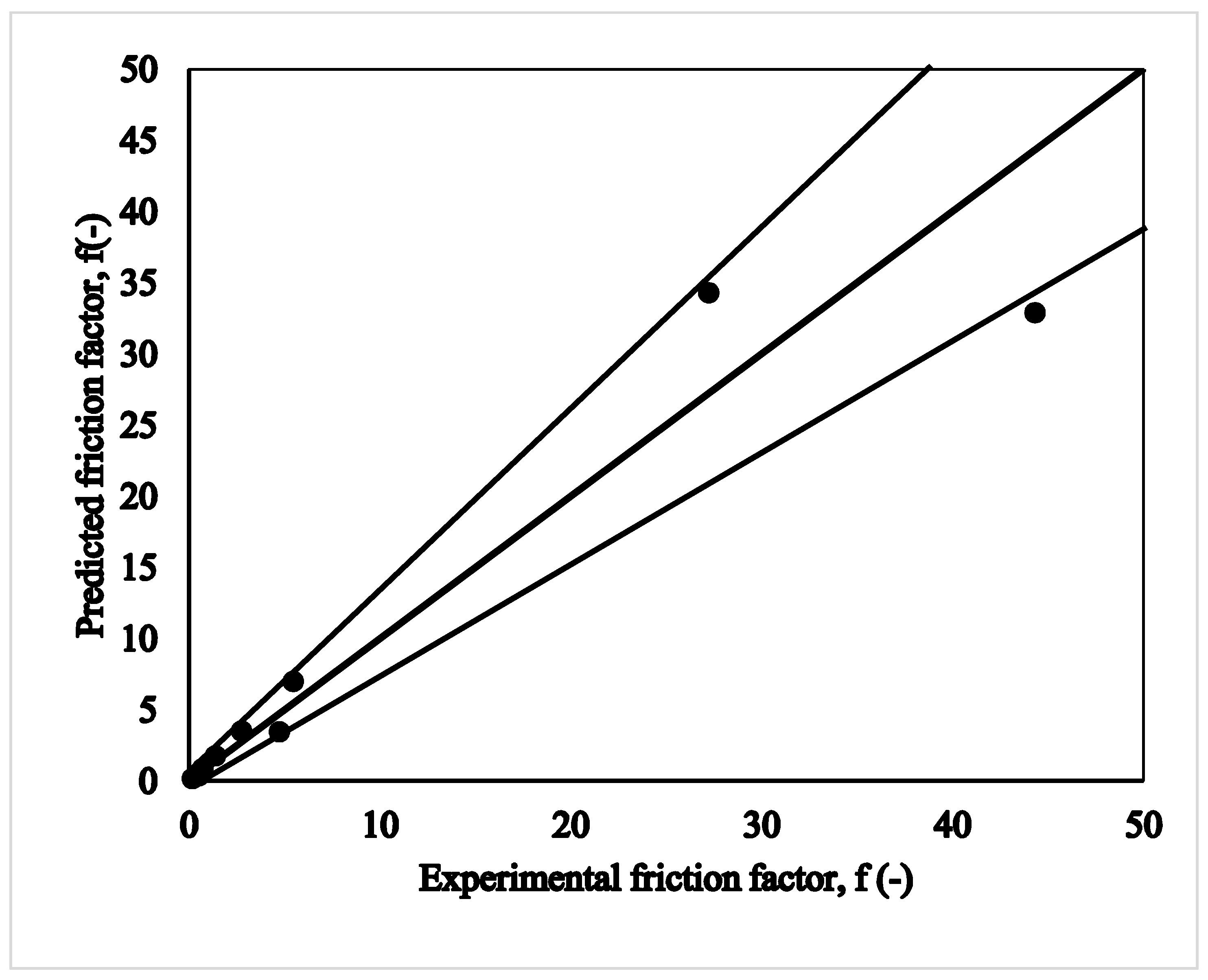
- A correlation for friction factors based on the pressure drop from the literature data has been developed which will help in also understanding the heat and mass transfer characteristics.
Funding
Conflicts of Interest
Abbreviations
| Alphabetical letters | ||
| A | Total Available Area for the Fluid Flow | m2 |
| ∆b | Buoyancy term | m·s−2 |
| Ca | Capillary Number | - |
| Ci | Concentration at the ith point | mol·m−3 |
| Average Concentration of the N points | mol·m−3 | |
| D | Diameter | m |
| DAB | Diffusivity of species A in species B | m2·s−1 |
| De | Dean number | - |
| DH | Hydraulic Diameter of the Geometry in which the Flowing Fluid | m |
| dh | Hydraulic diameter of the flowing fluid | m |
| f | fanning Friction Factor | - |
| fD | Darcy’s Friction Factor | - |
| L | Charasteristic length | m |
| MI | Mixing Index | - |
| N | Total Number of points used in the Calculation of the Mixing Index | - |
| Nu | Nusselt number | - |
| P | Total Available Perimeter for the Fluid Flow | M |
| Pe | Peclet Number | - |
| ∆P | Pressure Drop | Pa |
| Pr | Prandtl number | - |
| Q | The Volumetric Flow rate of the Fluid | m3·s−1 |
| R | radius | m |
| Rc | Radius of curvature | m |
| Ri | Richardson number | - |
| Re | Reynolds Number of the Flowing Fluid | - |
| Sc | Schmidt number | - |
| Sh | Sherwood number | - |
| St | Stanton number | - |
| ∆U | Difference in linear velocity | m·s−1 |
| U | Linear velocity | m·s−1 |
| K | Local Loss Factor | - |
| vqvg | Average Velocity of the Flowing Fluid | m·s−1 |
| vi | Velocity at the ith point | m·s−1 |
| Greek Alphabets | ||
| λ1 | Overall Friction Factor | - |
| λ | Mean free path in definition of Knudsen number | - |
| μ | Dynamics Viscosity of the Flowing Fluid | Pa·s |
| ξ | Effective Friction Factor | - |
| ρ | Density of the Flowing Fluid | kg·m−3 |
| σM | Standard Deviation of the Concentration Field of the Mixture | - |
| σM,max | Maximum Standard Deviation of the Concentration Field of the Mixture | - |
| σ | Surface tension, Nm−1 | |
References
- Hessel, V.; Löwe, H.; Schönfeld, F. Micromixers—A review on passive and active mixing principles. Chem. Eng. Sci. 2005, 60, 2479–2501. [Google Scholar] [CrossRef]
- Ganguli, A.A.; Pandit, A.B. Hydrodynamics of Liquid-Liquid Flows in Micro Channels and Its Influence on Transport Properties: A Review. Energies 2021, 14, 6066. [Google Scholar] [CrossRef]
- Ganguli, A.A.; Pandit, A.B.; Kunzru, D. Transport phenomena in microchannels in liquid–liquid extraction (LLE) systems operating in a slug flow regime—A review. Can. J. Chem. Eng. 2023, 102, 459–480. [Google Scholar] [CrossRef]
- Cai, G.; Xue, L.; Zhang, H.; Lin, J. A review on micromixers. Micromachines 2017, 8, 274. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.-Y.; Chang, C.-L.; Wang, Y.-N.; Fu, L.-M. Microfluidic mixing: A review. Int. J. Mol. Sci. 2011, 12, 3263–3287. [Google Scholar] [CrossRef] [PubMed]
- Khan, A.H.; Ganguli, A.; Edirisinghe, M.; Dalvi, S.V. Experimental and Computational Investigation of Microbubble Formation in a Single Capillary Embedded T-junction Microfluidic Device. Langmuir 2023, 39, 18971–18982. [Google Scholar] [CrossRef] [PubMed]
- Das, D.; Duraiswamy, S.; Yi, Z.; Chan, V.; Yang, C. Continuous droplet-based liquid-liquid extraction of phenol from oil. Sep. Sci. Technol. 2015, 50, 1023–1029. [Google Scholar] [CrossRef]
- Floris, A.; Staal, S.; Lenk, S.; Staijen, E.; Kohlheyer, D.; Eijkel, J.; van den Berg, A. A prefilled, ready-to-use electrophoresis based lab-on-a-chip device for monitoring lithium in blood. Lab A Chip 2010, 10, 1799–1806. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Li, L.; Xie, P.; Luo, G. Liquid–liquid microflow reaction engineering. React. Chem. Eng. 2017, 2, 611–627. [Google Scholar] [CrossRef]
- Liu, J.; Zhu, X.; Liao, Q.; Chen, R.; Ye, D.; Feng, H.; Liu, M.; Chen, G. Layer-by-layer self-assembly of palladium nanocatalysts with polyelectrolytes grafted on the polydopamine functionalized gas-liquid-solid microreactor. Chem. Eng. J. 2018, 332, 174–182. [Google Scholar] [CrossRef]
- Ter Schiphorst, J.; Melpignano, G.G.; Amirabadi, H.E.; Houben, M.H.; Bakker, S.; Den Toonder, J.M.; Schenning, A.P. Photoresponsive Passive Micromixers Based on Spiropyran Size-Tunable Hydrogels. Macromol. Rapid Commun. 2017, 39, 1700086. [Google Scholar] [CrossRef]
- Tamagawa, O.; Muto, A. Development of cesium ion extraction process using a slug flow microreactor. Chem. Eng. J. 2010, 167, 700–704. [Google Scholar] [CrossRef]
- Li, W.; Xia, F.; Qin, H.; Zhang, M.; Li, W.; Zhang, J. Numerical and experimental investigations of micromixing performance and efficiency in a pore-array intensified tube-in-tube microchannel reactor. Chem. Eng. J. 2019, 370, 1350–1365. [Google Scholar] [CrossRef]
- Lei, Y.; Tang, L.; Xie, Y.; Xianyu, Y.; Zhang, L.; Wang, P.; Hamada, Y.; Jiang, K.; Zheng, W.; Jiang, X. Gold nanoclusters-assisted delivery of NGF siRNA for effective treatment of pancreatic cancer. Nat. Commun. 2017, 8, 15130. [Google Scholar] [CrossRef] [PubMed]
- Rawal, S.; Yang, Y.-P.; Cote, R.; Agarwal, A. Identification and quantitation of circulating tumor cells. Annu. Rev. Anal. Chem. 2017, 10, 321–343. [Google Scholar] [CrossRef] [PubMed]
- Sun, J.; Xianyu, Y.; Jiang, X. Point-of-care biochemical assays using gold nanoparticle-implemented microfluidics. Chem. Soc. Rev. 2014, 43, 6239–6253. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Chen, X.; Ma, X.; Kong, X.; Xu, Z.; Wang, J. Fast DNA hybridization on a microfluidic mixing device based on pneumatic driving. Talanta 2011, 84, 565–571. [Google Scholar] [CrossRef] [PubMed]
- Papadopoulos, V.; Kefala, I.; Kaprou, G.; Kokkoris, G.; Moschou, D.; Papadakis, G.; Gizeli, E.; Tserepi, A. A passive micromixer for enzymatic digestion of DNA. Microelectron. Eng. 2014, 124, 42–46. [Google Scholar] [CrossRef]
- Nguyen, N.-T.; Hejazian, M.; Ooi, C.H.; Kashaninejad, N. Recent advances and future perspectives on microfluidic liquid handling. Micromachines 2017, 8, 186. [Google Scholar] [CrossRef]
- Wiggins, S.; Ottino, J.M. Foundations of chaotic mixing. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 2004, 362, 937–970. [Google Scholar] [CrossRef]
- Ottino, J.M. Mixing, chaotic advection, and turbulence. Annu. Rev. Fluid Mech. 1990, 22, 207–254. [Google Scholar] [CrossRef]
- Ottino, J.M. The Kinematics of Mixing: Stretching, Chaos, and Transport; Cambridge University Press: Cambridge, UK, 1989; Volume 3. [Google Scholar]
- Cieślik, A.; Akkermans, R.A.; Kamp, L.P.; Clercx, H.J.; van Heijst, G.J. Dipole-wall collision in a shallow fluid. Eur. J. Mech./Fluids 2009, 28, 397–404. [Google Scholar] [CrossRef]
- Mansur, E.A.; Mingxing, Y.; Yundong, W.; Youyuan, D. A state-of-the-art review of mixing in microfluidic mixers. Chin. J. Chem. Eng. 2008, 16, 503–516. [Google Scholar] [CrossRef]
- Raza, W.; Hossain, S.; Kim, K.-Y. A review of passive micromixers with a comparative analysis. Micromachines 2020, 11, 455. [Google Scholar] [CrossRef] [PubMed]
- Bahrami, D.; Bayareh, M. Experimental and numerical investigation of a novel spiral micromixer with sinusoidal channel walls. Chem. Eng. Technol. 2021, 45, 100–109. [Google Scholar] [CrossRef]
- Lobasov, A.; Minakov, A. Analyzing mixing quality in a T-shaped micromixer for different fluids properties through numerical simulation. Chem. Eng. Process. Process. Intensif. 2018, 124, 11–23. [Google Scholar] [CrossRef]
- Luo, J.-Z.; Luo, Y.; Chu, G.-W.; Arowo, M.; Xiang, Y.; Sun, B.-C.; Chen, J.-F. Micromixing efficiency of a novel helical tube reactor: CFD prediction and experimental characterization. Chem. Eng. Sci. 2016, 155, 386–396. [Google Scholar] [CrossRef]
- Jin, S.-Y.; Liu, Y.-Z.; Wang, W.-Z.; Cao, Z.-M.; Koyama, H.S. Numerical evaluation of two-fluid mixing in a swirl micro-mixer. J. Hydrodyn. 2006, 18, 542–546. [Google Scholar] [CrossRef]
- Akbarzadeh, M.; Rashidi, S.; Esfahani, J. Influences of corrugation profiles on entropy generation, heat transfer, pressure drop, and performance in a wavy channel. Appl. Therm. Eng. 2017, 116, 278–291. [Google Scholar] [CrossRef]
- Rahmannezhad, J.; Mirbozorgi, S.A. CFD analysis and RSM-based design optimization of novel grooved micromixers with obstructions. Int. J. Heat Mass Transf. 2019, 140, 483–497. [Google Scholar] [CrossRef]
- Niu, D.; Chen, J.; Xiong, M.; Cao, Y.; He, C.; Chen, J.; Liu, H. Numerical investigation of multiscale lateral microstructures enhancing passive micromixing efficiency via secondary vortex flow. Phys. Fluids 2022, 34, 092006. [Google Scholar] [CrossRef]
- Hou, C.; Li, L.; Lv, R.; Tian, Z.; Chen, X. Mixing Performance of Micromixers with Fractal Obstacles Based on Murray’s Law. Chem. Eng. Technol. 2021, 44, 2220–2227. [Google Scholar] [CrossRef]
- Rudyak, V.; Minakov, A. Modeling and optimization of Y-type micromixers. Micromachines 2014, 5, 886–912. [Google Scholar] [CrossRef]
- Zhang, S.; Chen, X.; Wu, Z.; Zheng, Y. Numerical Study on Koch Fractal Baffle Micromixer. Fractals 2019, 27, 1950026. [Google Scholar] [CrossRef]
- Adeosun, J.T.; Lawal, A. Residence-time distribution as a measure of mixing in T-junction and multilaminated/elongational flow micromixers. Chem. Eng. Sci. 2010, 65, 1865–1874. [Google Scholar] [CrossRef]
- Zhang, N.; Zha, K.; Wang, J. Exploring the Design Efficiency of Random Microfluidic Mixers. IEEE Access 2021, 9, 9864–9872. [Google Scholar] [CrossRef]
- Plouffe, P.; Roberge, D.M.; Macchi, A. Liquid–liquid flow regimes and mass transfer in various micro-reactors. Chem. Eng. J. 2016, 300, 9–19. [Google Scholar] [CrossRef]
- Nieves-Remacha, M.J.; Kulkarni, A.A.; Jensen, K.F. Hydrodynamics of liquid–liquid dispersion in an advanced-flow reactor. Ind. Eng. Chem. Res. 2012, 51, 16251–16262. [Google Scholar] [CrossRef]
- Ruijin, W.; Beiqi, L.; Dongdong, S.; Zefei, Z. Investigation on the splitting-merging passive micromixer based on Baker’s transformation. Sens. Actuators B Chem. 2017, 249, 395–404. [Google Scholar] [CrossRef]
- Okuducu, M.B.; Aral, M.M. Novel 3-d t-shaped passive micromixer design with helicoidal flows. Processes 2019, 7, 637. [Google Scholar] [CrossRef]
- Aubin, J.; Fletcher, D.F.; Xuereb, C. Design of micromixers using CFD modelling. Chem. Eng. Sci. 2005, 60, 2503–2516. [Google Scholar] [CrossRef]
- Malecha, K.; Golonka, L.J.; Bałdyga, J.; Jasińska, M.; Sobieszuk, P. Serpentine microfluidic mixer made in LTCC. Sens. Actuators B Chem. 2009, 143, 400–413. [Google Scholar] [CrossRef]
- Balan, C.M.; Broboana, D.; Balan, C. Mixing process of immiscible fluids in microchannels. Int. J. Heat Fluid Flow 2010, 31, 1125–1133. [Google Scholar] [CrossRef]
- Du, Y.; Zhang, Z.; Yim, C.; Lin, M.; Cao, X. Evaluation of floor-grooved micromixers using concentration-channel length profiles. Micromachines 2010, 1, 19–33. [Google Scholar] [CrossRef]
- Ait Mouheb, N.; Montillet, A.; Solliec, C.; Havlica, J.; Legentilhomme, P.; Comiti, J.; Tihon, J. Flow characterization in T-shaped and cross-shaped micromixers. Microfluid. Nanofluidics 2011, 10, 1185–1197. [Google Scholar] [CrossRef]
- Rudyak, V.Y.; Minakov, A.; Gavrilov, A.; Dekterev, A. Modelling of flows in micromixers. Thermophys. Aeromechanics 2010, 17, 565–576. [Google Scholar] [CrossRef]
- Fang, W.-F.; Hsu, M.-H.; Chen, Y.-T.; Yang, J.-T. Characterization of microfluidic mixing and reaction in microchannels via analysis of cross-sectional patterns. Biomicrofluidics 2011, 5, 014111. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.J.; Lai, Y.R.; Tsai, R.T.; Der Lin, J.; Wu, C.Y. Crosswise ridge micromixers with split and recombination helical flows. Chem. Eng. Sci. 2011, 66, 2164–2176. [Google Scholar] [CrossRef]
- Dreher, S.; Kockmann, N.; Woias, P. Characterization of laminar transient flow regimes and mixing in T-shaped micromixers. Heat Transf. Eng. 2009, 30, 91–100. [Google Scholar] [CrossRef]
- Du¨ chs, D.; Brunklaus, S.; Spang, P.; Ritzi-Lehnert, M.; Drese, K. Numerical Study of Micromixers for Stopped Flow. In Proceedings of the International Conference on Nanochannels, Microchannels, and Minichannels, Edmonton, AL, Canada, 19–22 June 2011; pp. 151–153. [Google Scholar]
- Tseng, L.-Y.; Yang, A.-S.; Lee, C.-Y.; Hsieh, C.-Y. CFD-based optimization of a diamond-obstacles inserted micromixer with boundary protrusions. Eng. Appl. Comput. Fluid Mech. 2011, 5, 210–222. [Google Scholar] [CrossRef]
- Fang, Y.; Ye, Y.; Shen, R.; Zhu, P.; Guo, R.; Hu, Y.; Wu, L. Mixing enhancement by simple periodic geometric features in microchannels. Chem. Eng. J. 2012, 187, 306–310. [Google Scholar] [CrossRef]
- Fischer, M.; Kockmann, N. Enhanced Convective Mixing and Residence Time Distribution in Advanced Micromixers. In Proceedings of the International Conference on Nanochannels, Microchannels, and Minichannels, Rio Grande, PR, USA, 8–12 July 2012; pp. 481–488. [Google Scholar]
- Roudgar, M.; Brunazzi, E.; Galletti, C.; Mauri, R. Numerical Study of Split T-Micromixers. Chem. Eng. Technol. 2012, 35, 1291–1299. [Google Scholar] [CrossRef]
- Leung, W.W.-F.; Ren, Y. Crossflow and mixing in obstructed and width-constricted rotating radial microchannel. Int. J. Heat Mass Transf. 2013, 64, 457–467. [Google Scholar] [CrossRef]
- Wang, L.; Wu, W.; Li, X. Numerical and experimental investigation of mixing characteristics in the constructal tree-shaped microchannel. Int. J. Heat Mass Transf. 2013, 67, 1014–1023. [Google Scholar] [CrossRef]
- Plouffe, P.; Macchi, A.; Donaldson, A.A. Enhancement of interphase transport in mini-/microscale applications using passive mixing. Heat Transf. Eng. 2013, 34, 159–168. [Google Scholar] [CrossRef]
- Cheri, M.S.; Latifi, H.; Moghaddam, M.S.; Shahraki, H. Simulation and experimental investigation of planar micromixers with short-mixing-length. Chem. Eng. J. 2013, 234, 247–255. [Google Scholar] [CrossRef]
- Su, Y.; Lautenschleger, A.; Chen, G.; Kenig, E.Y. A numerical study on liquid mixing in multichannel micromixers. Ind. Eng. Chem. Res. 2013, 53, 390–401. [Google Scholar] [CrossRef]
- Tran-Minh, N.; Dong, T.; Karlsen, F. An efficient passive planar micromixer with ellipse-like micropillars for continuous mixing of human blood. Comput. Methods Programs Biomed. 2014, 117, 20–29. [Google Scholar] [CrossRef] [PubMed]
- Parsa, M.K.; Hormozi, F.; Jafari, D. Mixing enhancement in a passive micromixer with convergent–divergent sinusoidal microchannels and different ratio of amplitude to wave length. Comput. Fluids 2014, 105, 82–90. [Google Scholar] [CrossRef]
- Picardo, J.R.; Pushpavanam, S. Laterally stratified flow in a curved microchannel. Int. J. Multiph. Flow 2015, 75, 39–53. [Google Scholar] [CrossRef]
- Chandra, A.; Kishor, K.; Mishra, P.; Alam, M. Numerical investigations of two-phase flows through enhanced microchannels. Chem. Biochem. Eng. Q. 2016, 30, 149–159. [Google Scholar] [CrossRef]
- Chen, X.; Li, T.; Zeng, H.; Hu, Z.; Fu, B. Numerical and experimental investigation on micromixers with serpentine microchannels. Int. J. Heat Mass Transf. 2016, 98, 131–140. [Google Scholar] [CrossRef]
- Huang, S.-W.; Wu, C.-Y.; Lai, B.-H.; Chien, Y.-C. Fluid mixing in a swirl-inducing microchannel with square and T-shaped cross-sections. Microsyst. Technol. 2016, 23, 1971–1981. [Google Scholar] [CrossRef]
- Ortega-Casanova, J. CFD study on mixing enhancement in a channel at a low Reynolds number by pitching a square cylinder. Comput. Fluids 2017, 145, 141–152. [Google Scholar] [CrossRef]
- Xiong, Q.-Q.; Chen, Z.; Li, S.-W.; Wang, Y.-D.; Xu, J.-H. Micro-PIV measurement and CFD simulation of flow field and swirling strength during droplet formation process in a coaxial microchannel. Chem. Eng. Sci. 2018, 185, 157–167. [Google Scholar] [CrossRef]
- Ansari, M.A.; Kim, K.-Y.; Kim, S.M. Numerical and experimental study on mixing performances of simple and vortex micro T-mixers. Micromachines 2018, 9, 204. [Google Scholar] [CrossRef] [PubMed]
- Khaydarov, V.; Borovinskaya, E.S.; Reschetilowski, W. Numerical and experimental investigations of a micromixer with chicane mixing geometry. Appl. Sci. 2018, 8, 2458. [Google Scholar] [CrossRef]
- Borgohain, P.; Arumughan, J.; Dalal, A.; Natarajan, G. Design and performance of a three-dimensional micromixer with curved ribs. Chem. Eng. Res. Des. 2018, 136, 761–775. [Google Scholar] [CrossRef]
- Silva Jr, J.; Santana, H.; Taranto, O. Numerical Simulations of New Uniform Circular Obstacles Cross-flow Micromixers. Chem. Eng. Trans. 2019, 74, 1045–1050. [Google Scholar]
- Cheng, K.; Liu, C.; Guo, T.; Wen, L. CFD and experimental investigations on the micromixing performance of single countercurrent-flow microchannel reactor. Chin. J. Chem. Eng. 2019, 27, 1079–1088. [Google Scholar] [CrossRef]
- Chen, Y.; Chen, X. Numerical investigations of a passive micromixer based on minkowski fractal principle. Int. J. Chem. React. Eng. 2019, 18, 20190105. [Google Scholar] [CrossRef]
- Farahinia, A.; Zhang, W. Numerical investigation into the mixing performance of micro T-mixers with different patterns of obstacles. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 491. [Google Scholar] [CrossRef]
- Hosseini, F.; Rahimi, M. Computational fluid dynamics and experimental investigations on liquid–liquid mass transfer in T-type microchannels with different mixing channel barrier shapes. Sep. Sci. Technol. 2019, 55, 3502–3516. [Google Scholar] [CrossRef]
- Amar, K.; Embarek, D.; Sofiane, K. Parametric study of the Crossing elongation effect on the mixing performances using short Two-Layer Crossing Channels Micromixer (TLCCM) geometry. Chem. Eng. Res. Des. 2020, 158, 33–43. [Google Scholar] [CrossRef]
- Okuducu, M.B.; Aral, M.M. Toward the next generation of passive micromixers: A novel 3-D design approach. Micromachines 2021, 12, 372. [Google Scholar] [CrossRef] [PubMed]
- Feng, Q.; Chen, X.; Wang, X.; Yu, X.; Zeng, X.; Ma, Y.; Wang, Q. Numerical simulation of a three dimensional electroosmotic micromixer with a flexible and controllable Rubik’s cube module. Int. Commun. Heat Mass Transf. 2021, 127, 105482. [Google Scholar] [CrossRef]
- Talebjedi, B.; Ghazi, M.; Tasnim, N.; Janfaza, S.; Hoorfar, M. Performance optimization of a novel passive T-shaped micromixer with deformable baffles. Chem. Eng. Process. Process Intensif. 2021, 163, 108369. [Google Scholar] [CrossRef]
- Lotfiani, A.; Rezazadeh, G. A new two-layer passive micromixer design based on SAR-vortex principles. Int. J. Chem. React. Eng. 2021, 19, 309–329. [Google Scholar] [CrossRef]
- Wang, X.; Yang, L.; Sun, F. CFD analysis and RSM optimization of obstacle layout in Tesla micromixer. Int. J. Chem. React. Eng. 2021, 19, 1045–1055. [Google Scholar] [CrossRef]
- Mahmud, M.; Hossain, S.; Kim, J.-H. A SAR Micromixer for Water-Water Mixing: Design, Optimization, and Analysis. Processes 2021, 9, 1926. [Google Scholar] [CrossRef]
- Tripathi, E.; Patowari, P.K.; Pati, S. Comparative assessment of mixing characteristics and pressure drop in spiral and serpentine micromixers. Chem. Eng. Process. Process Intensif. 2021, 162, 108335. [Google Scholar] [CrossRef]
- Tripathi, E.; Patowari, P.K.; Pati, S. Numerical investigation of mixing performance in spiral micromixers based on Dean flows and chaotic advection. Chem. Eng. Process. Process. Intensif. 2021, 169, 108609. [Google Scholar] [CrossRef]
- Valeh-e-Sheyda, P.; Yarmohammad, M. Numerical Modeling of Micromixing Performance of Five Generic Microchannel Reactors using Villermaux/Dushman Competing Test Reaction. J. Chem. Pet. Eng. 2022, 56, 257–272. [Google Scholar]
- Yuan, S.; Zhou, M.; Peng, T.; Li, Q.; Jiang, F. An investigation of chaotic mixing behavior in a planar microfluidic mixer. Phys. Fluids 2022, 34, 032007. [Google Scholar] [CrossRef]
- Shanbhag, V.V.; Mukherjee, J.; Pandit, A.B. Analytical and numerical investigations of mixing fluids in microchannel systems of different geometrical configurations. Can. J. Chem. Eng. 2022, 100, 2217–2229. [Google Scholar] [CrossRef]
- Karthikeyan, K.; Kandasamy, S.K.; Saravanan, P.; Alodhayb, A. Numerical simulation and parameter optimization of micromixer device using fuzzy logic technique. RSC Adv. 2023, 13, 4504–4522. [Google Scholar]
- Babu, H.V.; Sahu, S.; Haderlein, M.; Peukert, W.; Verma, N. Numerical investigation of flow patterns and concentration profiles in Y-mixers. Chem. Eng. Technol. 2016, 39, 1963–1971. [Google Scholar] [CrossRef]
- Mariotti, A.; Antognoli, M.; Galletti, C.; Mauri, R.; Salvetti, M.V.; Brunazzi, E. A Study on the Effect of Flow Unsteadiness on the Yield of a Chemical Reaction in a T Micro-Reactor. Micromachines 2021, 12, 242. [Google Scholar] [CrossRef]
- Fatima, U.; Shakaib, M. A Numerical Study of the Effects of Narrow Channel Dimensions on Pressure Drop and Mass Transfer Performance of a Mixer Device. Iran. J. Chem. Chem. Eng. 2022, 41, 1727–1739. [Google Scholar]
- Minakov, A.; Yagodnitsyna, A.; Lobasov, A.; Rudyak, V.; Bilsky, A. Study of fluid flow in micromixer with symmetrical and asymmetrical inlet conditions. La Houille Blanche 2013, 99, 12–21. [Google Scholar] [CrossRef]
- Strang, E.; Fernando, H. Entrainment and mixing in stratified shear flows. J. Fluid Mech. 2001, 428, 349–386. [Google Scholar] [CrossRef]
- Chung, C.-K.; Shih, T. Effect of geometry on fluid mixing of the rhombic micromixers. Microfluid. Nanofluidics 2007, 4, 419–425. [Google Scholar] [CrossRef]
- Hsiao, K.-Y.; Wu, C.-Y.; Huang, Y.-T. Fluid mixing in a microchannel with longitudinal vortex generators. Chem. Eng. J. 2014, 235, 27–36. [Google Scholar] [CrossRef]
- Nieves-Remacha, M.J.; Kulkarni, A.A.; Jensen, K.F. OpenFOAM computational fluid dynamic simulations of single-phase flows in an advanced-flow reactor. Ind. Eng. Chem. Res. 2015, 54, 7543–7553. [Google Scholar] [CrossRef]
- Sommer, K. The Variance as Measured Variable for the Evaluation of a Mixing Process or for the Comparison of Mixtures and Mixers. In Micro and Macro Mixing: Analysis, Simulation and Numerical Calculation; Springer: Berlin/Heidelberg, Germany, 2010; pp. 3–15. [Google Scholar]
- Engler, M.; Kockmann, N.; Kiefer, T.; Woias, P. Numerical and experimental investigations on liquid mixing in static micromixers. Chem. Eng. J. 2004, 101, 315–322. [Google Scholar] [CrossRef]
- Dundi, T.M.; Raju, V.; Chandramohan, V. Numerical evaluation of swirl effect on liquid mixing in a passive T-micromixer. Aust. J. Mech. Eng. 2019, 19, 363–377. [Google Scholar] [CrossRef]
- Kirby, B.J. Micro-and Nanoscale Fluid Mechanics: Transport in Microfluidic Devices; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Howell, G.W.; Weathers, T.M.; Trw Systems Group Redondo Beach Ca. Aerospace Fluid Component Designers’ Handbook, Volume II, Revision D.; Report No. RPL-TDR-64-25; TRW Systems Group, One Space Park: Redondo Beach, CA, USA, 1970. [Google Scholar]
- Viktorov, V.; Mahmud, M.R.; Visconte, C. Comparative analysis of passive micromixers at a wide range of Reynolds numbers. Micromachines 2015, 6, 1166–1179. [Google Scholar] [CrossRef]
- Cortes-Quiroz, C.A.; Azarbadegan, A.; Zangeneh, M.; Goto, A. Analysis and multi-criteria design optimization of geometric characteristics of grooved micromixer. Chem. Eng. J. 2010, 160, 852–864. [Google Scholar] [CrossRef]
- Ganguli, A.A.; Pandit, A.B. Computational fluid dynamics simulations to improve performance characteristics of a manifold having a central inlet and outlet. Front. Energy Res. 2022, 10, 1013540. [Google Scholar] [CrossRef]

 Khaydarov et al. [70] (LTFMSM);
Khaydarov et al. [70] (LTFMSM);  Chen and Chen [74] (SMFOM);
Chen and Chen [74] (SMFOM);  Chen and Chen [74] (PMFOM)
Chen and Chen [74] (PMFOM)  Okuducu and Aral [41] (TLM);
Okuducu and Aral [41] (TLM);  Okuducu and Aral [41] (CSCRM);
Okuducu and Aral [41] (CSCRM);  Lotfiani and Razazadeh [81] (YJM);
Lotfiani and Razazadeh [81] (YJM);  Lotfiani and Razazadeh [81] (TLM);
Lotfiani and Razazadeh [81] (TLM);  Mahmud et al. [83] (HCM);
Mahmud et al. [83] (HCM);  Mahmud et al. [83] (YUM);
Mahmud et al. [83] (YUM);  Tripathi et al. [85] (SpiM);
Tripathi et al. [85] (SpiM);  Tripathi et al. [85] (RsPM);
Tripathi et al. [85] (RsPM);  Tripathi et al. [85] (SESpM);
Tripathi et al. [85] (SESpM);  Niu et al. [32] (ESBM);
Niu et al. [32] (ESBM);  Niu et al. [32] (CSBM);
Niu et al. [32] (CSBM);  Niu et al. [32] (SBM);
Niu et al. [32] (SBM);  Yuan et al. [87] (ASZMMM);
Yuan et al. [87] (ASZMMM);  Hou et al. [33] (TSFOM).
Hou et al. [33] (TSFOM).
 Khaydarov et al. [70] (LTFMSM);
Khaydarov et al. [70] (LTFMSM);  Chen and Chen [74] (SMFOM);
Chen and Chen [74] (SMFOM);  Chen and Chen [74] (PMFOM)
Chen and Chen [74] (PMFOM)  Okuducu and Aral [41] (TLM);
Okuducu and Aral [41] (TLM);  Okuducu and Aral [41] (CSCRM);
Okuducu and Aral [41] (CSCRM);  Lotfiani and Razazadeh [81] (YJM);
Lotfiani and Razazadeh [81] (YJM);  Lotfiani and Razazadeh [81] (TLM);
Lotfiani and Razazadeh [81] (TLM);  Mahmud et al. [83] (HCM);
Mahmud et al. [83] (HCM);  Mahmud et al. [83] (YUM);
Mahmud et al. [83] (YUM);  Tripathi et al. [85] (SpiM);
Tripathi et al. [85] (SpiM);  Tripathi et al. [85] (RsPM);
Tripathi et al. [85] (RsPM);  Tripathi et al. [85] (SESpM);
Tripathi et al. [85] (SESpM);  Niu et al. [32] (ESBM);
Niu et al. [32] (ESBM);  Niu et al. [32] (CSBM);
Niu et al. [32] (CSBM);  Niu et al. [32] (SBM);
Niu et al. [32] (SBM);  Yuan et al. [87] (ASZMMM);
Yuan et al. [87] (ASZMMM);  Hou et al. [33] (TSFOM).
Hou et al. [33] (TSFOM).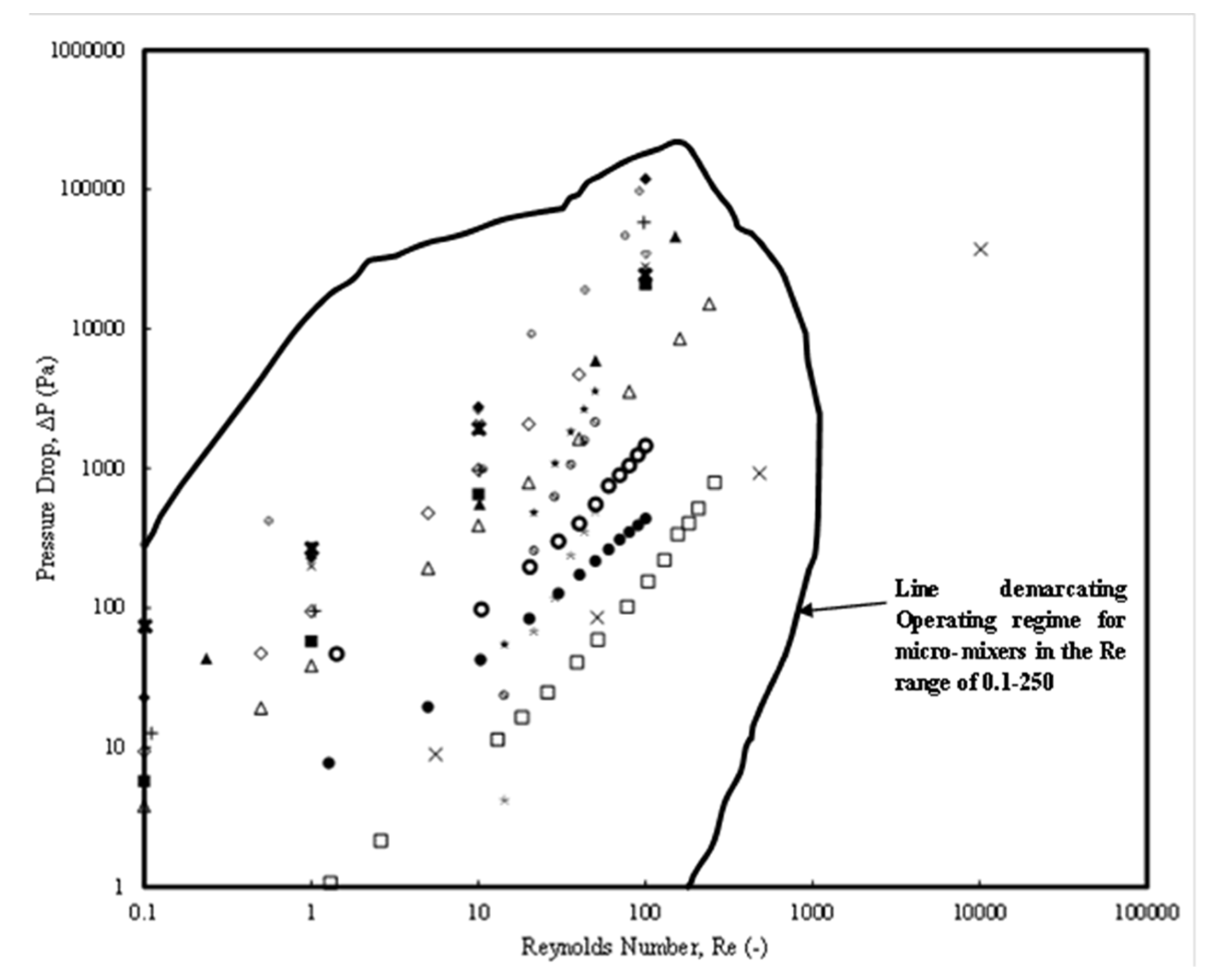
 Amar et al. [77] (TJM);
Amar et al. [77] (TJM);  Mahmud et al. [83] (YUM);
Mahmud et al. [83] (YUM);  Tripathi et al. [84] (YJM);
Tripathi et al. [84] (YJM);  Tripathi et al. [84] (SM);
Tripathi et al. [84] (SM);  Tripathi et al. [84] (SpiM);
Tripathi et al. [84] (SpiM);  Tripathi et al. [85] (SpiM);
Tripathi et al. [85] (SpiM);  Tripathi et al. [85] (RsPM);
Tripathi et al. [85] (RsPM);  Tripathi et al. [85] (SESpM);
Tripathi et al. [85] (SESpM);  Yuan et al. [87] (ASZMMM);
Yuan et al. [87] (ASZMMM);  Hou et al. [33] (TSFOM);
Hou et al. [33] (TSFOM);  Okuducu and Aral [78] (TJM);
Okuducu and Aral [78] (TJM);  Niu et al. [32] (ESBM);
Niu et al. [32] (ESBM);  Niu et al. [32] (CSBM);
Niu et al. [32] (CSBM);  Niu et al. [32] (SBM).
Niu et al. [32] (SBM).
 Amar et al. [77] (TJM);
Amar et al. [77] (TJM);  Mahmud et al. [83] (YUM);
Mahmud et al. [83] (YUM);  Tripathi et al. [84] (YJM);
Tripathi et al. [84] (YJM);  Tripathi et al. [84] (SM);
Tripathi et al. [84] (SM);  Tripathi et al. [84] (SpiM);
Tripathi et al. [84] (SpiM);  Tripathi et al. [85] (SpiM);
Tripathi et al. [85] (SpiM);  Tripathi et al. [85] (RsPM);
Tripathi et al. [85] (RsPM);  Tripathi et al. [85] (SESpM);
Tripathi et al. [85] (SESpM);  Yuan et al. [87] (ASZMMM);
Yuan et al. [87] (ASZMMM);  Hou et al. [33] (TSFOM);
Hou et al. [33] (TSFOM);  Okuducu and Aral [78] (TJM);
Okuducu and Aral [78] (TJM);  Niu et al. [32] (ESBM);
Niu et al. [32] (ESBM);  Niu et al. [32] (CSBM);
Niu et al. [32] (CSBM); 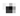 Niu et al. [32] (SBM).
Niu et al. [32] (SBM).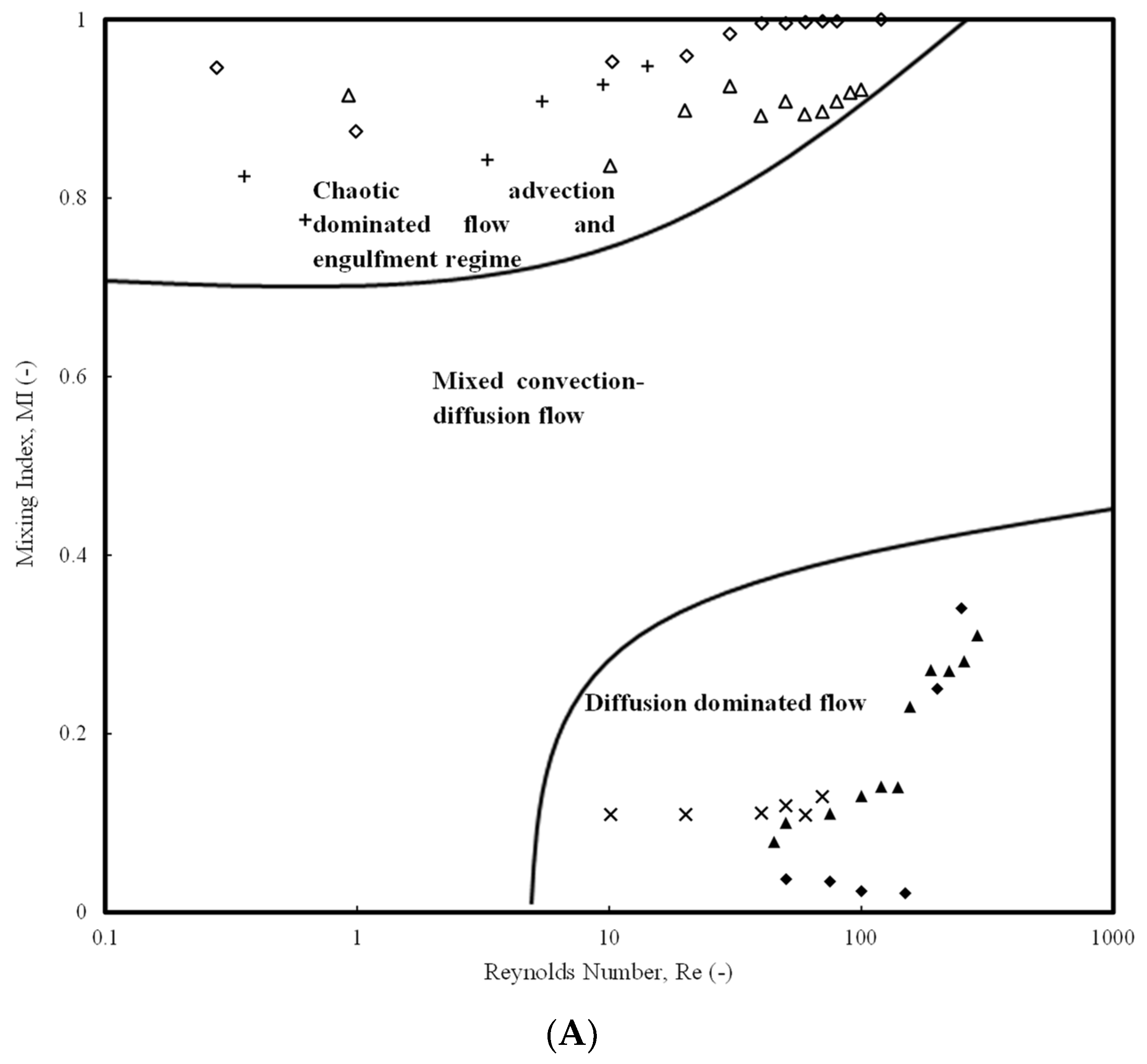
 Talebjedi et al. [80] (TJM);
Talebjedi et al. [80] (TJM);  Tripathi et al. [84] (YJM);
Tripathi et al. [84] (YJM);  Tripathi et al. [84] (SM);
Tripathi et al. [84] (SM);  Tripathi et al. [84] (SpiM);
Tripathi et al. [84] (SpiM);  Tripathi et al. [85] (SpiM);
Tripathi et al. [85] (SpiM);  Tripathi et al. [85] (RsPM)
Tripathi et al. [85] (RsPM)  Tripathi et al. [85] (SESpM);
Tripathi et al. [85] (SESpM);  Yuan et al. [87] (ASZMMM);
Yuan et al. [87] (ASZMMM);  Niu et al. [32] (ESBM);
Niu et al. [32] (ESBM);  Niu et al. [32] (CSBM);
Niu et al. [32] (CSBM);  Niu et al. [32] (SBM).
Niu et al. [32] (SBM).
 Talebjedi et al. [80] (TJM);
Talebjedi et al. [80] (TJM);  Tripathi et al. [84] (YJM);
Tripathi et al. [84] (YJM);  Tripathi et al. [84] (SM);
Tripathi et al. [84] (SM);  Tripathi et al. [84] (SpiM);
Tripathi et al. [84] (SpiM);  Tripathi et al. [85] (SpiM);
Tripathi et al. [85] (SpiM);  Tripathi et al. [85] (RsPM)
Tripathi et al. [85] (RsPM)  Tripathi et al. [85] (SESpM);
Tripathi et al. [85] (SESpM);  Yuan et al. [87] (ASZMMM);
Yuan et al. [87] (ASZMMM);  Niu et al. [32] (ESBM);
Niu et al. [32] (ESBM);  Niu et al. [32] (CSBM);
Niu et al. [32] (CSBM); 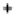 Niu et al. [32] (SBM).
Niu et al. [32] (SBM).
| Reference | Geometry | Geometry Abbreviation | Category |
|---|---|---|---|
| Aubin et al. [42] | Staggered Herringbone Micromixer | SHM | 2 |
| Jin et al. [29] | Swirl Micromixer | SwM | 2 |
| Adeosun and Lawal [36] | Multilaminated/Elongational Flow Micromixer | MEFM-4 | 2 |
| T-Junction Micromixer | TJM | 1 | |
| Malecha et al. [43] | I-Shaped Serpentine Micromixer | ISSM | 2 |
| Balan et al. [44] | Y-Junction Micromixer | YJM | 1 |
| Du et al. [45] | Slanted Groove Micromixer | SGM | 2 |
| Ait Mouheb et al. [46] | Cross Flow Junction Micromixer | CFJM | 1 |
| Rudyak et al. [47] | T-Junction Micromixer with Rectangular Inserts | RITJM | 3 |
| Fang et al. [48] | Overlapping Micromixer | OM | 3 |
| Chen et al. [49] | Crosswise Ridge Micromixer | CRM | 3 |
| Dreher et al. [50] | T-Junction Micromixers with Vertical Rectangular Cross Sections | TJMVRCS | 3 |
| Düchs et al. [51] | Berger Ball Micromixer | BBM | 4 |
| Tseng et al. [52] | Diamond Obstructed Y-Junction Micromixer | DOYJM | 3 |
| Fang et al. [53] | Periodically Obstructed Micromixer | POM | 3 |
| Nieves-Remacha et al. [39] | Corning Advanced Flow Reactor (Immiscible system) | CAFR | 4 |
| Fischer and Kockmann [54] | Tangential T-Junction Micromixer | TaTJM | 4 |
| Twisted Y-Junction Micromixer | TYJM | 4 | |
| Inverted Twisted Y-Junction Micromixer | ITYJM | 2 | |
| Roudgar et al. [55] | Horizontally Splited T-Junction Micromixer | HSTJM | 2 |
| Vertically Splited T-Junction Micromixer | VSTJM | 2 | |
| Leung and Ren [56] | Obstructed and Width Constricted Micromixer | OWCM | 3 |
| Wang et al. [57] | Tree-Shaped Micromixer | TSM | 4 |
| Plouffe et al. [58] | Venturi Micromixer (Immiscible system) | VM | 4 |
| Cylindrical Serpentine Micromixer (Immiscible System) | CSM | 2 | |
| Cheri et al. [59] | Hexagonal Chamber Micromixer | HeCM | 4 |
| Round Corner Rectangular Chamber Micromixer | RCRCM | 4 | |
| Su et al. [60] | Zigzag T-Junction Micromixer | ZTJM | 2 |
| Tran-Minh et al. [61] | Elliptical Obstructed Splitting and Recombination Micromixer | EOSARM | 4 |
| Parsa et al. [62] | Sinusoidal Micromixers | SiM | 2 |
| Rudyak and Minakov [34] | T-Type Micromixer with Rectangular Obstacles | ROTJM | 3 |
| Picardo and Pushpavanam [63] | Curved Micromixer | CM | 2 |
| Chandra et al. [64] | Enhanced Micromixer | EM | 3 |
| Chen et al. [65] | Square wave Serpentine Micromixer | SSM | 2 |
| Multi wave Serpentine Micromixer | MSM | 2 | |
| Zigzag Serpentine Micromixer | ZSM | 2 | |
| Luo et al. [28] | Helical Coiled Tube Micromixer | HCTM | 2 |
| Plouffe et al. [38] | SZ Micromixer (Immiscible system) | SZM | 4 |
| TG Micromixer (Immiscible system) | TGM | 4 | |
| Sickle Micromixer (Immiscible system) | SicM | 4 | |
| Spade Micromixer (Immiscible system) | SpM | 4 | |
| Huang et al. [66] | Longitudinal Modules Vortex Micromixer | LMVM | 3 |
| Ortega-Casanova [67] | Obstructed Micromixer with Square Cylinder | OMSC | 3 |
| Akbarzadeh et al. [30] | Sinusoidal Wavy Channel Micromixer | SWCM | 2 |
| Trapezoidal Wavy Channel Micromixer | TWCM | 2 | |
| Triangular Wavy Channel Micromixer | TrWCM | 2 | |
| Ruijin et al. [40] | Baker Micromixer | BM | 4 |
| Smale Micromixer | SmM | 4 | |
| Helical Micromixer | HM | 4 | |
| Xiong et al. [68] | Co-Axial Micromixer | CAM | 2 |
| Ansari et al. [69] | Vortex T-Junction Micromixer | VTJM | 2 |
| Khaydarov et al. [70] | LTF-MS Micromixer | LTFMSM | 2 |
| Borgohain et al. [71] | T-Junction Micromixer with Rectangular Winglet Pair | RWPTJM | 3 |
| Silva Jr et al. [72] | Uniform Circular Obstacles and Cross Flow | UCCFM | 3 |
| Zhang et al. [35] | Primary Koch Fractal Baffle Micromixer | PKFBM | 3 |
| Secondary Koch Fractal Baffle Micromixer | SKFBM | 3 | |
| Cheng et al. [73] | Single Countercurrent Flow Micromixer | SCFM | 2 |
| Li et al. [13] | Pore Array intensified Tube-in-tube Micromixer | PAITTM | 4 |
| Chen and Chen [74] | Primary Minkowski Fractal Obstacle Micromixer | PMFOM | 3 |
| Secondary Minkowski Fractal Obstacle Micromixer | SMFOM | 3 | |
| Rahmannezhad and Mirbozorgi [31] | Circular Obstructed Grooved Micromixer | COGM | 3 |
| Diamond Obstructed Grooved Micromixer | DOGM | 3 | |
| Square Obstructed Grooved Micromixer | SOGM | 3 | |
| Farahinia and Zhang [75] | Rectangular Obstacle T-Junction Micromixer | ROTJM | 3 |
| Diagonally Obstacle T-Junction Micromixer | DOTJM | 3 | |
| Diagonally Primary Minkowski Fractal Obstacle Micromixer | DPMFOM | 3 | |
| Hosseini and Rahimi [76] | Cylindrical Barrier Micromixer | CBM | 3 |
| Semi Cylindrical Barrier Micromixer | SCBM | 3 | |
| Conical Barrier Micromixer | CoBM | 3 | |
| Semi Conical Barrier Micromixer | SCoBM | 3 | |
| Okuducu and Aral [41] | Convex Semi-Circular Ridge Micromixer | CSCRM | 2 |
| Silv Jr. et al. [72] | Circular Obstacle Cross Flow Micromixer | COCFM | 3 |
| Amar et al. [77] | Two Layer Crossing Channel Micromixer | TLCCM | 4 |
| Hou et al. [33] | T Symmetry Fractal obstacle micromixer | TSFOM | 3 |
| T Asymmetry Fractal obstacle micromixer | TASFOM | 3 | |
| Semicircle T Symmetry Fractal obstacle micromixer | STSFOM | 3 | |
| Semicircle T Asymmetry Fractal obstacle micromixer | STASFOM | 3 | |
| Okuducu and Aral [78] | Circular-Shaped Fluid Overlapping Micromixer | CSFOM | 4 |
| Zhang et al. [37] | Random Micromixers | RM | 4 |
| Feng et al. [79] | Flexible Rubik’s Cube Module Micromixer | FRCMM | 4 |
| Talebjedi et al. [80] | Rigid Baffles T-Junction Micromixer | RBTJM | 3 |
| Deformable Baffles T Junction Micromixer | DBTJM | 3 | |
| Lotfiani and Rezazadeh [81] | Two Layer Micromixer | TLM | 4 |
| Wang et al. [82] | Diamond Obstacle Tesla Micromixer | DOTM | 3 |
| Mahmud et al. [83] | YU Micromixer | YUM | 4 |
| HC Micromixer | HCM | 4 | |
| Tripathi et al. [84] | Spiral Micromixer | SpiM | 2 |
| Tripathi et al. [85] | Sharp Edged Spiral Micromixer | SESpM | 2 |
| Rectangular Spiral Micromixer | RSpM | 2 | |
| Valeh-e-Sheyda and Yarmohammad [86] | Trapezoidal T-Junction Micromixer | TrTJM | 2 |
| Concentric Micromixer | CoM | 2 | |
| Caterpillar Micromixer | CaM | 2 | |
| Yuan et al. [87] | ASZMM Micromixer | ASZMMM | 3 |
| Bahrami and Bayareh [26] | Sinusoidal Walls Spiral Micromixer | SWSM | 2 |
| Niu et al. [32] | Staggered Baffles Micromixer | SBM | 3 |
| Cross Scale Staggered Baffles Micromixer | CSBM | 3 | |
| Equivalent Staggered Baffles Micromixer | ESBM | 3 | |
| Shanbhag et al. [88] | X-Shaped Micromixer | XsM | 2 |
| Karthikeyan et al. [89] | Obstacle Serpentine Micromixer | OSM | 3 |
| Reference | Geometric Dimensions | Fluid Details | Reynolds Number, Peclet Number, and Capillar Number | Pressure Drop (ΔP) Details | Mixing Index (MI) Details |
|---|---|---|---|---|---|
| Parsa et al. [62] | 0.06 cm < L < 1.4 cm; W = 200 mm; 100 μm < H < 322 μm | Methylene blue, water | Re < 50 | ΔP < 30,000 Pa | 0.2 < MI < 1 |
| Chen et al. [65] | - | Blue ink, yellow ink | 0.1 < Re < 200 | ΔP < 50,000 Pa | 0.3 < MI < 1 |
| Babu et al. [90] | W = 0.003 m; L = 0.0016 m; H = 0.001 m | Water–Water (with certain concentration) | 100 < Re < 400 | 10 Pa < ΔP < 10,000 Pa | 0.175 < MI < 0.4 |
| Ansari et al. [69] | W = 200 μm; H = 200 μm; L = 5 mm | Water, ethanol | 1 ≤ Re ≤ 80 | - | 0.01 < MI < 0.45 |
| Khaydarov et al. [70] | D = 1 mm; L = 21 mm | Colored ink, water | 1.3 ≤ Re ≤ 259.3 | 1 Pa < ΔP < 789 Pa | 0.33 ≤ MI ≤ 0.80 |
| Hosseini and Rahimi [76] | D = 0.9 mm; L = 10 cm | Water, ARS, Aliquat, 1-octanol | 47 ≤ Re ≤ 377 | 15 mbar < ΔP < 175 mbar | Performance ratio: 1 to 1.7 |
| Mariotti et al. [91] | W = 1 mm; L = 45 mm | Methylene blue, ascorbic acid | Re < 700 | - | - |
| Yuan et al. [87] | L > 4000 μm | Water, ethanol | 0.1 ≤ Re ≤ 50 | 0.002 kPa < ΔP < 100 kPa | 0.77 < MI < 0.99 |
| Bahrami and Bayareh [26] | W = 200 μm; L > 70 mm | Water–Concentrated water | 0 ≤ Re ≤ 100 | 0.1 kPa < ΔP < 40 kPa | 0.15 ≤ MI ≤ 0.99 |
| Reference | Geometric Dimensions | Fluid Details | CFD Software Details | Mesh Details | Reynolds Number Range | Pressure Drop (ΔP) Details | Mixing Index (MI)/Segregation Index (SI) Details |
|---|---|---|---|---|---|---|---|
| Amar et al. [77] | Dh = 350 μm | Water–dye | ANSYS Fluent 16.0 | M1 = 222,917 M2 = 429,113 M3 = 643,203 M4 = 1,083,357 | Re < 120; 150 ≤ Pe ≤ 13,840 | ΔP < 90 kPa | 0.1 < MI < 1 |
| Mariotti et al. [91] | H = W = 1 mm; L = 45 mm | Methylene blue, ascorbic acid | ANSYS Fluent v.19 | M = 4,700,000 | Re < 700 | - | - |
| Okuducu and Aral [78] | Inlet and exit W = 200 μm and diameter of 300 μm for circular part | Water and fluid with diffusivities 0.3, 1.5, and 3 nm2/s | OpenFOAM v5.0 | M1 = 3,900,000 M2 = 2,450,000 M3 = 1,580,000 M4 = 1,050,000 | 0.1 ≤ Re ≤ 300; 280 ≤ Pe ≤ 82,000 | 100 ≤ ΔP ≤ 14,000 | 0.5 ≤ MI ≤ 0.92 |
| Hou et al. [33] | Fractal shape with main channel W = 90 μm; L = 300 μm; other branches 45 and 22.5 μm width and 150 and 75 μm length | Water with concentration from 0 to 1 mol/lit | COMSOL Multiphysics 5.2a | M1 = 41,635 M2 = 79,089 M3 = 155,794 M4 = 338,441 | 0.1 ≤ Re ≤ 150 | ΔP < 50,000 Pa | 0.7 < MI < 1 |
| Talebjedi et al. [80] | H = 300 μm; L ≤ 8000 μm; deformable baffle heights < 125 μm and width = 20 μm | Water–water with concentration from 0 to 1 mol/m3 | COMSOL Multiphysics 5.4 | - | Re < 60 | ΔP < 3500 Pa | MI < 0.9 |
| Lotfiani and Rezazadeh [81] | 100 μm ≤ H ≤ 200 μm; 2800 μm ≤ L ≤ 20,000 μm | Water–water with concentration from 0–1 mol/m3 | ANSYS Fluent 17.0 | M1 = 107,078 M2 = 224,000 M3 = 233,600 | 1 < Re ≤ 100 | 10 Pa ≤ ΔP ≤ 100,000 Pa | MI ≤ 0.995 |
| Wang et al. [82] | W = H = 100 μm; L = 7 mm | Water–water | COMSOL Multiphysics 5.5 | M1 = 205,120 M2 = 353,085 M3 = 523,973 M4 = 710,479 M5 = 909,697 | 4 ≤ Re ≤ 200 | 198.4 ≤ ΔP < 536.02 Pa | 0.728 ≤ MI ≤ 0.964 |
| Mahmud et al. [83] | L = 7 mm; W = 400 μm | Water–water with diffusivity 1 nm2/s | ANSYS Fluent 15 | 200,000 < M < 1,000,000 | 0 ≤ Re ≤ 100 | ΔP < 1600 Pa | 0.4 < MI < 1 |
| Tripathi et al. [85] | H = 400 μm; W = 200 μm | Ethanol, water D = 1.24 × 10−9 m2/s | ANSYS Fluent 14 | M1 = 2,329,800 M2 = 2,447,975 M3 = 2,219,891 | 0.1 ≤ Re ≤ 100; 900 < Pe < 90,000 | ΔP < 35,000 Pa | MI < 0.9 |
| Tripathi et al. [84] | H = 400 μm; W = 200 μm; 12.24 mm ≤ L ≤ 31.65 mm | Ethanol, water D = 1.24 × 10−9 m2/s | ANSYS Fluent 18.1 | M < 3,500,000 | 1 ≤ Re ≤ 100; 900 < Pe < 90,000 | ΔP < 11,000 Pa | 0.1 < MI < 0.9 |
| Yuan et al. [87] | Width = 60 μm; H = 150 μm; L < 5000 μm | Ethanol, water | COMSOL Multiphysics 5.4; Ansys Fluent 15.0 | 1,250,000 ≤ M ≤ 1,850,000 | 0.1 ≤ Re ≤ 50; 52 < Pe < 7600 | 0.08 kPa < ΔP < 80 kPa | 0.8 < MI < 1 |
| Bahrami and Bayareh [26] | W = H = 600 μm; L < 80 mm | Water–water | ANSYS Fluent | 27,300 < M < 9,870,000 | 0 ≤ Re ≤ 100 | 0.1 kPa < ΔP < 45 kPa | 0.1 < MI < 1 |
| Niu et al. [32] | W = H = 600 μm; L = 10 mm | Water–water with concentration from 0 to 1 mol/m3 | COMSOL Multiphysics 5.4 | M1 = 33,976 M2 = 44,455 M3 = 62,207 M4 = 75,378 | 0.01 < Re < 50; 9 < Pe < 46,000 | 5 Pa < ΔP < 90,000 Pa | 0.2 < MI ≤ 1 |
| Fatima and Shakaib [92] | W = H = 400 μm; L = 6000 μm | Water–water with concentration from 0 to 1 mol/m3 | ANSYS Fluent | 19- | 20 ≤ Re ≤ 260 | ΔP < 6000 Pa | MI < 0.1; 0.8 < SI < 0.90 |
| Karthikeyan et al. [89] | W = 200 μm; L = 16.5 mm | Water–water with concentration from 0 to 1 mol/m3 | COMSOL Multiphysics 6.0 | M1 = 40,948 M2 = 55,750 M3 = 65,516 M4 = 137,008 | - | ΔP < 1400 Pa | 0.4 < MI < 1 |
| Author | Expression |
|---|---|
| Engler et al. [99] | ; ; |
| Rudyak et al. [34] | |
| Tseng et al. [52] | |
| Roudgar et al. [55] |
| Author | Expression |
|---|---|
| Kirby, B.J. [101] | |
| Howell and Weathers [102] | ; ; |
| Babu et al. [90] | ; |
| Khaydarov et al. [70] | |
| Hosseini and Rahimi [76] |
| Reference | Passive Micromixer | Category | Re | % Increase Pressure Drop Compared with Straight Channel | Mixing Index |
|---|---|---|---|---|---|
| Okuducu and Aral [41] | TJM | 1 | 40 | 51% | 25% |
| Lotfiani and Rezazadeh [81] | YJM | 1 | 100 | 164% | 58% |
| Mahmud et al. [83] | YUM | 2 | 50 | 83% | 51% |
| Khaydarov et al. [70] | LTFMSM | 2 | 52 | 69% | 25% |
| Mahmud et al. [83] | HCM | 2 | 50 | 163% | 94% |
| Okuducu and Aral [41] | CSCRM | 3 | 40 | 337% | 80% |
| Niu et al. [32] | SBM | 3 | 50 | 561% | 53% |
| Niu et al. [32] | CSBM | 3 | 50 | 2797% | 98% |
| Niu et al. [32] | ESBM | 3 | 50 | 4767% | 94% |
| Lotfiani and Rezazadeh [81] | TLM | 4 | 100 | 883% | 81% |
| Borgohain et al. [71] | TJMRWP | 3 | 51 | 430% | 85% |
| Yuan et al. [87] | ASZMMM | 3 | 50 | 225% | 100% |
| Tripathi et al. [84] | YJM | 2 | 50 | 150% | 90% |
| Wang et al. [57] | TSM | 4 | 50 | 110% | 75% |
| Ruijin et al. [40] | BM | 4 | 20 | 101% | 60% |
| Author | Total Mesh | Smallest Element, μm |
|---|---|---|
| Chen et al. [74] | 155,794 | 5 |
| Amar et al. [77] | 643,203 | 6 |
| Mahmud et al. [83] | 7.54 × 105 | 20 |
| Okuducu and Aral [78] | 1.45 × 106 | 5 |
| Tripathi et al. (a) [84] | 1,635,434 | 11 |
| Niu et al. [32] | 62,207 | 20 |
| Yuan et al. [87] | 3.87 × 106 | 5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ganguli, A.; Bhatt, V.; Yagodnitsyna, A.; Pinjari, D.; Pandit, A. A Review of Pressure Drop and Mixing Characteristics in Passive Mixers Involving Miscible Liquids. Micromachines 2024, 15, 691. https://doi.org/10.3390/mi15060691
Ganguli A, Bhatt V, Yagodnitsyna A, Pinjari D, Pandit A. A Review of Pressure Drop and Mixing Characteristics in Passive Mixers Involving Miscible Liquids. Micromachines. 2024; 15(6):691. https://doi.org/10.3390/mi15060691
Chicago/Turabian StyleGanguli, Arijit, Viraj Bhatt, Anna Yagodnitsyna, Dipak Pinjari, and Aniruddha Pandit. 2024. "A Review of Pressure Drop and Mixing Characteristics in Passive Mixers Involving Miscible Liquids" Micromachines 15, no. 6: 691. https://doi.org/10.3390/mi15060691
APA StyleGanguli, A., Bhatt, V., Yagodnitsyna, A., Pinjari, D., & Pandit, A. (2024). A Review of Pressure Drop and Mixing Characteristics in Passive Mixers Involving Miscible Liquids. Micromachines, 15(6), 691. https://doi.org/10.3390/mi15060691





