Design and Optimization of a Fan-Out Wafer-Level Packaging- Based Integrated Passive Device Structure for FMCW Radar Applications
Abstract
1. Introduction
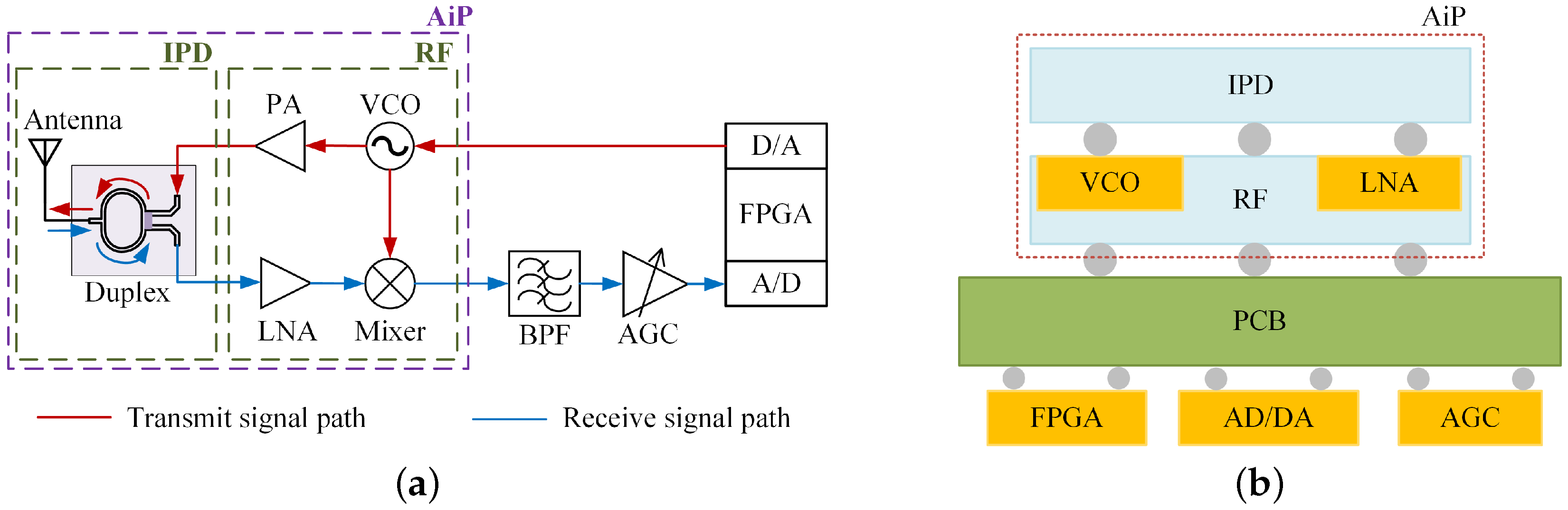
2. Structure, Process, and Empirical Formula-Based Dimensioning of the Integrated Passive Device
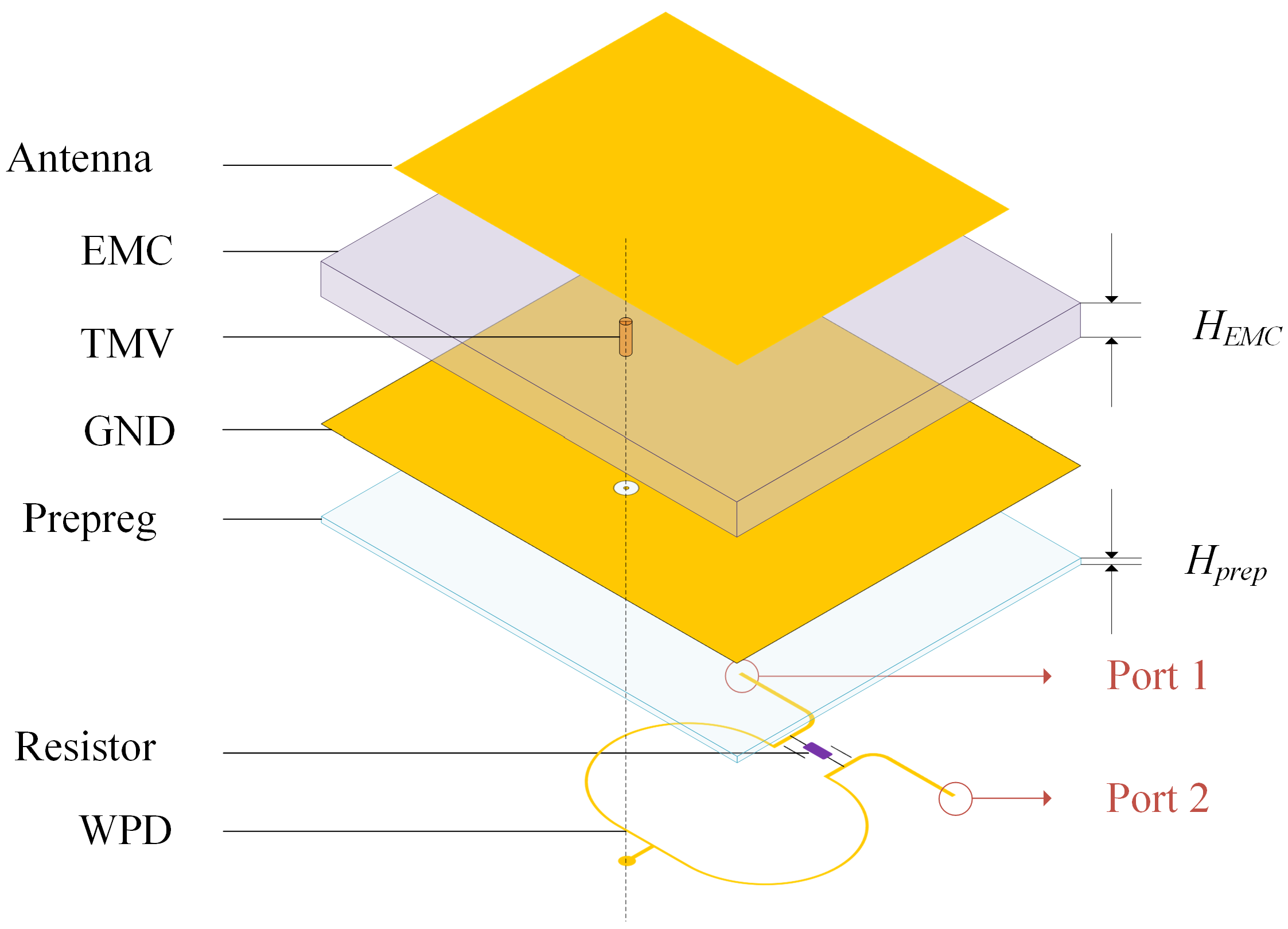
2.1. Structure Design of the Integrated Passive Device
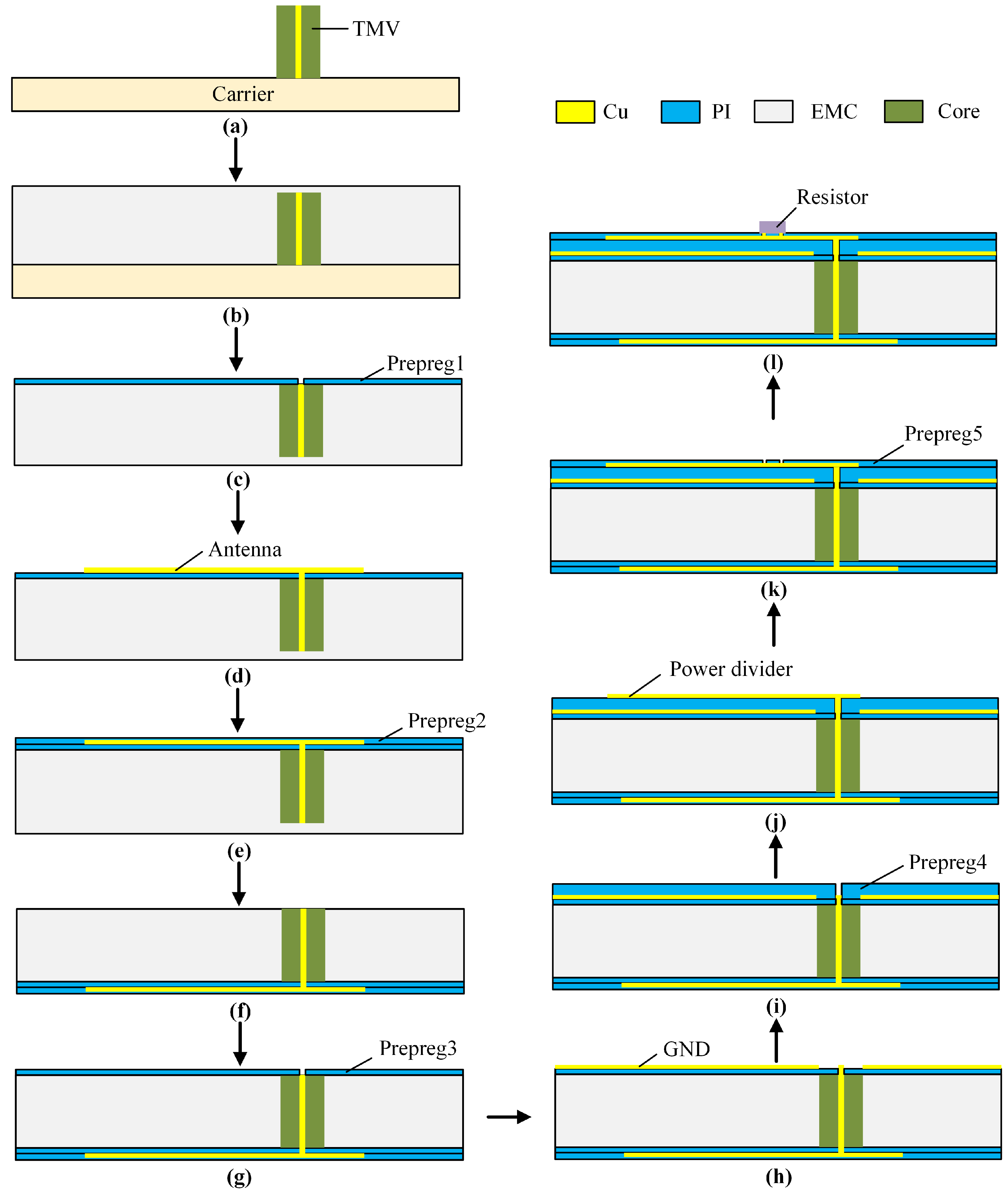
2.2. Process for Fabricating the Integrated Passive Device Using Fan-Out Wafer Level Package Technology
2.3. Empirical Formula-Based Dimension Design of the Integrated Passive Device
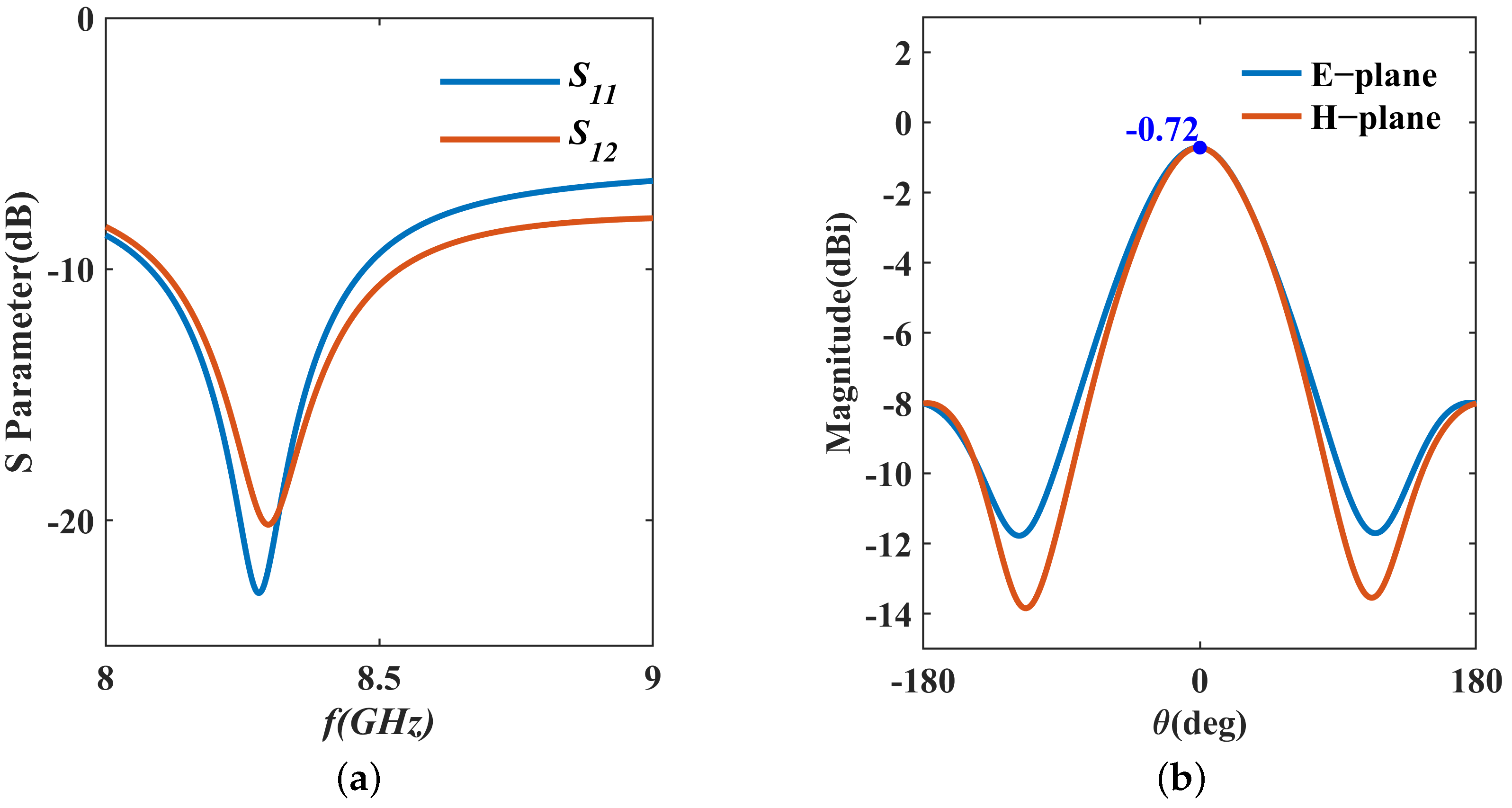
3. Evaluation and Analysis of Simulation Results for the Empirical Formula-Based Design
3.1. Simulation Results Corresponding to the Empirical Formula-Based Design
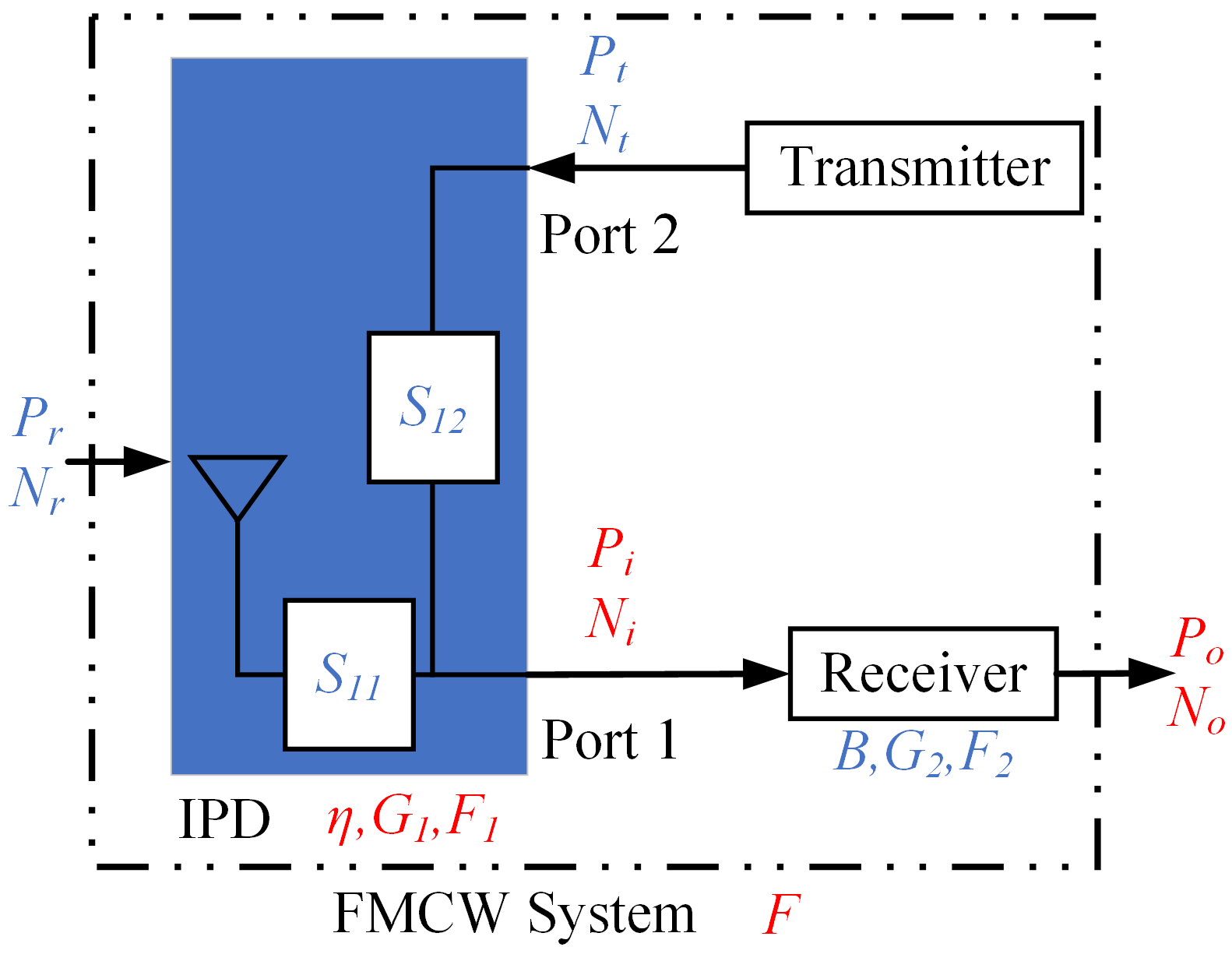
3.2. Modeling the Effect of IPD on the Receiver Noise Figure
4. Optimization for the Integrated Passive Device Structure
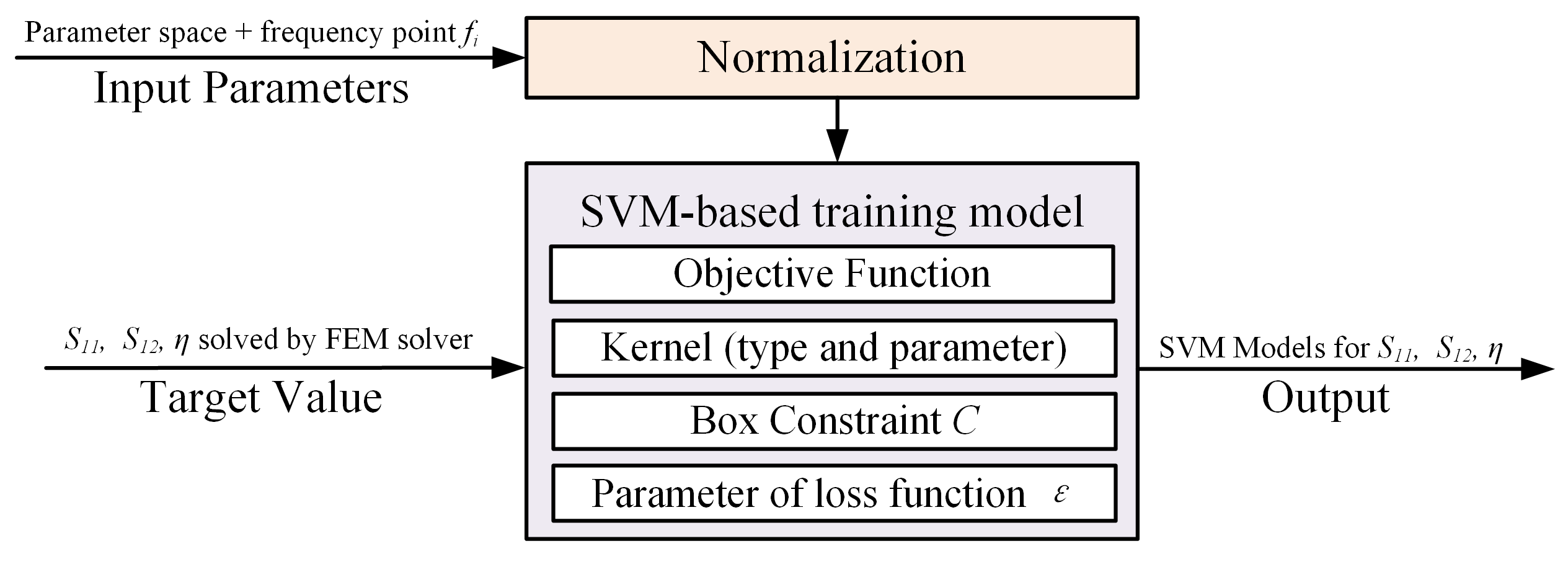
4.1. Optimization Strategy
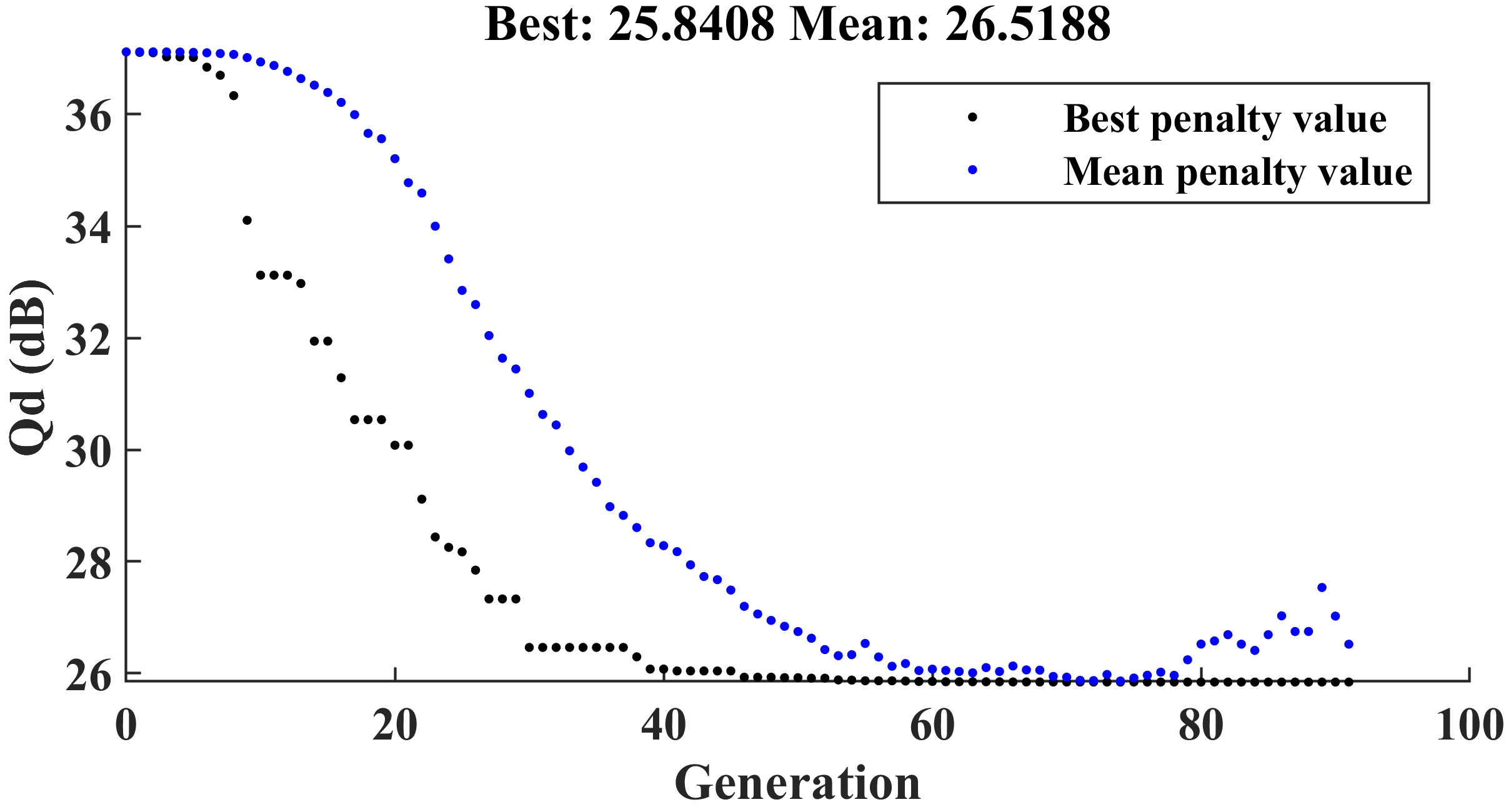
4.2. Process of the Optimization
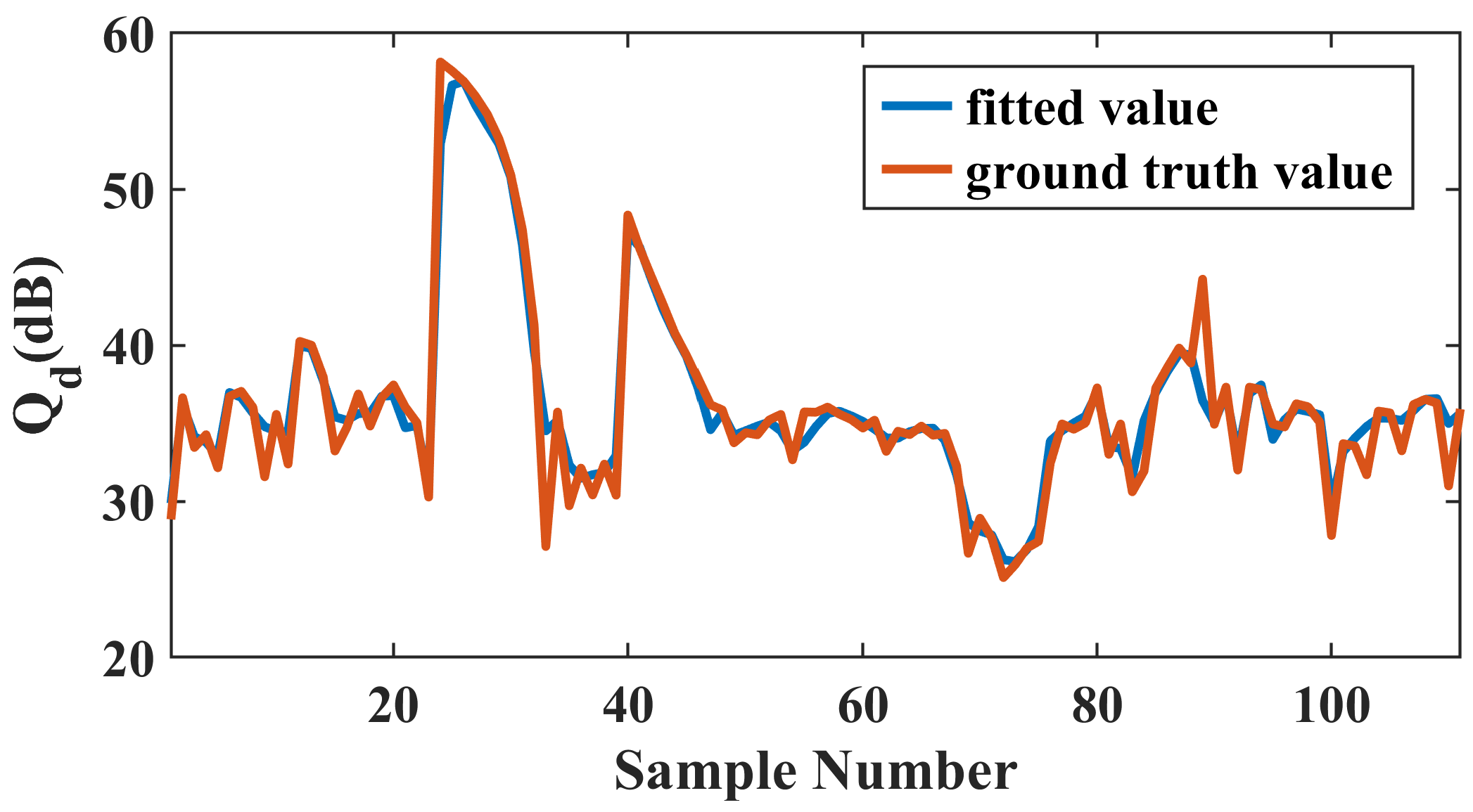
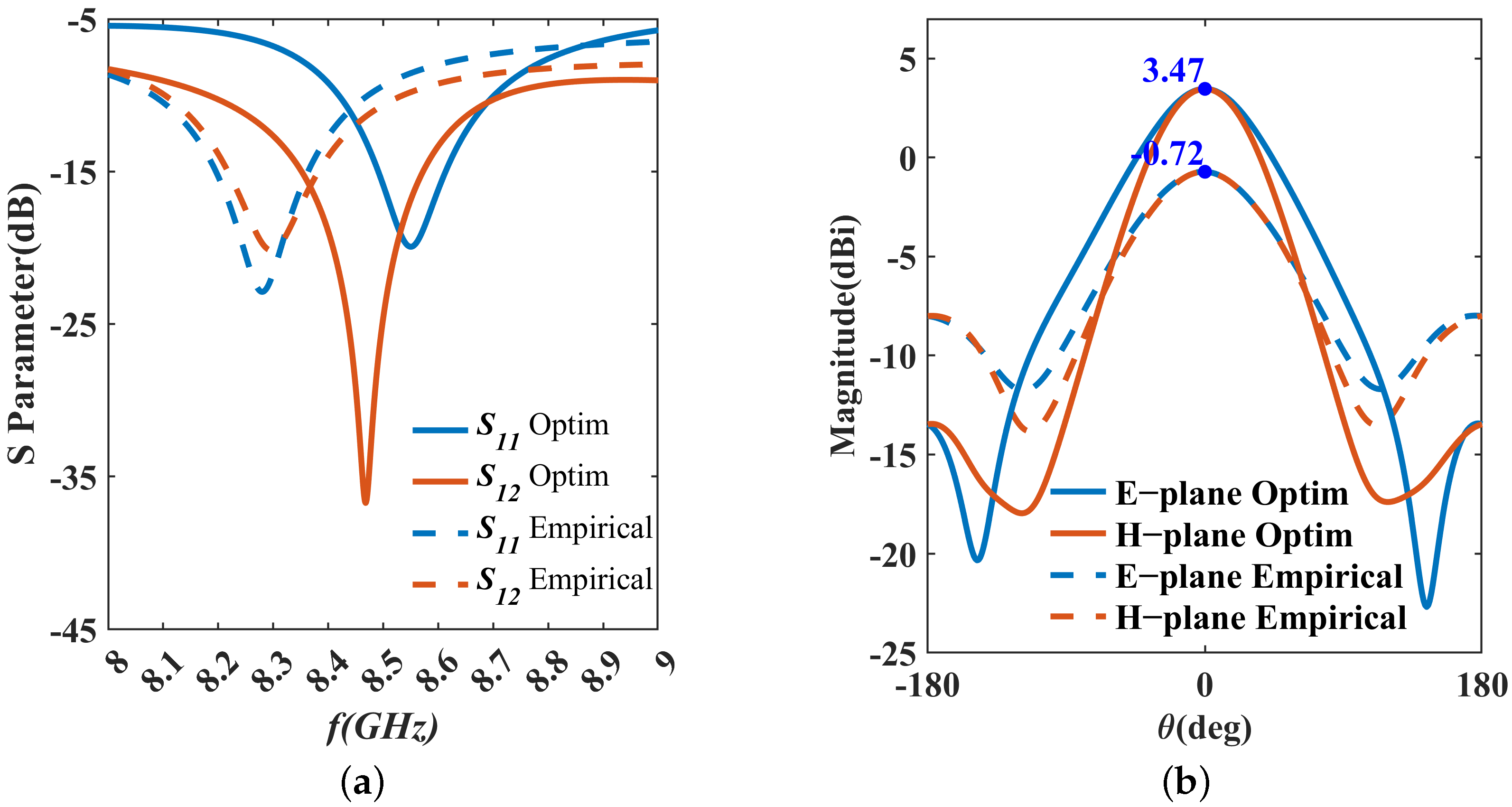
4.3. Analysis and Comparison of the Optimization Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Chou, H.C.; Kao, Y.H.; Peng, C.C.; Wang, Y.J.; Chu, T.S. An X-Band Frequency-Modulated Continuous-Wave Radar Sensor System With a Single-Antenna Interface for Ranging Applications. IEEE Trans. Microw. Theory Tech. 2018, 66, 4216–4231. [Google Scholar] [CrossRef]
- Esposito, C.; Natale, A.; Palmese, G.; Berardino, P.; Lanari, R.; Perna, S. On the Capabilities of the Italian Airborne FMCW AXIS InSAR System. Remote Sens. 2020, 12, 539. [Google Scholar] [CrossRef]
- Lee, J.; Park, M.; Eo, I.; Koo, B. An X-Band FMCW Radar for Detection and Tracking of Miniaturized UAVs. In Proceedings of the 2017 International Conference on Computational Science and Computational Intelligence (CSCI), Las Vegas, NV, USA, 14–16 December 2017; pp. 1844–1845. [Google Scholar] [CrossRef]
- Strohm, K.M.; Bloecher, H.L.; Schneider, R.; Wenger, J. Development of future short range radar technology. In Proceedings of the European Radar Conference, Paris, France, 3–4 October 2005; EURAD: Berlin, Germany, 2005; pp. 165–168. [Google Scholar]
- Li, C.; Peng, Z.; Huang, T.Y.; Fan, T.; Wang, F.K.; Horng, T.S.; Munoz-Ferreras, J.M.; Gomez-Garcia, R.; Ran, L.; Lin, J. A review on recent progress of portable short-range noncontact microwave radar systems. IEEE Trans. Microw. Theory Tech. 2017, 65, 1692–1706. [Google Scholar] [CrossRef]
- Dabi, A.; Rameshbabu, A.; Ghanvat, V.; Pinto, R. Compact Short Range X-Band FMCW Radar. In Proceedings of the 2021 IEEE MTT-S International Microwave and RF Conference (IMARC), Kanpur, India, 17–19 December 2021; pp. 1–4. [Google Scholar] [CrossRef]
- Huang, T.Y.; Shen, T.M.; Wu, R.B. A system-on-package integration of X-band FMCW sensor RF frontend module. In Proceedings of the 2009 Asia Pacific Microwave Conference, Singapore, 7–10 December 2009; pp. 147–150. [Google Scholar] [CrossRef]
- Zhan-gang, L.; Fa-lin, L.; Xing-guo, C. A Design of X-band Vertical Transmission T/R Module. In Proceedings of the 2022 International Conference on Microwave and Millimeter Wave Technology (ICMMT), Harbin, China, 12–15 August 2022; pp. 1–3. [Google Scholar] [CrossRef]
- Zhang, Y.; Mao, J. An overview of the development of antenna-in-package technology for highly integrated wireless devices. Proc. IEEE 2019, 107, 2265–2280. [Google Scholar] [CrossRef]
- Kam, D.G.; Liu, D.; Natarajan, A.; Reynolds, S.; Chen, H.C.; Floyd, B.A. LTCC packages with embedded phased-array antennas for 60 GHz communications. IEEE Microw. Wirel. Compon. Lett. 2011, 21, 142–144. [Google Scholar] [CrossRef]
- Chen, K.; Yu, B.; Wang, Z.; Zhao, B.; Qu, S.; Liu, J.; Pan, G.; Yan, B.; Xu, R.; Xu, Y. Broadband X-Band LTCC Front-End Prototype Integrated with Air-Cavities-Embedded Antenna-in-Package. IEEE Antennas Wirel. Propag. Lett. 2023, 22, 2437–2441. [Google Scholar] [CrossRef]
- Jin, C.; Sekhar, V.N.; Bao, X.; Chen, B.; Zheng, B.; Li, R. Antenna-in-Package Design Based on Wafer-Level Packaging With Through Silicon Via Technology. IEEE Trans. Compon. Packag. Manuf. Technol. 2013, 3, 1498–1505. [Google Scholar] [CrossRef]
- Zhao, Y.; Liang, L.; Hu, Y.; Xiao, Y.; Sun, H. A Ka-Band Silicon-Based Antenna-in-Package Design Using Characteristic Mode Analysis for Bandwidth Enhancement. Electronics 2023, 12, 4983. [Google Scholar] [CrossRef]
- Watanabe, A.O.; Lin, T.H.; Ali, M.; Ogawa, T.; Raj, P.M.; Tentzeris, M.M.; Tummala, R.R.; Swaminathan, M. 3D Glass-Based Panel-Level Package with Antenna and Low-Loss Interconnects for Millimeter-Wave 5G Applications. In Proceedings of the 2019 IEEE MTT-S International Microwave Conference on Hardware and Systems for 5G and Beyond (IMC-5G), Atlanta, GA, USA, 15–16 August 2019; pp. 1–3. [Google Scholar] [CrossRef]
- Galler, T.; Frey, T.; Waldschmidt, C.; Chaloun, T. High-Gain Millimeter-Wave Holographic Antenna in Package Using Glass Technology. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 2067–2071. [Google Scholar] [CrossRef]
- Ho, C.Y.; Hsieh, S.C.; Jhong, M.F.; Pan, P.C.; Wang, C.C.; Ting, C.Y. Millimeter-wave antenna in package on low-cost organic substrate for flip chip chip scale (FCCSP) package. In Proceedings of the 2017 IEEE CPMT Symposium Japan (ICSJ), Kyoto, Japan, 20–22 November 2017; pp. 67–70. [Google Scholar] [CrossRef]
- Yu, B.; Qian, Z.; Lin, C.; Lin, J.; Zhang, Y.; Yang, G.; Luo, Y. A Wideband mmWave Antenna in Fan-Out Wafer Level Packaging with Tall Vertical Interconnects for 5G Wireless Communication. IEEE Trans. Antennas Propag. 2021, 69, 6906–6911. [Google Scholar] [CrossRef]
- Ma, L.; Lai, J.; Yin, Y.; Xia, C.; Gu, C.; Mao, J. A Wideband Co-Linearly Polarized Full-Duplex Antenna-in-Package with High Isolation for Integrated Sensing and Communication. IEEE Antennas Wirel. Propag. Lett. 2023, 22, 2185–2189. [Google Scholar] [CrossRef]
- Yin, Y.; Xia, C.; Liu, S.; Zhang, Z.; Chen, C.; Wang, G.; Wang, C.; Wu, Y. Ultrathin Antenna-in-Package Based on TMV-Embedded FOWLP for 5G mm-Wave Applications. Electronics 2024, 13, 839. [Google Scholar] [CrossRef]
- Wang, C.T.; Tang, T.C.; Lin, C.W.; Hsu, C.W.; Hsieh, J.S.; Tsai, C.H.; Wu, K.C.; Pu, H.P.; Yu, D. InFO AiP Technology for High Performance and Compact 5G Millimeter Wave System Integration. In Proceedings of the 2018 IEEE 68th Electronic Components and Technology Conference (ECTC), San Diego, CA, USA, 29 May–1 June 2018; pp. 202–207. [Google Scholar] [CrossRef]
- Lee, D.; Lee, J.Y.; Lee, K.; Kim, M.; Kim, M.; Youn, Y.; Song, H.J.; Hong, W. Planar Asymmetric Fed Interdigital Coupling Antenna-in-Package Using FOWLP Process Operating at 60–90 GHz in Endfire Mode. IEEE Trans. Microw. Theory Tech. 2024, 72, 2378–2390. [Google Scholar] [CrossRef]
- Chen, Y.; Li, J.; Ding, F.; Cao, L. Antenna in package design and measurement for millimeter-wave applications in fan-out wafer-level package. IEICE Electron. Express 2022, 19, 20220122. [Google Scholar] [CrossRef]
- Boon, S.S.S.; Wee, D.H.S.; Yao, H.H.; Boon, S.L.S.; Shan, S.L.P.; Choong, C.S.; Chong, C.T. Development of Antenna on FO-WLP. In Proceedings of the 2018 IEEE 20th Electronics Packaging Technology Conference (EPTC), Singapore, 4–7 December 2018; pp. 504–508. [Google Scholar] [CrossRef]
- Braun, T.; Le, T.H.; Rossi, M.; Ndip, I.; Hölck, O.; Becker, K.F.; Böttcher, M.; Schiffer, M.; Aschenbrenner, R.; Müller, F.; et al. Development of a Scalable AiP Module for mmWave 5G MIMO Applications Based on a Double Molded FOWLP Approach. In Proceedings of the 2021 IEEE 71st Electronic Components and Technology Conference (ECTC), San Diego, CA, USA, 1 June–4 July 2021; pp. 2009–2015. [Google Scholar] [CrossRef]
- Wu, Z.; Zheng, S.; Pan, Y. A Rapid Optimization Scheme for the Simultaneous Optimization of S-Parameters and Radiation Patterns. IEEE Trans. Antennas Propag. 2023, 71, 9394–9404. [Google Scholar] [CrossRef]
- Brooker, G. Understanding millimetre wave FMCW radars. In Proceedings of the 1st International Conference on Sensing Technology, Palmerston North, New Zealand, 21–23 November 2005. [Google Scholar]
- Adela, B.B.; van Beurden, M.C.; Van Zeijl, P.; Smolders, A.B. High-Isolation Array Antenna Integration for Single-Chip Millimeter-Wave FMCW Radar. IEEE Trans. Antennas Propag. 2018, 66, 5214–5223. [Google Scholar] [CrossRef]
- Doerry, A.W. Noise and Noise Figure for Radar Receivers; Technical report; Sandia National Lab.(SNL-NM): Albuquerque, NM, USA, 2016. [Google Scholar]
- Best, S.R. Realized Noise Figure of the General Receiving Antenna. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 702–705. [Google Scholar] [CrossRef]
- Pozar, D.M. Microstrip Antennas; Artech House: Norwood, MA, USA, 1980. [Google Scholar]
- David, M.P. Microwave Engineering, 4th ed.; Publishing House of Electronics Industry: Beijing, China, 2019. [Google Scholar]
- Friis, H. Noise Figures of Radio Receivers. Proc. Ire 1944, 32, 419–422. [Google Scholar] [CrossRef]
- Koziel, S.; Ogurtsov, S. Simulation-Driven Design in Microwave Engineering: Methods. In Computational Optimization, Methods and Algorithms; Koziel, S., Yang, X.S., Eds.; Springer: Berlin/Heidelberg, Germany, 2011; pp. 153–178. [Google Scholar] [CrossRef]
- Zheng, Z.; Chen, X.; Huang, K. Application of support vector machines to the antenna design. Int. J. Microw.-Comput.-Aided Eng. 2011, 21, 85–90. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, H.C.; Cheng, Q.S. Surrogate-Assisted Quasi–Newton Enhanced Global Optimization of Antennas Based on a Heuristic Hypersphere Sampling. IEEE Trans. Antennas Propag. 2021, 69, 2993–2998. [Google Scholar] [CrossRef]
- Chauhan, N.; Mittal, A.; Kartikeyan, M.V. Support vector driven genetic algorithm for the design of circular polarized microstrip antenna. Int. J. Infrared Millim. Waves 2008, 29, 558–569. [Google Scholar] [CrossRef]




| Characters | Descriptions | Values |
|---|---|---|
| permittivity of EMC | 3.69 | |
| thickness of EMC substrate | 400 µm | |
| permittivity of prepreg | 3.5 | |
| thickness of the prepreg layer used as the substrate for the power divider | 30 µm | |
| thickness of the other prepreg layers | 10 µm | |
| diameter of the through mold via | 120 µm |
| Parameters | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Values (Units) | 297 µm | 13.9 mm | 11.5 mm | 11.5 mm | 9.1 mm | 2.5 mm | 68 µm | 37 µm | 5.4 mm | 100 | 0 mm |
| Characters | Descriptions |
|---|---|
| Echo signal power received by the antenna from space. | |
| Noise power received by the antenna from space. | |
| Signal power output from the transmitter. | |
| Noise power output from the transmitter. | |
| Voltage reflection coefficient at port 1 of IPD (connected to the receiver). | |
| Voltage transmission coefficient from port 2 to port 1 of IPD. | |
| Efficiency of IPD. | |
| Gain of IPD. | |
| Noise factor of IPD. | |
| Output signal power of IPD (also input signal power of the receiver). | |
| Output noise power of IPD (also input noise power of the receiver). | |
| Output signal power of the receiver. | |
| Output noise power of the receiver. | |
| B | Bandwidth of the received signal. |
| Gain of the receiver. | |
| Noise factor of the receiver. | |
| F | Noise factor of the FMCW system. |
| Parameters | Units | Upper Boundary | Lower Boundary | Resolution | Fabrication Accuracy | Fixed Values |
|---|---|---|---|---|---|---|
| µm | 400 | 200 | 25 | 1 | 284 | |
| mm | 35 | 20 | 2.5 | 0.01 | 31.7 | |
| mm | 20 | 10 | 1 | 0.01 | 15.7 | |
| mm | 35 | 20 | 2.5 | 0.01 | 24.75 | |
| mm | 9 | 7 | 0.2 | 0.01 | 8.85 | |
| mm | 3 | 1 | 0.2 | 0.001 | 2.41 | |
| µm | 100 | 40 | 5 | 1 | 66 | |
| µm | 50 | 20 | 5 | 1 | 36 | |
| mm | 3.74 | 6.88 | 0.314 | 0.001 | 1.51 | |
| 150 | 50 | 10 | 1 | 61 | ||
| mm | 1.2 | 0 | 0.1 | 0.01 | 0.17 |
| Model | Kernel Type | RBF Kernel Parameter | Box Constraint C | Loss Funciton Parameter | Objective Function |
|---|---|---|---|---|---|
| RBF | 0.53 | 74.17 | 7.42 | MSE | |
| RBF | 0.42 | 86.44 | 8.64 | MSE | |
| RBF | 0.53 | 26.78 | 2.68 | MSE |
| Parameters | (dB) | |||
|---|---|---|---|---|
| Mean squared error | 0.0008 | 0.0007 | 0.0004 | 2.4176 |
| Parameters | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Values | 288 µm | 31.17 mm | 15.21 mm | 23.69 mm | 8.82 mm | 2.472 mm | 66 µm | 39 µm | 5.432 mm | 62 | 0.14 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, J.; Xu, L.; Yang, K. Design and Optimization of a Fan-Out Wafer-Level Packaging- Based Integrated Passive Device Structure for FMCW Radar Applications. Micromachines 2024, 15, 1311. https://doi.org/10.3390/mi15111311
Yang J, Xu L, Yang K. Design and Optimization of a Fan-Out Wafer-Level Packaging- Based Integrated Passive Device Structure for FMCW Radar Applications. Micromachines. 2024; 15(11):1311. https://doi.org/10.3390/mi15111311
Chicago/Turabian StyleYang, Jiajie, Lixin Xu, and Ke Yang. 2024. "Design and Optimization of a Fan-Out Wafer-Level Packaging- Based Integrated Passive Device Structure for FMCW Radar Applications" Micromachines 15, no. 11: 1311. https://doi.org/10.3390/mi15111311
APA StyleYang, J., Xu, L., & Yang, K. (2024). Design and Optimization of a Fan-Out Wafer-Level Packaging- Based Integrated Passive Device Structure for FMCW Radar Applications. Micromachines, 15(11), 1311. https://doi.org/10.3390/mi15111311






