Dynamic Characteristic Model of Giant Magnetostrictive Transducer with Double Terfenol-D Rods
Abstract
1. Introduction
2. Dynamic Characteristic Model of Giant Magnetostrictive Transducer Considering Magneto-Mechanical Coupling Effect
2.1. Finite Element Model for Giant Magnetostrictive Transducer
2.2. The Structure and Materials of Giant Magnetostrictive Transducer
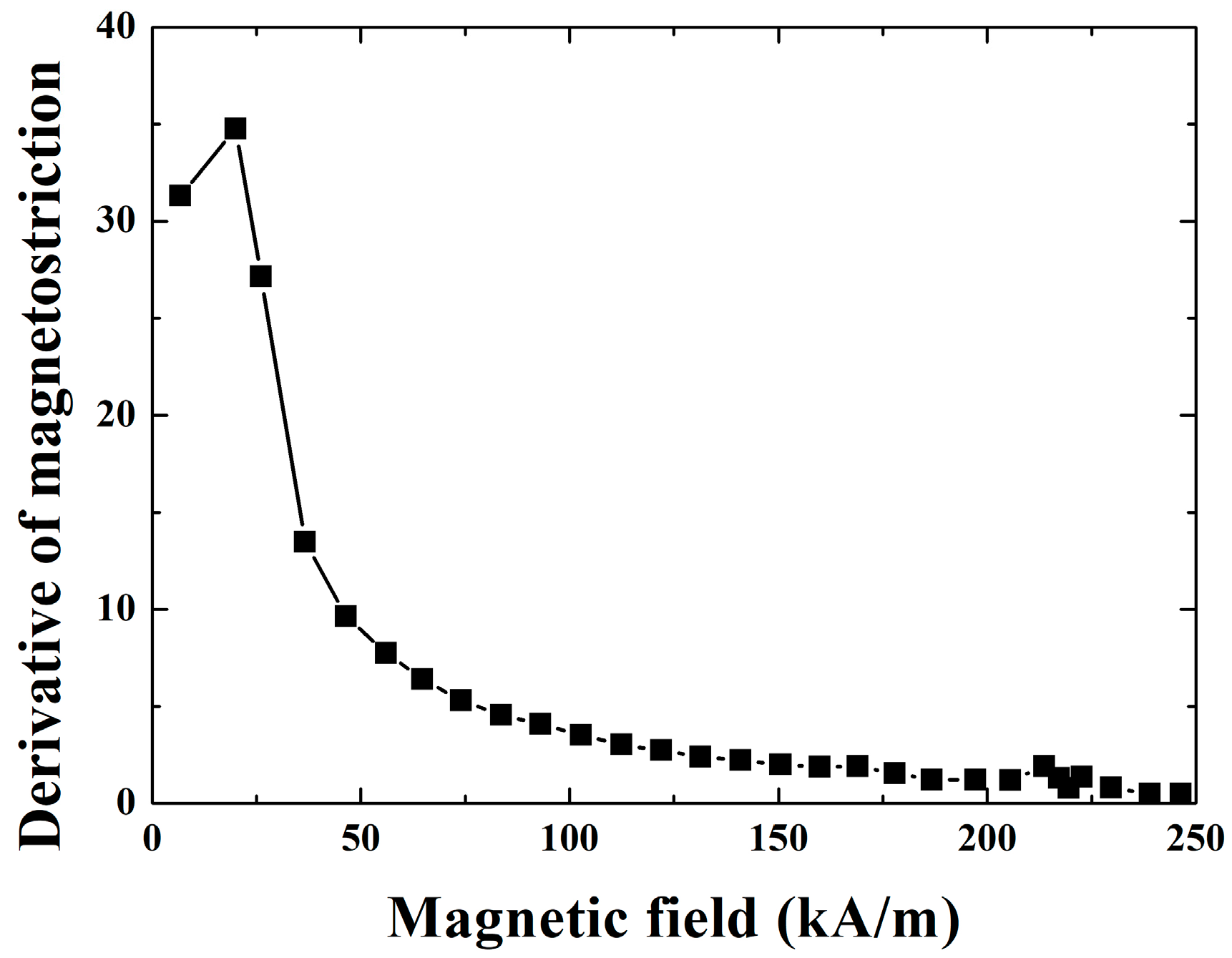
2.3. Finite Element Calculation of the Dynamic Characteristic Model
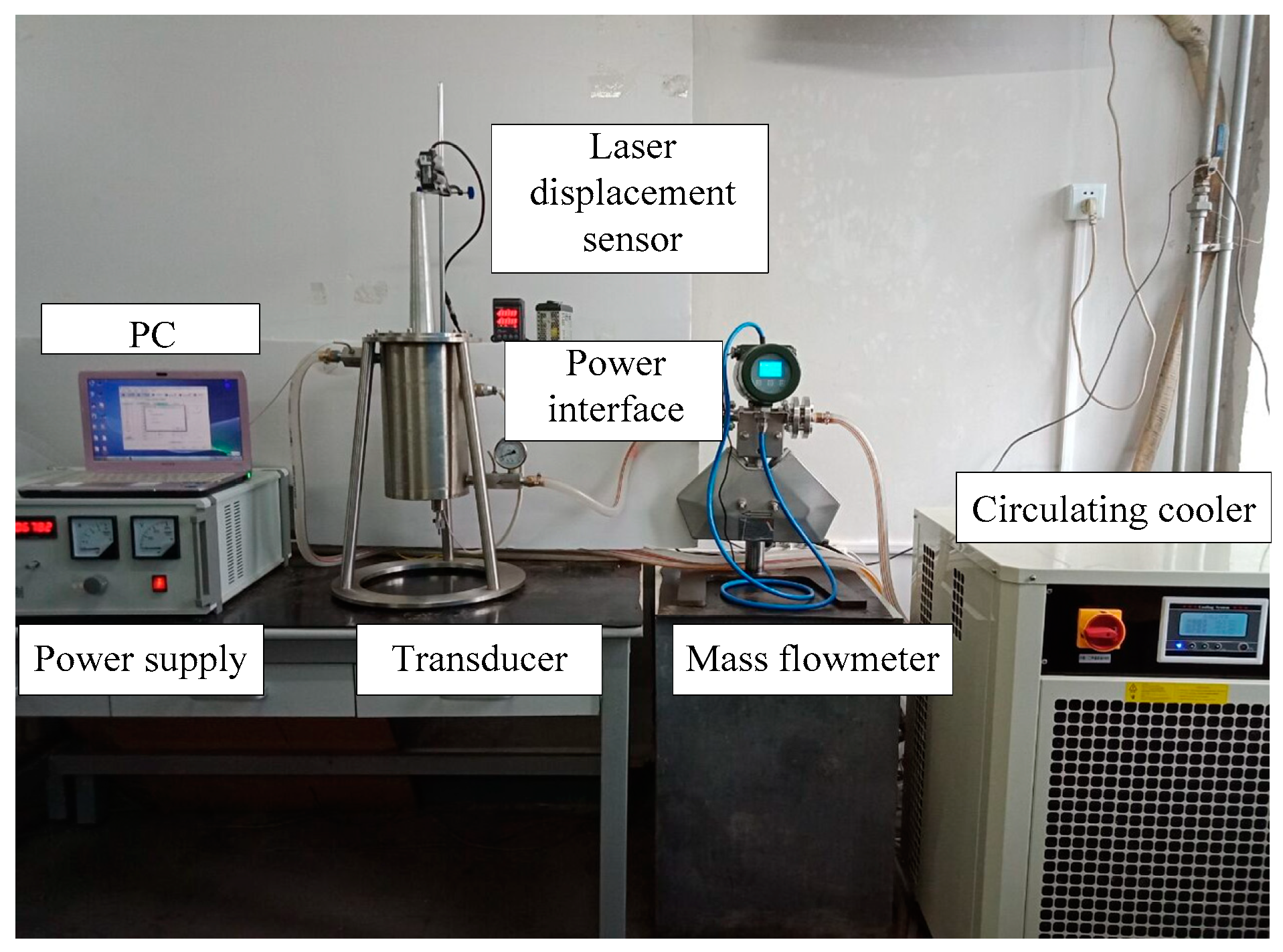
3. The Prototype of Giant Magnetostrictive Transducer and Experimental System
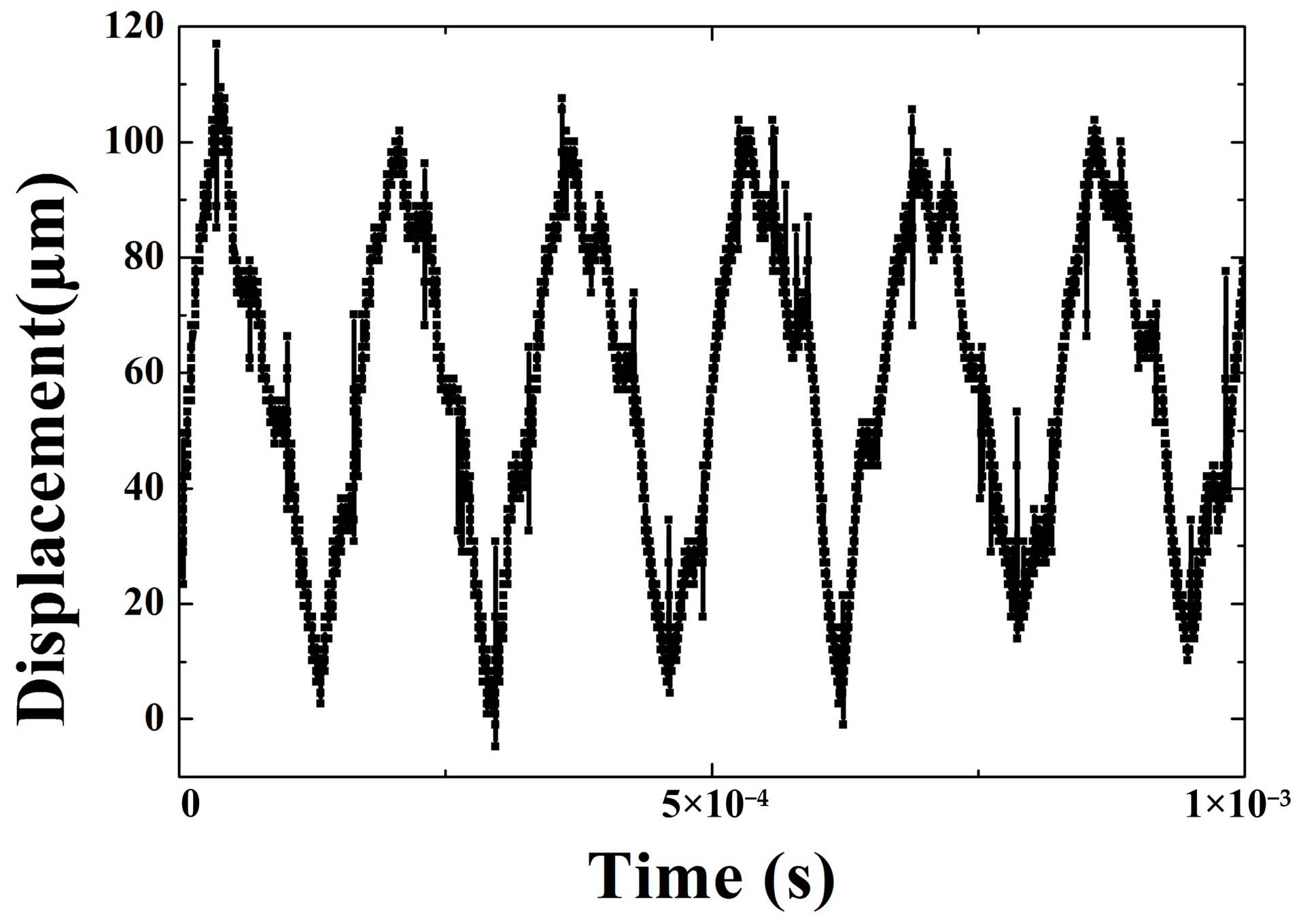
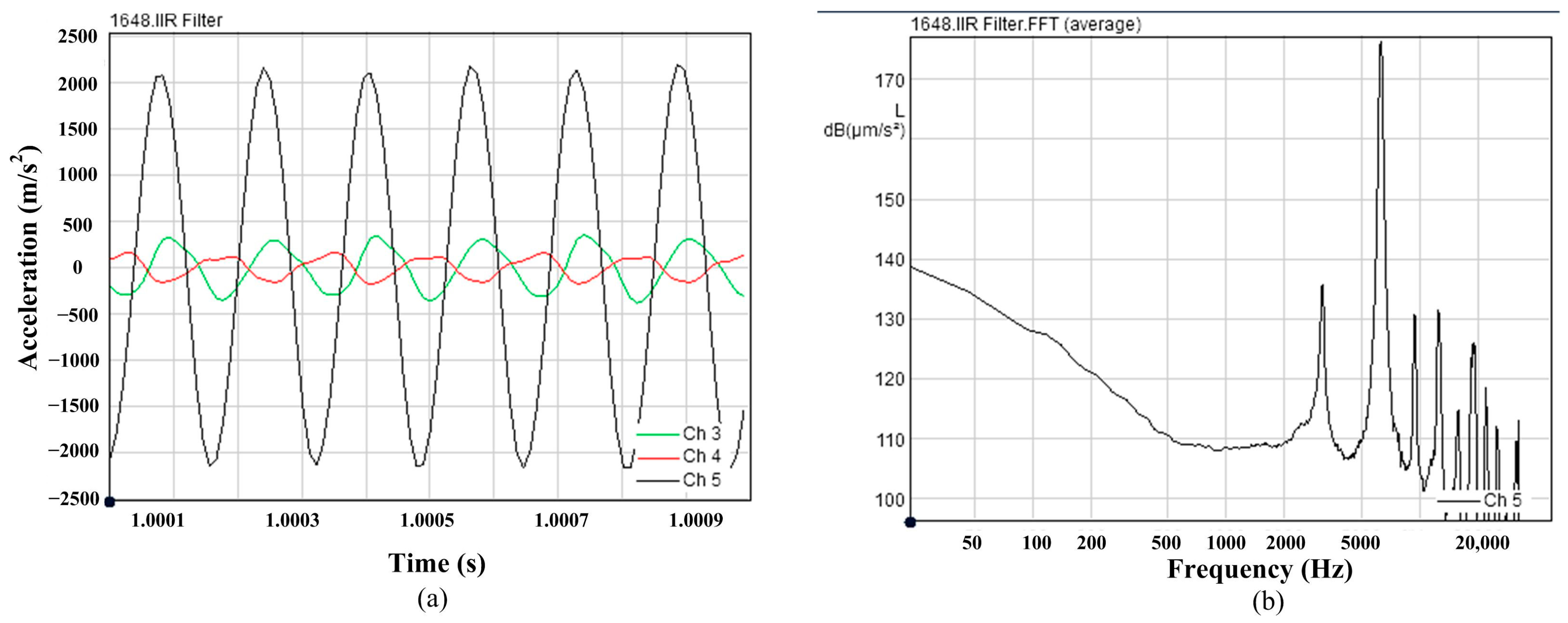
4. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, A.; Goto, K.; Kobayashi, Y.; Hara, Y.; Jia, Y.; Shi, Y.; Soutis, C.; Kurita, H.; Narita, F.; Otsuka, K.; et al. Energy harvesting using a magnetostrictive transducer based on switching control. Sensor Actuat. A Phys. 2023, 355, 114303. [Google Scholar] [CrossRef]
- Zhou, Z.Q.; He, Z.B.; Xue, G.M.; Zhou, J.T.; Rong, C.; Liu, G.P. Structure design and working characteristics analysis of direct-drive giant magnetostrictive injector. Micromachines 2022, 13, 1721. [Google Scholar] [CrossRef]
- Liu, X.H.; Gao, L.; Wu, Y.; Song, H.R.; He, Y. Micro-displacement amplifier of giant magnetostrictive actuator using flexure hinges. J. Magn. Magn. Mater. 2022, 556, 169415. [Google Scholar] [CrossRef]
- Zhao, N.T.; Gao, B.; Yang, W.H.; Li, H.S. Design of underwater magnetostrictive longitudinal-type transducers with circuit model considering eddy currents and multi-rod structure. Appl. Acoust. 2023, 202, 109112. [Google Scholar] [CrossRef]
- Xiao, Y.; Gou, X.F.; Zhang, D.G. A one-dimension nonlinear hysteretic constitutive model with elasto-thermo-magnetic coupling for giant magnetostrictive materials. J. Magn. Magn. Mater. 2017, 441, 642–649. [Google Scholar] [CrossRef]
- Zheng, X.J.; Sun, L.; Jin, K. A dynamic hysteresis constitutive relation for giant magnetostrictive materials. Mech. Adv. Mater. Struct. 2009, 16, 516–521. [Google Scholar] [CrossRef]
- Talebian, S.; Hojjat, Y.; Ghodsi, M.; Karafi, M.R. Study on classical and excess eddy currents losses of Terfenol-D. J. Magn. Magn. Mater. 2015, 388, 150–159. [Google Scholar] [CrossRef]
- Talebian, S.; Hojjat, Y.; Ghodsi, M.; Karafi, M.R.; Mirzamohammadi, S. A combined Preisach-Hyperbolic Tangent model for magnetic hysteresis of Terfenol-D. J. Magn. Magn. Mater. 2015, 396, 38–47. [Google Scholar] [CrossRef]
- Braghin, F.; Cinquemani, S.; Resta, F. A model of magnetostrictive actuators for active vibration control. Sens. Actuat. A Phys. 2011, 165, 342–350. [Google Scholar] [CrossRef]
- Zhang, T.; Yang, B.T.; Li, H.G.; Meng, G. Dynamic modeling and adaptive vibration control study for giant magnetostrictive actuators. Sens. Actuat. A Phys. 2013, 190, 96–105. [Google Scholar] [CrossRef]
- Xue, G.M.; He, Z.B.; Li, D.W.; Yang, Z.S.; Zhao, Z.L. Analysis of the giant magnetostrictive actuator with strong bias magnetic field. J. Magn. Magn. Mater. 2015, 394, 416–421. [Google Scholar] [CrossRef]
- Scheidler, J.J.; Asnani, V.M. Validated linear dynamic model of electrically-shunted magnetostrictive transducers with application. Smart Mater. Struct. 2017, 26, 035057. [Google Scholar] [CrossRef]
- Huang, W.M.; Song, G.Y.; Sun, Y. Numerical dynamic strong coupled model of linear magnetostrictive actuators. IEEE T. Magn. 2012, 48, 391–394. [Google Scholar]
- Chakrabarti, S.; Dapino, M.J. Coupled axisymmetric finite element model of a hydraulically amplified magnetostrictive actuator for active powertrain mounts. Finite Elem. Anal. Des. 2012, 60, 25–34. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, X.Y.; Gu, G.Y.; Chen, X.K.; Su, C.Y. A comprehensive dynamic model for magnetostrictive actuators considering different input frequencies with mechanical loads. IEEE T. Ind. Inform. 2016, 12, 980–990. [Google Scholar] [CrossRef]
- Wang, W.; Thomas, P. Low-frequency active noise control of an underwater large-scale structure with distributed giant magnetostrictive actuators. Sens. Actuat. A Phys. 2017, 263, 113–121. [Google Scholar] [CrossRef]
- Ma, K.; Wang, J.J.; Zhang, J.F.; Feng, P.F.; Yu, D.W.; Ahmad, S. A highly temperature-stable and complete-resonance rotary giant magnetostrictive ultrasonic system. Int. J. Mech. Sci. 2022, 214, 106927. [Google Scholar] [CrossRef]
- Fang, S.X.; Zhang, Q.J.; Zhao, H.L.; Yu, J.Z.; Chu, Y.C. The design of rare-earth giant magnetostrictive ultrasonic transducer and experimental study on its application of ultrasonic surface strengthening. Micromachines 2018, 9, 98. [Google Scholar] [CrossRef]
- Zhou, H.L.; Zhang, J.F.; Feng, P.F.; Yu, D.W.; Wu, Z.J. Investigations on a mathematical model for optimum impedance compensation of a giant magnetostrictive ultrasonic transducer and its resonance characteristics. Ultrasonics 2021, 110, 106286. [Google Scholar] [CrossRef]
- Li, Y.F.; Huang, W.M.; Wang, B.W. Design and output characteristics of ultrasonic transducer based on rare-earth giant magnetostrictive material. IEEE Trans. Magn. 2022, 58, 4000406. [Google Scholar] [CrossRef]
- Li, Y.F.; Dong, X.; Yu, X.D. Magnetic energy losses and temperature control system for giant magnetostrictive transducer. Micromachines 2023, 14, 177. [Google Scholar] [CrossRef] [PubMed]
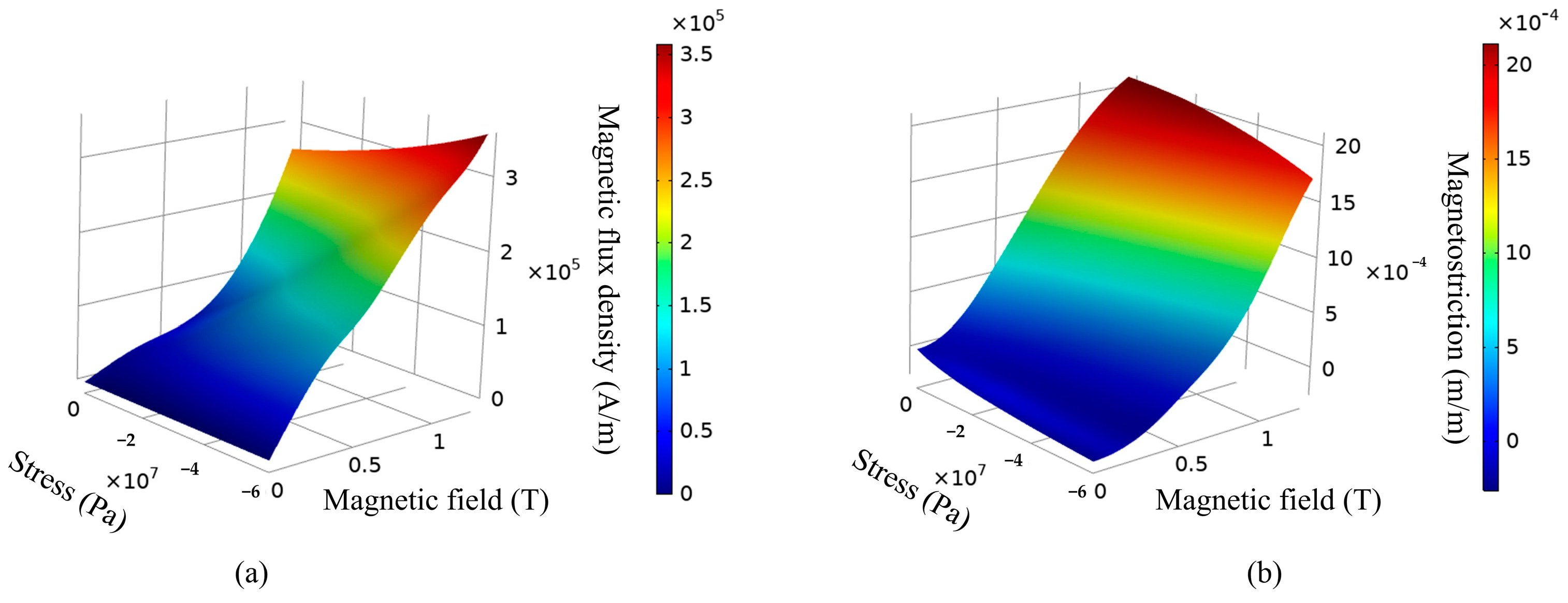
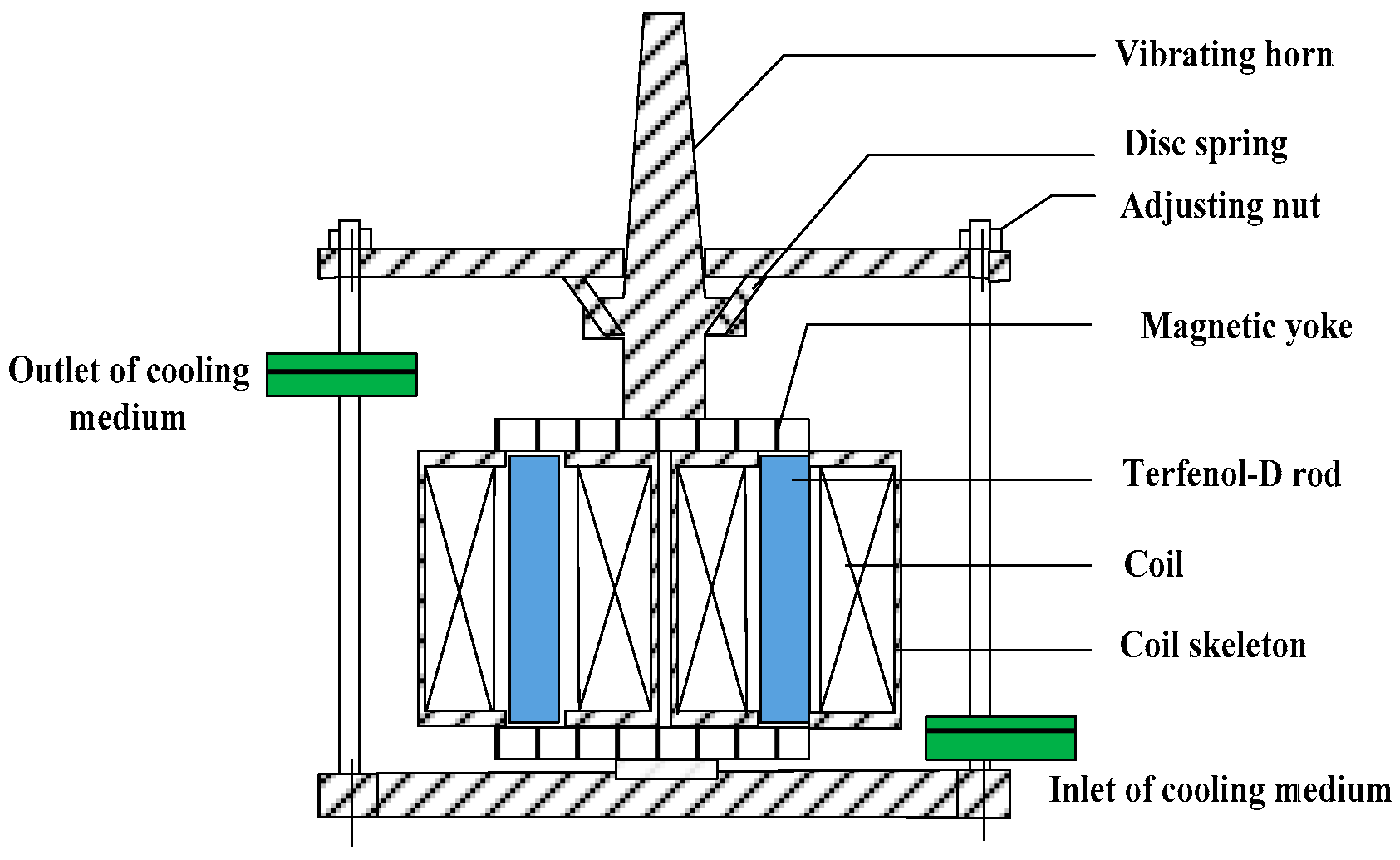
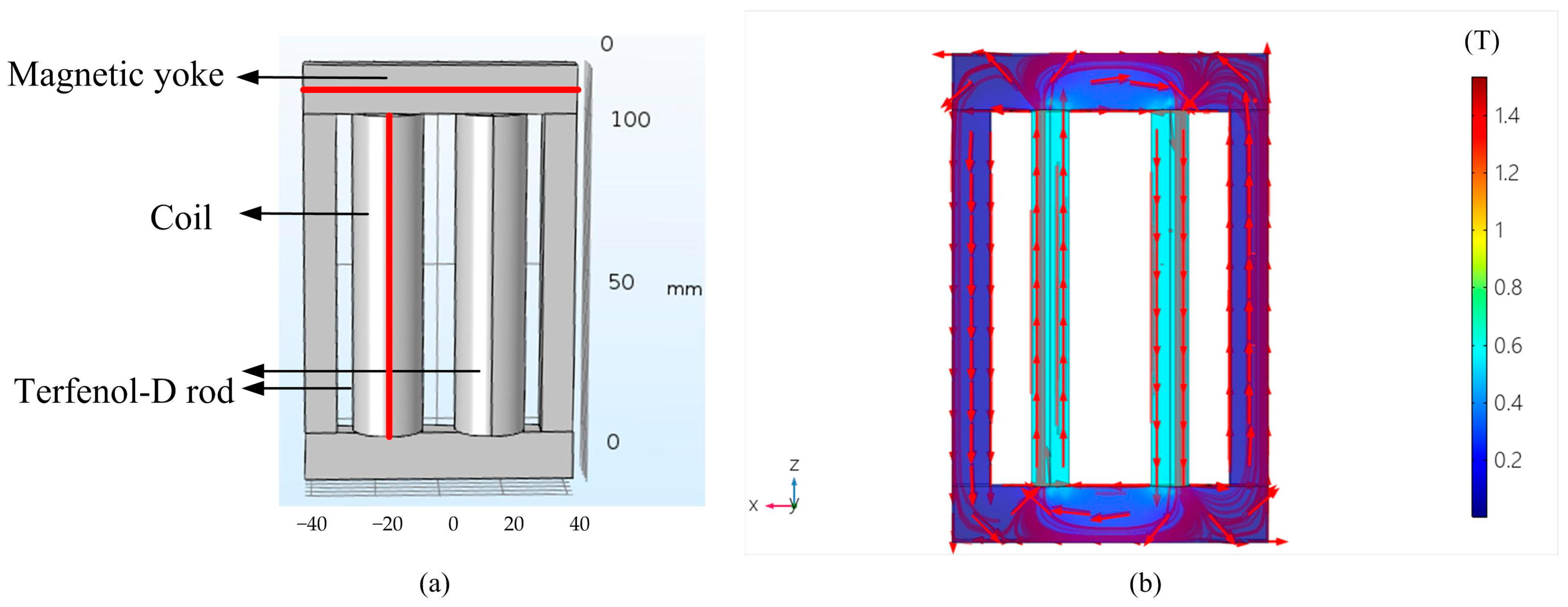

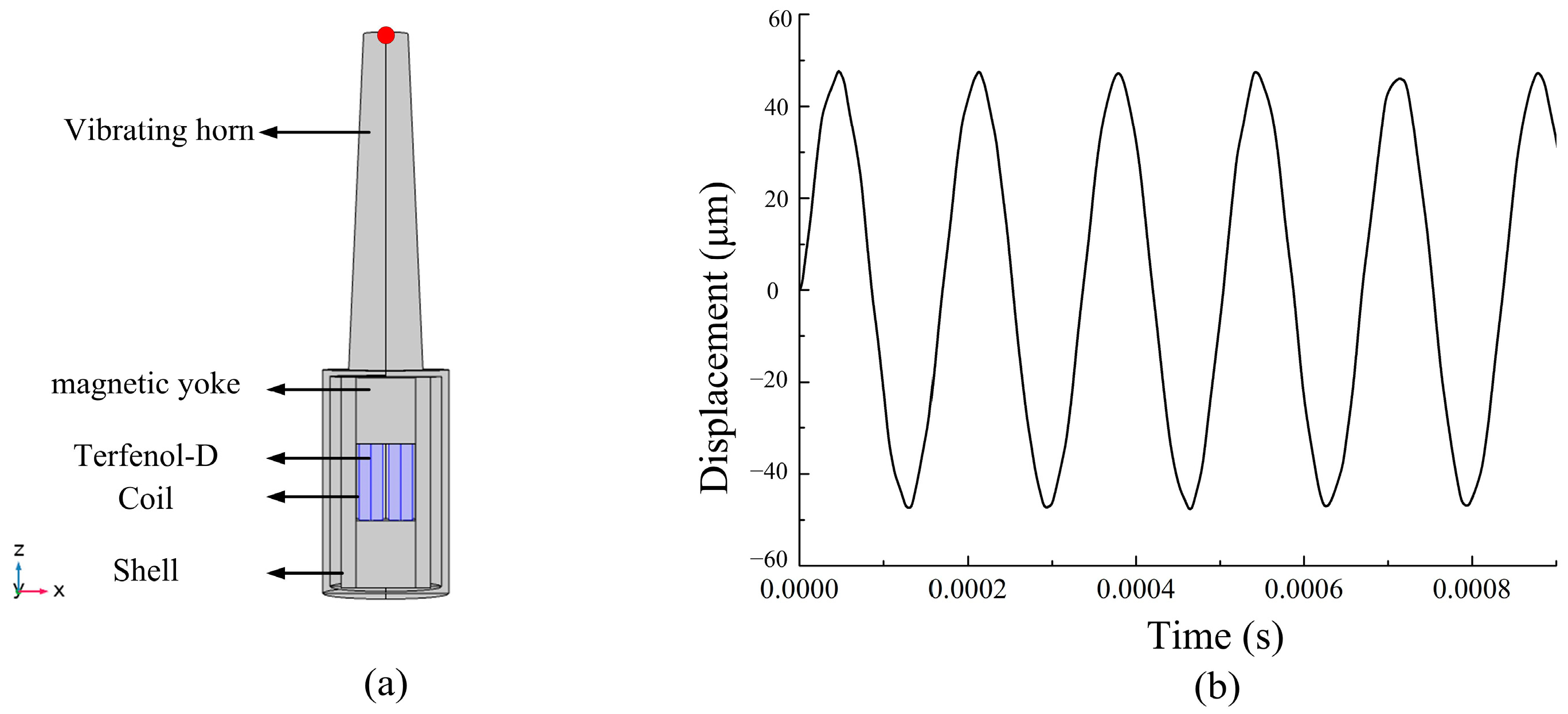



| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Young’s modulus | 3 × 1010 Pa | Relative permeability | 9.2 |
| Poisson’s ratio | 0.45 | Density | 9250 kg/m3 |
| Heat Expansion Coefficient | 12 × 10−6 °C−1 | Curie temperature | 380 °C |
| Relative dielectric constant | 1 | Conductivity | 1.7 × 106 S/m |
| Resistivity | 6 × 10−7 Ω·m | Specific heat capacity | 0.35 kJ/(kg·K) |
| Saturation magnetostriction | 1555 ppm | Saturation magnetization | 6.65 × 105 A/m |
| Heat conductivity | 13.5 W/(m·K) |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Terfenol-D rod | 2 rods | Coil turns | 150 turns |
| Terfenol-D rod radius | 7.5 mm | Coil bobbin length | 120 mm |
| Terfenol-D rod length | 102 mm | Coil outward radius | 14 mm |
| Horn top diameter | 28 mm | Horn length | 310 mm |
| Horn bottom diameter | 67 mm | Shell radius | 76 mm |
| Magnetic yoke length | 60 mm | Magnetic yoke width | 16 mm |
| Frequency | Current | Voltage | Temperature | Temperature Difference | Amplitude |
|---|---|---|---|---|---|
| 5.6 kHz | 1 A | 150 V | 21.1 °C | 1.1 °C | 17 μm |
| 5.6 kHz | 2 A | 240 V | 21.6 °C | 1.6 °C | 29 μm |
| 5.8 kHz | 1 A | 143 V | 21.3 °C | 1.3 °C | 18 μm |
| 5.8 kHz | 2 A | 220 V | 21.7 °C | 1.7 °C | 31 μm |
| 6 kHz | 1 A | 130 V | 21.5 °C | 1.5 °C | 21 μm |
| 6 kHz | 2 A | 200 V | 21.9 °C | 1.9 °C | 35 μm |
| 6.2 kHz | 1 A | 115 V | 22.0 °C | 2.0 °C | 28 μm |
| 6.2 kHz | 2 A | 190 V | 22.2 °C | 2.2 °C | 41 μm |
| 6.4 kHz | 1 A | 105 V | 22.4 °C | 2.4 °C | 33 μm |
| 6.4 kHz | 2 A | 175 V | 22.6 °C | 2.6 °C | 49 μm |
| 6.6 kHz | 1 A | 95 V | 22.6 °C | 2.6 °C | 26 μm |
| 6.6 kHz | 2 A | 155 V | 22.8 °C | 2.8 °C | 40 μm |
| 6.8 kHz | 1 A | 85 V | 22.8 °C | 2.8 °C | 18 μm |
| 6.8 kHz | 2 A | 140 V | 22.9 °C | 2.9 °C | 32 μm |
| 7 kHz | 1 A | 77 V | 22.9 °C | 2.9 °C | 15 μm |
| 7 kHz | 2 A | 125 V | 23.0 °C | 3.0 °C | 26 μm |
| Frequency | Current | Acceleration | Frequency | Current | Acceleration |
|---|---|---|---|---|---|
| 5.6 kHz | 1 A | 570 m/s2 | 5.6 kHz | 2 A | 1260 m/s2 |
| 5.8 kHz | 1 A | 650 m/s2 | 5.8 kHz | 2 A | 1428 m/s2 |
| 6 kHz | 1 A | 778 m/s2 | 6 kHz | 2 A | 1581 m/s2 |
| 6.2 kHz | 1 A | 820 m/s2 | 6.2 kHz | 2 A | 1700 m/s2 |
| 6.4 kHz | 1 A | 925 m/s2 | 6.4 kHz | 2 A | 1943 m/s2 |
| 6.6 kHz | 1 A | 860 m/s2 | 6.6 kHz | 2 A | 1680 m/s2 |
| 6.8 kHz | 1 A | 790 m/s2 | 6.8 kHz | 2 A | 1400 m/s2 |
| 7 kHz | 1 A | 600 m/s2 | 7 kHz | 2 A | 1100 m/s2 |
| Frequency | Current | Force | Frequency | Current | Force |
|---|---|---|---|---|---|
| 5.6 kHz | 1 A | 4 N | 5.6 kHz | 2 A | 10 N |
| 5.8 kHz | 1 A | 5 N | 5.8 kHz | 2 A | 11 N |
| 6 kHz | 1 A | 6 N | 6 kHz | 2 A | 14 N |
| 6.2 kHz | 1 A | 8 N | 6.2 kHz | 2 A | 16 N |
| 6.4 kHz | 1 A | 10 N | 6.4 kHz | 2 A | 20 N |
| 6.6 kHz | 1 A | 7 N | 6.6 kHz | 2 A | 11 N |
| 6.8 kHz | 1 A | 6 N | 6.8 kHz | 2 A | 10 N |
| 7 kHz | 1 A | 5 N | 7 kHz | 2 A | 8 N |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Dong, X.; Yu, X. Dynamic Characteristic Model of Giant Magnetostrictive Transducer with Double Terfenol-D Rods. Micromachines 2023, 14, 1103. https://doi.org/10.3390/mi14061103
Li Y, Dong X, Yu X. Dynamic Characteristic Model of Giant Magnetostrictive Transducer with Double Terfenol-D Rods. Micromachines. 2023; 14(6):1103. https://doi.org/10.3390/mi14061103
Chicago/Turabian StyleLi, Yafang, Xia Dong, and Xiaodong Yu. 2023. "Dynamic Characteristic Model of Giant Magnetostrictive Transducer with Double Terfenol-D Rods" Micromachines 14, no. 6: 1103. https://doi.org/10.3390/mi14061103
APA StyleLi, Y., Dong, X., & Yu, X. (2023). Dynamic Characteristic Model of Giant Magnetostrictive Transducer with Double Terfenol-D Rods. Micromachines, 14(6), 1103. https://doi.org/10.3390/mi14061103





