Push–Pull Inverter Using Amplitude Control and Frequency Tracking for Piezoelectric Transducers
Abstract
:1. Introduction
2. Theoretical Analysis
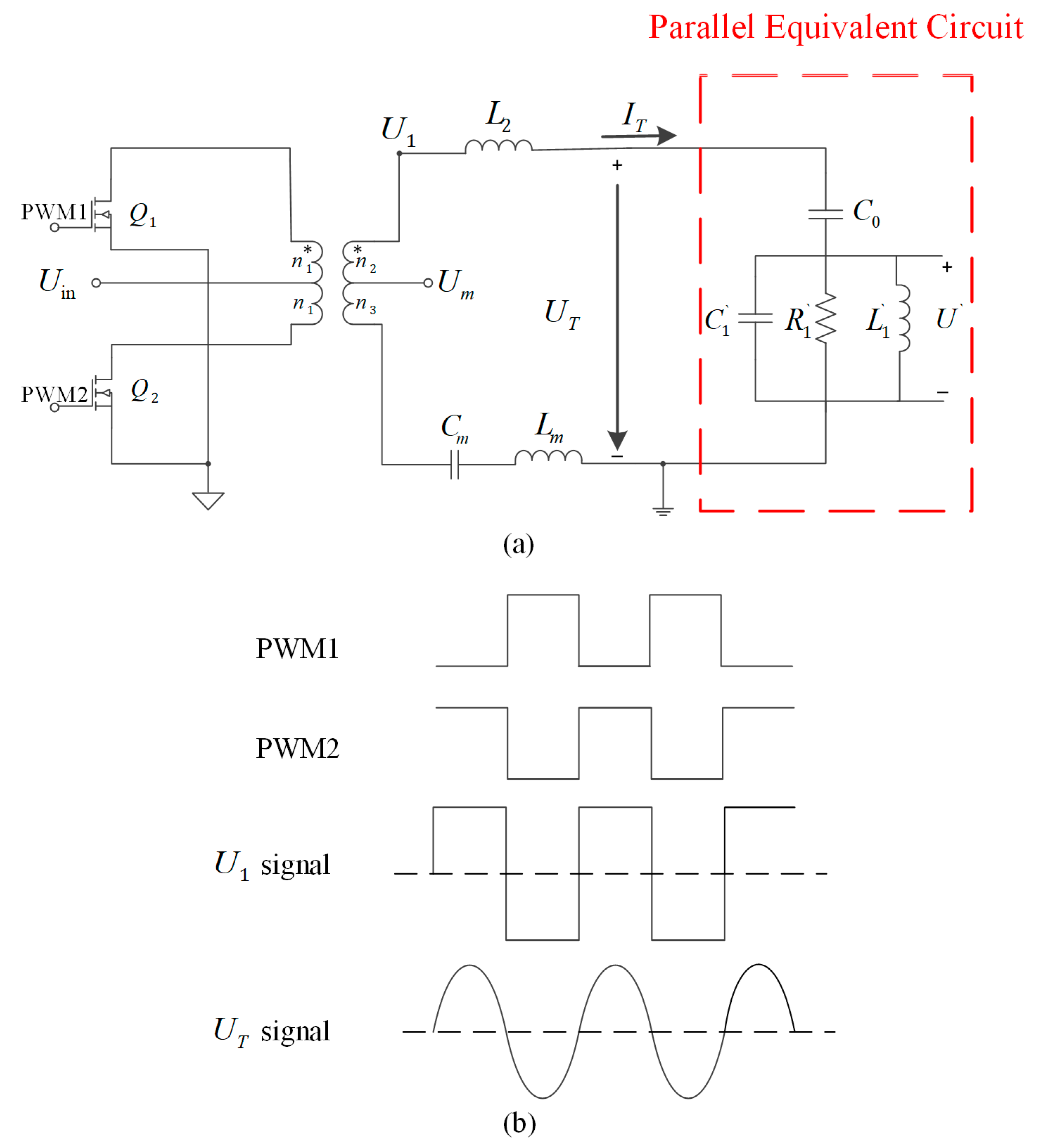
2.1. Equivalent Circuit Model
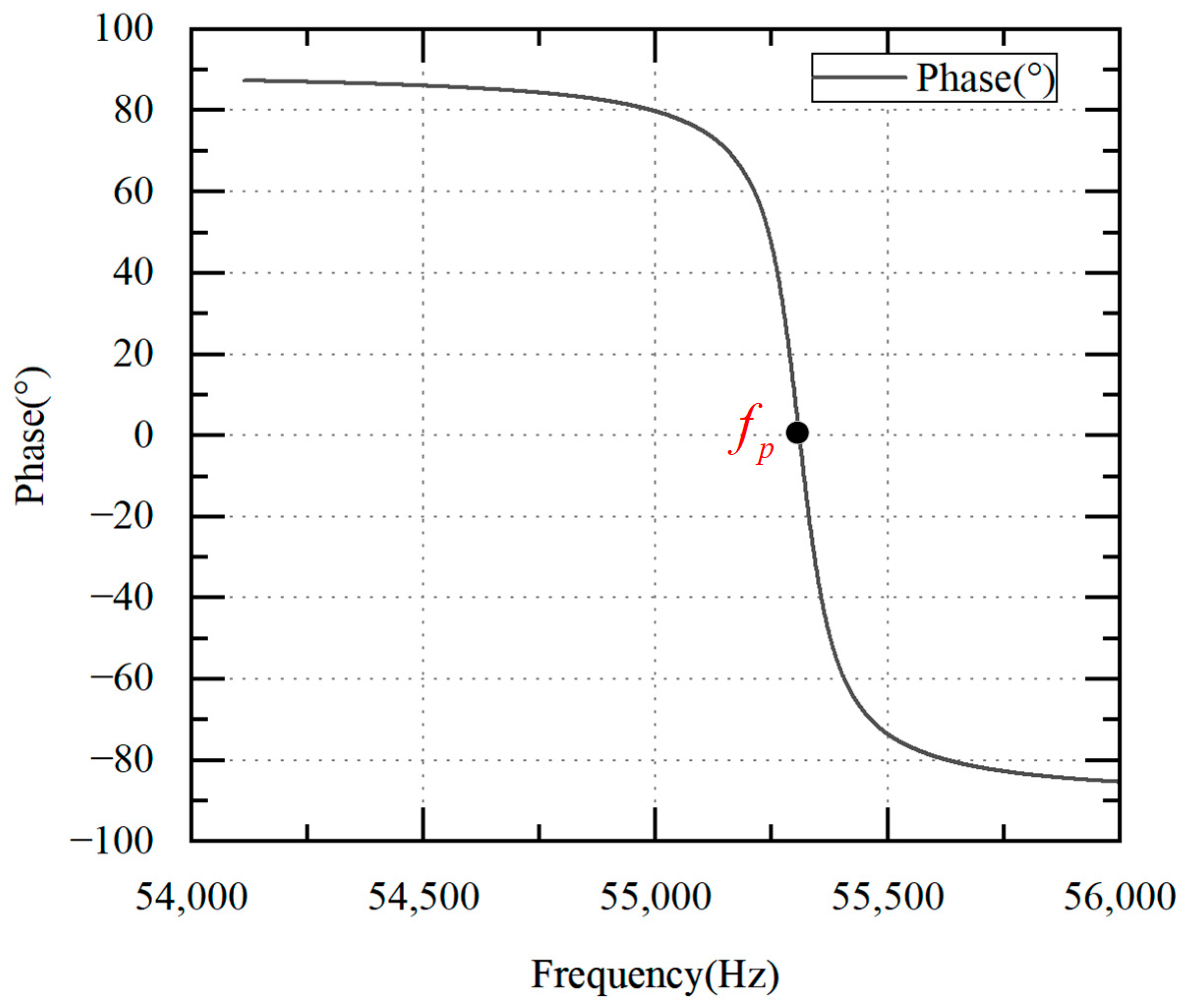
2.2. The Extraction of
2.3. Detection of Amplitude
3. Implementation of the Proposed Scheme
3.1. Voltage Sensing Scheme in Push–Pull Topology
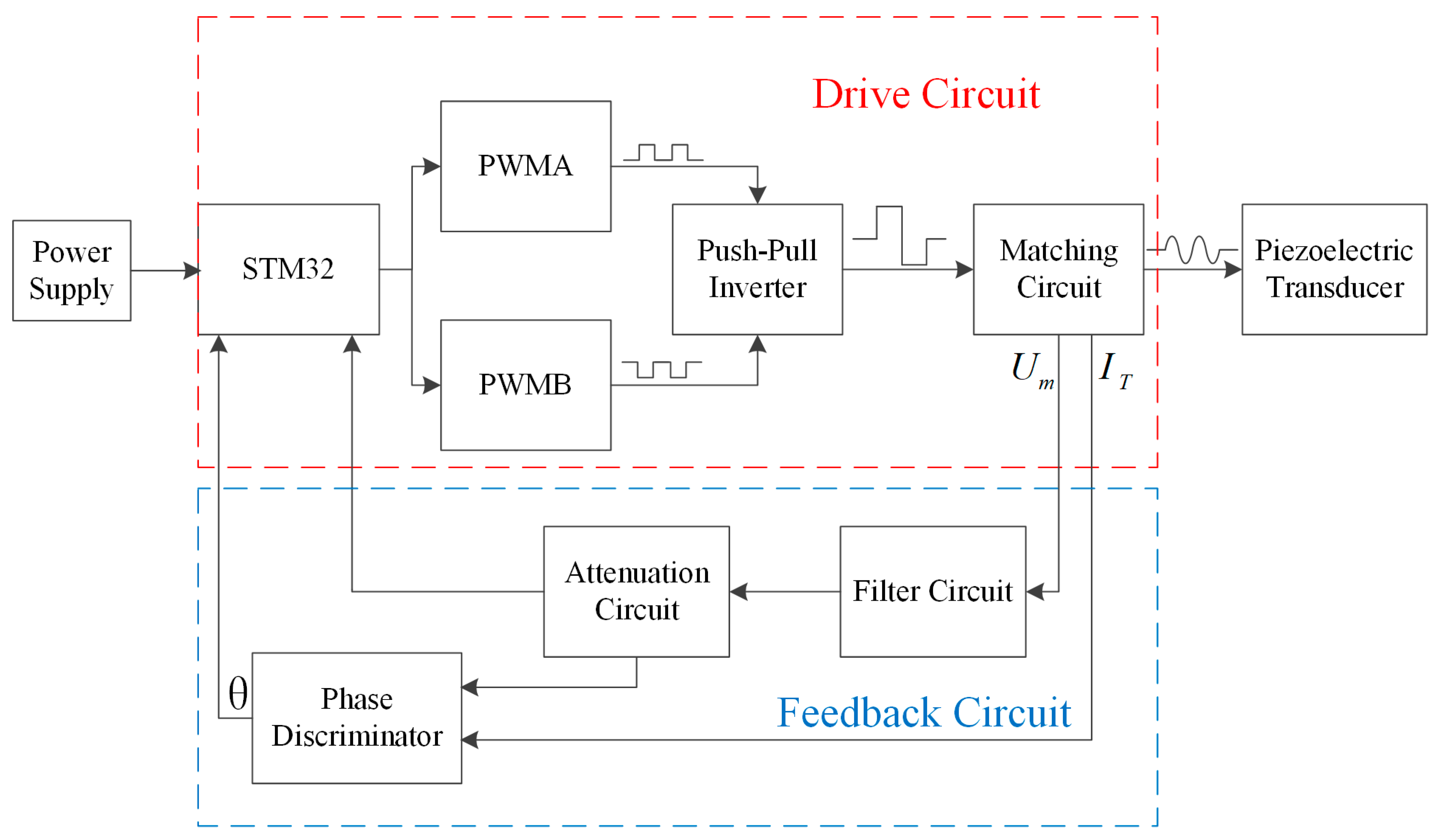
3.2. Hardware Structure
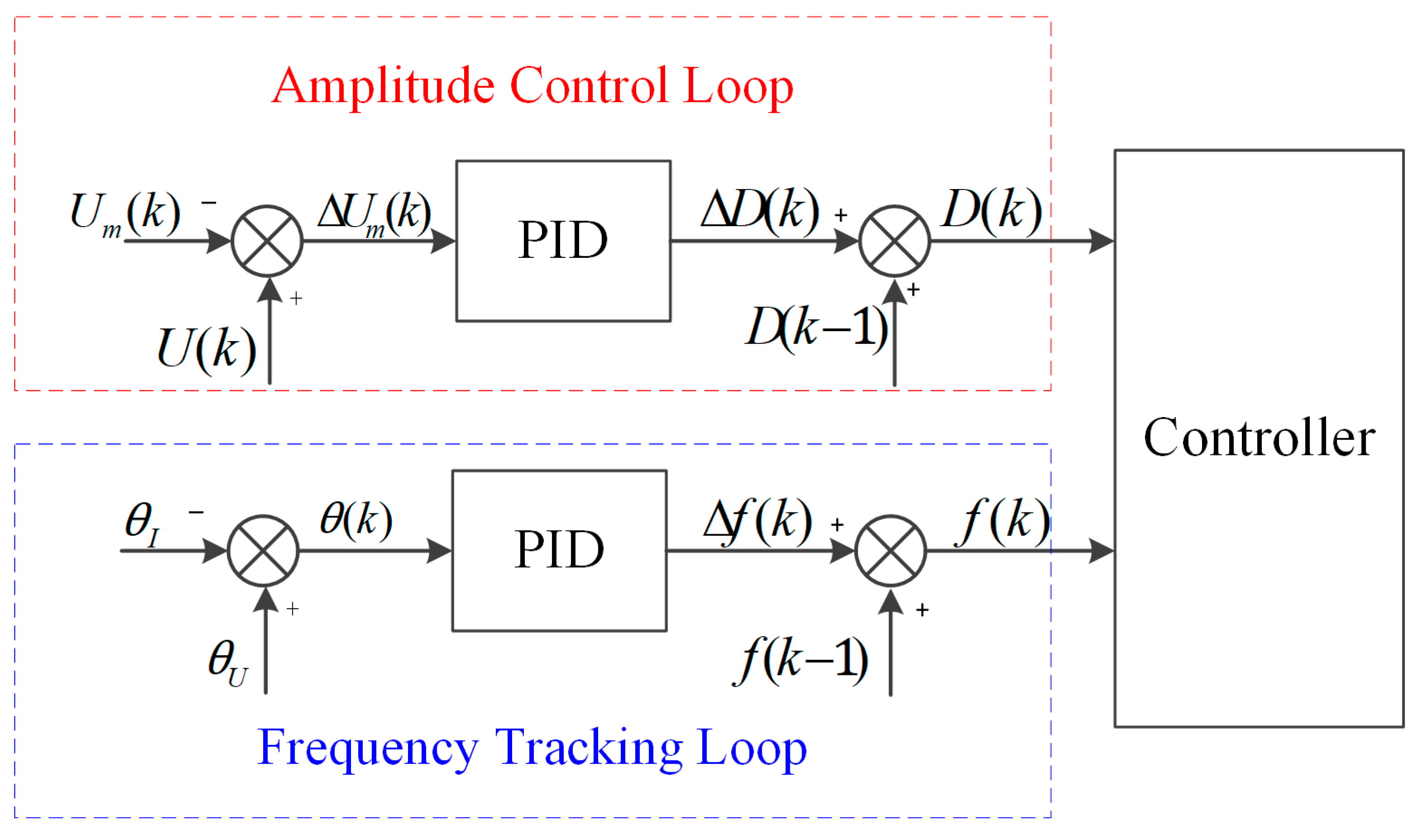
3.3. Control Structure
4. Experiment Results
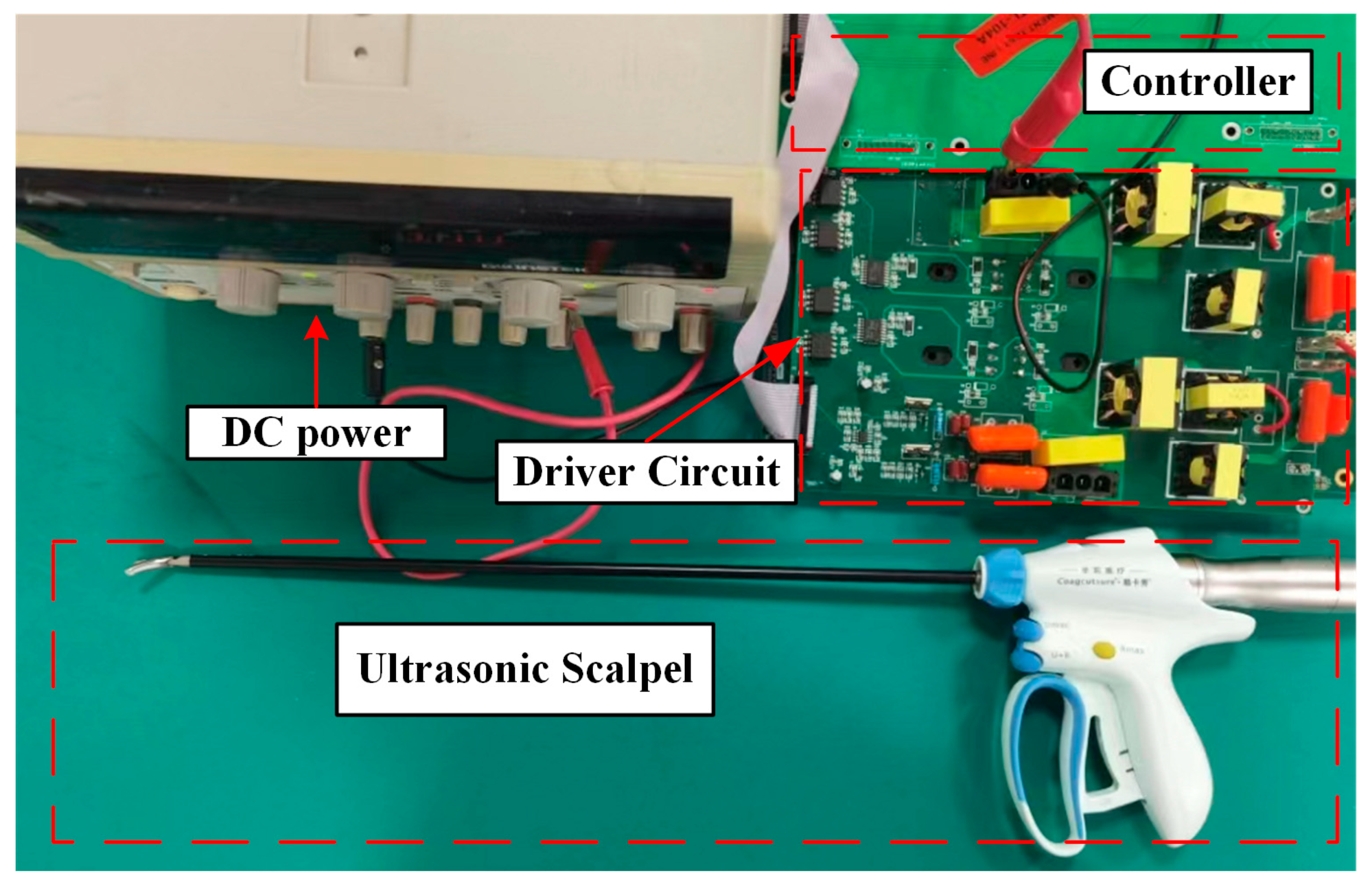
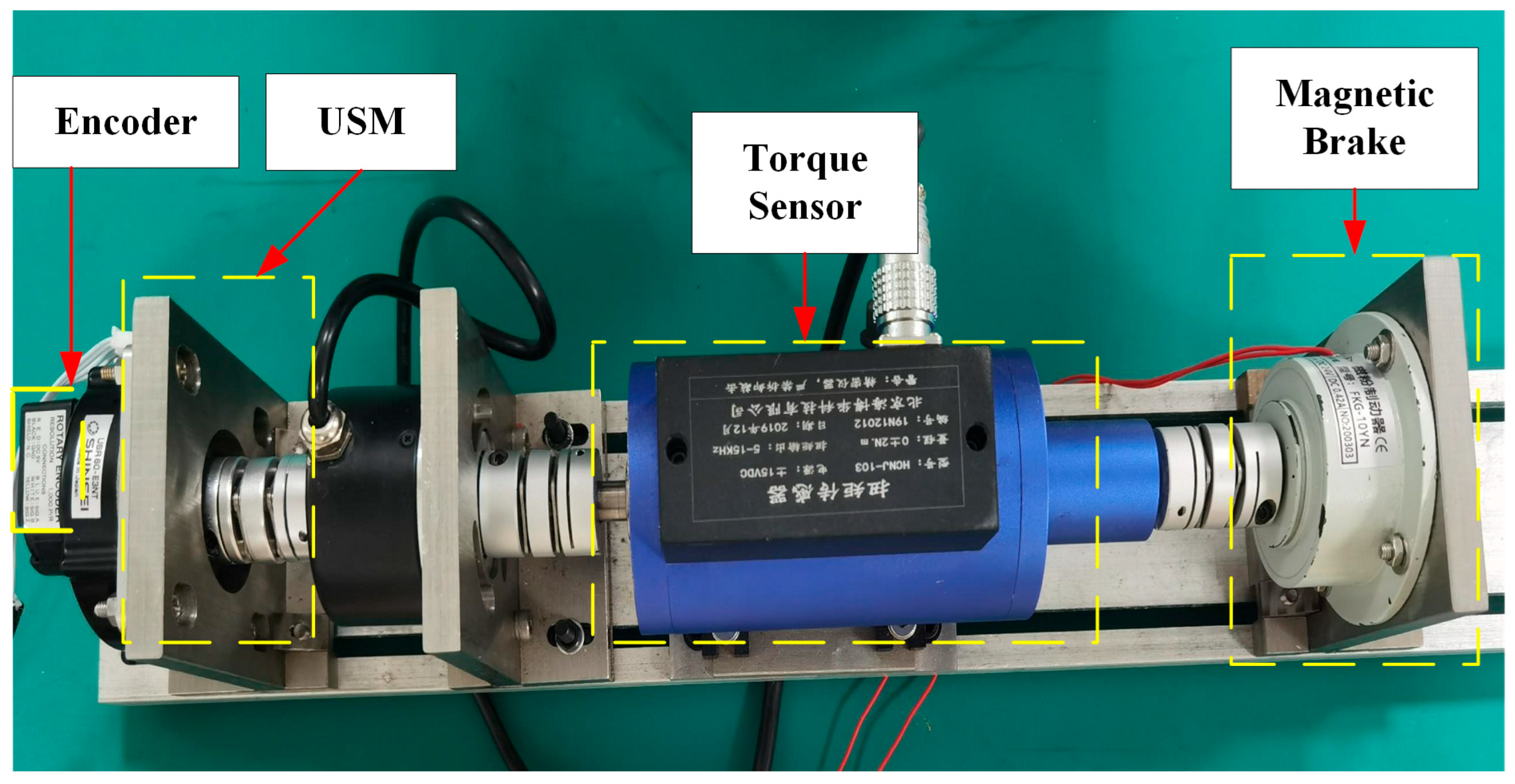
4.1. Experiment Setup
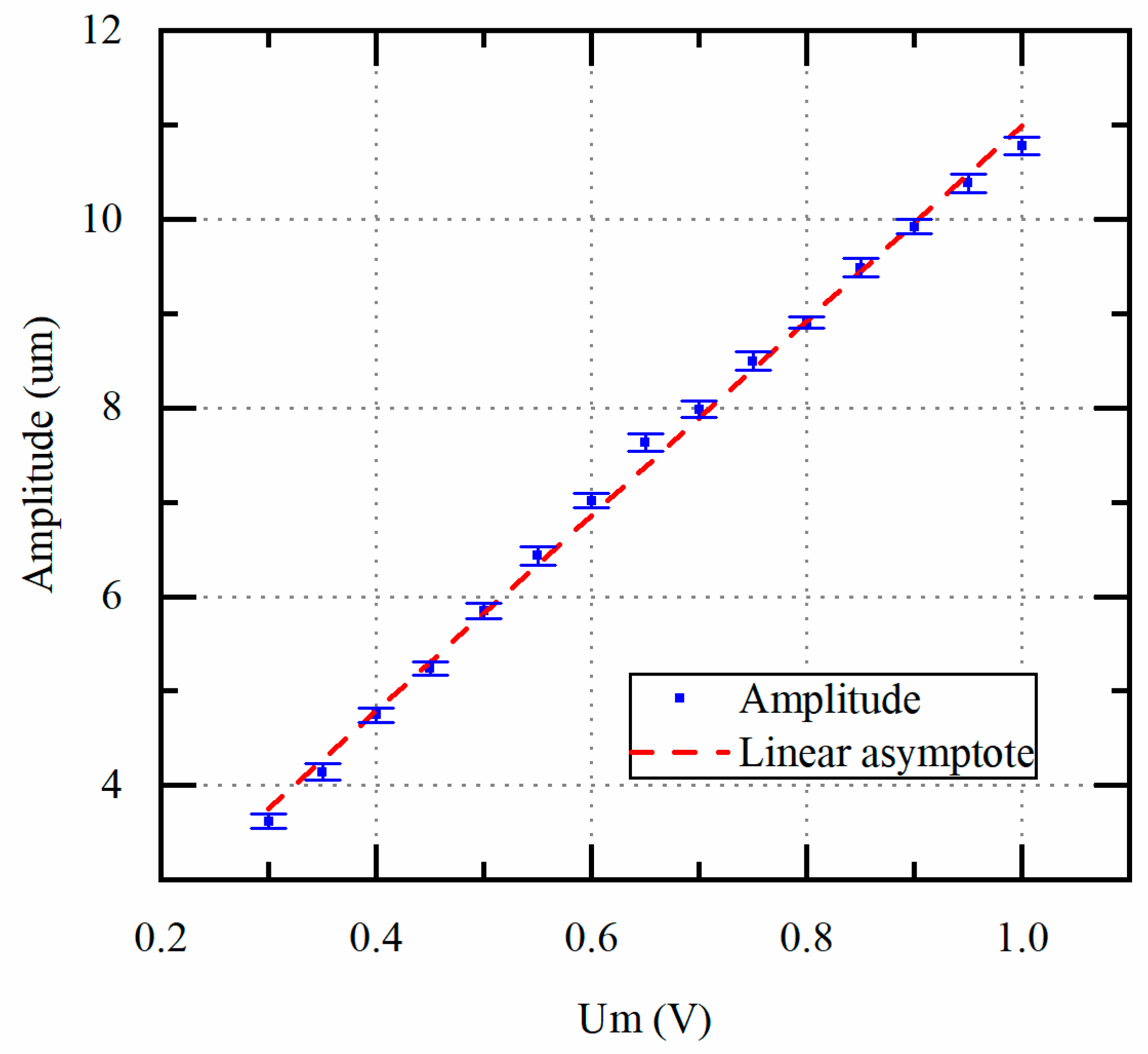
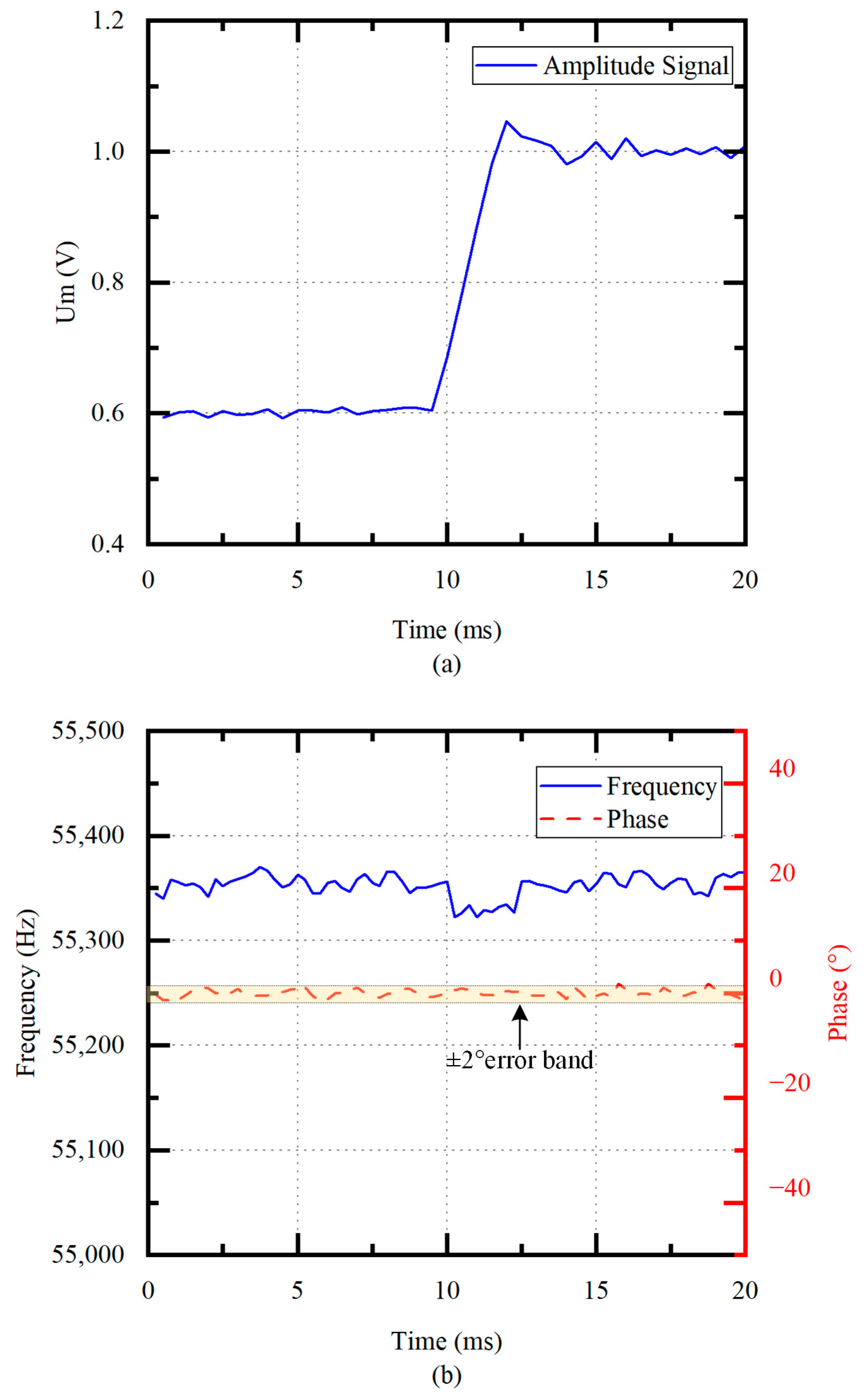
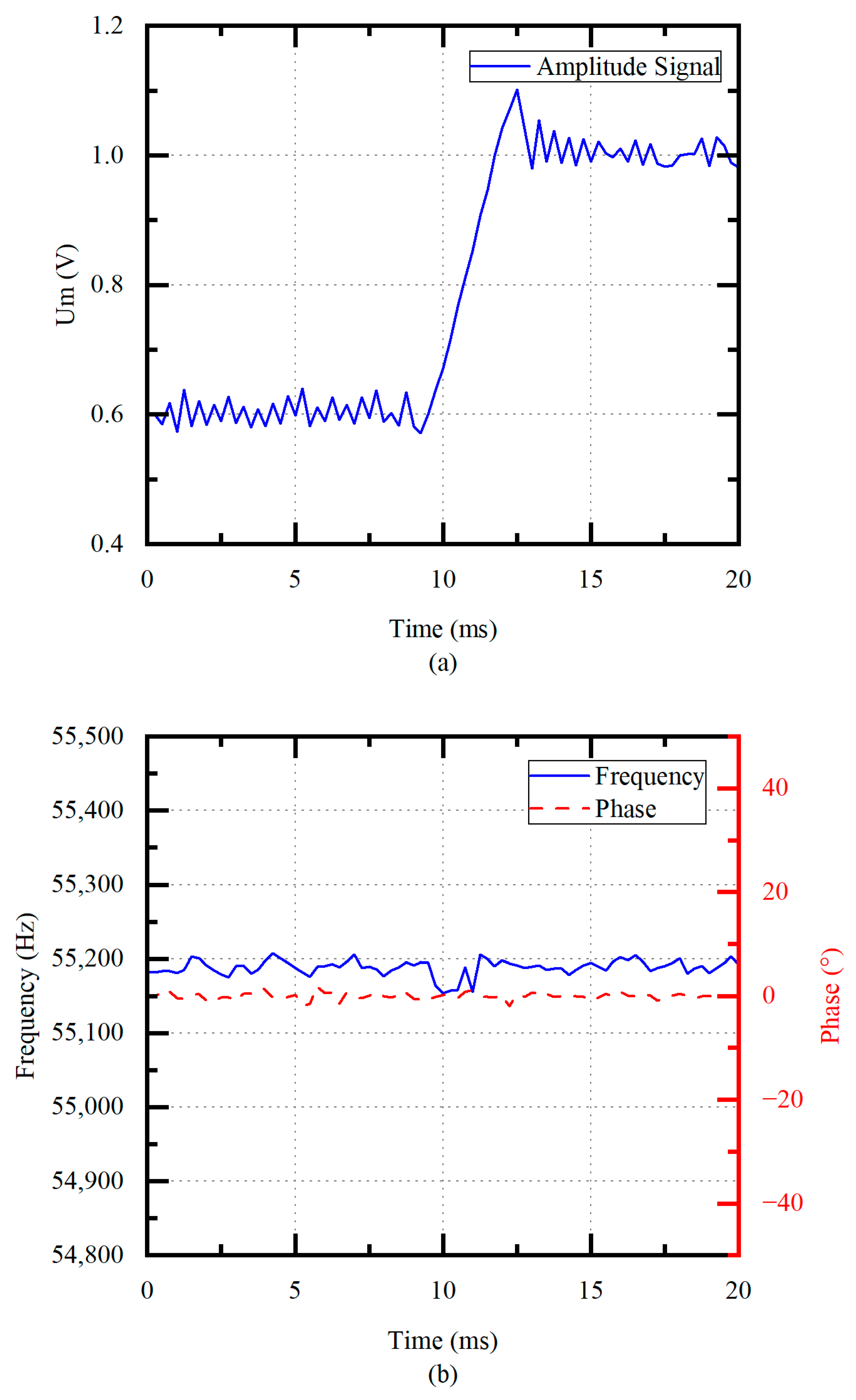
4.2. Verification of Amplitude and Feedback Voltage
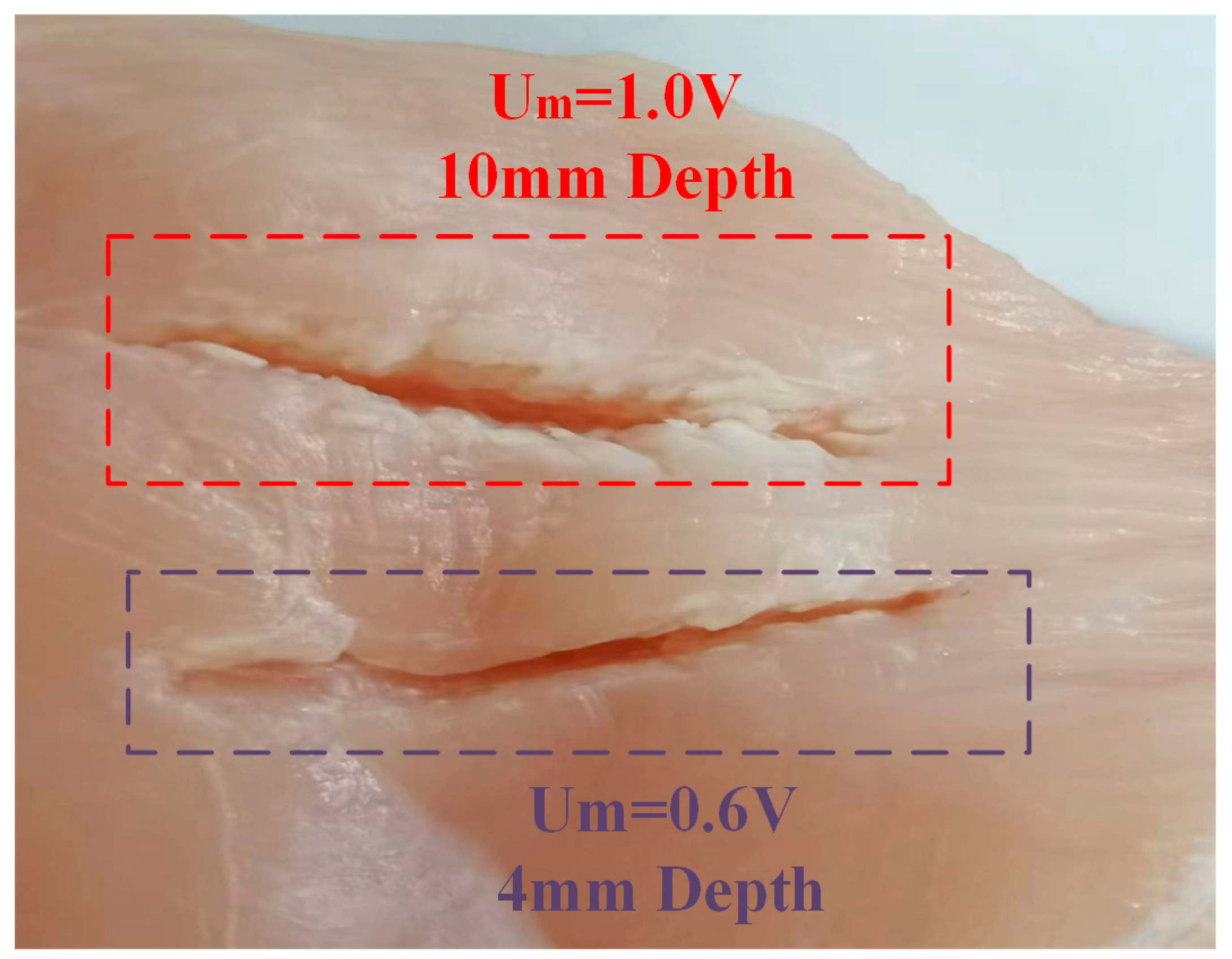
4.3. Experimental Results in Glycerin and Chicken Tissue
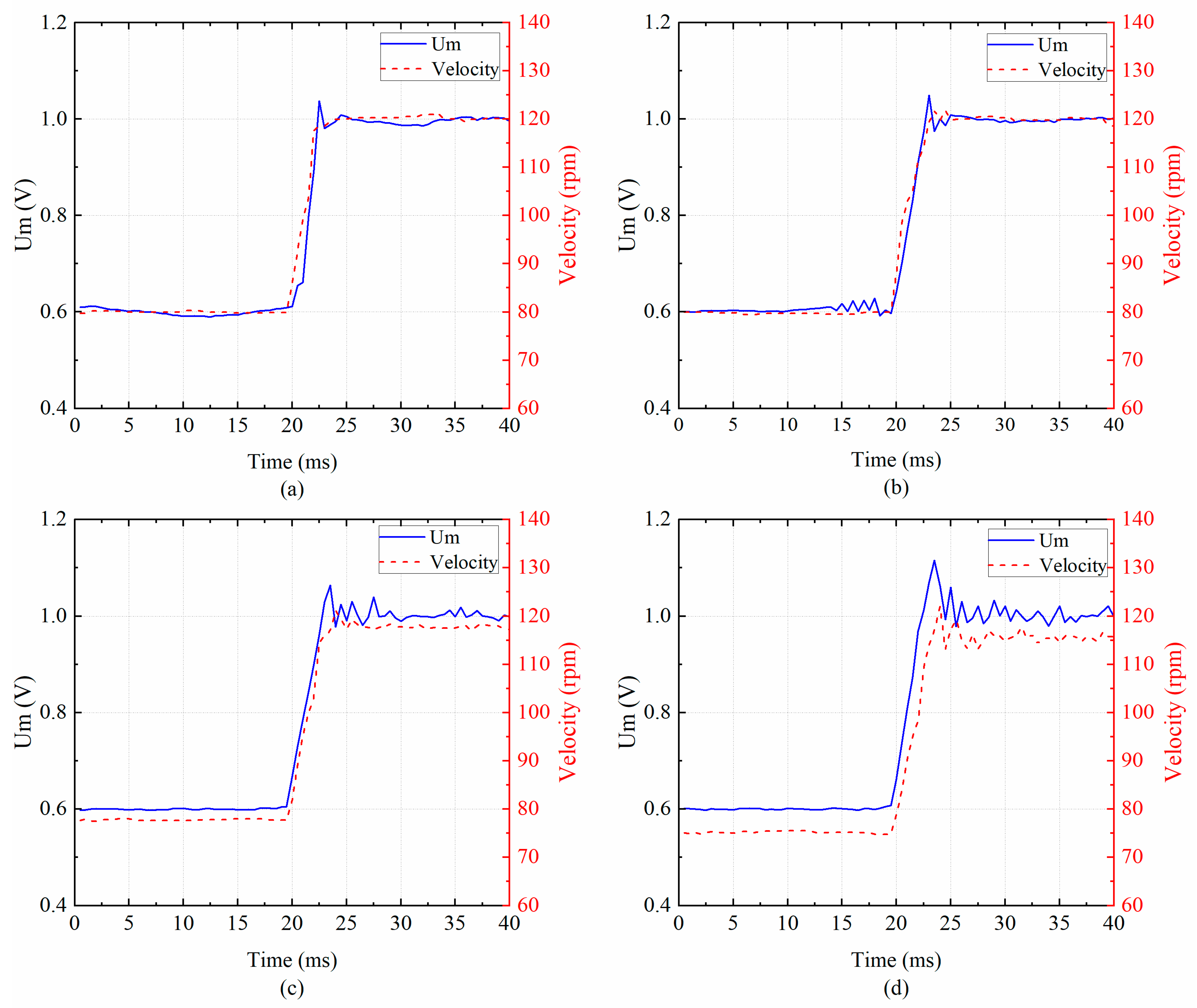
4.4. Experimental Results in Ultrasonic Motors
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zeng, Y.; Wang, X.K.; Qin, X.P.; Hua, L.; Xu, M. Laser Ultrasonic inspection of a Wire plus Arc Additive Manufactured (WAAM) sample with artificial defects. Ultrasonics 2021, 110, 15. [Google Scholar] [CrossRef]
- Perez-Sanchez, A.; Segura, J.A.; Rubio-Gonzalez, C.; Baldenegro-Perez, L.A.; Soto-Cajiga, J.A. Numerical design and analysis of a langevin power ultrasonic transducer for acoustic cavitation generation. Sens. Actuator A-Phys. 2020, 311, 112035. [Google Scholar] [CrossRef]
- Yu, P.P.; Wang, L.; Jin, J.M.; Ye, Z.L.; Chen, D. A novel piezoelectric actuated underwater robotic finger. Smart Mater. Struct. 2019, 28, 105047. [Google Scholar] [CrossRef]
- Cui, X.Y.; Yu, Y.H.; Liu, Q.J.; Liu, X.; Qing, X.L. Full-field monitoring of the resin flow front and dry spot with noninvasive and embedded piezoelectric sensor networks. Smart Mater. Struct. 2023, 32, 085021. [Google Scholar] [CrossRef]
- He, S.F.; Tang, H.; Zhu, Z.Y.; Zhang, P.Y.; Xu, Y.; Chen, X. A Novel Flexure Piezomotor With Minimized Backward and Nonlinear Motion Effect. IEEE Trans. Ind. Electron. 2022, 69, 652–662. [Google Scholar] [CrossRef]
- Wang, J.D.; Jiang, J.J.; Duan, F.J.; Zhang, F.M.; Liu, W.; Qu, X.H. A Novel Fast Resonance Frequency Tracking Method Based on the Admittance Circle for Ultrasonic Transducers. IEEE Trans. Ind. Electron. 2020, 67, 6864–6873. [Google Scholar] [CrossRef]
- Gao, L.; Yang, S.L.; Meng, B.; Tong, G.X.; Fan, H.P.; Yang, G.S. Frequency matching optimization model of ultrasonic scalpel transducer based on neural network and reinforcement learning. Eng. Appl. Artif. Intell. 2023, 117, 105572. [Google Scholar] [CrossRef]
- Quan, Q.Q.; Wang, T.Z.; Yu, H.Y.; Deng, Q.Y.; Tang, D.W.; Deng, Z.Q. An Ultrasonic Drilling System for Fast Drilling Speed With Uncertain Load. IEEE-ASME Trans. Mechatron. 2023, 28, 1477–1487. [Google Scholar] [CrossRef]
- Zhao, C.S. Ultrasonic Motors: Technologies and Applications; Science Press Beijing: Beijing, China, 2011; pp. 1–494. [Google Scholar]
- Zhang, K.; Gao, G.F.; Zhao, C.Y.; Wang, Y.; Wang, Y.; Li, J.F. Review of the design of power ultrasonic generator for piezoelectric transducer. Ultrason. Sonochem. 2023, 96, 106438. [Google Scholar] [CrossRef]
- Jiang, X.X.; Ng, W.T.; Chen, J. A Miniaturized Low-Intensity Ultrasound Device for Wearable Medical Therapeutic Applications. IEEE Trans. Biomed. Circuits Syst. 2019, 13, 1372–1382. [Google Scholar] [CrossRef]
- Peng, H.; Sabate, J.; Wall, K.A.; Glaser, J.S. GaN-Based High-Frequency High-Energy Delivery Transformer Push-Pull Inverter for Ultrasound Pulsing Application. IEEE Trans. Power Electron. 2018, 33, 6794–6806. [Google Scholar] [CrossRef]
- Kuang, Y.; Jin, Y.; Cochran, S.; Huang, Z. Resonance tracking and vibration stablilization for high power ultrasonic transducers. Ultrasonics 2014, 54, 187–194. [Google Scholar] [CrossRef] [PubMed]
- Martin, R.W.; Vaezy, S.; Proctor, A.; Myntti, T.; Lee, J.B.J.; Crum, L.A. Water-cooled, high-intensity ultrasound surgical applicators with frequency tracking. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2003, 50, 1305–1317. [Google Scholar] [CrossRef] [PubMed]
- Ben-Yaakov, S.; Peretz, M.M. A self-adjusting sinusoidal power source suitable for driving capacitive loads. IEEE Trans. Power Electron. 2006, 21, 890–898. [Google Scholar] [CrossRef]
- Pfeiffer, J.; Kuster, P.; Schulz, I.E.M.; Friebe, J.; Zacharias, P. Review of Flux Interaction of Differently Aligned Magnetic Fields in Inductors and Transformers. IEEE Access 2021, 9, 2357–2381. [Google Scholar] [CrossRef]
- Viguier, C.; Nadal, C.; Rouchon, J.F. Feasibility investigation of a static force measurement with longitudinal piezoelectric resonant sensor. Solid State Phenom. 2009, 147–149, 876–881. [Google Scholar] [CrossRef]
- Liu, C.; Xu, Z.; Xu, B.; Zhang, H.; Jin, L.; Sui, Q.; Shen, Z. Design and Research of Ultrasonic Motor Drive Based on LCLC Resonance Matching. In Proceedings of the 17th Annual Conference of China Electrotechnical Society, Beijing, China, 17–18 September 2022; Lecture Notes in Electrical Engineering (1013). Springer: Singapore, 2023; pp. 982–990. [Google Scholar]
- Fang, Z.W.; Yang, T.Y.; Zhu, Y.F.; Li, S.Y.; Yang, M. Velocity Control of Traveling-Wave Ultrasonic Motors Based on Stator Vibration Amplitude. Sensors 2019, 19, 5326. [Google Scholar] [CrossRef] [PubMed]
- Van Dyke, K.S. The piezo-electric resonator and its equivalent network. Proc. Inst. Radio Eng. 1928, 16, 742–764. [Google Scholar] [CrossRef]
- Liu, X.S.; Colli-Menchi, A.I.; Gilbert, J.; Friedrichs, D.A.; Malang, K.; Sanchez-Sinencio, E. An Automatic Resonance Tracking Scheme With Maximum Power Transfer for Piezoelectric Transducers. IEEE Trans. Ind. Electron. 2015, 62, 7136–7145. [Google Scholar] [CrossRef]
- Yang, T.Y.; Zhu, Y.F.; Li, S.Y.; An, D.W.; Yang, M.; Cao, W.W. Dielectric loss and thermal effect in high power piezoelectric systems. Sens. Actuator A-Phys. 2020, 303, 111724. [Google Scholar] [CrossRef]
- Di, S.S.; Fan, W.; Li, H.F. Parallel resonant frequency tracking based on the static capacitance online measuring for a piezoelectric transducer. Sens. Actuator A-Phys. 2018, 270, 18–24. [Google Scholar] [CrossRef]
- Wang, T.Z.; Quan, Q.Q.; Tang, D.W.; Yang, Z.; Huang, J.C.A.; Guo, F.; Meng, L.Z.; Zhao, Z.J.; Deng, Z.Q. Effect of hyperthermal cryogenic environments on the performance of piezoelectric transducer. Appl. Therm. Eng. 2021, 193, 116725. [Google Scholar] [CrossRef]
- Ebina, K.; Hasegawa, H.; Kanai, H. Investigation of frequency characteristics in cutting of soft tissue using prototype ultrasonic knives. Jpn. J. Appl. Phys. Part 1-Regul. Pap. Brief Commun. Rev. Pap. 2007, 46, 4793–4800. [Google Scholar] [CrossRef]
- Yang, T.Y.; Zhu, Y.F.; Fang, Z.W.; Wu, H.Y.; Jiang, W.L.; Yang, M. A Driving and Control Scheme of High Power Piezoelectric Systems over a Wide Operating Range. Sensors 2020, 20, 4401. [Google Scholar] [CrossRef]
- Gao, X.C.; Yang, M.; Zhu, Y.F.; Hu, Y.H. Two-Phase Stator Vibration Amplitude Compensation of Traveling-Wave Ultrasonic Motor. Actuators 2022, 11, 278. [Google Scholar] [CrossRef]





| This Work | [21] | |
|---|---|---|
| Resonant Point | ||
| Build-up Time | 3 ms | 10 ms |
| Unloaded Frequency Variation | 50 Hz | 80 Hz |
| Loaded Frequency Variation | 200 Hz | 250 Hz |
| Inverter Topology | Push–Pull Inverter | Full bridge Inverter |
| The Number of Used MOSFET Switching Tubes in the Inverter | Two | Four |
| Inverter Complexity | Simple | Complex |
| Controller Form | Discrete | Discrete |
| Parameters | Values |
|---|---|
| Drive Frequency | 40–45 kHz |
| Drive Voltage | 130 Vrms |
| Rated Torque | 1.0 Nm |
| Rated Output | 5.0 W |
| Maximum Velocity | 150 rpm |
| Proposed Scheme | [27] | |||
|---|---|---|---|---|
| Load (Nm) | Overshoot (%) | Velocity Fluctuation (rpm) | Overshoot (%) | Velocity Fluctuation (rpm) |
| 0 | 0.8 | 0.96 | 0.96 | 1.68 |
| 0.1 | 1.6 | 1.44 | 1.92 | 1.68 |
| 0.2 | 2.5 | 1.88 | 1.94 | 1.92 |
| 0.3 | 5.4 | 2.26 | 6.9 | 2.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Y.; Yang, M.; Zhu, Y.; Wang, S. Push–Pull Inverter Using Amplitude Control and Frequency Tracking for Piezoelectric Transducers. Micromachines 2023, 14, 2147. https://doi.org/10.3390/mi14122147
Hu Y, Yang M, Zhu Y, Wang S. Push–Pull Inverter Using Amplitude Control and Frequency Tracking for Piezoelectric Transducers. Micromachines. 2023; 14(12):2147. https://doi.org/10.3390/mi14122147
Chicago/Turabian StyleHu, Yinghua, Ming Yang, Yuanfei Zhu, and Shangting Wang. 2023. "Push–Pull Inverter Using Amplitude Control and Frequency Tracking for Piezoelectric Transducers" Micromachines 14, no. 12: 2147. https://doi.org/10.3390/mi14122147
APA StyleHu, Y., Yang, M., Zhu, Y., & Wang, S. (2023). Push–Pull Inverter Using Amplitude Control and Frequency Tracking for Piezoelectric Transducers. Micromachines, 14(12), 2147. https://doi.org/10.3390/mi14122147






