A Novel Type of Pseudo-Decoupling Method for Two Degree-of-Freedom Piezoelectrically Driven Compliant Mechanisms Based on Elliptical Parameter Compensation
Abstract
:1. Introduction
2. Pseudo-Decoupling Method Based on Phase Difference Compensation
2.1. Pseudo-Decoupling Principle of PDC-Based Method
2.2. Pseudo-Decoupling Optimization of PDC-Based Method
3. Pseudo-Decoupling Method Based on Elliptical Parameter Compensations
3.1. Basic Principle of Elliptical Parameter Compensations
3.2. Optimization of Amplification and Coupling Coefficients
3.3. Verification of Elliptical Parameter Compensations
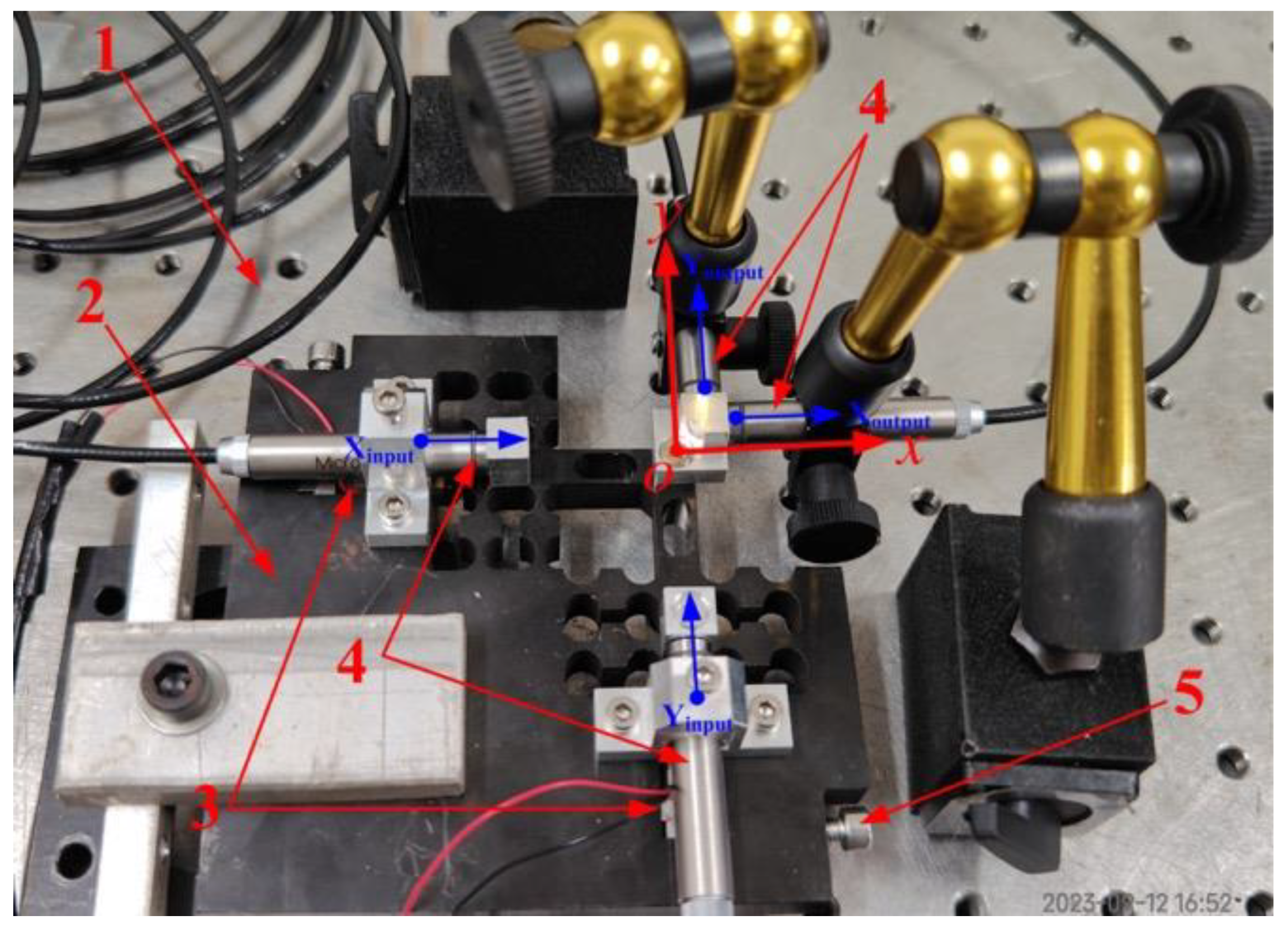
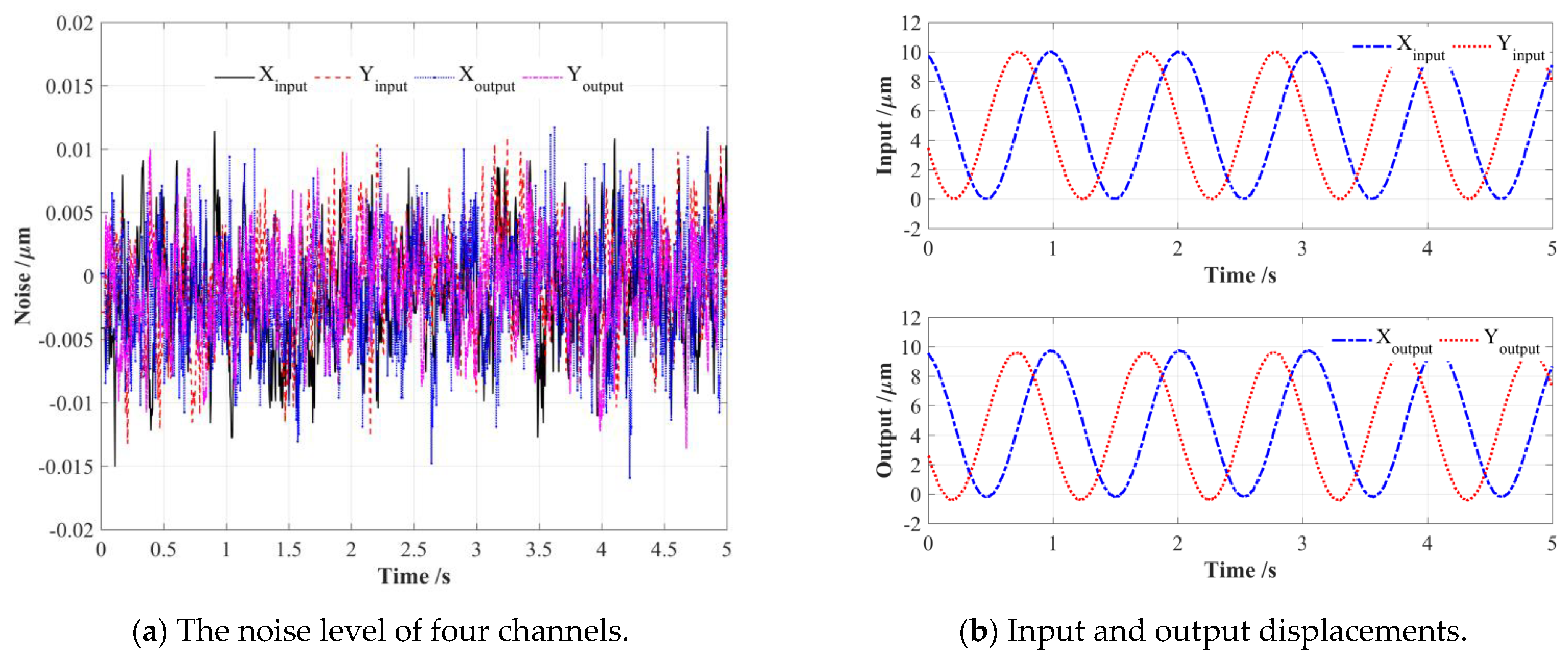
4. Experiments and Discussion
5. Conclusions
- (a)
- The developed PDC-based pseudo-decoupling method could effectively eliminate the attitude rotations of the elliptical trajectories with different motion parameters, but some degrees of dimension amplifications could obviously be observed on almost all of the elliptical trajectories. This as mainly because the decoupling substructures of 2-DOF PDCMs have a certain level of displacement magnification, so their trajectory tracking precision makes it very difficult to satisfy the practical demands in many EVM processes of high-precision functional microstructured surfaces.
- (b)
- The EPC-based pseudo-decoupling method was mathematically modeled through defining an amplification coefficient and a coupling coefficient that could effectively describe the relationships between the input motion parameters and output geometrical features of different elliptical trajectories. Under different input motion parameters, the output elliptical trajectories theoretically calculated by the EPC-based model were a very close approximation to the FEA-based simulation results, and their corresponding error distances were very slight and so can be fully negligible, which can strongly prove the high accuracy and validity of the constructed EPC model.
- (c)
- The actual input motion parameters compensated by the inverse EPC model were respectively applied on the 2-DOF PDCM to generate the expected elliptical trajectories through FEA-based simulation and EPC-based calculation. Under four series of different input motion parameters, the FEA simulated and EPC-calculated output elliptical trajectories both had very good approximation with our expected trajectories, and their corresponding error distances were very slight and could be completely ignored, which strongly prove the high effectiveness and feasibility of the EPC-based pseudo-decoupling method in improving the trajectory tracking precision of conventional 2-DOF PDCMs.
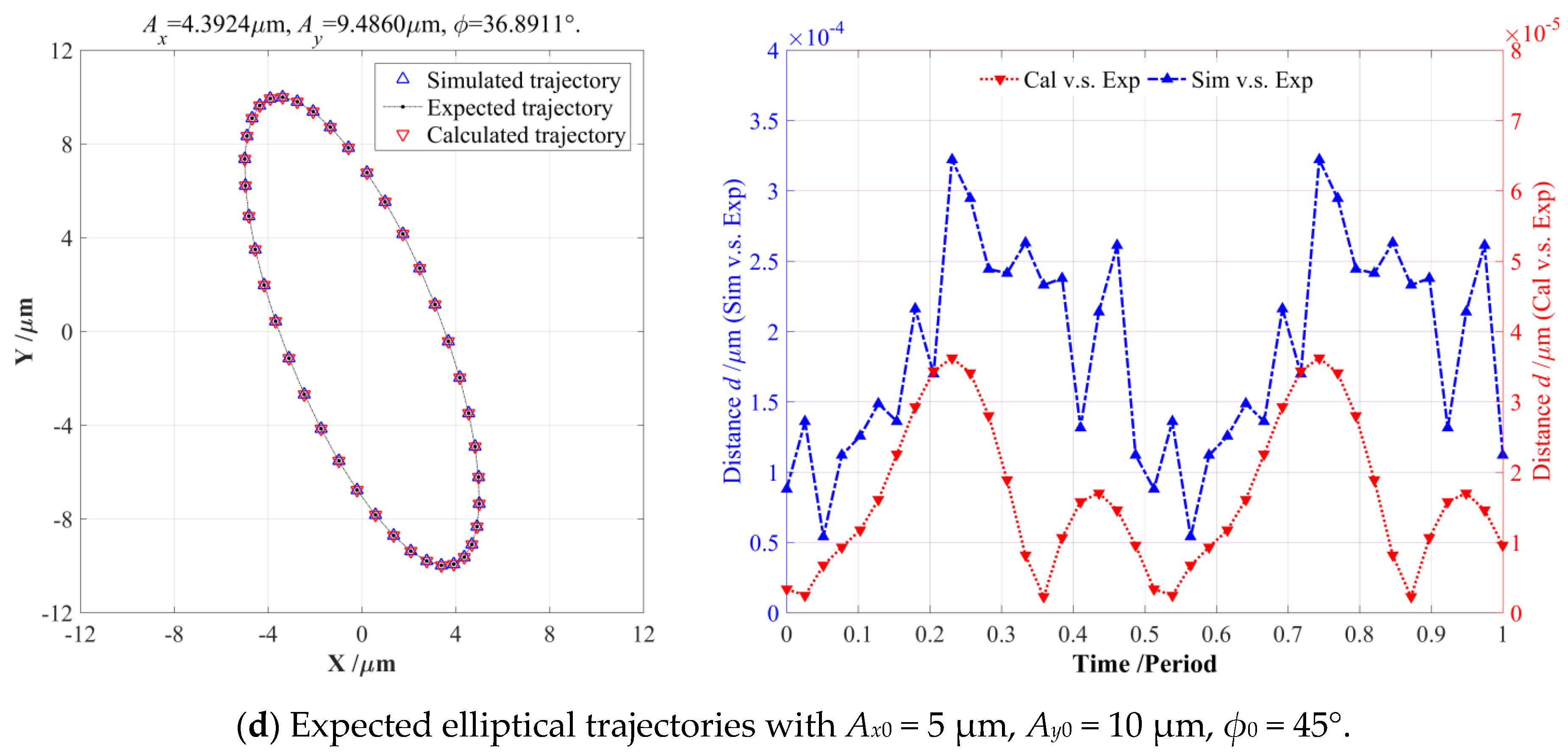
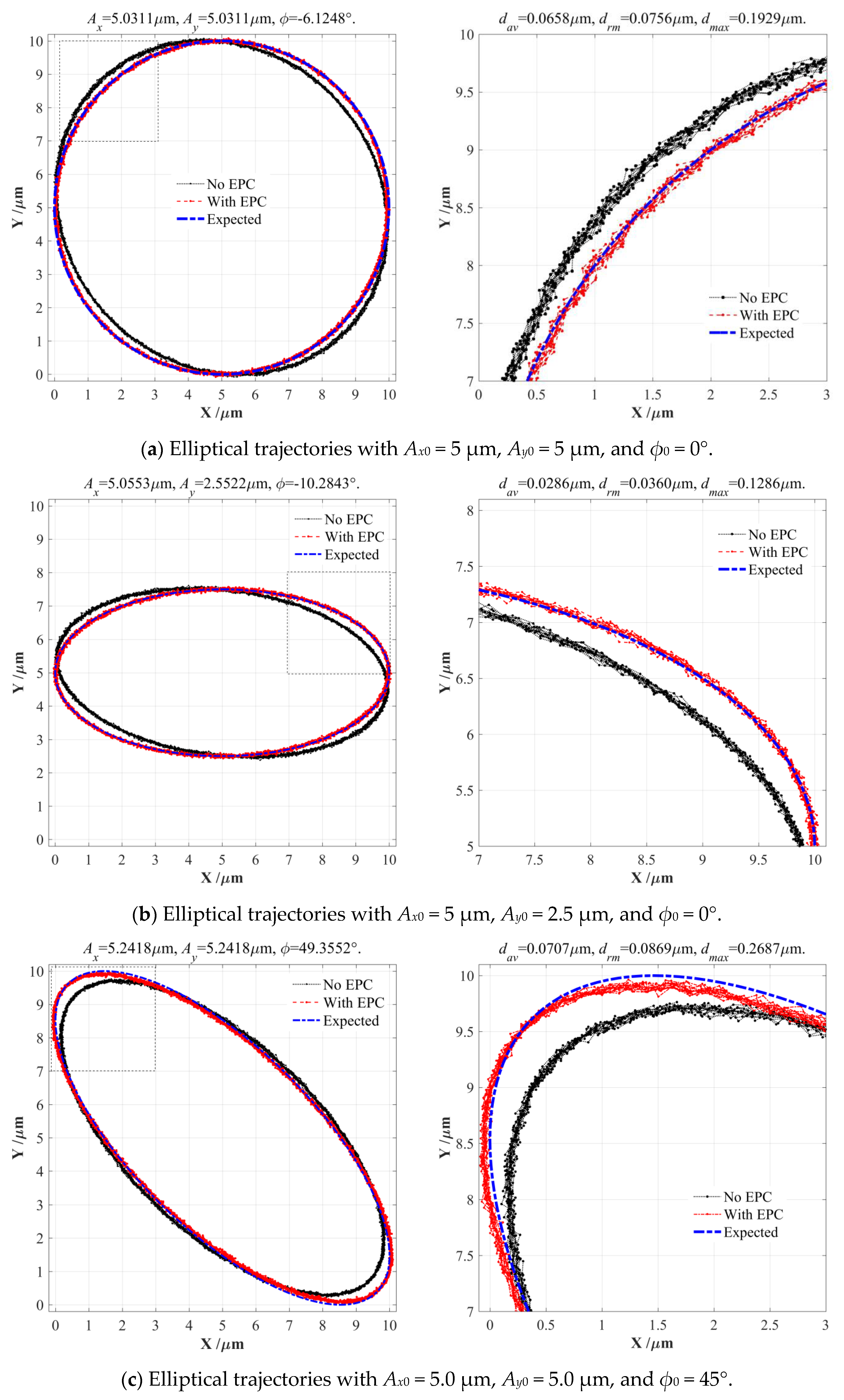
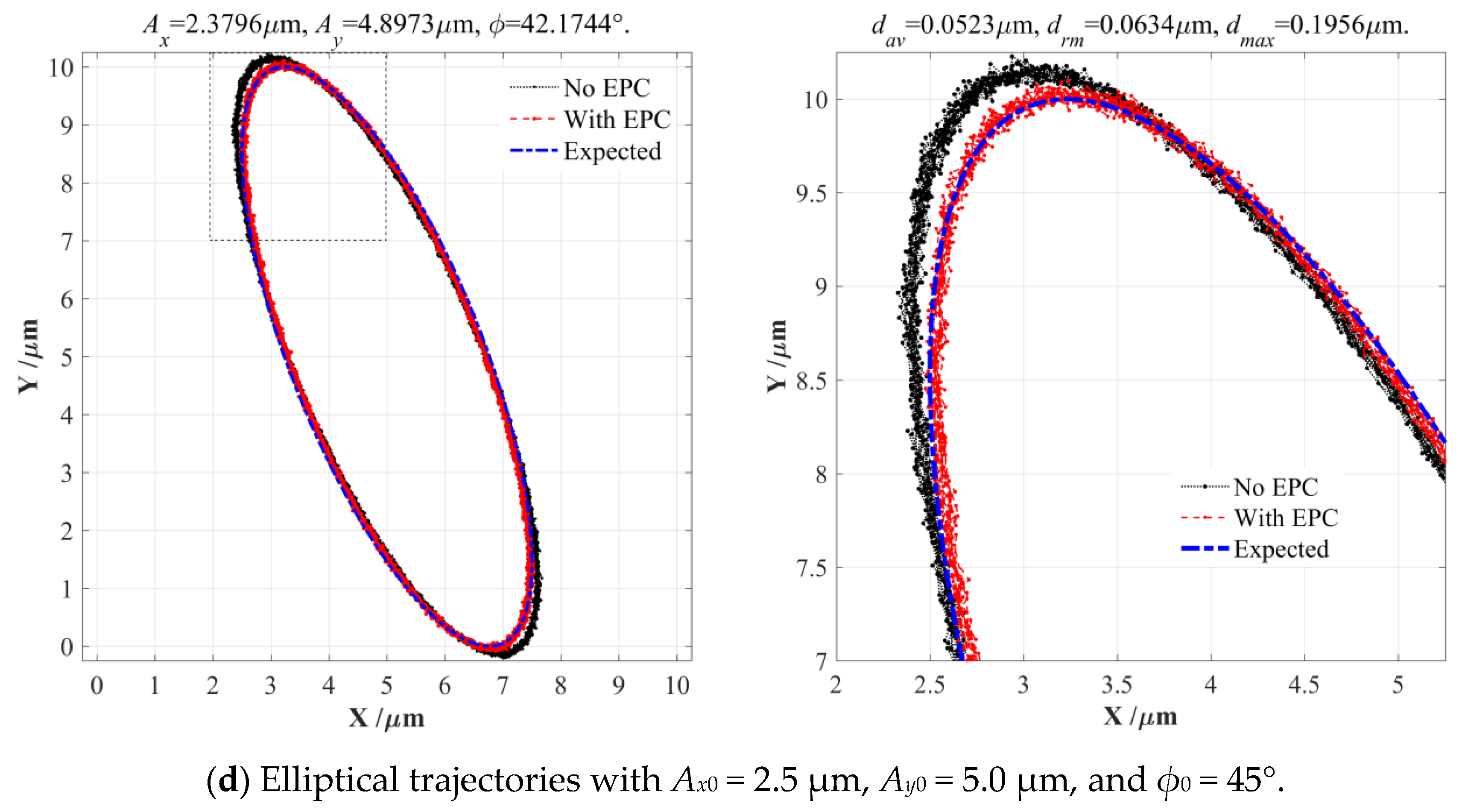
- (d)
- The input motion parameters compensated by the inverse EPC-based model were practically employed in exciting the 2-DOF PDCM of the built EVM experimental system, then the output elliptical trajectories were measured and collected. However, all of the obtained results clearly indicated the absolute error distances between the expected and experimental elliptical trajectories under four series of different motion parameters, which well demonstrated that this newly proposed EPC-based method exhibits strong feasibility and high effectiveness in eliminating the bad cross-coupling motions of conventional 2-DOF PDCMs, especially with parallel configuration.
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, J.; Cui, T.; Ge, C.; Sui, Y.; Yang, H. Review of micro/nano machining by utilizing elliptical vibration cutting. Int. J. Mach. Tools Manuf. 2016, 106, 109–126. [Google Scholar] [CrossRef]
- Zhang, J.G.; Suzuki, N.; Shamoto, E. Advanced applications of elliptical vibration cutting in micro/nano- machining of difficult-to-cut materials. In Simulation and Experiments of Material-Oriented Ultra-Precision Machining; Springer: Singapore, 2019; pp. 167–200. ISBN 978-981-13-3334-7. [Google Scholar]
- Yuan, Y.; Zhang, D.; Jing, X.; Ehmann, K.F. Freeform surface fabrication on hardened steel by double frequency vibration cutting. J. Am. Acad. Dermatol. 2019, 275, 116369. [Google Scholar] [CrossRef]
- Li, G.; Che, L.; Wang, B.; Ding, F.; Zhang, C.F. Experimental study on elliptical vibration cutting for optical microstructures. In Proceedings of the 7th International Symposium on Advanced Optical Manufacturing and Testing Technologies: Design, Manufacturing, and Testing of Micro-and Nano-Optical Devices and Systems, Harbin, China, 26–29 April 2014; pp. 19–27. [Google Scholar]
- Lin, J.Q.; Lu, M.M.; Zhou, X.Q. Development of a Non-Resonant 3D Elliptical Vibration Cutting Apparatus for Diamond. Turning. Exp. Technol. 2016, 40, 173–183. [Google Scholar] [CrossRef]
- Han, J.; Lin, J.; Li, Z.; Lu, M.; Zhang, J. Design and Computational Optimization of Elliptical Vibration-Assisted Cutting System With a Novel Flexure Structure. IEEE Trans. Ind. Electron. 2019, 66, 1151–1161. [Google Scholar] [CrossRef]
- Wang, R.; Zhou, X.; Meng, G. Development of a new type of elliptical/non-elliptical vibration coining for manufacturing functional microstructure surfaces. J. Micromech. Microeng. 2019, 29, 025012–025027. [Google Scholar] [CrossRef]
- Kim, G.D.; Loh, B.G. Machining of micro-channels and pyramid patterns using elliptical vibration cutting. Int. J. Adv. Manuf. Technol. 2009, 49, 961–968. [Google Scholar] [CrossRef]
- Li, Y.; Huang, J.; Tang, H. A Compliant Parallel XY Micromotion Stage with Complete Kinematic Decoupling. IEEE Trans. Autom. Sci. Eng. 2012, 9, 538–553. [Google Scholar] [CrossRef]
- Lai, L.-J.; Gu, G.-Y.; Zhu, L.-M. Design and control of a decoupled two degree of freedom translational parallel micro-positioning stage. Rev. Sci. Instruments 2012, 83, 045105. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Xu, Q. Development and assessment of a novel decoupled XY parallel micropositioning platform. IEEE/ASME Trans. Mechatron. 2010, 15, 125–135. [Google Scholar]
- Xu, Q. XY Flexure Stage; John Wiley & Sons Singapore Pte. Ltd.: Singapore, 2016. [Google Scholar] [CrossRef]
- Xiao, X.; Li, Y.; Xiao, S. Development of a novel large stroke 2-DOF micromanipulator for micro/nano manipulation. Microsyst. Technol. 2017, 23, 2993–3003. [Google Scholar] [CrossRef]
- Sun, X.; Chen, W.; Tian, Y.; Fatikow, S.; Zhou, R.; Zhang, J.; Mikczinski, M. A novel flexure-based microgripper with double amplification mechanisms for micro/nano manipulation. Rev. Sci. Instrum. 2013, 84, 085002. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.Q.; Zou, Q.; Wang, T.; Zhang, Q.C.; Zhang, Y.L.; Yao, J.N. Structures Analysis and Optimum Design of the Large Stroke Two-Axis Fast Tool Servo. Adv. Mater. Res. 2013, 853, 477–481. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhou, X.; Liu, Z.; Wang, R.; Zhu, L. Development of a piezoelectrically actuated two-degree-of-freedom fast tool servo with decoupled motions for micro-/nanomachining. Precis. Eng. 2014, 38, 809–820. [Google Scholar] [CrossRef]
- Polit, S.; Dong, J. Development of a High-Bandwidth XY Nanopositioning Stage for High-Rate Micro-/Nanomanufacturing. IEEE/ASME Trans. Mechatron. 2011, 16, 724–733. [Google Scholar] [CrossRef]
- Yong, Y.K.; Aphale, S.S.; Moheimani, S.O.R. Design, identification, and control of a flexure-based XY stage for fast nanoscale positioning. IEEE Trans. Nanotechnol. 2009, 8, 46–54. [Google Scholar] [CrossRef]
- Xiao, S.; Li, Y.; Zhao, X. Design and analysis of a novel flexure-based XY micro-positioning stage driven by electromagnetic actuators. In Proceedings of the International Conference on Fluid Power and Mechatronics (FPM), Beijing, China, 17–20 August 2011. [Google Scholar]
- Wang, R.; Zhou, X.; Meng, G. Development of A New Type of 2-DOF Piezo-Actuated Pseudo-Decoupled Compliant Mechanism for Elliptical Vibration Machining. Micromachines 2019, 10, 122. [Google Scholar] [CrossRef]
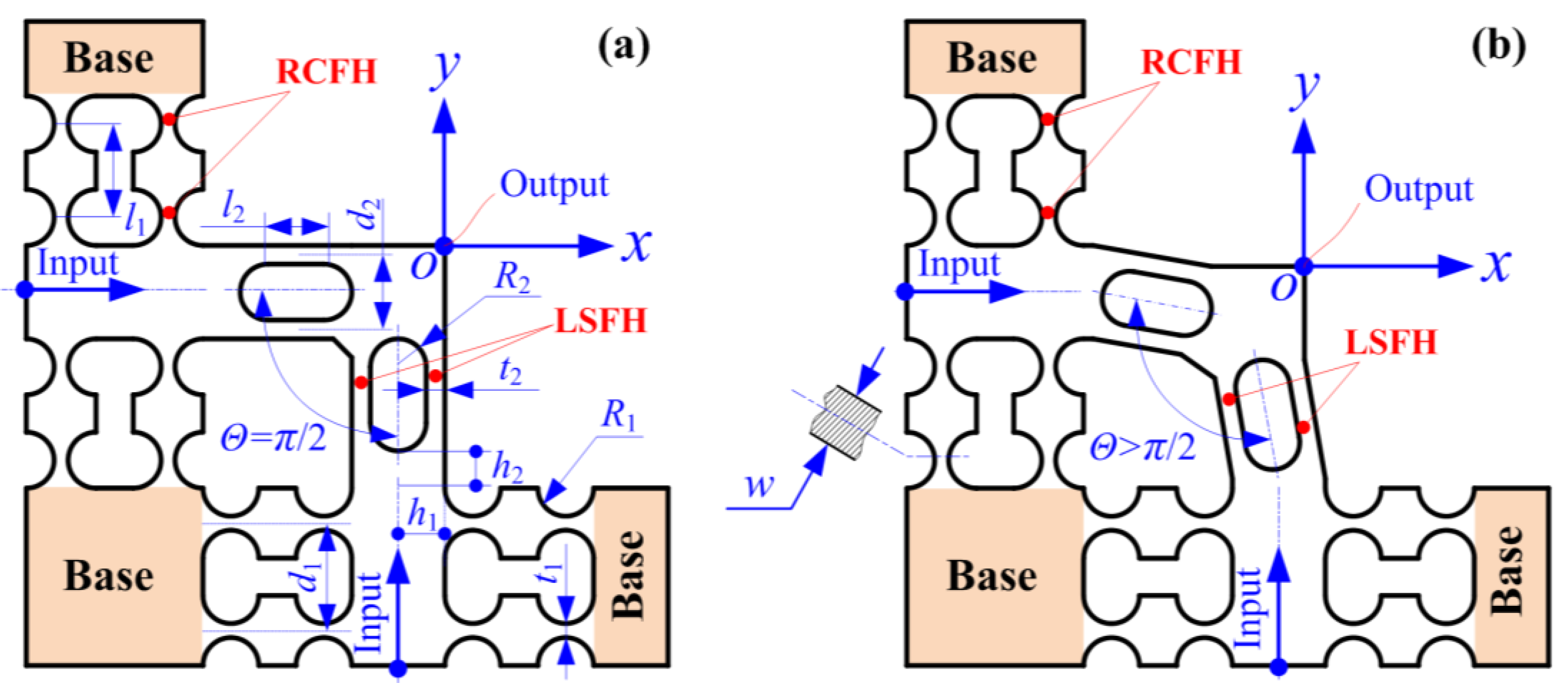





| LSFH | t1 = 1 mm | R1 = 2.5 mm | l1 = 6 mm | h1 = 4 mm | d1 = 7.0 mm | w = 10 mm |
| RCFH | t2 = 1 mm | R2 = 3.0 mm | l2 = 10 mm | h2 = 4 mm | d2 = 10 mm | w = 10 mm |
| Geometric Parameters of Output Elliptical Trajectory | Phase Difference Compensation (PDC) Angles Δϕ (Ax = 10 μm, Ay = 10 μm, ϕ = 0°) | |||||
|---|---|---|---|---|---|---|
| Δϕ = 0° | Δϕ = 2.5° | Δϕ = 5.0° | Δϕ = 7.5° | Δϕ = 8.25° | Δϕ = 10.0° | |
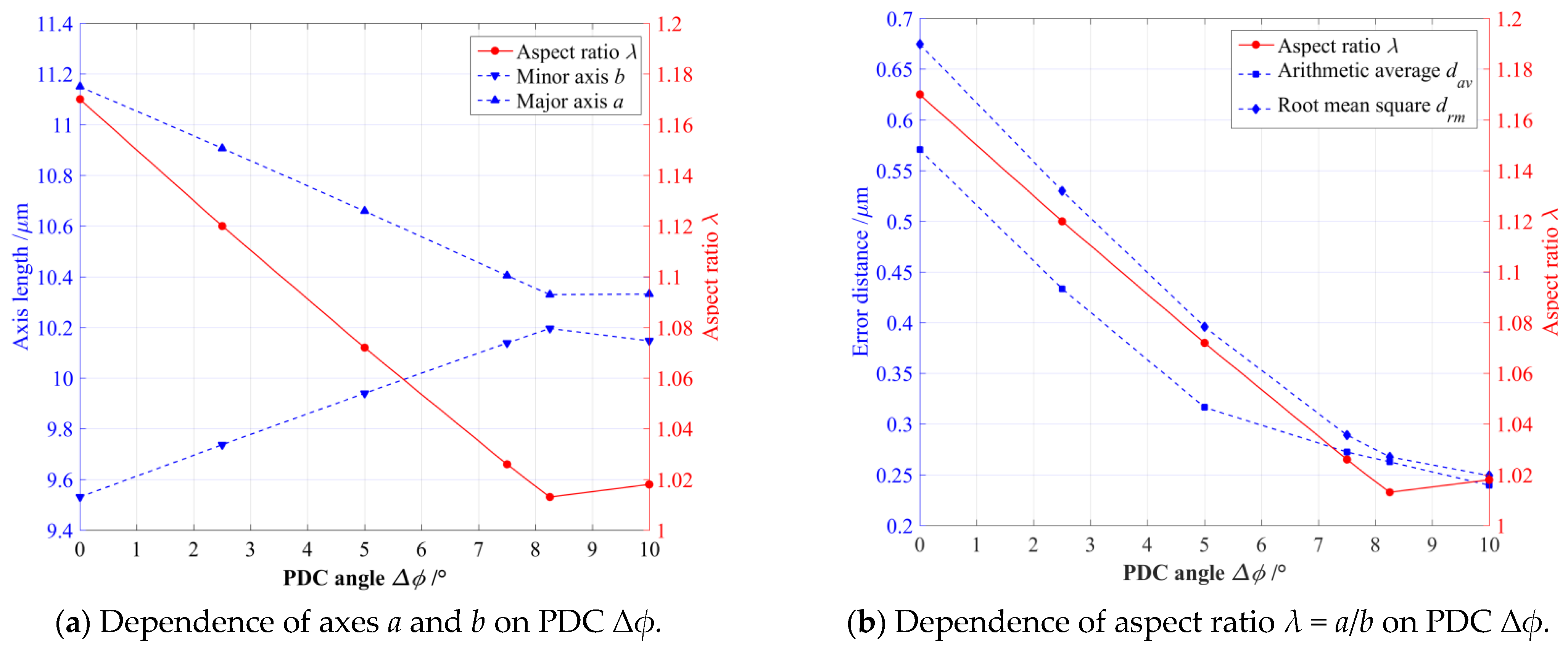
| Semi-major axis a/μm | 11.150 | 10.907 | 10.659 | 10.405 | 10.329 | 10.331 |
| Semi-minor axis b/μm | 9.530 | 9.737 | 9.940 | 10.138 | 10.196 | 10.147 |
| Relative aspect ratio λ = a/b | 1.170 | 1.120 | 1.072 | 1.026 | 1.013 | 1.018 |
| Attitude rotation angle θ/° | −44.88 | −44.82 | −44.72 | −44.17 | −43.42 | 43.76 |
| Arithmetic average distance dav/μm | 0.5706 | 0.4335 | 0.3165 | 0.2723 | 0.2627 | 0.2396 |
| Root mean square distance drm/μm | 0.6747 | 0.5299 | 0.3958 | 0.2890 | 0.2676 | 0.2491 |
| The Fitting Results of Geometrical Parameters of FEA-Based Output Elliptical Trajectories | Motion Parameters and PDC Angles of Input Elliptical Trajectories | ||||||
|---|---|---|---|---|---|---|---|
| Ay = 10 μm, Az = 5 μm, ϕ = 0°, Δϕ = 10.5° | Ay = 5 μm, Az = 10 μm, ϕ = 0°, Δϕ = 10.5° | Ay = 10 μm, Az = 10 μm, ϕ = 45°, Δϕ = 8.25° | Ay = 10 μm, Az = 10 μm, ϕ = −45°, Δϕ = 8.25° | Ay = 10 μm, Az = 5 μm, ϕ = 30°, Δϕ = 15.0° | Ay = 5 μm, Az = 10 μm, ϕ = −30°, Δϕ = 12.0° | ||
| Semi-major axis length a/μm | Expected | 10.000 | 10.000 | 13.066 | 13.066 | 10.372 | 10.370 |
| Simulated | 10.282 | 10.274 | 12.826 | 14.054 | 10.501 | 10.843 | |
| Error | 2.82% | 2.74% | 1.84% | 7.57% | 1.25% | 4.57% | |
| Semi-minor axis length b/μm | Expected | 5.000 | 5.000 | 5.412 | 5.412 | 4.175 | 4.175 |
| Simulated | 5.090 | 5.094 | 5.025 | 6.012 | 3.614 | 4.644 | |
| Error | 1.81% | 1.89% | 7.14% | 11.09% | 13.43% | 11.23% | |
| Relative aspect ratio λ = a/b | Expected | 2.000 | 2.000 | 2.414 | 2.414 | 2.484 | 2.484 |
| Simulated | 2.020 | 2.017 | 2.552 | 2.338 | 2.906 | 2.335 | |
| Error | 0.99% | 0.84% | 5.72% | 3.17% | 16.96% | 5.99% | |
| Altitude rotation angle θ/° | Expected | 0 | 0 | 45.000 | −45.000 | 16.845 | 16.841 |
| Simulated | −0.400 | 0.398 | 44.923 | −44.950 | 17.397 | 17.018 | |
| Error | --- | --- | 0.17% | 0.12% | 3.26% | 1.05% | |
| Arithmetic average distance dav/μm | 0.1853 | 0.1840 | 0.3862 | 0.8608 | 0.3907 | 0.5444 | |
| Root mean square distance drm/μm | 0.1999 | 0.1973 | 0.3956 | 0.8721 | 0.4597 | 0.5473 | |
| Motion Parameters of Input Elliptical Trajectories | Error Distances dav and drm Relative to Expected Elliptical Trajectories | |||
|---|---|---|---|---|
| No. | Expected Output Elliptical Parameters | Actual Input Elliptical Parameters | FEA-Simulation | EPC-Calculation |
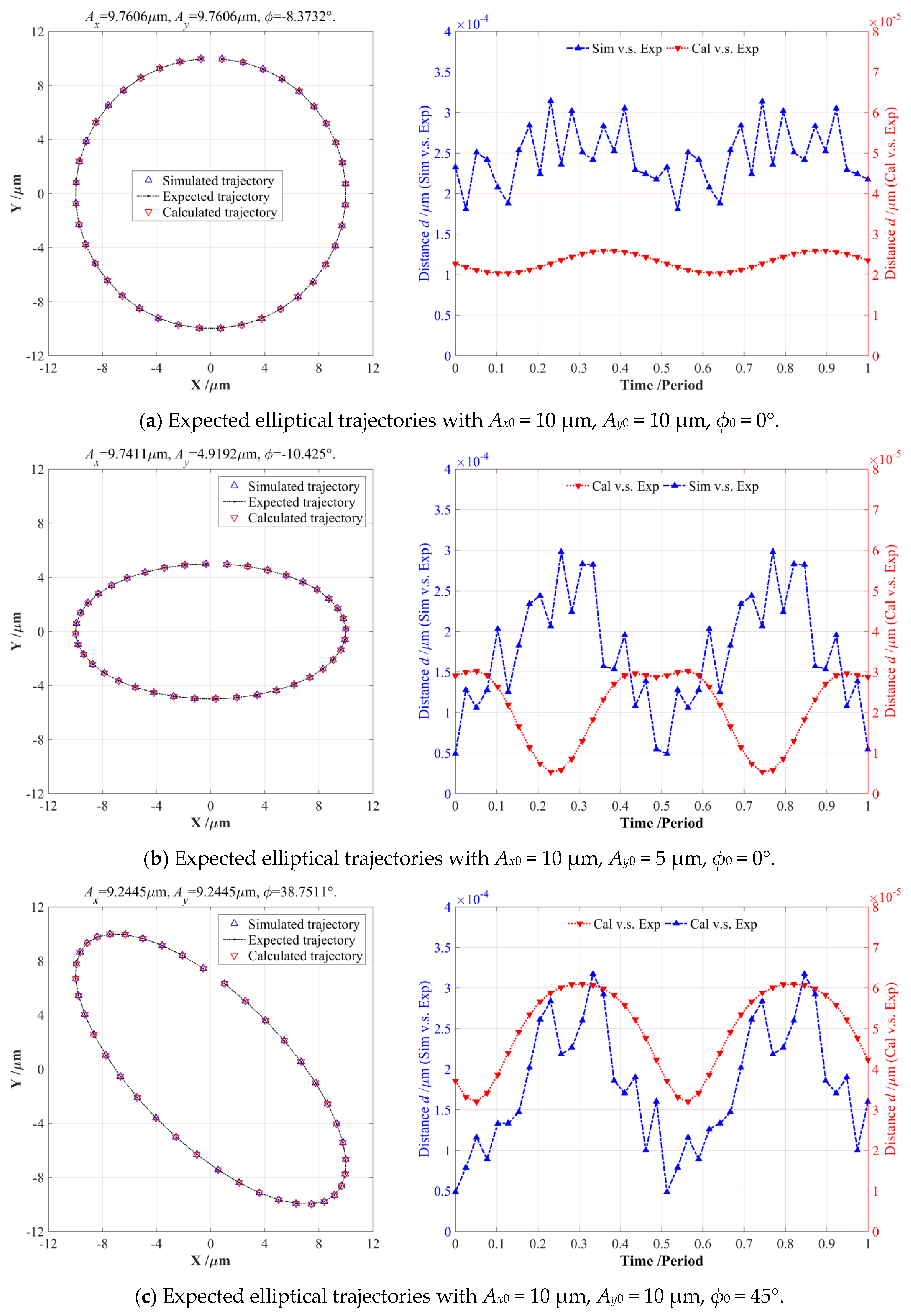
| 1 | Ax0 = 10 μm; Ay0 = 10 μm; ϕ0 = 0°. | Ax = 9.7606 μm; Ay = 9.7606 μm; ϕ = −8.3732° (Δϕ = −8.3732°). | dav1 = 2.4589 × 10−4 μm drm1 = 2.4848 × 10−4 μm | dav2 = 2.3139 × 10−5 μm drm2 = 2.3224 × 10−5 μm |
| 2 | Ax0 = 10 μm; Ay0 = 5 μm; ϕ0 = 0°. | Ax = 9.7411 μm; Ay = 4.9192 μm; ϕ = −10.425° (Δϕ = −10.425°). | dav1 = 1.7499 × 10−4 μm drm1 = 1.8870 × 10−4 μm | dav2 = 2.0978 × 10−5 μm drm2 = 2.2844 × 10−5 μm |
| 3 | Ax0 = 10 μm; Ay0 = 10 μm; ϕ0 = 45° | Ax = 9.2445 μm; Ay = 9.2445 μm; ϕ = 38.7511° (Δϕ = −6.2489°). | dav1 = 1.8040 × 10−4 μm drm1 = 1.9528 × 10−4 μm | dav2 = 4.9751 × 10−5 μm drm2 = 5.0771 × 10−5 μm |
| 4 | Ax0 = 5 μm; Ay0 = 10 μm; ϕ0 = 45° | Ax = 4.3924 μm; Ay = 9.4860 μm; ϕ = 36.8911° (Δϕ = −8.1089°). | dav1 = 1.8700 × 10−4 μm drm1 = 2.0069 × 10−4 μm | dav2 = 1.6555 × 10−5 μm drm2 = 1.9665 × 10−5 μm |
| Motion Parameters of Input Elliptical Trajectories | Analyses on Experimental Results | |||
|---|---|---|---|---|
| No. | Expected Output Elliptical Parameters | Actual Input Elliptical Parameters with EPC | Coefficients ω and κ Distance Deviations ξrm | Error Distances with Expected Results |
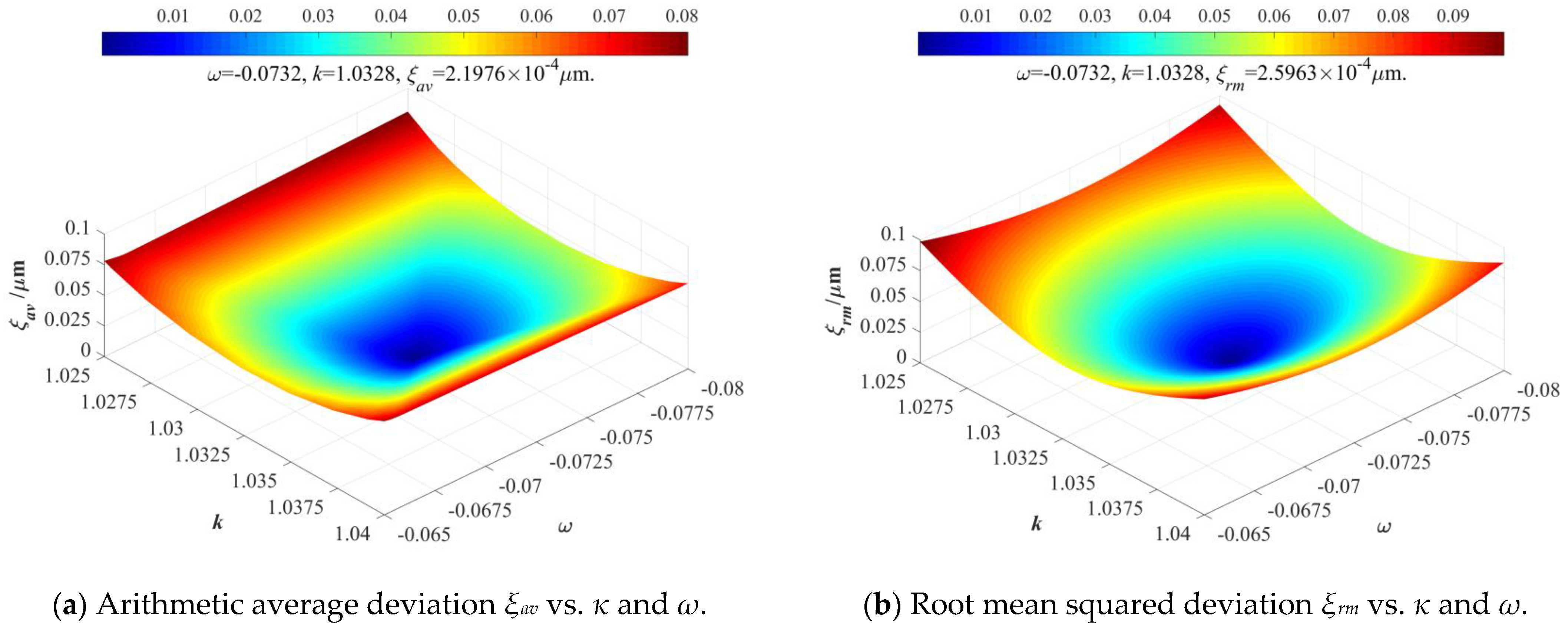
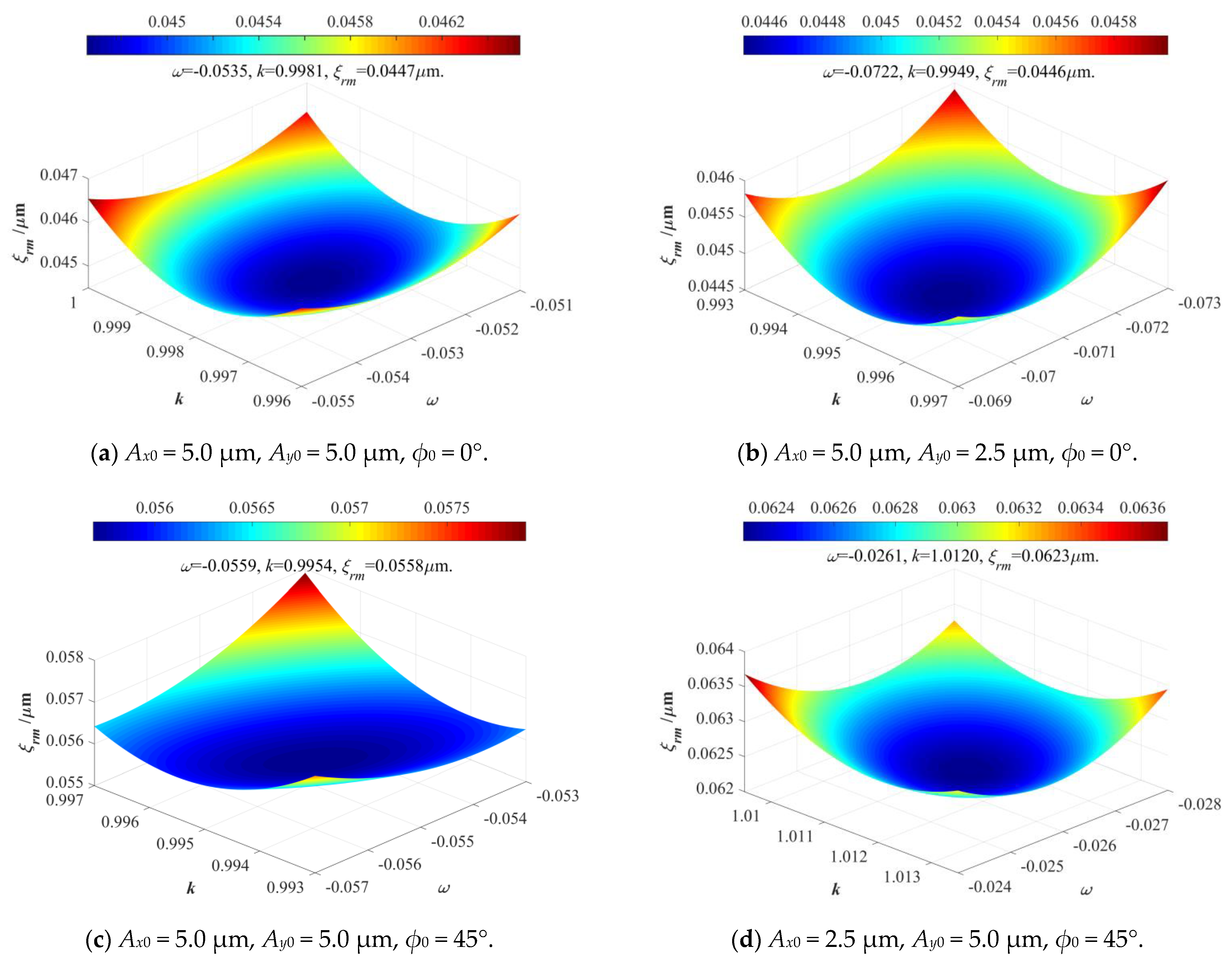
| 1 | Ax0 = 5.0 μm; Ay0 = 5.0 μm; ϕ0 = 0°. | Ax = 5.0311 μm; Ay = 5.0311 μm; ϕ = −6.1248°. (Δϕ = −6.1248°) | ω = −0.0535; κ = 0.9981; ξrm = 0.0447 μm. | dav = 0.0658 μm; drm = 0.0756 μm. |
| 2 | Ax0 = 5.0 μm; Ay0 = 2.5 μm; ϕ0 = 0°. | Ax = 5.0553 μm; Ay = 2.5522 μm; ϕ = −10.2843°. (Δϕ = −10.2842°) | ω = −0.0722; κ = 0.9949; ξrm = 0.0446 μm. | dav = 0.0286 μm; drm = 0.0360 μm. |
| 3 | Ax0 = 5.0 μm; Ay0 = 5.0 μm; ϕ0 = 45° | Ax = 5.2418 μm; Ay = 5.2418 μm; ϕ = 49.3552°. (Δϕ = 4.3552°) | ω = −0.0559; κ = 0.9954; ξrm = 0.0558 μm. | dav = 0.0707 μm; drm = 0.0869 μm. |
| 4 | Ax0 = 2.5 μm; Ay0 = 5.0 μm; ϕ0 = 45° | Ax = 2.3796 μm; Ay = 4.8973 μm; ϕ = 42.1744°. (Δϕ = −2.8256°) | ω = −0.0270; κ = 1.0120; ξrm = 0.0623 μm. | dav = 0.0523 μm; drm = 0.0634 μm. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, R.; Zhou, X.; Meng, H.; Liu, B. A Novel Type of Pseudo-Decoupling Method for Two Degree-of-Freedom Piezoelectrically Driven Compliant Mechanisms Based on Elliptical Parameter Compensation. Micromachines 2023, 14, 2043. https://doi.org/10.3390/mi14112043
Wang R, Zhou X, Meng H, Liu B. A Novel Type of Pseudo-Decoupling Method for Two Degree-of-Freedom Piezoelectrically Driven Compliant Mechanisms Based on Elliptical Parameter Compensation. Micromachines. 2023; 14(11):2043. https://doi.org/10.3390/mi14112043
Chicago/Turabian StyleWang, Rongqi, Xiaoqin Zhou, Haonan Meng, and Baizhi Liu. 2023. "A Novel Type of Pseudo-Decoupling Method for Two Degree-of-Freedom Piezoelectrically Driven Compliant Mechanisms Based on Elliptical Parameter Compensation" Micromachines 14, no. 11: 2043. https://doi.org/10.3390/mi14112043
APA StyleWang, R., Zhou, X., Meng, H., & Liu, B. (2023). A Novel Type of Pseudo-Decoupling Method for Two Degree-of-Freedom Piezoelectrically Driven Compliant Mechanisms Based on Elliptical Parameter Compensation. Micromachines, 14(11), 2043. https://doi.org/10.3390/mi14112043








