Vibrating Flexoelectric Micro-Beams as Angular Rate Sensors
Abstract
:1. Introduction
2. Theory of Flexoelectricity
3. One-Dimensional Equations for a Flexoelectric Beam in Bending Vibrations
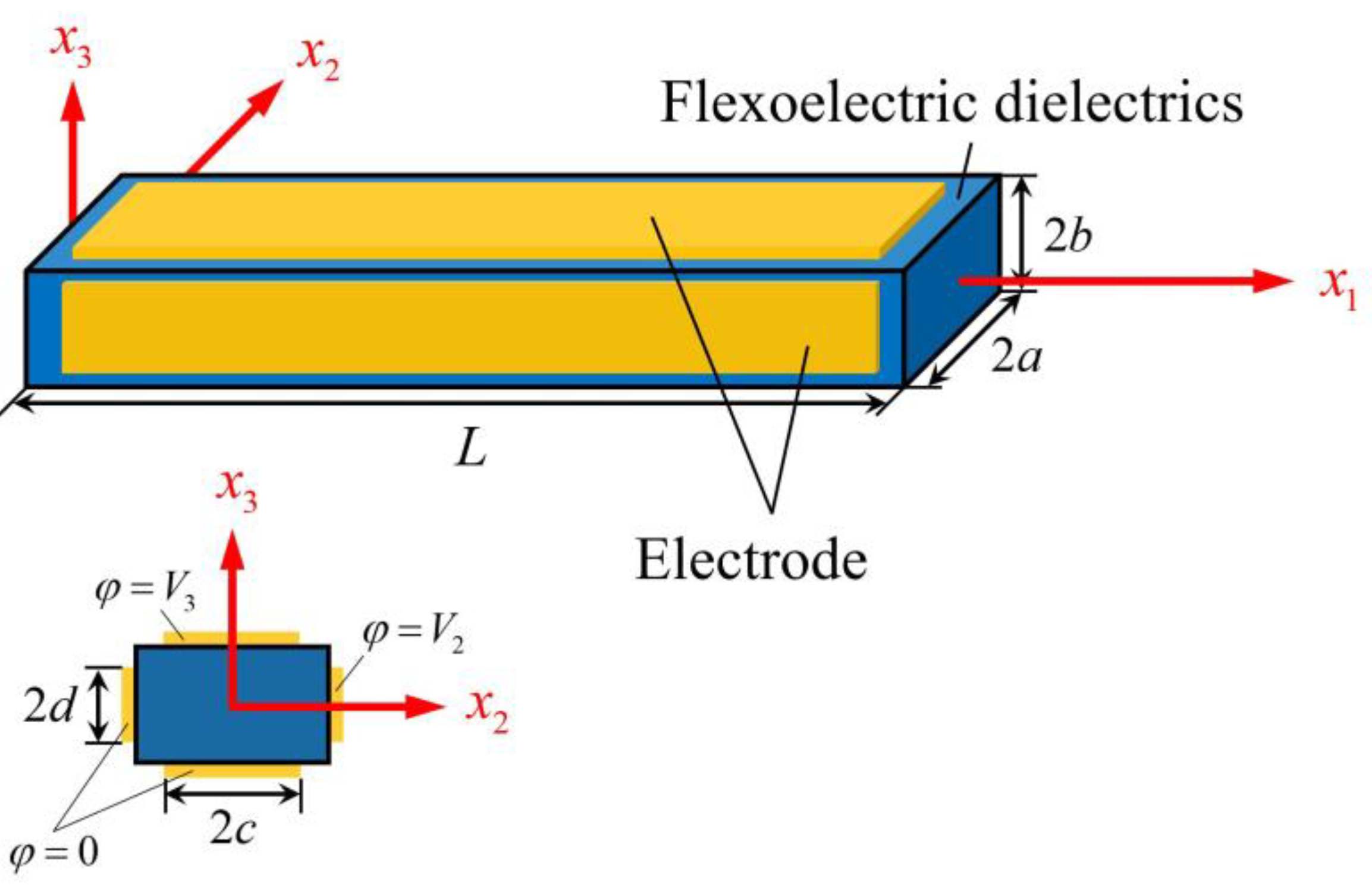
4. Analysis of a Flexoelectric Gyroscope
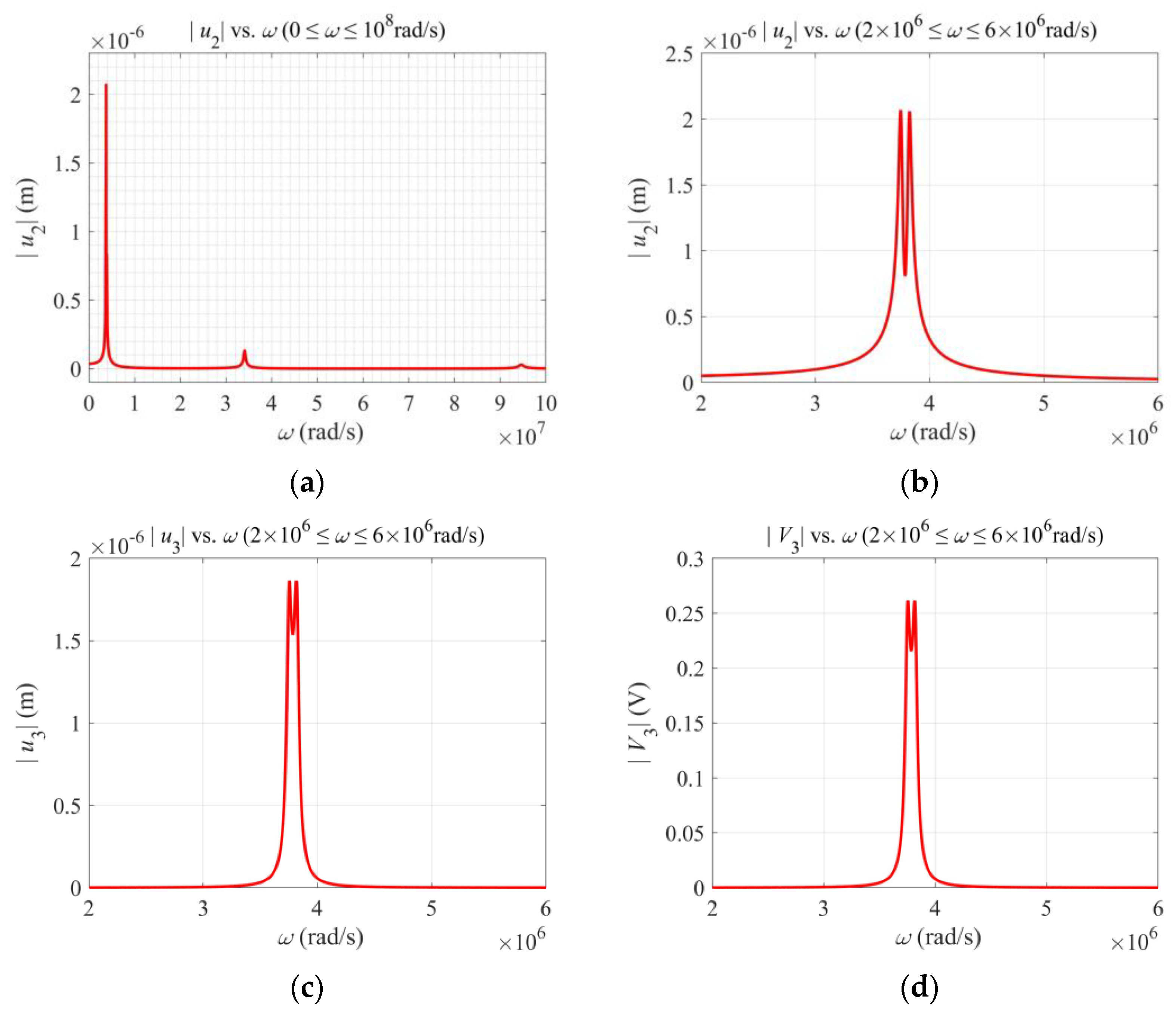
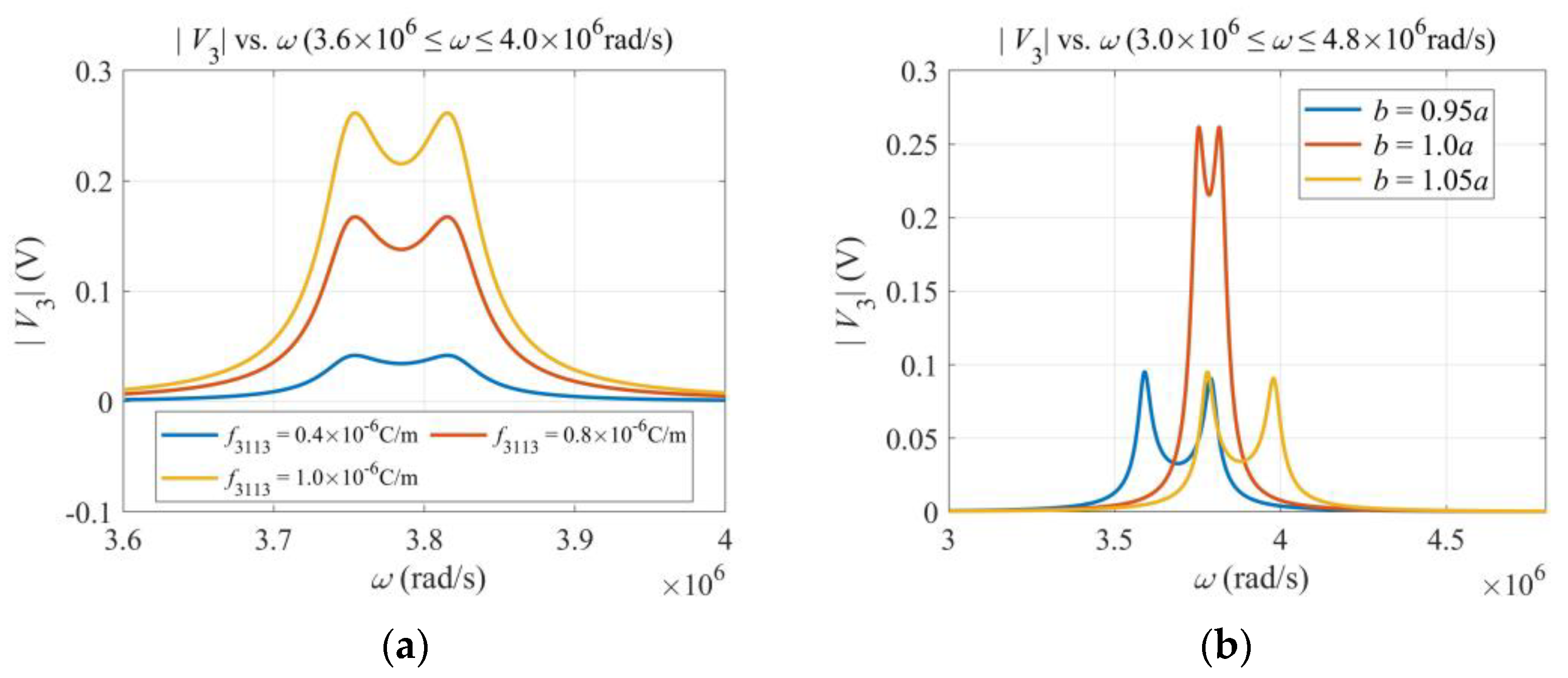
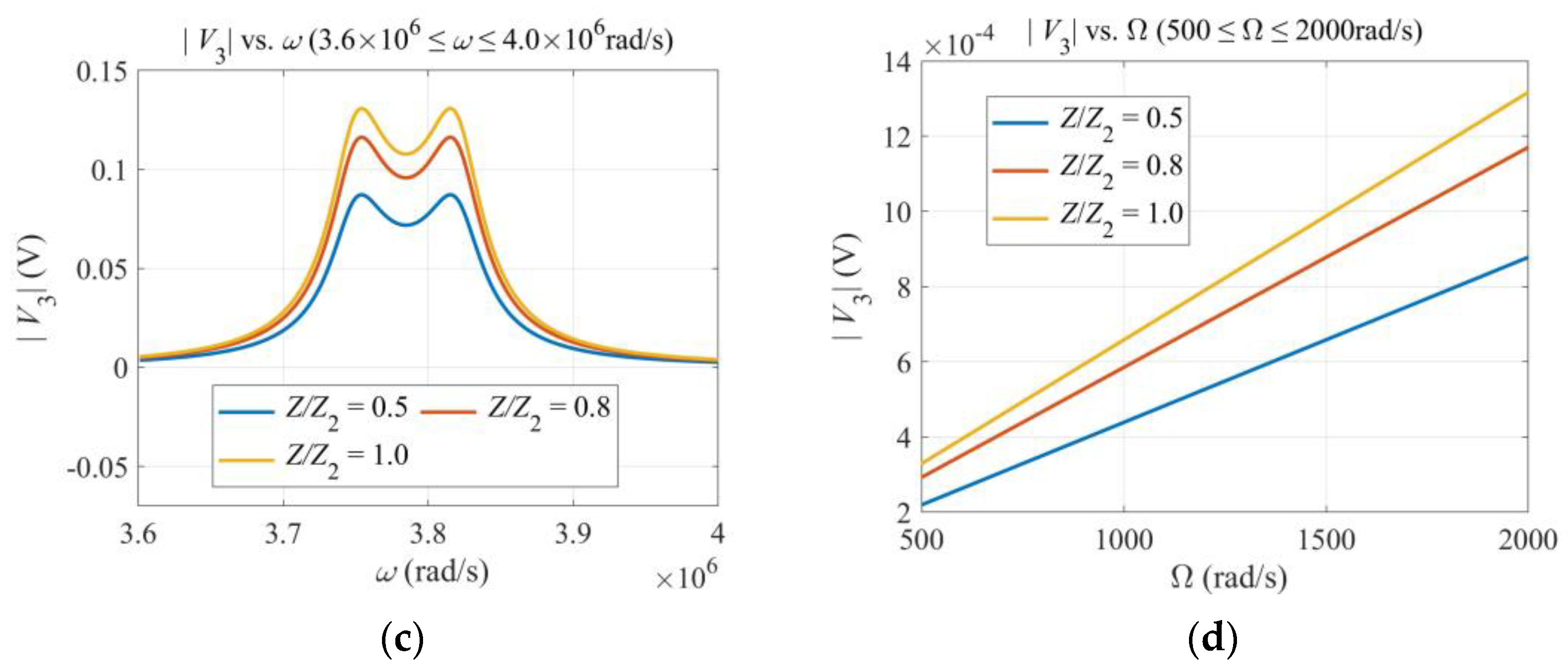
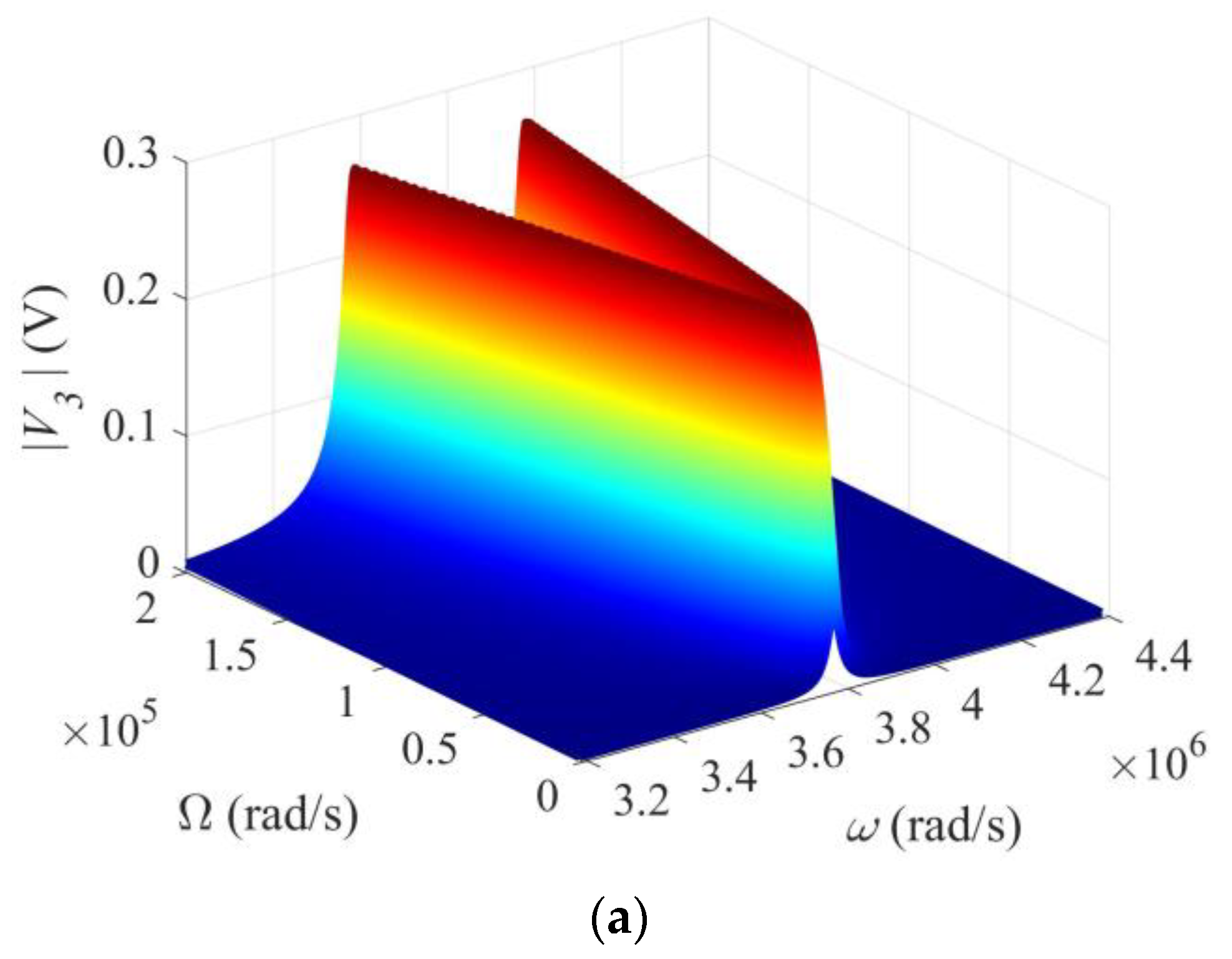
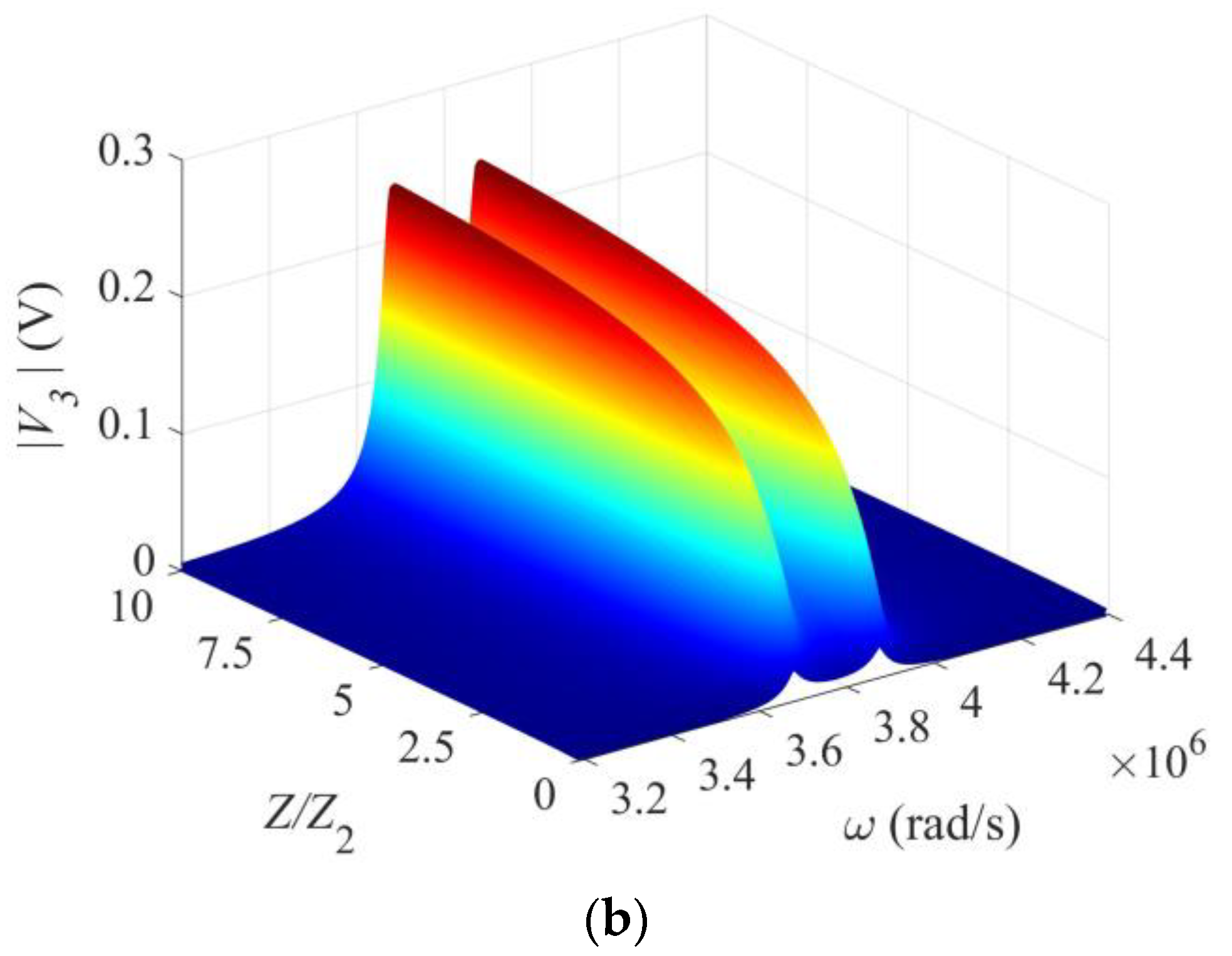
5. Numerical Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Burdess, J.S.; Harris, A.J.; Cruickshank, J.; Wood, D.; Cooper, G. A review of vibratory gyroscopes. Eng. Sci. Edu. J. 1994, 3, 249–254. [Google Scholar] [CrossRef]
- Soderkvist, J. Micromachined gyroscopes. Sens. Actuators A 1994, 43, 65–71. [Google Scholar] [CrossRef]
- Yang, J.S. A review of analyses related to vibrations of rotating piezoelectric bodies and gyroscopes. IEEE Trans. Ultrason. Ferroelect. Freq. Contr. 2005, 52, 698–706. [Google Scholar] [CrossRef] [PubMed]
- Loveday, P.W. Analysis and Compensation of Imperfection Effects in Piezoelectric Vibratory Gyroscopes. Ph.D. Dissertation, Virginia Polytechnic Institute and State University, Blacksburg, VA, USA, 1999. [Google Scholar]
- Fang, H.Y. Vibrations of a Rotating Piezoelectric Body and Applications in Gyroscopes. Ph.D. Dissertation, University of Nebraska–Lincoln, Lincoln, NE, USA, 2000. [Google Scholar]
- Liang, F.; Liang, D.D.; Qian, Y.J. Dynamical analysis of an improved MEMS ring gyroscope encircled by piezoelectric film. Int. J. Mech. Sci. 2020, 187, 105915. [Google Scholar] [CrossRef]
- Hodjat-Shamami, M.; Ayazi, F. Eigenmode operation of piezoelectric resonant gyroscopes. Microsyst. Nanoeng. 2020, 6, 108. [Google Scholar] [CrossRef] [PubMed]
- Obitani, K.; Qian, J.; Tsuchiya, T.; Araya, K.; Yachi, M. Electrode design of single crystal lithium niobate piezoelectric disk gyroscope. In Proceedings of the 2020 IEEE International Symposium on Inertial Sensors and Systems (INERTIAL), Hiroshima, Japan, 23–26 March 2020; pp. 1–2. [Google Scholar]
- Qu, T.; Zhou, G.; Xue, X.; Teng, J. Cylindrical shell vibration gyroscope excited and detected by high-temperature-sintered piezoelectric ceramic electrodes. Sensors 2020, 20, 5972. [Google Scholar] [CrossRef] [PubMed]
- Larkin, K.; Ghommem, M.; Serrano, M.; Abdelkefi, A. A review on vibrating beam-based micro/nano-gyroscopes. Microsyst. Technol. 2021, 27, 4157–4181. [Google Scholar] [CrossRef]
- Gates, W.D. Vibrating angular rate sensor may threaten the gyroscope. Electronics 1968, 41, 130–134. [Google Scholar]
- Soderkvist, J. Piezoelectric beams and vibrating angular rate sensors. IEEE Trans. Ultrason. Ferroelec. Freq. Contr. 1991, 38, 271–280. [Google Scholar] [CrossRef]
- Yang, J.S.; Fang, H.Y. Analysis of a rotating elastic beam with piezoelectric films as an angular rate sensor. IEEE Trans. Ultrason. Ferroelec. Freq. Contr. 2002, 49, 798–804. [Google Scholar] [CrossRef] [PubMed]
- Sahin, E.; Dost, S. A strain-gradients theory of elastic dielectrics with spatial dispersion. Int. J. Engng. Sci. 1988, 26, 1231–1245. [Google Scholar] [CrossRef]
- Tagantsev, A.K. Piezoelectricity and flexoelectricity in crystalline dielectrics. Phys. Rev. B 1986, 32, 5883–5889. [Google Scholar] [CrossRef] [PubMed]
- Shen, S.; Hu, S. A theory of flexoelectricity with surface effect for elastic dielectrics. J. Mech. Phys. Solids 2010, 58, 665–677. [Google Scholar] [CrossRef]
- Zhang, R.; Liang, X.; Shen, S. A Timoshenko dielectric beam model with flexoelectric effect. Meccanica 2015, 51, 1181–1188. [Google Scholar] [CrossRef]
- Zhou, Z.D.; Yang, C.P.; Su, Y.X.; Huang, R.; Lin, X.L. Electromechanical coupling in piezoelectric nanobeams due to the flexoelectric effect. Smart Mater. Struct. 2017, 26, 095025. [Google Scholar] [CrossRef]
- Hu, Y.T.; Wang, J.N.; Yang, F.; Xue, H.; Hu, H.P.; Wang, J. The effect of first-order strain gradient in micro piezoelectric-bimorph power harvester. IEEE Trans. Ultrason. Ferroelec. Freq. Contr. 2011, 58, 849–852. [Google Scholar]
- Qu, Y.L.; Jin, F.; Yang, J.S. Buckling of flexoelectric semiconductor beams. Acta Mech. 2021, 232, 2623–2633. [Google Scholar] [CrossRef]
- Yang, W.J.; Liang, X.; Shen, S.P. Electromechanical responses of piezoelectric nanoplates with flexoelectricity. Acta Mech. 2015, 226, 3097–3110. [Google Scholar] [CrossRef]
- Ray, M.C. Mesh free model of nanobeam integrated with a flexoelectric actuator layer. Compos. Struct. 2017, 159, 63–71. [Google Scholar] [CrossRef]
- Fan, M.; Min, H. Active actuating of a simply supported beam with the flexoelectric effect. Materials 2020, 13, 1735. [Google Scholar] [CrossRef] [Green Version]
- Mase, G.T.; Mase, G.E. Continuum Mechanics for Engineers, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1990. [Google Scholar]
- Auld, B.A. Acoustic Fields and Waves in Solids; Wiley: New York, NY, USA, 1973. [Google Scholar]
- Shu, L.; Wei, X.; Pang, T.; Yao, X.; Wang, C. Symmetry of flexoelectric coefficients in crystalline medium. J. Appl. Phys. 2011, 110, 104106. [Google Scholar] [CrossRef]
- Zaki, N.A.F.; Aziz, A.A.; Khairudin, N.; Burham, N. Simulation of zinc oxide, barium sodium niobate, and barium titanate as lead-free piezoelectric materials. In Proceedings of the 2021 IEEE Regional Symposium on Micro and Nanoelectronics (RSM), Kuala Lumpur, Malaysia, 2–4 August 2021; pp. 38–41. [Google Scholar]
- Bhaskar, U.; Banerjee, N.; Abdollahi, A.; Wang, Z.; Schlom, D.G.; Rijnders, G.; Catalan, G. A flexoelectric microelectromechanical system on silicon. Nat. Nanotech. 2016, 11, 263–266. [Google Scholar] [CrossRef] [PubMed]
- Yamaguchi, H.; Tatami, J.; Iijima, M. Measurement of mechanical properties of BaTiO3 layer in multilayered ceramic capacitor using a microcantilever beam specimen. J. Ceram. Soc. Jpn. 2019, 127, 335–338 2019. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qu, Y.; Jin, F.; Yang, J. Vibrating Flexoelectric Micro-Beams as Angular Rate Sensors. Micromachines 2022, 13, 1243. https://doi.org/10.3390/mi13081243
Qu Y, Jin F, Yang J. Vibrating Flexoelectric Micro-Beams as Angular Rate Sensors. Micromachines. 2022; 13(8):1243. https://doi.org/10.3390/mi13081243
Chicago/Turabian StyleQu, Yilin, Feng Jin, and Jiashi Yang. 2022. "Vibrating Flexoelectric Micro-Beams as Angular Rate Sensors" Micromachines 13, no. 8: 1243. https://doi.org/10.3390/mi13081243
APA StyleQu, Y., Jin, F., & Yang, J. (2022). Vibrating Flexoelectric Micro-Beams as Angular Rate Sensors. Micromachines, 13(8), 1243. https://doi.org/10.3390/mi13081243





