Mechanical Behavior and Constitutive Model Characterization of Optically Clear Adhesive in Flexible Devices
Abstract
1. Introduction
2. Constitutive Model
2.1. Hyperelastic Constitutive Models
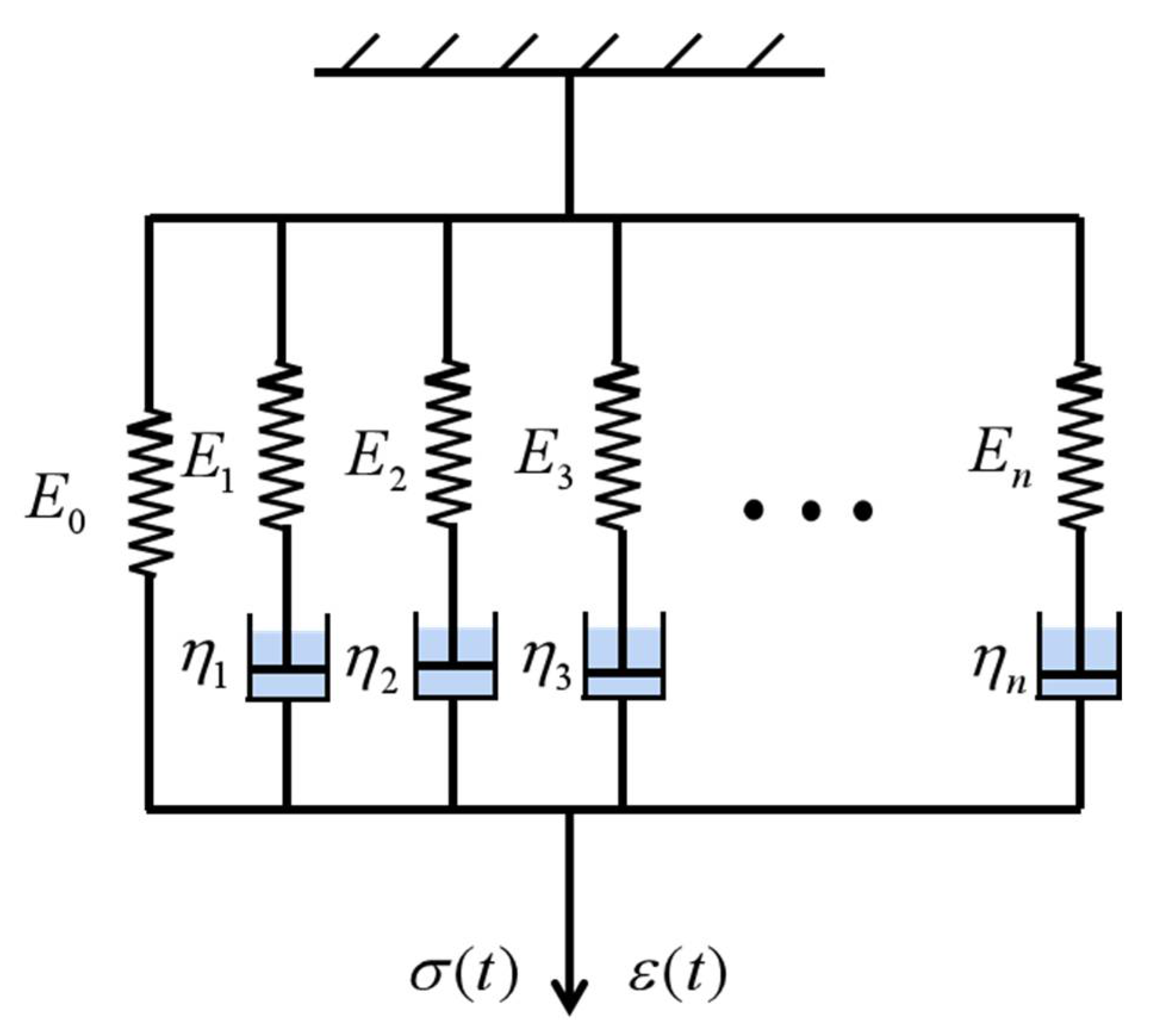
2.2. Viscoelastic Constitutive Models
3. Materials and Methods
3.1. Sample Preparation
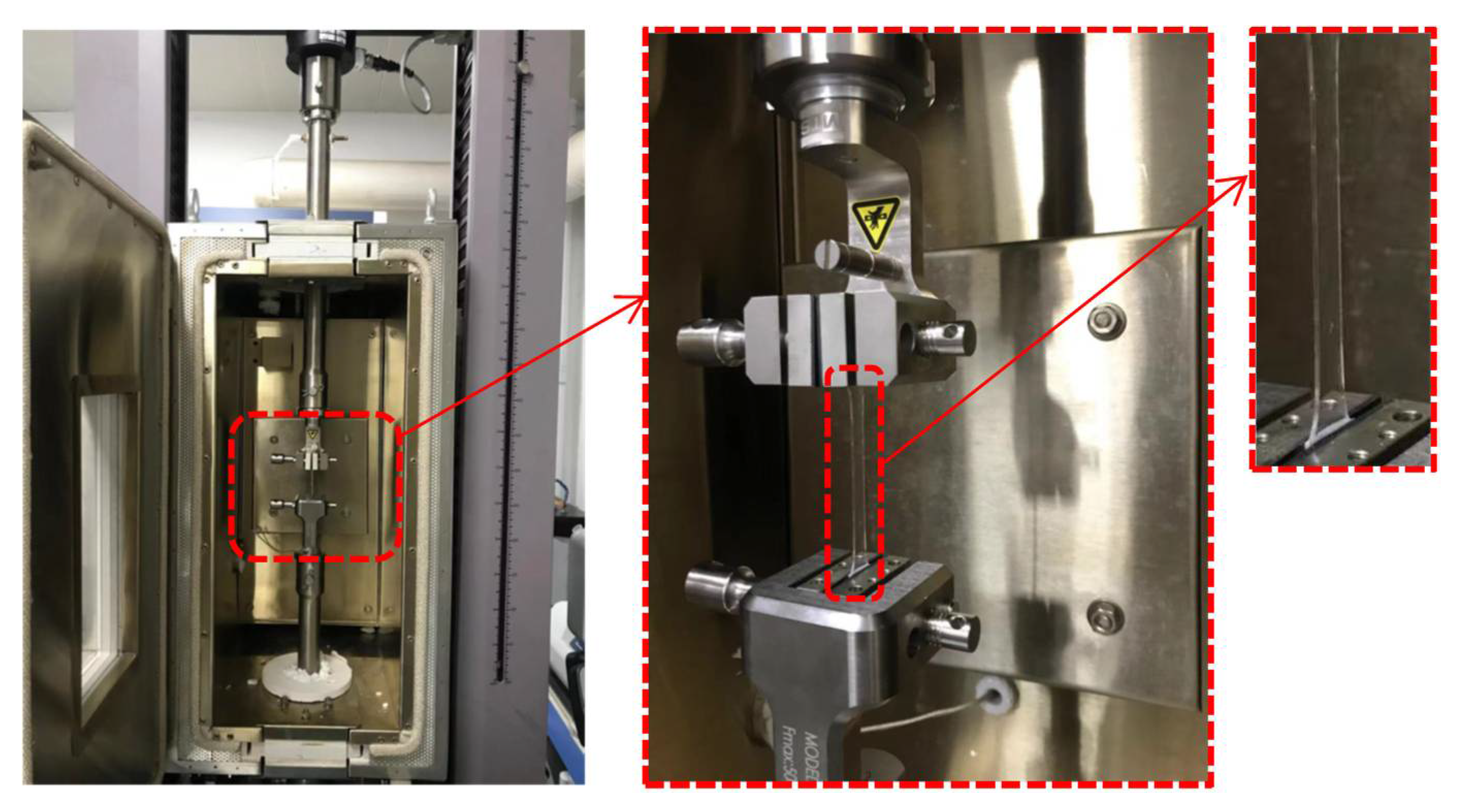
3.2. Experimental Procedure
3.2.1. Hyperelastic Experiment
3.2.2. Viscoelastic Experiment
4. Results and Discussion
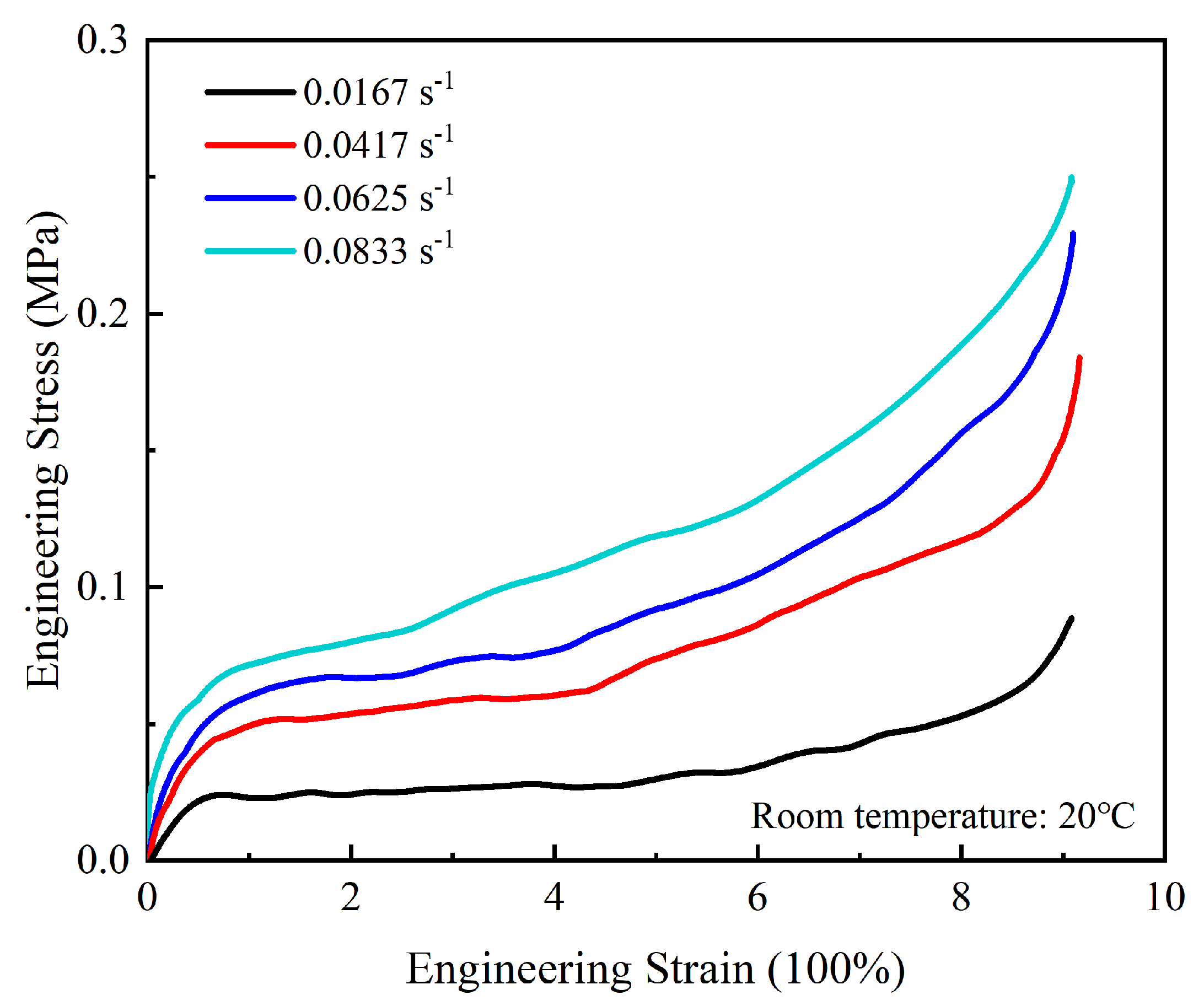
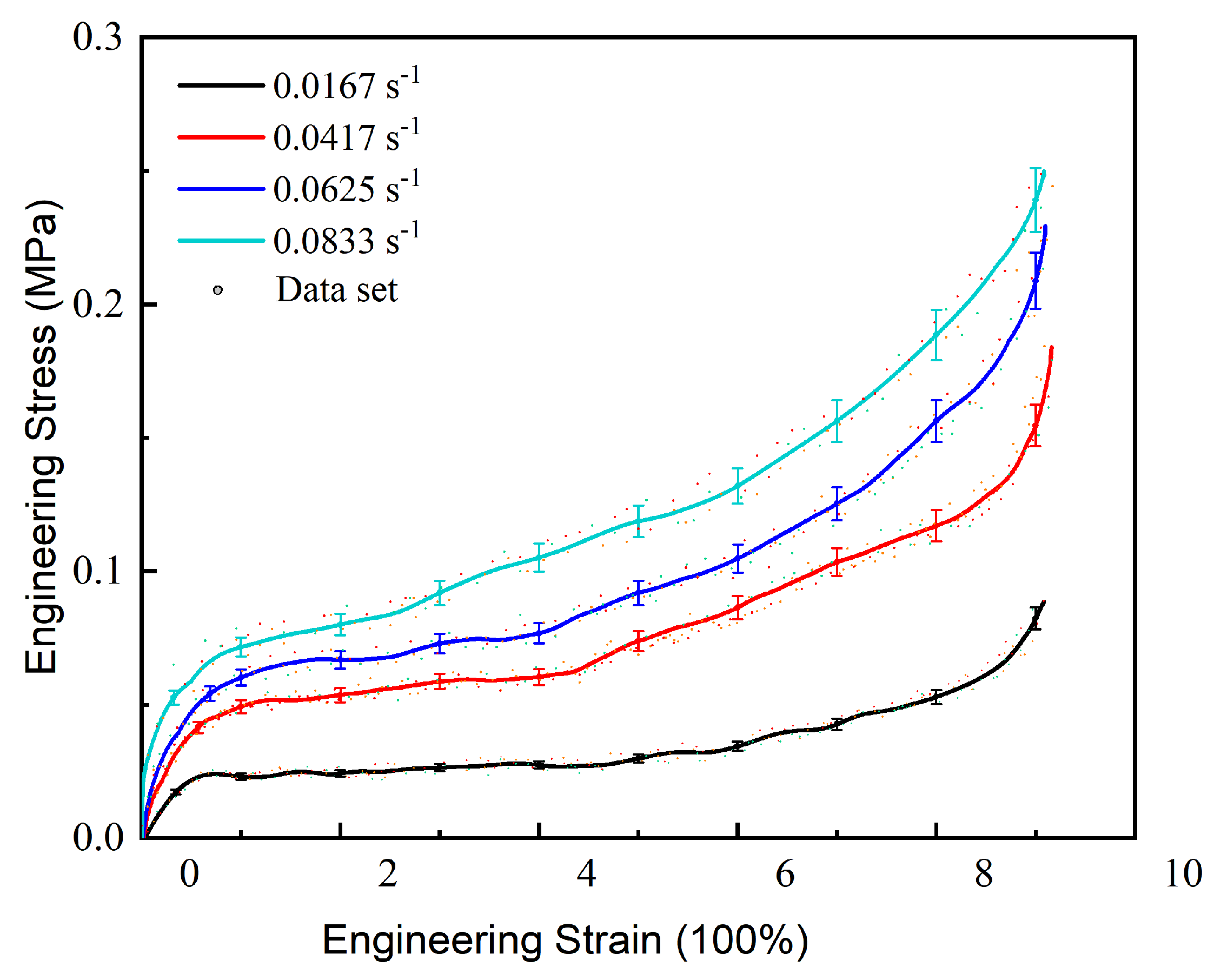
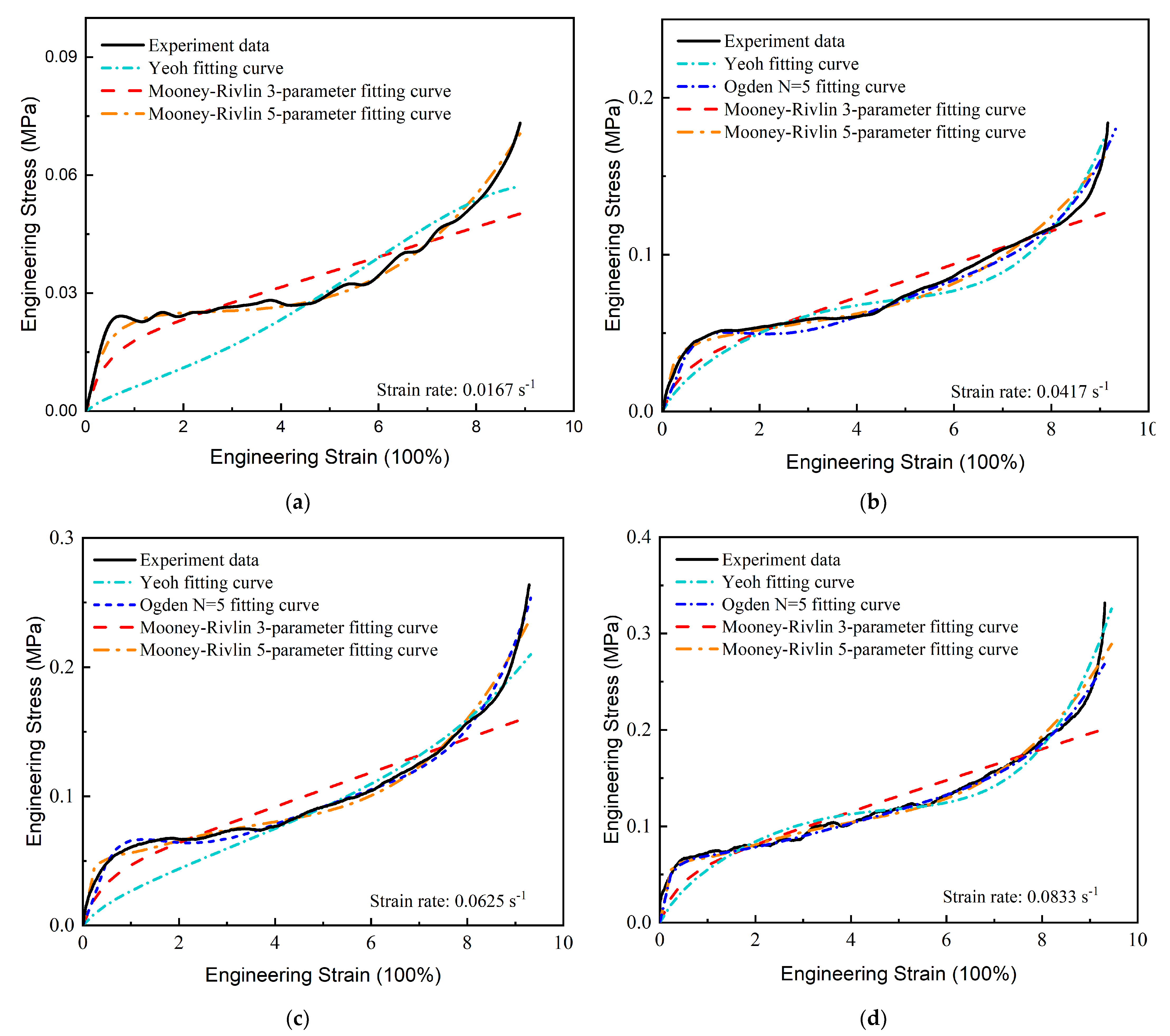
4.1. Hyperelasticity of OCA Material
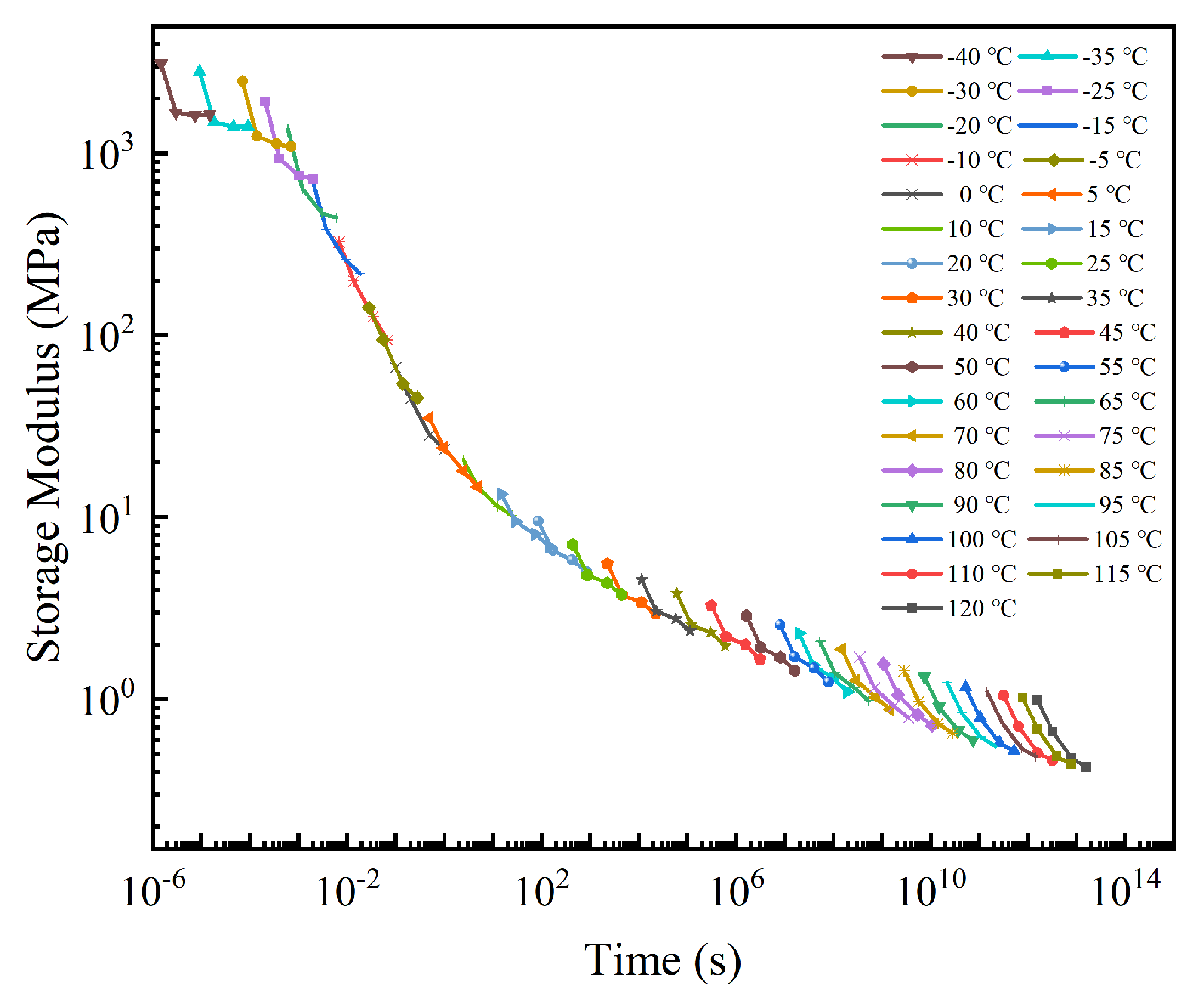
4.2. Static Viscoelasticity of OCA Material
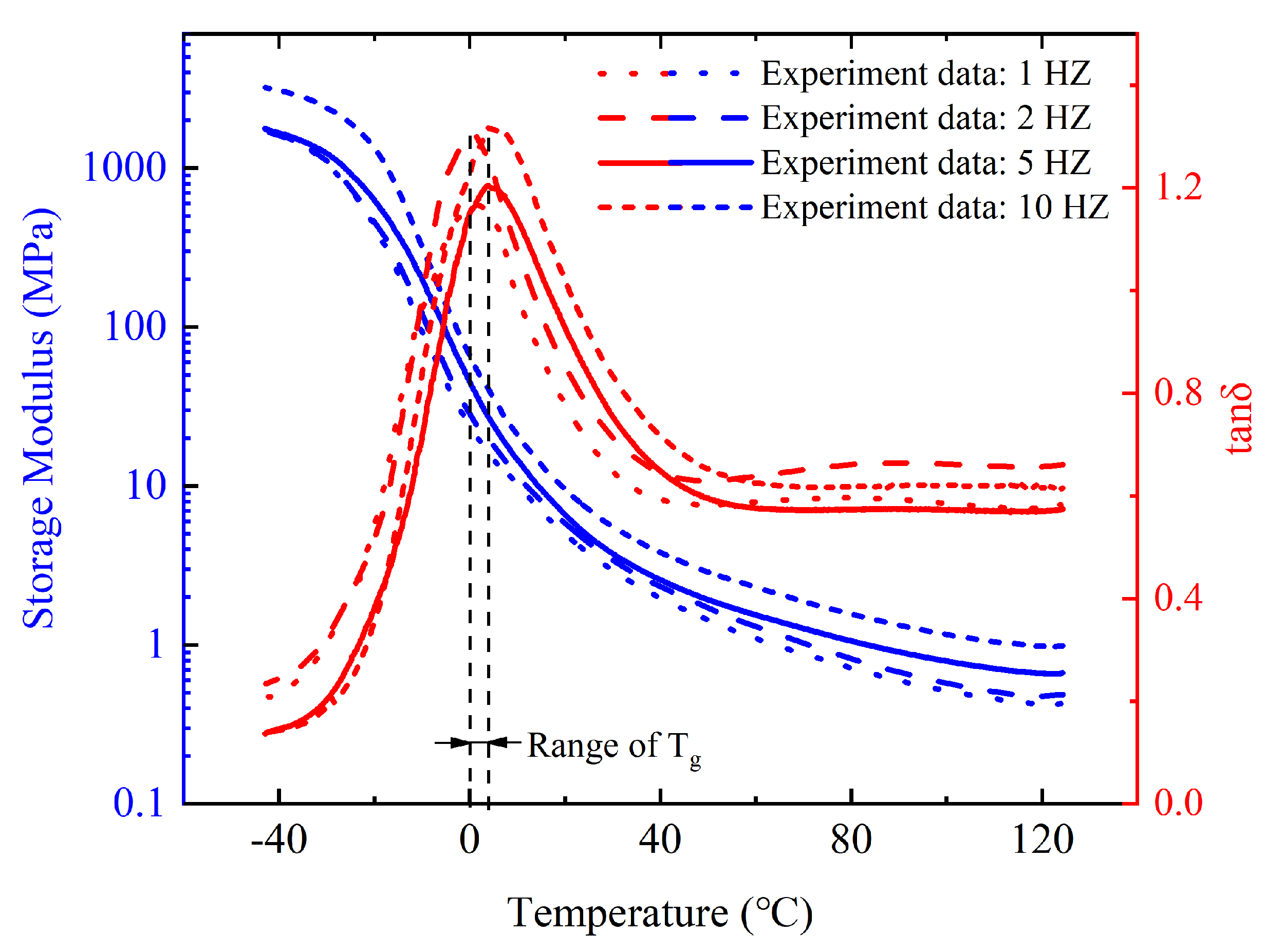
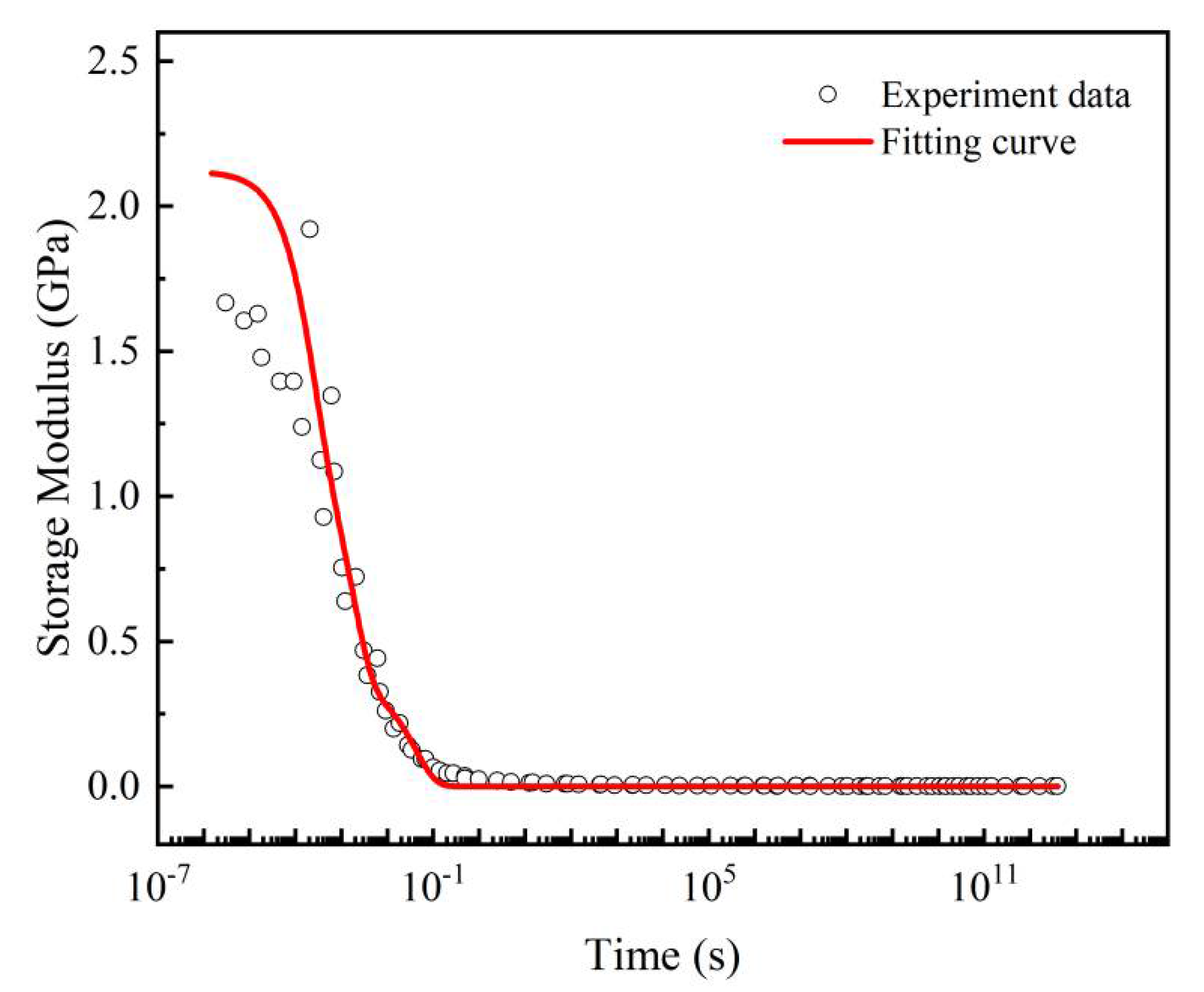
4.3. Dynamic Viscoelasticity of OCA Material
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chiu, T.C.; Yeh, E.Y. Warpage simulation for the reconstituted wafer used in fan-out wafer level packaging. Microelectron. Reliab. 2018, 80, 14–23. [Google Scholar] [CrossRef]
- Sarvar, F.; Hutt, D.A.; Whalley, D.C. Application of adhesives in MEMS and MOEMS assembly: A review. In Proceedings of the 2nd International IEEE Conference on Polymers and Adhesives in Microelectronics and Photonics, Zalaegerszeg, Hungary, 23–26 June 2002; pp. 22–28. [Google Scholar]
- Priyadarshi, A.; Shimin, L.; Mhaisalkar, S.G.; Rajoo, R.; Wong, E.H.; Kripesh, V.; Namdas, E.B. Characterization of optical properties of acrylate based adhesives exposed to different temperature conditions. J. Appl. Polym. Sci. 2010, 98, 950–956. [Google Scholar] [CrossRef]
- Leo, K. Organic light-emitting diodes: Efficient and flexible solution. Nat. Photonics 2011, 5, 716–718. [Google Scholar] [CrossRef]
- Usafsson, H.; Cao, Y.; Racy, M.; Kavr, F.; Hrvol, A.J. Flexible light-emitting diodes made from soluble conducting polymers. Nature 1992, 357, 477–479. [Google Scholar]
- Min, S.H.; Kang, M.K.; Kim, I.Y.; Kim, H.S.; Chang, K.K. A study on flexible OLED employing cellulose paper as a substrate. Mol. Cryst. Liq. Cryst. 2012, 563, 159–165. [Google Scholar] [CrossRef]
- Chwang, A.B.; Rothman, M.A.; Mao, S.Y.; Hewitt, R.H.; Weaver, M.S.; Silvernail, J.A.; Rajan, K.; Hack, M.; Brown, J.J.; Chu, X.; et al. Thin film encapsulated flexible organic electroluminescent displays. Appl. Phys. Lett. 2003, 83, 413–415. [Google Scholar] [CrossRef]
- Li, L.; Yu, Z.; Hu, W.L.; Chang, C.H.; Chen, Q.; Pei, Q.B. Efficient flexible phosphorescent polymer light-emitting diodes based on silver nanowire-polymer composite electrode. Adv. Mater. 2011, 23, 5563–5567. [Google Scholar] [CrossRef]
- Jin, D.U.; Kim, T.W.; Koo, H.W.; Stryakhilev, D.; Kim, H.S.; Seo, S.J.; Kim, M.J.; Min, H.K.; Chung, H.K.; Kim, S.S. Highly robust flexible amoled display on plastic substrate with new structure. SID Int. Symp. Dig. Tech. Pap. 2010, 41, 703–705. [Google Scholar] [CrossRef]
- Campbell, C.J.; Clapper, J.; Behling, R.E.; Erdogan, B.; Beagi, H.Z.; Abrahamson, J.T.; Everaerts, A.I. P-198: Optically clear adhesives enabling foldable and flexible OLED displays. SID Int. Symp. Dig. Tech. Pap. 2017, 48, 2009–2011. [Google Scholar] [CrossRef]
- Wang, H.; Deng, X.; Wu, H.; Pi, A.; Huang, F. Investigating the dynamic mechanical behaviors of polyurea through experimentation and modeling. Def. Technol. 2019, 15, 10. [Google Scholar] [CrossRef]
- Qian, Z.F.; Wang, J.J.; Yang, J.; Liu, S. Visco-elastic-plastic properties and constitutive modeling of underfills. IEEE Trans. Compon. Packag. Technol. 1999, 22, 152–157. [Google Scholar] [CrossRef]
- Fu, X.; Wang, Z.; Ma, L.; Zou, Z.; Zhang, Z.; Guan, X. Temperature-Dependence of rubber hyperelasticity based on the eight-chain model. Polymers 2020, 12, 932. [Google Scholar] [CrossRef] [PubMed]
- Yeh, M.; Chang, L.; Cheng, H.; Wang, P. Bending stress analysis of laminated foldable touch panel. Procedia Eng. 2014, 79, 189–193. [Google Scholar] [CrossRef][Green Version]
- Salmon, F.; Everaerts, A.; Campbell, C.; Pennington, B.; Erdogan-Haug, B.; Caldwell, G. 64-1: Modeling the mechanical performance of a foldable display panel bonded by 3M optically clear adhesives. SID Int. Symp. Dig. Tech. Pap. 2017, 48, 938–941. [Google Scholar] [CrossRef]
- Ha, M.H.; Choi, J.K.; Park, B.M.; Han, K.Y. Highly flexible cover window using ultra-thin glass for foldable displays. J. Mech. Sci. Technol. 2021, 35, 661–668. [Google Scholar] [CrossRef]
- Yunsik, C.; Chae, G.S.; Youn, Y.O.; Woo, S.; Shin, S.K.; Lee, J. Optimal design of thickness and Young’s modulus of multi-layered foldable structure considering bending stress, neutral plane and delamination under 2.5 mm radius of curvature. Int. J. Precis. Eng. Manuf. 2018, 19, 1143–1154. [Google Scholar]
- Cheng, A.; Chen, Y.; Jin, J.; Su, T. Study on mechanical behavior and effect of adhesive layers in foldable AMOLED display by finite element analysis. SID Symp. Dig. Tech. Pap. 2019, 50, 1060–1063. [Google Scholar] [CrossRef]
- Nishimura, M.; Hishinuma, M.; Yamaguchi, H.; Murayama, A. Quantitative evaluation of neutral-plane splitting in foldable displays using folding stiffness measurements and finite element method simulations. SID Symp. Dig. Tech. Pap. 2020, 56, 834–837. [Google Scholar] [CrossRef]
- Ma, L.; Gu, J. 3D bending simulation and mechanical properties of the OLED bending area. Open Phys. 2020, 18, 397–407. [Google Scholar] [CrossRef]
- Suchocki, C.; Jemioo, S. Polyconvex hyperelastic modeling of rubberlike materials. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 352. [Google Scholar] [CrossRef]
- Huang, Z.P. A novel constitutive formulation for rubberlike materials in thermoelasticity. J. Appl. Mech. 2014, 81, 041013. [Google Scholar] [CrossRef]
- Beda, T.; Chevalier, Y. Hybrid continuum model for large elastic deformation of rubber. J. Appl. Phys. 2003, 94, 2701–2706. [Google Scholar] [CrossRef]
- Thanakhun, K.; Puttapitukporn, T. PDMS material models for anti-fouling surfaces using finite element method. Eng. J. 2019, 23, 381–398. [Google Scholar] [CrossRef]
- Darijani, H.; Naghdabadi, R. Hyperelastic materials behavior modeling using consistent strain energy density functions. Acta Mech. 2010, 213, 235–254. [Google Scholar] [CrossRef]
- Yeoh, O.H. Some forms of the strain energy function for rubber. Rubber Chem. Technol. 2012, 66, 754–771. [Google Scholar] [CrossRef]
- Mao, Y.; Li, Y.; Chen, Y.; Miao, Y.; Deng, Q.; Niu, W. Hyperelastic behavior of two rubber materials under quasistatic and dynamic compressive loadings-testing, modeling and application. Polimery 2015, 60, 516–522. [Google Scholar] [CrossRef]
- Dorfmann, A.; Ogden, R.W. Nonlinear electroelastic deformations. J. Elast. 2006, 82, 99–127. [Google Scholar] [CrossRef]
- Peng, M.; Xu, Z. Research on nonlinear constitutive relationship of permanent deformation in asphalt pavements. Sci. China Ser. G Phys. Mech. Astron. 2006, 49, 671–682. [Google Scholar] [CrossRef]
- Renaud, F.; Dion, J.; Chevallier, G.; Tawfiq, I.; Lemaire, R. A new identification method of viscoelastic behavior: Application to the generalized Maxwell model. Mech. Syst. Signal Processing 2011, 25, 991–1010. [Google Scholar] [CrossRef]
- Zhao, B.; Hu, J.; Chen, W.; Chen, J.; Jing, Z. A nonlinear uniaxial stress-strain constitutive model for viscoelastic membrane materials. Polym. Test. 2020, 90, 106633. [Google Scholar] [CrossRef]
- Ghaffari, S.; Ng, E.J.; Ahn, C.H.; Yang, Y.; Wang, S.; Hong, V.A.; Kenny, T.W. Accurate modeling of quality factor behavior of complex silicon MEMS resonators. J. Microelectromech. Syst. 2015, 24, 276–288. [Google Scholar] [CrossRef]
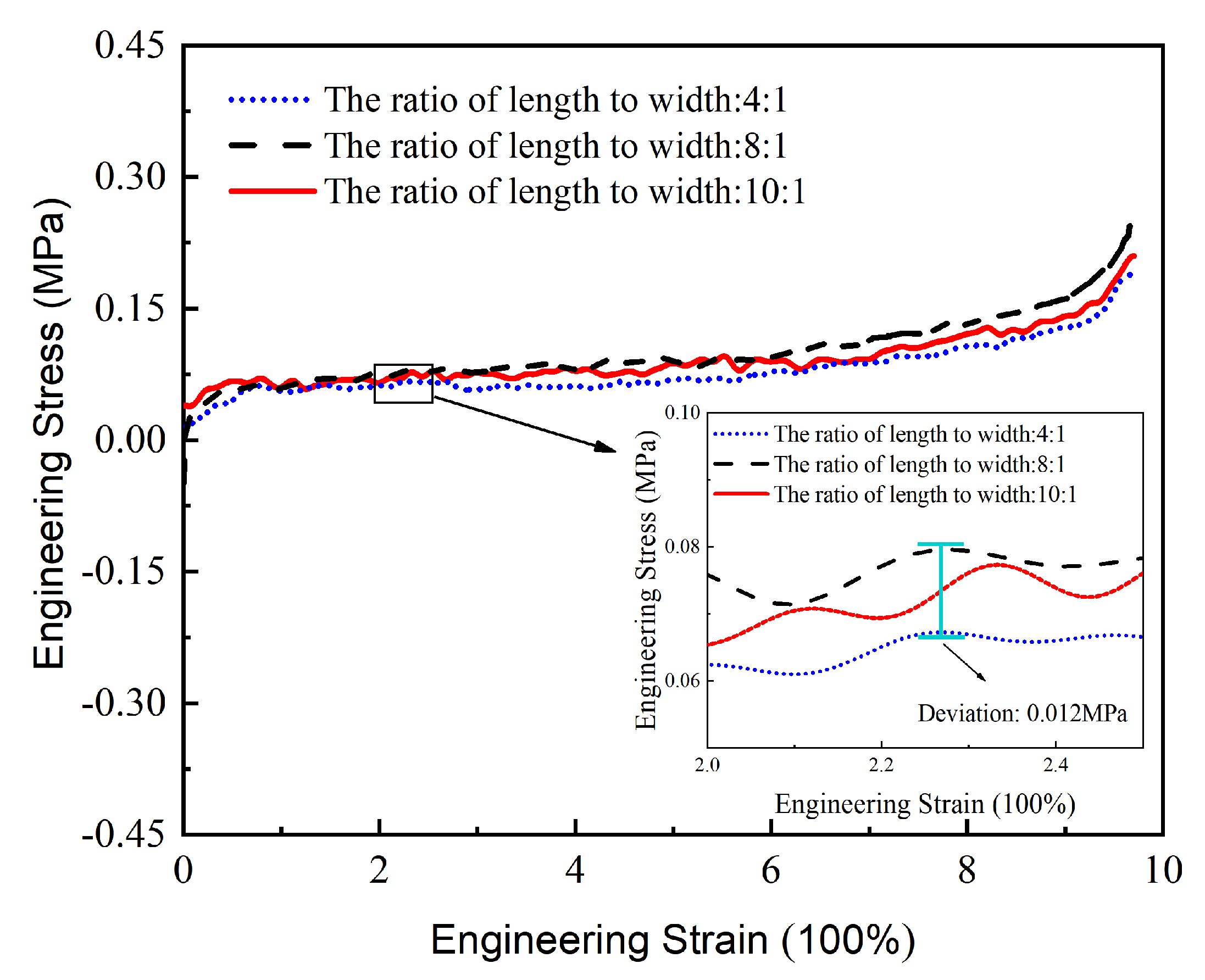





| ID | Length (mm) | Width (mm) | Thickness (mm) | Length to Width Ratio |
|---|---|---|---|---|
| 1 | 40 | 10 | 1 | 4:1 |
| 2 | 40 | 5 | 1 | 8:1 |
| 3 | 50 | 5 | 1 | 10:1 |
| Strain Rate/s−1 | Order | Fitting Parameters | |||
|---|---|---|---|---|---|
| 0.0167 | 5-parameter | C10 = −1.357 × 10−4 C20 = 3.454 × 10−5 | C01 = 1.302 × 10−2 C11 = −2.053 × 10−4 | C02 = 3.639 × 10−4 | D1 = 0 D2 = 0 |
| 0.0417 | 5-parameter | C10 = −4.258 × 10−2 C20 = 7.826 × 10−5 | C01 = 8.166 × 10−2 C11 = −6.182 × 10−4 | C02 = 1.284 × 10−2 | D1 = 0 D2 = 0 |
| 0.0625 | 5-parameter | C10 = −0.1214 C20 = 1.966 × 10−4 | C01 = 0.1885 C11 = −1.961 × 10−3 | C02 = 3.749 × 10−2 | D1 = 0 D2 = 0 |
| 0.0833 | 5-parameter | C10 = −0.1616 C20 = 2.270 × 10−4 | C01 = 0.2463 C11 = −2.385 × 10−3 | C02 = 4.976 × 10−2 | D1 = 0 D2 = 0 |
| Temperature (°C) | i | 1 | 2 | 3 |
|---|---|---|---|---|
| 30 | gi | 0.4248 | 0.2436 | 0.3315 |
| τi | 14.368 | 37.209 | 161.71 | |
| 60 | gi | 0.8166 | 0.1666 | - |
| τi | 15.034 | 203.425 | - | |
| 85 | gi | 0.6172 | 0.2256 | 0.1563 |
| τi | 16.281 | 118.25 | 236.26 |
| i | 1 | 2 | 3 | |
|---|---|---|---|---|
| Prony Series Parameters | gi | 0.171 | 0.222 | 0.0713 |
| τi | 2.19 × 10−4 | 1.77 × 10−3 | 0.0910 |
| WLF | C1 | C2 | Residual Sum of Squares |
| −81.3 | 550.4 | 0.0014 | |
| Arrhenius | k | b | Residual Sum of Squares |
| 8.98 | −0.00231 | 0.0032 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Wang, S.; Dong, F.; Sun, Y.; Sheng, C.; Ma, K.; Tian, Z.; Qian, Z.; Wong, C.; Liu, S. Mechanical Behavior and Constitutive Model Characterization of Optically Clear Adhesive in Flexible Devices. Micromachines 2022, 13, 301. https://doi.org/10.3390/mi13020301
Zhang Y, Wang S, Dong F, Sun Y, Sheng C, Ma K, Tian Z, Qian Z, Wong C, Liu S. Mechanical Behavior and Constitutive Model Characterization of Optically Clear Adhesive in Flexible Devices. Micromachines. 2022; 13(2):301. https://doi.org/10.3390/mi13020301
Chicago/Turabian StyleZhang, Yuexin, Shizhao Wang, Fang Dong, Yameng Sun, Can Sheng, Kun Ma, Zhiqiang Tian, Zhengfang Qian, Chingping Wong, and Sheng Liu. 2022. "Mechanical Behavior and Constitutive Model Characterization of Optically Clear Adhesive in Flexible Devices" Micromachines 13, no. 2: 301. https://doi.org/10.3390/mi13020301
APA StyleZhang, Y., Wang, S., Dong, F., Sun, Y., Sheng, C., Ma, K., Tian, Z., Qian, Z., Wong, C., & Liu, S. (2022). Mechanical Behavior and Constitutive Model Characterization of Optically Clear Adhesive in Flexible Devices. Micromachines, 13(2), 301. https://doi.org/10.3390/mi13020301






