Tailoring the Topological Charge of a Superposition of Identical Parallel Laguerre–Gaussian Beams
Abstract
1. Introduction
2. TC of the Superposition of Identical Parallel LG Beams in the Initial Plane
3. TC of the Superposition of Parallel Identical LG Beams with Different Weight Coefficients in the Far Field
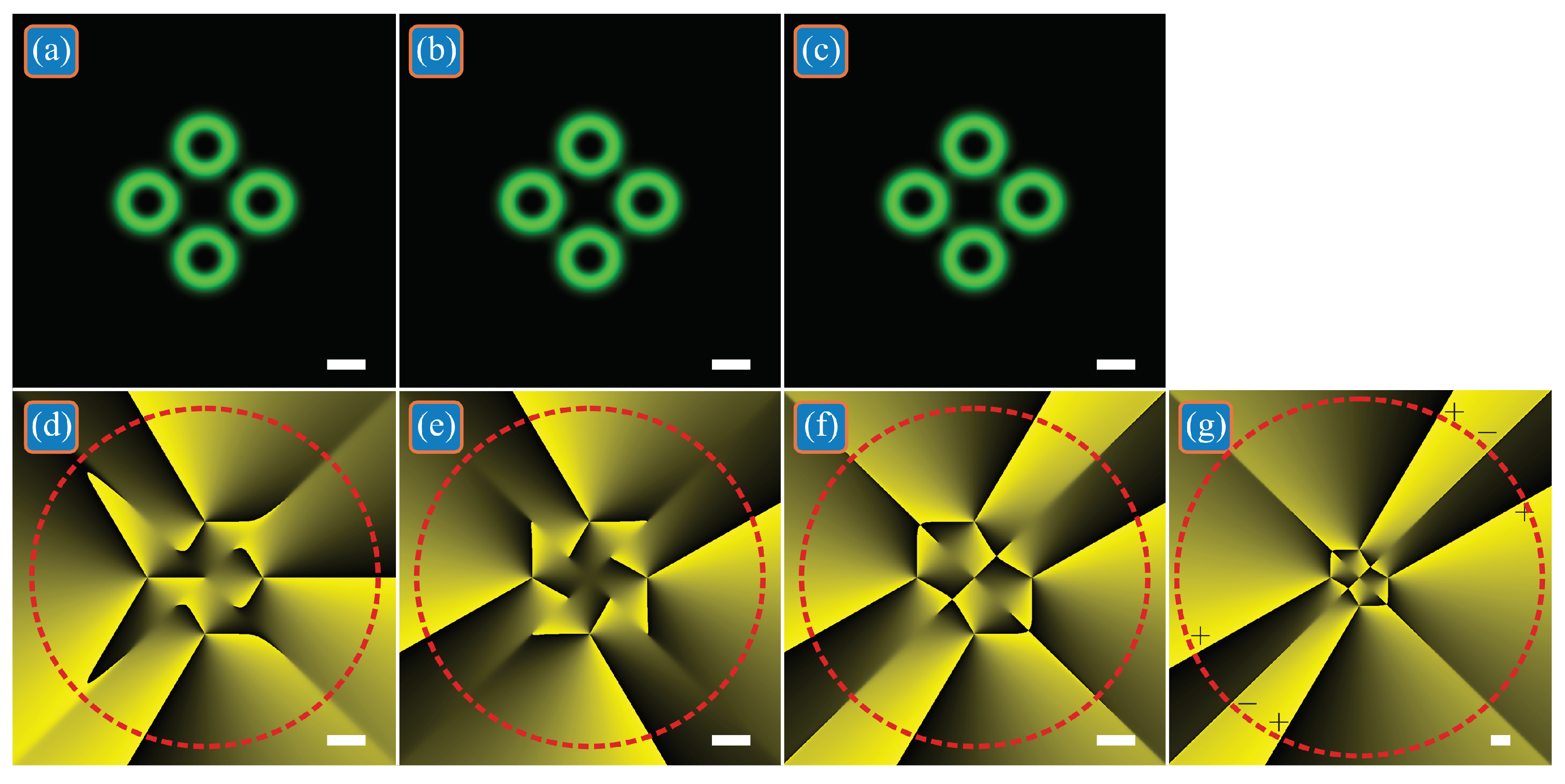
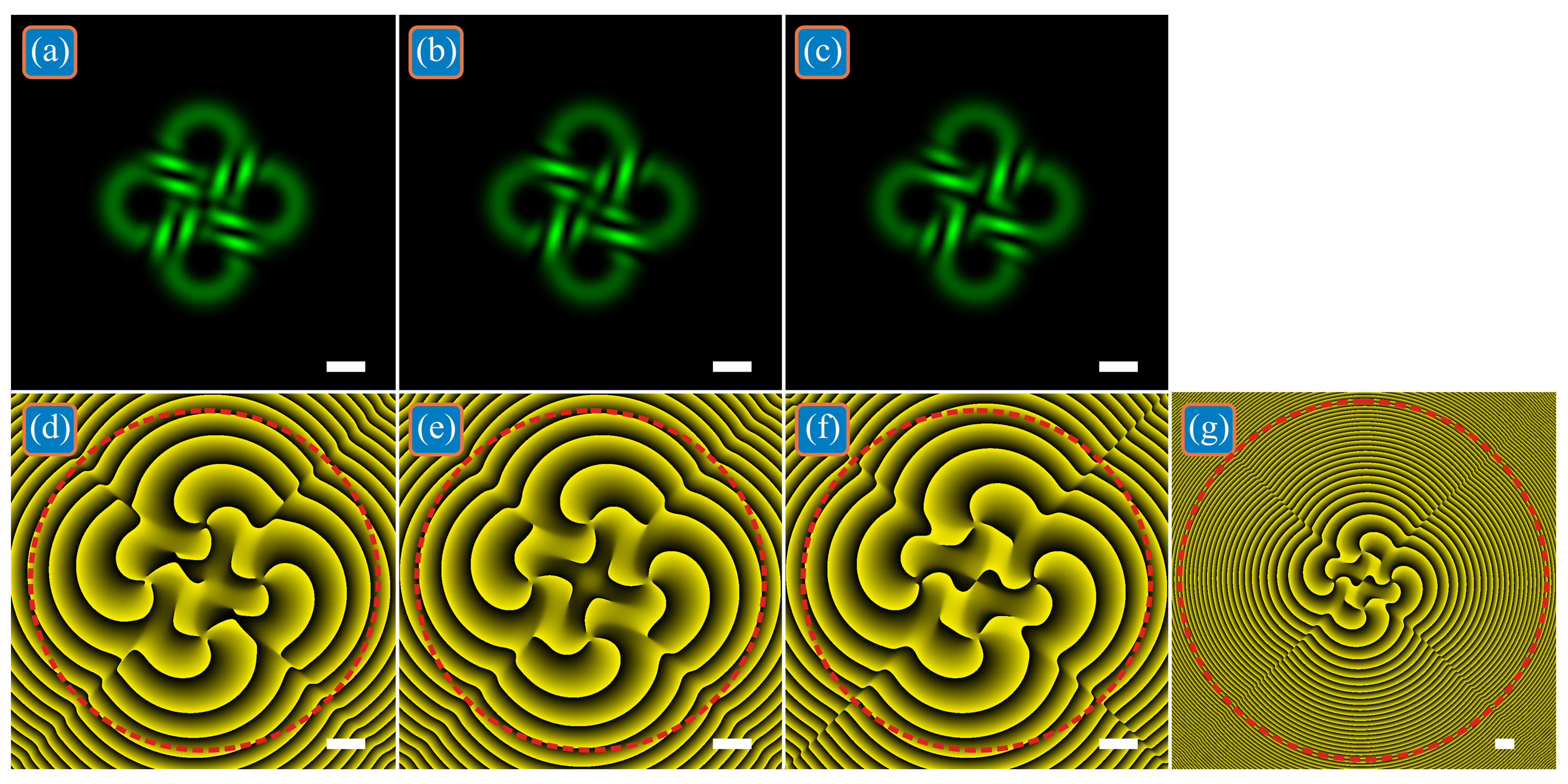
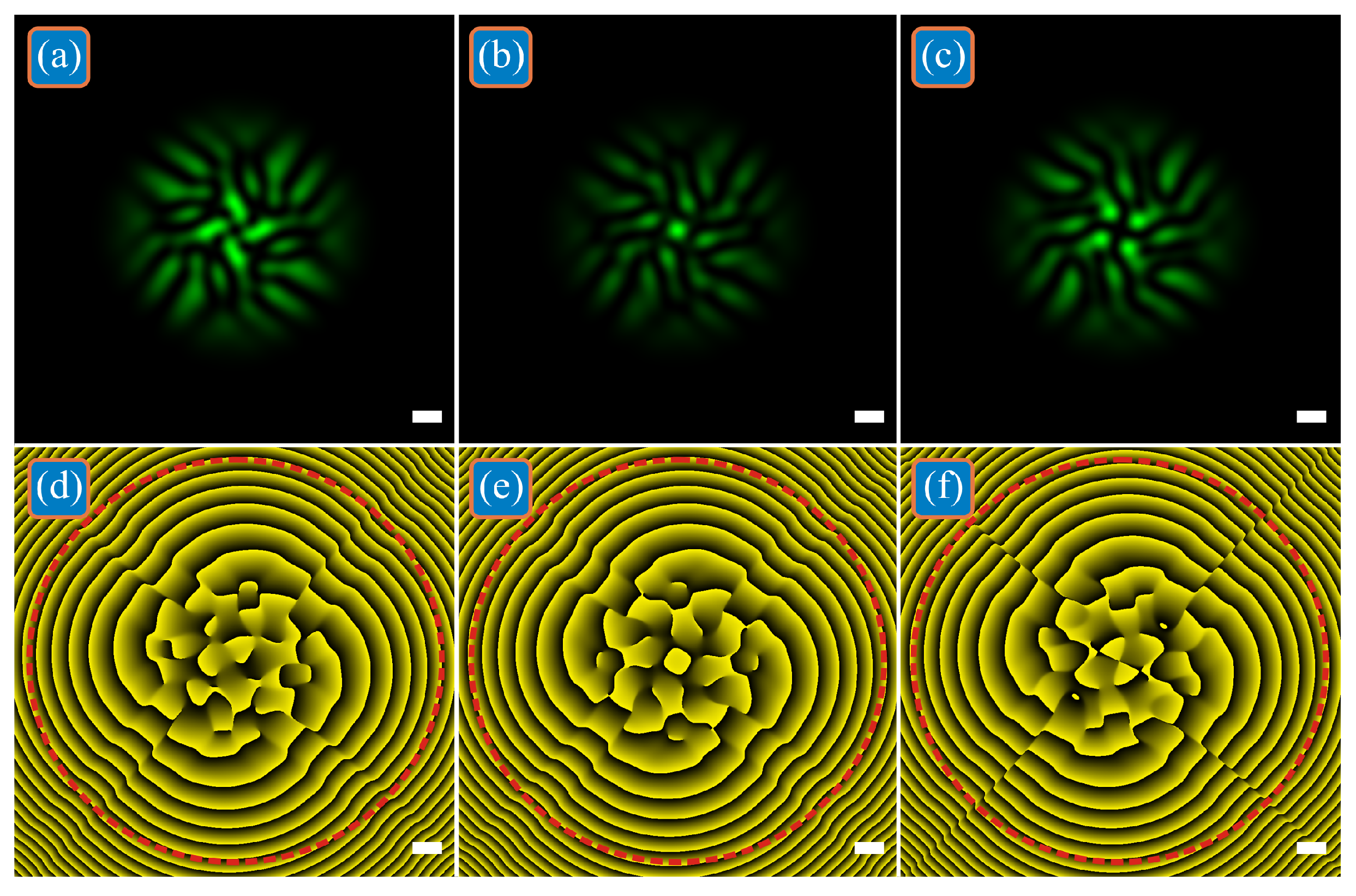
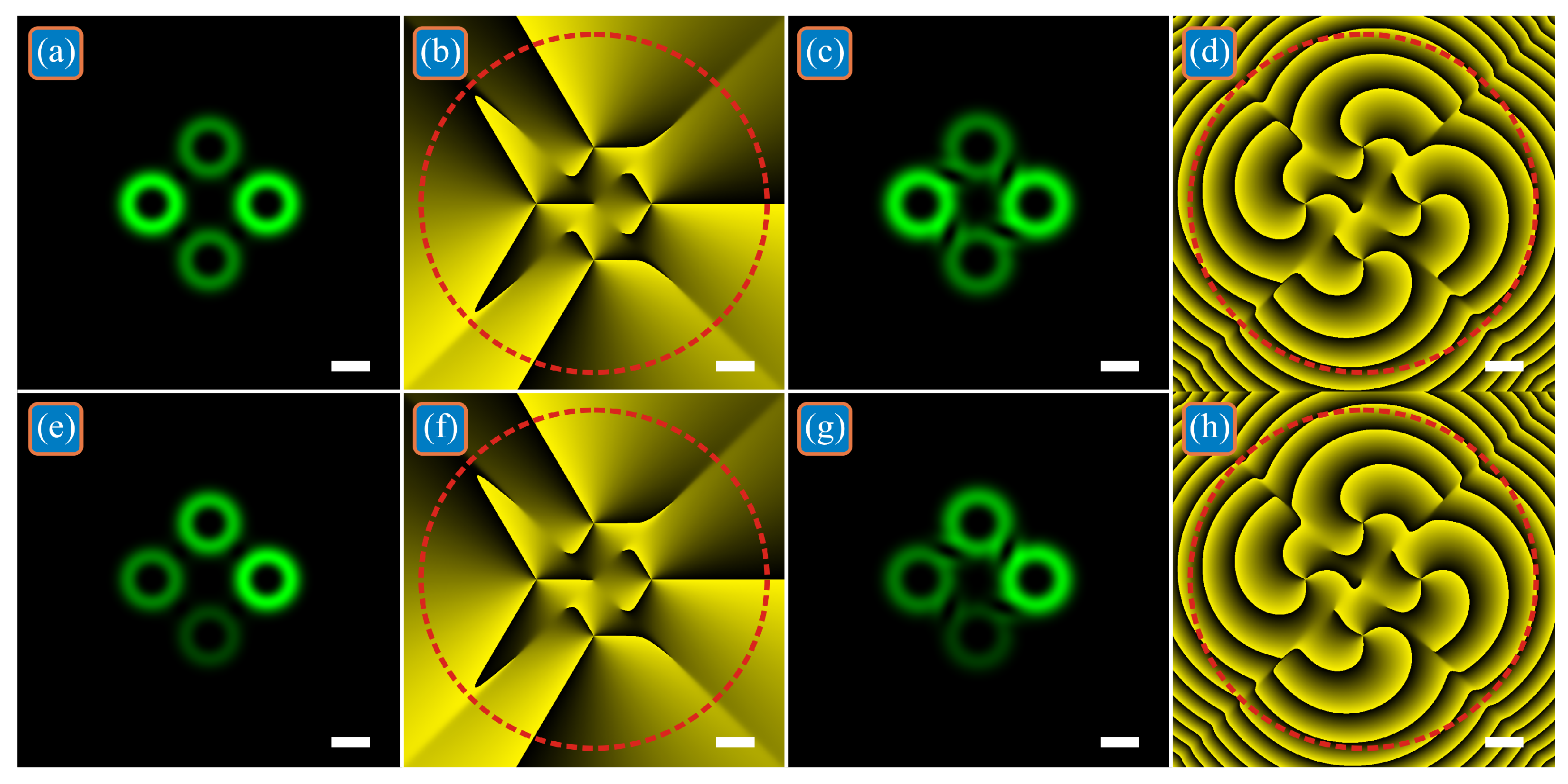
4. Modeling
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shen, Y.; Wang, X.; Xie, Z.; Min, C.; Fu, X.; Liu, Q.; Gong, M.; Yuan, X. Optical vortices 30 years on: OAM manipulation from topological charge to multiple singularities. Light Sci. Appl. 2019, 8, 90. [Google Scholar] [CrossRef] [PubMed]
- Lin, X.J.; Feng, Q.C.; Zhu, Y.; Ji, S.H.; Xiao, B.; Xu, H.Y.; Li, W.S.; Cai, Z.P. Diode-pumped wavelength-switchable visible Pr3+:YLF laser and vortex laser around 670 nm. Opto-Electron. Adv. 2021, 4, 210006. [Google Scholar] [CrossRef]
- Zhang, D.S.; Li, X.Z.; Fu, Y.; Yao, Q.H.; Li, Z.G.; Sugioka, K. Liquid vortexes and flows induced by femtosecond laser ablation in liquid governing formation of circular and crisscross LIPSS. Opto-Electron. Adv. 2022, 5, 210066. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, X.; Lin, H.; Wang, D.; Cao, E.; Liu, S.; Nie, Z.; Jia, B. Ultrafast Multi-Target Control of Tightly Focused Light Fields. Opto-Electron. Adv. 2022, 5, 210026. [Google Scholar] [CrossRef]
- Allen, L.; Beijersbergen, M.; Spreeuw, R.; Woerdman, J. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185–8189. [Google Scholar] [CrossRef]
- Berry, M.V. Optical vortices evolving from helicoidal integer and fractional phase steps. J. Opt. A Pure Appl. Opt. 2004, 6, 259–268. [Google Scholar] [CrossRef]
- Molina-Terriza, G.; Recolons, J.; Torner, L. The curious arithmetic of optical vortices. Opt. Lett. 2000, 25, 1135–1137. [Google Scholar] [CrossRef][Green Version]
- Pyragaite, V.; Smilgevicius, V.; Stabinis, A.; Maslinska, V. The interference of higher order Laguerre-Gaussian beams. Lith. J. Phys. 2007, 47, 21–26. [Google Scholar] [CrossRef]
- Pyragaite, V.; Stabinis, A. Free-space propagation of overlapping light vortex beams. Opt. Commun. 2002, 213, 187–191. [Google Scholar] [CrossRef]
- Lopez-Mago, D.; Perez-Garcia, B.; Yepiz, A.; Hernandez-Aranda, R.I.; Gutiérrez-Vega, J.C. Dynamics of polarization singularities in composite optical vortices. J. Opt. 2013, 15, 044028. [Google Scholar] [CrossRef]
- Naik, D.N.; Viswanathan, N.K. Generation of singular optical beams from fundamental Gaussian beam using Sagnac interferometer. J. Opt. 2016, 18, 095601. [Google Scholar] [CrossRef]
- Cheng, K.; Lü, B. Composite coherence vortices in coherent and incoherent superpositions of two off-axis partially coherent vortex beams. J. Mod. Opt. 2008, 55, 2751–2764. [Google Scholar] [CrossRef]
- Sukhorukov, A.P.; Kalinovich, A.A.; Molina-Terriza, G.; Torner, L. Superposition of noncoaxial vortices in parametric wave mixing. Phys. Rev. E 2002, 66, 036608. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Wu, Z.; Yang, K.; Li, P.; Wen, F.; Gu, Y. Splitting, generation, and annihilation of phase singularities in non-coaxial interference of Bessel-Gaussian beams. Phys. Scr. 2021, 96, 125105. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A.; Amiri, P.; Soltani, P.; Rasouly, S. Topological charge of two parallel Laguerre-Gaussian beams. Opt. Express 2021, 29, 42962–42977. [Google Scholar] [CrossRef]
- Kovalev, A.A.; Kotlyar, V.V. Orbital angular momentum of superposition of identical shifted vortex beams. J. Opt. Soc. Am. A 2015, 32, 1805–1810. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Kovalev, A.A. Topological Charge of Optical Vortices; Novaya Technika: Samara, Russia, 2021; ISBN 978-5-88940-157-5. (In Russian) [Google Scholar]
- Kotlyar, V.V.; Kovalev, A.A.; Volyar, A.V. Topological charge of a linear combination of optical vortices: Topological competition. Opt. Express 2020, 28, 8266–8281. [Google Scholar] [CrossRef]
- Zhang, Q.; Ni, J.; Qiu, C.W. Vortex 4.0 on chip. Light Sci. Appl. 2020, 9, 103. [Google Scholar] [CrossRef]
- Akulshin, A.M.; Novikova, I.; Mikhailov, E.E.; Suslov, S.A.; McLean, R.J. Arithmetic with optical topological charges in stepwise-excited Rb vapor. Opt. Lett. 2016, 41, 1146–1149. [Google Scholar] [CrossRef]
- Lee, I.J.; Kim, S. Directional coupler design for orbital angular momentum mode-based photonic integrated circuits. Opt. Express 2020, 28, 30085. [Google Scholar] [CrossRef]
- Lee, I.J.; Kim, S. On-Chip Guiding of Higher-Order Orbital Angular Momentum Modes. Photonics 2019, 6, 72. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kotlyar, V.V.; Kovalev, A.A.; Kozlova, E.S.; Savelyeva, A.A. Tailoring the Topological Charge of a Superposition of Identical Parallel Laguerre–Gaussian Beams. Micromachines 2022, 13, 2227. https://doi.org/10.3390/mi13122227
Kotlyar VV, Kovalev AA, Kozlova ES, Savelyeva AA. Tailoring the Topological Charge of a Superposition of Identical Parallel Laguerre–Gaussian Beams. Micromachines. 2022; 13(12):2227. https://doi.org/10.3390/mi13122227
Chicago/Turabian StyleKotlyar, Victor V., Alexey A. Kovalev, Elena S. Kozlova, and Alexandra A. Savelyeva. 2022. "Tailoring the Topological Charge of a Superposition of Identical Parallel Laguerre–Gaussian Beams" Micromachines 13, no. 12: 2227. https://doi.org/10.3390/mi13122227
APA StyleKotlyar, V. V., Kovalev, A. A., Kozlova, E. S., & Savelyeva, A. A. (2022). Tailoring the Topological Charge of a Superposition of Identical Parallel Laguerre–Gaussian Beams. Micromachines, 13(12), 2227. https://doi.org/10.3390/mi13122227







