An Analytic Model of Transient Heat Conduction for Bi-Layered Flexible Electronic Heaters by Symplectic Superposition
Abstract
1. Introduction
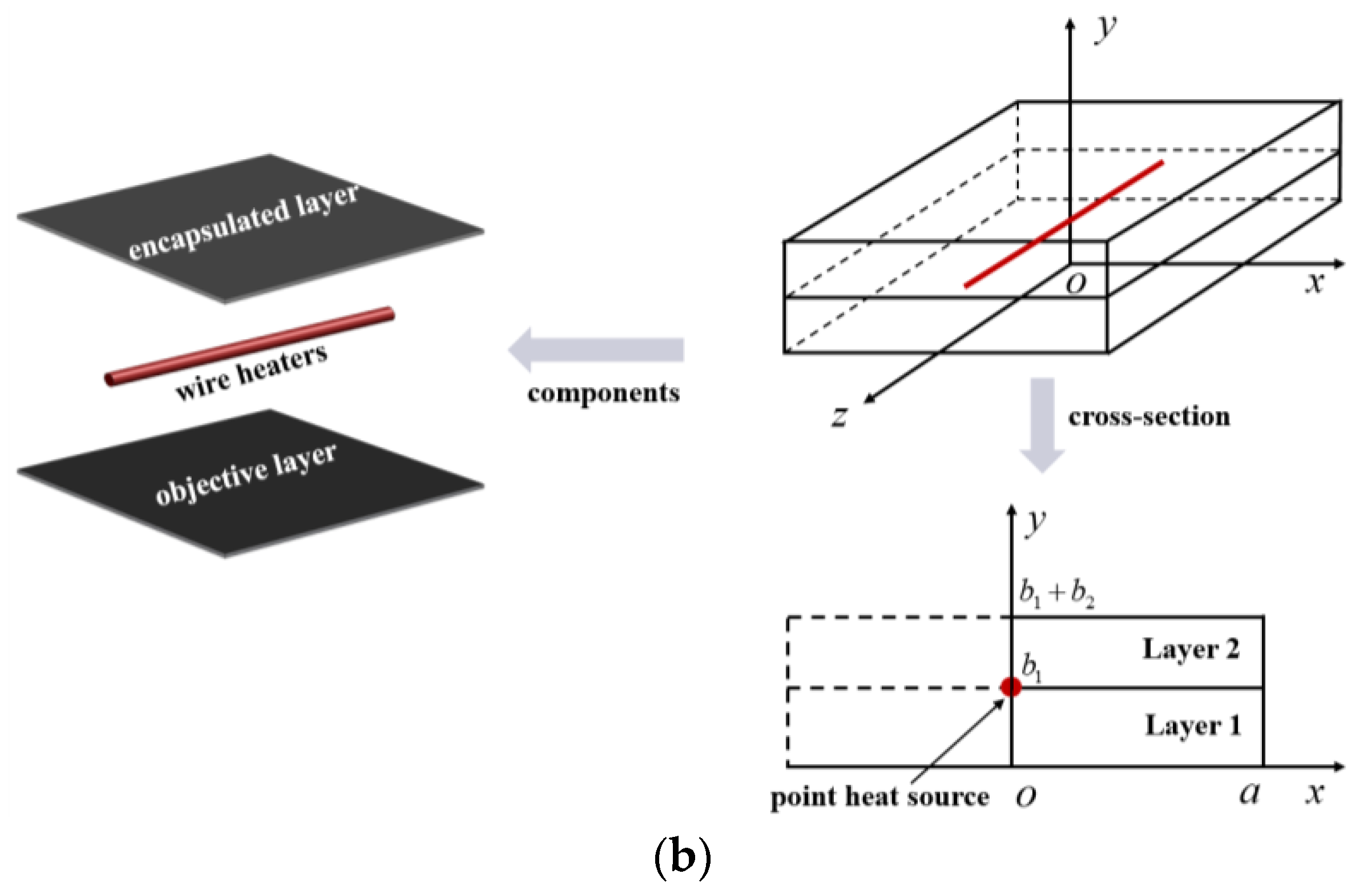
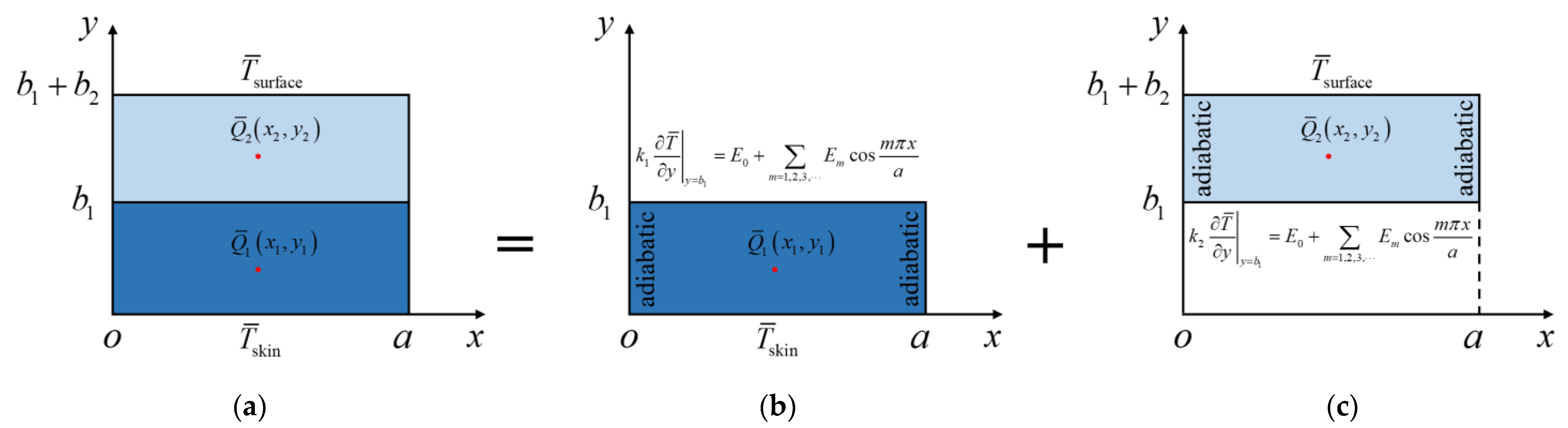
2. Governing Equation for Bi-Layered Transient Heat Conduction within the Hamiltonian Framework
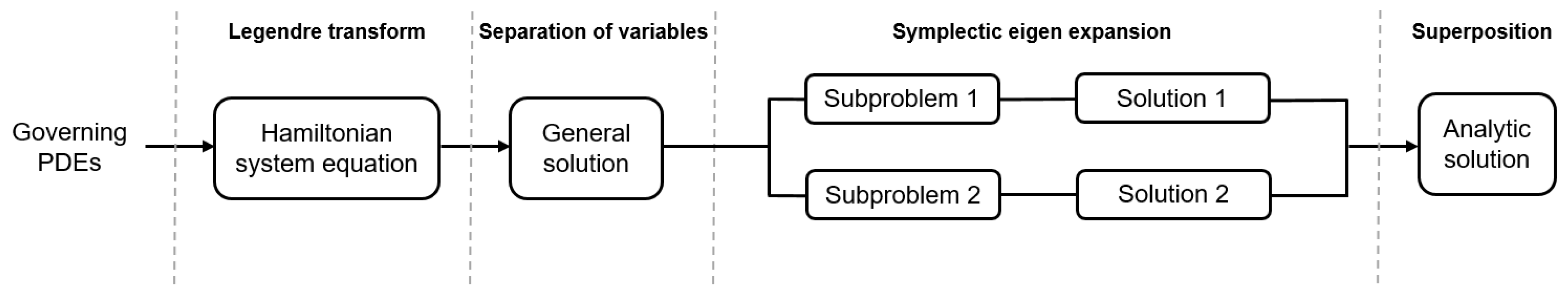
3. New Analytic Solution by the SSM
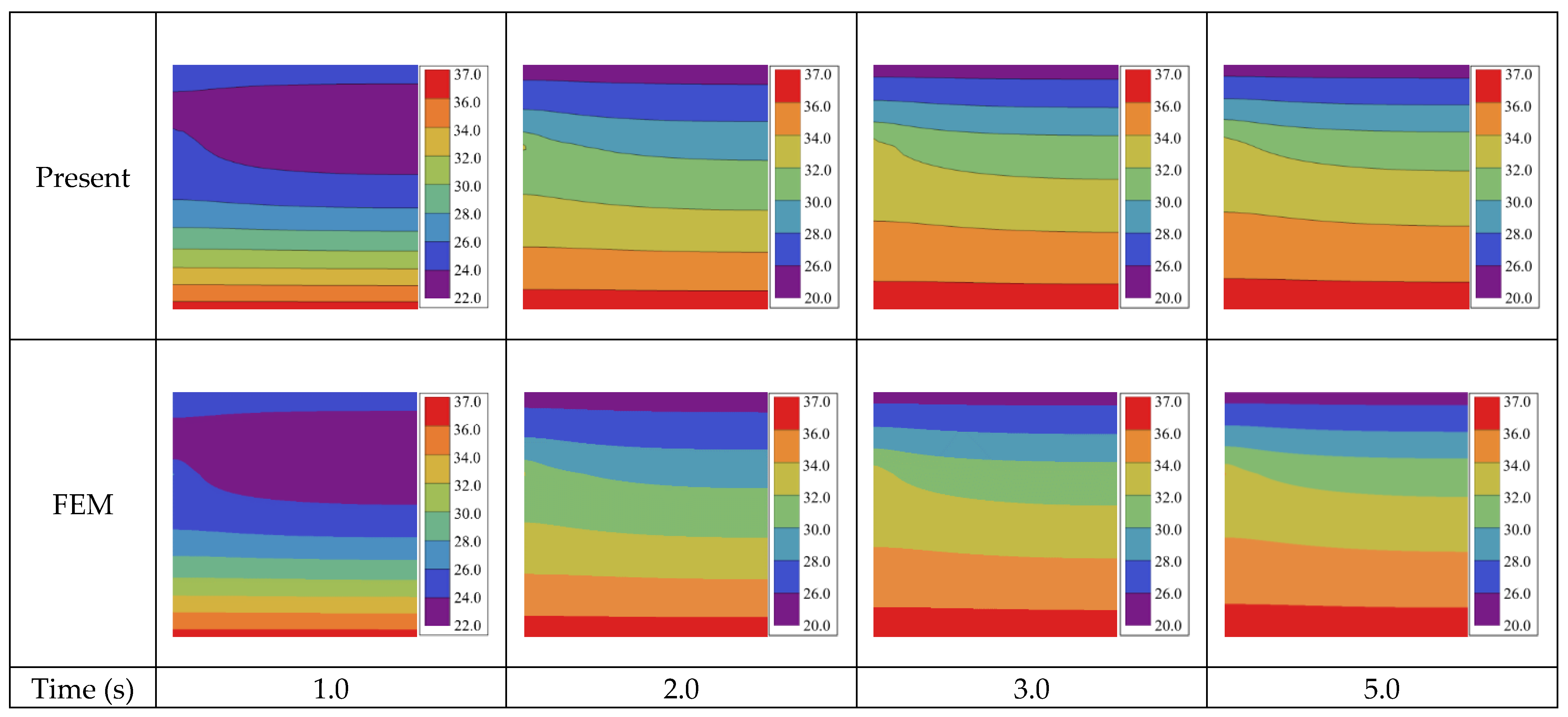
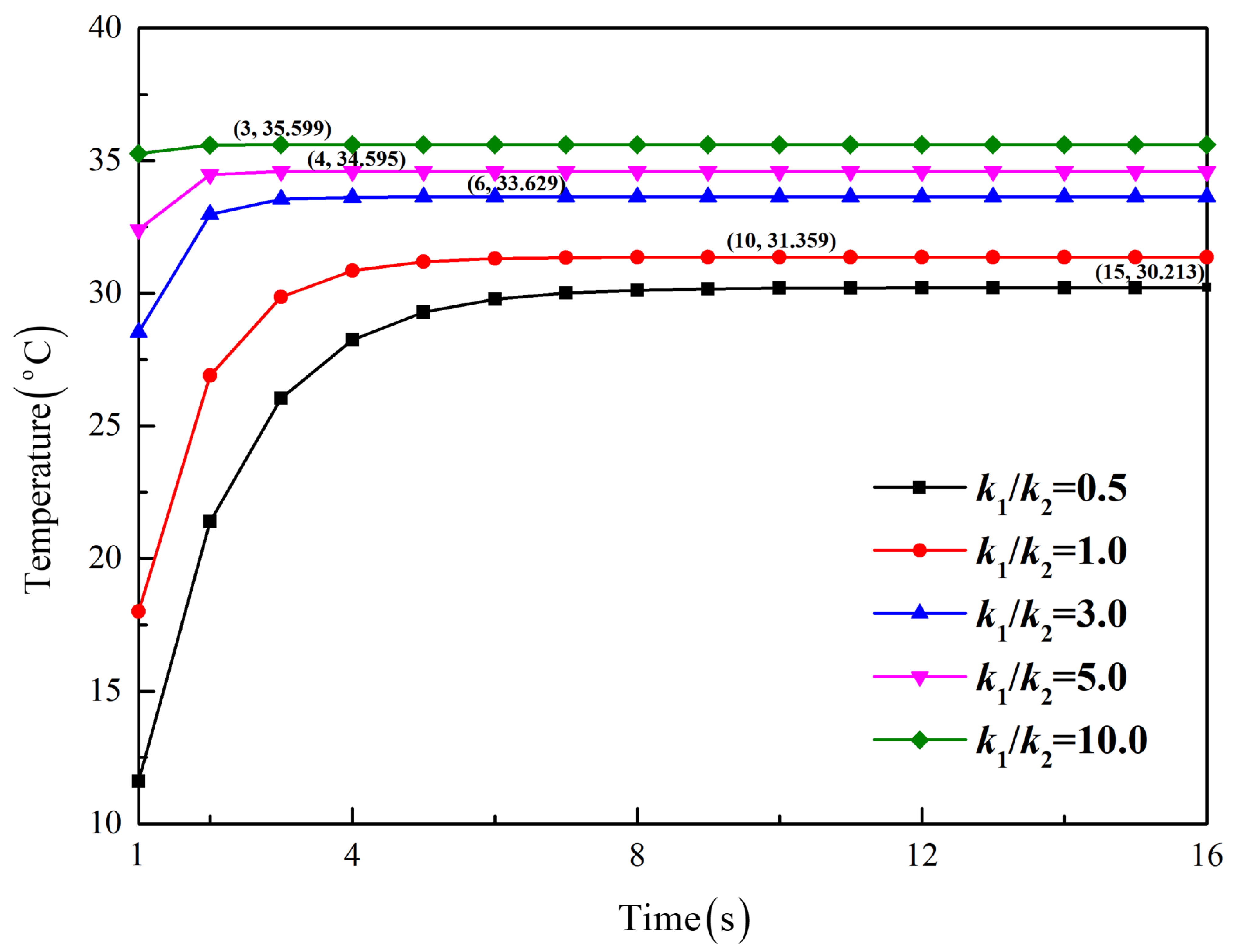
4. Comprehensive Benchmark Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kwon, J.; Hong, S.; Kim, G.; Suh, Y.D.; Lee, H.; Choo, S.; Lee, D.; Kong, H.; Yeo, J.; Ko, S.H. Digitally patterned resistive micro heater as a platform for zinc oxide nanowire based micro sensor. Appl. Surf. Sci. 2018, 447, 1–7. [Google Scholar] [CrossRef]
- Williams, S.J.; Green, N.G. Electrothermal pumping with interdigitated electrodes and resistive heaters. Electrophoresis 2015, 36, 1681–1689. [Google Scholar] [CrossRef]
- Zainal, M.A.; Ahmad, A.; Ali, M.S.M. Frequency-controlled wireless shape memory polymer microactuator for drug delivery application. Biomed. Microdevices 2017, 19, 8. [Google Scholar] [CrossRef]
- Kersemans, V.; Gilchrist, S.; Allen, P.D.; Beech, J.S.; Kinchesh, P.; Vojnovic, B.; Smart, S.C. A resistive heating system for homeothermic maintenance in small animals. Magn. Reson. Imaging 2015, 33, 847–851. [Google Scholar] [CrossRef]
- Jo, H.S.; An, S.; Lee, J.G.; Park, H.G.; Al-Deyab, S.S.; Yarin, A.L.; Yoon, S.S. Highly flexible, stretchable, patternable, transparent copper fiber heater on a complex 3D surface. NPG Asia Mater. 2017, 9, e347. [Google Scholar] [CrossRef]
- Hong, S.; Lee, H.; Lee, J.; Kwon, J.; Han, S.; Suh, Y.D.; Cho, H.; Shin, J.; Yeo, J.; Ko, S.H. Highly stretchable and transparent metal nanowire heater for wearable electronics applications. Adv. Mater. 2015, 27, 4744–4751. [Google Scholar] [CrossRef]
- Oh, J.Y.; Kim, S.; Baik, H.K.; Jeong, U. Conducting polymer dough for deformable electronics. Adv. Mater. 2016, 28, 4455–4461. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Z.; Li, X.; Zhang, J.; Lou, H.; Shi, X.; Cheng, X.; Peng, H. A smart, stretchable resistive heater textile. J. Mater. Chem. C 2017, 5, 41–46. [Google Scholar] [CrossRef]
- Stier, A.; Halekote, E.; Mark, A.; Qiao, S.; Yang, S.; Diller, K.; Lu, N. Stretchable tattoo-like heater with on-site temperature feedback control. Micromachines 2018, 9, 170. [Google Scholar] [CrossRef]
- Jang, N.S.; Kim, K.H.; Ha, S.H.; Jung, S.H.; Lee, H.M.; Kim, J.M. Simple approach to high-performance stretchable heaters based on kirigami patterning of conductive paper for wearable thermotherapy applications. ACS Appl. Mater. Interfaces 2017, 9, 19612–19621. [Google Scholar] [CrossRef]
- Li, K.; Cheng, X.; Zhu, F.; Li, L.; Xie, Z.; Luan, H.; Wang, Z.; Ji, Z.; Wang, H.; Liu, F.; et al. A generic soft encapsulation strategy for stretchable electronics. Adv. Funct. Mater. 2019, 29, 1806630. [Google Scholar] [CrossRef]
- Yan, D.; Chang, J.; Zhang, H.; Liu, J.; Song, H.; Xue, Z.; Zhang, F.; Zhang, Y. Soft three-dimensional network materials with rational bio-mimetic designs. Nat. Commun. 2020, 11, 1180. [Google Scholar] [CrossRef] [PubMed]
- Xu, L.; Gutbrod, S.R.; Ma, Y.; Petrossians, A.; Liu, Y.; Webb, R.C.; Fan, J.A.; Yang, Z.; Xu, R.; Whalen, J.J., III; et al. Materials and fractal designs for 3D multifunctional integumentary membranes with capabilities in cardiac electrotherapy. Adv. Mater. 2015, 27, 1731–1737. [Google Scholar] [CrossRef] [PubMed]
- Cai, Y.; Piao, X.; Yao, X.; Nie, E.; Zhang, Z.; Sun, Z. A facile method to prepare silver nanowire transparent conductive film for heaters. Mater. Lett. 2019, 249, 66–69. [Google Scholar] [CrossRef]
- Yoon, Y.H.; Song, J.W.; Kim, D.; Kim, J.; Park, J.K.; Oh, S.K.; Han, C.S. Transparent film heater using single-walled carbon nanotubes. Adv. Mater. 2007, 19, 4284–4287. [Google Scholar] [CrossRef]
- Gueye, M.N.; Carella, A.; Demadrille, R.; Simonato, J.P. All-polymeric flexible transparent heaters. ACS Appl. Mater. Interfaces 2017, 9, 27250–27256. [Google Scholar] [CrossRef]
- Ji, S.; He, W.; Wang, K.; Ran, Y.; Ye, C. Thermal response of transparent silver nanowire/ PEDOT: PSS film heaters. Small 2014, 10, 4951–4960. [Google Scholar] [CrossRef]
- Zheng, X.; Sun, Y.; Huang, M.; An, D.; Li, P.; Wang, B.; Li, R. Symplectic superposition method-based new analytic bending solutions of cylindrical shell panels. Int. J. Mech. Sci. 2019, 152, 432–442. [Google Scholar] [CrossRef]
- Zheng, X.; Ni, Z.; Xu, D.; Wang, Z.; Liu, M.; Li, Y.; Du, J.; Li, R. New analytic buckling solutions of non-Lévy-type cylindrical panels within the symplectic framework. Appl. Math. Model. 2021, 98, 398–415. [Google Scholar] [CrossRef]
- Xu, D.; Ni, Z.; Li, Y.; Hu, Z.; Tian, Y.; Wang, B.; Li, R. On the symplectic superposition method for free vibration of rectangular thin plates with mixed boundary constraints on an edge. Theor. Appl. Mech. Lett. 2021, 11, 100293. [Google Scholar] [CrossRef]
- Li, R.; Li, M.; Su, Y.; Song, J.; Ni, X. An analytical mechanics model for the island-bridge structure of stretchable electronics. Soft Matter 2013, 9, 8476. [Google Scholar] [CrossRef]
- Yao, W.; Zhong, W.; Lim, C. Symplectic Elasticity; World Scientific: Singapore, 2009. [Google Scholar]
- Fourier, J.B.J. The Analytical Theory of Heat; The University Press: Cambridge, UK, 1878. [Google Scholar]
- Honig, G.; Hirdes, U. A method for the numerical inversion of Laplace transforms. Comput. Appl. Math. 1984, 10, 113–132. [Google Scholar] [CrossRef]
- Xu, C.; Rong, D.; Zhou, Z.; Xu, X. A novel Hamiltonian-based method for two-dimensional transient heat conduction in a rectangle with specific mixed boundary conditions. J. Therm. Sci. Technol. 2017, 12, JTST0021. [Google Scholar] [CrossRef][Green Version]
- Hua, Y.; Zhao, T.; Guo, Z. Optimization of the one-dimensional transient heat conduction problems using extended entransy analyses. Int. J. Heat Mass Transf. 2018, 116, 166–172. [Google Scholar] [CrossRef]
- Zhao, T.; Ai, W.; Ma, H.; Liang, Z.; Chen, Q. Integral identification of fluid specific heat capacity and heat transfer coefficient distribution in heat exchangers based on multiple-case joint analysis. Int. J. Heat Mass Transf. 2022, 185, 122394. [Google Scholar] [CrossRef]
- Hua, Y.; Zhao, T.; Guo, Z. Irreversibility and Action of the Heat Conduction Process. Entropy 2018, 20, 206. [Google Scholar] [CrossRef]


| Case | Location | Number of Series Terms | FEM | ||||
|---|---|---|---|---|---|---|---|
| 5 | 10 | 20 | 30 | 50 | |||
| 1 | (a/6, a/6) | 35.699 | 35.699 | 35.699 | 35.699 | 35.699 | 35.699 |
| (a/2, a/6) | 35.613 | 35.613 | 35.613 | 35.613 | 35.613 | 35.613 | |
| (5a/6, a/6) | 35.563 | 35.563 | 35.563 | 35.563 | 35.563 | 35.563 | |
| (a/6, a/2) | 33.197 | 33.190 | 33.190 | 33.190 | 33.190 | 33.190 | |
| (a/2, a/2) | 32.784 | 32.781 | 32.781 | 32.781 | 32.781 | 32.781 | |
| (5a/6, a/2) | 32.644 | 32.641 | 32.641 | 32.641 | 32.641 | 32.641 | |
| (a/6, 5a/6) | 28.499 | 28.492 | 28.492 | 28.492 | 28.492 | 28.492 | |
| (a/2, 5a/6) | 28.171 | 28.167 | 28.167 | 28.167 | 28.167 | 28.167 | |
| (5a/6, 5a/6) | 28.081 | 28.078 | 28.077 | 28.077 | 28.077 | 28.077 | |
| 2 | (a/6, a/6) | 37.662 | 37.662 | 37.662 | 37.662 | 37.662 | 37.662 |
| (a/2, a/6) | 37.378 | 37.378 | 37.378 | 37.378 | 37.378 | 37.378 | |
| (5a/6, a/6) | 37.210 | 37.210 | 37.210 | 37.210 | 37.210 | 37.210 | |
| (a/6, a/2) | 39.324 | 39.300 | 39.301 | 39.301 | 39.301 | 39.302 | |
| (a/2, a/2) | 37.947 | 37.935 | 37.935 | 37.935 | 37.935 | 37.935 | |
| (5a/6, a/2) | 37.479 | 37.469 | 37.468 | 37.468 | 37.468 | 37.468 | |
| (a/6, 5a/6) | 38.662 | 38.638 | 38.639 | 38.639 | 38.639 | 38.640 | |
| (a/2, 5a/6) | 37.570 | 37.557 | 37.558 | 37.558 | 37.558 | 37.557 | |
| (5a/6, 5a/6) | 37.269 | 37.259 | 37.258 | 37.258 | 37.258 | 37.258 | |
| Time (s) | Method | Location | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| (a/6, a/6) | (a/2, a/6) | (5a/6, a/6) | (a/6, a/2) | (a/2, a/2) | (5a/6, a/2) | (a/6, 5a/6) | (a/2, 5a/6) | (5a/6, 5a/6) | ||
| 0.5 | Present | 27.648 | 27.586 | 27.557 | 15.266 | 14.913 | 14.824 | 17.501 | 17.221 | 17.170 |
| FEM | 27.580 | 27.519 | 27.490 | 15.150 | 14.799 | 14.712 | 17.443 | 17.165 | 17.114 | |
| 1.0 | Present | 32.134 | 32.053 | 32.007 | 25.171 | 24.771 | 24.641 | 23.513 | 23.195 | 23.113 |
| FEM | 32.088 | 32.007 | 31.961 | 25.068 | 24.669 | 24.539 | 23.450 | 23.133 | 23.051 | |
| 1.5 | Present | 34.101 | 34.016 | 33.967 | 29.594 | 29.186 | 29.047 | 26.257 | 25.934 | 25.845 |
| FEM | 34.070 | 33.986 | 33.936 | 29.524 | 29.116 | 28.978 | 26.214 | 25.891 | 25.802 | |
| 2.0 | Present | 34.982 | 34.896 | 34.846 | 31.576 | 31.167 | 31.027 | 27.489 | 27.165 | 27.075 |
| FEM | 34.963 | 34.878 | 34.828 | 31.535 | 31.125 | 30.986 | 27.463 | 27.139 | 27.049 | |
| 2.5 | Present | 35.377 | 35.292 | 35.241 | 32.466 | 32.056 | 31.916 | 28.042 | 27.717 | 27.627 |
| FEM | 35.366 | 35.281 | 35.231 | 32.443 | 32.033 | 31.893 | 28.027 | 27.703 | 27.613 | |
| 3.0 | Present | 35.554 | 35.469 | 35.419 | 32.865 | 32.455 | 32.315 | 28.290 | 27.965 | 27.875 |
| FEM | 35.548 | 35.463 | 35.413 | 32.853 | 32.443 | 32.303 | 28.282 | 27.957 | 27.868 | |
| 5.0 | Present | 35.693 | 35.607 | 35.557 | 33.177 | 32.767 | 32.627 | 28.484 | 28.159 | 28.069 |
| FEM | 35.692 | 35.607 | 35.557 | 33.176 | 32.767 | 32.626 | 28.483 | 28.159 | 28.069 | |
| 7.5 | Present | 35.698 | 35.613 | 35.563 | 33.190 | 32.780 | 32.640 | 28.492 | 28.167 | 28.077 |
| FEM | 35.698 | 35.613 | 35.563 | 33.190 | 32.780 | 32.640 | 28.492 | 28.167 | 28.077 | |
| 10.0 | Present | 35.699 | 35.613 | 35.563 | 33.190 | 32.781 | 32.641 | 28.492 | 28.167 | 28.077 |
| FEM | 35.699 | 35.613 | 35.563 | 33.190 | 32.781 | 32.641 | 28.492 | 28.167 | 28.077 | |
| 15.0 | Present | 35.699 | 35.613 | 35.563 | 33.190 | 32.781 | 32.641 | 28.492 | 28.167 | 28.077 |
| FEM | 35.699 | 35.613 | 35.563 | 33.190 | 32.781 | 32.641 | 28.492 | 28.167 | 28.077 |
| Time (s) | Method | Location | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| (a/6, a/6) | (a/2, a/6) | (5a/6, a/6) | (a/6, a/2) | (a/2, a/2) | (5a/6, a/2) | (a/6, 5a/6) | (a/2, 5a/6) | (5a/6, 5a/6) | ||
| 0.5 | Present | 28.216 | 28.008 | 27.912 | 18.086 | 16.909 | 16.614 | 25.448 | 24.514 | 24.342 |
| FEM | 28.147 | 27.941 | 27.847 | 17.953 | 16.781 | 16.490 | 25.359 | 24.432 | 24.262 | |
| 1.0 | Present | 33.454 | 33.183 | 33.029 | 29.828 | 28.494 | 28.059 | 32.750 | 31.692 | 31.416 |
| FEM | 33.399 | 33.130 | 32.976 | 29.706 | 28.373 | 27.940 | 32.674 | 31.618 | 31.344 | |
| 1.5 | Present | 35.777 | 35.495 | 35.330 | 35.058 | 33.697 | 33.236 | 36.002 | 34.924 | 34.629 |
| FEM | 35.740 | 35.459 | 35.294 | 34.976 | 33.615 | 33.155 | 35.951 | 34.873 | 34.579 | |
| 2.0 | Present | 36.816 | 36.533 | 36.365 | 37.398 | 36.033 | 35.567 | 37.457 | 36.375 | 36.077 |
| FEM | 36.794 | 36.511 | 36.344 | 37.349 | 35.984 | 35.518 | 37.426 | 36.345 | 36.046 | |
| 2.5 | Present | 37.282 | 36.998 | 36.831 | 38.447 | 37.081 | 36.615 | 38.109 | 37.027 | 36.728 |
| FEM | 37.270 | 36.986 | 36.819 | 38.420 | 37.053 | 36.587 | 38.092 | 37.010 | 36.711 | |
| 3.0 | Present | 37.492 | 37.208 | 37.040 | 38.918 | 37.552 | 37.085 | 38.401 | 37.319 | 37.020 |
| FEM | 37.485 | 37.201 | 37.033 | 38.903 | 37.537 | 37.070 | 38.392 | 37.310 | 37.011 | |
| 5.0 | Present | 37.655 | 37.371 | 37.203 | 39.286 | 37.920 | 37.453 | 38.630 | 37.548 | 37.249 |
| FEM | 37.655 | 37.370 | 37.203 | 39.285 | 37.919 | 37.452 | 38.629 | 37.547 | 37.248 | |
| 7.5 | Present | 37.662 | 37.378 | 37.210 | 39.301 | 37.935 | 37.468 | 38.639 | 37.557 | 37.258 |
| FEM | 37.662 | 37.378 | 37.210 | 39.301 | 37.935 | 37.468 | 38.640 | 37.557 | 37.258 | |
| 10.0 | Present | 37.662 | 37.378 | 37.210 | 39.301 | 37.935 | 37.468 | 38.639 | 37.557 | 37.258 |
| FEM | 37.662 | 37.378 | 37.210 | 39.302 | 37.935 | 37.468 | 38.640 | 37.557 | 37.258 | |
| 15.0 | Present | 37.662 | 37.378 | 37.210 | 39.301 | 37.935 | 37.468 | 38.639 | 37.558 | 37.258 |
| FEM | 37.662 | 37.378 | 37.210 | 39.302 | 37.935 | 37.468 | 38.640 | 37.557 | 37.258 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, D.; Xiong, S.; Meng, F.; Wang, B.; Li, R. An Analytic Model of Transient Heat Conduction for Bi-Layered Flexible Electronic Heaters by Symplectic Superposition. Micromachines 2022, 13, 1627. https://doi.org/10.3390/mi13101627
Xu D, Xiong S, Meng F, Wang B, Li R. An Analytic Model of Transient Heat Conduction for Bi-Layered Flexible Electronic Heaters by Symplectic Superposition. Micromachines. 2022; 13(10):1627. https://doi.org/10.3390/mi13101627
Chicago/Turabian StyleXu, Dian, Sijun Xiong, Fanxing Meng, Bo Wang, and Rui Li. 2022. "An Analytic Model of Transient Heat Conduction for Bi-Layered Flexible Electronic Heaters by Symplectic Superposition" Micromachines 13, no. 10: 1627. https://doi.org/10.3390/mi13101627
APA StyleXu, D., Xiong, S., Meng, F., Wang, B., & Li, R. (2022). An Analytic Model of Transient Heat Conduction for Bi-Layered Flexible Electronic Heaters by Symplectic Superposition. Micromachines, 13(10), 1627. https://doi.org/10.3390/mi13101627





