Vibration Control of Time-Varying Delay under Complex Excitation
Abstract
:1. Introduction
2. Delay Dynamic Vibration Absorber
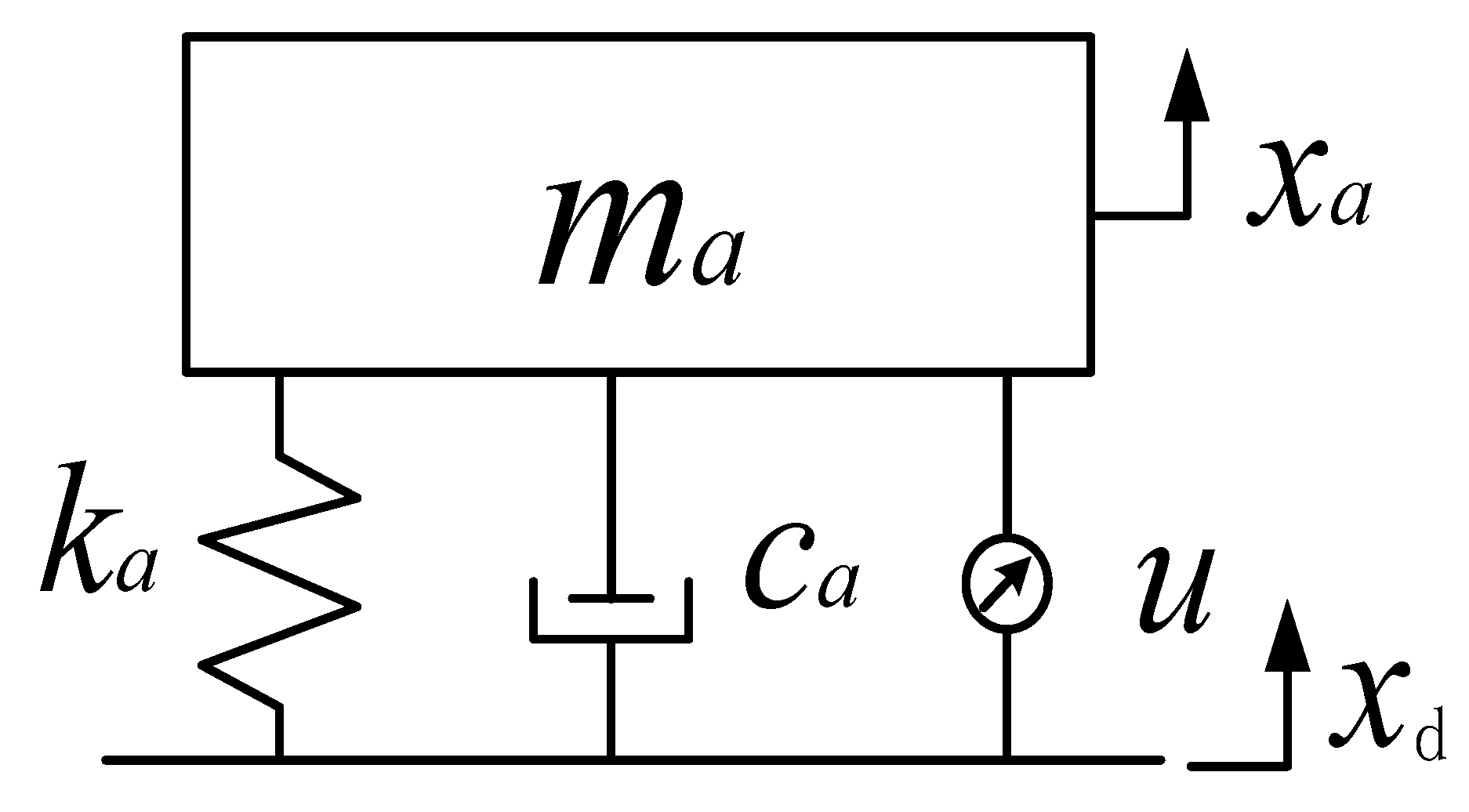
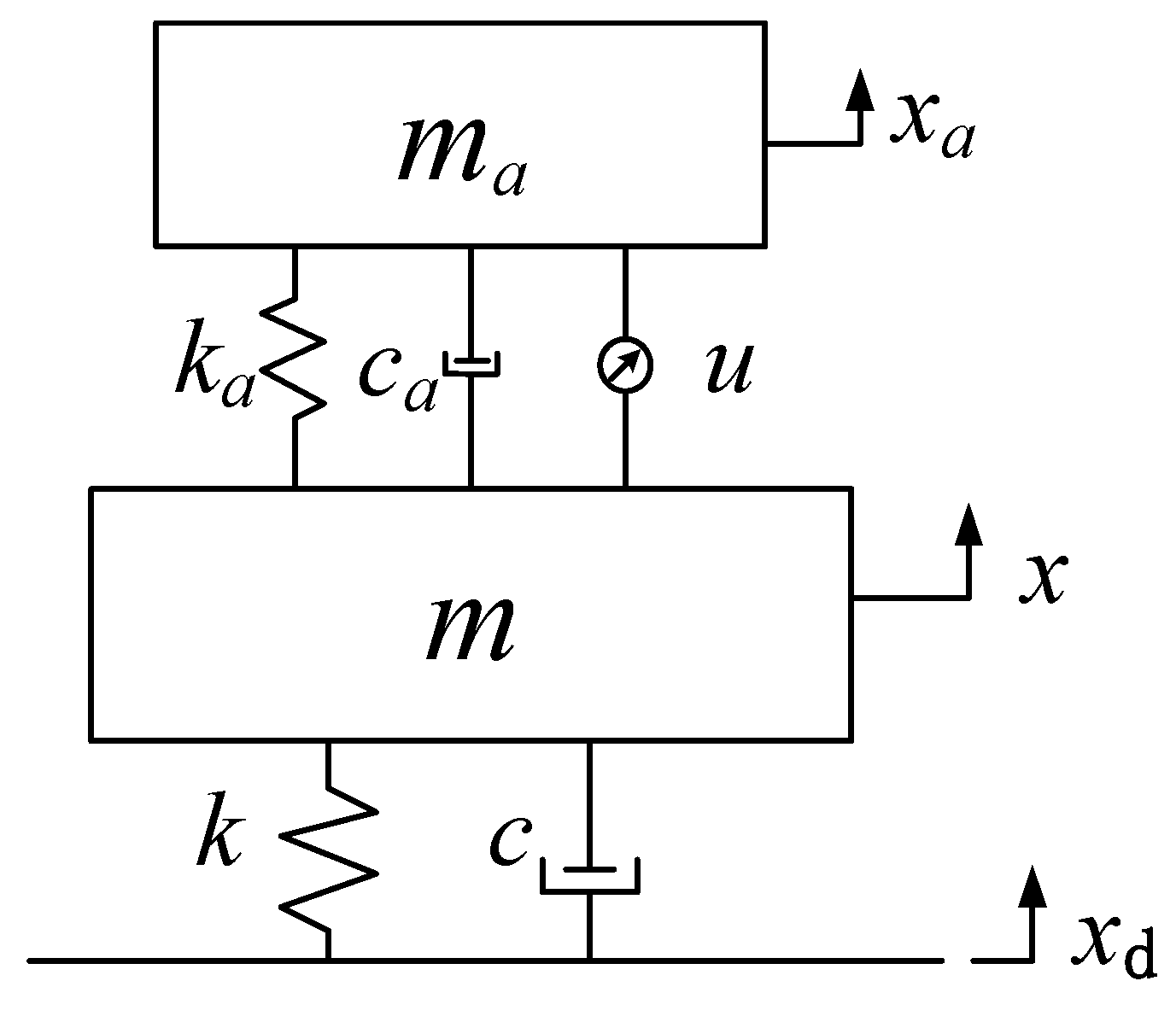
2.1. Delay Dynamic Vibration Absorber Model
2.2. Critical Stability Parameters of Delay Dynamic Vibration Absorber
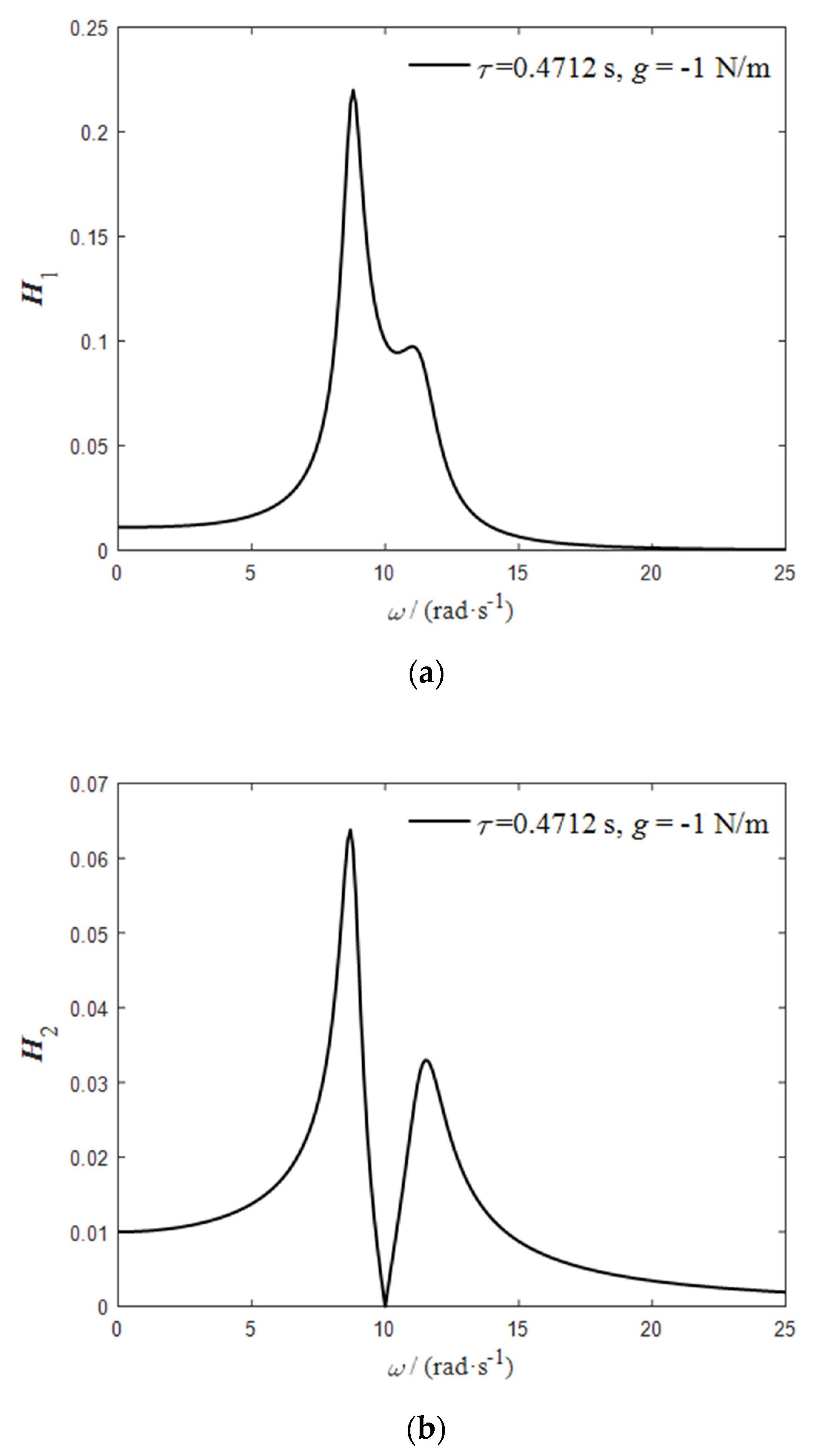
2.3. Research on Damping Efficiency of Delay Dynamic Vibration Absorber
3. Harmonic Equivalent Method for Complex Excitation
4. Time-Varying Delay Feedback Control Parameters
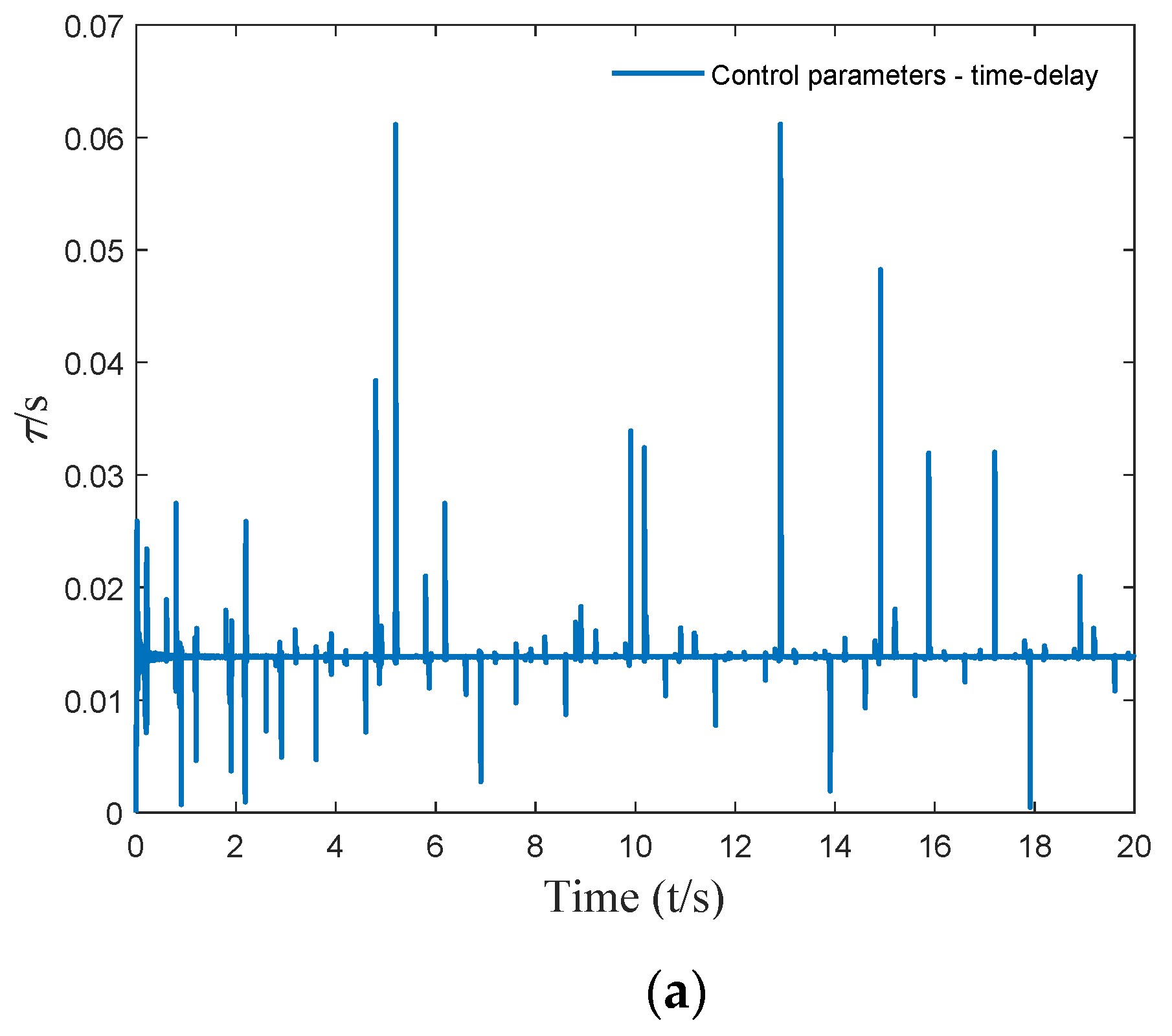
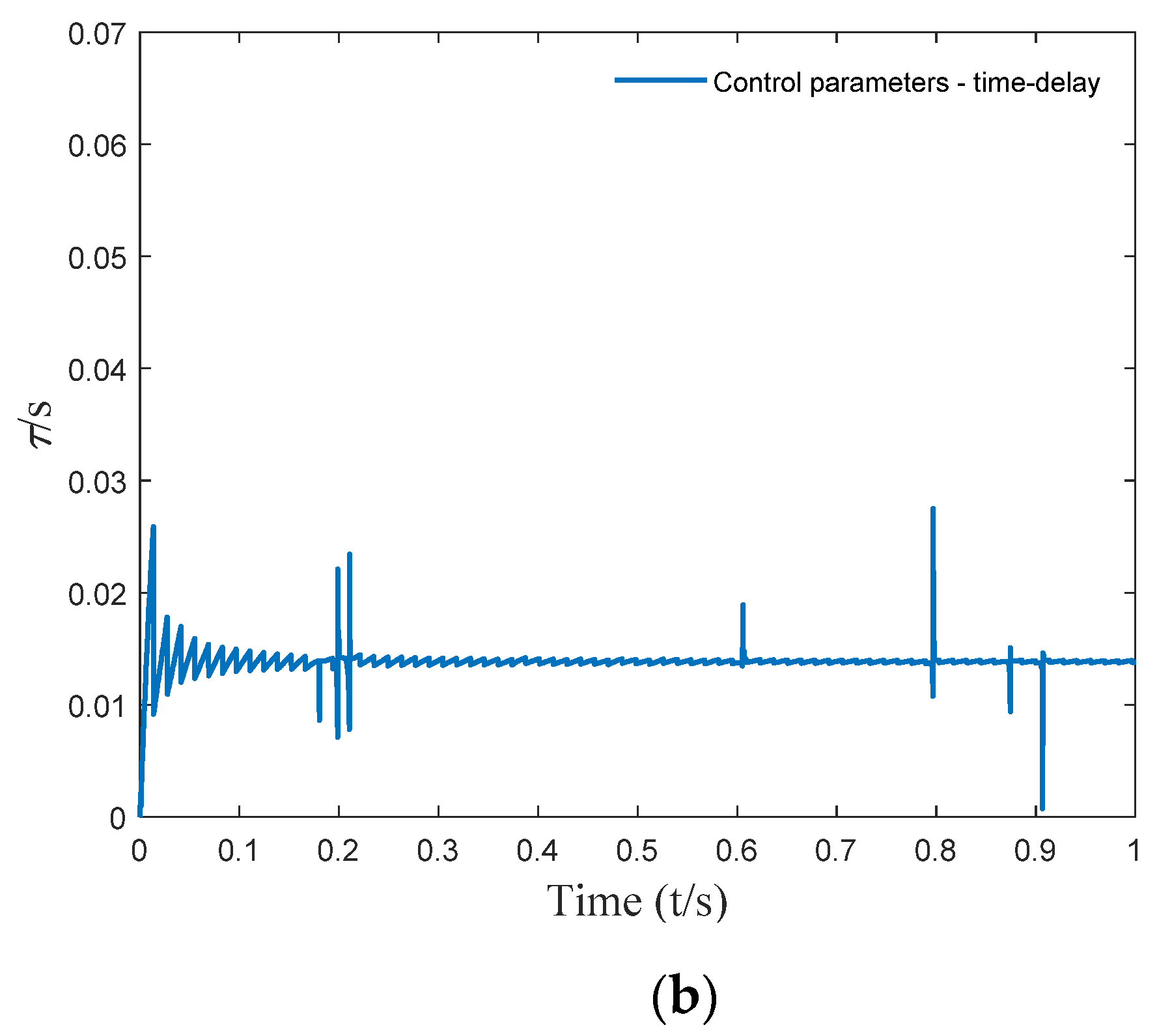
4.1. Determination of Time-Varying Delay Parameters
4.2. Determination of Feedback Gain Based on Hybrid Particle Swarm Optimization Algorithm
- The location and velocity of each particle in the random initial population were determined;
- The fitness of each particle was evaluated, and the current position and adaptation value of each particle in the Pbest of each particle was stored. Then, the position and adaptation value of the individual with the optimal adaptation value was stored in all Pbest in Gbest;
- The speed and position of each particle were updated;
- For each particle, the adaptation value and its experienced best position were compared. When the outcome was great, the most current best position was compared;
- The current values of all Pbest and Gbest were compared, and Gbest was updated;
- The whole particle population was sorted according to the adaptation value. The position and velocity of the worst half of the particle was replaced with that of the best half in the population; then, the unchanged status of Pbest and Gbest remained;
- The search was ended when the stop condition was satisfied (usually a preset operation accuracy or iteration times). Then, the result was outputted. Otherwise, the procedure returned to step 3.
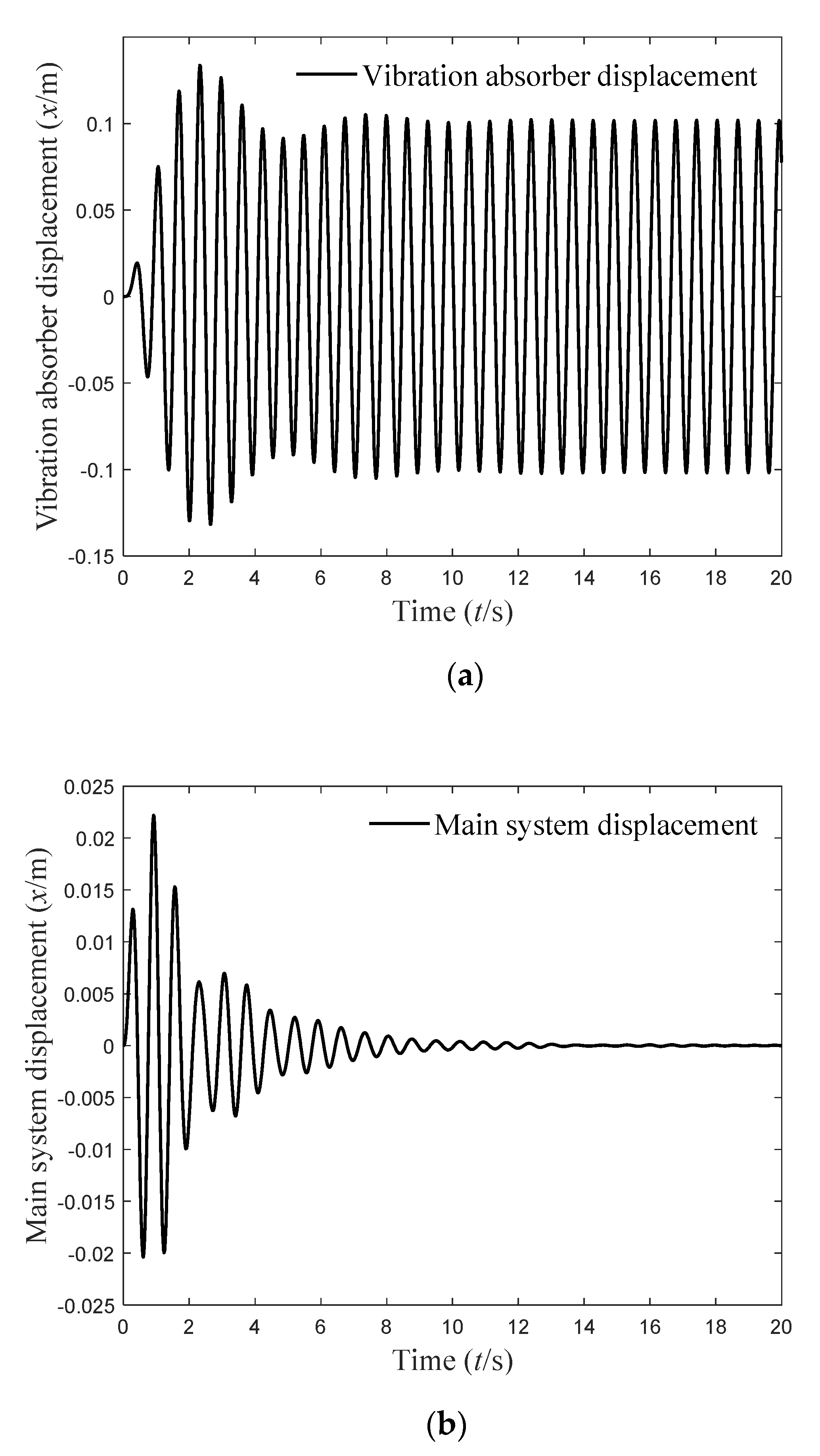
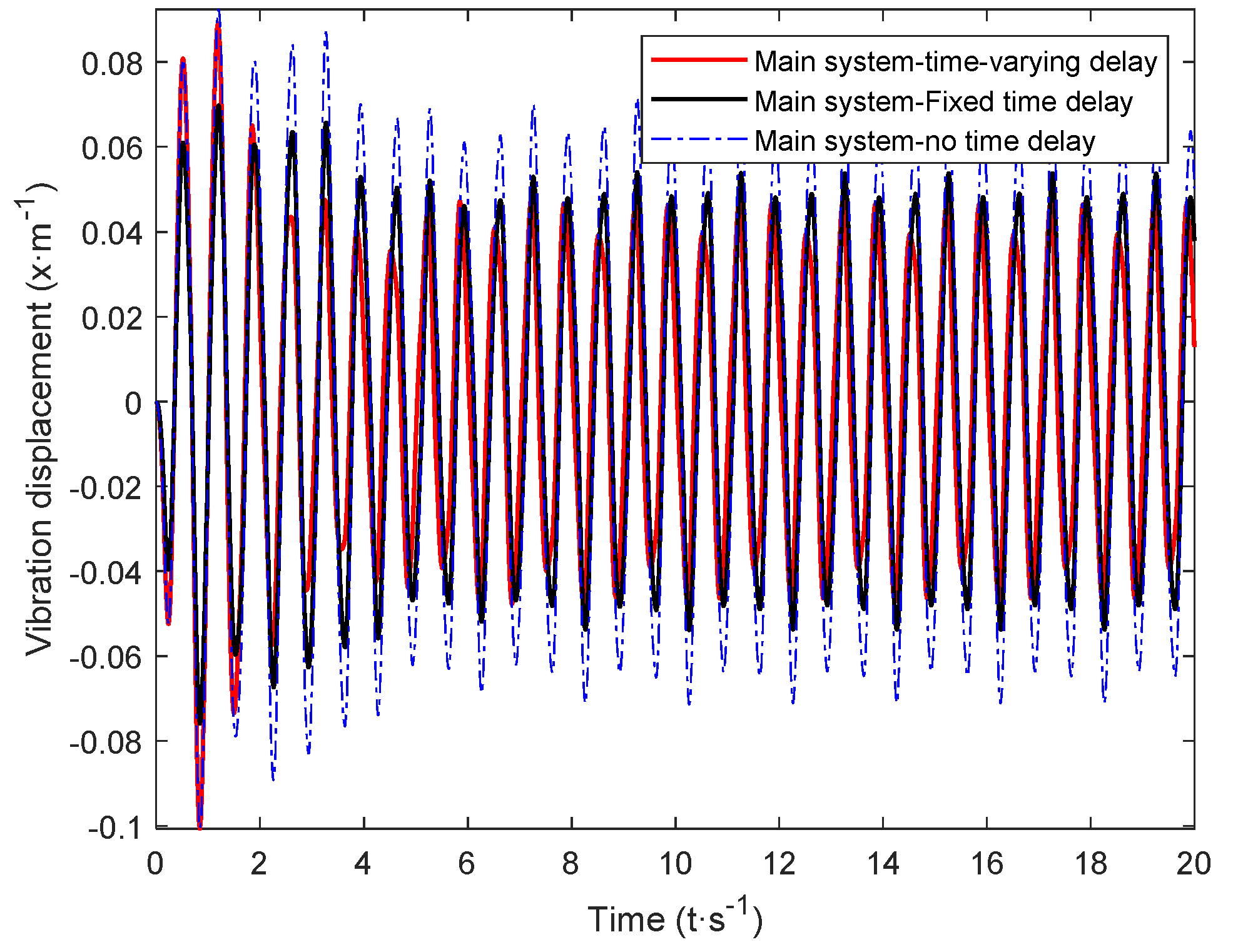
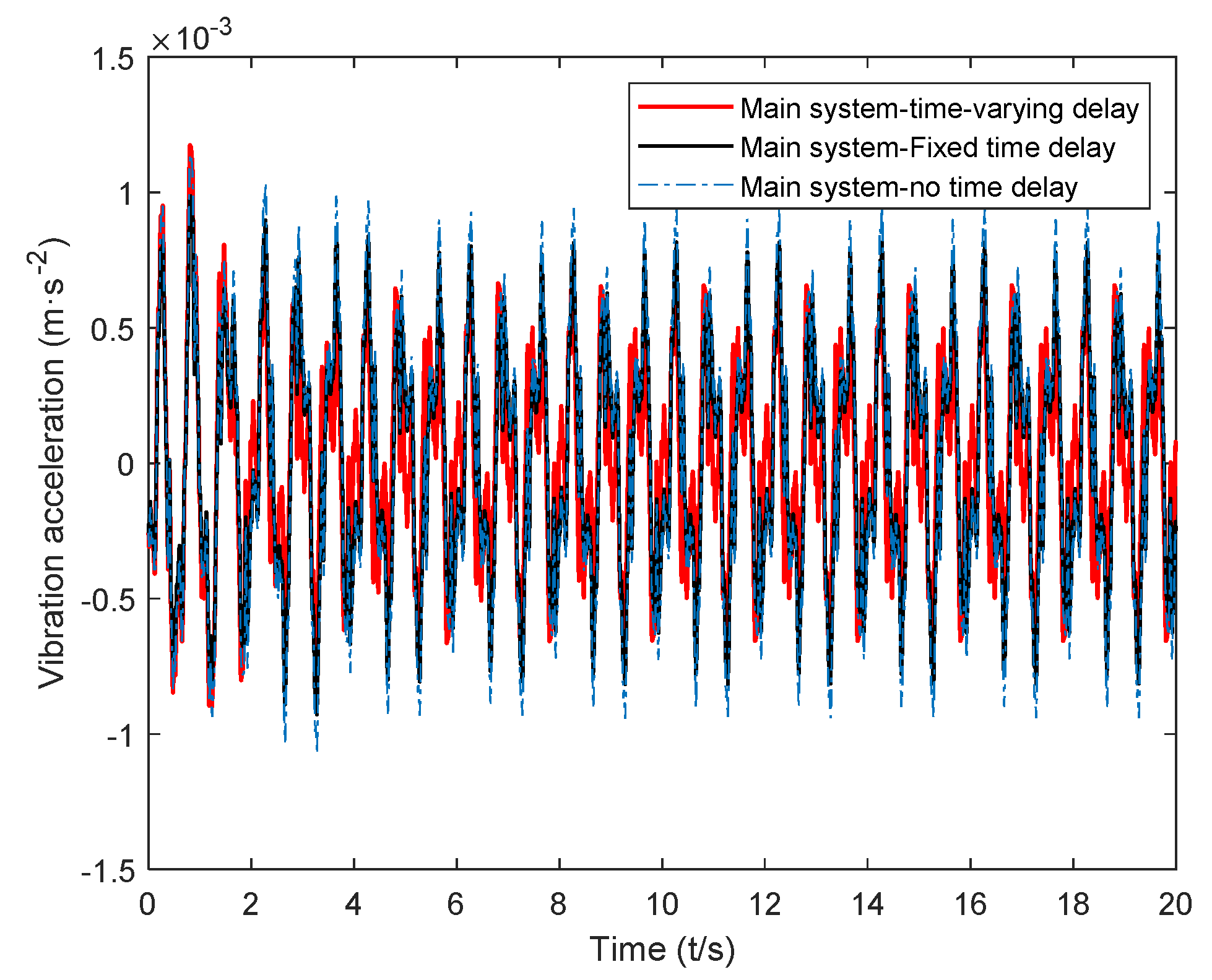
5. Time-Domain Simulation and Result Analysis
6. Conclusions
- (1)
- When the external excitation frequency ω was 5, 8, 10, 12, or 15 rad/s, the appropriate time-delay control parameters were selected. The vibration absorber could also achieve a better control effect when the external excitation deviated from the natural frequency of the vibration absorber. Time-delay control could effectively widen the effective damping bandwidth of the vibration absorber.
- (2)
- Time-varying delay feedback control could change the delay control parameters in real time according to the equivalent frequency. In the continuous time interval, the time-delay dynamic vibration absorber behaved as time-varying time-delay fixed gain control. This overcame the problem of active control requiring a large energy input.
- (3)
- The dynamic vibration absorber with time-varying delay obtained using the simple harmonic equivalent method of complex excitation was better than the dynamic vibration absorber with a fixed parameter and passive vibration absorber with time-varying delay. Compared with the passive vibration absorber, the vibration of the main system could be reduced by about 30% under complex excitation. This shows that the time-varying delay dynamic vibration absorber can reduce the vibration of the main system more effectively.
Author Contributions
Funding
Conflicts of Interest
References
- Chu, S.Y.; Song, T.T.; Lin, C.C. Time-delay effect and compensation on direct output feedback controlled mass damper systems. Earthq. Eng. Struct. Dyn. 2002, 31, 121–137. [Google Scholar] [CrossRef]
- Du, H.; Zhang, N.; Lam, J. Parameter-dependent input-delayed control of uncertain vehicle suspensions. J. Sound Vib. 2008, 317, 537–556. [Google Scholar] [CrossRef]
- Ebrahimi, B.; Tafreshi, R.; Franchek, M.; Grigoriadis, K. A dynamic feedback control strategy for control loops with time-varying delay. Int. J. Control 2014, 87, 887–897. [Google Scholar] [CrossRef]
- Gouaisbaut, F.; Dambrine, M.; Richard, J.P. Robust control of delay systems: A sliding mode control design via LMI. Syst. Control Lett. 2015, 46, 219–230. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Zhang, B.; Zhang, N.; Wang, C.Y.; Chen, Y.C. A novel reliable robust adaptive event-triggered automatic steering control approach of autonomous land vehicles under communication delay. Int. J. Robust Nonlinear Control 2021, 31, 2436–2464. [Google Scholar] [CrossRef]
- Su, H.; Tang, G.Y. Vibration reduction control of active suspension system with input time delay. Control Theory Appl. 2016, 33, 552–558. [Google Scholar]
- Qi, H.M.; Chen, Y.C.; Zhang, N.; Zhang, B.J.; Wang, D.; Tan, B.H. Improvement of both handling stability and ride comfort of a vehicle via coupled hydraulically interconnected suspension and electronic controlled air spring. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2020, 234, 552–571. [Google Scholar] [CrossRef]
- Qi, H.M.; Zhang, N.; Chen, Y.C.; Tan, B.H. A comprehensive tune of coupled roll and lateral dynamics and parameter sensitivity study for a vehicle fitted with hydraulically interconnected suspension system. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2021, 235, 143–161. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, B.J.; Chen, Y.C.; Zhang, N.; Zhang, Y. Frequency-Based Modeling of a Vehicle Fitted with Roll-Plane Hydraulically Interconnected Suspension for Ride Comfort and Experimental Validation. IEEE Access 2020, 8, 1091–1104. [Google Scholar] [CrossRef]
- Qi, H.M.; Zhang, B.J.; Zhang, N.; Zheng, M.Y.; Chen, Y.C. Enhanced Lateral and Roll Stability Study for a Two-Axle Bus via Hydraulically Interconnected Suspension Tuning. SAE Int. J. Veh. Dyn. Stab. NVH 3 2018. [Google Scholar] [CrossRef]
- Tan, B.H.; Wu, Y.; Zhang, N.; Zhang, B.J.; Chen, Y.C. Improvement of ride quality for patient lying in ambulance with a new hydro-pneumatic suspension. Adv. Mech. Eng. 2019, 11, 4. [Google Scholar] [CrossRef] [Green Version]
- Wu, Z.; Zhou, X.; Hu, D. Active vibration reduction of concrete pump truck boom based on time-delay compensation method. Adv. Mech. 2013, 24, 3283–3288. [Google Scholar]
- Olgac, N.; McFarland, D.M.; Holm-Hansen, B.T. Position feedback-induced resonance: The delayed resonator. Winter Annu. Meet. 1992, 38, 113–119. [Google Scholar]
- Valášek, M.; Olgac, N.; Neusser, Z. Real-time tunable single-degree of freedom, multiple-frequency vibration absorber. Mech. Syst. Signal Process. 2019, 133, 106244. [Google Scholar] [CrossRef]
- Olgac, N.; Jenkins, R. Time-Delayed Tuning of Vibration Absorbers for Non-collocated Suppression. In Proceedings of the IEEE 2020 American Control Conference (ACC), Denver, CO, USA, 1–3 July 2020; pp. 1381–1386. [Google Scholar]
- Olgac, N.; Hosek, M. Active vibration absorption using delayed resonator with relative position measurement. J. Vib. Acoust. 1997, 119, 131–136. [Google Scholar] [CrossRef]
- Alhazza, K.A.; Masoud, Z.N.; Alajmi, M. Nonlinear free vibration control of beams using acceleration delayed-feedback control. Smart Mater. Struct. 2008, 17, 015002. [Google Scholar] [CrossRef]
- Alhazza, K.A. Non-linear vibrations of parametrically excited cantilever beams subjected to non-linear delayed-feedback control. Int. J. Non-Linear Mech. 2008, 43, 801–812. [Google Scholar] [CrossRef]
- Alhazza, K.A.; Nayfeh, A.H.; Mohammed, F.D. On utilizing delayed feedback for active-multimode vibration control of cantilever beams. J. Sound Vib. 2009, 319, 735–752. [Google Scholar] [CrossRef]
- Mirafzal, S.H. Optimizing time delay feedback for active vibration control of a cantilever beam using a genetic algorithm. J. Vib. Control 2015, 22, 4047–4061. [Google Scholar] [CrossRef]
- Kandil, A.; El-Ganaini, W.A. Investigation of the time delay effect on the control of rotating blade vibrations. Eur. J. Mech.-A/Solids 2018, 72, 16–40. [Google Scholar] [CrossRef]
- Shao, S.; Masri, K.M.; Younis, M.I. The effect of time-delayed feedback controller on an electrically actuated resonator. Nonlinear Dyn. 2013, 74, 257–270. [Google Scholar] [CrossRef]
- Saeed, N.A.; Moatimid, G.M.; Elsabaa, F.M. Time-Delayed Nonlinear Integral Resonant Controller to Eliminate the Nonlinear Oscillations of a Parametrically Excited System. IEEE Access 2021, 9, 74836–74854. [Google Scholar] [CrossRef]
- Wang, Z.H.; Hu, H.Y. Stability and bifurcation of time-delay dynamic systems: From theory to application. Mech. Prog. 2013, 43, 3–20. [Google Scholar]
- Hu, H.Y.; Wang, Z.H. Research progress of nonlinear time-delay dynamic systems. Adv. Mech. 1999, 29, 501–512. [Google Scholar]
- Chen, L.X.; Cai, G.P. Experimental study on active control of a rotating flexible beam with time-delay. Chin. J. Theor. Appl. Mech. 2008, 40, 520–527. [Google Scholar]
- Chen, L.X.; Cai, G.P. Experimental study on time-delay variable structure control of a flexible beam under forced vibration. Chin. J. Theor. Appl. Mech. 2009, 41, 410–417. [Google Scholar]
- Zhao, Y.Y.; Xu, J. Vibration reduction mechanism of time-delay nonlinear dynamic vibration absorber. Chin. J. Theor. Appl. Mech. 2008, 40, 98–106. [Google Scholar]
- Zhang, X.X.; Xu, J. Modelling and Tuning for the Time-delayed Vibration Absorber with Friction. J. Sound Vib. 2018, 424, 137–157. [Google Scholar] [CrossRef]
- Chen, Y.C.; Mendoza, A.; Todd, D. Experimental and numerical study of high-order complex curvature mode shape and mode coupling on a three-bladed wind turbine assembly. Mech. Syst. Signal Process. 2021, 160, 3. [Google Scholar] [CrossRef]
- Chen, Y.C.; Peter, A.; Christopher, P.; Jacob, D. A polynomial based dynamic expansion and data consistency assessment and modification for cylindrical shell structures. Mech. Syst. Signal Process. 2021, 154, 107574. [Google Scholar] [CrossRef]
- Chen, Y.C.; Dagny, J.; Peter, A. Underwater dynamic response at limited points expanded to full-field strain response. J. Vib. Acoust. 2018, 140, 5. [Google Scholar] [CrossRef] [Green Version]
- Han-Kwan, D.; Iacobelli, M. From Newton’s second law to Euler’s equations of perfect fluids. Proc. Am. Math. Soc. 2021, 149, 3045–3061. [Google Scholar] [CrossRef]
- Zhang, Y.L. Reconstruction of a time-domain model of random road irregularity elevation with harmonic superposition method. Trans. Chin. Soc. Agric. Eng. 2003, 19, 32–35. [Google Scholar]
- Zhong, W.X. Precise time integration method for structural dynamic equations. J. Dalian Univ. Technol. 1994, 34, 131–136. [Google Scholar]
- Zhong, W.X. The fine calculation method of transient history. Comput. Mech. 1995, 12, 1–6. [Google Scholar]
- Ren, C.B.; He, G.Z.; Li, Z.F. A high-precision general calculation format for structural dynamics fine integration. Mech. Sci. Technol. Aerosp. Eng. 2005, 24, 1507–1509. [Google Scholar]
- Tian, X.T.; Ren, C.B. Precise integration method for linear time-delay dynamics. J. Shandong Univ. Technol. 2006, 2, 53–56. [Google Scholar]
- Xu, J.J.; Huang, Y.H.; Wang, Q.; Chen, H. Reactive power optimization of distribution network with DG access based on natural selection particle swarm optimization. Electr. Meas. Instrum. 2014, 51, 33–88. [Google Scholar]

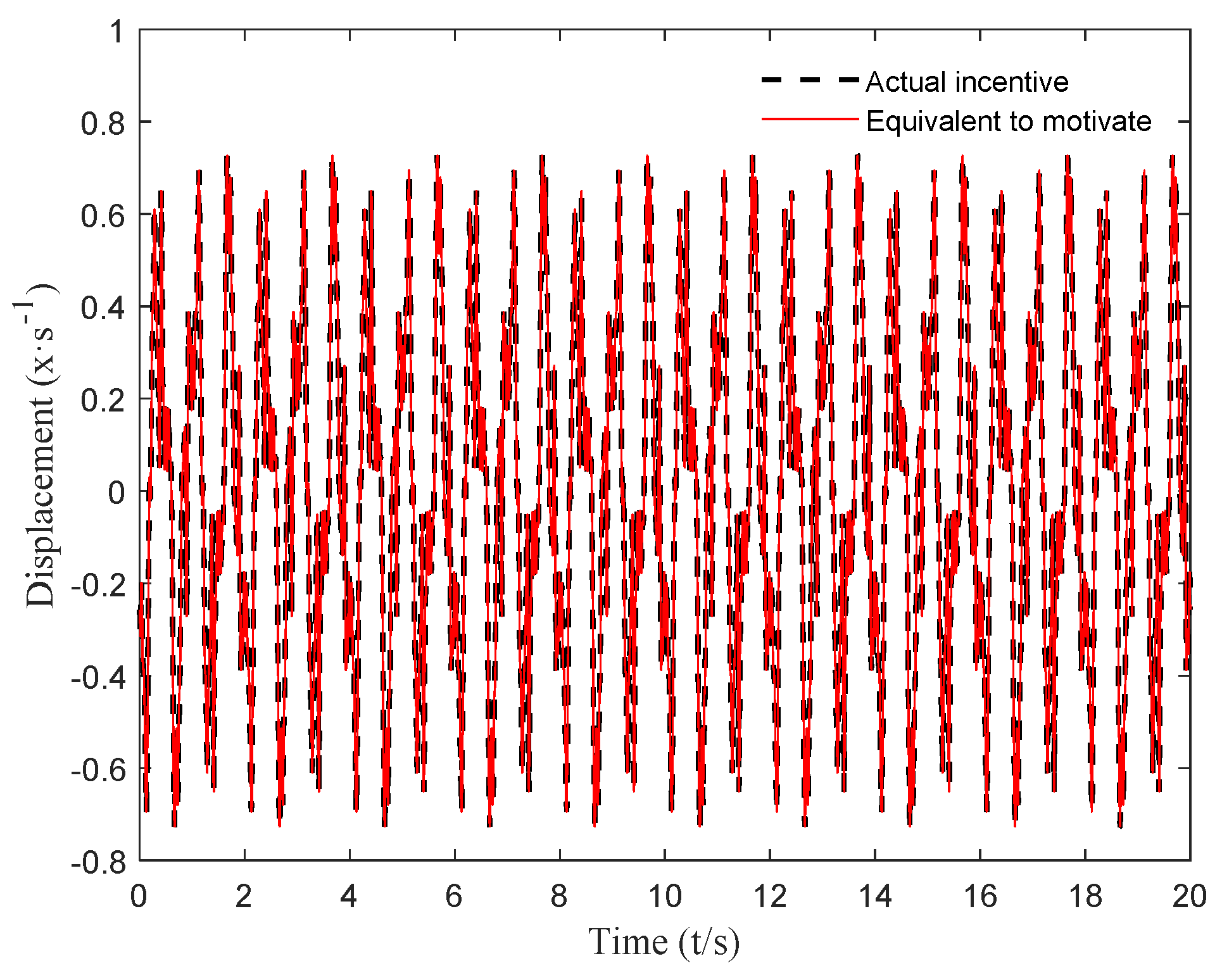
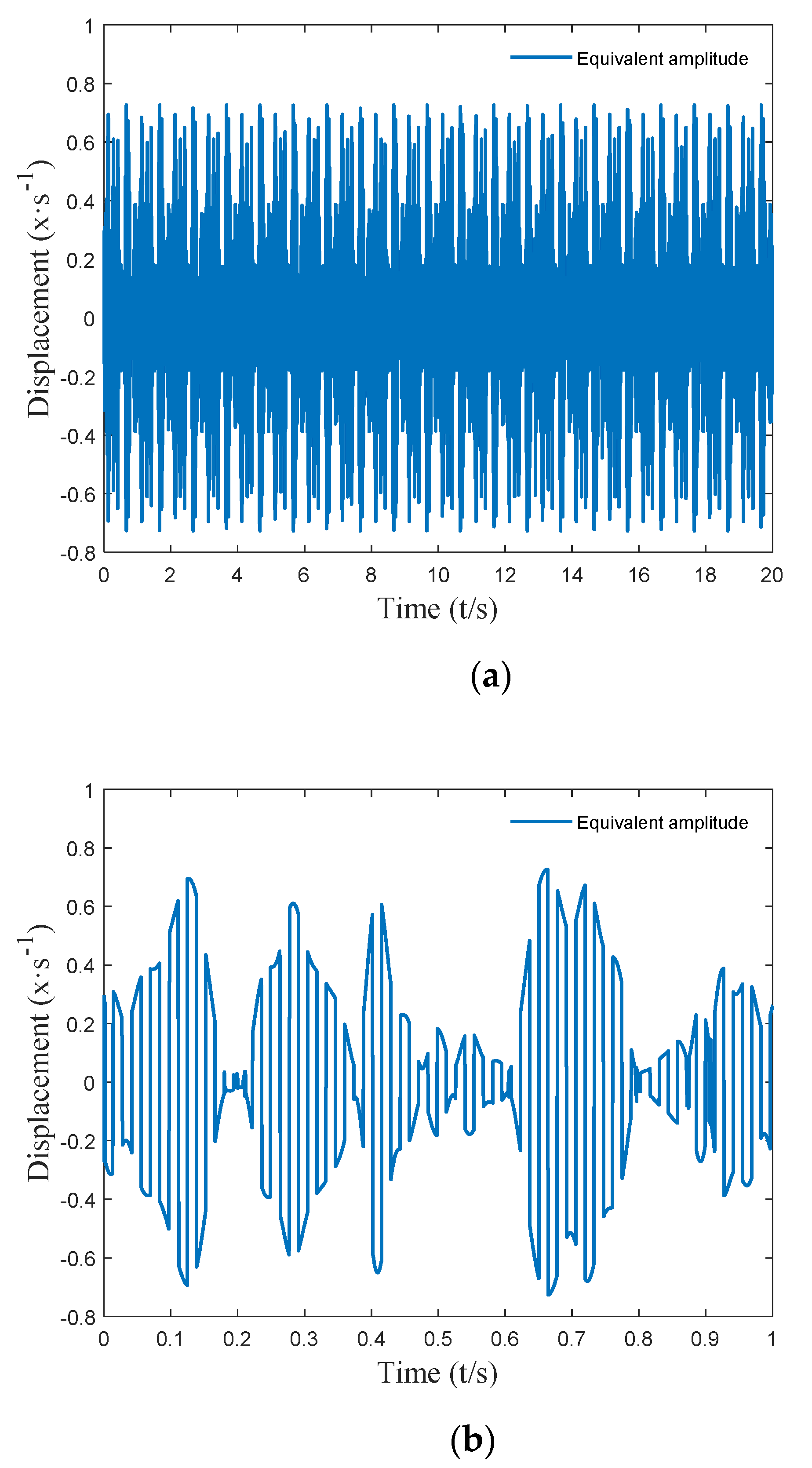
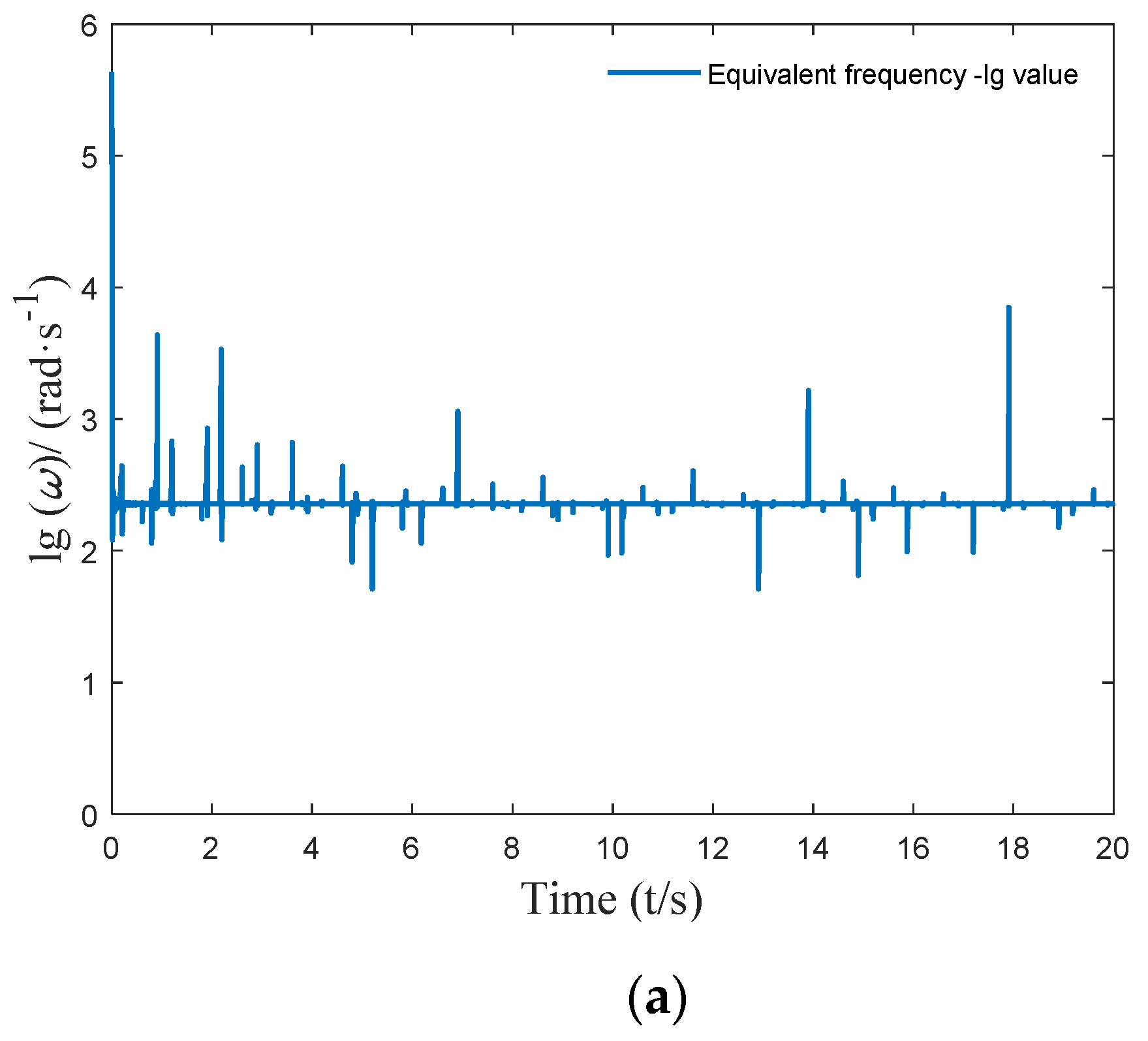
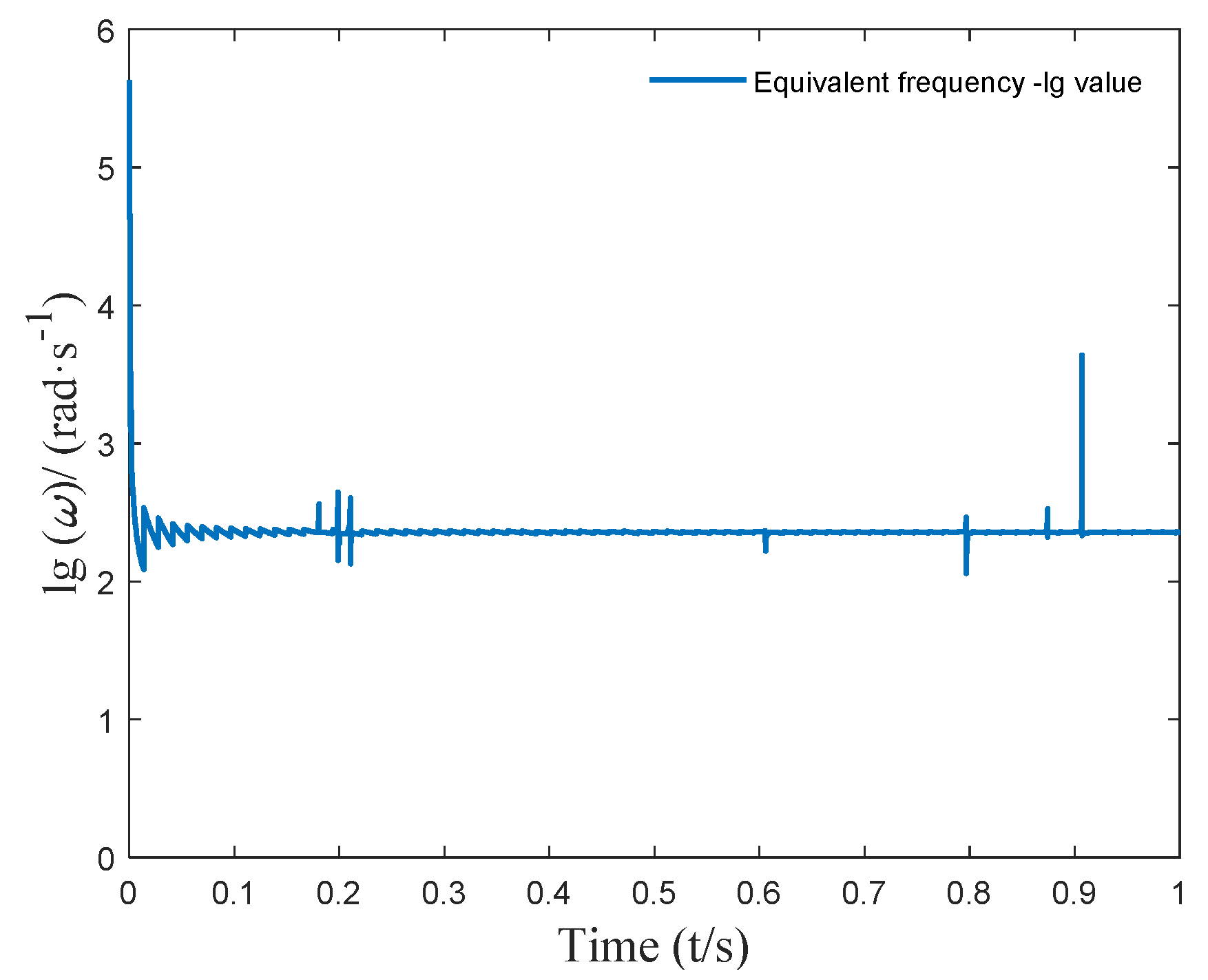


| ma (kg) | m (kg) | ka (N/m) | k (N/m) | ca (N·s/m) | c (N·s/m) |
|---|---|---|---|---|---|
| 0.1 | 1 | 10 | 100 | 0.1 | 2 |
| RMS | Passive Absorber | Fixed Time-Delay Absorber | Time-Varying Delay Absorber | Vibration Reduction Efficiency of Time-Varying Delay Absorber Relative to Passive Absorber | Fixed Time-Delay Absorber Relative to Passive Absorber |
|---|---|---|---|---|---|
| RMS value of main system displacement (m) | 0.0470 | 0.0355 | 0.0308 | 34.58% | 24.53% |
| RMS value of main system velocity (m/s) | 0.4503 | 0.3348 | 0.3006 | 33.25% | 25.65% |
| RMS value of main system acceleration (m/s2) | 0.2174 | 0.1882 | 0.1690 | 22.26% | 13.45% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, K.; Ren, C.; Chen, Y.; Shao, S.; Zhou, J.; Ma, C.; Li, L. Vibration Control of Time-Varying Delay under Complex Excitation. Micromachines 2021, 12, 1081. https://doi.org/10.3390/mi12091081
Wu K, Ren C, Chen Y, Shao S, Zhou J, Ma C, Li L. Vibration Control of Time-Varying Delay under Complex Excitation. Micromachines. 2021; 12(9):1081. https://doi.org/10.3390/mi12091081
Chicago/Turabian StyleWu, Kaiwei, Chuanbo Ren, Yuanchang Chen, Sujuan Shao, Jilei Zhou, Chicheng Ma, and Lin Li. 2021. "Vibration Control of Time-Varying Delay under Complex Excitation" Micromachines 12, no. 9: 1081. https://doi.org/10.3390/mi12091081
APA StyleWu, K., Ren, C., Chen, Y., Shao, S., Zhou, J., Ma, C., & Li, L. (2021). Vibration Control of Time-Varying Delay under Complex Excitation. Micromachines, 12(9), 1081. https://doi.org/10.3390/mi12091081








