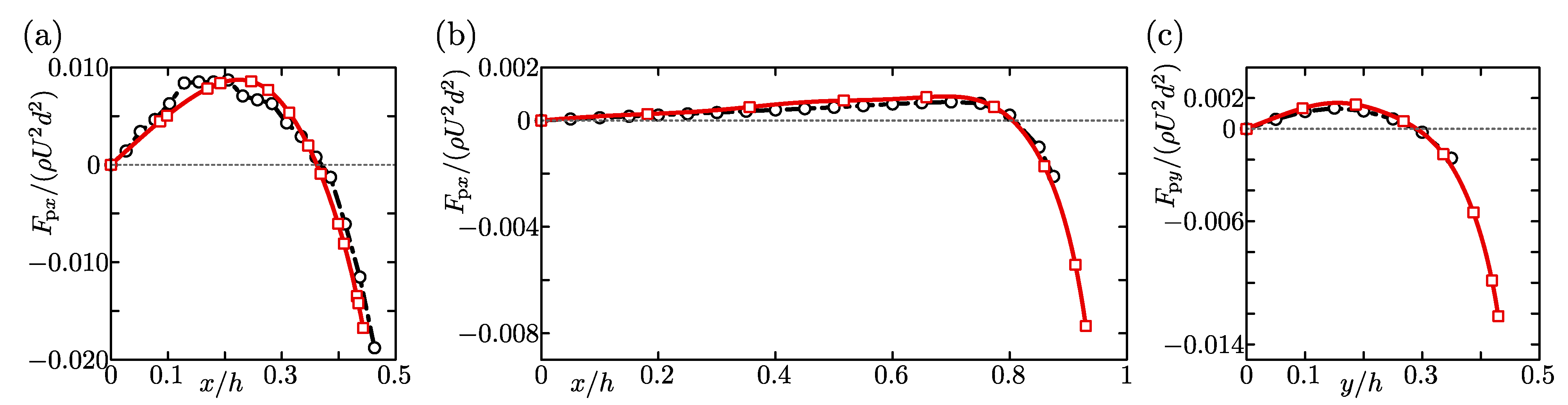
3.1. Two Types of Particle-Focusing Pattern for and
The inertial-focusing phenomena of a neutrally buoyant spherical particle suspended in rectangular duct flows were numerically investigated for the aspect ratio
, various blockage ratios from
to
and above, and Reynolds numbers from 50 to 400. In this section, we report our numerical results for
and
, as a representative example.
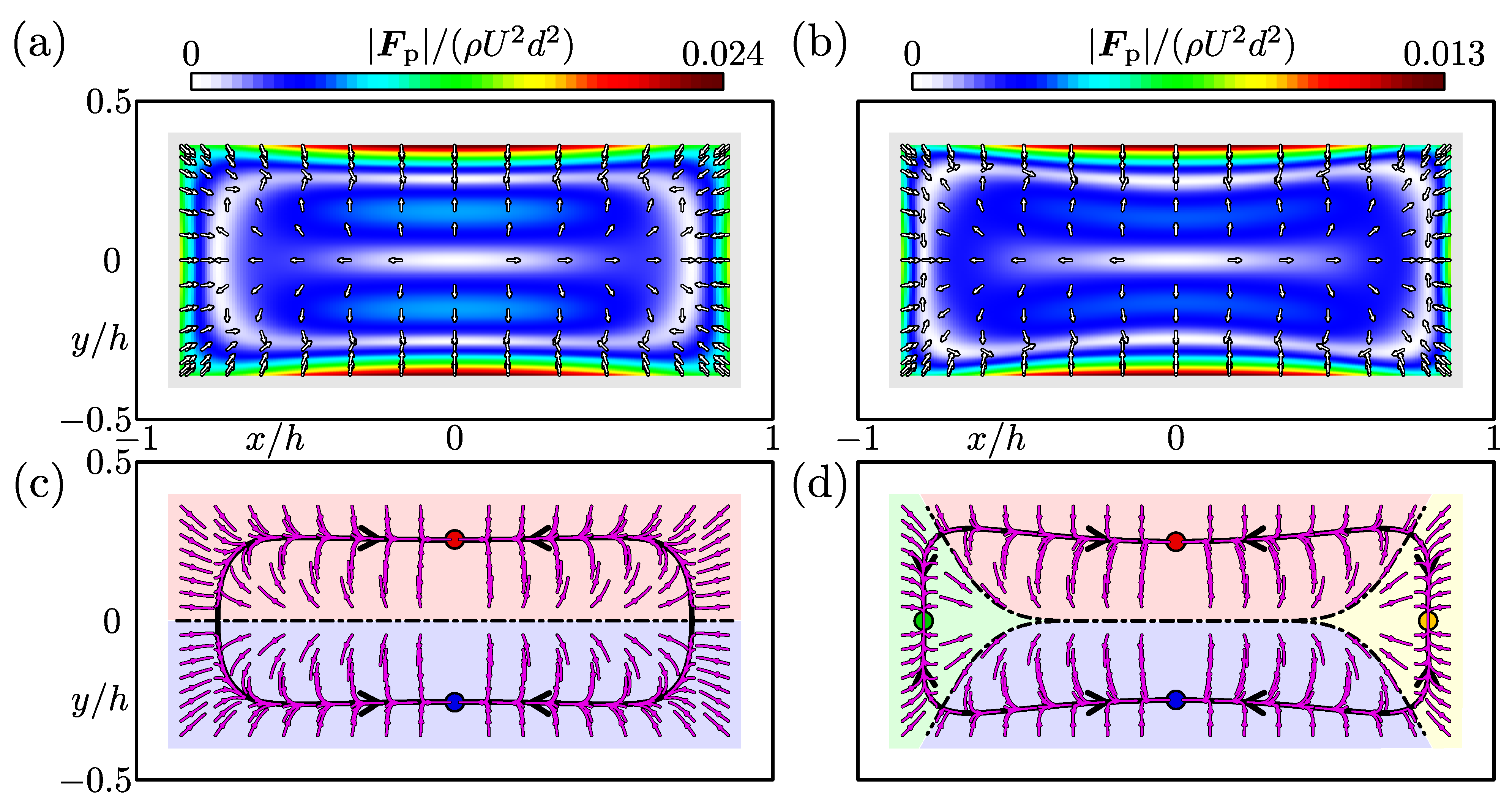
Figure 3a,b show the lift maps for the representative Reynolds numbers
and 200, respectively. The calculated lift forces are depicted with arrows, and their magnitudes are shown in graded colours in the background. It can be seen from both
Figure 3a,b that regardless of the Reynolds numbers, the particles near the side walls experience lift pointing in the opposite direction to the wall, and the lift forces acting on a particle located near the centre of the cross-section are directed towards the side walls.
The particle trajectories estimated from the lift map for
(
Figure 3a) are shown in
Figure 3c. Curves with arrows represent the trajectories of the particle centre. This figure indicates the two-stage migration property of particles suspended in a duct flow, which are well-known features of the inertial migration of suspended particles [
9,
13]. Firstly, particles starting from various positions in the duct cross-section move towards the thick black trajectories depicted in
Figure 3c. The closed curve consisting of the thick black particle trajectories correspond to the Segré–Silberberg annulus appeared in suspension flows through a circular pipe, and is, therefore, named “pseudo Segré–Silberberg (pSS) ring” [
9]. Secondly, particles migrate along the pSS ring and settle at certain points. In this case of
Figure 3c, it is found that particle-focusing points exist near the centres of the long sides of the duct cross-section, as indicated by circles. The dash-dotted line on the
x-axis in
Figure 3c represents the separatrix for the regions in terms of particle-focusing points. The particles initially located inside the region of
(red region) and
(blue region) focus on the points drawn in red and blue, respectively.
Figure 3d shows the particle trajectories and the focusing points obtained for
. The trajectories estimated from the lift map shown in
Figure 3b indicate that the pSS ring is slightly deformed compared to that for
, and there are particle-focusing points near the centres of not only the long sides but also the short sides of the cross-section. It was found that the focusing pattern of suspended particles for
is different from that for
. The existence region of the particle centre, shown in grey in
Figure 3b, is divided into four regions by the separatrices. Suspended particles initially located inside each coloured region focus at the position plotted by circles with the corresponding colour. Our calculation suggests that it is relatively difficult to experimentally observe the focusing points near the short sides because the green and yellow regions are smaller than the red and blue regions.
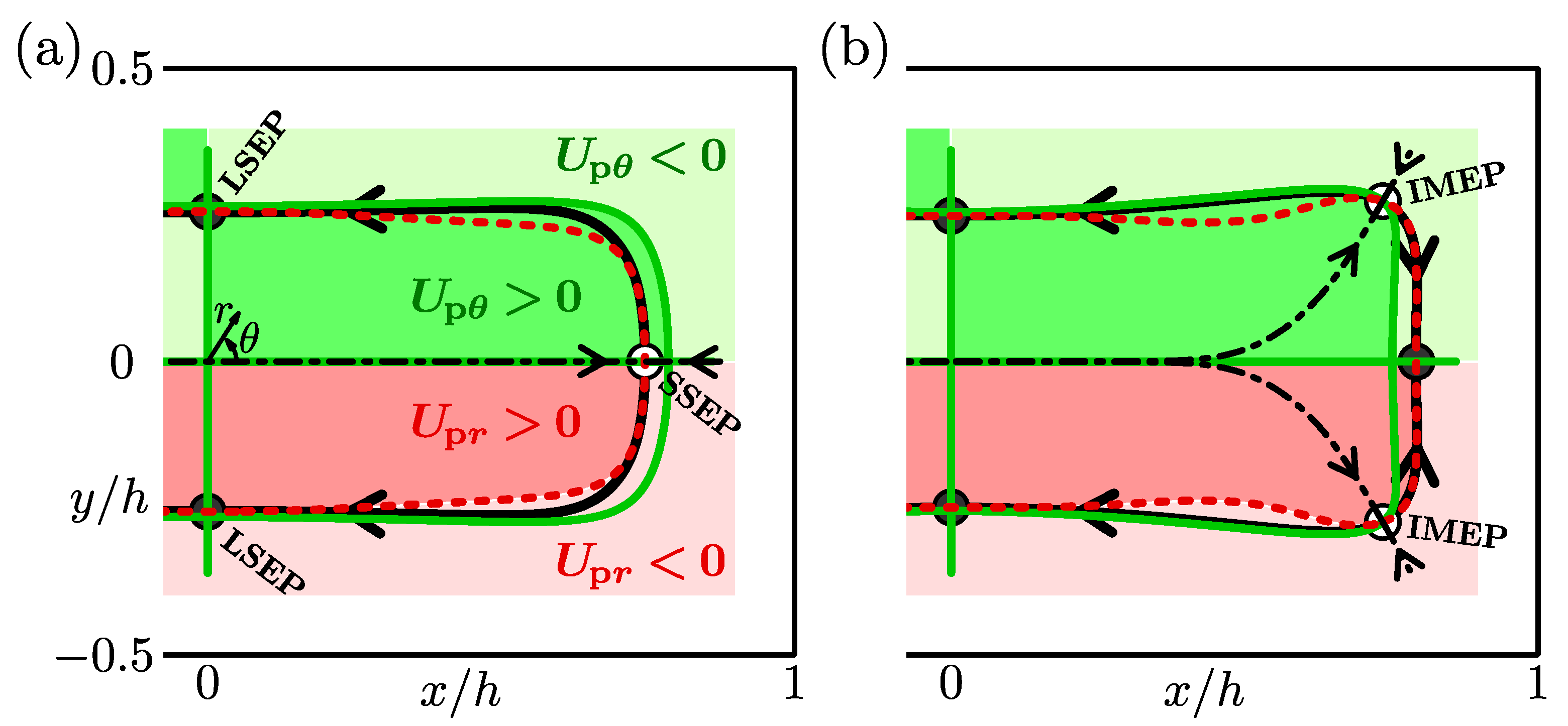
We have shown two types of particle-focusing patterns at
and 200 for the aspect ratio
and blockage ratio
. To catch the structure of the transition between these two patterns, the nullclines of the particle trajectories for
and 200 are illustrated in
Figure 4a,b, respectively. We determined the nullclines of the radial and circumferential components of the particle velocity estimated based on the lift-force map and Stokes drag by interpolating the map.
In
Figure 4, the red dashed curve shows the zero-level set contour of the radial component of the particle velocity
, which is named “
r-nullcline” in the present study. In both
Figure 4a,b,
is positive inside the
r-nullcline, whereas it is negative between the nullcline and the side walls. This primarily determines the feature of the first stage for the migration property of suspended particles—particles move towards the pSS ring.
The green solid curve (closed curve in the entire cross-section) and the
x- and
y-axes represent the zero-level set contour of the circumferential component of the particle velocity
. We call this contour “
-nullcline”. The cross-section is divided into eight regions by the
-nullcline, and the sign of
changes between adjacent regions, as in the case of the
r-nullcline. In the first quadrant of the cross-section, the circumferential component of the particle velocity
is positive (anticlockwise) inside the
-nullcline (green regions in
Figure 4), whereas
is negative (clockwise) outside it (light green region). This is related to the second stage of the migration property—particles move along the pSS ring and settle at certain points.
The intersections between the nullclines of radial and circumferential motions of the particle, at which
is satisfied, correspond to the particle equilibrium points because we assume that the particle velocity is proportional to the lift acting on a particle (see Equation (
18)). In
Figure 4a, for the case of
, the
r-nullcline and the
x-axis (the
-nullcline) intersect. This equilibrium point plotted by an open circle is called “Short Side Equilibrium Point (SSEP)” in the present study. The
r-nullcline also crosses the
y-axis (the
-nullcline). We call this intersection depicted by a filled circle “Long Side Equilibrium Point (LSEP)”. The pSS ring (thick solid curve) consists of heteroclinic orbits joining the SSEP to the LSEP. Arrows depicted on the separatrix (dash-dotted line) and pSS ring indicate that the SSEP is a saddle equilibrium position, whereas the LSEP is stable. Therefore, the particles focus near the centres of the long sides and do not focus near the short sides of the duct cross-section when
as shown in
Figure 3c. Note that the centre of the cross-section is also the equilibrium point. We have found that this point is always unstable; that is, the particles do not focus at the duct centre in the Re range investigated in our study.
The nullclines for
are shown in
Figure 4b.
Figure 4b indicates that the particle equilibrium points appear not only on the
x- and
y-axes but also near the duct corners. The equilibrium points near the corner (open circles) is named “Intermediate Equilibrium Point (IMEP)”. The emergence of the IMEP is caused by the slight deformation of the nullclines, depending on Re. The
r-nullcline is located inside the curved
-nullcline along the side walls when
; that is, they do not intersect (see
Figure 4a). As Re increases, both the nullclines are slightly deformed, and a part of the
r-nullcline near the short side lies outside the curved
-nullcline. Therefore, the nullclines intersect near the duct corners and the IMEP emerges. The pSS ring for
consists of heteroclinic orbits connecting the IMEP to the LSEP and the IMEP to the SSEP. The separatrices and pSS ring indicate that the IMEP is a saddle equilibrium point and the LSEP is a stable node. It was also found that the SSEP is changed to be a stable node. At
, the heteroclinic orbit in the first quadrant of the cross-section is located in the
region (green region in
Figure 4a) so that the SSEP is a saddle equilibrium point. In contrast, the SSEP is stable when
because the heteroclinic orbit joining the IMEP to the SSEP lies in the
region (light green region in
Figure 4b).
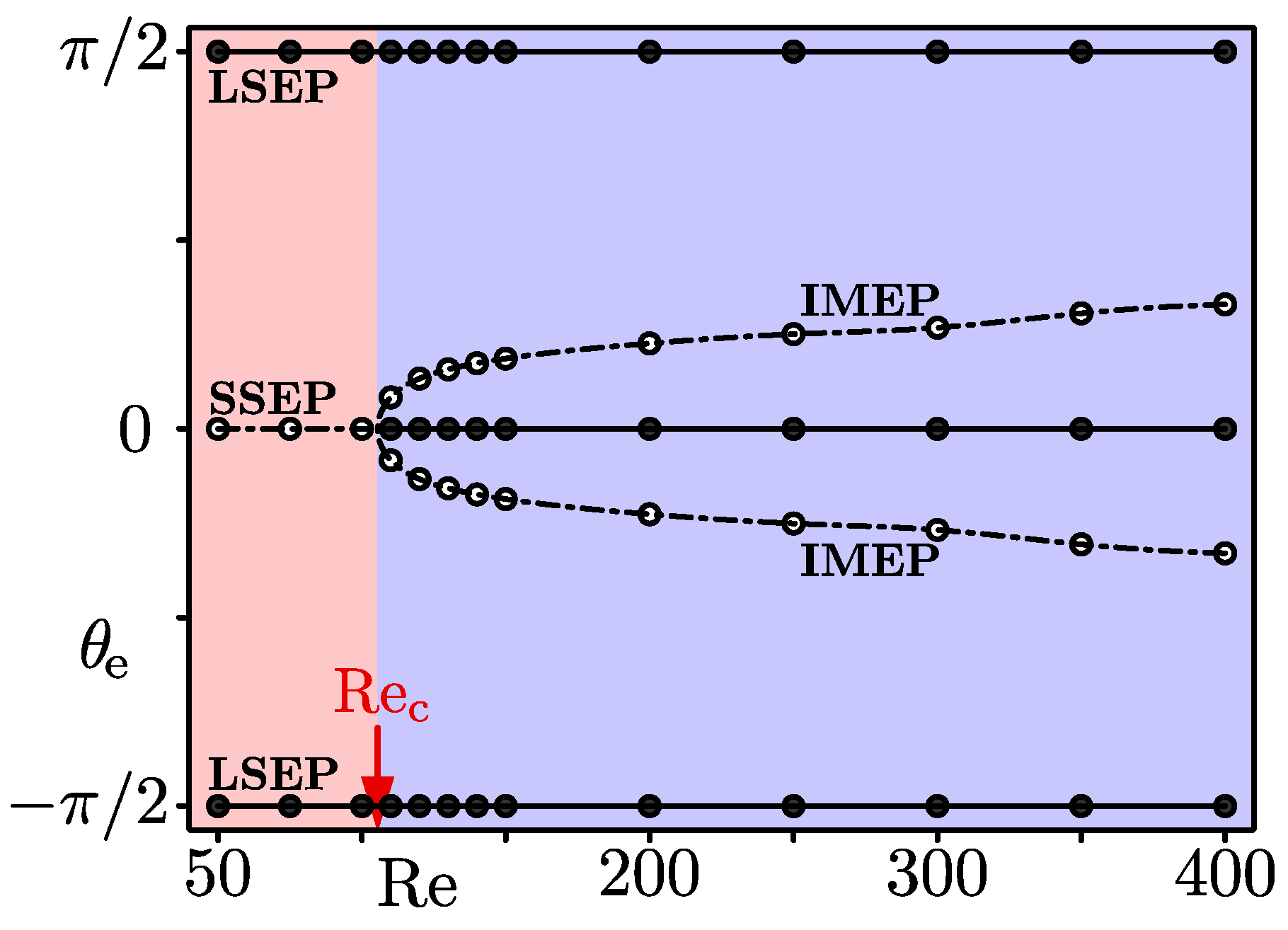
Figure 5 shows the changes, depending on the Re, of the particle equilibrium points appeared in the first and fourth quadrants of the duct cross-section, that is, in the
region. We plotted the azimuthal angle of the equilibrium point
as the vertical axis in this diagram. The equilibrium point
corresponds to the SSEP, and
are the LSEPs, and the others are the IMEP. Open circles and dash-dotted lines represent saddle equilibrium points. Filled circles and solid lines represent stable equilibrium points where suspended particles are focused. The diagram indicates that the particles focus at the centres of the long sides (the LSEP) in the Re region with red, whereas they focus at the centres of both the long and short sides (the LSEP and the SSEP) when the Re value is in the blue region. The critical Reynolds number
, which corresponds to the border between the red and blue regions, represents the Re value when the transition of the particle-focusing patterns occurs. Our computation showed that the
is greater than 100 and less than 110. This value is comparable to the previous estimate
for
and
by Liu et al. [
15]
Our calculation suggests that the pattern transition on the inertial particle focusing for rectangular duct flows for
and
is explained as a subcritical pitchfork bifurcation. When
, the
r-nullcline lies inside the curved
-nullclines along the walls, as shown in
Figure 4a, so that there are the stable LSEP and the saddle SSEP in the cross-section. At
, the
r-nullcline and the curved
-nullcline touch each other on the
x-axis near the short sides. When Re exceeds
, a part of the
r-nullcline near the short sides is outside the curved
-nullcline, and the SSEP changes from a saddle point to a stable node together with the emergence of the saddle IMEP.
In addition to the case of , we performed numerical computations for other blockage ratios at . As far as we examined in the rage of , it was inferred that the transition of the particle focusing pattern with Re has the same structure with that for , i.e., pitchfork bifurcation from the focusing on the LSEP at low Re to that on the LSEP and SSEP at elevated Re.
3.2. Three Types of Particle-Focusing Pattern for and
For
, we have found another focusing pattern between the two focusing regimes reported in the previous section. As a representative example,
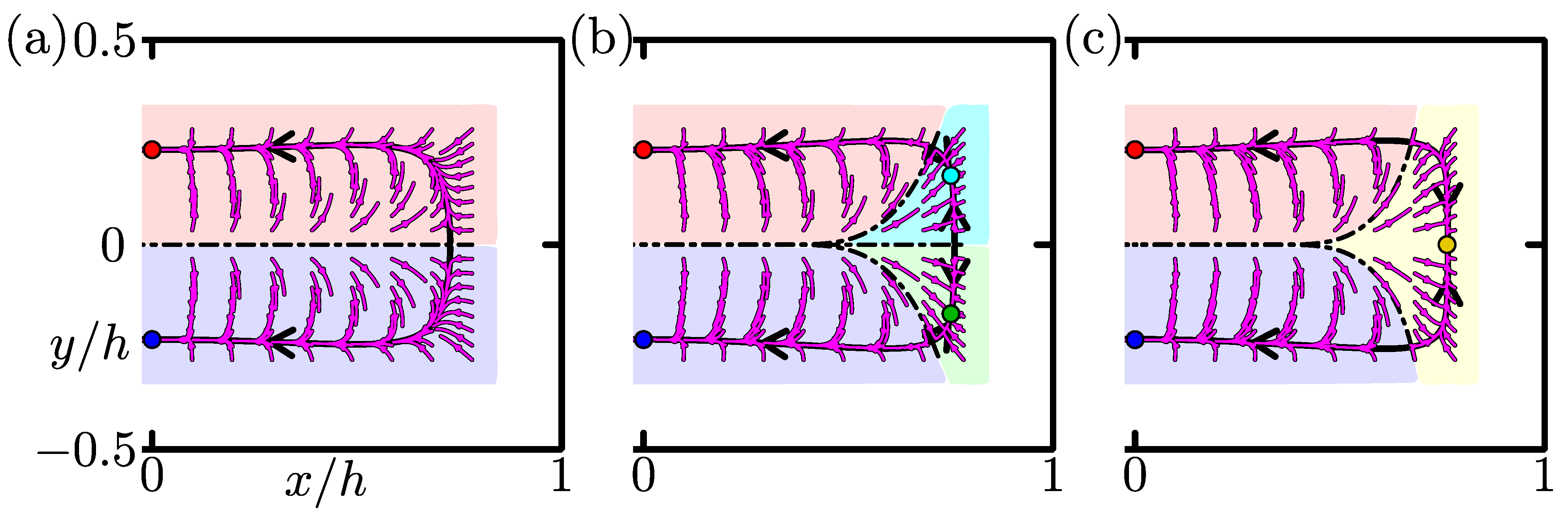
Figure 6,
Figure 7 and
Figure 8 show our numerical results for
and
, corresponding to
Figure 3,
Figure 4 and
Figure 5, respectively. In this case, the focusing patterns at a low Re (=100,
Figure 6a) and at an elevated Re (=300,
Figure 6c) are similar to those for
at
and 200, respectively. That is, particles focus on the LSEP at
, and they focus on both the LSEP and SSEP at
. At
between these Re values, there is a new type of particle focusing pattern as shown in
Figure 6b, where particle focusing points are on the LSEP and IMEP near the duct corners. The particle focusing on the IMEP emerges due to crossings of the
r-nullcline and
-nullcline near the corner as shown in the inset of
Figure 7b. As a result of these crossings, two IMEPs (IMEP
and IMEP
in the inset) appear near the long side and short side of the cross-section. The IMEP
is unstable (saddle point) but the IMEP
is stable, judging from the particle trajectories shown in
Figure 6b. To the authors’ knowledge, this is the first time report of non-LSEP and non-SSEP particle focusing in rectangular duct flows, except for square duct flows. In square duct flows, particle focusing on the IMEP has been observed experimentally and the experimental results were reproduced by numerical simulations [
9,
10]. Furthermore, the particle focusing on the IMEP was accounted for by crossing of the
r-nullcline and
-nullcline, similar to the present study [
10]. Our preliminary experiments for rectangular duct flows also indicated the particle focusing on the IMEP for
and
, although parameter values are different from the present case [
18]. In
Figure 7b, relative positions of the
r-nullcline and
-nullcline near the SSEP and LSEP are the same with those at low Re (
Figure 7a), leading to that their stabilities are the same with those at low Re. Thus, the particle focusing positions are on the LSEP and IMEP at
.
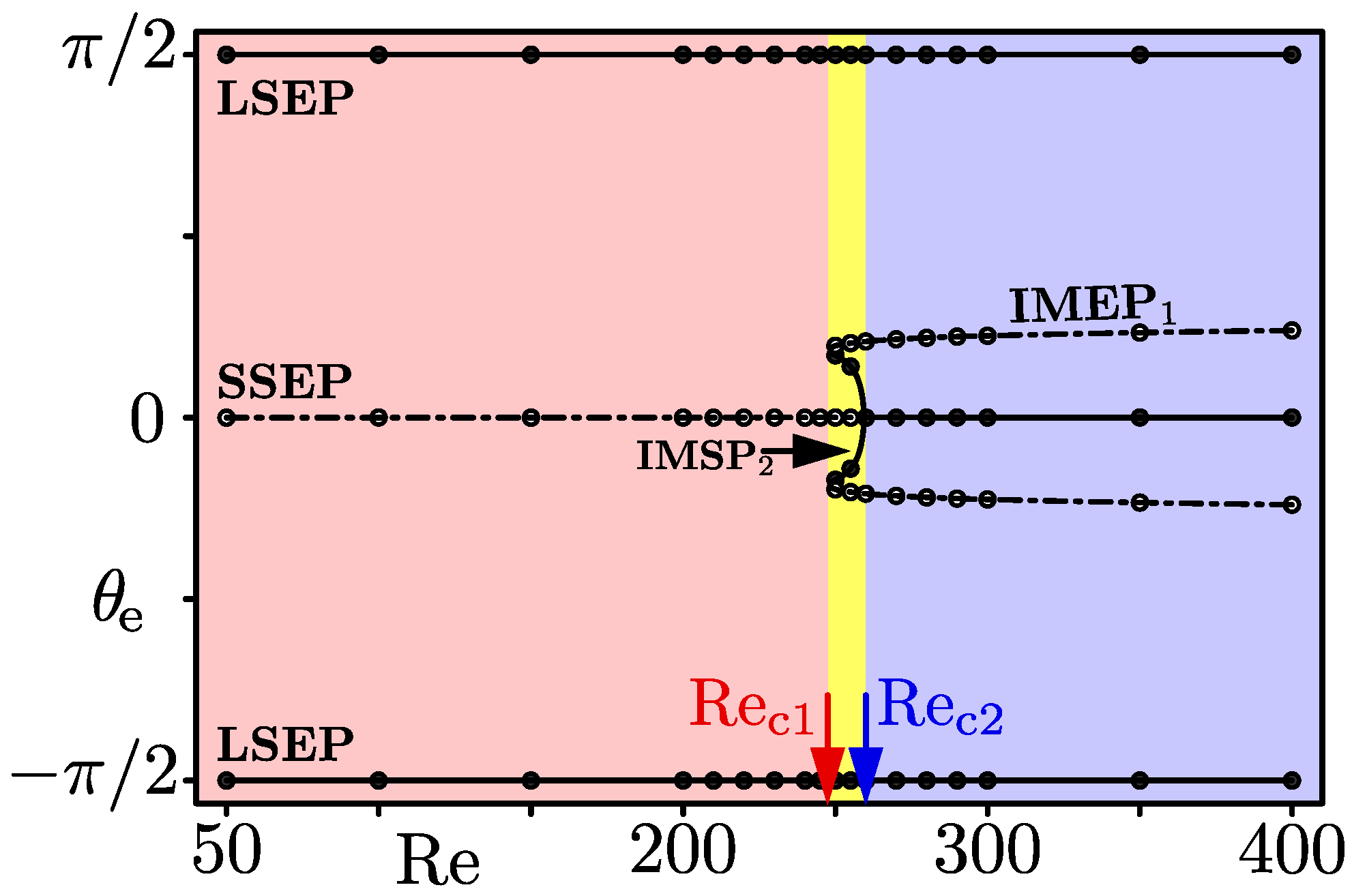
Figure 8 shows a phase diagram of the particle focusing for
and
. There are two critical Re,
and
, between adjacent regimes. At
, a saddle-node bifurcation occurs, where the
r-nullcline and
-nullcline touch each other. At
, a supercritical pitchfork bifurcation occurs, where the stable IMEP (IMEP
) coincides with the SSEP. To summarise the case of
and
, the LSEP is stable but the SSEP is a saddle for
; stabilities of the LSEP and SSEP are unchanged but stable and unstable (saddle) IMEPs emerge for
; the stable IMEP disappears and the SSEP becomes stable for
.
We showed that for
, the transition structure changes depending on whether the blockage ratio is larger or smaller than about
. Harding et al. [
12] predicted by a perturbation expansion method that particles would focus on the LSEP and SSEP at low Re for
, suggesting the extinction of the regime where particles focus only on the LSEP. Consequently, there may be another transition structure for small blockage ratios for
. Although more detailed study is necessary to elucidate these properties in a wider range of the blockage ratio, we have found that the present method of analysis in terms of
r-nullcline and
-nullcline is critical to determine the particle focusing position and to trace the variation of the focusing pattern with parameter values. In addition, the bifurcation diagram in terms of the azimuthal angle of particle equilibrium points is appropriate for intuitive visualisation of the change of the transition structure. The variations in a wide range of
are currently under study and the results will be reported in the near future.