Enhancing the Electromechanical Coupling in Soft Energy Harvesters by Using Graded Dielectric Elastomers
Abstract
:1. Introduction
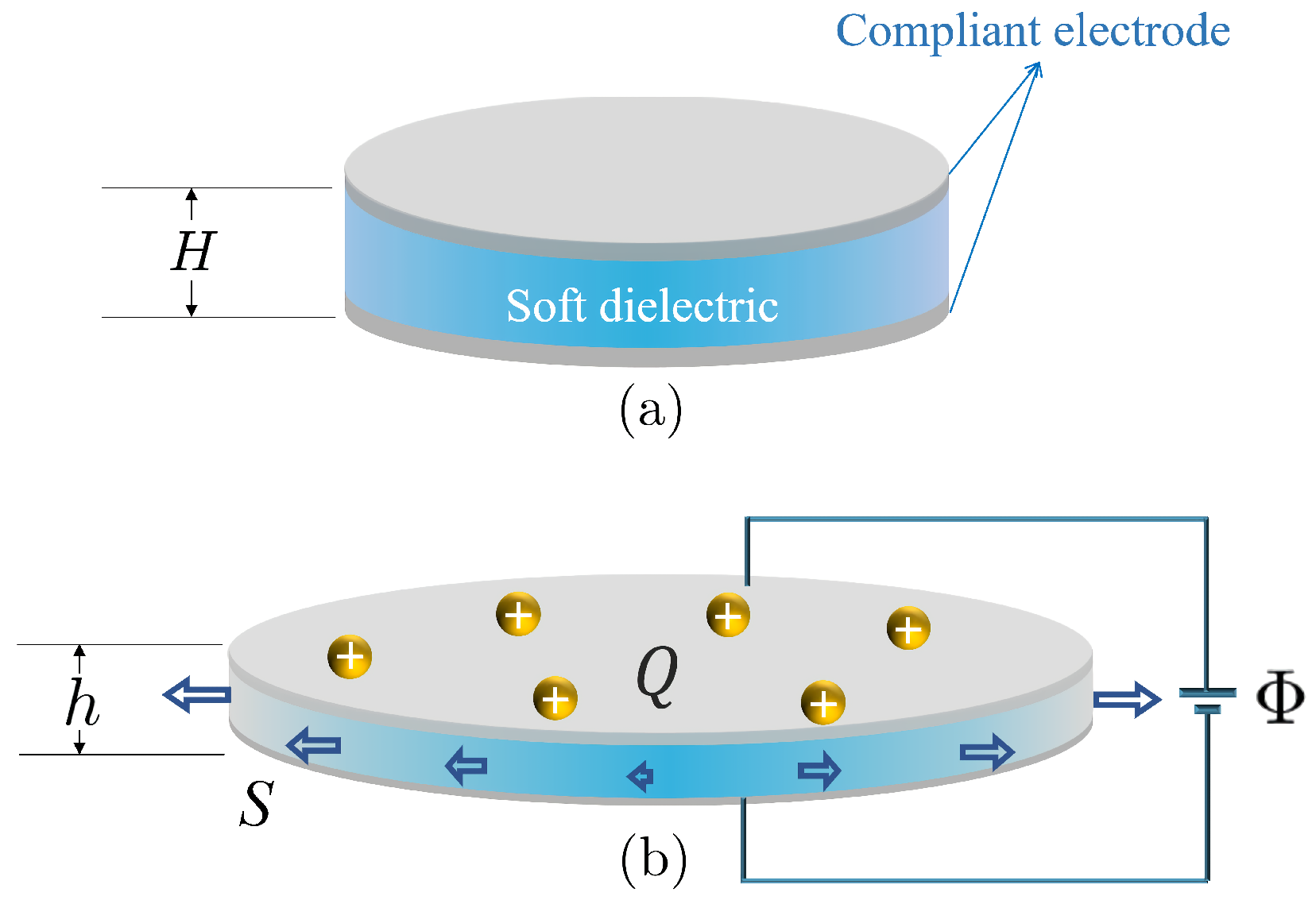
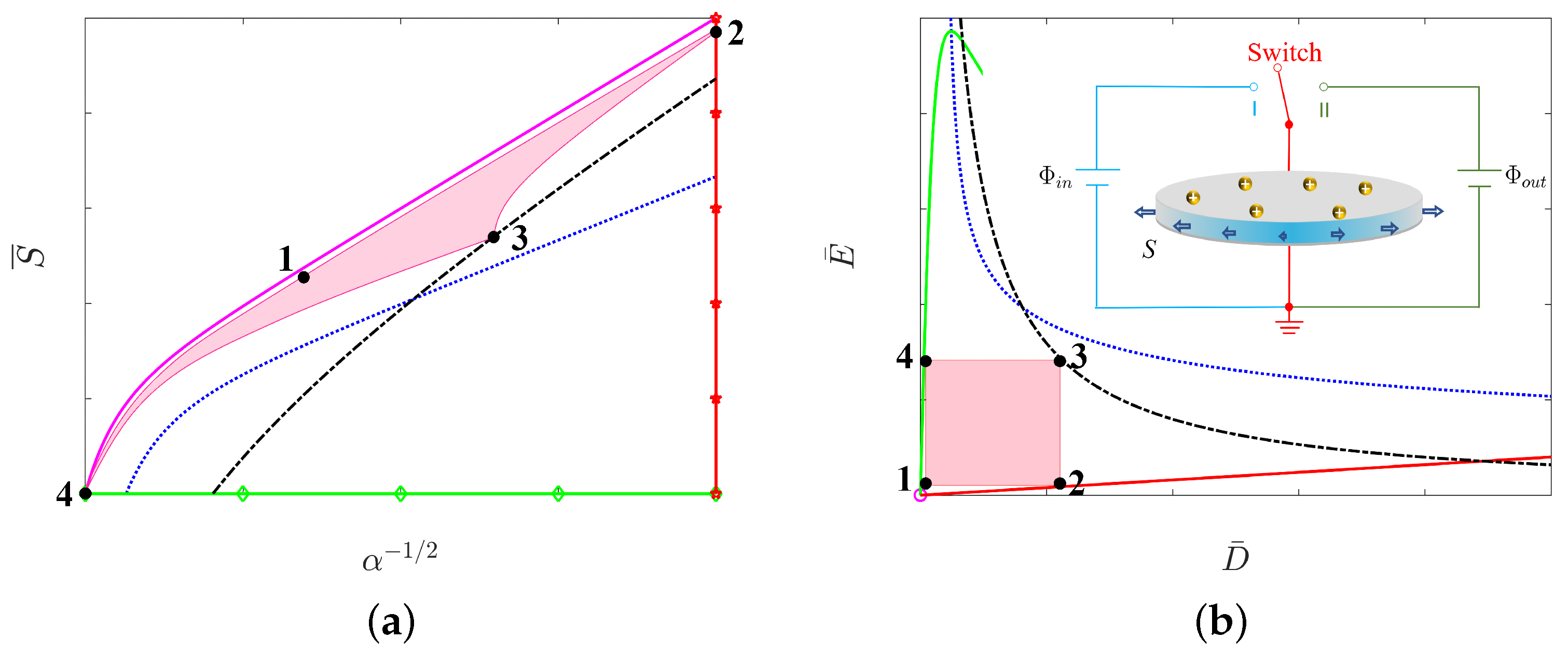
2. A Typical Energy Harvester of Dielectric Elastomers
3. Electromechanical Couplings in a Circular Film of Homogeneous Dielectric Elastomers
3.1. Equilibrium States
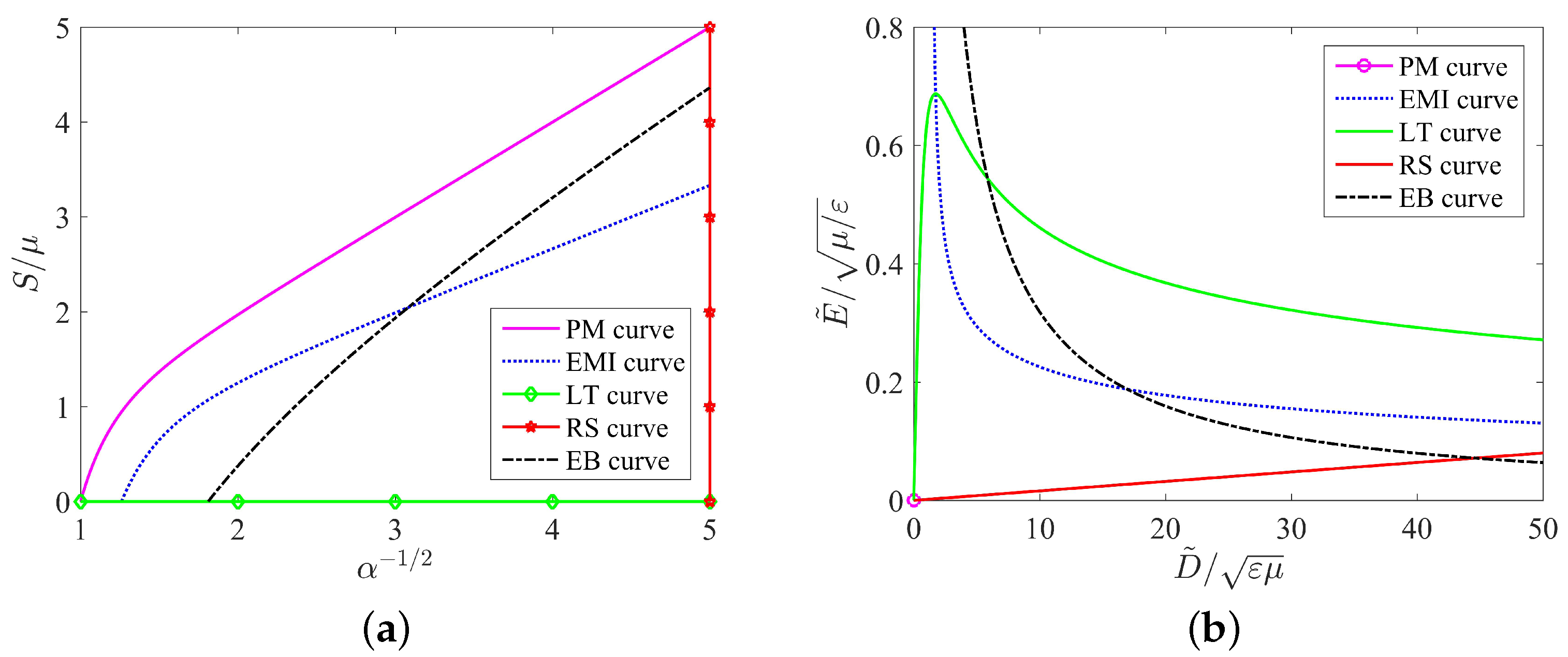
3.2. Four Modes of Failure
- Electromechanical instability (EMI): When the electromechanical loads reach the threshold, the condition for the onset of electromechanical instability is as follows [31]:
- Loss of tension (LT): When the nominal stress S in (2) becomes zero, it is the so-called state of loss of tension, and the following is the case
- Rupture by stretch (RS): The film ruptures when the in-plane stretch , i.e., , reaches a critical value, and the following is the caseUsually, the critical stretch for rupture in the experiment [19] of equal biaxial stretch is suggested as . In this paper, we chose the same value as that used in the work [32] in order to show how the graded modulus affects energy conversion. Note that the stretch here is governed by the equilibrium Equations (2) and (3).
- Electric breakdown (EB): When the true electric field , i.e., , reaches a critical value (), the dielectric film accompanies the occurrence of electric breakdown, and the following is the caseBased on the existing experiments [10], the critical electric field for the onset of EB is chosen as . Other material parameters used in the numerical calculations are and , as well as the mass density .
3.3. In-Plane Stress vs. Stretch Curves
3.4. Nominal Electric Field vs. Nominal Electric Displacement Curves
- (1)
- Purely mechanical (PM) curve: By setting in (3), the PM curve corresponds to the origin of the vs. plane, i.e., .
- (2)
- (3)
- (4)
- (5)
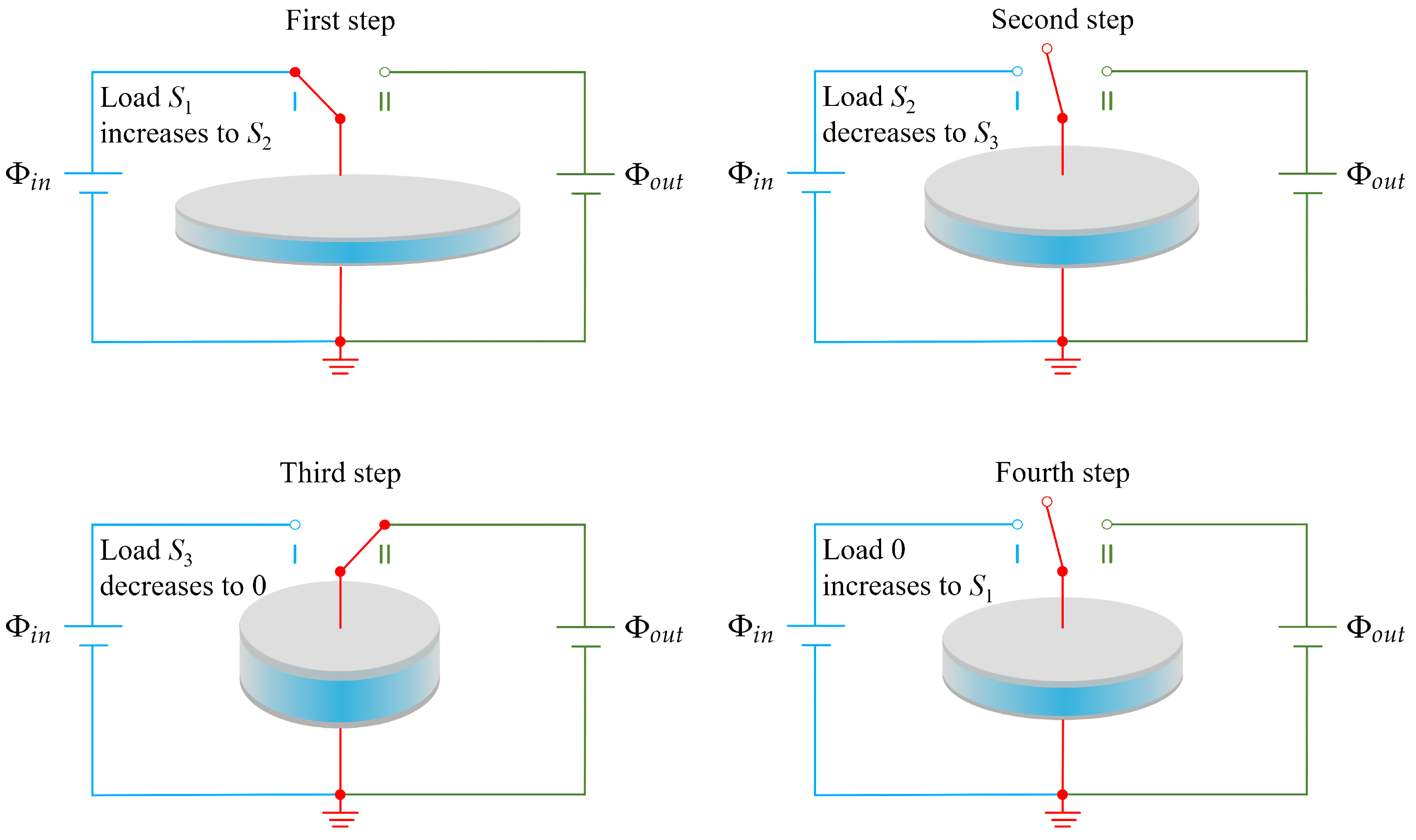
3.5. Energy of Conversion
4. Electromechanical Couplings in a Circular Film of Graded Dielectric Elastomers
4.1. Equilibrium Equation and Four Modes of Failure
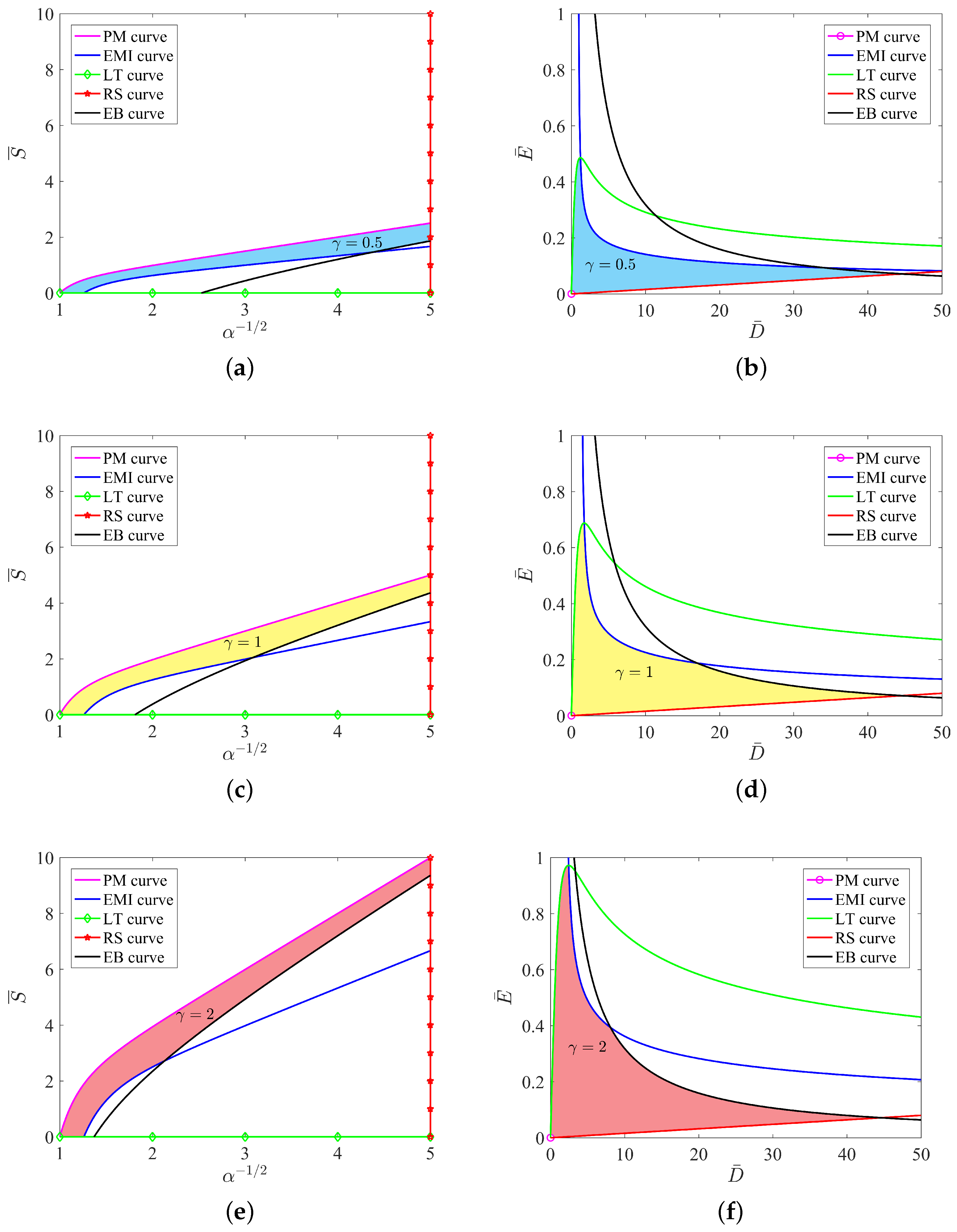
4.2. Curves on the Two Planes
4.3. Scaling
4.3.1. Dimensionless Equations of Equilibrium and Four Modes of Failure
4.3.2. Dimensionless Equations of Five Curves
4.4. Energy of Conversion
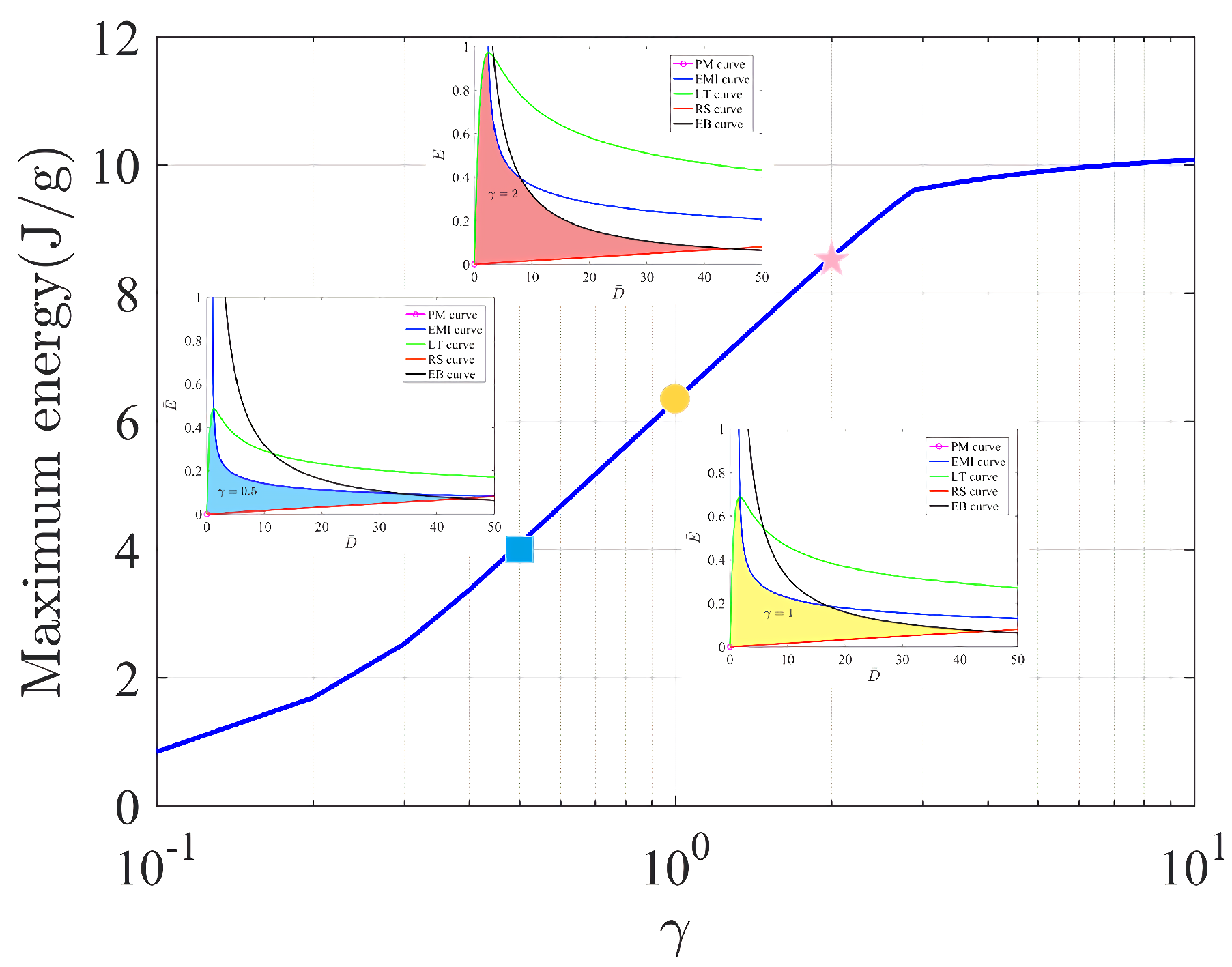
5. Results and Discussion
5.1. Specific Energy and Output Voltage
5.2. Maximum Energy
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Priya, S. Modeling of electric energy harvesting using piezoelectric windmill. Appl. Phys. Lett. 2005, 87, 42. [Google Scholar] [CrossRef]
- Anton, S.R.; Sodano, H.A. A review of power harvesting using piezoelectric materials. Smart Mater. Struct. 2007, 16, R1. [Google Scholar] [CrossRef]
- Sodano, H.A.; Simmers, G.E.; Dereux, R.; Inman, D.J. Recharging Batteries Using Energy Harvested from Thermal Gradients. J. Intell. Mater. Syst. Struct. 2006, 18, 3–10. [Google Scholar] [CrossRef]
- Liao, Y.; Sodano, H.A. Model of a single mode energy harvester and properties for optimal power generation. Smart Mater. Struct. 2008, 17, 065026. [Google Scholar] [CrossRef]
- Zhu, M.; Worthington, E.; Tiwari, A. Design study of piezoelectric energy-harvesting devices for generation of higher electrical power using a coupled piezoelectric-circuit finite element method. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 427–437. [Google Scholar] [PubMed]
- Song, H.C.; Kim, H.C.; Kang, C.Y.; Kim, H.J.; Yoon, S.J.; Jeong, D.Y. Multilayer piezoelectric energy scavenger for large current generation. J. Electroceramics 2009, 23, 301–304. [Google Scholar] [CrossRef]
- Guan, M.; Liao, W.H.; Clark, W.W.; Ahmadian, M.; Lumsdaine, A. On the energy storage devices in piezoelectric energy harvesting. In Proceedings of the Spie the International Society for Optical Engineering, San Diego, CA, USA, 26 February–2 March 2006; p. 6169. [Google Scholar]
- Deng, Q.; Kammoun, M.; Erturk, A.; Sharma, P. Nanoscale flexoelectric energy harvesting. Int. J. Solids Struct. 2014, 51, 3218–3225. [Google Scholar] [CrossRef] [Green Version]
- Wang, B.; Yang, S.; Sharma, P. Flexoelectricity as a universal mechanism for energy harvesting from crumpling of thin sheets. Phys. Rev. B 2019, 100, 035438. [Google Scholar] [CrossRef]
- Pelrine, R.; Kornbluh, R.; Pei, Q.; Joseph, J. High-speed electrically actuated elastomers with strain greater than 100%. Science 2000, 287, 836–839. [Google Scholar] [CrossRef]
- Rui, Z.; Lochmatter, P.; Kovacs, G.; Kunz, A.; Conti, F. Dielectric Elastomers as Electromechanical Transducers; Fundamentals, Materials, Devices, Models and Applications ofan Emerging Electroactive Polymer Technology; Elsevier Science: Oxford, UK, 2008. [Google Scholar]
- Rus, D.L.; Tolley, M.T. Design, fabrication and control of soft robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef] [Green Version]
- Kofod, G.; Wirges, W.; Paajanen, M.; Bauer, S. Energy minimization for self-organized structure formation and actuation. Appl. Phys. Lett. 2007, 90, 081916. [Google Scholar] [CrossRef]
- Anderson, I.A.; Gisby, T.A.; Mckay, T.G.; OBrien, B.; Calius, E.P. Multi-functional dielectric elastomer artificial muscles for soft and smart machines. J. Appl. Phys. 2012, 112, 041101. [Google Scholar] [CrossRef]
- Zhao, X.; Wang, Q. Harnessing large deformation and instabilities of soft dielectrics: Theory, experiment, and application. Appl. Phys. Rev. 2014, 1, 021304. [Google Scholar] [CrossRef] [Green Version]
- Invernizzi, F.; Dulio, S.; Patrini, M.; Guizzetti, G.; Mustarelli, P. Energy harvesting from human motion: Materials and techniques. Chem. Soc. Rev. 2016, 45, 5455–5473. [Google Scholar] [CrossRef]
- Chen, F.; Liu, K.; Wang, Y.; Zou, J.; Gu, G.; Zhu, X. Automatic Design of Soft Dielectric Elastomer Actuators with Optimal Spatial Electric Fields. IEEE Trans. Robot. 2019, 35, 1150–1165. [Google Scholar] [CrossRef] [Green Version]
- Chen, F.; Wang, M.Y. Design Optimization of Soft Robots: A Review of the State of the Art. IEEE Robot. Autom. Mag. 2020, 27, 27–43. [Google Scholar] [CrossRef]
- Plante, J.S.; Dubowsky, S. Large-scale failure modes of dielectric elastomer actuators. Int. J. Solids Struct. 2006, 43, 7727–7751. [Google Scholar] [CrossRef] [Green Version]
- Keplinger, C.; Li, T.; Baumgartner, R.; Suo, Z.; Bauer, S. Harnessing snap-through instability in soft dielectrics to achieve giant voltage-triggered deformation. Soft Matter 2012, 8, 285–288. [Google Scholar] [CrossRef]
- Zhao, X.; Suo, Z. Method to analyze electromechanical stability of dielectric elastomers. Appl. Phys. Lett. 2007, 91, 061921. [Google Scholar] [CrossRef]
- Wang, Q.; Suo, Z.; Zhao, X. Bursting drops in solid dielectrics caused by high voltages. Nat. Commun. 2012, 3, 1157. [Google Scholar] [CrossRef]
- Cheng, S.; Lu, T.; An, L.; Wang, F.; Wang, T. Experimental investigation of the electromechanical phase transition in a dielectric elastomer tube. Smart Mater. Struct. 2015, 24, 035006. [Google Scholar]
- Liu, X.; Li, B.; Chen, H.; Jia, S.; Zhou, J. Voltage-induced wrinkling behavior of dielectric elastomer. J. Appl. Polym. Sci. 2016, 133. [Google Scholar] [CrossRef]
- Yang, S.; Zhao, X.; Sharma, P. Avoiding the pull-in instability of a dielectric elastomer film and the potential for increased actuation and energy harvesting. Soft Matter 2017, 13, 4552–4558. [Google Scholar] [CrossRef]
- Yang, S.; Zhao, X.; Sharma, P. Revisiting the instability and bifurcation behavior of soft dielectrics. J. Appl. Mech. 2017, 84, 031008. [Google Scholar] [CrossRef] [Green Version]
- Su, Y.; Broderick, H.C.; Chen, W.; Destrade, M. Wrinkles in soft dielectric plates. J. Mech. Phys. Solids 2018, 119, 298–318. [Google Scholar] [CrossRef] [Green Version]
- Dorfmann, L.; Ogden, R.W. Instabilities of soft dielectrics. Philos. Trans. R. Soc. Math. Phys. Eng. Sci. 2019, 377, 20180077. [Google Scholar] [CrossRef] [Green Version]
- Zurlo, G.; Destrade, M.; DeTommasi, D.; Puglisi, G. Catastrophic thinning of dielectric elastomers. Phys. Rev. Lett. 2017, 118, 078001. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.; Yang, X.; Wang, B.; Yang, S.; Dayal, K.; Sharma, P. The interplay between symmetry-breaking and symmetry-preserving bifurcations in soft dielectric films and the emergence of giant electro-actuation. Extrem. Mech. Lett. 2021, 43, 101151. [Google Scholar] [CrossRef]
- Chen, L.; Yang, X.; Wang, B.; Yang, S. Nonlinear electromechanical coupling in graded soft materials: Large deformation, instability, and electroactuation. Phys. Rev. E 2020, 102, 023007. [Google Scholar] [CrossRef]
- Koh, S.J.A.; Zhao, X.; Suo, Z. Maximal energy that can be converted by a dielectric elastomer generator. Appl. Phys. Lett. 2009, 94, 262902. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Yang, S. Enhancing the Electromechanical Coupling in Soft Energy Harvesters by Using Graded Dielectric Elastomers. Micromachines 2021, 12, 1187. https://doi.org/10.3390/mi12101187
Chen L, Yang S. Enhancing the Electromechanical Coupling in Soft Energy Harvesters by Using Graded Dielectric Elastomers. Micromachines. 2021; 12(10):1187. https://doi.org/10.3390/mi12101187
Chicago/Turabian StyleChen, Lingling, and Shengyou Yang. 2021. "Enhancing the Electromechanical Coupling in Soft Energy Harvesters by Using Graded Dielectric Elastomers" Micromachines 12, no. 10: 1187. https://doi.org/10.3390/mi12101187
APA StyleChen, L., & Yang, S. (2021). Enhancing the Electromechanical Coupling in Soft Energy Harvesters by Using Graded Dielectric Elastomers. Micromachines, 12(10), 1187. https://doi.org/10.3390/mi12101187





