1. Introduction
Liquid droplets are utilized in many different ways in a wide variety of applications [
1]. Their use is especially suitable for biochemical analysis, as only a small amount of volume is required to perform the required task [
2,
3].
In this work, we present a facile setup, which is capable of parallel droplet deposition with precise droplet volume (dosage). The core functionality is based on evaporation of droplets resting on a superhydrophobic hole plate, the concept of which we recently introduced [
4,
5]. The system can be fabricated using simple methods and is highly parallelizable, allowing for large-scale droplet deposition. In Tröls et al.’s research [
4], the working principle was first introduced where evaporation was not aided by heating of the substrate. Measurements were performed with different diameters of the holes of the dosage plate showing the applicability of the underlying principles. Also, considerations of droplet shape during transition through the hole and the dependence of droplet mass on the hole diameter were presented. In addition to the previously reported setup, here, microheaters are implemented on the bottom surface of the device, in order to accelerate the dosing process. Research concerning the evaporation of sessile droplets on heated substrates revealed that the decrease in total evaporation time due to the heating follows a power law trend [
6,
7]. Assuming similar conditions are present with our setup, a significant reduction in evaporation time can be expected due to the implementation of the microheaters. Also, resistance temperature detectors (RTD) are deposited on the top surface to monitor the temperature during the entire application process. Preliminary results regarding the use of screen printed microheaters were presented in Hintermüller et al.’s research [
5]. The additional implementation of temperature sensors directly on the dosage plate allows for a more reliable measurement of the temperature of the plate. This aids the quantification of the reduction in total evaporation time depending on temperature and allows comparison to similar findings in literature.
The proposed system was introduced for the potential application of coating of microneedle arrays for transdermal drug delivery systems by exact droplet deposition [
4]. Here, the surface of the microneedles needs to be coated with a precise amount of the drug. Simply dip-coating the microneedle array may lead to patchy coverage [
8], and other methods such as electrohydrodynamic atomization, ink-jet printing, and jet- and spray drying require special and often expensive equipment [
9,
10,
11].
When using microtiter plates, for example, for enzyme-linked immunosorbent arrays (ELISA) [
12,
13], parallel and precise dosing is also required. Instead of using multichannel pipettes, the proposed system may be utilized for this task by directly depositing the required liquid volume as a droplet into the wells.
So-called dispensed droplet systems require deposition of sessile droplets in a well-ordered manner on a substrate to create a planar droplet array [
14,
15,
16]. Here, the proposed setup may also be advantageous to replace the manual deposition of droplets with a predefined volume.
In the current state, the presented system is comparable to conventional pipettes. When working with, for example, microwell plates, using single channel pipettes can be tedious, as the same operation must be performed hundreds of times. Multichannel pipettes improve that situation but are often less accurate and precise. Also, these conventional systems are prone to human error, as it is not uncommon to lose track during repetitions [
17]. Such errors not only cost time, but also the sample material is wasted, which might be scarce. This can be improved by using an automated liquid handling system, as such a system can perform many pipetting steps in a short amount of time, operating with robotic precision. Unfortunately, such systems are usually very expensive. The proposed setup is an alternative to the aforementioned systems. Its fabrication is simple, as well as cost effective, and it works semi-automatic, helping to reduce human error, while the dosing performance is comparable to the methods mentioned above.
With the evolution of microfluidics, the employed mechanisms were also utilized for pipetting and dosing methods. Huang et al. developed a hybrid polydimethylsiloxane (PDMS)-glass chip with a series of pneumatic valves to perform precise dosing processes [
18]. A collection reservoir was filled by repeatedly combining one of three predefined volumes that could be set with the device. After achieving the desired dosage in the reservoir, it could be dispensed for further usage. More recently, an improved version of a standard micropipette was reported [
19,
20]. Standard pipettes become increasingly unreliable at volumes below 1 µL, featuring a precision of about 20% or worse [
20]. Researchers modified a standard micropipette and combined it with a microfluidic PDMS chip, featuring a flexible membrane. After filling the pipette cartridge, an electromagnetic actuator pushes on the PDMS membrane to release a defined dosage. Using this approach, precision was improved to about 5% in the range of 100 nL–1000 nL. Additionally, the system is programmable, helping to reduce human error.
Over the past years, the analysis of single cells became an important topic in cell biology. Therefore, there was a demand for single-cell dispensation systems. In Yusof and Gross et al.’s researches [
21,
22], a system for single-cell dispensation was presented. It consisted of a microfluidic dispenser chip, which was filled with fluid and cells through an inlet. Through piezo actuation (similar to a regular inkjet printer) on a deformable silicon membrane, picoliter-sized droplets were generated. An optical system at the end of the nozzle of the dispenser chip was able to detect if a single cell was contained inside the droplet. If so, the droplet was dispensed into a microtiter plate; if not, the droplet was sucked away using a high-speed pneumatic shutter system, which was also located at the end of the nozzle. As of now, commercially available single-cell dispenser systems are made by, for example, NanoCellect Biomedical, Cellenion or Namocell.
We consider the presented system to be appealing due to its simple fabrication, high adaptability, and parallelization. It can be easily adjusted to meet required specifications for certain tasks regarding droplet positioning (by, e.g., changing distances between the holes in the array or even organizing them in completely different patterns) and dosing (by adjusting the hole diameters; also, different sized holes in one plate are possible). The hole plates can be swapped out readily, in order to adjust to varying specifications, making the setup highly flexible. The system can also be scaled up easily to support many droplets at once.
2. Principle of Operation
The terminology introduced in Tröls et al.’s research [
4] will be adopted here, which means that a distinction between “dispensation” (i.e., rough amount of liquid volume) and “dosage” (i.e., precise amount of liquid volume) will be made.
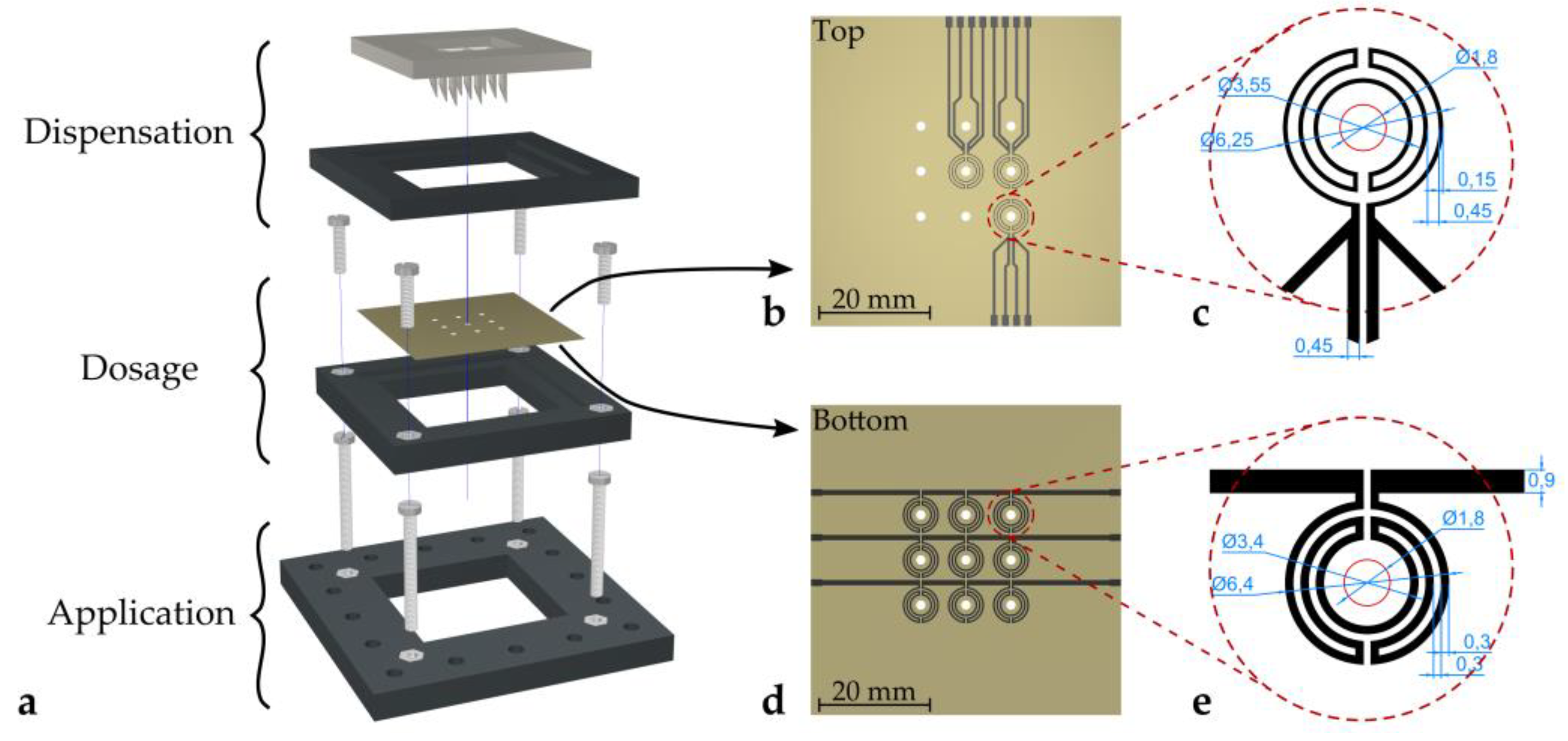
Figure 1a shows the full setup of the proposed deposition concept. The system can be divided into three separate stages, (i) the dispensation stage, (ii) the dosage stage, and (iii) the application stage. The dispensation stage features a 3D-printed hollow needle array, whereas the dosage stage is comprised of a metal plate featuring an array of holes.
The principle of operation is illustrated in
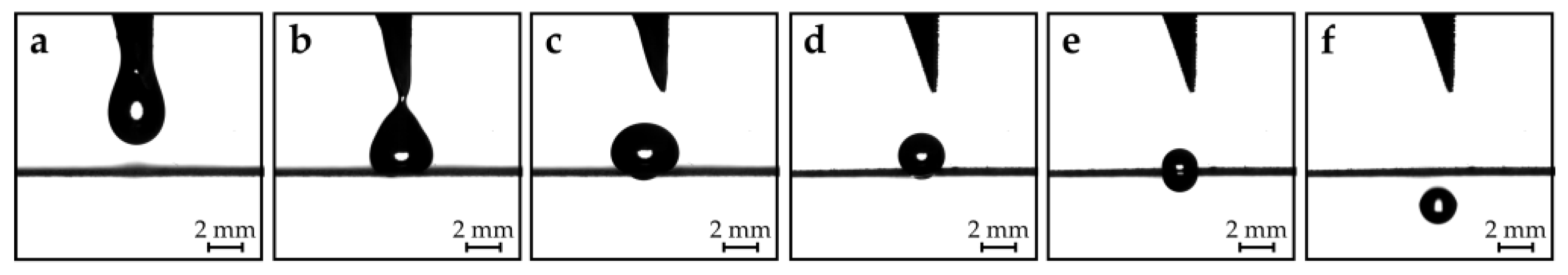
Figure 2. The topside of the hollow needle array structure features a reservoir with individual small wells for each needle. If liquid is placed in the reservoir (e.g., by pipetting or simply pouring), liquid is sucked into the hollow needle channels by capillary forces. If enough liquid is placed in the reservoir, gravitational forces can overcome the capillary forces and a single droplet slides along the needle tip. Eventually, the droplet detaches from the tip and subsequently lands on a hole of the metal plate (dosage pate) beneath (
Figure 2a,b). The remaining liquid remains inside the capillaries, as long as no additional liquid is placed in the reservoir. During this step, only an approximate amount of the required dosage needs to be dispensed from the needles. The channels of the hollow needles in this study have a diameter of 1.8 mm. The dispensed droplets have an initial mass of about 15 mg.
The precise dosing takes place in the dosage stage as follows. Sessile droplets resting on a plane behave differently during evaporation, depending on the properties of the surface. In general, evaporation takes place in three stages. First, (i) in a constant contact radius (CCR) mode (contact radius remains constant and contact angle decreases), followed by (ii) a constant contact angle (CCA) mode (contact angle remains constant and contact radius decreases), and lastly, (iii) in a mixed mode (both contact radius and contact angle decrease) [
23,
24,
25,
26]. For our purposes, the CCA mode is the preferred one, because the droplets need to reduce in diameter to eventually be able to pass through the hole. However, at the beginning of the evaporation process, the droplets evaporate in a CCR mode due to contact line pinning resulting from contact angle hysteresis [
27,
28,
29]. In order to minimize the unwanted evaporation in CCR mode, a superhydrophobic surface with low contact angle hysteresis is required [
30]. Usually, this is achieved by structuring the surface, so the droplets rest in a suspended Cassie–Baxter state [
31]; the contact angle hysteresis of such surfaces is insignificant [
32]. Droplets resting on a superhydrophobic hole were observed to evaporate in a mixed mode [
33], but the superhydrophobicity is still required to avoid contact line pinning. The droplets dispensed from the needle array rest on the superhydrophobic hole plate and shrink in size due to evaporation (
Figure 2c,d). If the sizes of the droplets eventually match the size of the holes, the droplets are pulled through the hole by gravitational forces (
Figure 2e,f). The precisely dosed droplets can then be used within the application stage. High-speed camera recordings of the dispensing and dosing process can be found in the supplementary materials provided in Tröls et al.’s research [
4].
For a given liquid, the mass of the dosed droplets is defined only by the geometry of the holes, resulting in the desired exact dosage of the liquid. Different liquids may not necessarily be dosed with the same mass, as fluid properties can affect the dosing process. Liquids with higher density have a higher mass at the same size as lower density liquids. In such a case, gravitational forces are higher; therefore, it can be expected that the liquid droplet is pulled earlier through the hole than it would be for lower density liquids. As discussed in Tröls et al.’s research [
4], the droplet’s shape is not perfectly spherical when it passes through the hole. For bigger hole sizes, the deviation from a sphere was higher, which was caused by the increased gravitational forces due to bigger droplets. Similar reasoning can be applied to higher density liquids. As the system relies on evaporation in the CCA mode, contact line pinning (caused by contact angle hysteresis) needs to be avoided as this would inhibit the principle of operation. Being able to evaporate in the CCA mode usually requires high initial contact angles. Therefore, also the surface tension of the liquid can influence the performance of the setup. The evaporation is mainly diffusion driven. Therefore, the diffusion coefficient of the vapor in the gas phase greatly effects the total evaporation time [
34].
In order to speed up this evaporation process, microheaters were applied by screen printing on the bottom side of the hole plate (schematics are depicted in
Figure 1d,e). As heat is transferred from the plate to the droplets, the liquid is warmed up, leading to an increased evaporation flux [
35]. Additionally, temperature sensors were applied to the top surface of the hole plate (schematics are depicted in
Figure 1b,c) to monitor the temperature of the dosage plate. The microheaters were designed to be radially symmetric to provide uniform heating of a droplet. The linewidth was chosen to ensure reasonable resistance to be able to apply power using a voltage source. The RTD design was chosen accordingly to provide the same symmetry as the microheaters. Linewidths were designed to be narrower in order to achieve a higher resistance, which simplifies the measurement. Compared to, e.g., using a Pt100 temperature probe, which gives only pointwise information, the distributed design of the presented temperature sensor yields a mean temperature around the hole. This value allows for better comparison, as this is also the value a droplet experiences.
With the presented evaporation-based system, exact dosing can be ensured for homogenous liquids. If solutions evaporate, the solute concentration increases as the solvent evaporates. In some applications, the increased concentration may be unacceptable. Nevertheless, it was demonstrated in Hernandez-Perez et al.’s research [
14], that for dispensed droplet systems, a preconcentration step caused by solvent evaporation, can be beneficial, especially for samples with low abundance.
3. Fabrication
The frames of the three stages of the setup shown in black in
Figure 1a were fabricated using a standard desktop 3D-printer (Ultimaker 3 extended, Ultimaker, Utrecht, The Netherlands). The hollow needle array was also 3D-printed (Stratasys Objet 30 Pro, Stratasys Ltd., Eden Prairie, MN, USA).
Figure 3 shows the fabrication process of the superhydrophobic hole plate. Starting with a 100 µm thick nickel silver (or German nickel) plate (size 55 mm × 55 mm), which was coated with photoresist on both sides, the hole layout was transferred by a standard photolithography process (
Figure 3a). After development of the photoresist (
Figure 3b), the transferred pattern was etched away, leaving the holes in the metal plate (
Figure 3c). In this example, a 3 × 3 array of holes with a diameter of 1.8 mm was etched. Next, the metal plate was insulated to avoid shorting of the microheaters and RTDs (
Figure 3d). To this end, an insulation coating, made from 33 wt% Rhodeftal 210 ES (Huntsman International LLC, The Woodlands, TX, USA), 40 wt% N-methyl-2-pyrrolidone (NMP), and 27 wt% p-xylene, was prepared. After mixing of the components, 0.1 wt% of the surface additive BYK 310 (BYK Additives and Instruments, Wesel, Germany) was also incorporated to reduce the surface tension of the coating [
36,
37]. Using a spray coating process, the insulating coating was applied to the top and bottom surfaces. Between layer applications, the plate was preheated on a hotplate to approximately 230 °C and finally cured at 250 °C for approximately 10–15 min. Next, the microheaters and RTDs were applied by the screen printing process (
Figure 3e). First, the RTDs were printed using a 180 threads/cm PET (polyethylene terephthalate) mesh and then the microheaters, using a 100 treads/cm PET mesh. A conductive polyimide silver ink (KA 801, DuPont, Wilmington, DE, USA) was used in both cases. After each printing step, the ink was cured for about 15 min on a hotplate at about 150 °C. Lastly, the top and bottom sides of the dosage plate were coated using the commercially available impregnation spray NeverWet (Rust-Oleum, Vernon Hills, IL, USA) following the guidelines provided by the manufacturer (
Figure 3f). Using this spray, the contact angle between a plane and water was measured to be about
θ0 = 154° after coating [
38].
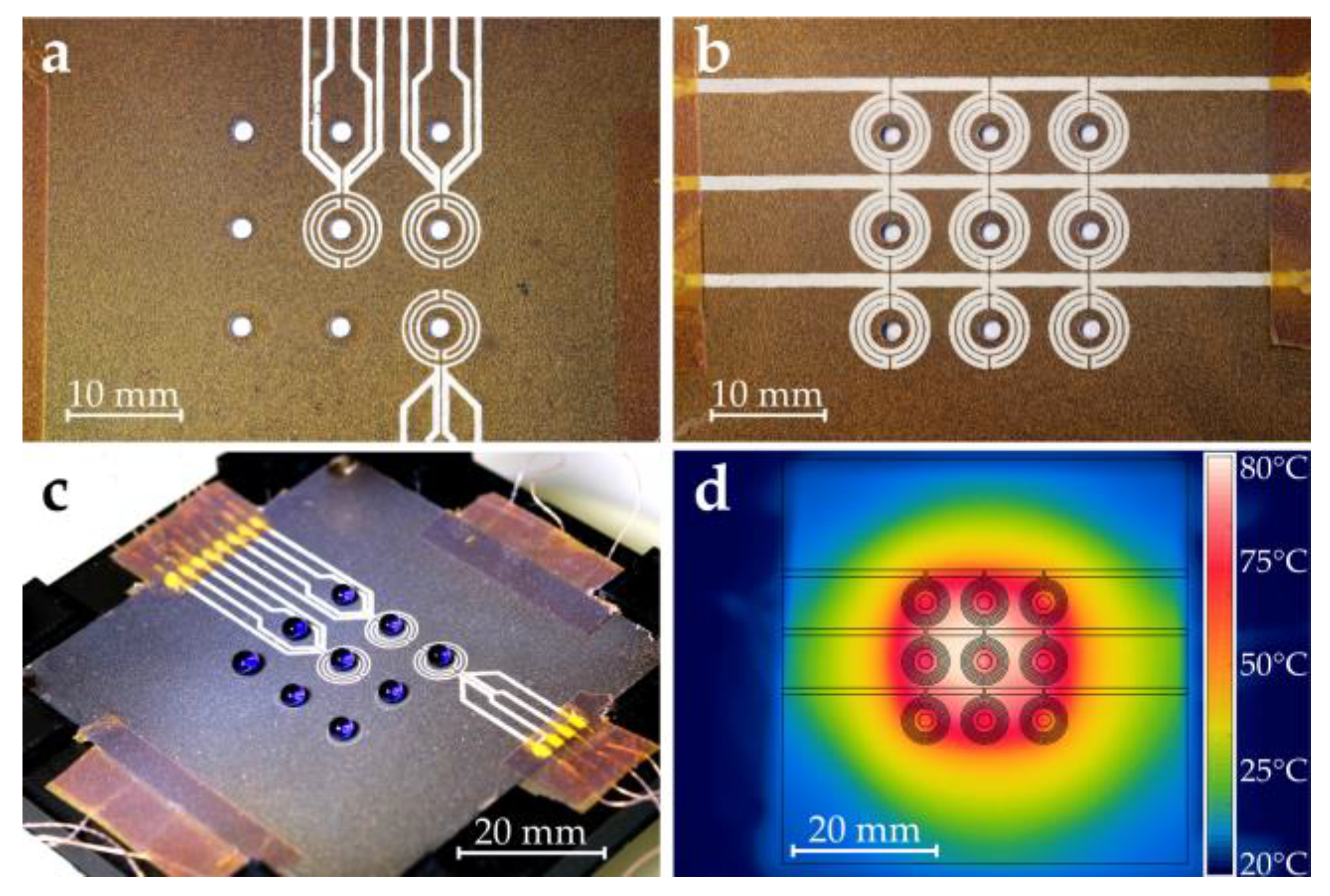
Figure 4a,b show close-up photographs of the fabricated RTDs and microheaters on the dosage plate after applying the superhydrophobic coating, respectively.
Figure 4c shows a picture of the fully loaded dosage plate. Note that deionized (DI) water mixed with blue ink was used for this picture to enhance contrast. The measurements below were performed using only DI water.
Each of the microheater meanders had an electrical resistance of about 4.5 Ω. The three traces of the microheater design were connected in parallel via the connection cables.
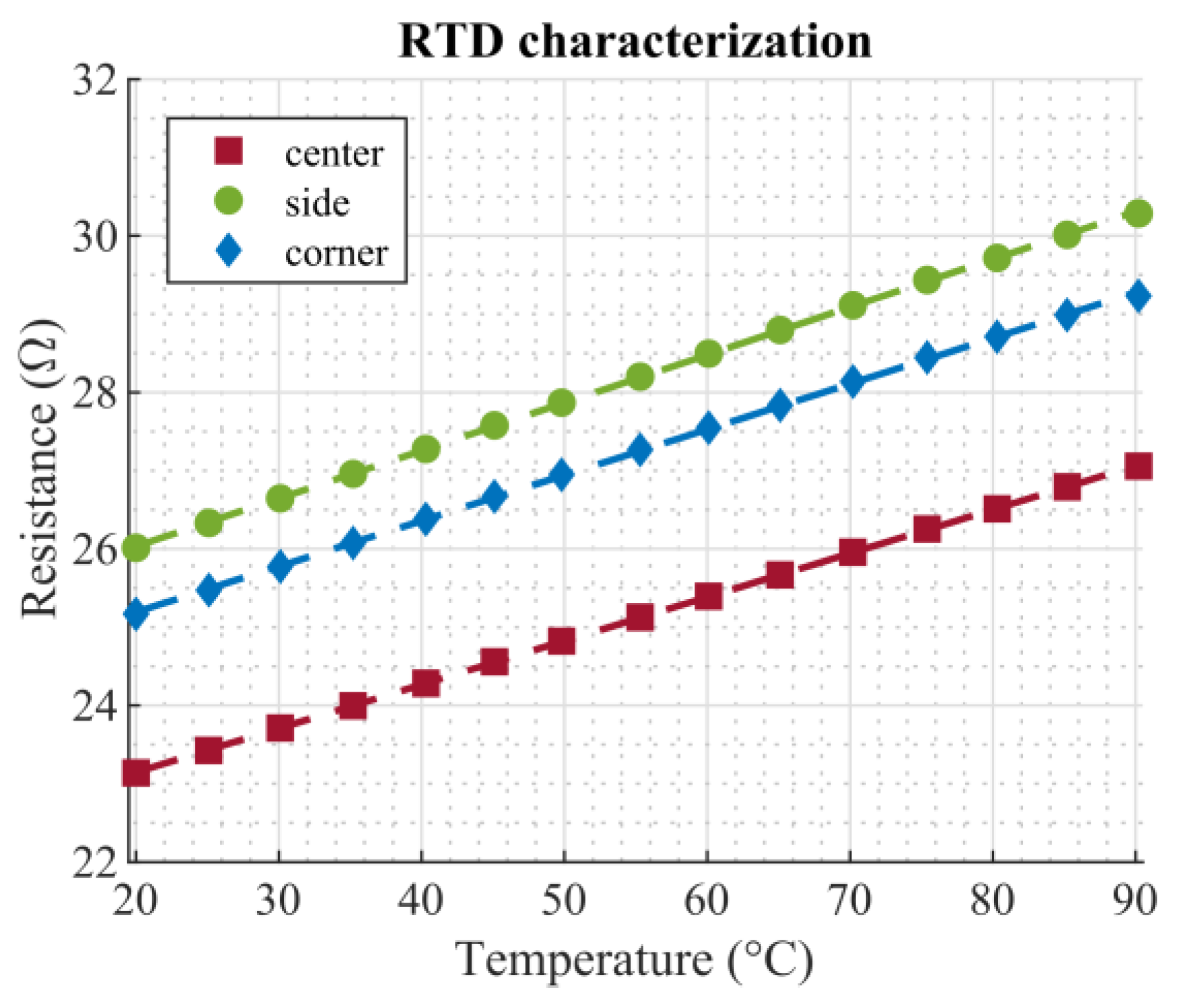
Figure 4d shows a thermal image (FLIR E4, FLIR Systems Inc., Wilsonville, OR, USA) of the topside of the dosage plate after applying DC voltage (4 V) to the heaters. Since the heat distribution is not uniform, droplets in the center of the plate tend to evaporate faster than corner droplets due to the higher temperature in the center. The layout of the RTDs was designed in a way to capture all tree temperature zones, ranging from the hottest area (center) to slightly cooler parts (in the middle of the sides) to the sections with the lowest temperature (corners). Considering the symmetry of the temperature distribution, these three points were sufficient to characterize the temperature conditions of the dosage plate.
5. Discussion and Conclusions
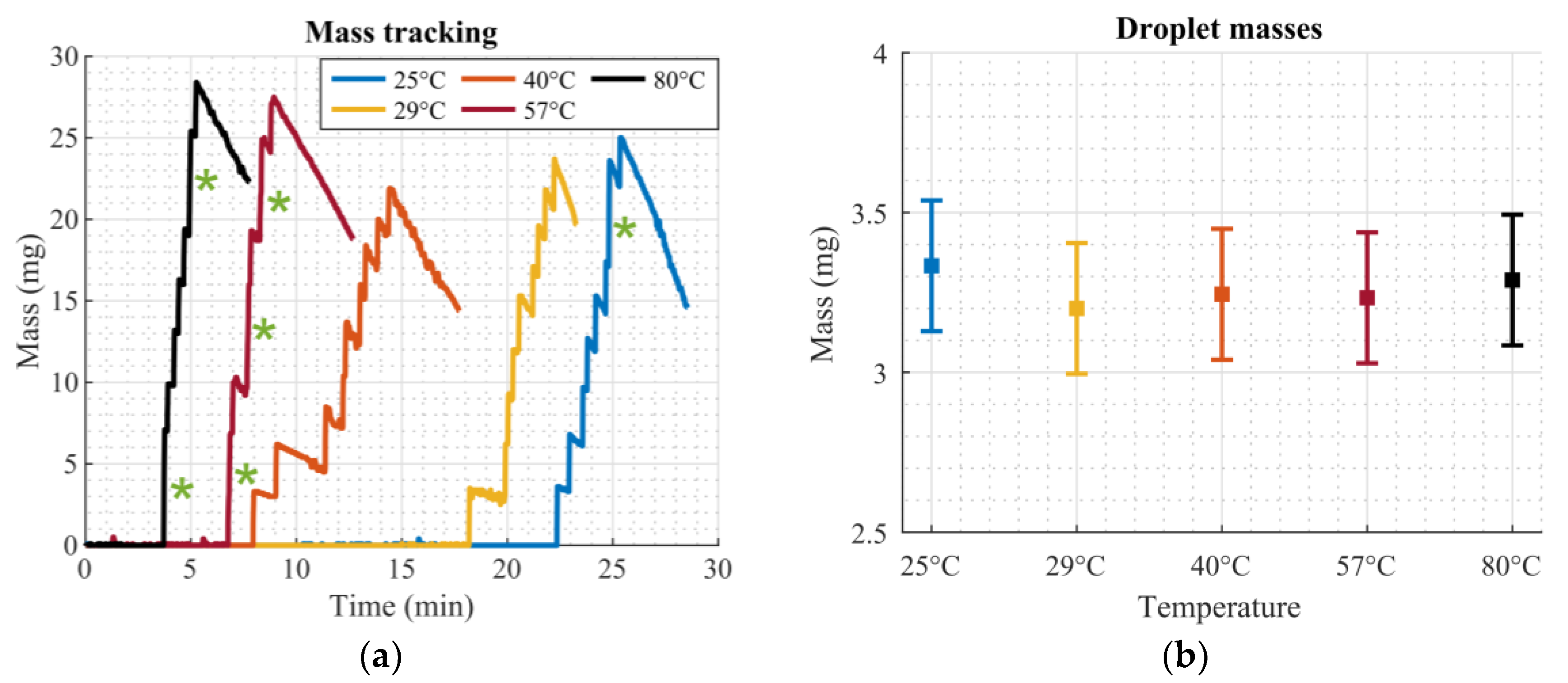
This work presented a simple setup which is suitable for precise deposition and dosing of homogenous liquids. The dosing was performed by means of droplet evaporation on a superhydrophobic hole plate. Additionally, screen-printed microheaters on the bottom side of this plate were utilized to accelerate the evaporation process significantly without compromising the functionality of the system. The improvement of the total evaporation time could be described by a power law, which is in agreement with findings in the literature concerning similar problems. The relative improvement in total evaporation time due to the heated plate, compared to evaporation at room temperature, was 12.34% (at 29 °C), 43.16% (at 40 °C), 64.76% (at 57 °C), and 79.27% (at 80 °C). The addition of screen-printed temperature sensors helped to monitor the temperature directly on the superhydrophobic plate.
The precision of the presented system can be compared with commercially available systems by introducing the coefficient of variation
cv (which is defined as the ratio of the standard deviation to the mean value). From
Table 3, this value was calculated being about 6% for the proposed setup. The performance is compared to commercially available single channel and multichannel pipettes (brand name: Eppendorf Research® plus, Eppendorf, Hamburg, Germany) and an automated liquid handling system (brand name: epMotion® Dispensing Tools, Eppendorf, Hamburg, Germany). Values were taken from operating manuals of the manual pipettes [
39] and the automated system [
40], respectively. For single channel pipettes with variable volume between 0.5 µL–10 µL at a test volume of 5 µL, the
cv value was found to be 0.8%, and for pipettes with variable volume between 2 µL–20 µL at a test volume of 2 µL, the value is 1.5%. Multichannel pipettes with variable volume between 0.5 µL–10 µL with 8 or 12 channels have a
cv value of 2% at a test volume of 5 µL, and ones with 16 or 24 channels and volumes between 1 µL–20 µL at a test volume of 2 µL have a
cv value of 5%. Lastly, the single tip tool (1 µL–50 µL) and the multi tip tool (0.2 µL–10 µL, 8 channels) of the automated system have
cv values of 3% and 2%, respectively, both at test volumes of 5 µL. The performance of the presented setup is comparable with already commercially available systems.
The dosed mass can be adjusted by changing the hole diameter for a given liquid. In Tröls et al.’s research [
4], the dosed mass was measured for varying hole diameters. No obvious change in precision due to the differently sized holes was observed. For small hole diameters, and therefore corresponding small droplets, the dosed mass tends to approach the expected mass of a perfectly spherical droplet. Gravitational forces are so low that the droplet is required to evaporate until the hole diameter is met, at which time it can pass through the hole. For larger hole diameters, gravitational forces help pull the droplets through the hole by slightly squeezing them. Such droplets take on an oval shape during transition through the hole. Their mass is higher than that of a spherical droplet of the same diameter as the hole. The accuracy of the present system is mainly affected by the fabrication process. Misalignments of the photomasks on the top and bottom of the metal plate during UV exposure can result in holes that deviate from a perfectly shaped circle after the etching process. Also, uneven etching can have an effect on the hole geometry. Lastly, uneven application of the insulation and especially the superhydrophobic coating can affect accuracy and precision negatively. Most of these shortcomings could be potentially improved by an automated fabrication process, as in this work each step was performed manually.
The temperature measurements confirmed that the temperature distribution is not uniform across the hole plate. A hotspot was prominent in the center of the plate. This might become an issue when liquids are to be dosed, that should not be heated above a certain temperature. Although the supplied power could be lowered, in order to reduce the temperature of the hotspot to an allowed level, this would impair the overall performance of the device in terms of evaporation time, as the rest of the dosage plate would also be cooler. Adjusting the design, by, for example, reducing the resistance of the center microheater (e.g., by broadening the printed lines), would probably result in a more uniform temperature distribution. Then, the entire plate could be operated at the maximum allowed temperature, resulting in the shortest possible evaporation time.
The combination of microheaters and temperature sensors, which can be processed directly onto the plate, can be used to further study the evaporation behavior of droplets resting on a superhydrophobic hole on a heated substrate. Results obtained using this approach could be compared to models considering the evaporation of sessile droplets.
The proposed system is captivating due to its simple implementation. It can be easily scaled up and is therefore capable of highly parallelized droplet deposition and dosing processes. Additionally, the setup is flexible, as the position and size of the holes of the superhydrophobic dosage plate can be adjusted straightforwardly in order to meet required specifications. By simplify swapping out the dosage plate, the setup can be adjusted for a different task very quickly. The proposed system can therefore be of particular interest for various automated or parallelized tasks in biochemical laboratories, e.g., the coating of microneedle arrays, liquid handling on microtiter plates or for dispensed droplet systems.