1. Introduction
In recent years, microfluidic devices have become an important tool for use in lab-on-a-chip (LoC) processes, including drug screening and delivery, biochemical reactions, sample preparation and analysis, separation, chemotaxis, and cell manipulation [
1,
2,
3,
4,
5,
6]. Compared with traditional methods, these devices have the potential to simplify laboratory processes while reducing the time and cost required. Generally, fluids containing a sample are driven through a device consisting of microfabricated channels that complete the required experimental steps. To transport the fluid, either a pressure gradient or electroosmotic force is commonly used [
7,
8,
9,
10].
In order to maintain precise solute transport inside the microchannel, which is paramount for molecular- or cellular-level application, a flat cross-sectional concentration profile is desired [
11]. For example, the flat concentration profile has been demonstrated to improve biochemical assay technology by allowing a uniform fluid velocity profile to disperse an equal amount of sample to be analyzed in parallel [
12]. The most common technique to achieve a flat concentration profile is by applying electrical potential to drive the liquid motion, which is popularly described as electroosmotic flow (EOF). The implementation of EOF inherently produces a nearly uniform velocity profile across a channel [
13,
14,
15]. However, EOF heavily relies on the electrical properties of the medium and surface, for instance, imperfections or chemical coatings on the channel surface can distort the concentration profile [
16,
17]. Moreover, the application of an electric field can potentially be incompatible with nonhomogeneous solutes/buffers used in the device and may have negative effects on biological samples [
18,
19]. It can also be difficult to use electroosmotic flows for particular processes, such as mixing particles, due to the irrotational nature of the flow [
20,
21]. Recently, flat concentration profiles could be generated through magnetohydrodynamic (MHD) pumping [
22]. To allow for effective MHD, the introduction of redox species to the fluid element is necessary. Thus, controlling the concentration profile in a microfluidics device without dependency on the liquid composition remains a challenge.
Pressure-driven flows introduced with a mechanical syringe pump provide a simple and inexpensive alternative when EOF is not compatible with a device or the sample [
13,
23]. However, it is well known that a pressure gradient through a channel will introduce a parabolic flow profile in the axial direction. This is due to the no-slip condition found at the channel wall, which causes a non-uniform velocity gradient along the cross section of the channel [
24]. Considering a two-dimensional (2D) cross-section of a rectangular channel, this effect is proportional to channel dimensions and much more significant in the axial dimension of a typical device. Accordingly, the introduction of such a parabolic profile prevents precise solute transport within the device.
Related literature on the topic of Taylor dispersion in microfluidic channels has suggested that passive geometric features can be utilized to control the solute profile. Dutta et al. showed in theory and simulation that the length of a solute slug undergoing Taylor dispersion can be significantly reduced in rectangular and isotropically wet-etched microfluidic channels by increasing the cross-sectional area near channel side walls [
25]. This reduces the effective drag of the walls on the fluid as a result of no-slip conditions. Dutta et al. expounded on this work to include elliptical and trapezoidal channel geometries [
26]. Despite significant theoretical contributions, these works lack experimental proceedings to test the developed theories and simulations. Similarly, Aminian et al. reported with theoretical and experimental evidence that cross-sectional aspect ratio alone could control the shape of the concentration profile of a solute plug in the axial direction [
27], however, they primarily concerned themselves with long-term flow behavior, which is not directly applicable to many LoC processes requiring well-behaved solute distribution within a short timescale of injection. Recently, Gökçe et al. reported self-coalescence-based control of reagent reconstitution by implementing a special geometric feature—capillary pinning line—into the shallow microfluidic channel [
28]. Although reagent delivery was effectively demonstrated, the design is limited by the fact that dried reagents need to be pre-positioned at specific locations of the device, and the delivery area transverse dimension (1.5 mm) is quite small.
Here, we introduce an elegantly simple method for achieving a flat solute concentration profile in a microfluidic channel, solely relying on the modified geometry of merely the entry segment of the channel. We start with a typical microchannel found in LoC applications (hereto referred to as the “Control” channel design) and draw inspiration from finite element fluid dynamics simulations to design and fabricate passive geometric features within the diverging entry segment to achieve a flat solute concentration profile (hereto referred to as the “Curve” channel design). We fabricate the device by utilizing conventional machining technology due to its complex shape. For analyzing the device performance, we concern ourselves with achieving a flat solute profile across the channel width (y). Due to the 2D nature of the microchannel, a flat solute profile across the channel depth (z) is not considered. Performance of the features, as characterized by the transformation of an inherent parabolic solute profile into a flat solute profile, is measured experimentally and compared with simulation results.
2. Theoretical Methods
2.1. Theory
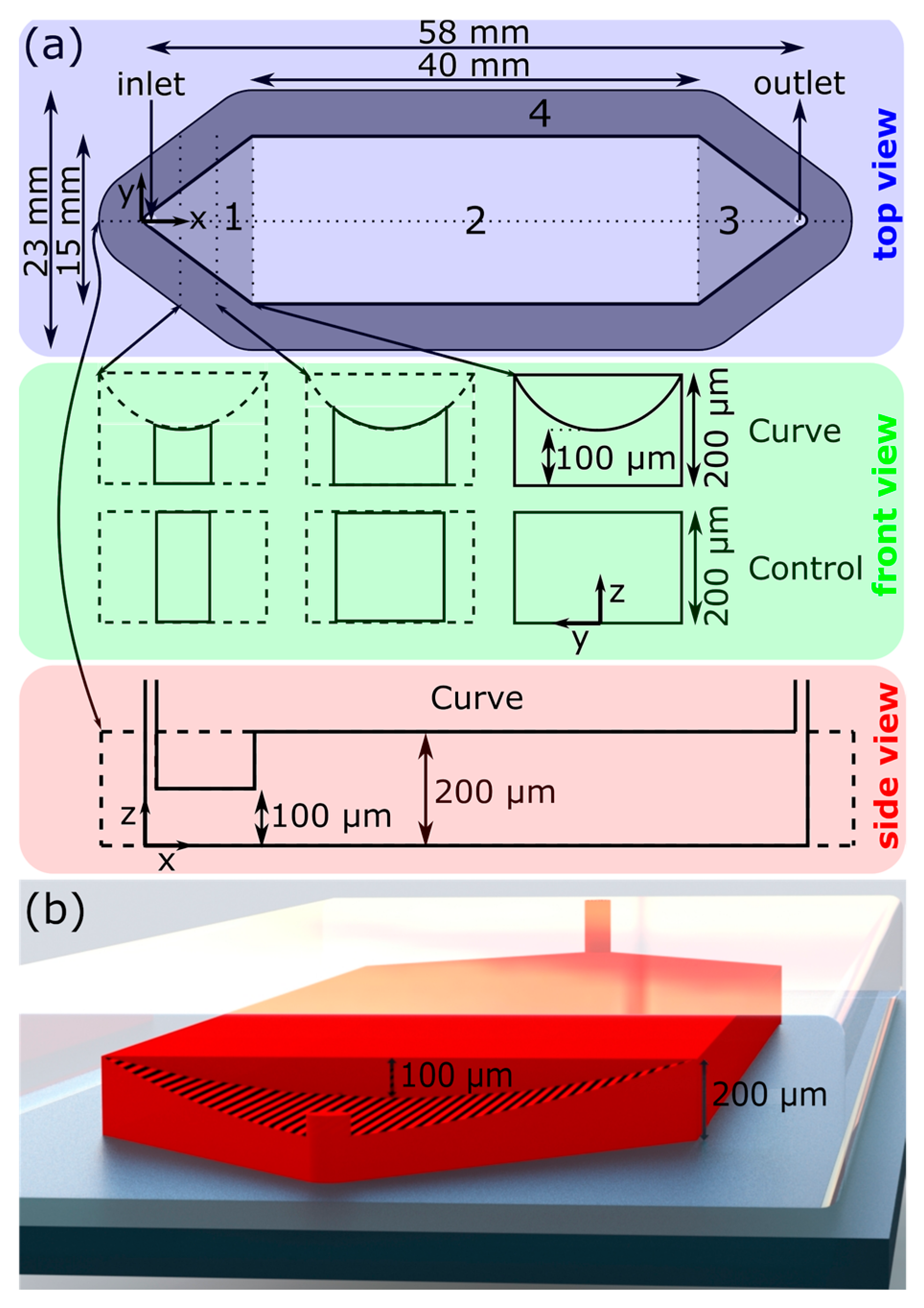
In a microfluidic channel as shown in
Figure 1a, the straight segment in the middle of the channel is connected to either inlet or outlet through a diverging triangle segment. The aspect ratio, that is, depth to width, of the straight segment is 0.0133, and the depth is constant over this segment. Hence, the horizontal velocity profile does not change significantly, except near the side walls of the channel. In the Control design, the depth of the channel is constant everywhere in both diverging and straight segments, the depth-averaged constant pressure lines can be approximated by concentric arcs. The fluid velocity field is dominated by the radial velocity, where the center is located at the intersection of the two vertical walls of the diverging segment. When a solute slug is introduced at the inlet, the horizontal profile—viewed from the top—is predominantly circular. This circular profile enters the straight segment of the channel.
To start with a nearly straight-line horizontal profile of the second liquid at the joining region of the diverging and straight segments of the channel, the proposed design has a modified cross-sectional area in the diverging segment. Here, the roof of the channel is such that the depth varies as a parabola where the apex—shallowest point of the channel—coincides with the line between the inlet and the outlet. To gain some insight about how this design contributes toward correcting the circular horizontal profile of the second liquid, let any point on the top surface of the channel be described by z = y2, where the origin is located at the intersection of the two side walls on the bottom surface of the channel, z is the vertical position, y is the position along the breadth, and x is the axial position. In addition, we consider a cylindrical coordinate system (r,θ,z) with the same origin, where θ is the angular position with respect to the straight line between the centers of the inlet and outlet. For any given θ, we find ∂z/∂r = 2r∙sin2θ, and for any given radial position, r: ∂z/∂θ = r2∙sin2θ. Therefore, the depth increases linearly with the distance from the origin at any given angular position. This effect is stronger with higher values of θ, toward the side walls, due to the multiplying factor sin2θ. The second equation implies that for a given radial position, depth increases nonlinearly as sin2θ. Again, this effect is intensified by the factor r2, signifying that further from the inlet, the increase in depth is significant as we move near the side walls. Thus, we can qualitatively assess the contribution of the modified diverging segment to the correction of velocity field. The depth-averaged pressure at any given radial position will vary significantly with angular position. Specifically, the pressure along the central axial line will be minimal, and will increase significantly near the side walls. Consequently, there will be a significant tangential component of velocity (uθ) in addition to the radial component (ur). The horizontal front/profile of the second liquid will no longer appear circular due to increased flow near the side walls. Overall, the front of the second liquid will be straightened as it enters the main uniform segment of the channel.
2.2. Simulation
Using the theoretical intuition discussed above, several geometric modifications to the upstream region of the Control channel were considered to yield the flat concentration profile. Various Curve channel designs were drafted with the intent of increasing cross-sectional area near the side walls of the upstream region of the channel relative to the cross-sectional area in the center of the channel (y = 0). In turn, fluid velocity near the edges will increase such that the solute profile near the edges will “catch up” to the profile in the center. Taylor’s hypothesis suggests that extension of the geometric features to the entirety of the channel is not necessary on the relevant time and length scales. Moreover, such features further complicate manufacturing and may cause unpredictable biases in applications which require cell culture or other operations in which oxygen permeability of the polydimethylsiloxane (PDMS)-based microchannel is a factor.
The Curve channel designs under consideration were modelled in SolidWorks and simulated in COMSOL Multiphysics (COMSOL Inc., Stockholm, Sweden). Simulations were performed with volumetric flow rates of 115, 230, 460, and 920 µL/min, a relevant range for LoC applications. In all models, the inlets to the channel were modelled as a planar face perpendicular to the flow (
) and coincident with the central axis of the inlet. COMSOL simulations made use of the Laminar Flow and Transport of a Diluted Species physics modules. The velocity field from the laminar flow module was used for the transport properties of the diluted species module. Studies were performed in a two-step process: Step 1 was a Stationary step, which was used to simulate the developed laminar flow at steady state, and Step 2 was a Time-Dependent step, which was used to simulate the species transport in the steady-state flow with respect to time. COMSOL simulation parameters are listed in
Table S1 in the supplementary materials.
2.3. Channel Design
Ultimately, we chose a design resembling a typical microchannel with an extruded parabolic mass in the upper region. A schematic of the channel design is presented in
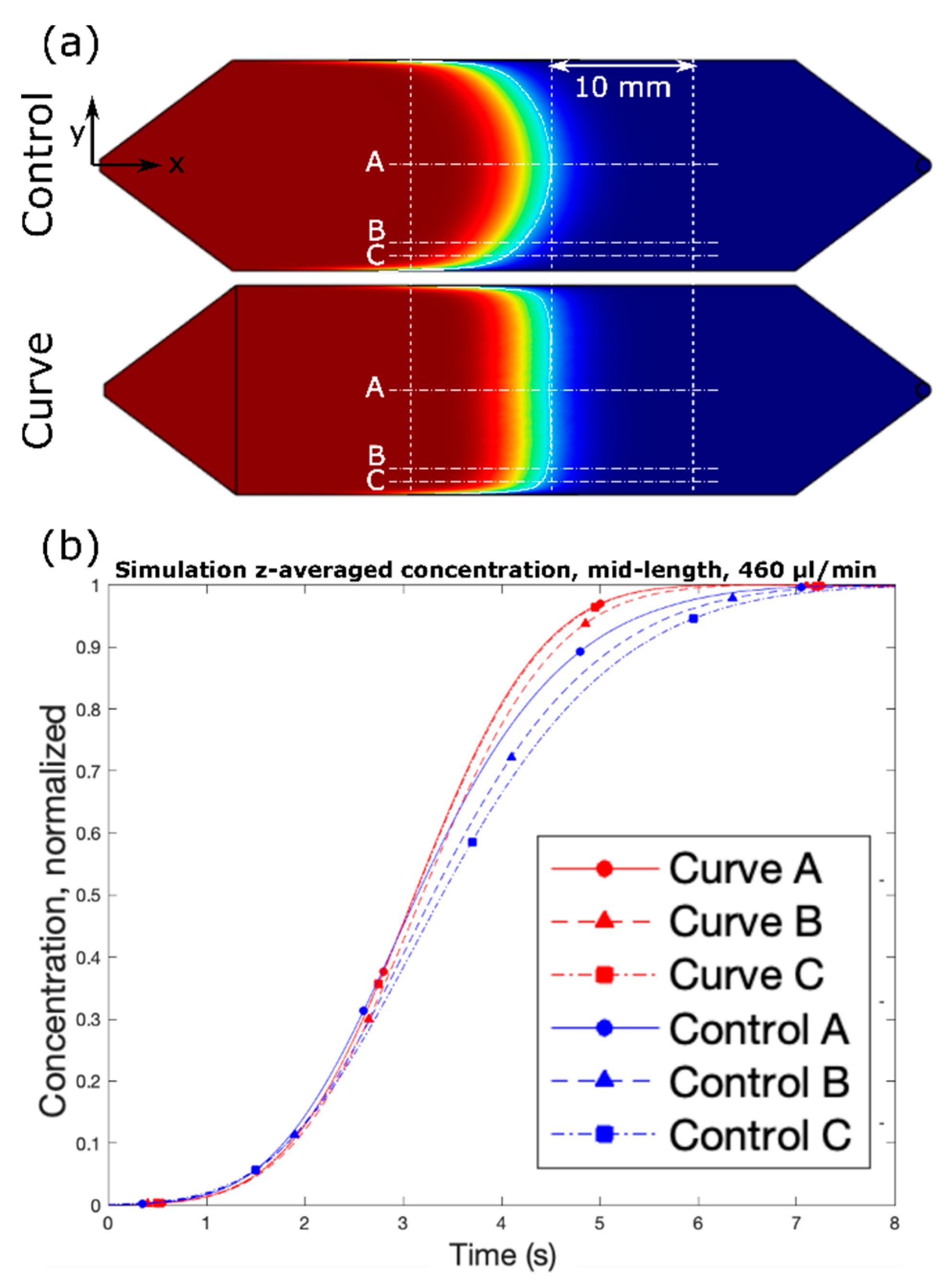
Figure 1. The design was selected due to its superior performance in simulation and constitutes the “Curve” channel for the remainder of the study. The design effectively increases the cross-sectional area near the side walls, as discussed above, by squeezing the fluid near the center of the channel. Under the range of flow rates and timescales, the simulated solute profile is extremely flat with respect to the y–z plane. Furthermore, despite the complex shape of the channel in the context of microfabrication, it was one of the simpler designs under consideration in terms of fabrication.
As mentioned, the Curve channel is best described as a typical microchannel (“Control”) with minor geometric modifications only to the upstream region (region 1 in
Figure 1a). Each channel features one inlet and one outlet, both diameter 1 mm, separated by a straight channel. The midstream rectangular region (Region 2 in
Figure 1a) is uniform with a cross-section of 15 mm by 40 mm, and is the primary space in which the LoC operations take place. The upstream and downstream 9.7 mm long sections of the channel (1 and 3 in
Figure 1, respectively) are triangular: the upstream triangular region is purposed to expand the fluid from the inlet to the midstream straight region, and the downstream triangular region contracts the fluid from the midstream region to the outlet. The Control channel was 200 µm tall everywhere. Due to manufacturing difficulties discussed in
Section 4.2, the Curve channel was 225 µm tall throughout the majority of Region 2 (
Figure S1 in supplementary materials). The geometric modifications which constitute the Curve design can be described by a parabolic curve drawn at the upstream–midstream intersectional plane (x = 0), with endpoints at the corners of the ceiling–side wall intersectional edges ((y,z) = (±7.5 mm, 200 µm)) and a depth of 100 µm (z = 100 µm). This curve is then extruded in the −x direction up to and beyond the inlet of the channel.
3. Experimental Methods
3.1. Channel Fabrication
Since the Curve channel design requires a smooth, multidimensional curved surface, traditional microfabrication techniques such as photolithography or deep reactive-ion etching (DRIE) were not an option. Initially, we experimented with fabricating via high-resolution three-dimensional (3D) printing (Stratasys Objet Pro, vertical resolution 16 µm), as this new technology very readily lends itself to this difficult fabrication problem. We followed the protocol outlined in King’s research to enable curing of PDMS in contact with the cured objet material, but this resulted in thermal warping and inconsistent performance results [
29].
The final Curve channel was fabricated from a solid aluminum block via computer numerical control machining. Prior to machining, the aluminum stock was finely lapped and polished. This polished face is planar with the “ceiling” of the final channel mold in the midstream and downstream regions, in an effort to minimize artifacts of the machining process. The upstream region of the mold was machined with only flow-parallel (x-parallel) tool paths so to reduce asymmetric species transport in the region. Sylgard 184 (Dow Corning, Midland, MI, USA) PDMS was prepared by mixing the monomer with the curing agent in a 10:1 mass ratio and poured over the respective channel mold. The PDMS was allowed to cure at 70 °C for 1 h. After curing, the PDMS was carefully peeled from the mold, inlet/outlet holes were punched, and the PDMS was bonded to a standard glass microscope slide via oxygen–plasma bonding. A glass microscope slide was also cut to length and bonded on top to prevent deflection of the PDMS under pressure, however, this is not depicted in the figures presented for visualization purpose.
3.2. Optical Concentration Measurement
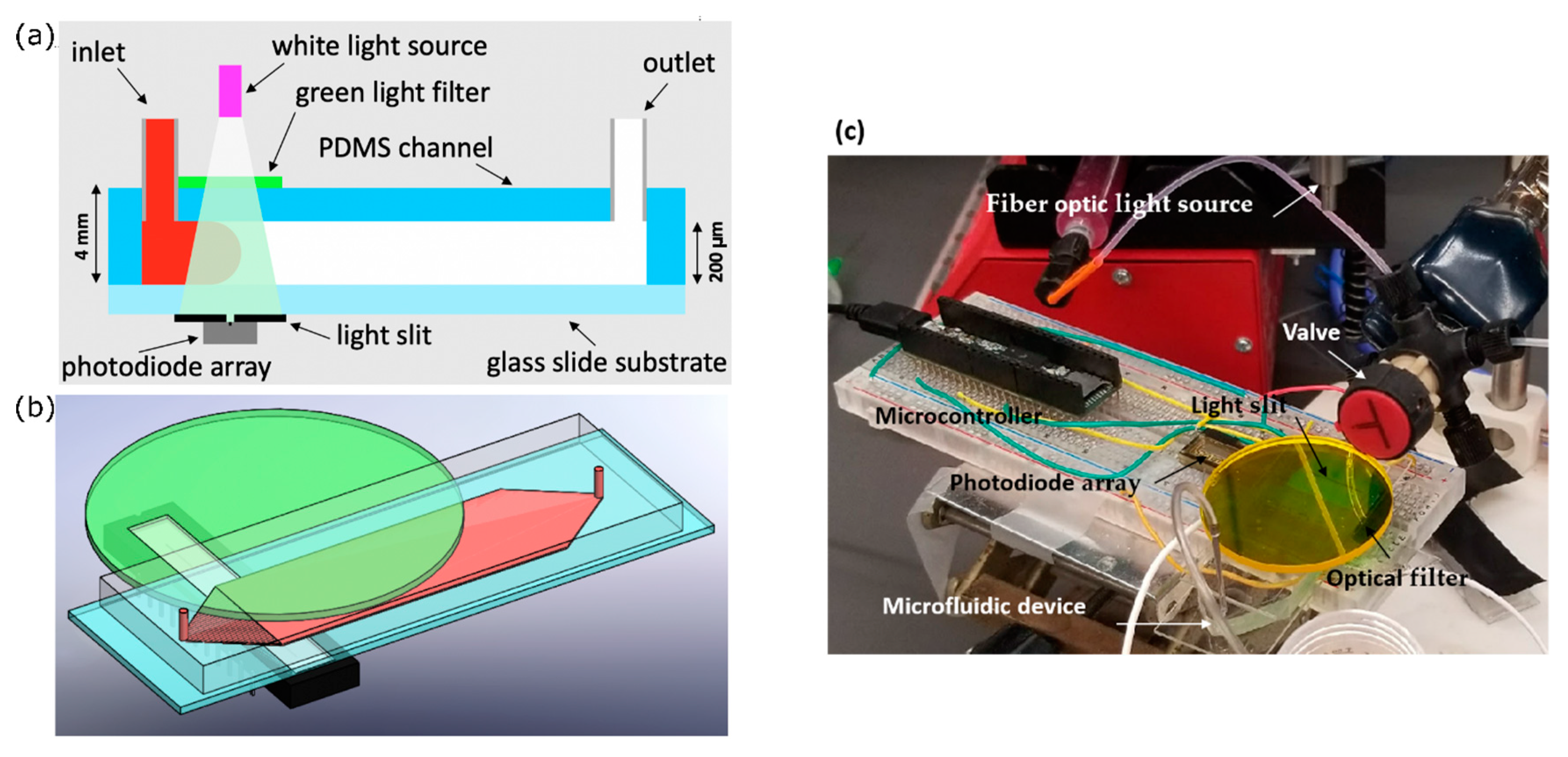
An optical absorption system was used to experimentally assess Curve channel performance. The setup for this optical absorption system is depicted in
Figure 2. Phenol red, a pH-sensitive red dye, was prepared in 40 µM DI (deionized water) water solution and adjusted to pH = 7.2. At this pH, phenol red exhibits peak absorption at 560 nm, which falls in the visible green spectrum.
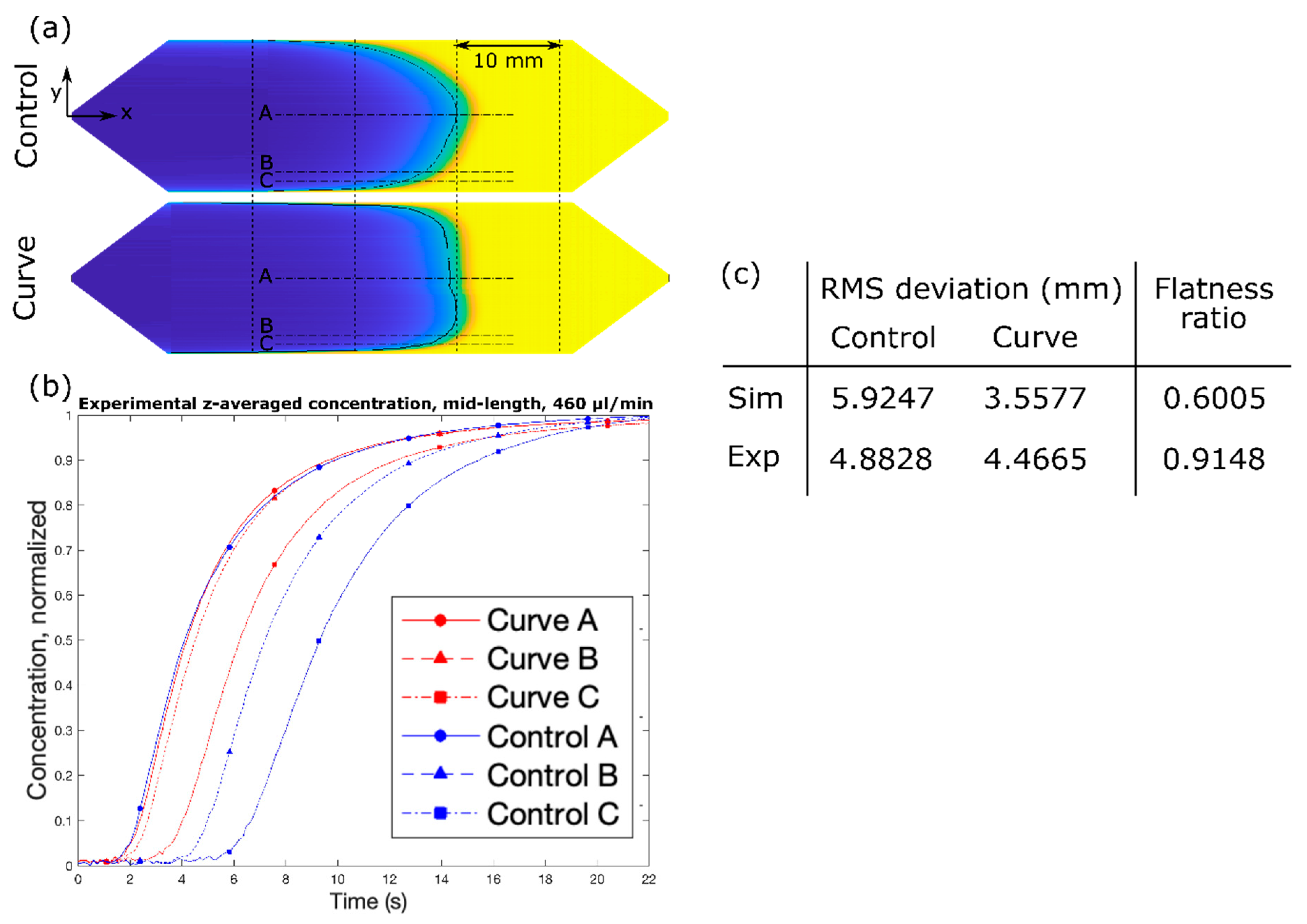
First, white light was passed through a green light filter. The resulting green light passed through the channel, and then through a light slit to block reflected light, and finally into a one-dimensional (1D) linear photodiode array (TAOS TSL1406, pixel resolution 63.5 µm, ams AG, Premstaetten, Austria) placed parallel to the y-direction, so as to capture the transmitted light intensity (z-averaged concentration) across the entire width of the channel. Next, the microchannel was flushed with DI water, and then a slug of phenol red solution was introduced into the inlet via syringe pump. The outlet was left to drain freely. Incident light intensities were read by the photodiode array every tPD = 50 ms by a Teensy 3.6 with custom Teensy-duino code (an Arduino wrapper for the Teensy, Teensy 3.6). A “pseudo-snapshot” of the concentration profile was then derived by measuring concentration across the width of the channel at some longitudinal x position over time. These measurements are generated into a single image by calculating linear fluid velocity from the channel dimensions and volumetric flow rate. This is justified by an alternative perspective on Taylor’s “frozen turbulence” Hypothesis—an observation of a concentration slug in laminar flow at a single point will agree well with the actual progression of the concentration slug in time. Furthermore, while the concentration profile gap does noticeably widen with respect to flow rate, it does not do so with respect to time, thus providing a sufficient means of capturing the concentration distribution in the whole channel at a given point in time.
In order to assess performance, incident light measurements were recorded from the initial presence of the DI water until the phenol red slug had fully saturated the channel. The first and last few measurements were discarded to prevent anomalies resulting from transient fluid flow. Measurements from pixels outside the boundaries of the channel were also discarded. Of the remaining data, for each pixel, measurements were normalized by the first measurement, which corresponds to the pixel value for DI water.