How Plant Toxins Cause Early Larval Mortality in Herbivorous Insects: An Explanation by Modeling the Net Energy Curve
Abstract
1. Introduction
2. Results
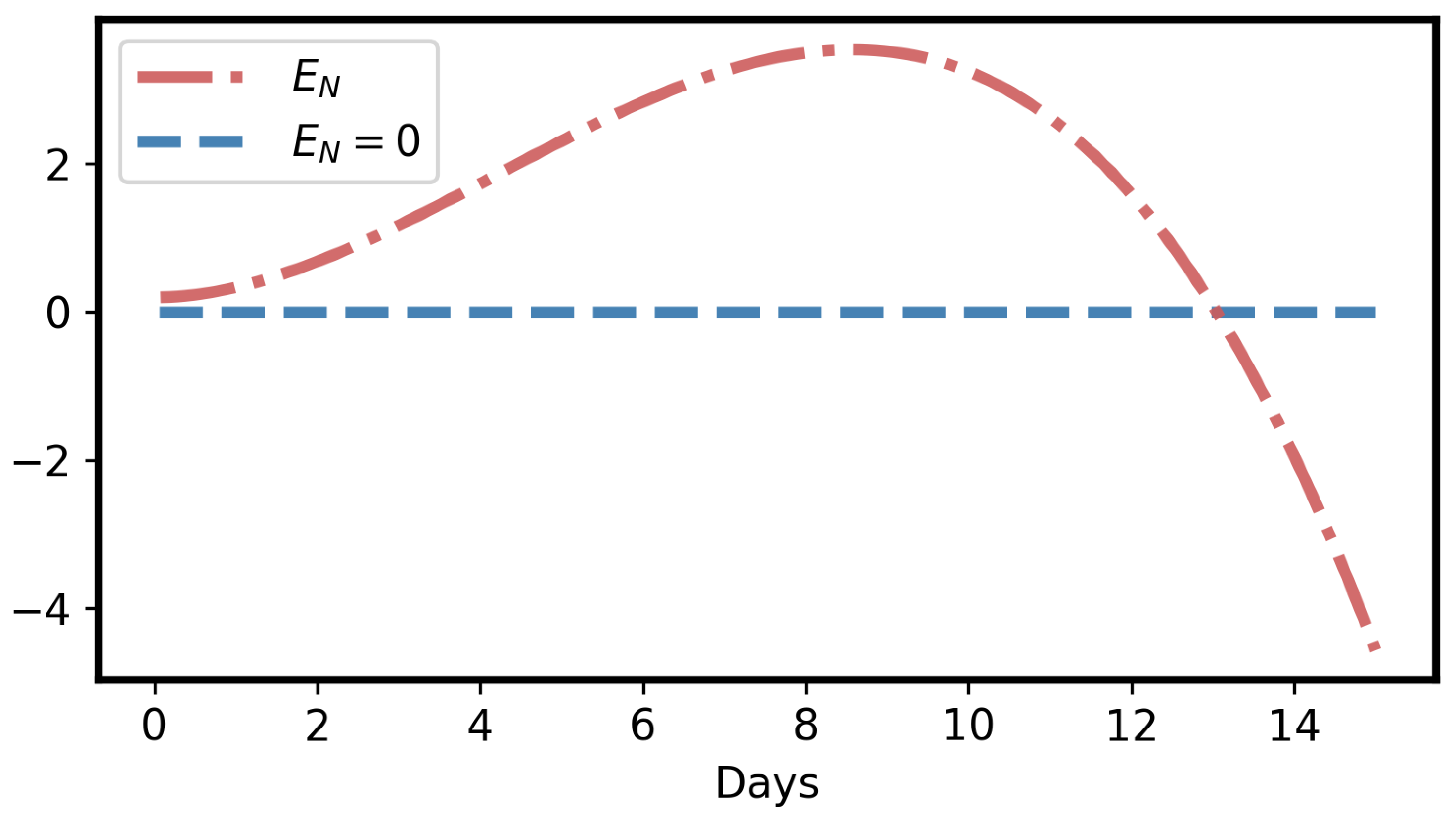
- When net energy becomes zero, a larva stops herbivory because further feeding leads to negative net energy, as shown in Equations (11) and (15) and Figure 3. The energy benefit of herbivory and the metabolic costs of toxin exposure and foraging determine the particular time, T, as shown in Figure 4.
- If the net energy reaches zero at an early time point (Figure 4), i.e., T is small in comparison to larval development time, the larva dies prematurely. A larva does not necessarily die as soon as it stops herbivory; there could be a time lag, but eventually it dies without nutrition. If net energy becomes zero close to the end of the larval instar stages (Figure 4), i.e., T is approximately equal to larval development time, then the larva survives the plant toxin.
3. Discussion
4. Materials and Methods
- Energy benefit: A larva obtains energy benefit from herbivory. Therefore, this function is proportional to herbivory: Equations (1) and (2). Let the increase in energy growth rate per unit time due to the growth of larvae be a constant . Then, the energy growth rate is a linear function (like Equation (1)) and larval energy is a monotonic increasing quadratic function of time (like Equation (2)) if metabolic costs (of toxin and foraging) are absent.
- Toxin cost: The metabolic costs of toxins in a larva should be proportional to the ITC concentration I. Assuming is the proportionality constant, a simple function for the metabolic cost of toxin is .
- Foraging cost: This is the metabolic cost of herbivory, i.e., the energy expended for herbivory. Therefore, the foraging cost is proportional to the herbivory function in Equation (2), denoted as , where is the proportionality constant.
Analytical Approximate Calculation
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
List of Symbols and Acronyms
| Symbols | Definitions | Types |
| t | Time or instar in continuous form | Independent variable |
| H | Herbivory by a larva at time t | Dependent variable |
| G | Ingested GLSs by a larva at time t | Dependent variable |
| F | Free GLSs in larval gut at time t | Dependent variable |
| I | ITCs in a larval gut at time t | Dependent variable |
| Net energy of a larva at time t | Dependent variable | |
| Larval herbivory growth rate constant per unit time | Parameter | |
| GLS ingestion growth rate constant per unit time | Parameter | |
| Degradation rate constant of GLS ingestion in larval gut | Parameter | |
| Hydrolysis rate constant at which ITCs are formed | Parameter | |
| Excretion rate constant of ITCs | Parameter | |
| Energy growth rate constant per unit time | Parameter | |
| Metabolic cost (constant) of ITCs | Parameter | |
| Metabolic cost (constant) of foraging | Parameter | |
| T | Maximum duration of herbivory for a larva | Solution of |
| Important acronyms | Full name | Type |
| GLSs | Glucosinolates | Precursor of plant toxins |
| ITCs | Isothiocyanates | Plant toxins |
| OFT | Optimal Foraging Theory | Theory |
| ODEs | Ordinary differential equations | Equations |
Appendix A. Solution of Differential Equations
Appendix A.1. Solution of Equation (6)
Appendix A.2. Solution to Equation (9)
Appendix A.3. Behavior of EN in Equation (9)
References
- Bones, A.M.; Rossiter, J.T. The myrosinase-glucosinolate system, its organisation and biochemistry. Physiol. Plant. 1996, 97, 194–208. [Google Scholar] [CrossRef]
- Halkier, B.A.; Gershenzon, J. Biology and biochemistry of glucosinolates. Annu. Rev. Plant Biol. 2006, 57, 303–333. [Google Scholar] [CrossRef]
- Wittstock, U.; Kliebenstein, J.D.; Lambrix, V.; Reichelt, M.; Gershenzon, J. Glucosinolate hydrolysis and its impact on generalist and specialist insect herbivores. Recent Adv. Phytochem. 2003, 37, 101–125. [Google Scholar]
- Hopkins, R.J.; van Dam, N.M.; van Loon, J.J.A. Role of glucosinolates in insect plant relationships and multitrophic interactions. Annu. Rev. Entomol. 2009, 54, 57–83. [Google Scholar] [CrossRef]
- Sun, R.; Jiang, X.; Reichelt, M.; Gershenzon, J.; Pandit, S.S.; Vassão, D.G. Tritrophic metabolism of plant chemical defenses and its effects on herbivore and predator performance. eLife 2019, 8, e51029. [Google Scholar] [CrossRef]
- Wittstock, U.; Burow, M. Glucosinolate breakdown in Arabidopsis: Mechanism, regulation and biological significance. Arab. Book 2010, 8, e0134. [Google Scholar] [CrossRef]
- Jeschke, V.; Gershenzon, J.; Vassão, D.G. Insect detoxification of glucosinolates and their hydrolysis products. In Advances in Botanical Research; Kopriva, S., Ed.; Elsevier Ltd.: Amsterdam, The Nertherland, 2016; Volume 80, pp. 199–245. [Google Scholar]
- Petschenka, G.; Agrawal, A. How herbivores coopt plant defenses: Natural selection, specialization, and sequestration. Curr. Opin. Insect Sci. 2016, 14, 17–24. [Google Scholar] [CrossRef] [PubMed]
- Ratzka, A.; Vogel, H.; Kliebenstein, D.J.; Mitchell-Olds, T.; Kroymann, J. Disarming the mustard oil bomb. Proc. Natl. Acad. Sci. USA 2002, 99, 11223–11228. [Google Scholar] [CrossRef] [PubMed]
- Sporer, T.; Körnig, J.; Wielsch, N.; Gebauer-Jung, S.; Reichelt, M.; Hupfer, Y.; Beran, F. Hijacking the Mustard-Oil Bomb: How a Glucosinolate-Sequestering Flea Beetle Copes with Plant Myrosinases. Front. Plant Sci. 2021, 12, 645030. [Google Scholar] [CrossRef] [PubMed]
- Wittstock, U.; Agerbirk, N.; Stauber, E.J.; Olsen, C.E.; Hippler, M.; Mitchell-Olds, T.; Gershenzon, J.; Vogel, H. Successful herbivore attack due to metabolic diversion of a plant chemical defense. Proc. Natl. Acad. Sci. USA 2004, 101, 4859–4864. [Google Scholar] [CrossRef] [PubMed]
- Müller, C.; Agerbirk, N.; Olsen, C.; Boevé, J.-L.; Schaffner, U.; Brakefield, P. Sequestration of host plant glucosinolates in the defensive hemolymph of the sawfly Athalia rosae. J. Chem. Ecol. 2001, 27, 2505–2516. [Google Scholar] [CrossRef]
- Jeschke, V.; Kearney, E.E.; Schramm, K.; Kunert, G.; Shekhov, A.; Gershenzon, J.; Vassão, D.G. How Glucosinolates Affect Generalist Lepidopteran Larvae: Growth, Development and Glucosinolate Metabolism. Front. Plant Sci. 2017, 8, 1995. [Google Scholar] [CrossRef]
- Schramm, K.; Vassão, D.G.; Reichelt, M.; Gershenzon, J.; Wittstock, U. Metabolism of glucosinolate-derived isothiocyanates to glutathione conjugates in generalist lepidopteran herbivores. Insect Biochem. Mol. Biol. 2012, 42, 174–182. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, S.; Gershenzon, J.; Schuster, S. Comparing two strategies of counter-defense against plant toxins: A modeling study on plant–herbivore interactions. Front. Ecol. Evol. 2023, 11, 1197757. [Google Scholar] [CrossRef]
- Santolamazza-Carbone, S.; Sotelo, T.; Velasco, P.; Cartea, M.E. Antibiotic properties of the glucosinolates of Brassica oleracea var. acephala similarly affect generalist and specialist larvae of two lepidopteran pests. J. Pest Sci. 2016, 89, 195–206. [Google Scholar] [CrossRef]
- Johansen, N.S. Mortality of eggs, larvae and pupae and larval dispersal of the cabbage moth, Mamestra brassicae, in white cabbage in south-eastern Norway. Entomol. Exp. Appl. 1997, 83, 347–360. [Google Scholar] [CrossRef]
- Agrawal, A.A.; Kurashige, S.N. A role for isothiocyanates in plant resistance against the specialist herbivore Pieris rapae. J. Chem. Ecol. 2003, 29, 1403–1415. [Google Scholar] [CrossRef] [PubMed]
- Gols, R.; Wagenaar, R.; Bukovinszky, T.; van Dam, N.M.; Dicke, M.; Bullock, J.M.; Harvey, J.A. Genetic variation in defense chemistry in wild cabbages affects herbivores and their endoparasitoids. Ecology 2008, 89, 1616–1626. [Google Scholar] [CrossRef] [PubMed]
- Ahuja, I.; Rohloff, J.; Bones, A.M. Defence mechanisms of Brassicaceae: Implications for plant-insect interactions and potential for integrated pest management: A review. Agron. Sustain. Dev. 2010, 30, 311–348. [Google Scholar] [CrossRef]
- Noble, R.R.P.; Harvey, S.G.; Sams, C.E. Toxicity of Indian mustard and allyl isothiocyanate to masked chafer beetle larvae. Plant Health Prog. 2002, 3, 9. [Google Scholar] [CrossRef]
- Cerón, D.A.C.; de Alencar, E.R.; Faroni, L.R.D.; Silva, M.V.D.A.; Salvador, D.V. Toxicity of allyl isothiocyanate applied in systems with or without recirculation for controlling Sitophilus zeamais, Rhyzopertha dominica, and Tribolium castaneum in corn grains. J. Sci. Food Agric. 2023, 103, 6373–6382. [Google Scholar] [CrossRef]
- Stephens, D.W.; Krebs, J.R. Monographs in Behavior and Ecology. In Foraging Theory, 1st ed.; Princeton University Press: Princeton, NJ, USA, 1986. [Google Scholar]
- Werner, E.E.; Hall, D.J. Optimal Foraging and the Size Selection of Prey by the Bluegill Sunfish (Lepomis macrochirus). Ecology 1974, 55, 1042–1052. [Google Scholar] [CrossRef]
- MacArthur, R.H.; Pianka, E.R. On Optimal Use of a Patchy Environment. Am. Nat. 1966, 100, 603–609. [Google Scholar] [CrossRef]
- Merritt, E.J. The Role of Time and Energy in Food Preference. Am. Nat. 1966, 100, 611–617. [Google Scholar]
- Parker, G.; Smith, J.M. Optimality theory in evolutionary biology. Nature 1990, 348, 27–33. [Google Scholar] [CrossRef]
- Schoener, T.W. Theory of Feeding Strategies. Annu. Rev. Ecol. Syst. 1971, 2, 369–404. [Google Scholar] [CrossRef]
- Gripenberg, S.; Mayhew, P.J.; Parnell, M.; Roslin, T. A meta-analysis of preference–performance relati onships in phytophagous insects. Ecol. Lett. 2010, 13, 383–393. [Google Scholar] [CrossRef] [PubMed]
- Videla, M.; Valladares, G.R.; Salvo, A. Choosing between good and better: Optimal oviposition drives host plant selection when parents and offspring agree on best resources. Oecologia 2012, 169, 743–751. [Google Scholar] [CrossRef] [PubMed]
- Srinivasan, B. A guide to the Michaelis–Menten equation: Steady state and beyond. FEBS J. 2022, 289, 6086–6098. [Google Scholar] [CrossRef]
- Alexander, R.A. Chapters “Optimum behaviour”, “Dangers and difficulties” and “Mathematical summary”. In Optima for Animals: Revised Edition; Princeton University Press: Princeton, NJ, USA, 1996; ISBN 9780691027982. [Google Scholar]
- Pszczolkowski, M.A.; Matos, L.F.; Brown, R.; Brown, J.J. Feeding and development of Cydia pomonella (Lepidoptera: Tortricidae) larvae on apple Leaves. Ann. Entomol. Soc. Am. 2002, 95, 603–607. [Google Scholar] [CrossRef]
- Krebs, J.R.; Davies, N.B. An Introduction to Behavioral Ecology, 4th ed.; Blackwell Scientific Publications: Oxford, UK, 1989. [Google Scholar]
- Wolf, T.J.; Schmid-Hempel, P. Extra Loads and Foraging Life Span in Honeybee Workers. J. Anim. Ecol. 1989, 58, 943. [Google Scholar] [CrossRef]
- Manatunge, J.; Asaeda, T. Optimal foraging as the criteria of prey selection by two centrarchid fishes. Hydrobiologia 1998, 391, 221–239. [Google Scholar] [CrossRef]
- Beumer, L.T.; Pohle, J.; Schmidt, N.M.; Chimienti, M.; Desforges, J.-P.; Hansen, L.H.; Langrock, R.; Pedersen, S.H.; Stelvig, M.; van Beest, F.M. An application of upscaled optimal foraging theory using hidden Markov modelling: Year-round behavioural variation in a large arctic herbivore. Mov. Ecol. 2020, 8, 25. [Google Scholar] [CrossRef] [PubMed]
- Smith, E.A.; Bettinger, R.L.; Bishop, C.A.; Blundell, V.; Cashdan, E.; Casimir, M.J.; Christenson, A.L.; Cox, B.; Dyson-Hudson, R.; Hayden, B.; et al. Anthropological Applications of Optimal Foraging Theory: A Critical Review [and Comments and Reply]. Curr. Anthropol. 1983, 24, 625–651. [Google Scholar] [CrossRef]
- Pierce, G.J.; Ollason, J.G. Eight reasons why optimal foraging theory is a complete waste of time. Oikos 1987, 49, 111–118. [Google Scholar] [CrossRef]
- Brauer, F.; Castillo-Chavez, C. Mathematical Models in Population Biology and Epidemiology, 2nd ed.; Springer: New York, NY, USA, 2001. [Google Scholar]
- Martcheva, M. An Introduction to Mathematical Epidemiology, 1st ed.; Springer: New York, NY, USA, 2015. [Google Scholar]
- Chakraborty, S.; Gershenzon, J.; Schuster, S. Selection pressure by specialist and generalist insect herbivores leads to optimal constitutive plant defense. A mathematical model. Ecol. Evol. 2023, 13, e10763. [Google Scholar] [CrossRef]
- Chattopadhyay, J.; Bairagi, N. Pelicans at risk in Salton sea—An eco-epidemiological model. Ecol. Model. 2001, 136, 103–112. [Google Scholar] [CrossRef]
- Chattopadhyay, J.; Pal, S. Viral infection on phytoplankton–zooplankton system—A mathematical model. Ecol. Model. 2002, 151, 15–28. [Google Scholar] [CrossRef]
- Murray, J.D. Mathematical Biology I. An Introduction, 3rd ed.; Springer: New York, NY, USA, 2002. [Google Scholar]
- Knoke, B.; Textor, S.; Gershenzon, J.; Schuster, S. Mathematical modelling of aliphatic glucosinolate chain length distribution in Arabidopsis thaliana leaves. Phytochem. Rev. 2009, 8, 39–51. [Google Scholar] [CrossRef][Green Version]
- Schauer, M.; Heinrich, R. Quasi-steady-state approximation in the mathematical modeling of biochemical reaction networks. Math. Biosci. 1983, 65, 155–170. [Google Scholar]
- Schuster, S.; Ewald, J.; Dandekar, T.; Dühring, S. Optimizing defence, counter-defence and counter-counter defence in parasitic and trophic interactions—A modelling study. arXiv 2019, arXiv:1907.04820. [Google Scholar]
- Jolakoski, P.; Pal, A.; Sandev, T.; Kocarev, L.; Metzler, R.; Stojkoski, V. A first passage under resetting approach to income dynamics. Chaos Solitons Fractals 2023, 175, 113921. [Google Scholar] [CrossRef]
- Stojkoski, V.; Sandev, T.; Kocarev, L.; Pal, A. Geometric Brownian motion under stochastic resetting: A stationary yet nonergodic process. Am. Phys. Soc. 2021, 104, 014121. [Google Scholar] [CrossRef]
- Stojkoski, V.; Karbevski, M. Ergodicity breaking in wealth dynamics: The case of reallocating geometric Brownian motion. Am. Phys. Soc. 2022, 105, 024107. [Google Scholar] [CrossRef]
- Vinod, D.; Cherstvy, A.G.; Metzler, R.; Sokolov, I.M. Time-averaging and nonergodicity of reset geometric Brownian motion with drift. Phys. Rev. E 2022, 106, 034137. [Google Scholar] [CrossRef] [PubMed]
- Furusawa, C.; Suzuki, T.; Kashiwagi, A.; Yomo, T.; Kaneko, K. Ubiquity of log-normal distributions in intra-cellular reaction dynamics. Biophysics 2005, 21, 25–31. [Google Scholar] [CrossRef] [PubMed]
- Iyer-Biswas, S.; Wright, C.S.; Henry, J.T.; Lo, K.; Burov, S.; Lin, Y.; Crooks, G.E.; Crosson, S.; Dinner, A.R.; Scherer, N.F. Scaling laws governing stochastic growth and division of single bacterial cells. Proc. Natl. Acad. Sci. USA 2014, 111, 15912. [Google Scholar] [CrossRef] [PubMed]
- Balaban, N.Q.; Helaine, S.; Lewis, K.; Ackermann, M.; Aldridge, B.; DIAndersson, D.I.; Brynildsen, M.P.; Bumann, D.; Camilli, A.; Collins, J.J.; et al. Definitions and guidelines for research on antibiotic persistence. Nat. Rev. Microbiol. 2019, 17, 441. [Google Scholar]
- Yang, Z.-L.; Kunert, G.; Sporer, T.; Körnig, J.; Beran, F. Glucosinolate abundance and composition in Brassicaceae influence sequestration in a specialist flea beetle. J. Chem. Ecol. 2021, 46, 186–197. [Google Scholar] [CrossRef]
- Ali, J.G.; Agrawal, A.A. Specialist versus generalist insect herbivores and plant defense. Trends Plant Sci. 2012, 17, 293–302. [Google Scholar] [CrossRef] [PubMed]
- Bahar, M.H.; Al Parvez, M.; Rahman, S.; Islam, R. Performance of polyvoltine silkworm Bombyx mori L. on different mulberry plant varieties. Entomol. Res. 2011, 41, 46–52. [Google Scholar] [CrossRef]
- Benesh, D.P.; Weinreich, F.; Kalbe, M. The relationship between larval size and fitness in the tapeworm Schistocephalus solidus: Bigger is better? Oikos 2012, 121, 1391–1399. [Google Scholar] [CrossRef]
- Gligorescu, A.; Toft, S.; Hauggaard-Nielsen, H.; Axelsen, J.A.; Nielsen, S.A. Development, growth and metabolic rate of Hermetia illucens larvae. J. Appl. Entomol. 2019, 143, 875–881. [Google Scholar] [CrossRef]
- Grunert, L.W.; Clarke, J.W.; Ahuja, C.; Eswaran, H.; Nijhout, H.F. A quantitative analysis of growth and size regulation in Manduca sexta: The physiological basis of variation in size and age at metamorphosis. PLoS ONE 2015, 10, e0127988. [Google Scholar] [CrossRef]
- Hota, A.K. Growth in amphibians. Gerontology 1994, 40, 147–160. [Google Scholar] [CrossRef]
- Kivelä, S.M.; Davis, R.B.; Esperk, T.; Gotthard, K.; Mutanen, M.; Valdma, D.; Tammaru, T. Comparative analysis of larval growth in Lepidoptera reveals instar-level constraints. Funct. Ecol. 2020, 34, 1391–1403. [Google Scholar] [CrossRef]
- Nijhout, H.F.; Davidowitz, G.; Roff, D.A. A quantitative analysis of the mechanism that controls body size in Manduca sexta. J. Biol. 2006, 5, 16. [Google Scholar] [CrossRef] [PubMed]
- Jeschke, V.; Zalucki, J.M.; Raguschke, B.; Gershenzon, J.; Heckel, D.G.; Zalucki, M.P.; Vassão, D.G. So much for glucosinolates: A generalist does survive and develop on brassicas, but at what cost? Plants 1997, 10, 962. [Google Scholar] [CrossRef] [PubMed]
- Wittstock, U.; Gershenzon, J. Constitutive plant toxins and their role in defense against herbivores and pathogens. Curr. Opin. Plant Biol. 2002, 5, 300–307. [Google Scholar] [CrossRef] [PubMed]
- Zalucki, J.M.; Heckel, D.G.; Wang, P.; Kuwar, S.; Vassão, D.G.; Perkins, L.; Zalucki, M.P. A Generalist Feeding on Brassicaceae: It Does Not Get Any Better with Selection. Plants 2021, 10, 954. [Google Scholar] [CrossRef] [PubMed]
- Ibanez, S.; Gallet, C.; Després, L. Plant Insecticidal Toxins in Ecological Networks. Toxins 2012, 4, 228–243. [Google Scholar] [CrossRef]
- Wang, S.-D.; Liu, W.; Xue, C.-B.; Luo, W.-C. The effects of luteolin on phenoloxidase and the growth of Spodoptera exigua (Hubner) larvae (Lepidoptera: Noctuidae). J. Pestic. Sci. 2010, 35, 483–487. [Google Scholar] [CrossRef]
- Singh, D.; Bapatla, K.G. Toxicity and lethal effects of herbaceous plant crude extracts against Spodoptera litura. J. Basic Appl. Zool. 2022, 83, 8. [Google Scholar] [CrossRef]
- Zulhussnain, M.; Zahoor, M.K.; Rizvi, H.; Zahoor, M.A.; Rasul, A.; Ahmad, A.; Majeed, H.N.; Rasul, A.; Ranian, K.; Jabeen, F. Insecticidal and Genotoxic effects of some indigenous plant extracts in Culex quinquefasciatus Say Mosquitoes. Sci. Rep. 2020, 10, 6826. [Google Scholar] [CrossRef]
- Dicke, M.; Baldwin, I.T. The evolutionary context for herbivore-induced plant volatiles: Beyond the ‘cry for help’. Trends Plant Sci. 2010, 15, 167–175. [Google Scholar] [CrossRef]
- Gatehouse, J.A. Plant resistance towards insect herbivores: A dynamic interaction. New Phytol. 2002, 156, 145–169. [Google Scholar] [CrossRef]
- Blande, J.D.; Pickett, J.A.; Poppy, G.M. A comparison of semiochemically mediated interactions involving specialist and generalist Brassica-feeding aphids and the braconid parasitoid Diaeretiella rapae. J. Chem. Ecol. 2007, 33, 767–779. [Google Scholar] [CrossRef] [PubMed]
- Mumm, R.; Burow, M.; Bukovinszkine’Kiss, G.; Kazantzidou, E.; Wittstock, U.; Dicke, M.; Gershenzon, J. Formation of simple nitriles upon glucosinolate hydrolysis affects direct and indirect defense against the specialist herbivore, Pieris rapae. J. Chem. Ecol. 2008, 34, 1311–1321. [Google Scholar] [CrossRef] [PubMed]
- van der Meijden, E. Plant defence, an evolutionary dilemma: Contrasting effects of (specialist and generalist) herbivores and natural enemies. Entomol. Exp. Appl. 1996, 80, 307–310. [Google Scholar] [CrossRef]
- Van Poecke, R.M.P.; Posthumus, M.A.; Dicke, M. Herbivore-induced volatile production by Arabidopsis thaliana leads to attraction of the parasitoid Cotesia rubecula: Chemical, behavioral, and gene-expression analysis. J. Chem. Ecol. 2001, 27, 1911–1928. [Google Scholar] [CrossRef] [PubMed]
- Reddy, G.V.; Holopainen, J.K.; Guerrero, A. Olfactory responses of Plutella xylostella natural enemies to host pheromone, larval frass, and green leaf cabbage volatiles. J. Chem. Ecol. 2002, 28, 131–143. [Google Scholar] [CrossRef] [PubMed]
- Fergola, P.; Wang, W. On the influences of defensive volatiles of plants in tritrophic interactions. J. Biol. Syst. 2011, 19, 345–363. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, D.; An, M.; Fu, Y.; Zeng, R.; Luo, S.; Wu, H.; Pratley, J. Modelling tritrophic interactions mediated by induced defence volatiles. Ecol. Model. 2009, 220, 3241–3247. [Google Scholar] [CrossRef]
- Oerke, E.C.; Dehne, H.W. Safeguarding production—losses in major crops and the role of crop protection. Crop Prot. 2004, 23, 275–285. [Google Scholar] [CrossRef]
- Zhang, Z.; Sun, P.; Zhao, J.; Zhang, H.; Wang, X.; Li, L.; Xiong, L.; Yang, N.; Li, Y.; Yuchi, Z.; et al. Design, synthesis and biological activity of diamide compounds based on 3-substituent of the pyrazole ring†. Pest Manag. Sci. 2022, 78, 2022–2033. [Google Scholar] [CrossRef] [PubMed]
- Bhuvaneswari, K.; Mani, M.; Suganthi, A.; Manivannan, A. Novel Insecticides and Their Application in the Management of Horticultural Crop Pests. In Trends in Horticultural Entomology; Mani, M., Ed.; Springer: Singapore, 2022. [Google Scholar]
- Cassereau, J.; Ferré, M.; Chevrollier, A.; Codron, P.; Verny, C.; Homedan, C.; Lenaers, G.; Procaccio, V.; May-Panloup, P.; Reynier, P. Neurotoxicity of Insecticides. Curr. Med. Chem. 2017, 24, 2988–3001. [Google Scholar] [CrossRef] [PubMed]
- Mansour, R.; Grissa-Lebdi, K.; Suma, P.; Mazzeo, G.; Russo, A. Key scale insects (Hemiptera: Coccoidea) of high economic importance in a Mediterranean area: Host plants, bio-ecological characteristics, natural enemies and pest management strategies—A review. Plant Prot. Sci. Czech Acad. Agric. Sci. 2017, 53, 1–14. [Google Scholar] [CrossRef]
- Guedes, R.N.C.; Smagghe, G.; Stark, J.D.; Desneux, N. Pesticide-induced stress in arthropod pests for optimized integrated pest management programs. Annu. Rev. Entomol. Annu. Rev. 2016, 61, 43–62. [Google Scholar] [CrossRef]
- Dyck, V.A.; Hendrichs, J.; Robinson, A.S. (Eds.) Sterile Insect Technique: Principles And Practice. In Area-Wide Integrated Pest Management, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2021. [Google Scholar]
- Letourneau, D.K.; Armbrecht, I.; Rivera, B.S.; Lerma, J.M.; Carmona, E.J.; Daza, M.C.; Escobar, S.; Galindo, V.; Gutiérrez, C.; López, S.D.; et al. Does plant diversity benefit agroecosystems? A synthetic review. Ecol. Appl. 2011, 21, 9–21. [Google Scholar] [CrossRef]
- Bond, R.A.B.; Martincigh, B.S.; Mika, J.R.; Simoyi, R.H. The Quasi-Steady-State Approximation: Numerical Validation. J. Chem. Educ. 1998, 75, 1158–1165. [Google Scholar] [CrossRef]

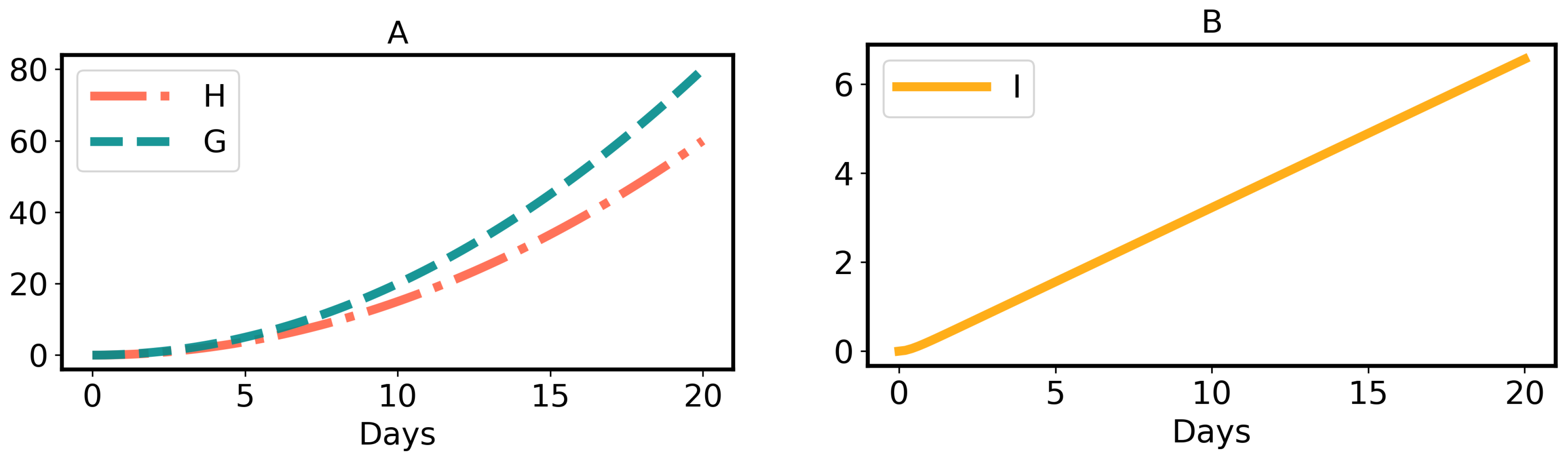


| Instar | Cumulative Leaf Consumption (or Herbivory) per Larva (Mean) | Duration of Instar (Mean) |
|---|---|---|
| 1 | 0.588 mg | 3.54 days |
| 2 | 2.713 mg | 3.27 days |
| 3 | 8.001 mg | 3.27 days |
| 4 | 37.667 mg | 4.5 days |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chakraborty, S.; Schuster, S. How Plant Toxins Cause Early Larval Mortality in Herbivorous Insects: An Explanation by Modeling the Net Energy Curve. Toxins 2024, 16, 72. https://doi.org/10.3390/toxins16020072
Chakraborty S, Schuster S. How Plant Toxins Cause Early Larval Mortality in Herbivorous Insects: An Explanation by Modeling the Net Energy Curve. Toxins. 2024; 16(2):72. https://doi.org/10.3390/toxins16020072
Chicago/Turabian StyleChakraborty, Suman, and Stefan Schuster. 2024. "How Plant Toxins Cause Early Larval Mortality in Herbivorous Insects: An Explanation by Modeling the Net Energy Curve" Toxins 16, no. 2: 72. https://doi.org/10.3390/toxins16020072
APA StyleChakraborty, S., & Schuster, S. (2024). How Plant Toxins Cause Early Larval Mortality in Herbivorous Insects: An Explanation by Modeling the Net Energy Curve. Toxins, 16(2), 72. https://doi.org/10.3390/toxins16020072





