On-Orbit Radiometric Calibration for a Space-Borne Multi-Camera Mosaic Imaging Sensor
Abstract
:1. Introduction
2. Satellites, Test Sites and Datasets
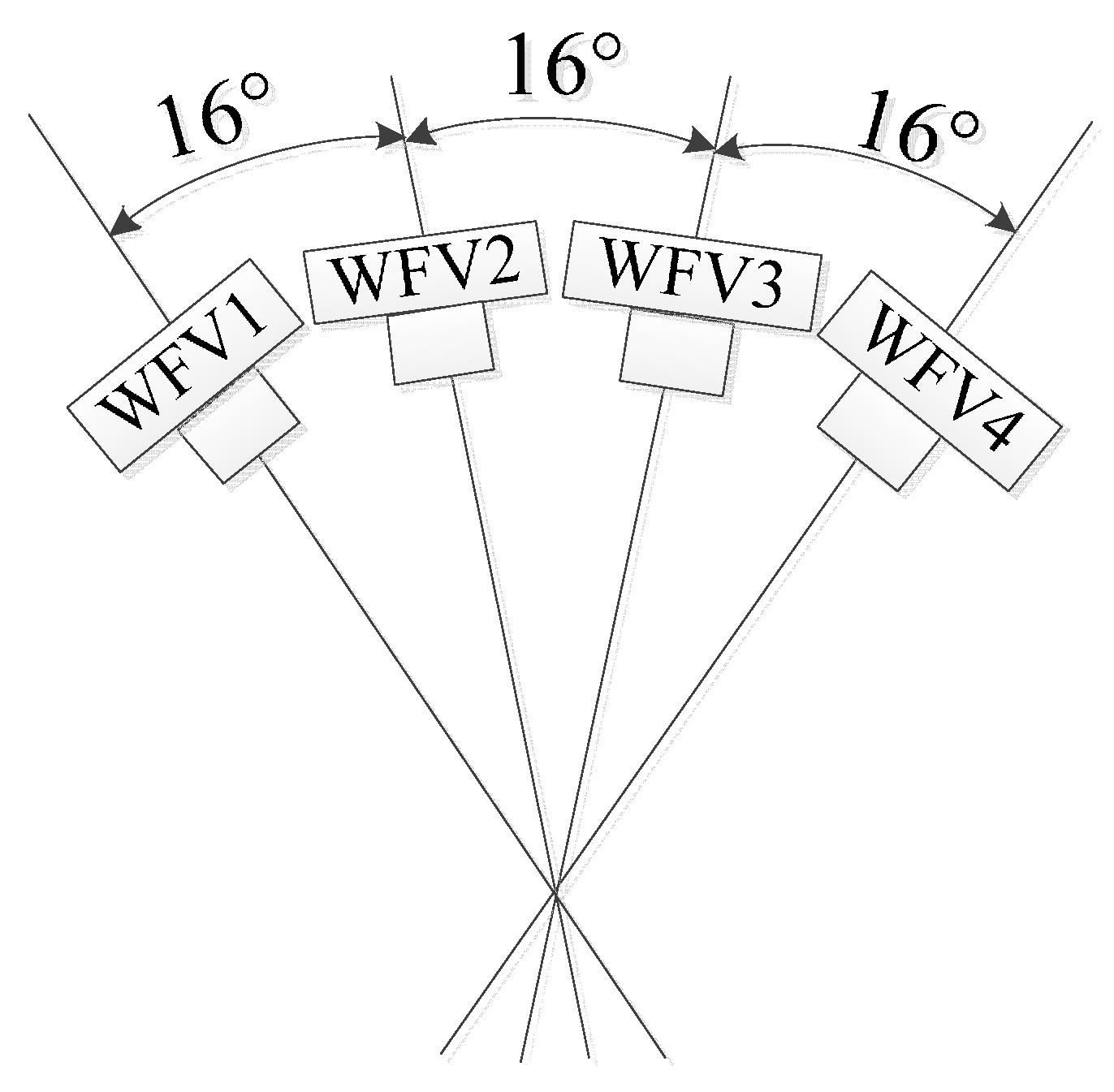
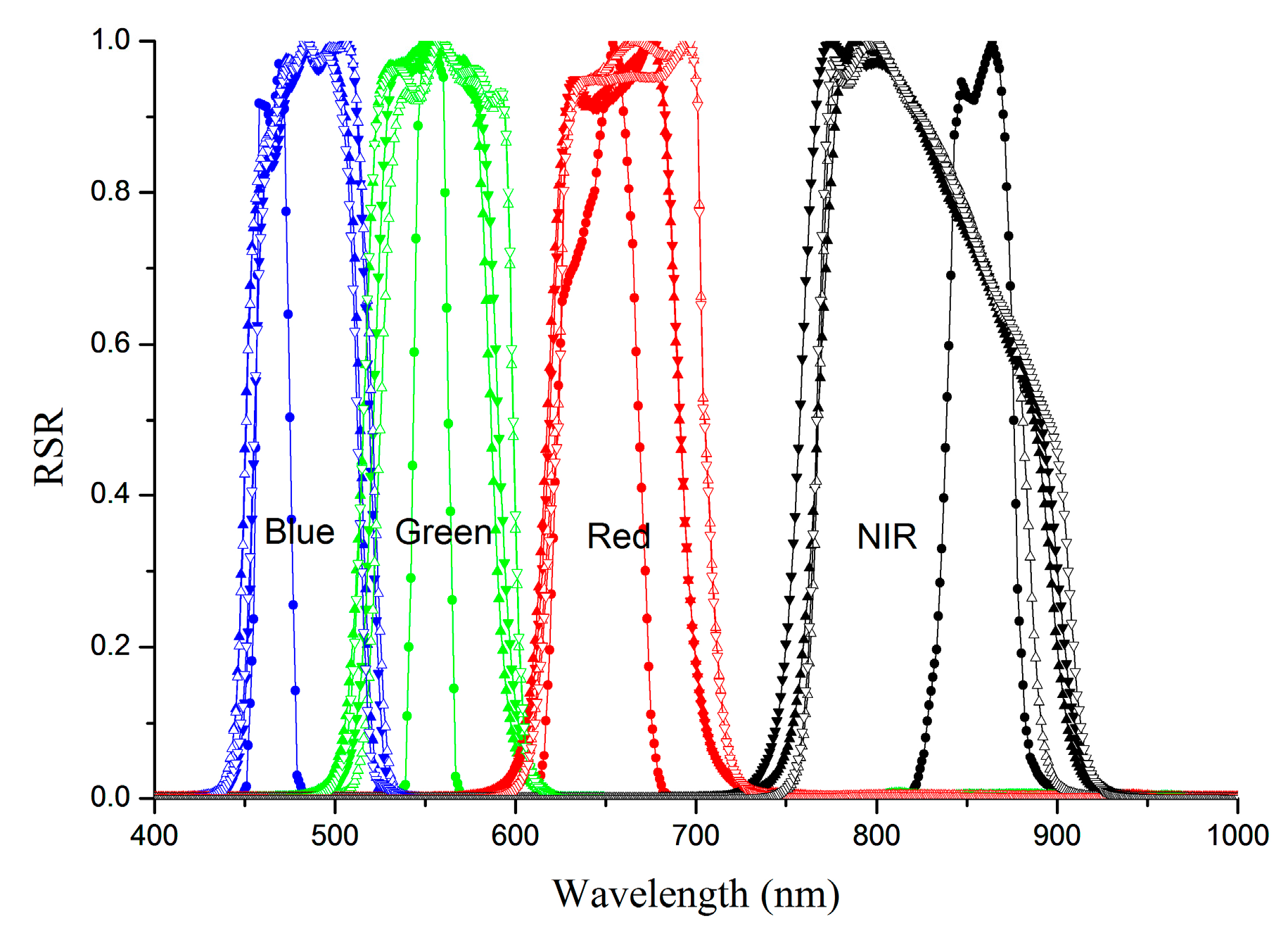
2.1. Satellites
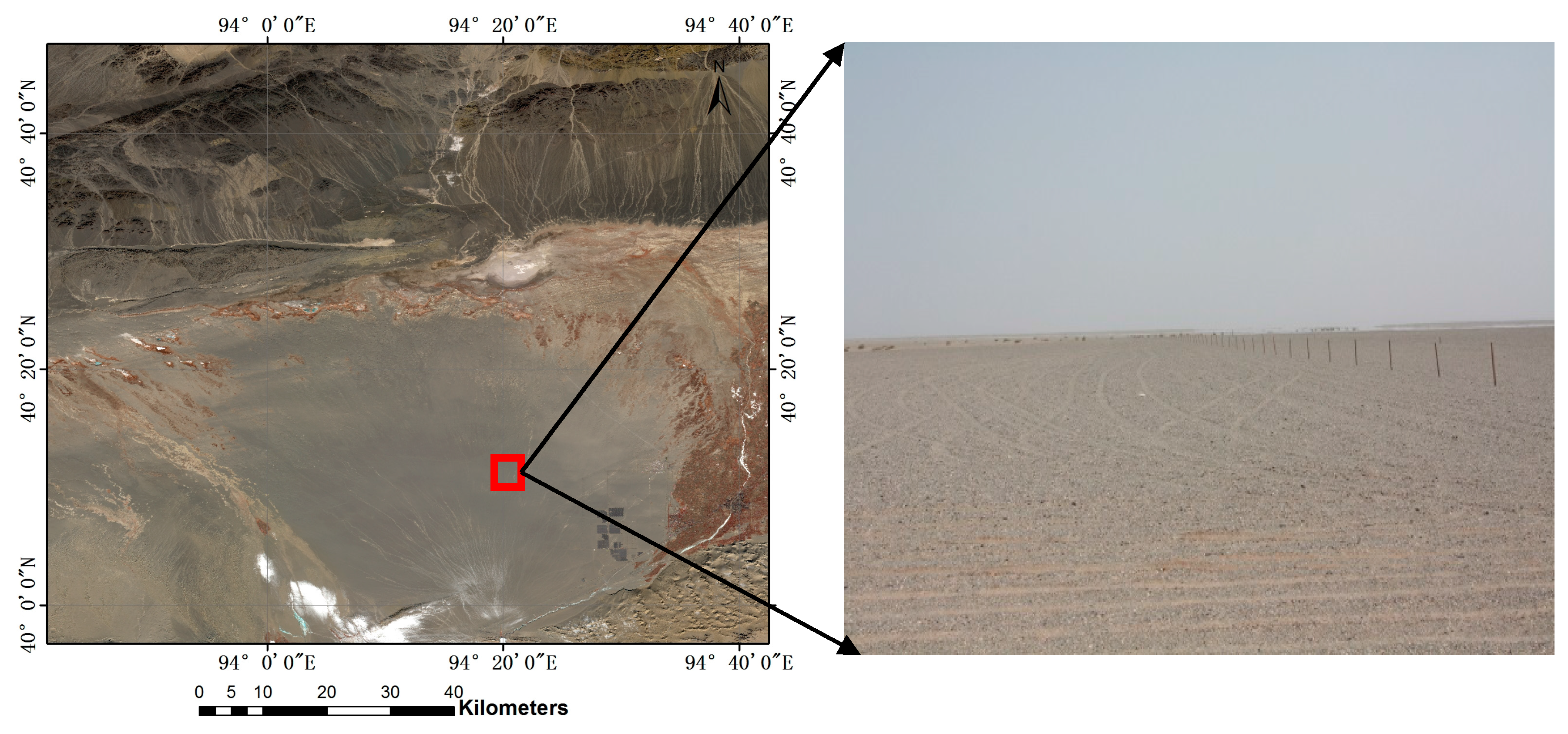
2.2. Test Sites
2.3. Datasets
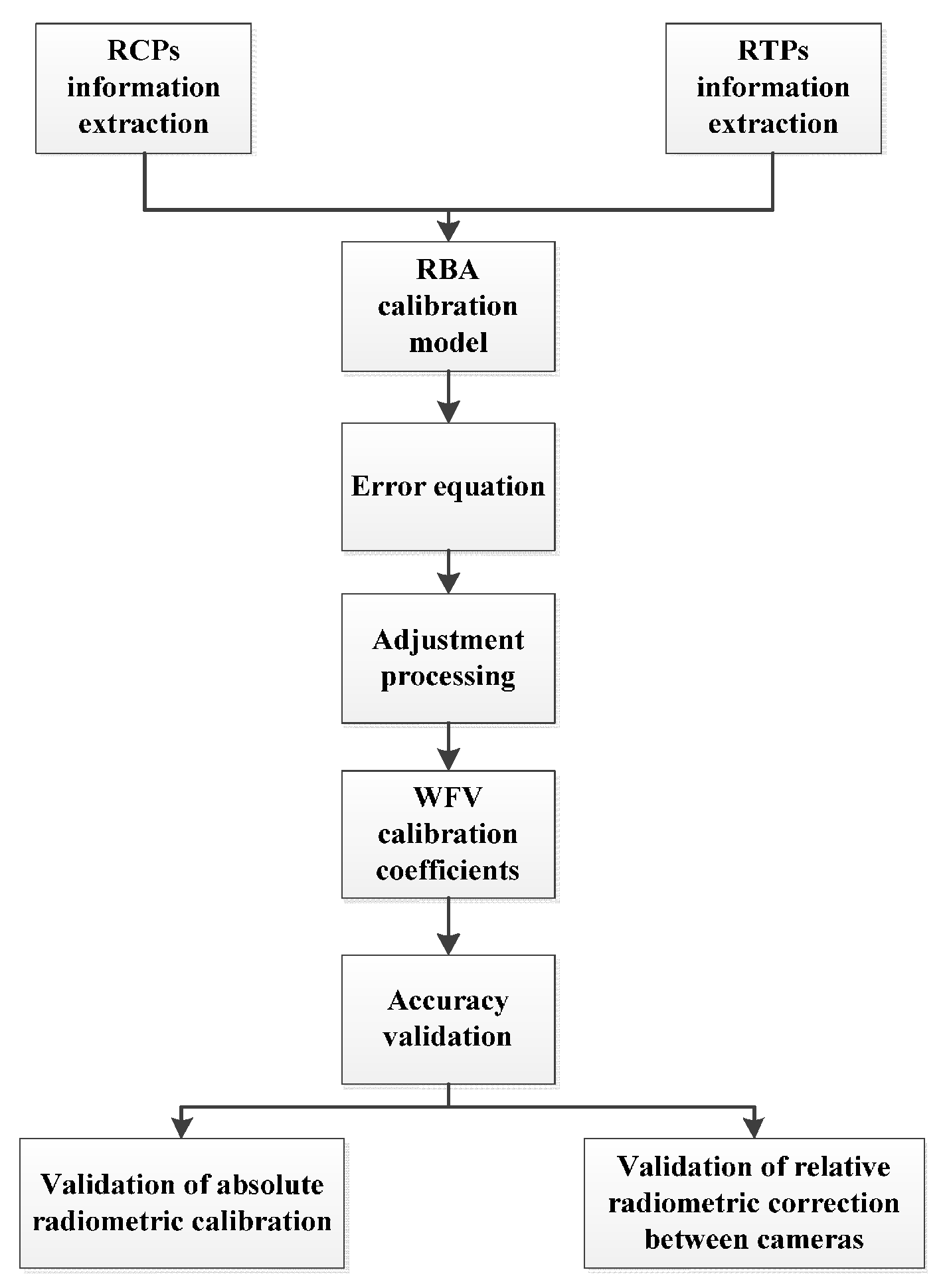
3. Methodology
3.1. Brief Review of the RBA
3.2. On-Orbit Radiometric Calibration Model Based on the RBA
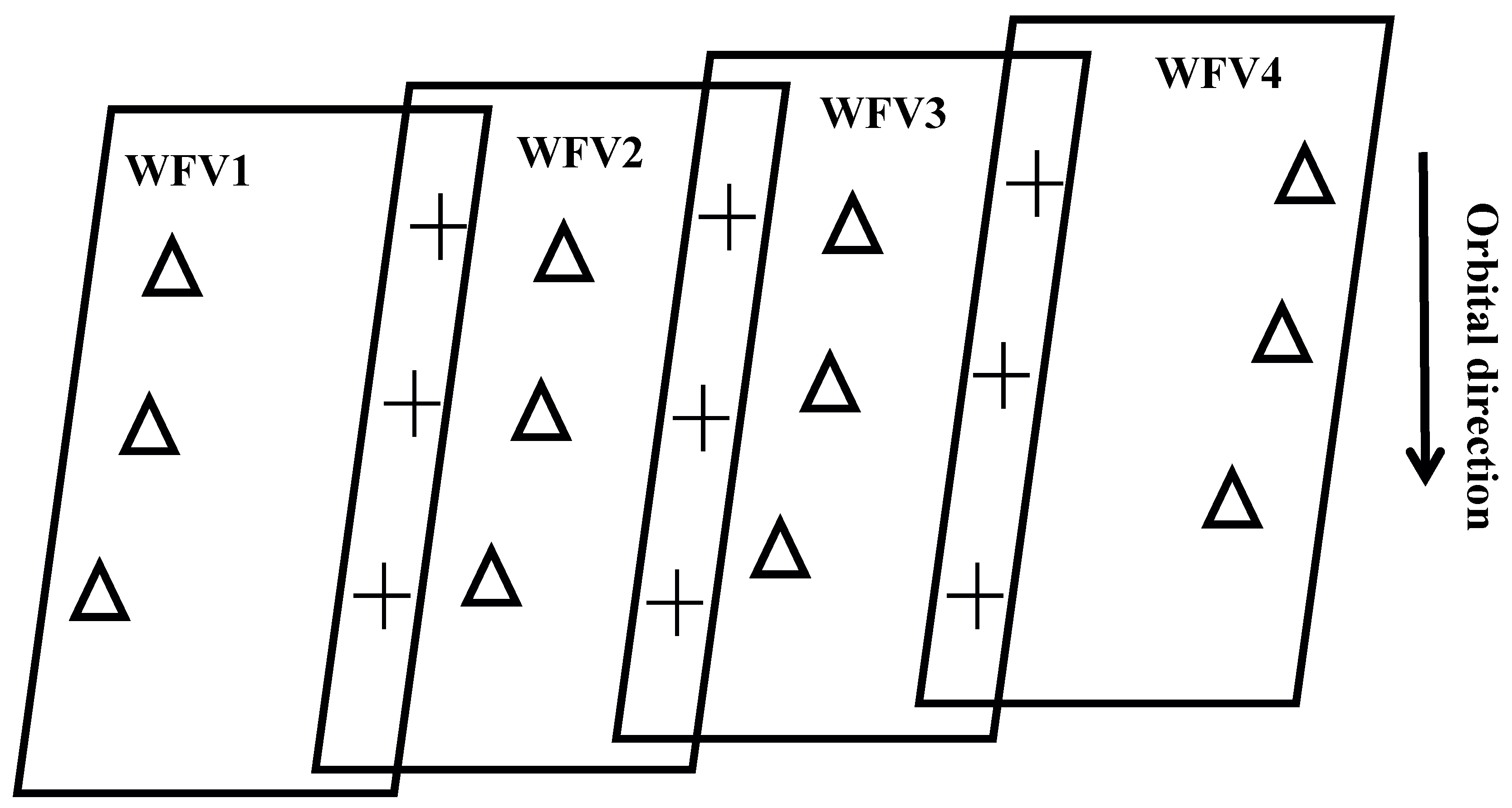
3.2.1. RCPs Information Extraction
3.2.2. RTPs’ Information Extraction
Geometrical Misalignment Elimination
RTPs’ Extraction Standard
Radiometric Constraint Condition
3.2.3. Radiometric Calibration Model Establishment
4. Results
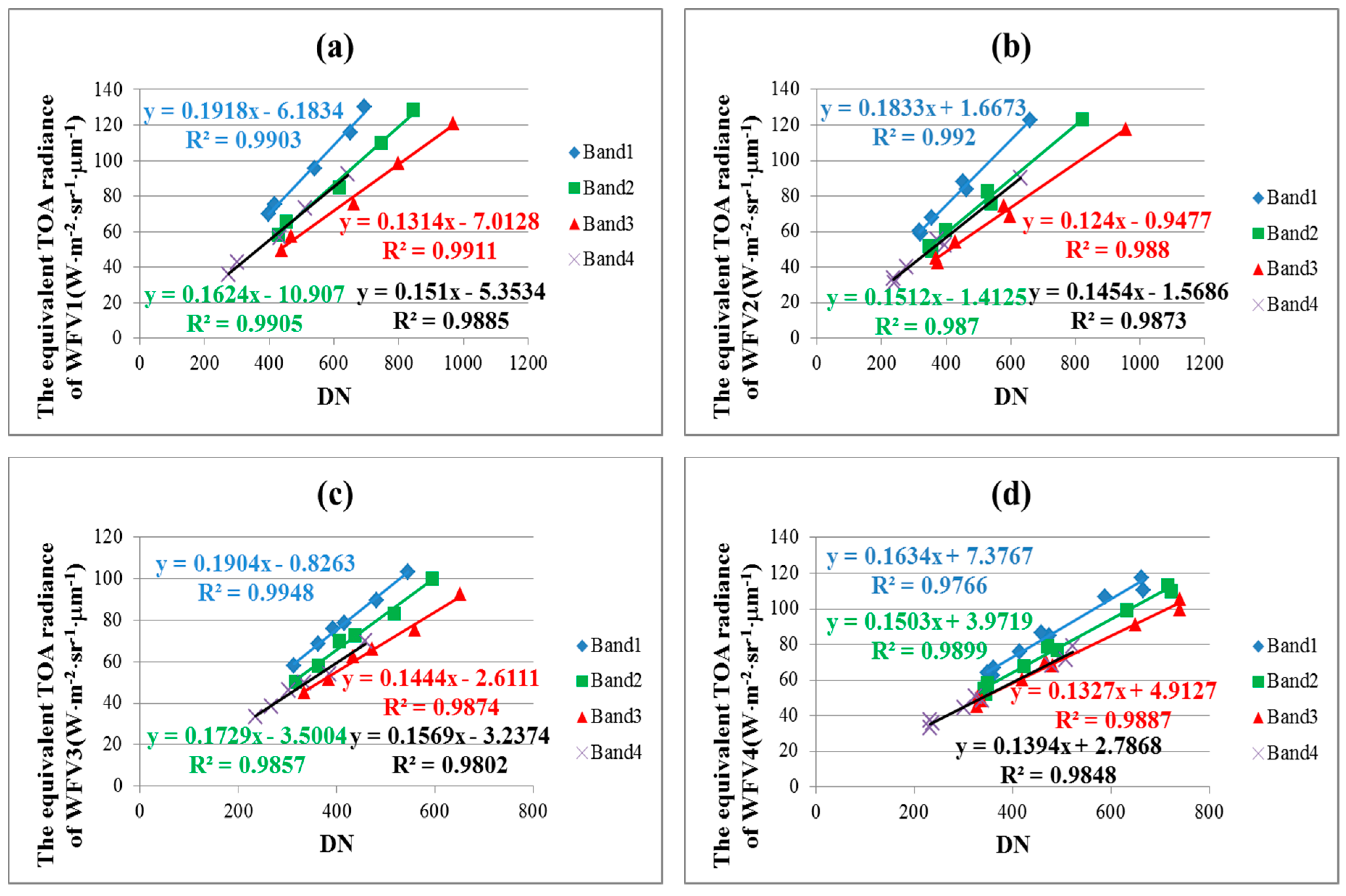
4.1. Calibration Results
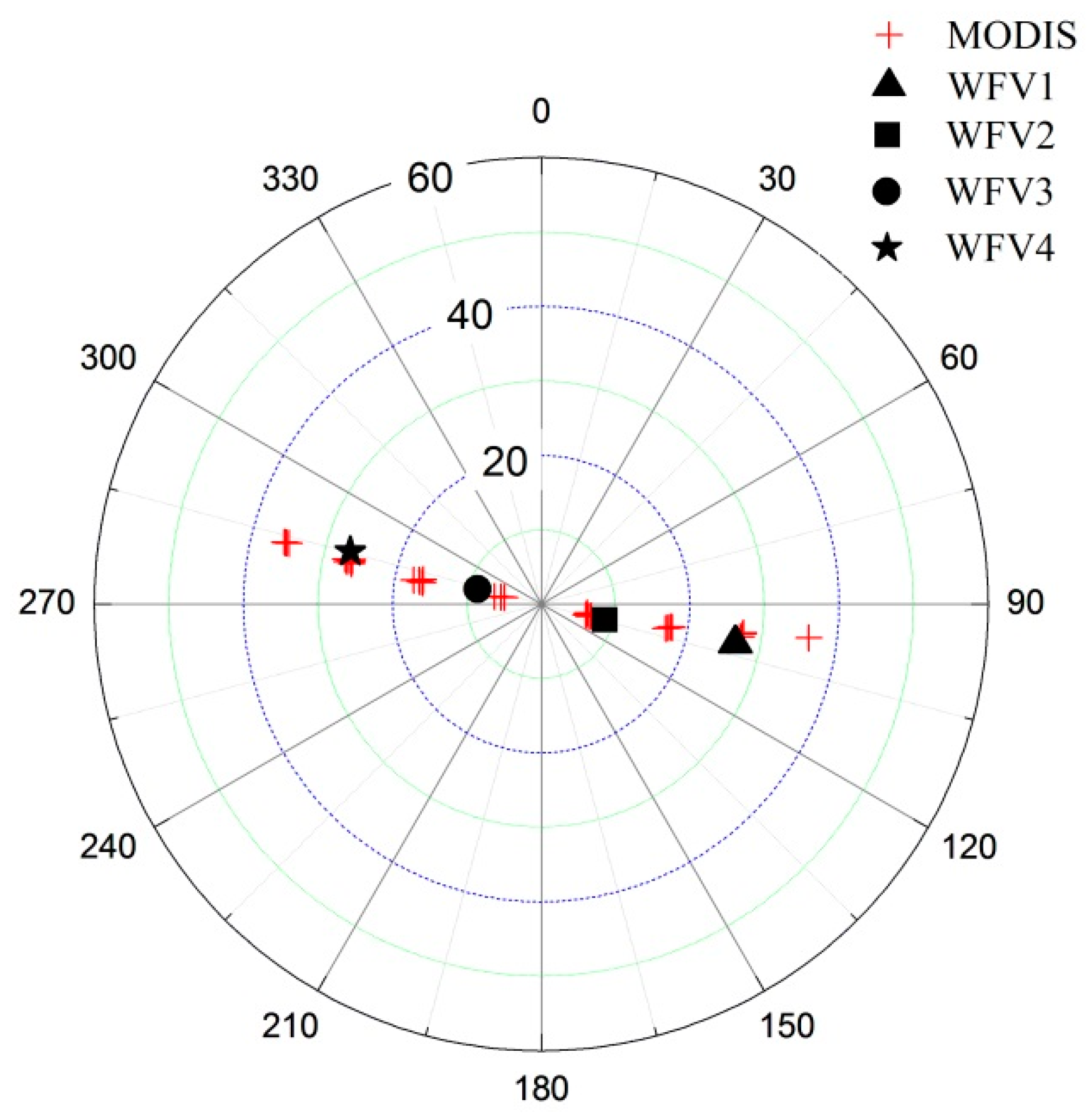
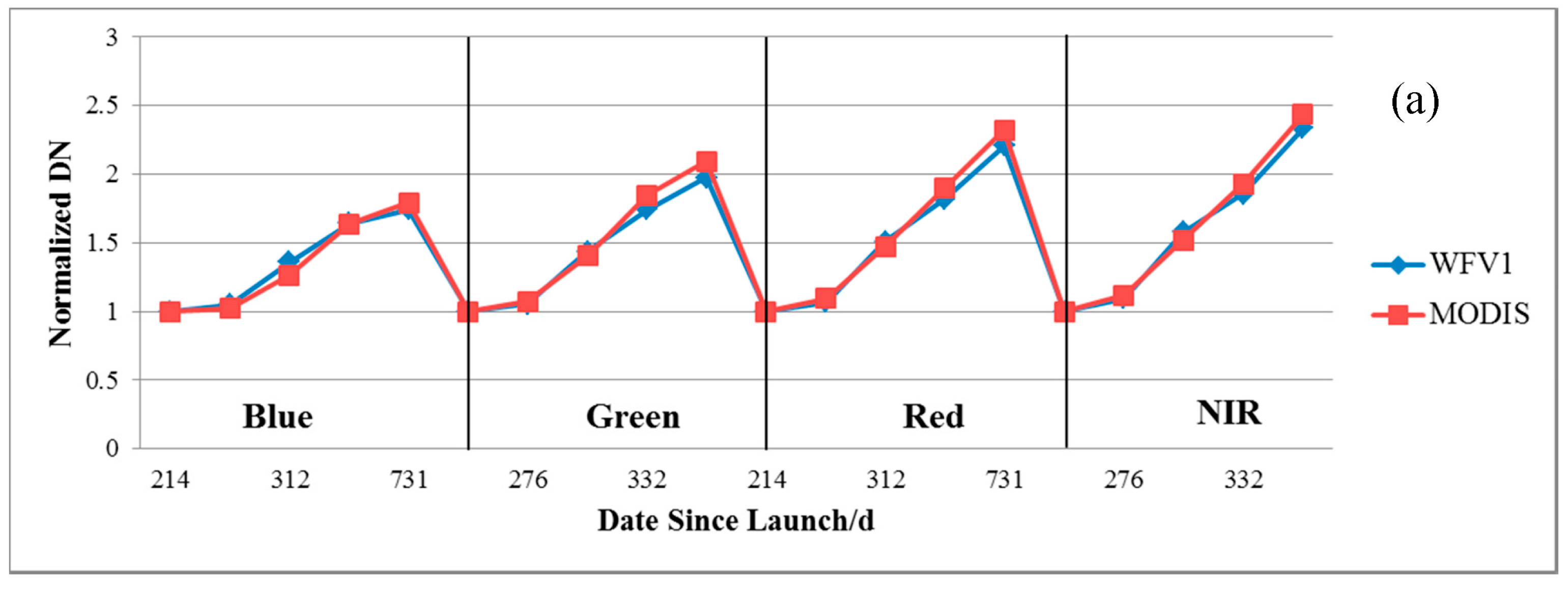
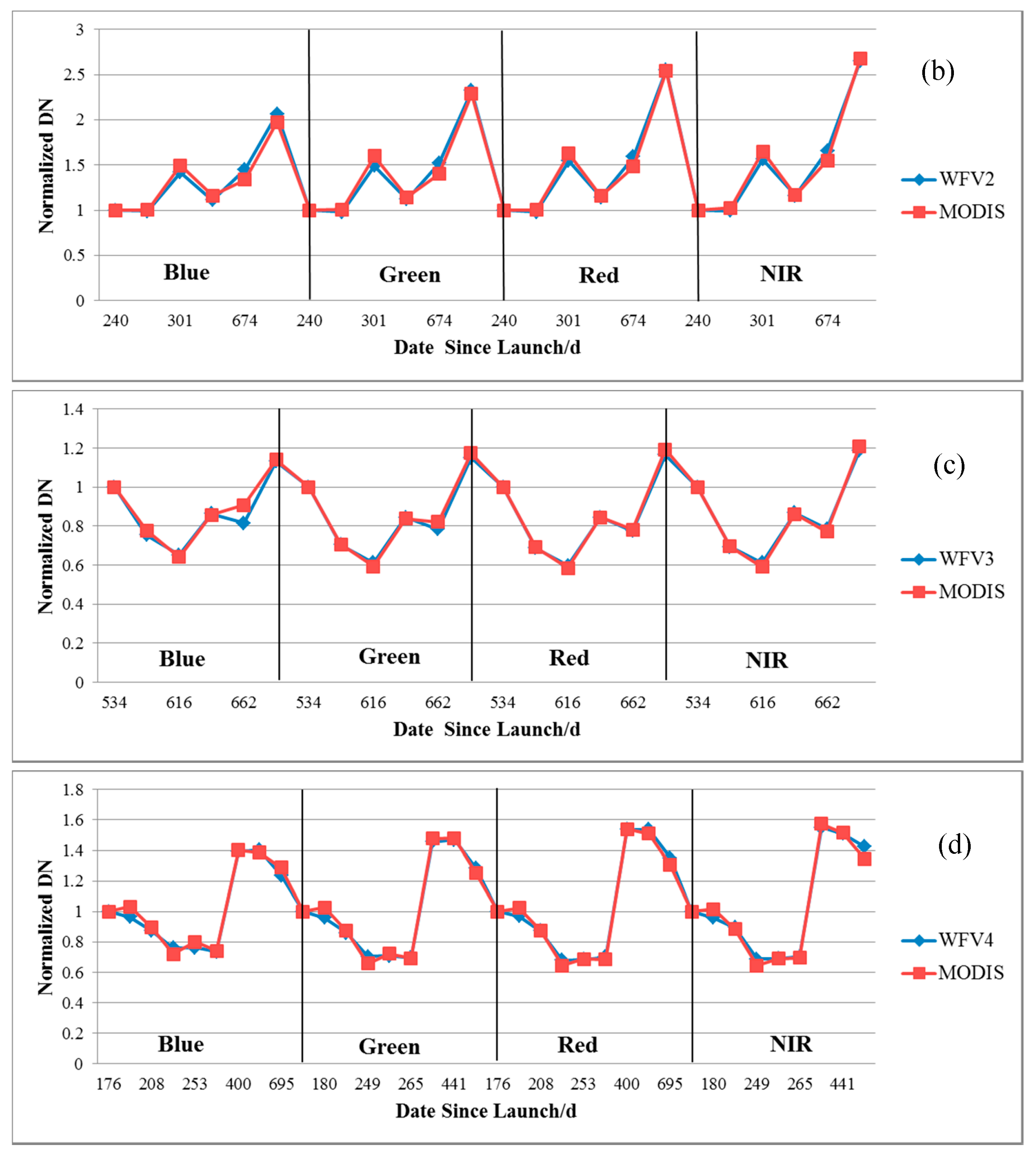
4.2. Validation of Absolute Radiometric Calibration
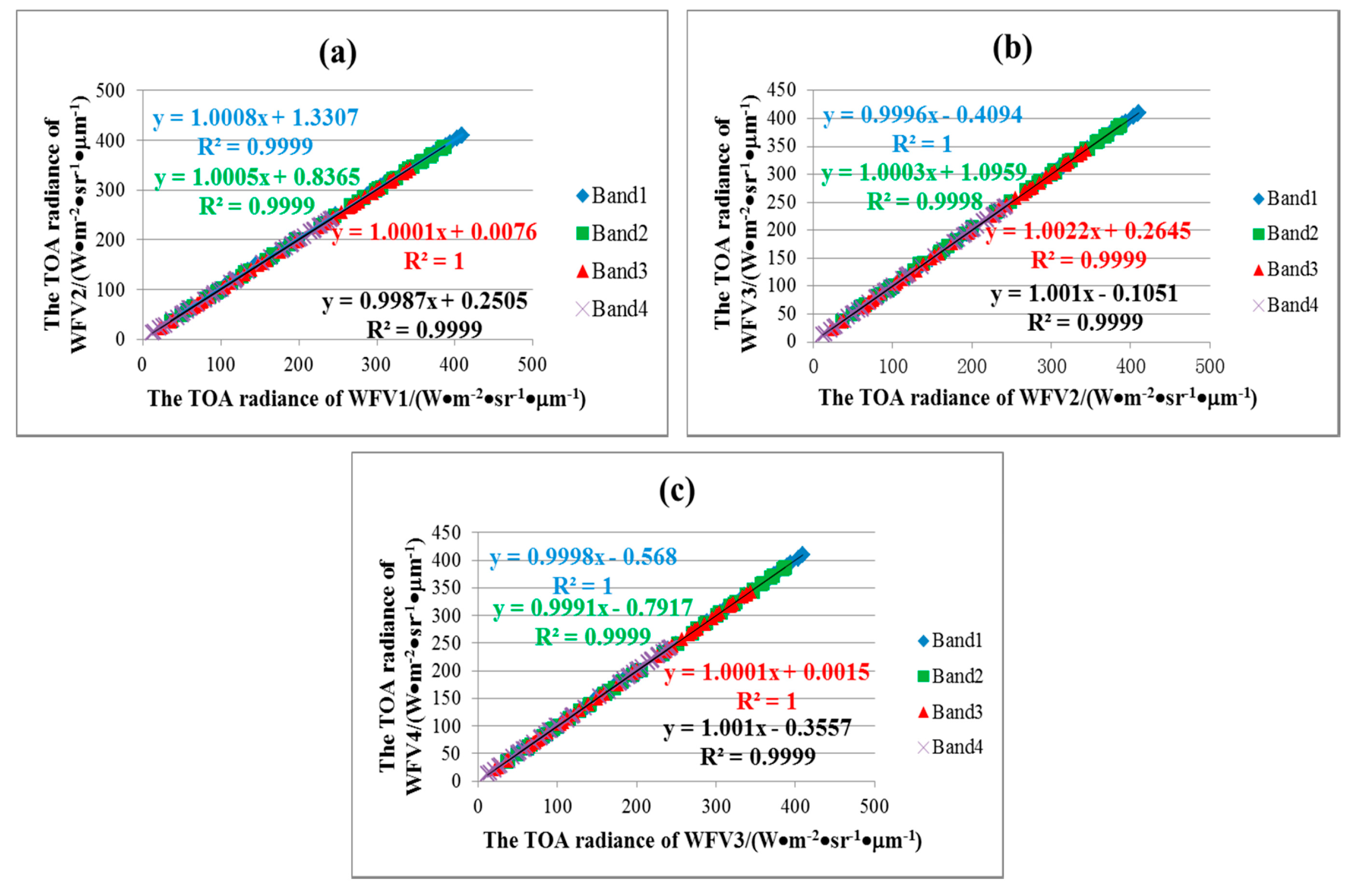
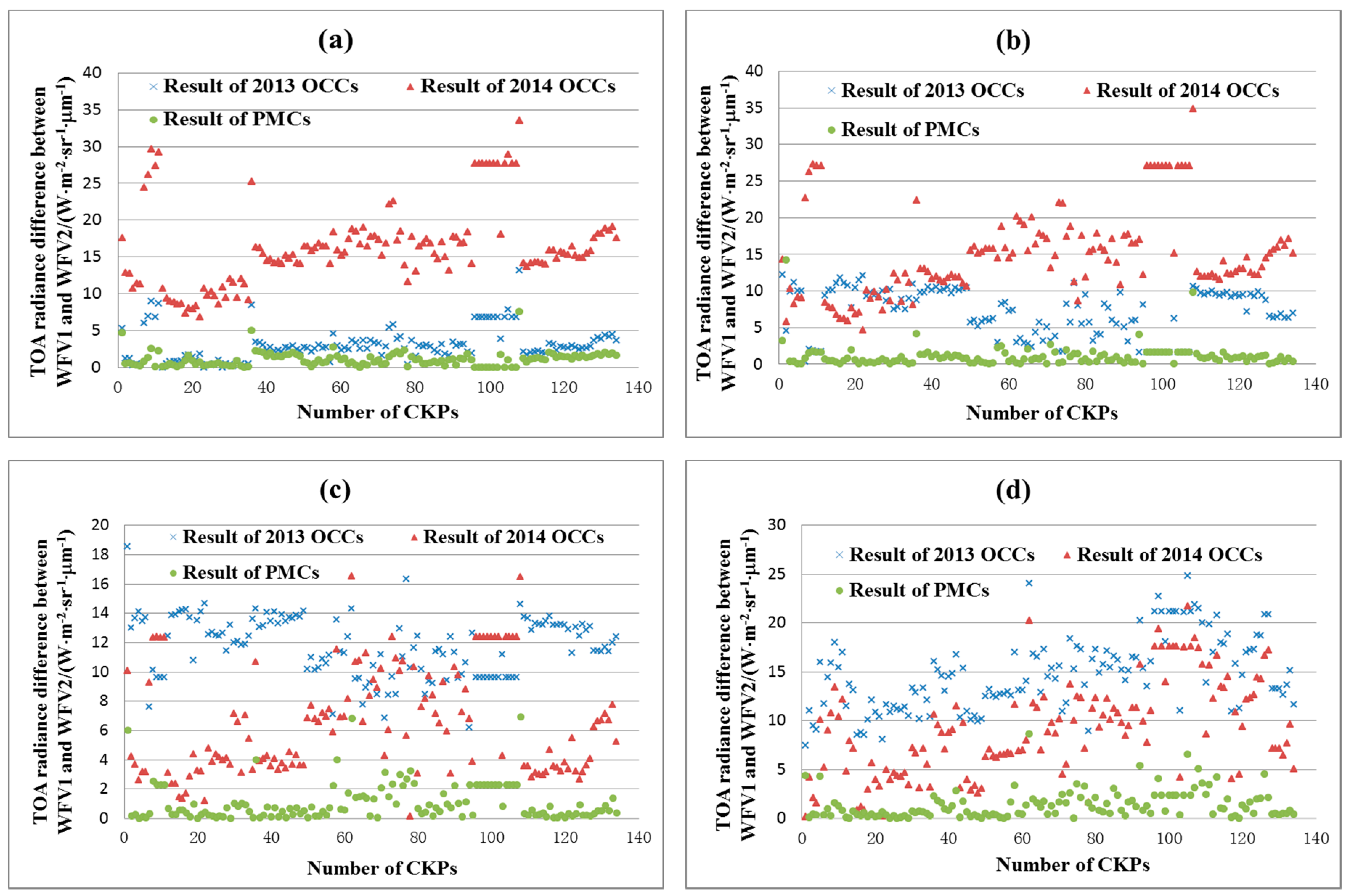
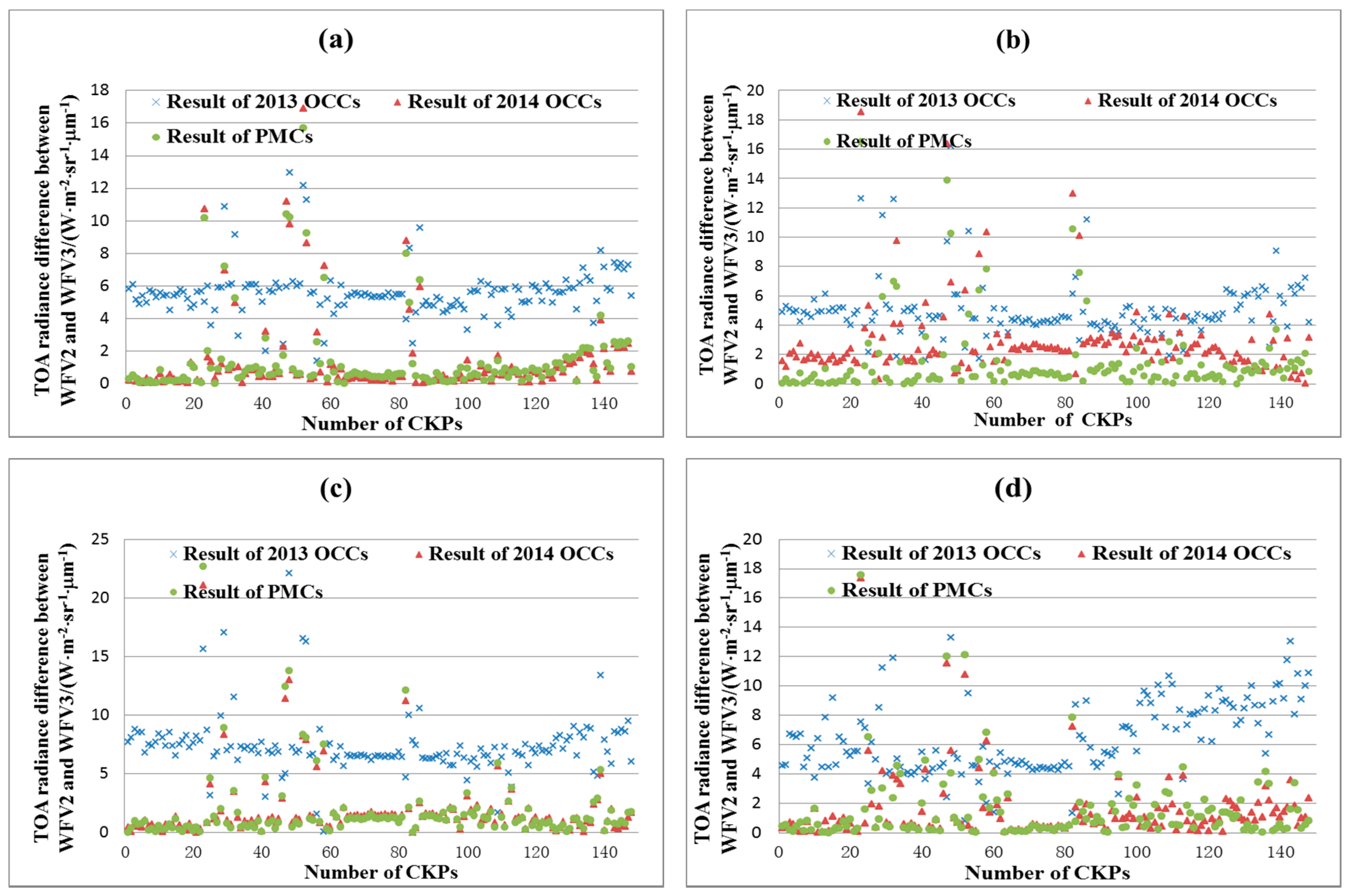
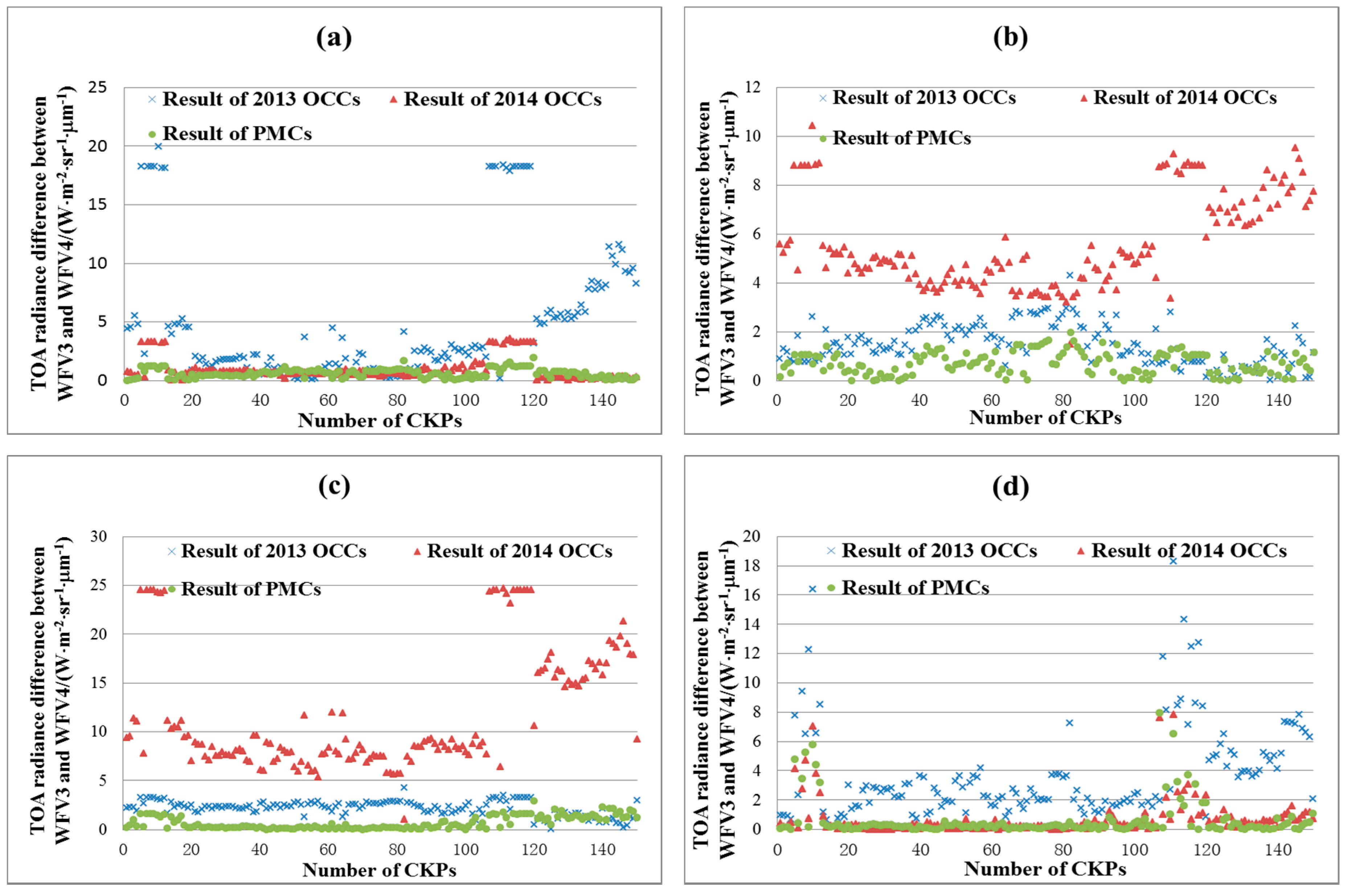
4.3. Validation of Relative Radiometric Correction between Cameras
5. Discussion
5.1. Each WFV Camera Calibrated by the Cross-Calibration Method Independently
5.2. Uncertainty Caused by RCPs Distribution
- On the whole, the REs of the calibration coefficients for each WFV camera are down or stable at a small value when using more RCPs located in different WFV cameras. Taking the WFV1 camera as an example, the REs of each band become lower from 8.39%, 8.78%, 4.89% and 14.95% to 6.61%, 7.91%, 4.39% and 6.50%. This is because more RCPs extracted from different cameras can effectively reduce the calibration uncertainty.
- The REs of the WFV1 camera calculated by the RBA only with RCPs in the WFV1 camera are larger than that of the WFV1 camera calculated by cross-calibration listed in Table 6. The reason may be that the different accuracies and distributions of RTPs in different overlapping regions have an impact on the absolute radiometric calibration to some degree. However, when not all WFV cameras have RCPs, although the calibration coefficients calculated by RBA have slightly larger REs than those of the traditional cross-calibration, except for the near-infrared band, the RBA can obtain the calibration coefficients of other cameras without RCPs based on the radiometric constraint condition between the adjacent cameras.
- Only four cameras’ RCPs are introduced in the RBA model, the REs of all cameras become lower in the near-infrared band. However, the REs of the first three bands are all less than 8.78% even if only one camera’s RCPs are used. This is probably because the hypothesis is more suitable for the first three bands so that the actual radiometric performance differences of different cameras in the same band can be neglected.
6. Summary and Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Thome, K.J. Absolute radiometric calibration of Landsat 7 ETM+ using the reflectance-based method. Remote Sens. Environ. 2001, 78, 27–38. [Google Scholar] [CrossRef]
- Chander, G.; Markham, B. Revised Landsat-5 TM radiometric calibration procedures and postcalibration dynamic ranges. IEEE Trans. Geosci. Remote Sens. 2003, 41, 2674–2677. [Google Scholar] [CrossRef]
- Mishra, N.; Helder, D.; Angal, A.; Choi, J.; Xiong, X. Absolute calibration of optical satellite sensors using Libya 4 pseudo invariant calibration site. Remote Sens. 2014, 6, 1327–1346. [Google Scholar] [CrossRef]
- Thome, K.J.; Hekder, D.L.; Aaron, D.; Dewald, J.D. Landsat-5 TM and Landsat-7 ETM+ absolute radiometric calibration using the reflectance-based method. IEEE Trans. Geosci. Remote Sens. 2004, 42, 2777–2785. [Google Scholar] [CrossRef]
- Gao, H.; Gu, X.; Yu, T.; Sun, Y.; Liu, Q. Cross-calibration of gf-1 pms sensor with landsat 8 oli and terra modis. IEEE Trans. Geosci. Remote Sens. 2016, 54, 4847–4854. [Google Scholar] [CrossRef]
- Xiong, X.; Barnes, W. An overview of MODIS radiometric calibration and characterization. Adv. Atmos. Sci. 2006, 23, 69–79. [Google Scholar] [CrossRef]
- Xiong, X.; Sun, J.; Barnes, W.; Salomonson, V.; Esposito, J.; Erives, H.; Guenther, B. Multiyear on-orbit calibration and performance of terra MODIS reflective solar bands. IEEE Trans. Geosci. Remote Sens. 2007, 45, 879–889. [Google Scholar] [CrossRef]
- Han, J.; Xie, Y. Image dodging algorithm for gf-1 satellite wfv imagery. Acta Geodaeticaet Cartogr. Sin. 2016, 45, 1423–1433. [Google Scholar]
- Du, Y.; Cihlar, J.; Beaubien, J.; Latifovic, R. Radiometric normalization, compositing, and quality control for satellite high resolution image mosaics over large areas. IEEE Trans. Geosci. Remote Sens. 2001, 39, 623–634. [Google Scholar]
- Zhang, Y.; Yu, L.; Sun, M.; Zhu, X. A mixed radiometric normalization method for mosaicking of high-resolution satellite imagery. IEEE Trans. Geosci. Remote Sens. 2017, 55, 2972–2984. [Google Scholar] [CrossRef]
- Gao, H.; Gu, X.; Yu, T.; Li, L.; Sun, Y.; Xie, Y.; Liu, Q. Validation of the calibration coefficient of the gaofen-1 pms sensor using the landsat 8 Oli. Remote Sens. 2016, 8, 132. [Google Scholar] [CrossRef]
- Bai, Z. The Technical Characteristics of GF-1 Satellite. Aerospace China 2013, 5–9. (In Chinese) [Google Scholar]
- Hu, X.; Liu, J.; Sun, L.; Rong, Z.; Li, Y.; Zhang, Y.; Zheng, Z.; Wu, R.; Zhang, L.; Gu, X. Characterization of CRCS dunhuang test site and vicarious calibration utilization for fengyun (FY) series sensors. Can. J. Remote Sens. 2010, 36, 566–582. [Google Scholar] [CrossRef]
- Liu, J.; Li, Z. A new method for cross-calibration of two satellite sensors. Int. J. Remote Sens. 2004, 25, 5267–5281. [Google Scholar] [CrossRef]
- Wang, Z.; Xiao, P.; Gu, X.; Feng, X.; Li, X.; Gao, H.; Li, H.; Lin, J.; Zhang, X. Uncertainty analysis of cross-calibration for hj-1 CCD camera. Sci. China Tech. Sci. 2013, 56, 713–723. [Google Scholar] [CrossRef]
- Sun, L.; Hu, X.; Guo, M.; Xu, N. Multisite calibration tracking for FY-3A MERSI solar bands. IEEE Trans. Geosci. Remote Sens. 2012, 50, 4929–4942. [Google Scholar] [CrossRef]
- Chen, Y.; Sun, K.; Li, D.; Bai, T.; Huang, C. Radiometric cross-calibration of gf-4 pms sensor based on assimilation of landsat-8 oli images. Remote Sens. 2017, 9, 811. [Google Scholar] [CrossRef]
- Chen, S.; Zhang, T. An improved cloud masking algorithm for MODIS ocean colour data processing. Remote Sens. Lett. 2015, 6, 218–227. [Google Scholar] [CrossRef]
- Wolfe, R.E.; Nishihama, M.; Fleig, A.J.; Kuyper, J.A.; Roy, D.P.; Storey, J.C.; Patt, F.S. Achieving sub-pixel geolocation accuracy in support of MODIS land science. Remote Sens. Environ. 2002, 83, 31–49. [Google Scholar] [CrossRef]
- Chandelier, L.; Martinoty, G. Radiometric aerial triangulation for the equalization of digital aerial images and orthoimages. Photogramm. Eng. Remote Sens. 2009, 75, 193–200. [Google Scholar] [CrossRef]
- López, D.H.; García, B.F.; Piqueras, J.G.; Alcázar, G.V. An approach to the radiometric aerotriangulation of photogrammetric images. ISPRS J. Photogramm. Remote Sens. 2011, 66, 883–893. [Google Scholar] [CrossRef]
- Falala, L.; Gachet, R.; Cunin, L. Radiometric block-adjustment of satellite images reference3D production line improvement. In Proceedings of the XXI ISPRS Congress, Beijing, China, 3–11 July 2008. [Google Scholar]
- Gehrke, S. Radiometric processing of ADS imagery: Mosaicking of large image blocks. In Proceedings of the ASPRS Annual Conference, San Diego, CA, USA, 26–30 April 2010. [Google Scholar]
- Honkavaara, E.; Saari, H.; Kaivosoja, J.; Pölönen, I.; Hakala, T.; Litkey, P.; Mäkynen, J.; Pesonen, L. Processing and assessment of spectrometric, stereoscopic imagery collected using a lightweight uav spectral camera for precision agriculture. Remote Sens. 2013, 5, 5006–5039. [Google Scholar] [CrossRef] [Green Version]
- Yan, W.; Shaker, A. Radiometric correction and normalization of airborne LIDAR intensity data for improving land-cover classification. IEEE Trans. Geosci. Remote Sens. 2014, 52, 7658–7673. [Google Scholar]
- Gehrke, S.; Beshah, B. Radiometric normalization of large airborne image data sets acquired by different sensor types. In Proceedings of the XXIII ISPRS Congress, Prague, Czech Republic, 12–19 July 2016. [Google Scholar]
- Pros, A.; Colomina, I.; Navarro, J.A.; Antequera, R.; Andrinal, P. Radiometric block adjustment and digital radiometric model generation. In Proceedings of the ISPRS Hannover Workshop 2013, Hannover, Germany, 21–24 May 2013. [Google Scholar]
- Teo, T.A.; Wu, H.M. Radiometric block adjustment for multi-strip airborne waveform lidar data. Remote Sens. 2015, 7, 16831–16848. [Google Scholar] [CrossRef]
- Chander, G.; Mishra, N.; Helder, D.L.; Aaron, D.B.; Angal, A.; Choi, T.; Xiong, X.; Doelling, D.R. Applications of spectral band adjustment factors (SBAF) for cross-calibration. IEEE Trans. Geosci. Remote Sens. 2013, 51, 1267–1281. [Google Scholar] [CrossRef]
- Lachérade, S.; Fougnie, B.; Henry, P.; Gamet, P. Cross calibration over desert sites: Description, methodology, and operational implementation. IEEE Trans. Geosci. Remote Sens. 2013, 51, 1098–1113. [Google Scholar] [CrossRef]
- Yang, A.; Zhong, B.; Lv, W.; Wu, S.; Liu, Q. Cross-calibration of GF-1/WFV over a desert site using Landsat-8/OLI imagery and ZY-3/TLC data. Remote Sens. 2015, 7, 10763–10787. [Google Scholar] [CrossRef]
- Teillet, P.M.; Fedosejevs, G.; Thome, K.J.; Barker, J.L. Impacts of spectral band difference effects on radiometric cross-calibration between satellite sensors in the solar-reflective spectral domain. Remote Sens. Environ. 2007, 110, 393–409. [Google Scholar] [CrossRef]
- Chander, G.; Helder, D.L.; Aaron, D.; Mishra, N.; Shrestha, A.K. Assessment of spectral, misregistration, and spatial uncertainties inherent in the cross-calibration study. IEEE Trans. Geosci. Remote Sens. 2013, 51, 1282–2196. [Google Scholar] [CrossRef]
- Gao, H.; Gu, X.; Yu, T.; Li, X.; Gong, H.; Li, J. Time-series calibration for ccd camera: Taking cbers02b satellite as an example. Acta Geodaeticaet Cartogr. Sin. 2011, 40, 180–187. [Google Scholar]
- Tong, X.; Liu, S.; Weng, Q. Bias-corrected rational polynomial coefficients for high accuracy geo-positioning of QuickBird stereo imagery. ISPRS J. Photogramm. Remote Sens. 2010, 65, 218–226. [Google Scholar] [CrossRef]
| No. | Date Since Launched/Day | GF-1 Camera | WFV Time | MODIS Time | △t/’ |
|---|---|---|---|---|---|
| 1 | 214 | WFV1 | 12:33 | 13:00 | 27 |
| 2 | 276 | WFV1 | 12:37 | 12:30 | 7 |
| 3 | 312 | WFV1 | 12:46 | 12:45 | 1 |
| 4 | 332 | WFV1 | 12:36 | 12:25 | 11 |
| 5 | 731 | WFV1 | 12:55 | 12:26 | 29 |
| 6 | 240 | WFV2 | 12:50 | 12:55 | 5 |
| 7 | 244 | WFV2 | 12:48 | 12:30 | 18 |
| 8 | 301 | WFV2 | 12:50 | 12:25 | 25 |
| 9 | 637 | WFV2 | 13:00 | 12:25 | 35 |
| 10 | 674 | WFV2 | 13:03 | 12:40 | 23 |
| 11 | 715 | WFV2 | 13:04 | 12:35 | 29 |
| 12 | 534 | WFV3 | 13:08 | 13:00 | 8 |
| 13 | 575 | WFV3 | 13:10 | 12:55 | 15 |
| 14 | 616 | WFV3 | 13:11 | 12:45 | 26 |
| 15 | 657 | WFV3 | 13:12 | 12:40 | 32 |
| 16 | 662 | WFV3 | 13:10 | 12:15 | 55 |
| 17 | 669 | WFV3 | 13:13 | 12:35 | 38 |
| 18 | 176 | WFV4 | 13:07 | 12:55 | 12 |
| 19 | 180 | WFV4 | 13:06 | 12:30 | 36 |
| 26 | 208 | WFV4 | 12:59 | 12:55 | 4 |
| Camera | Blue | Green | Red | NIR |
|---|---|---|---|---|
| WFV1 | 1.45% | −2.02% | −1.70% | −1.21% |
| WFV2 | 0.36% | −0.29% | −0.08% | −0.37% |
| WFV3 | −1.82% | −0.49% | −0.27% | 0.72% |
| WFV4 | −1.40% | −0.21% | 0.83% | 0.85% |
| Parameters | Value |
|---|---|
| Sun zenith angle | 30° |
| Sun azimuth angle | 150° |
| Satellite zenith angle | 17° for WFV1 and WFV2, 0° for WFV2 and WFV3, 17° for WFV3 and WFV4 |
| Satellite azimuth angle | 102° for WFV1 and WFV2, 193° for WFV2 and WFV3, 284° for WFV3 and WFV4 |
| Atmospheric conditions | Mid-latitude Summer |
| Aerosol model | Continent |
| 550-nm AOT | 0.15 |
| Altitude | 1200 m |
| Surface profiles | 50 different spectral profiles from the USGS spectral library |
| Band | WFV1 | WFV2 | WFV3 | WFV4 | ||||
|---|---|---|---|---|---|---|---|---|
| Gain | Offset | Gain | Offset | Gain | Offset | Gain | Offset | |
| 1 | 0.1723 | 3.9090 | 0.1699 | 6.4417 | 0.1725 | 6.1388 | 0.1740 | 3.4047 |
| 2 | 0.1442 | 0.4192 | 0.1414 | 1.6595 | 0.1581 | 2.5134 | 0.1598 | -0.2751 |
| 3 | 0.1239 | -0.3238 | 0.1211 | 0.2630 | 0.1345 | 0.9027 | 0.1353 | 1.6649 |
| 4 | 0.1359 | 2.2127 | 0.1334 | 2.4679 | 0.1373 | 2.8715 | 0.1340 | 2.7709 |
| Camera | RE/% | |||
|---|---|---|---|---|
| Band 1 | Band 2 | Band 3 | Band 4 | |
| WFV1 | 6.61 | 7.02 | 4.45 | 6.82 |
| WFV2 | 7.91 | 4.91 | 5.16 | 7.61 |
| WFV3 | 4.39 | 3.43 | 4.84 | 4.02 |
| WFV4 | 6.50 | 4.84 | 6.67 | 5.76 |
| Overlaps | Results | Average Absolute Value of Difference (W·m−2·sr−1·μm−1) | |||
|---|---|---|---|---|---|
| Band 1 | Band 2 | Band 3 | Band 4 | ||
| WFV1 and WFV2 | 2013 OCCs results | 3.08 | 7.10 | 11.79 | 14.75 |
| 2014 OCCs results | 16.45 | 15.02 | 6.53 | 9.09 | |
| PMCs results | 1.12 | 1.06 | 1.04 | 1.34 | |
| WFV2 and WFV3 | 2013 OCCs results | 5.56 | 5.00 | 7.32 | 6.35 |
| 2014 OCCs results | 1.30 | 2.82 | 1.80 | 1.44 | |
| PMCs results | 1.39 | 1.43 | 1.76 | 1.50 | |
| WFV3 and WFV4 | 2013 OCCs results | 6.05 | 1.47 | 2.36 | 4.46 |
| 2014 OCCs results | 1.17 | 6.08 | 10.61 | 0.93 | |
| PMCs results | 0.80 | 0.93 | 0.64 | 0.71 | |
| Camera | Band 1 | Band 2 | Band 3 | Band 4 | |
|---|---|---|---|---|---|
| WFV1 | Gain/W·m−2·sr−1·μm−1 | 0.1918 | 0.1624 | 0.1314 | 0.1510 |
| Offset/W·m−2·sr−1·μm−1 | −6.1834 | −10.907 | −7.0128 | −5.3534 | |
| RE/% | 6.59 | 5.66 | 6.14 | 5.32 | |
| WFV2 | Gain/W·m−2·sr−1·μm−1 | 0.1833 | 0.1512 | 0.1240 | 0.1454 |
| Offset/W·m−2·sr−1·μm−1 | 1.6673 | −1.4125 | −0.9477 | −1.5686 | |
| RE/% | 6.13 | 4.26 | 4.89 | 7.98 | |
| WFV3 | Gain/W·m−2·sr−1·μm−1 | 0.1904 | 0.1729 | 0.1444 | 0.1569 |
| Offset/W·m−2·sr−1·μm−1 | −0.8263 | −3.5004 | −2.6111 | −3.2374 | |
| RE/% | 5.84 | 3.29 | 3.96 | 3.25 | |
| WFV4 | Gain/W·m−2·sr−1·μm−1 | 0.1634 | 0.1503 | 0.1327 | 0.1394 |
| Offset/W·m−2·sr−1·μm−1 | 7.3767 | 3.9719 | 4.9127 | 2.7868 | |
| RE/% | 6.22 | 4.24 | 5.43 | 4.61 |
| Overlaps | Average Absolute Value of Difference (W·m−2·sr−1·μm−1) | |||
|---|---|---|---|---|
| Band 1 | Band 2 | Band 3 | Band 4 | |
| WFV1 and WFV2 | 1.68 | 3.82 | 2.95 | 2.12 |
| WFV2 and WFV3 | 1.35 | 2.63 | 2.27 | 1.94 |
| WFV3 and WFV4 | 4.00 | 3.71 | 3.00 | 2.77 |
| RCPs Position | Band | RE/% | |||
|---|---|---|---|---|---|
| WFV1 | WFV2 | WFV3 | WFV4 | ||
| WFV1 | 1 | 8.39 | 8.06 | 4.35 | 4.48 |
| 2 | 8.78 | 6.25 | 6.18 | 3.89 | |
| 3 | 4.89 | 5.45 | 6.21 | 7.32 | |
| 4 | 14.95 | 15.52 | 36.03 | 7.52 | |
| WFV1 and WFV2 | 1 | 6.95 | 7.09 | 4.00 | 5.63 |
| 2 | 8.07 | 5.51 | 5.20 | 3.96 | |
| 3 | 4.6 | 5.16 | 5.62 | 6.94 | |
| 4 | 10.89 | 10.5 | 28.35 | 6.22 | |
| WFV1, WFV2 and WFV3 | 1 | 6.35 | 6.78 | 4.22 | 6.30 |
| 2 | 7.02 | 4.64 | 4.05 | 4.49 | |
| 3 | 4.48 | 5.11 | 5.52 | 6.67 | |
| 4 | 10.40 | 9.66 | 23.84 | 6.22 | |
| WFV1, WFV2, WFV3 and WFV4 | 1 | 6.61 | 7.02 | 4.45 | 6.28 |
| 2 | 7.91 | 4.91 | 5.16 | 7.61 | |
| 3 | 4.39 | 3.43 | 4.84 | 4.02 | |
| 4 | 6.50 | 4.84 | 6.67 | 5.76 | |
| RCPs Position | Band | Average Absolute Value of Difference (W·m−2·sr−1·μm−1) | ||
|---|---|---|---|---|
| WFV1 and WFV2 | WFV2 and WFV3 | WFV3 and WFV4 | ||
| WFV1 | 1 | 1.06 | 1.39 | 0.77 |
| 2 | 0.94 | 1.38 | 0.91 | |
| 3 | 0.76 | 1.75 | 0.63 | |
| 4 | 0.95 | 1.33 | 0.61 | |
| WFV1 and WFV2 | 1 | 1.08 | 1.46 | 0.81 |
| 2 | 0.97 | 1.43 | 0.95 | |
| 3 | 0.77 | 1.77 | 0.64 | |
| 4 | 1.01 | 1.49 | 0.69 | |
| WFV1, WFV2 and WFV3 | 1 | 1.14 | 1.47 | 0.82 |
| 2 | 0.99 | 1.45 | 0.96 | |
| 3 | 0.80 | 1.77 | 0.64 | |
| 4 | 1.21 | 1.50 | 0.70 | |
| WFV1, WFV2, WFV3 and WFV4 | 1 | 1.12 | 1.39 | 0.80 |
| 2 | 1.06 | 1.43 | 0.93 | |
| 3 | 1.04 | 1.76 | 0.64 | |
| 4 | 1.34 | 1.50 | 0.71 | |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, Y.; Han, J.; Gu, X.; Liu, Q. On-Orbit Radiometric Calibration for a Space-Borne Multi-Camera Mosaic Imaging Sensor. Remote Sens. 2017, 9, 1248. https://doi.org/10.3390/rs9121248
Xie Y, Han J, Gu X, Liu Q. On-Orbit Radiometric Calibration for a Space-Borne Multi-Camera Mosaic Imaging Sensor. Remote Sensing. 2017; 9(12):1248. https://doi.org/10.3390/rs9121248
Chicago/Turabian StyleXie, Yong, Jie Han, Xingfa Gu, and Qiyue Liu. 2017. "On-Orbit Radiometric Calibration for a Space-Borne Multi-Camera Mosaic Imaging Sensor" Remote Sensing 9, no. 12: 1248. https://doi.org/10.3390/rs9121248
APA StyleXie, Y., Han, J., Gu, X., & Liu, Q. (2017). On-Orbit Radiometric Calibration for a Space-Borne Multi-Camera Mosaic Imaging Sensor. Remote Sensing, 9(12), 1248. https://doi.org/10.3390/rs9121248





