Application of Quasi-Continuous Waveform Coding in Spaceborne Synthetic Aperture Radar
Abstract
1. Introduction
2. Design for System Model
2.1. Principle
2.2. The Monopulse Group Model with Reduced Dimensionality under Approximate Conditions
3. Simulation and Experiment
3.1. Validation of Point Target Simulations
3.2. Testing and Evaluation of Pulse Coding and Reconstruction
4. Error Analysis
4.1. Ambiguity Analysis of Reconstruction
- 1.
- Sampling according to the code scheme;
- 2.
- Calculating the reconstruction matrix by the code to reconstruct the echo;
- 3.
- Imaging with the reconstructed echo.
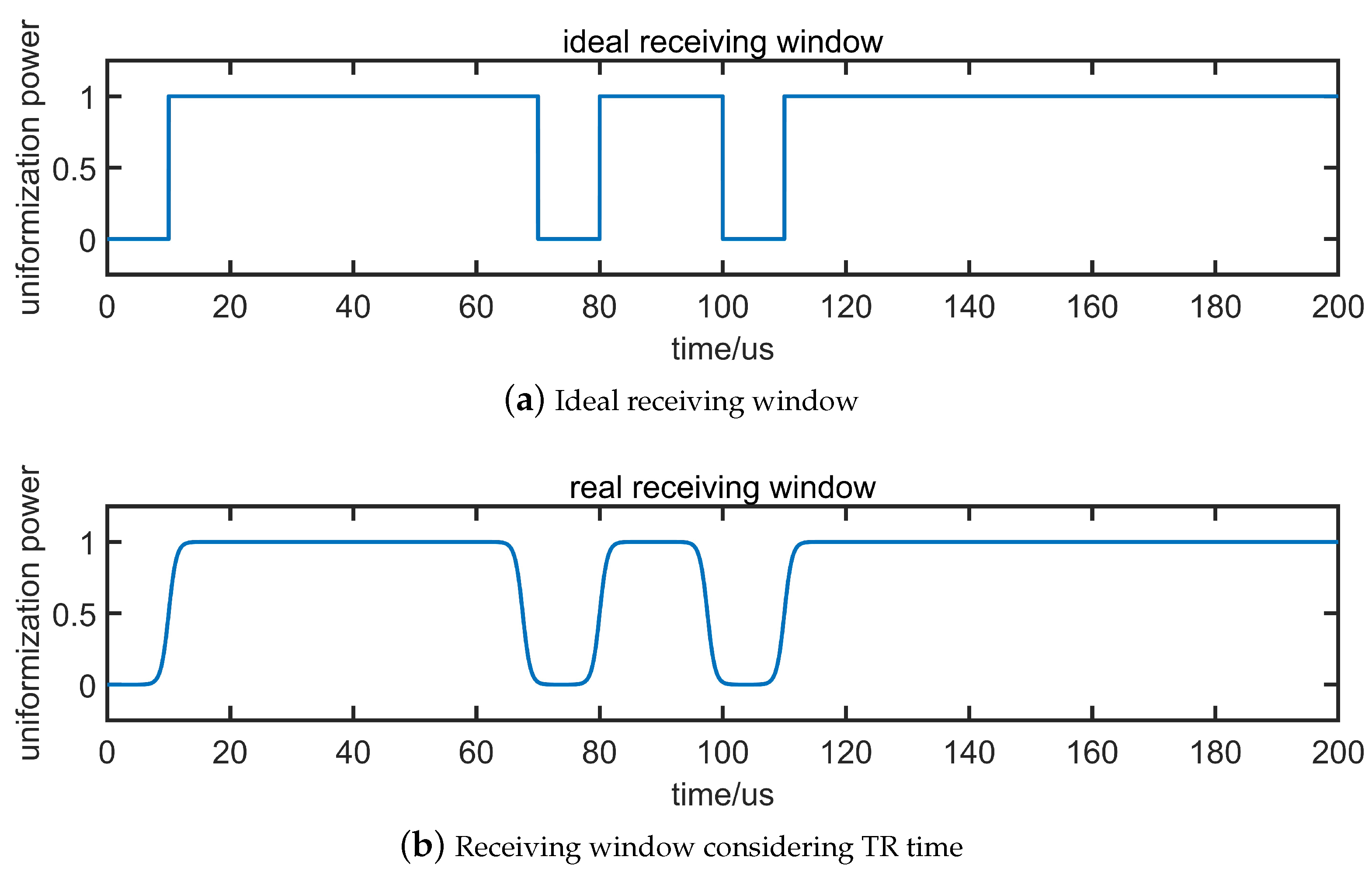
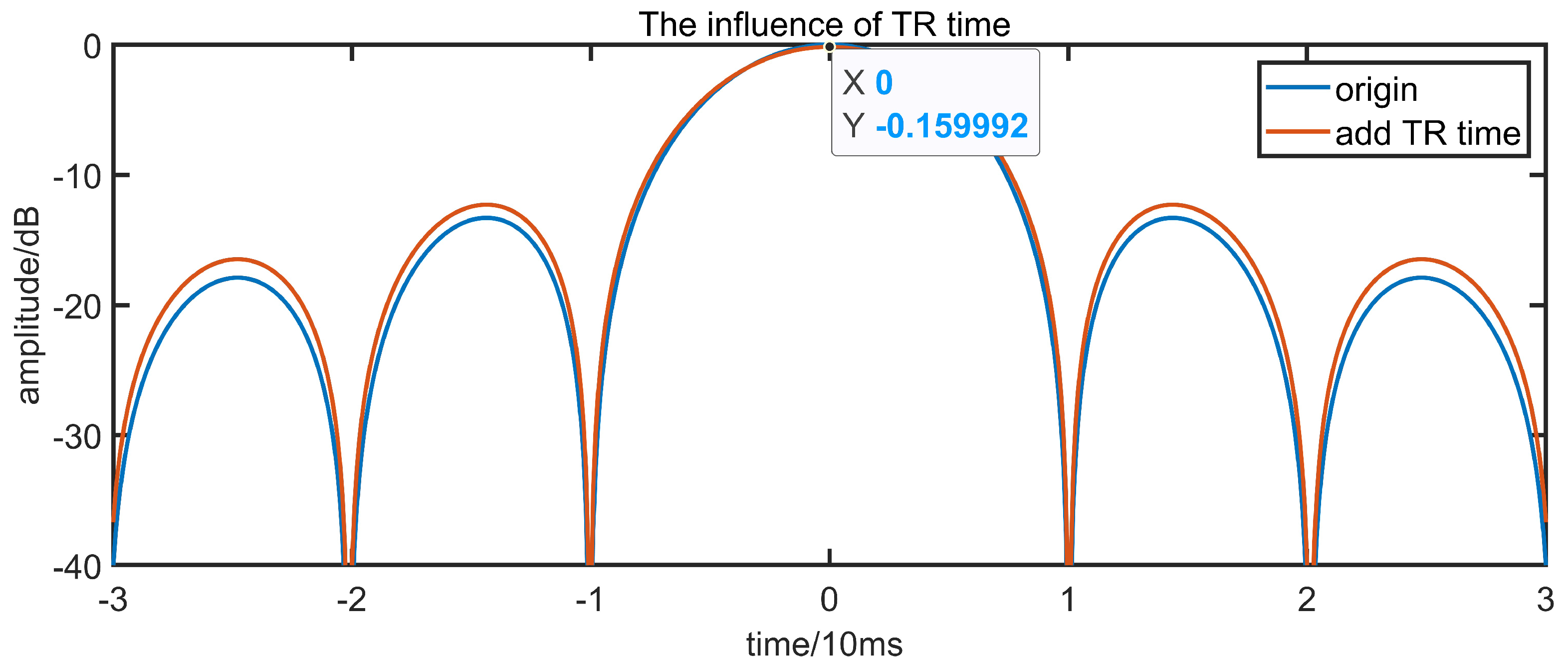
4.2. Transmit–Receive Conversion Time
5. Coding Scheme Design
- (1)
- Calculate by beam position design.
- (2)
- Determine the echo window width under the constraint of design and obtain a sum of the maximum subpulse widths in .
- (3)
- Determine an encoding length L and divide equally into L parts.
- (4)
- If there are M subpulses in total, the length of the receiving window is .
- (5)
- Evaluate the resulting construction matrix to see if it is full rank.
- (6)
- Verify the recovery effects of our coding and evaluate its performance.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| SAR | Synthetic aperture radar |
| SNR | Signal-to-noise ratio |
| HRWS | High-resolution wide swath |
| TR | Transmit–receive |
References
- Wang, Y.; Li, H.; Han, S. The theory and method of pulse coding for radar and its applications. J. Radars 2019, 8, 1–16. [Google Scholar]
- Wang, Y.; Liu, C.; Zhan, X.; Han, S. Technology and applications of UAV synthetic aperture radar system. J. Radars 2016, 5, 333–349. [Google Scholar]
- Khan, R.H.; Mitchel, D.K. Wave analysis for high frequency FMICW radar. IEEE Process.-F 1991, 138, 411–419. [Google Scholar]
- Yuan, S.; Chen, G.; Li, G. The digital signal processing system of quasi-continuous wave radar. J. Inst. Command. Technol. 2002, 13, 67–69. [Google Scholar]
- Zeng, T.; Long, T.; Wang, H. The design and realization of quasi-continuous tracking radar signal processor. J. Beijing Inst. Technol. 1999, 19, 604–606. [Google Scholar]
- Gao, B.; Ye, Z. The algorithm selection of maximum entropy spectral estimation based on quasi-continuous wave radar. Electron. Warf. Technol. 2003, 18, 26–31. [Google Scholar]
- Kovregin, V.N.; Kovregina, G.M.; Murzaev, A.S. Method for Detection/Identification of Several Air Objects with a Complex Spectrum in Radars with Quasi-Continuous Chirp Radiation. In Proceedings of the 2022 Wave Electronics and its Application in Information and Telecommunication Systems (WECONF), St. Petersburg, Russia, 30 May–3 June 2022; pp. 1–5. [Google Scholar]
- Kalenichenko, S.P.; Mettus, L.; Veremjev, I. The opportunity of applying a complex modulation laws radar signals for remote sensing low atmosphere and water surface by ground-based radar. In Proceedings of the Fifth International Conference on Remote Sensing of the Marine and Coastal Environments, San Diego, CA, USA, 5–7 October 1998; pp. 1–3. [Google Scholar]
- Barkhatov, A.V.; Kalenichenko, S.P.; Kutuzov, V.M. Multiband radar complex for remote monitoring of water areas. J. Min. Inst. 2001, 149, 244–247. [Google Scholar]
- Toutin, T. Impact of RADARSAT-2 SAR ultrafine-mode parameters on stereo-radargrammetric DEMs. IEEE Trans. Geosci. Remote Sens. 2010, 48, 3816–3823. [Google Scholar] [CrossRef]
- Mcgregor, J.A.; Poulter, E.M.; Smith, M.J. Switching system for single antenna operation of an S-band radar. IEEE Proc-Radar Sonar Navig. 1994, 1, 141–144. [Google Scholar] [CrossRef]
- Yuan, W. Study on the design method of quasi-CW radar waveform. In Proceedings of the CIE International Conference on Radar, Shanghai, China, 16–19 October 2006; pp. 1–4. [Google Scholar]
- Yuan, W. Study on the Design Method of a Novel Quasi-CW Radar Waveform. Morden Radar 2007, 29, 16–19. [Google Scholar]
- Su, F.; Gao, M.; Tian, L. Research on eclipse problem of quasi-CW phase-coded radar and solutions. Acta Armamentarii 2009, 30, 714–718. [Google Scholar]
- Wang, H.; Zhang, J.; Li, Y.; Zhu, X. Anti-range eclipse waveform optimization method for quasi-CW radar. J. Data Acquis. Process. 2011, 26, 531–535. [Google Scholar]
- Zeng, W.; Sun, Y.; Xu, H.; Pan, L. Waveform design and parameters calculation for quasi-continuous wave radar system. Syst. Eng. Electron. 2013, 35, 517–521. [Google Scholar]
- Gu, H.; Li, X.; Shang, W. A new approach of periodic square wave interruption to solution of the leakage of CW radar. Acta Electron. Sin. 1998, 26, 7–11. [Google Scholar]
- Peng, W.; Wang, X.; Zhao, J. Methods of eliminating Doppler dispersion in synthetic wideband signal. In Proceedings of the International Conference on Microwave and Millimeter Wave Technology, Nanjing, China, 21–24 April 2008; pp. 1540–1543. [Google Scholar]
- Li, B.; Zhou, S.; Stojanovie, M.; Freitag, L.; Willett, P. Multicarrier communication over underwater acoustic channels with nonuniform Doppler shifts. IEEE J. Ocean. Eng. 2008, 33, 198–209. [Google Scholar]
- Zeng, X.; Liu, X.; Bai, J.; Zhang, Y.S. Study of space borne SAR multidimensional waveform encoding technology based on azimuth multi-beams multi-phase centers. Acta Electron. Sin. 2013, 41, 1863–1868. [Google Scholar]
- Xu, W.; Deng, Y. Multi-channel SAR with reflector antenna for highresolution wide-swath imaging. IEEE Antennas Wirel. Propag. Lett. 2010, 9, 1123–1127. [Google Scholar] [CrossRef]
- Liang, D.; He, F.; Dong, Z. A Novel Space-Time Coding Alamouti Waveform Scheme for MIMO-SAR Implementation. IEEE Geosci. Remote Sens. Lett. 2015, 12, 229–233. [Google Scholar]
- He, F.; Zhang, Y.; Sun, Z.; Jin, G.; Dong, Z. Performance investigation on elevation cascaded digital beamforming for multidimensional waveform encoding SAR imaging. J. Radars 2020, 9, 828–855. [Google Scholar]
- Villano, M.; Krieger, G. Staggered SAR: High-Resolution Wide-Swath Imaging by Continuous PRI Variation. IEEE Trans. Geosci. Remote Sens. 2014, 52, 4462–4479. [Google Scholar] [CrossRef]
- Shao, W.; Huang, H. Study on Improving Imaging Quality of Spaceborne SAR by Pulse Coding Technology. In Proceedings of the International Academic Exchange Conference on Science and Technology Innovation, Chongqing, China, 8–9 December 2022; pp. 771–775. [Google Scholar]
- Bordoni, F.; Younis, M.; Krieger, G. Ambiguity Suppression by Azimuth Phase Coding in Multichannel SAR Systems. IEEE Trans. Geosci. Remote Sens. 2012, 50, 617–629. [Google Scholar] [CrossRef]
- Liu, J.; Xu, S.; Gao, X.; Li, X.; Zhuang, Z.W. A review of radar imaging technique based on compressed sensing. IEEE Signal Process. 2011, 27, 251–260. [Google Scholar]
- Basit, A.; Khan, W.; Khan, S.; Qureshi, I. Development of frequency diverse array radar technology: A review. IET Radar Sonar Navig. 2018, 12, 165–175. [Google Scholar] [CrossRef]
- Zhao, Q.; Zhang, Y.; Wang, W.; Deng, Y.; Yu, W.; Zhou, Y.; Wang, R. Echo separation for space-time waveform-encoding SAR with digital scalloped beamforming and adaptive multiple null-steering. IEEE Geosci. Remote Sens. Lett. 2020, 18, 92–96. [Google Scholar] [CrossRef]
- Zhao, Q.; Zhang, Y.; Wang, W.; Liu, K.; Deng, Y.; Zhang, H.; Wang, Y.; Zhou, Y.; Wang, R. On the frequency dispersion in DBF SAR and digital scalloped beamforming. IEEE Trans. Geosci. Remote Sens. 2020, 58, 3619–3632. [Google Scholar] [CrossRef]
- Ding, L.; Geng, F. Principle of Radar, 3rd ed.; Xidian University Press: Xi’an, China, 2002; pp. 1–22. [Google Scholar]
- Chen, S.; Wang, X.; Sato, M. PolInSAR complex coherence estimation based on covariance matrix similarity test. IEEE Trans. Geosci. Remote Sens. 2012, 50, 4699–4710. [Google Scholar] [CrossRef]
- Touzi, R.; Lopes, A.; Bousquet, P. A statistical and geometrical edge detector for SAR images. IEEE Trans. Geosci. Remote Sens. 1988, 26, 764–773. [Google Scholar] [CrossRef]
- Shen, P.; Wang, C. HoMeNL: A Homogeneity Measure-Based NonLocal Filtering Framework for Detail-Enhanced (Pol)(In)SAR Image Denoising. ISPRS J. Photogramm. Remote Sens. 2023, 197, 212–227. [Google Scholar] [CrossRef]
- Lan, L.; Liao, G. SAR Range Ambiguity Resolution Based on Element-Pulse-Coding. Radio Eng. 2021, 51, 675–680. [Google Scholar]
- Wang, C.; Xu, J.; Liao, G. A Range Ambiguity Resolution Approach for High-Resolution and Wide-Swath SAR Imaging Using Frequency Diverse Array. IEEE J. Sel. Top. Signal Process. 2017, 11, 336–346. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Y.; Liang, J.; Wang, Y. An Improved Omega-K Algorithm for Squinted SAR with Curved Trajectory. IEEE Geosci. Remote Sens. Lett. 2023, 21, 1–5. [Google Scholar] [CrossRef]
- Li, Y.; Huo, T.; Cao, C. An Efficient Imaging Method for Medium-Earth-Orbit Multichannel SAR-GMTI Systems. Remote Sens. 2022, 14, 5453. [Google Scholar] [CrossRef]


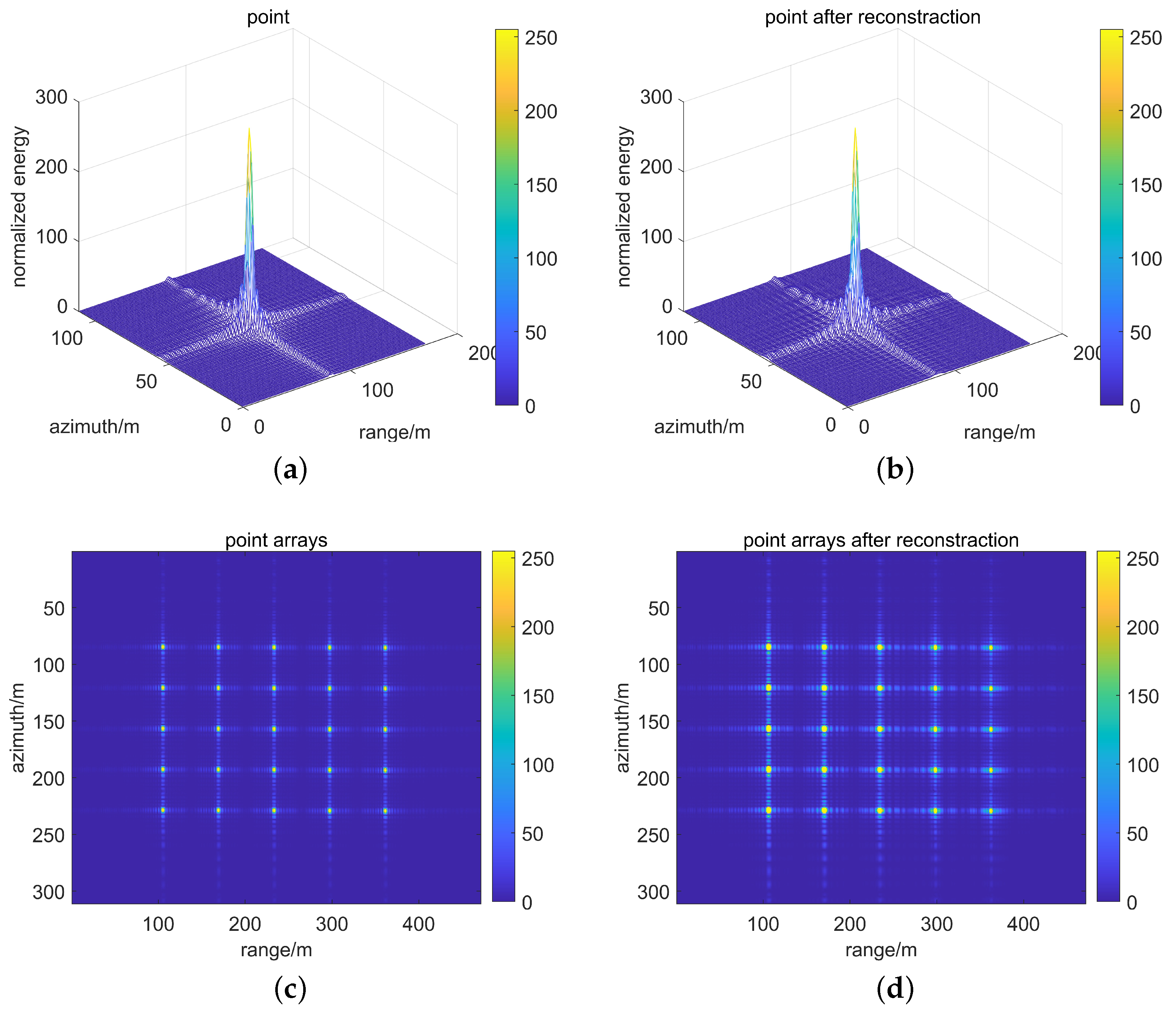
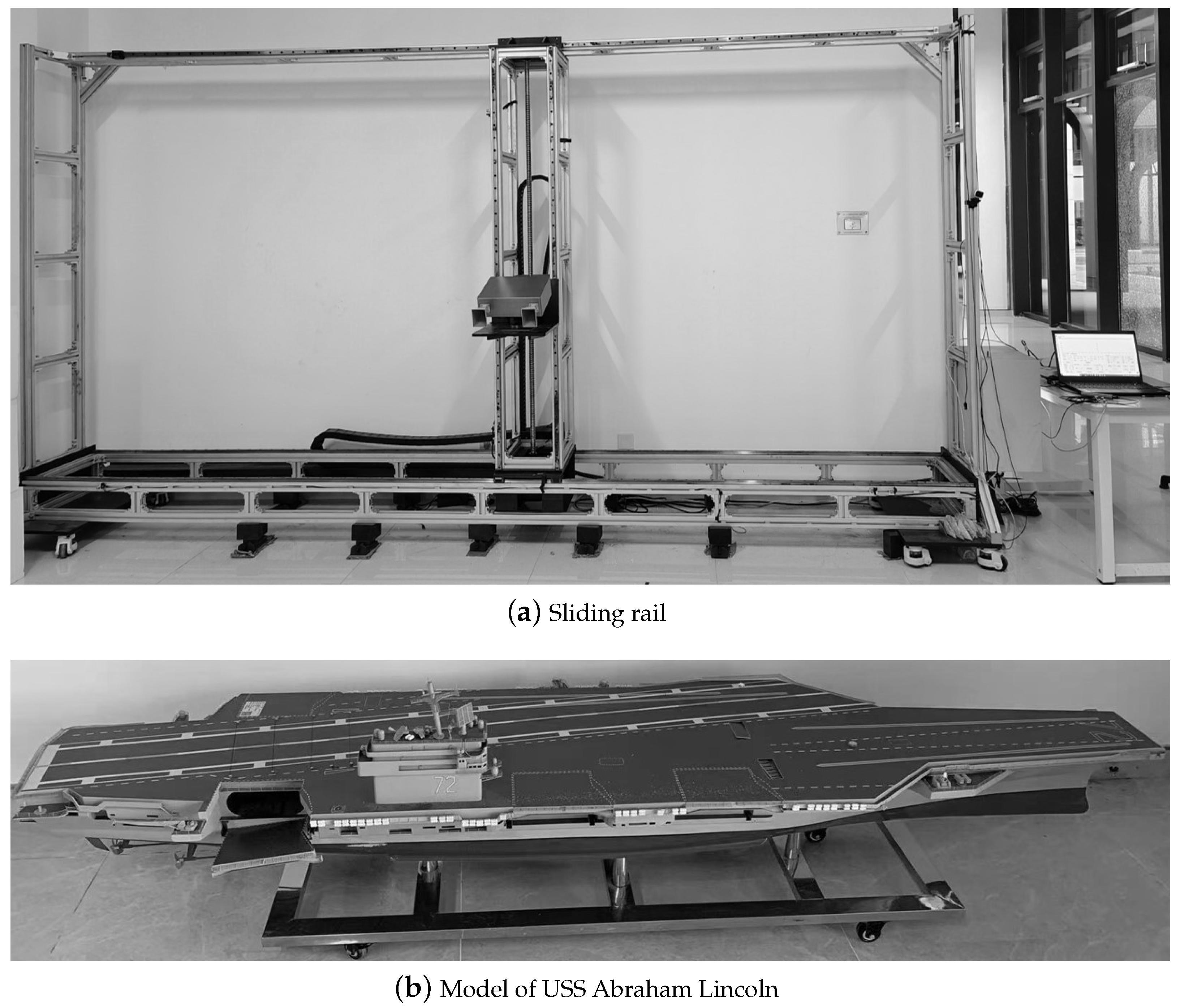
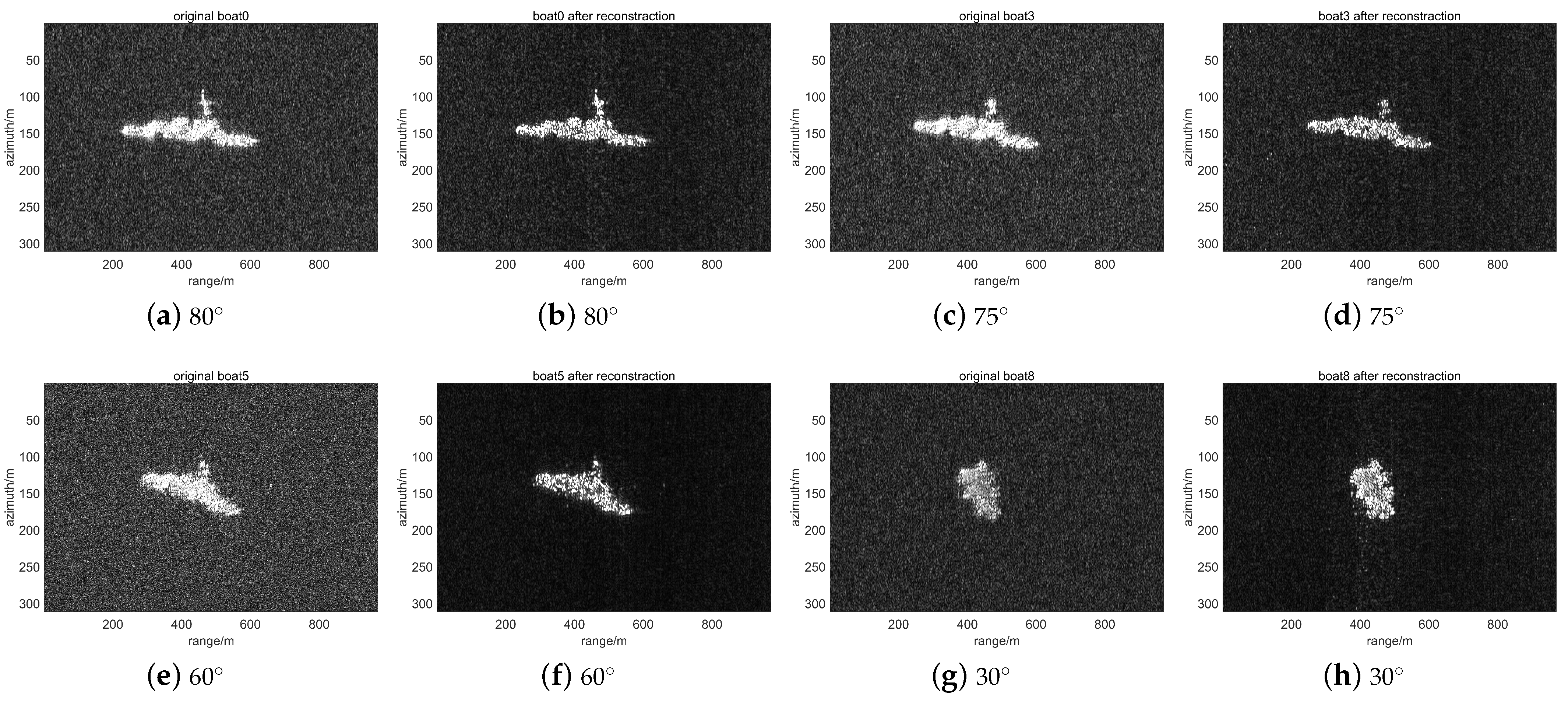
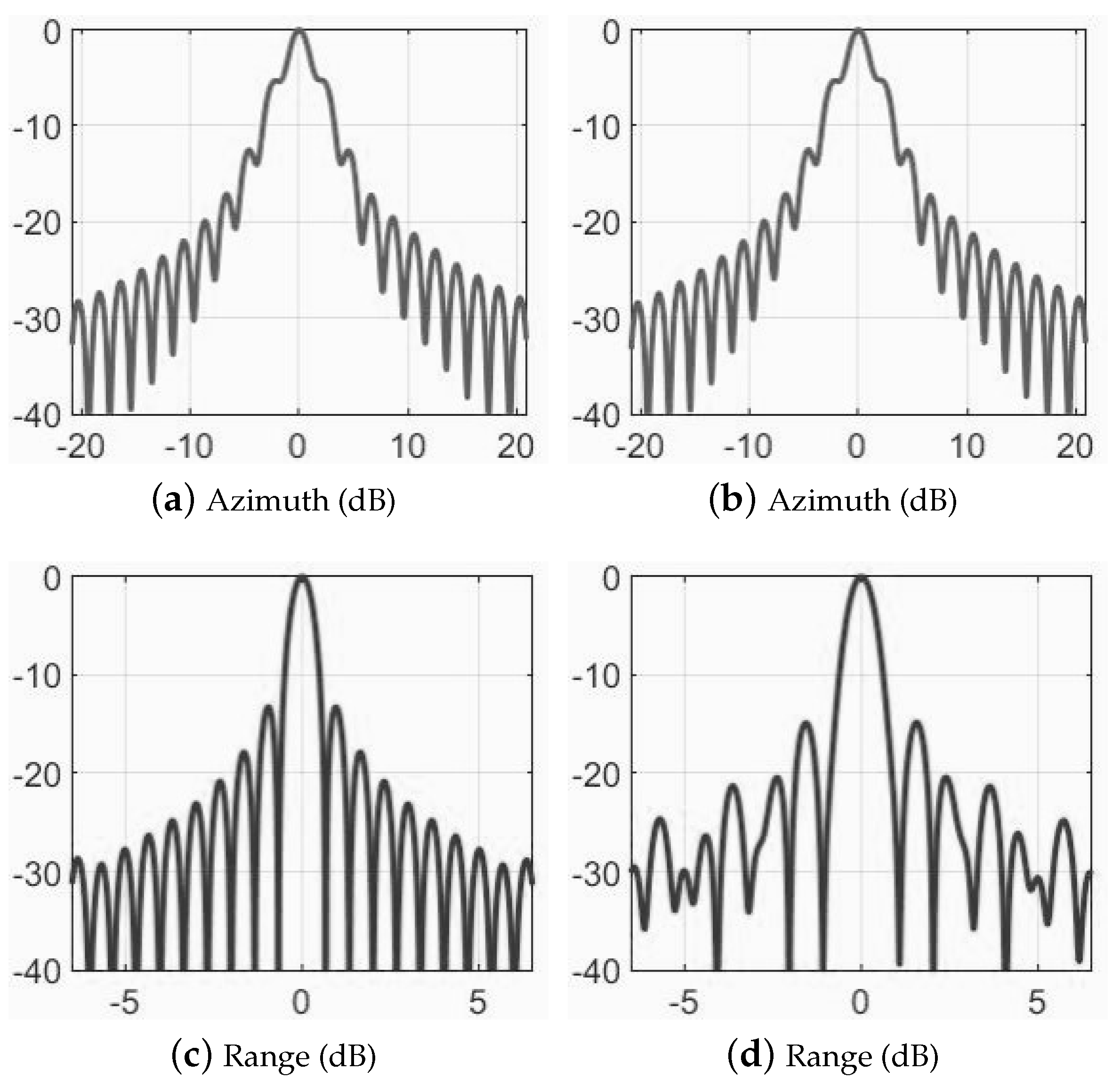
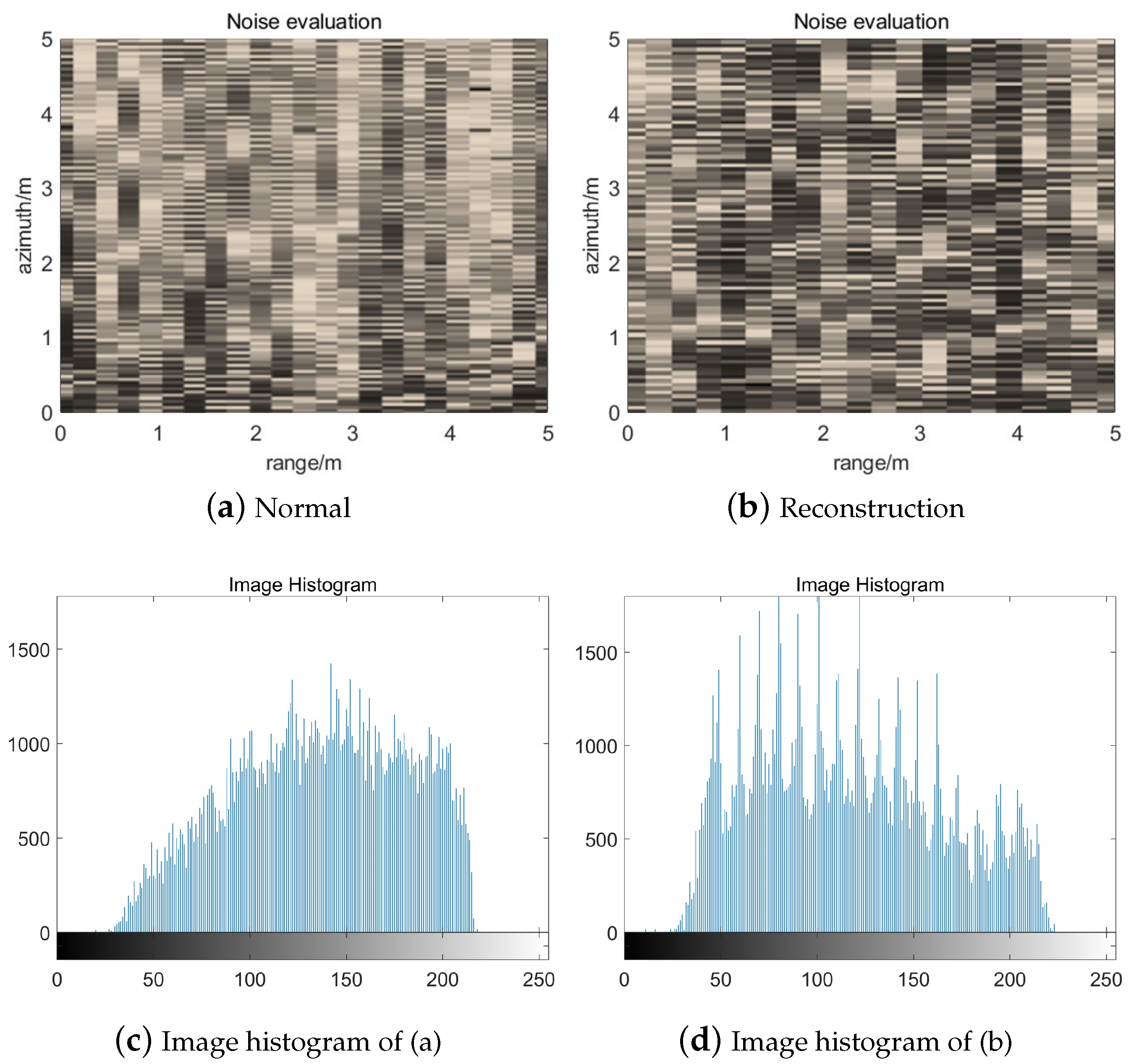
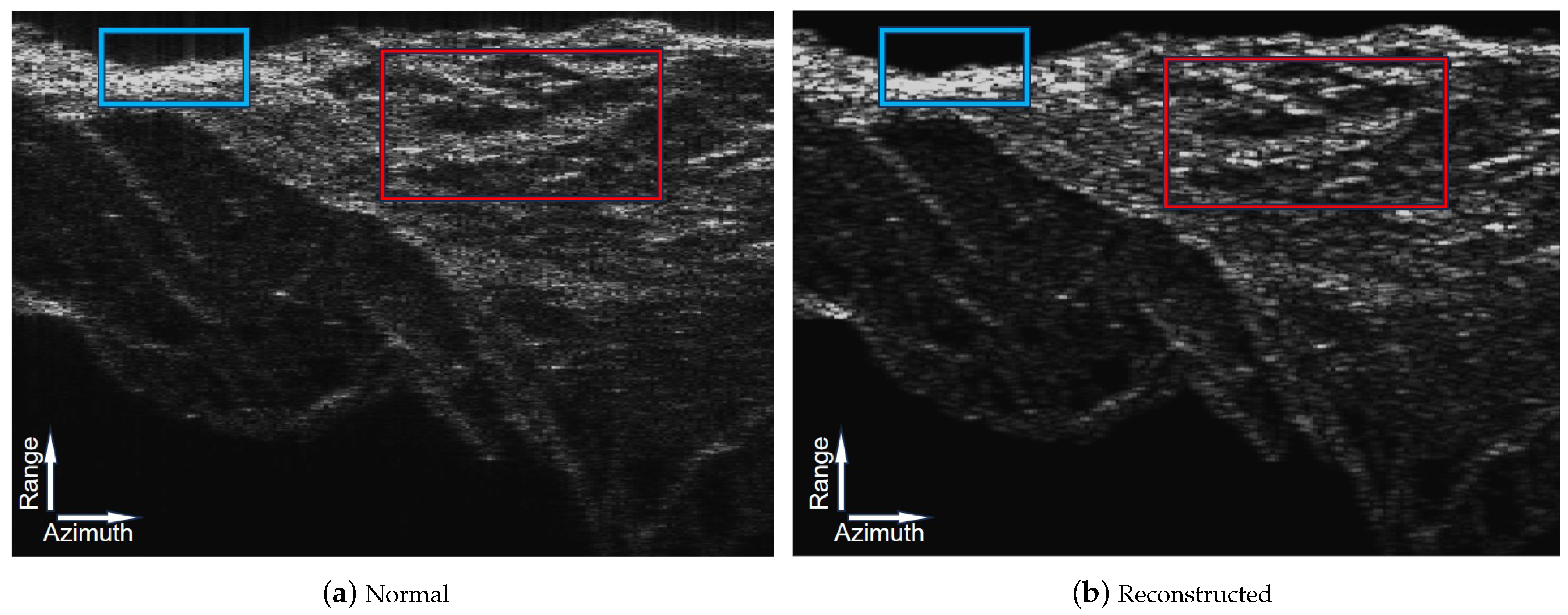


| Parameter | Value |
|---|---|
| Orbit height | 500 (km) |
| Right ascension of ascending node | 347.0438 () |
| Orbit inclination | 97.4334 () |
| Argument of perigee | 270 () |
| True anomaly | 359.8414 () |
| Duty cycle | 10% |
| eccentricity ratio | 0.11% |
| Carrier freq | 9.6 (GHz) |
| Azimuth resolution | 2 (m) |
| Signal bandwidth | 300 (MHz) |
| PRF | 4300 (Hz) |
| Pulse width | 23 (us) |
| Wave gate width | 50 (us) |
| Signal bandwidth | 300 (MHz) |
| Parameter | Origin | Reconstructed |
|---|---|---|
| Peak | (dB) | (dB) |
| Phase | −127.6212 | −127.6212 |
| A resolution | 1.7182 (m) | 1.7709 (m) |
| R resolution | 0.5895 (m) | 0.5824 (m) |
| PSLR (Azimuth) | −11.9538 (dB) | −11.9689 (dB) |
| PSLR (Range) | −13.2694 (dB) | −14.8749 (dB) |
| ISLR (Azimuth) | −4.2717 (dB) | −4.2679 (dB) |
| ISLR (Range) | −9.9110 (dB) | −10.3557 (dB) |
| Expansion factor | 0.9979 | 1.1864 |
| Parameter | Origin | Reconstructed |
|---|---|---|
| Range dimension | 4.837 (m) | 4.837 (m) |
| Azimuth dimension | 4.787 (m) | 4.787 (m) |
| Maximum value | ||
| Minimum value | ||
| Dynamic range | 48.94 (dB) | 51.89 (dB) |
| Mean | ||
| Variance | ||
| ENL | 0.734 | 0.943 (dB) |
| Radiation resolution | 3.359 (dB) | 3.074 (dB) |
| Beam Position | s1 | s2 | s3 | s4 | s5 | s6 | s7 |
|---|---|---|---|---|---|---|---|
| PRF (Hz) | 4285 | 4265 | 4265 | 4365 | 4315 | 4265 | 4265 |
| Pulse width (us) | 12 | 12 | 12 | 12 | 12 | 12 | 12 |
| Bandwidth (MHz) | 225 | 225 | 225 | 180 | 180 | 180 | 180 |
| Angle of incidence (°) | 25 | 26.35 | 27.67 | 30.23 | 31.47 | 32.68 | 33.86 |
| Gate start time (us) | 3752.0 | 3782.4 | 3825.1 | 3913.4 | 3958.1 | 4004.4 | 4052.4 |
| Gate end time (us) | 3813.4 | 3843.8 | 3886.5 | 3995.3 | 4040.0 | 4086.3 | 4134.3 |
| Gate width (us) | 61.44 | 61.44 | 61.44 | 81.92 | 81.92 | 81.92 | 81.92 |
| Sampling rate (MHz) | 400 | 400 | 400 | 200 | 200 | 200 | 200 |
| Duty ratio | 5.1% | 5.1% | 5.1% | 5.2% | 5.2% | 5.1% | 5.1% |
| Beam Position | s1 | s2 | s3 | s4 | s5 | s6 | s7 |
|---|---|---|---|---|---|---|---|
| PRT length | 24 | 24 | 24 | 24 | 24 | 24 | 24 |
| Subpulse width (us) | 9.7238 | 9.7694 | 9.7694 | 9.5456 | 9.6562 | 9.7694 | 9.7694 |
| Launches | 4 | 4 | 4 | 4 | 4 | 4 | 3 |
| Receving windows | 20 | 20 | 20 | 20 | 20 | 20 | 21 |
| Gate width (us) | 61.44 | 61.44 | 61.44 | 81.92 | 81.92 | 81.92 | 81.92 |
| Equivalent duty ratio | 16.6667% | 16.6667% | 16.6667% | 16.6667% | 16.6667% | 16.6667% | 12.5% |
| Coding scheme | |||||||
| Duty ratio | 5.142% | 5.118% | 5.118% | 5.238% | 5.178% | 5.118% | 5.118% |
| Beam Position | The Position of the Echo Window to Be Recovered and Its Corresponding Gain (dB) | Ambiguity (dB) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| s1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | −21.62 | ||
| 4.2184 | 3.127 | 4.7293 | 4.5707 | 3.127 | 3.4799 | 3.2156 | 4.1624 | ||||
| s2 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | −21.63 | |||
| 4.3641 | 4.3455 | 3.9903 | 3.6409 | 3.0166 | 4.3641 | 3.3962 | |||||
| s3 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | −21.63 | |||
| 4.3768 | 3.4717 | 3.015 | 4.4194 | 4.2929 | 4.4877 | 3.4603 | |||||
| s4 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | −22.54 |
| 3.1814 | 2.6767 | 3.7566 | 2.8474 | 3.8674 | 2.3702 | 2.9048 | 2.3778 | 2.5404 | 3.4039 | ||
| s5 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | −22.04 |
| 3.1785 | 2.0843 | 2.3568 | 2.0641 | 3.4966 | 2.0329 | 3.1575 | 2.1889 | 3.6534 | 3.5992 | ||
| s6 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | −21.51 |
| 3.9872 | 2.7762 | 4.0583 | 2.1836 | 2.6545 | 4.1102 | 3.9159 | 2.7916 | 2.4033 | 2.9579 | ||
| s7 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | −21.45 |
| 3.2979 | 3.2979 | 3.3661 | 3.3661 | 2.7864 | 2.7864 | 3.3661 | 3.3661 | 3.2979 | 3.2979 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shao, W.; Lai, T.; Huang, H.; Wang, Q.; Shen, P. Application of Quasi-Continuous Waveform Coding in Spaceborne Synthetic Aperture Radar. Remote Sens. 2024, 16, 348. https://doi.org/10.3390/rs16020348
Shao W, Lai T, Huang H, Wang Q, Shen P. Application of Quasi-Continuous Waveform Coding in Spaceborne Synthetic Aperture Radar. Remote Sensing. 2024; 16(2):348. https://doi.org/10.3390/rs16020348
Chicago/Turabian StyleShao, Weizheng, Tao Lai, Haifeng Huang, Qingsong Wang, and Peng Shen. 2024. "Application of Quasi-Continuous Waveform Coding in Spaceborne Synthetic Aperture Radar" Remote Sensing 16, no. 2: 348. https://doi.org/10.3390/rs16020348
APA StyleShao, W., Lai, T., Huang, H., Wang, Q., & Shen, P. (2024). Application of Quasi-Continuous Waveform Coding in Spaceborne Synthetic Aperture Radar. Remote Sensing, 16(2), 348. https://doi.org/10.3390/rs16020348






