Robust Underwater Direction-of-Arrival Estimation Method Using Acoustic Sensor Array under Unknown Swing Deviation Elements
Abstract
1. Introduction
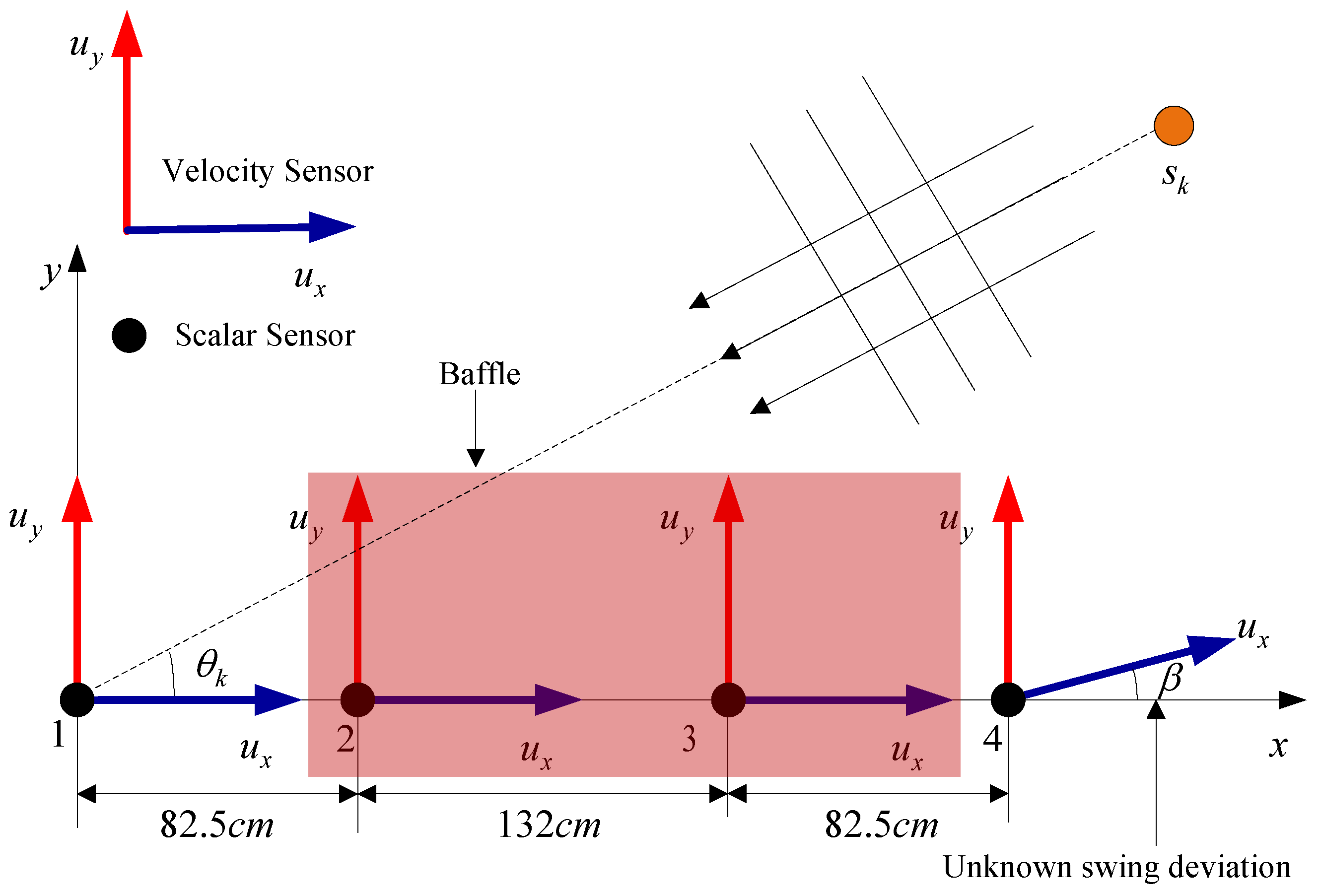
2. Problem Description
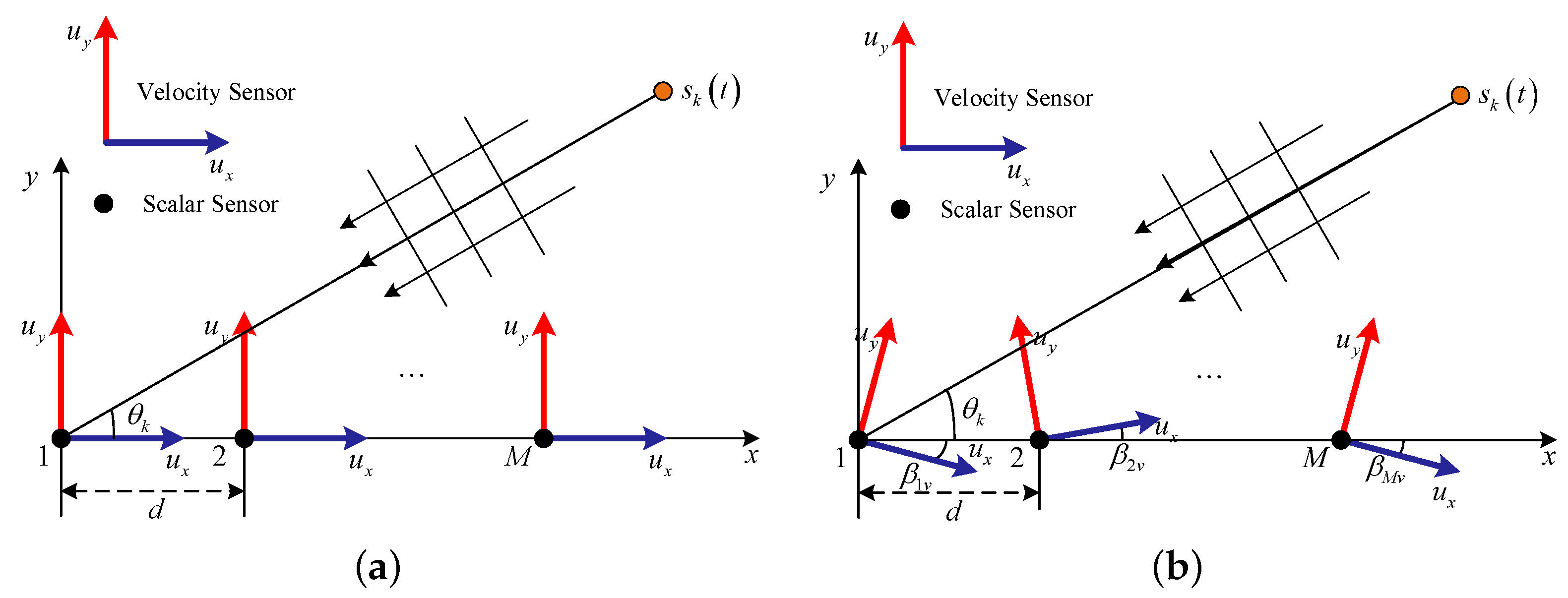
2.1. The Ideal Model of AVSA
2.2. The Model of AVSA with SDE
3. The Proposed Technique
3.1. Estimating the SDM
3.2. Estimating the Sparse Signal Power
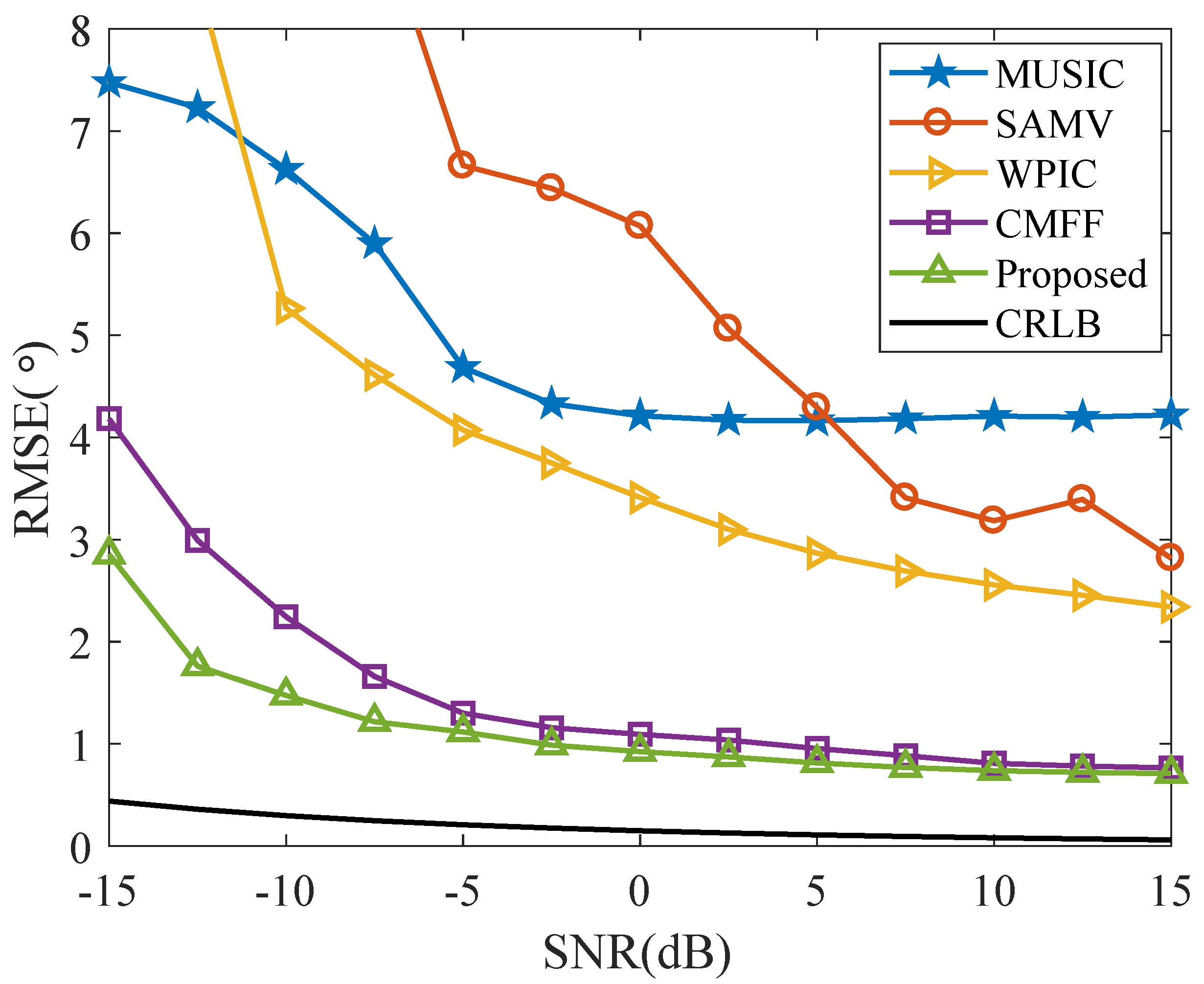
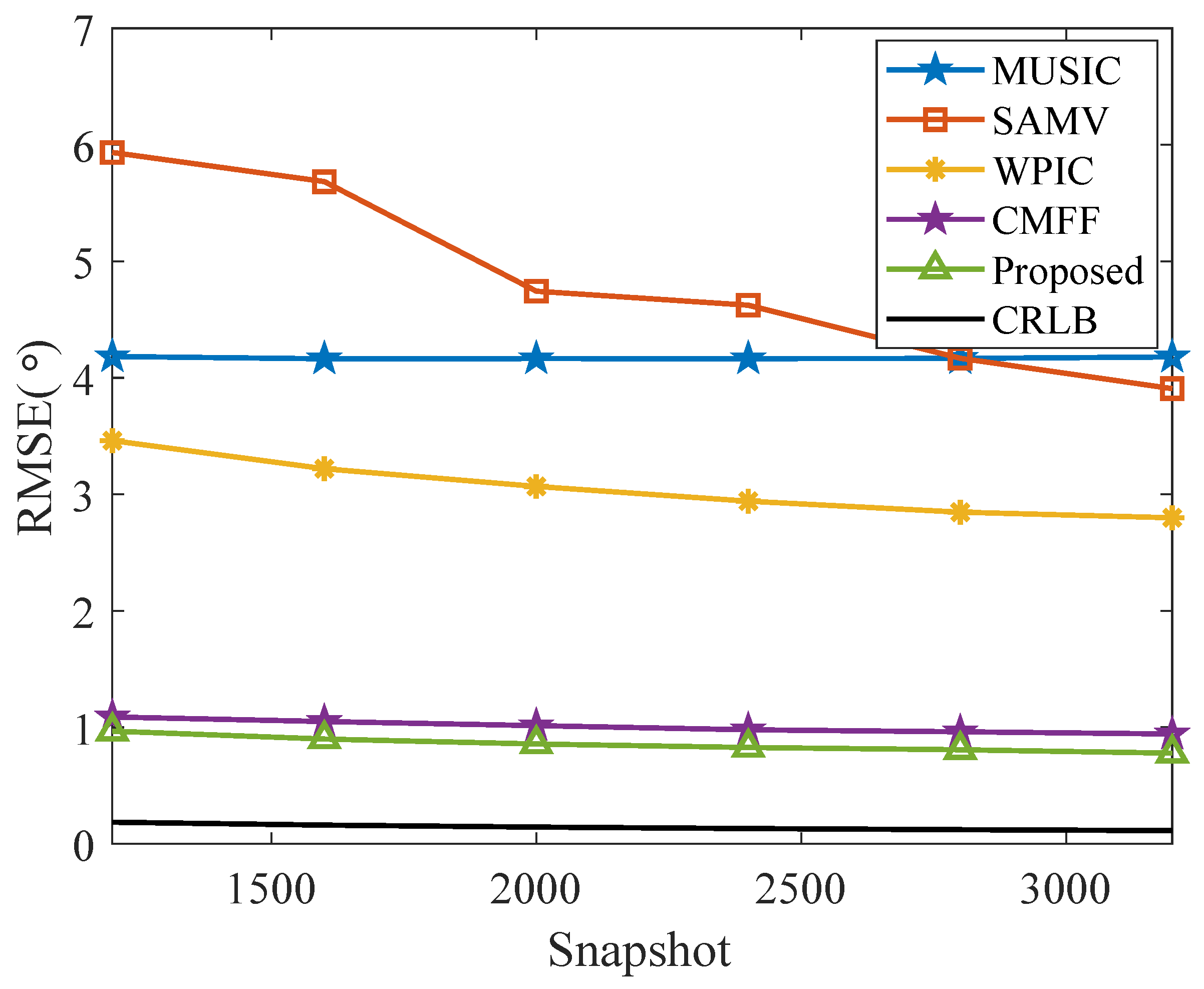
4. Simulation Results
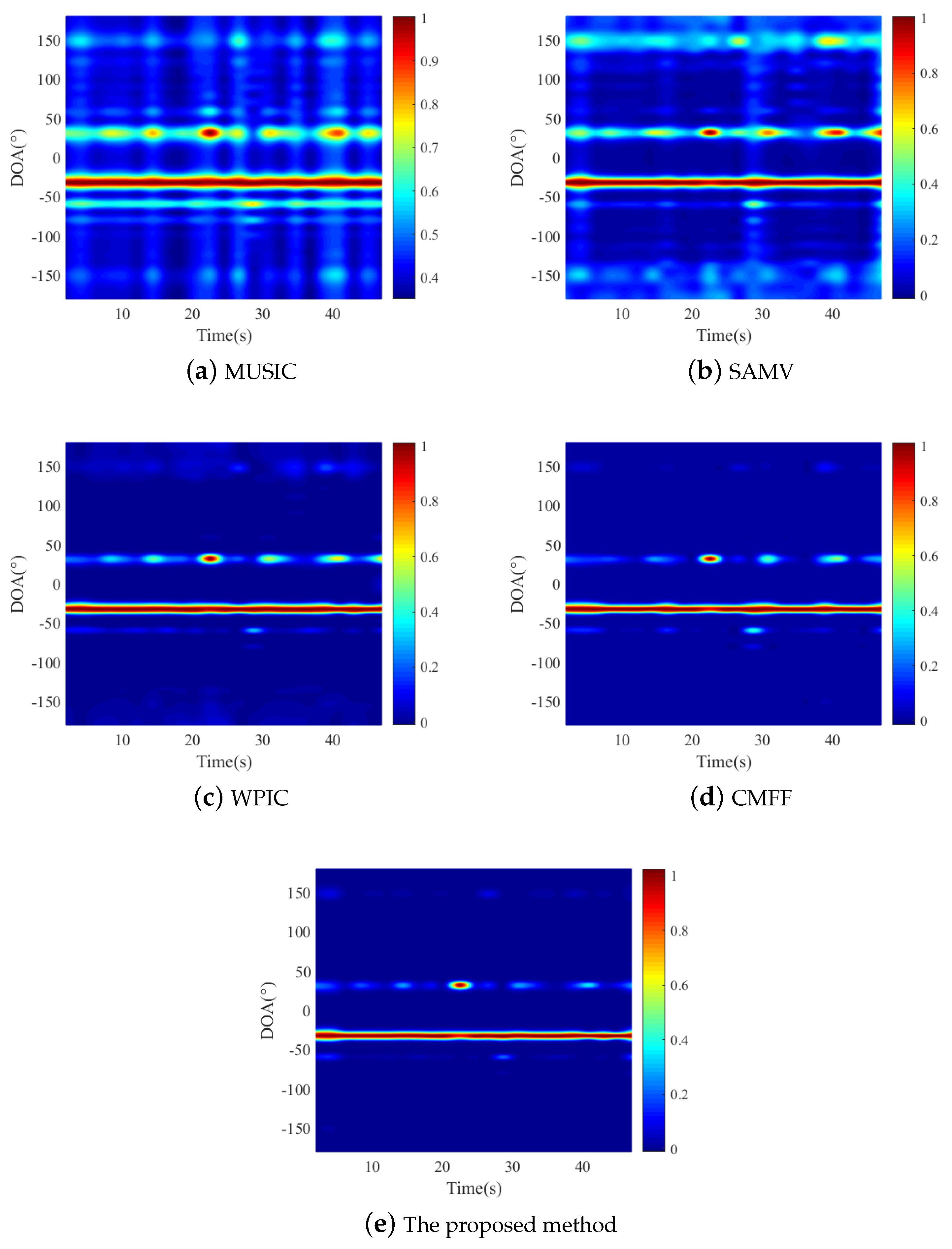
5. Experimental Results and Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nehorai, A.; Paldi, E. Acoustic vector-sensor array processing. IEEE Trans. Signal Process. 1994, 42, 2481–2491. [Google Scholar] [CrossRef]
- Chen, Q.; Li, J.; Wang, D.; Zhao, Y.; Fan, M. Cumulant-based 2-D Direction Estimation Using an Acoustic Vector Sensor Array. IEEE Sens. J. 2019, 19, 11698–11707. [Google Scholar]
- Chen, Y.; Zhang, G.; Wang, R.; Rong, H.; Yang, B. Acoustic vector sensor multi-source detection based on multimodal fusion. Sensors 2023, 23, 1301. [Google Scholar] [CrossRef]
- Shi, S.; Yang, D.; Liu, A.; Zhu, Z.; Li, Y. Augmented subspace music method for doa estimation using acoustic vector sensor array. IET Radar Sonar Navig. 2019, 13, 969–975. [Google Scholar]
- Shang, Z.; Zhang, W.; Zhang, G.; Zhang, X.; Ji, S.; Wang, R. Mixed near field and far field sources localization algorithm based on MEMS vector hydrophone array. Measurement 2020, 151, 107109. [Google Scholar] [CrossRef]
- Sharma, U.; Agrawal, M. 2qth-Order cumulants based virtual array of a single acoustic vector sensor. Digit. Signal Process. 2022, 123, 103438. [Google Scholar] [CrossRef]
- Heath, R.W.; Gonzalez-Prelcic, N.; Rangan, S.; Roh, W.; Sayeed, A.M. An overview of signal processing techniques for millimeter wave MIMO systems. IEEE J. Sel. Top. Signal Process. 2016, 10, 436–453. [Google Scholar] [CrossRef]
- He, Y.; Yang, J. Polarization Estimation with a Single Vector Sensor for Radar Detection. Remote Sens. 2022, 14, 1137. [Google Scholar] [CrossRef]
- Sinha, S.K.; Kumar, A.; Bahl, R. Study of acoustic vector sensor based direction of arrival estimation of in-air maneuvering tonal source. Appl. Acoust. 2022, 199, 109033. [Google Scholar] [CrossRef]
- Diao, Y.; Yu, L.; Jiang, W. High-resolution DOA estimation achieved by a single acoustic vector sensor under anisotropic noise. Appl. Acoust. 2023, 211, 109432. [Google Scholar] [CrossRef]
- Lou, Y.; Qu, X.; Wang, D.; Cheng, J. Direction-of-arrival estimation for nested acoustic vector-sensor arrays using quaternions. IEEE Trans. Geosci. Remote Sens. 2023, 61, 4204714. [Google Scholar] [CrossRef]
- Fischer, J.; Orescanin, M.; Leary, P.; Smith, K.B. Active Bayesian deep learning with vector sensor for passive sonar sensing of the ocean. IEEE J. Ocean. Eng. 2023, 48, 837–852. [Google Scholar] [CrossRef]
- Ma, L.; Yang, Y.; Wang, Z.; Wu, G.; Khan, A.Y.; Liu, S. Further results on maximal ratio combining under correlated noise for multi-carrier underwater acoustic communication using vector sensors. Appl. Acoust. 2023, 214, 109637. [Google Scholar] [CrossRef]
- Luo, L.; Qin, H.; Song, X.; Wang, M.; Qiu, H.; Zhou, Z. Wireless sensor networks for noise measurement and acoustic event recognitions in urban environments. Sensors 2020, 20, 2093. [Google Scholar] [CrossRef] [PubMed]
- Najeem, S.; Kiran, K.; Malarkodi, A.; Latha, G. Open lake experiment for direction of arrival estimation using acoustic vector sensor array. Appl. Acoust. 2017, 119, 94–100. [Google Scholar] [CrossRef]
- Wang, B.; Chen, F.; Ge, H. Subspace projection semi-real-valued MVDR algorithm based on vector sensors array processing. Neural Comput. Appl. 2020, 32, 173–181. [Google Scholar] [CrossRef]
- Wong, K.T.; Zoltowski, M.D. Self-initiating MUSIC-based direction finding in underwater acoustic particle velocity-field beamspace. IEEE J. Ocean. Eng. 2000, 25, 262–273. [Google Scholar] [CrossRef]
- Shi, S.G.; Li, Y.; Zhu, Z.R.; Shi, J. Real-valued robust DOA estimation method for uniform circular acoustic vector sensor arrays based on worst-case performance optimization. Appl. Acoust. 2019, 148, 495–502. [Google Scholar] [CrossRef]
- Dong, X.; Zhang, X.; Zhao, J.; Sun, M.; Wu, Q. Multi-maneuvering sources DOA tracking with improved interactive multi-model multi-bernoulli filter for acoustic vector sensor (AVS) array. IEEE Trans. Veh. Technol. 2021, 70, 7825–7838. [Google Scholar] [CrossRef]
- Malioutov, D.; Cetin, M.; Willsky, A.S. A sparse signal reconstruction perspective for source localization with sensor arrays. IEEE Trans. Signal Process. 2005, 53, 3010–3022. [Google Scholar] [CrossRef]
- Abeida, H.; Zhang, Q.; Li, J.; Merabtine, N. Iterative Sparse Asymptotic Minimum Variance Based Approaches for Array Processing. IEEE Trans. Signal Process. 2013, 61, 933–944. [Google Scholar] [CrossRef]
- Zhao, A.; Ma, L.; Hui, J.; Zeng, C.; Bi, X. Open-lake experimental investigation of azimuth angle estimation using a single acoustic vector sensor. J. Sens. 2018, 2018, 4324902. [Google Scholar] [CrossRef]
- Shi, S.; Li, Y.; Yang, D.; Liu, A.; Shi, J. Sparse representation based direction-of-arrival estimation using circular acoustic vector sensor arrays. Digit. Signal Process. 2020, 99, 102675. [Google Scholar] [CrossRef]
- Wang, W.; Tan, W.; Li, H.; Zhang, Q.; Shi, W. Source localization utilizing weighted power iterative compensation via acoustic vector hydrophone array. Appl. Acoust. 2021, 182, 108228. [Google Scholar] [CrossRef]
- Liu, A.; Shi, S.; Wang, X. Robust DOA Estimation Method for Underwater Acoustic Vector Sensor Array in Presence of Ambient Noise. IEEE Trans. Geosci. Remote Sens. 2023, 61, 4206014. [Google Scholar] [CrossRef]
- Lai, X.; Zhang, X.; Zheng, W.; Li, J.; Zhou, F. Fragmented coprime arrays with optimal inter subarray spacing for DOA estimation: Increased DOF and reduced mutual coupling. Signal Process. 2024, 215, 109273. [Google Scholar] [CrossRef]
- Vaidyanathan, P.P.; Pal, P. Sparse sensing with co-prime samplers and arrays. IEEE Trans. Signal Process. 2010, 59, 573–586. [Google Scholar] [CrossRef]
- Pan, J.; Sun, M.; Dong, X.; Wang, Y.; Zhang, X. Enhanced doa estimation with co-prime array in the scenario of impulsive noise: A pseudo snapshot augmentation perspective. IEEE Trans. Veh. Technol. 2023, 72, 11603–11616. [Google Scholar] [CrossRef]
- Pal, P.; Vaidyanathan, P.P. Nested arrays: A novel approach to array processing with enhanced degrees of freedom. IEEE Trans. Signal Process. 2010, 58, 4167–4181. [Google Scholar] [CrossRef]
- Shaalan, A.M.; Du, J. High-Order Dilated Nested Arrays with Increased Degrees of Freedom and Reduced Mutual Coupling. Digit. Signal Process. 2024, 153, 104650. [Google Scholar] [CrossRef]
- Liu, S.; Zhao, J.; Zhang, Y.; Wu, D. 2D DOA Estimation Algorithm by Nested Acoustic Vector-Sensor Array. Circuits Syst. Signal Process. 2022, 41, 1115–1130. [Google Scholar] [CrossRef]
- Zhang, J.; Zhang, G.; Geng, Y.; Ren, W.; Zhu, S.; Liu, G.; Liu, Y.; Jia, L.; Kong, X.; Wang, J.; et al. Research on the nested package structure of a MEMS vector hydrophone. IEEE Trans. Instrum. Meas. 2024, 73, 7502813. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, H.; Lv, Y. Improving the beamforming performance of a vector sensor line array with a coprime array configuration. Appl. Acoust. 2023, 207, 109329. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, H.; Gao, Y.; Wang, Z. DOA estimation of underwater acoustic co-frequency sources for the coprime vector sensor array. Front. Mar. Sci. 2023, 10, 1211234. [Google Scholar] [CrossRef]
- Wu, X.; Yang, X.; Jia, X.; Tian, F. A gridless DOA estimation method based on convolutional neural network with Toeplitz prior. IEEE Signal Process. Lett. 2022, 29, 1247–1251. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, H.; Wang, B. DOA estimation based on CNN for underwater acoustic array. Appl. Acoust. 2021, 172, 107594. [Google Scholar] [CrossRef]
- Xie, Y.; Wang, B. Data-Driven DOA Estimation Methods Based on Deep Learning for Underwater Acoustic Vector Sensor Array. Mar. Technol. Soc. J. 2023, 57, 16–29. [Google Scholar] [CrossRef]
- Cao, H.; Wang, W.; Su, L.; Ni, H.; Gerstoft, P.; Ren, Q.; Ma, L. Deep transfer learning for underwater direction of arrival using one vector sensor. J. Acoust. Soc. Am. 2021, 149, 1699–1711. [Google Scholar] [CrossRef]
- Xie, Y.; Wang, B. Direction-of-arrival estimation method based on neural network with temporal structure for underwater acoustic vector sensor array. Sensors 2023, 23, 4919. [Google Scholar] [CrossRef]
- Swindlehurst, A.L.; Kailath, T. A performance analysis of subspace-based methods in the presence of model errors. I. The MUSIC algorithm. IEEE Trans. Signal Process. 1992, 40, 1758–1774. [Google Scholar] [CrossRef]
- Ramamohan, K.N.; Chepuri, S.P.; Comesaña, D.F.; Leus, G. Self-calibration of acoustic scalar and vector sensor arrays. IEEE Trans. Signal Process. 2022, 71, 61–75. [Google Scholar] [CrossRef]
- Jacobsen, F.; Jaud, V. A note on the calibration of pressure-velocity sound intensity probes. J. Acoust. Soc. Am. 2006, 120, 830–837. [Google Scholar] [CrossRef]
- Basten, T.G.; de Bree, H.E. Full bandwidth calibration procedure for acoustic probes containing a pressure and particle velocity sensor. J. Acoust. Soc. Am. 2010, 127, 264–270. [Google Scholar] [CrossRef] [PubMed]
- Wajid, M.; Kumar, A.; Bahl, R. Microphone Based Acoustic Vector Sensor for Direction Finding with Bias Removal. Arch. Acoust. 2022, 47, 151–167. [Google Scholar]
- Kotus, J.; Szwoch, G. Calibration of acoustic vector sensor based on MEMS microphones for DOA estimation. Appl. Acoust. 2018, 141, 307–321. [Google Scholar] [CrossRef]
- Yuan, L.; Jiang, R.; Chen, Y. Gain and Phase Autocalibration of Large Uniform Rectangular Arrays for Underwater 3-D Sonar Imaging Systems. IEEE J. Ocean. Eng. 2014, 39, 458–471. [Google Scholar] [CrossRef]
- Viberg, M.; Swindlehurst, A.L. A Bayesian approach to auto-calibration for parametric array signal processing. IEEE Trans. Signal Process. 1994, 42, 3495–3507. [Google Scholar] [CrossRef]
- Cheng, Q.; Hua, Y.; Stoica, P. Asymptotic performance of optimal gain-and-phase estimators of sensor arrays. IEEE Trans. Signal Process. 2000, 48, 3587–3590. [Google Scholar]
- Wang, W.; Tan, W.J. Alternating iterative adaptive approach for DOA estimation via acoustic vector sensor array under directivity bias. IEEE Commun. Lett. 2020, 24, 1944–1948. [Google Scholar] [CrossRef]
- Shi, S.; Xu, F.; Zhang, X.; Zhu, X.; Shen, N.; Gui, C. Eigenstructure methods for DOA estimation of circular acoustic vector sensor array with axial angle bias in nonuniform noise. Digit. Signal Process. 2024, 147, 104404. [Google Scholar] [CrossRef]
- Wang, W.; Li, X.; Liu, Z.; Shi, W.; Li, H. Direction finding method via acoustic vector sensor array with fluctuating misorientation. Appl. Acoust. 2023, 211, 109469. [Google Scholar] [CrossRef]
- Zhang, Z.; Wu, X.; Li, C.; Zhu, W.P. An ℓp-norm based method for off-grid doa estimation. Circuits Syst. Signal Process. 2019, 38, 904–917. [Google Scholar] [CrossRef]
- Wu, X.; Zhu, W.P.; Yan, J.; Zhang, Z. Two sparse-based methods for off-grid direction-of-arrival estimation. Signal Process. 2018, 142, 87–95. [Google Scholar] [CrossRef]
- Xu, Z.; Chang, X.; Xu, F.; Zhang, H. L1/2 regularization: A thresholding representation theory and a fast solver. IEEE Trans. Neural Netw. Learn. Syst. 2012, 23, 1013–1027. [Google Scholar]

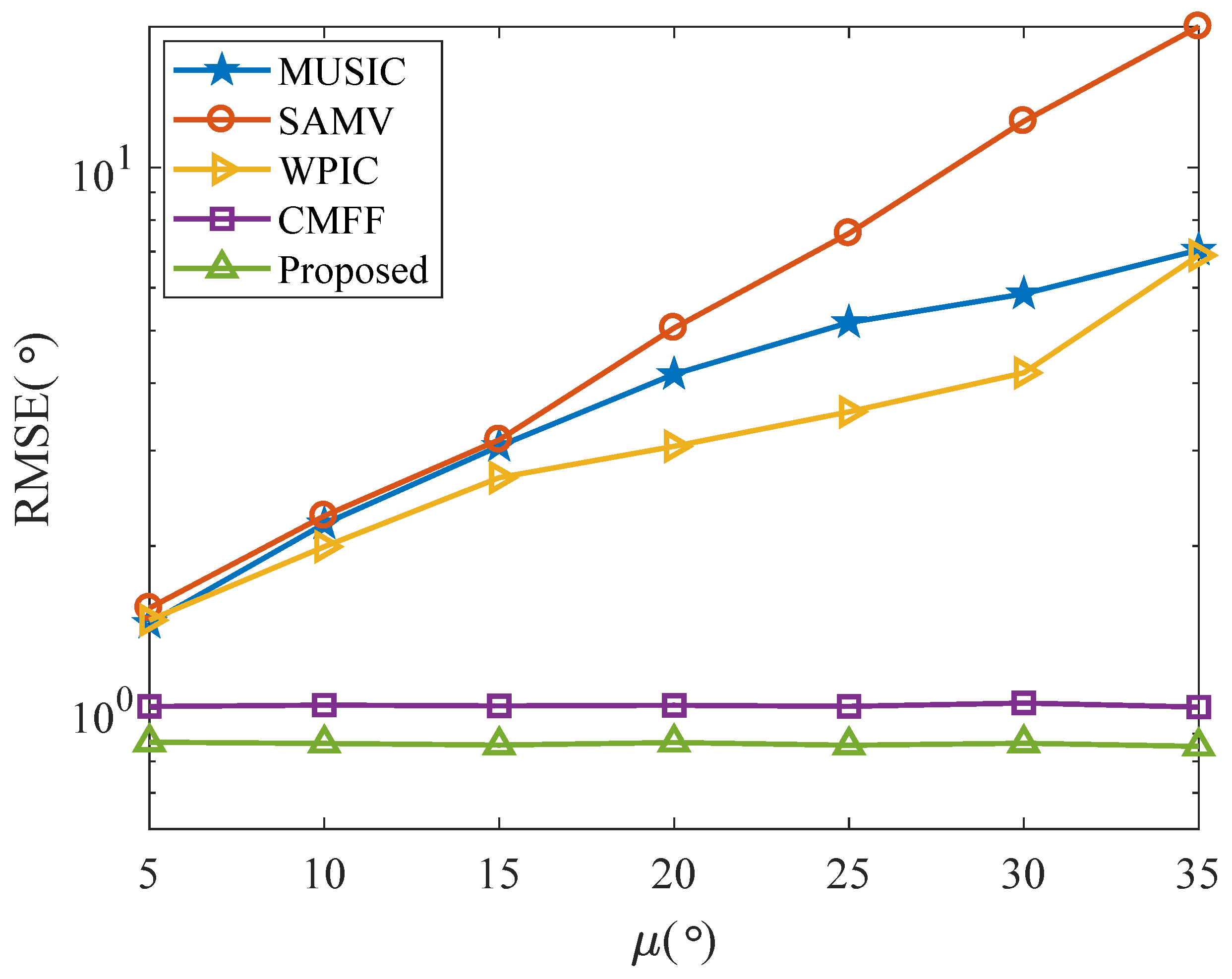

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Ma, L.; Shi, W.; Ali, W. Robust Underwater Direction-of-Arrival Estimation Method Using Acoustic Sensor Array under Unknown Swing Deviation Elements. Remote Sens. 2024, 16, 3634. https://doi.org/10.3390/rs16193634
Wang W, Ma L, Shi W, Ali W. Robust Underwater Direction-of-Arrival Estimation Method Using Acoustic Sensor Array under Unknown Swing Deviation Elements. Remote Sensing. 2024; 16(19):3634. https://doi.org/10.3390/rs16193634
Chicago/Turabian StyleWang, Weidong, Linya Ma, Wentao Shi, and Wasiq Ali. 2024. "Robust Underwater Direction-of-Arrival Estimation Method Using Acoustic Sensor Array under Unknown Swing Deviation Elements" Remote Sensing 16, no. 19: 3634. https://doi.org/10.3390/rs16193634
APA StyleWang, W., Ma, L., Shi, W., & Ali, W. (2024). Robust Underwater Direction-of-Arrival Estimation Method Using Acoustic Sensor Array under Unknown Swing Deviation Elements. Remote Sensing, 16(19), 3634. https://doi.org/10.3390/rs16193634






