On a Correlation Model for Laser Scanners: A Large Eddy Simulation Experiment
Abstract
1. Introduction
- estimates from simulated phase spectra for various meteorological conditions with a statistically based method rather than an empirical one,
- provides new insights into and its variability with height depending on the turbulent conditions (wind shear, buoyancy) to derive an atmospheric correlation model for terrestrial laser scanners,
- allows gaining a better understanding of its relationship to the local as derived by Tatarskii and measured by radiosondes to avoid confusion.
2. Methods: Wave Propagation and Large Eddy Simulation
2.1. Wave Propagation through Simulated Atmosphere
- Simulation of a spatio-temporal 4D field of temperature and pressure using LES as described in Section 2.6.
- Generation of phases starting from the Rytov approximation. More specifically, I make use of the Rytov first iteration solution for the phase. This latter is known to be valid also in the strong fluctuation regions [31]. Its exponential representation is favorable to represent a wave propagating through a random medium.
- Statistical estimation of the parameters of the power spectrum from the generated phases propagating in the simulated atmosphere (from the first step). I fully exploit the potential of LES to determine the temporal refractivity index fluctuations at any location along the propagation path using virtual measurements [32]. Thus, I do not rely on any apriori model as in [33], and the references inside.
2.2. Refractivity Index
2.3. Theoretical Power Spectrum of Phase Fluctuations
2.4. Whittle Maximum Likelihood Estimation (WMLE)
The Debiased WMLE
2.5. LES: Principle
2.6. LES: Simulation Setup
3. Results
3.1. Presentation of the Results
- generate three turbulent atmospheres following Table 1 using LES as described in Section 2.6,
- simulate an optical wave propagating along the x-axis, perpendicular to the geostropic wind component [38] and set m,
- estimate statistically the cutoff frequency , the parameter and the variance using the debiased WMLE,
- compute for the inertial subrange following Equation (7). I compare this value to the one found in step 3.
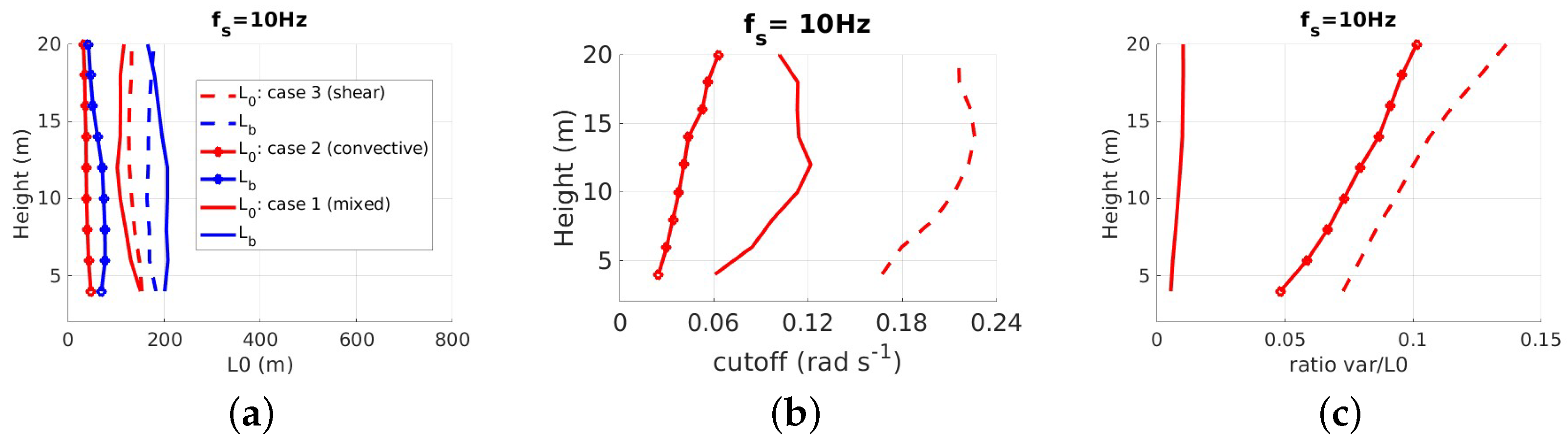
3.2. Case 1: Mixed Turbulence
3.3. Case 2: Convective-Driven Turbulence
3.4. Case 3: Shear-Driven Turbulence
Nesting: m
- similar to the non-nested cases, the variability of of with height is low, particularly for case 2 (convective-driven turbulence). The values found are compatible for all cases with the previous results from m.
- No clear linear dependency neither of nor of with height can be deduced from the analysis but I note that increases linearly with height in the first 10 m above the ground. The slope is the same for cases 2 and 3 and slightly lower for case 1. It cannot be linked to the power laws sometimes found in the literature. increases slightly with height (not shown as similar to the non-nested case) but is higher for the convective case, as in the non-nested simulations. This shape could be related to variations on smaller scales and the stronger impact of the surface layer, i.e., the shorter reorganization rate of the turbulent structure, in that case [52].
4. Discussion and Application
4.1. General Consideration
4.2. Atmospheric Correlation Model
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| LES | Large Eddy Simulation |
| WMLE | Whittle Maximum Likelihood Estimation |
| MDPI | Multidisciplinary Digital Publishing Institute |
| DOAJ | Directory of open access journals |
| TLA | Three letter acronym |
| LD | Linear dichroism |
References
- Andrews, L.C.; Phillips, R.L. Laser Beam Propagation through Random Media; SPIE Optical Engineering Press: San Francisco, CA, USA, 2005. [Google Scholar] [CrossRef]
- Rod, F. Effects of refractive turbulence on coherent laser radar. Appl. Opt. 1993, 32, 2122–2139. [Google Scholar] [CrossRef]
- Rod, F. Effects of global intermittency on laser propagation in the atmosphere. Appl. Opt. 1994, 33, 5764–5769. [Google Scholar] [CrossRef]
- Stull, R. An Introduction to Boundary Layer Meteorology; Atmospheric and Oceanographic Sciences Library; Springer: Dordrecht, The Netherlands, 1988. [Google Scholar]
- Bonnefois, A.M.; Velluet, M.T.; Cissé, M.; Lim, C.B.; Conan, J.M.; Petit, C.; Sauvage, J.F.; Meimon, S.; Perrault, P.; Montri, J.; et al. Feasibility demonstration of AO pre-compensation for GEO feeder links in a relevant environment. Opt. Express 2022, 30, 47179–47198. [Google Scholar] [CrossRef] [PubMed]
- Avila, R.; Ziad, A.; Borgnino, J.; Martin, F.; Agabi, A.; Tokovinin, A. Theoretical spatiotemporal analysis of angle of arrival induced by atmospheric turbulence as observed with the grating scale monitor experiment. J. Opt. Soc. Am. A 1997, 14, 3070–3082. [Google Scholar] [CrossRef]
- Ziad, A. Review of the Outer Scale of the Atmospheric Turbulence. In Proceedings of the Adaptive Optics Systems V, Edinburgh, UK, 26 June–1 July 2016; SPIE: San Francisco, CA, USA, 2016; Volume 9909, p. 99091K. [Google Scholar] [CrossRef]
- Kermarrec, G.; Lösler, M.; Hartmann, J. Analysis of the temporal correlations of TLS range observations from plane fitting residuals. ISPRS J. Photogramm. Remote Sens. 2021, 171, 119–132. [Google Scholar] [CrossRef]
- Weinstock, J. On the Theory of Turbulence in the Buoyancy Subrange of Stably Stratified Flows. J. Atmos. Sci. 1978, 35, 634–649. [Google Scholar] [CrossRef]
- Basu, S.; Holtslag, A.A.M. Revisiting and revising Tatarskii’s formulation for the temperature structure parameter (CT2) in atmospheric flows. Environ. Fluid Mech. 2022, 22, 1107–1119. [Google Scholar] [CrossRef]
- Ziad, A.; Conan, R.; Tokovinin, A.; Martin, F.; Borgnino, J. From the grating scale monitor to the generalized seeing monitor. Appl. Opt. 2000, 39, 5415–5425. [Google Scholar] [CrossRef]
- Kermarrec, G.; Lösler, M.; Guerrier, S.; Schön, S. The variance inflation factor to account for correlations in likelihood ratio tests: Deformation analysis with Terrestrial Laser Scanners. J. Geod. 2022, 96. [Google Scholar] [CrossRef]
- Védrenne, N.; Michau, V.; Robert, C.; Conan, J.M. Cn2 profile measurement from Shack-Hartmann data. Opt. Lett. 2007, 32, 2659–2661. [Google Scholar] [CrossRef]
- Cyril, P.; Aurélie, B.; Jean-Marc, C.; Anne, D.; François, G.; Caroline, L.; Joseph, M.; Laurie, P.; Philippe, P.; Marie-Thérèse, V.; et al. FEELINGS: The ONERA’s optical ground station for Geo Feeder links demonstration. In Proceedings of the 2022 IEEE International Conference on Space Optical Systems and Applications (ICSOS), Virtual, 29–31 March 2022; pp. 255–260. [Google Scholar] [CrossRef]
- Jakobsson, H. Simulations of time series of atmospherically distorted wave fronts. Appl. Opt. 1996, 35, 1561–1565. [Google Scholar] [CrossRef] [PubMed]
- Chen, Z.; Zhang, D.; Xiao, C.; Qin, M. Precision analysis of turbulence phase screens and their influence on the simulation of Gaussian beam propagation in turbulent atmosphere. Appl. Opt. 2020, 59, 3726–3735. [Google Scholar] [CrossRef] [PubMed]
- Mahe, F.; Michau, V.; Rousset, G.; Conan, J.M. Scintillation effects on wavefront sensing in the Rytov regime. In Proceedings of the Propagation and Imaging through the Atmosphere IV, San Diego, CA, USA, 3 August 2000; Roggemann, M.C., Ed.; International Society for Optics and Photonics. SPIE: San Francisco, CA, USA, 2000; Volume 4125, pp. 77–86. [Google Scholar] [CrossRef]
- Winker, D.M. Effect of a finite outer scale on the Zernike decomposition of atmospheric optical turbulence. J. Opt. Soc. Am. A 1991, 8, 1568–1573. [Google Scholar] [CrossRef]
- Rao, R.; Wang, S.; Liu, X.; Gong, Z. Turbulence spectrum effect on wave temporal-frequency spectra for light propagating through the atmosphere. J. Opt. Soc. Am. A 1999, 16, 2755–2762. [Google Scholar] [CrossRef]
- Gilbert, K.E.; Di, X.; Khanna, S.; Otte, M.J.; Wyngaard, J.C. Electromagnetic wave propagation through simulated atmospheric refractivity fields. Radio Sci. 1999, 34, 1413–1435. [Google Scholar] [CrossRef]
- Ishimaru, A. Wave Propagation and Scattering in Random Media; IEEE Press: Piscataway, NJ, USA, 2005. [Google Scholar]
- Sykulski, A.M.; Olhede, S.C.; Guillaumin, A.P.; Lilly, J.M.; Early, J.J. The debiased Whittle likelihood. Biometrika 2019, 106, 251–266. [Google Scholar] [CrossRef]
- Kulikov, V.; Andreeva, M.; Koryabin, A.; Shmalhausen, V. Method of estimation of turbulence characteristic scales. Appl. Opt. 2012, 51, 8505–8515. [Google Scholar] [CrossRef][Green Version]
- van Dinther, D.; Hartogensis, O.K. Using the Time-Lag Correlation Function of Dual-Aperture Scintillometer Measurements to Obtain the Crosswind. J. Atmos. Ocean. Technol. 2014, 31, 62–78. [Google Scholar] [CrossRef]
- Lilly, J.M.; Sykulski, A.M.; Early, J.J.; Olhede, S.C. Fractional Brownian motion, the Matérn process, and stochastic modeling of turbulent dispersion. Nonlinear Process. Geophys. 2017, 24, 481–514. [Google Scholar] [CrossRef]
- Taylor, G.I. The Spectrum of Turbulence. Proc. R. Soc. London. Ser. A-Math. Phys. Sci. 1938, 164, 476–490. [Google Scholar] [CrossRef]
- Cui, L.; Xue, B.; Zhou, F. Generalized anisotropic turbulence spectra and applications in the optical waves’ propagation through anisotropic turbulence. Opt. Express 2015, 23, 30088–30103. [Google Scholar] [CrossRef] [PubMed]
- Weichel, H. Laser Beam Propagation in the Atmosphere; SPIE Press: San Francisco, CA, USA, 1990; Volume TT03, p. 108. [Google Scholar]
- Cheinet, S.; Cumin, P. Local Structure Parameters of Temperature and Humidity in the Entrainment-Drying Convective Boundary Layer: A Large-Eddy Simulation Analysis. J. Appl. Meteorol. Climatol. 2011, 50, 472–481. [Google Scholar] [CrossRef]
- Moene, A. Effects of water vapour on the structure parameter of the refractive index for near-infrared radiation. Bound.-Layer Meteorol. 2003, 107, 635–653. [Google Scholar] [CrossRef]
- Barabanenkov, Y.N.; Kravtsov, Y.A.; Ozrin, V.; Saichev, A. II Enhanced Backscattering in Optics. Prog. Opt. 1991, 29, 65–197. [Google Scholar] [CrossRef]
- Rahlves, C.; Beyrich, F.; Raasch, S. Scan strategies for wind profiling with Doppler lidar – an large-eddy simulation (LES)-based evaluation. Atmos. Meas. Tech. 2022, 15, 2839–2856. [Google Scholar] [CrossRef]
- Jia, P.; Cai, D.; Wang, D.; Basden, A. Simulation of atmospheric turbulence phase screen for large telescope and optical interferometer. Mon. Not. R. Astron. Soc. 2015, 447, 3467–3474. [Google Scholar] [CrossRef]
- Andreas, E.L. Estimating Cn2 over snow and sea ice from meteorological data. J. Opt. Soc. Am. A 1988, 5, 481–495. [Google Scholar] [CrossRef]
- Arthur, D.W.; Davis, P.J.; Rabinowitz, P. Methods of numerical Integration, 2nd ed.; Davis, P.J., Rabinowitz, P., Eds.; Academic Press: Cambridge, MA, USA, 1984; p. 612. ISBN 0-12-206360-0. [Google Scholar]
- Tatarski, V.I.; Silverman, R.A.; Chako, N. Wave Propagation in a Turbulent Medium. Phys. Today 1961, 14, 46. [Google Scholar] [CrossRef]
- Masciadri, E.; Vernin, J.; Bougeault, P. 3D mapping of optical turbulence using an atmospheric numerical model-I. A useful tool for ground-based astronomy. Astron. Astrophys. Suppl. Ser. 1999, 137, 185–202. [Google Scholar] [CrossRef]
- Wheelon, A.D. Electromagnetic Scintillation; Cambridge University Press: Cambridge, UK, 2001; Volume 1. [Google Scholar] [CrossRef]
- Williamson, J. Low-storage Runge-Kutta schemes. J. Comput. Phys. 1980, 35, 48–56. [Google Scholar] [CrossRef]
- Perez, D.G.; Sepulveda, M.; Farfan, H. Spatiotemporal statistics of optical turbulence beyond Taylor’s frozen turbulence hypothesis. J. Opt. Soc. Am. A 2024, 41, B135–B143. [Google Scholar] [CrossRef] [PubMed]
- Hocking, W.K. Measurement of turbulent energy dissipation rates in the middle atmosphere by radar techniques: A review. Radio Sci. 1985, 20, 1403–1422. [Google Scholar] [CrossRef]
- Klipp, C. Turbulence Anisotropy in the Near-Surface Atmosphere and the Evaluation of Multiple Outer Length Scales. Bound.-Layer Meteorol. 2014, 151. [Google Scholar] [CrossRef]
- Du, W.; Tan, L.; Ma, J.; Jiang, Y. Temporal-frequency spectra for optical wave propagating through non-Kolmogorov turbulence. Opt. Express 2010, 18, 5763–5775. [Google Scholar] [CrossRef]
- Kermarrec, G.; Schön, S. On the Mátern covariance family: A proposal for modeling temporal correlations based on turbulence theory. J. Geod. 2014, 88, 1061–1079. [Google Scholar] [CrossRef]
- Brockwell, P.J.; Davis, R.A. Time Series: Theory and Methods; Springer Series in Statistics; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar] [CrossRef]
- Montillet, J.P.; Bos, M.S. Geodetic Time Series Analysis in Earth Sciences; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Davis, J.; Lawson, P.R.; Booth, A.J.; Tango, W.J.; Thorvaldson, E.D. Atmospheric path variations for baselines up to 80 m measured with the Sydney University Stellar Interferometer. Mon. Not. R. Astron. Soc. 1995, 273, L53–L58. [Google Scholar] [CrossRef]
- Pérez, D.G.; González, I.R.; Farfán, H. Beyond Taylor’s frozen turbulence hypothesis in ground-layer turbulence. In Proceedings of the Environmental Effects on Light Propagation and Adaptive Systems VI, Amsterdam, The Netherlands, 5–6 September 2023; Stein, K., Gladysz, S., Eds.; International Society for Optics and Photonics. SPIE: San Francisco, CA, USA, 2023; Volume 12731, p. 127310. [Google Scholar] [CrossRef]
- Maronga, B.; Banzhaf, S.; Burmeister, C.; Esch, T.; Forkel, R.; Fröhlich, D.; Fuka, V.; Gehrke, K.F.; Geletič, J.; Giersch, S.; et al. Overview of the PALM model system 6.0. Geosci. Model Dev. 2020, 13, 1335–1372. [Google Scholar] [CrossRef]
- Wicker, L.J.; Skamarock, W.C. Time-Splitting Methods for Elastic Models Using Forward Time Schemes. Mon. Weather Rev. 2002, 130, 2088–2097. [Google Scholar] [CrossRef]
- Deardorff, J.W. Stratocumulus-capped mixed layers derived from a three-dimensional model. Bound.-Layer Meteorol. 1980, 18, 495–527. [Google Scholar] [CrossRef]
- Cheinet, S.; Siebesma, A.P. The impact of boundary layer turbulence on optical propagation. In Proceedings of the Optics in Atmospheric Propagation and Adaptive Systems X, Warsaw, Poland, 13–14 September 2017; Stein, K., Kohnle, A., Gonglewski, J.D., Eds.; International Society for Optics and Photonics. SPIE: San Francisco, CA, USA, 2007; Volume 6747, p. 67470A. [Google Scholar] [CrossRef]
- Wilson, C.; Fedorovich, E. Direct Evaluation of Refractive-Index Structure Functions from Large-Eddy Simulation Output for Atmospheric Convective Boundary Layers. Acta Geophys. 2012, 60, 1474–1492. [Google Scholar] [CrossRef]
- Klemp, J.; Lilly, D. Numerical Simulation of Hydrostatic Mountain Waves. J. Atmos. Sci. 1978, 35, 78–107. [Google Scholar] [CrossRef]
- Mauritsen, T.; Svensson, G. Observations of Stably Stratified Shear-Driven Atmospheric Turbulence at Low and High Richardson Numbers. J. Atmos. Sci. 2007, 64, 645–655. [Google Scholar] [CrossRef]
- Mahrt, L. Nocturnal boundary-layer regimes. Bound.-Layer Meteorol. 1998, 88, 255–278. [Google Scholar] [CrossRef]
- Abahamid, A.; Jabiri, A.; Vernin, J.; Benkhaldoun, Z.; Azouit, M.; Agabi, A. Optical turbulence modeling in the boundary layer and free atmosphere using instrumented meteorological balloons. Astron. Astrophys. 2004, 416, 1193–1200. [Google Scholar] [CrossRef]
- Sauvage, C.; Robert, C.; Mugnier, L.M.; Conan, J.M.; Cohard, J.M.; Nguyen, K.L.; Irvine, M.; Lagouarde, J.P. Near ground horizontal high resolution Cn2 profiling from Shack–Hartmann slopeand scintillation data. Appl. Opt. 2021, 60, 10499–10519. [Google Scholar] [CrossRef] [PubMed]
- Thiermann, V.; Karipot, A.; Dirmhirn, I.; Poschl, P.; Czekits, C. Optical turbulence over paved surfaces. In Proceedings of the Atmospheric Propagation and Remote Sensing IV, Orlando, FL, USA, 17–19 April 1995; Dainty, J.C., Ed.; International Society for Optics and Photonics. SPIE: San Francisco, CA, USA, 1995; Volume 2471, pp. 197–203. [Google Scholar] [CrossRef]
- Pérez Muñoz, P.; Albajez García, J.A.; Santolaria Mazo, J. Analysis of the initial thermal stabilization and air turbulences effects on Laser Tracker measurements. J. Manuf. Syst. 2016, 41, 277–286. [Google Scholar] [CrossRef]
- Sühring, M.; Maronga, B.; Herbort, F.; Raasch, S. On the effect of surface heat-flux heterogeneities on the mixed-layer-top entrainment. Bound.-Layer Meteorol. 2014, 151, 531–556. [Google Scholar] [CrossRef]
- Kermarrec, G.; Schild, N.; Hartmann, J. Using Least-Squares Residuals to Assess the Stochasticity of Measurements—Example: Terrestrial Laser Scanner and Surface Modeling. In Proceedings of the 7th International conference on Time Series and Forecasting, Gran Canaria, Spain, 19–21 July 2021. [Google Scholar] [CrossRef]
- Brunner, F.K. Modelling of Atmospheric Effects on Terrestrial Geodetic Measurements. In Geodetic Refraction: Effects of Electromagnetic Wave Propagation Through the Atmosphere; Brunner, F.K., Ed.; Springer: Berlin/Heidelberg, Germany, 1984; pp. 143–162. [Google Scholar]
- Teunissen, P. Adjustment Theory; Vol. Series on Mathematical Geodesy and Positioning, DUP Blueprint; 2003. [Google Scholar]
- Wu, C.; Yuan, Y.; Tang, Y.; Tian, B. Application of Terrestrial Laser Scanning (TLS) in the Architecture, Engineering and Construction (AEC) Industry. Sensors 2021, 22, 265. [Google Scholar] [CrossRef]
- Zhao, L.; Ma, X.; Xiang, Z.; Zhang, S.; Hu, C.; Zhou, Y.; Chen, G. Landslide Deformation Extraction from Terrestrial Laser Scanning Data with Weighted Least Squares Regularization Iteration Solution. Remote Sens. 2022, 14, 2897. [Google Scholar] [CrossRef]
- Vos, S.; Kuschnerus, M.; Lindenbergh, R. Assessing the Error Budget for Permanent Laser Scanning in Coastal Areas. In Proceedings of the Proceedings FIG Working Week, Amsterdam, The Netherlands, 10–14 May 2020; International Federation of Surveyors (FIG): Copenhagen, Denmark, 2020. [Google Scholar]
- Kermarrec, G.; Czerwonka-Schröder, D.; Holst, C. Does atmospheric turbulence affect long-range terrestrial laser scanner observations? A case study in alpine region. In Proceedings of the Environmental Effects on Light Propagation and Adaptive Systems VI, Amsterdam, The Netherlands, 5–6 September 2023; Stein, K., Gladysz, S., Eds.; International Society for Optics and Photonics. SPIE: San Francisco, CA, USA, 2023; Volume 12731, p. 127310H. [Google Scholar] [CrossRef]
- Di Biase, V.; Kuschnerus, M.; Lindenbergh, R.C. Permanent Laser Scanner and Synthetic Aperture Radar Data: Correlation Characterisation at a Sandy Beach. Sensors 2022, 22, 2311. [Google Scholar] [CrossRef]
- Schröder, D.; Anders, K.; Winiwarter, L.; Wujanz, D. Permanent Terrestrial Lidar monitoring in mining, natural hazard prevention and Infrastructure Protection—Chances, risks, and challenges: A case study of a rockfall in Tyrol, Austria. In Proceedings of the 5th Joint International Symposium on Deformation Monitoring—JISDM 2022, Valencia, Spain, 20–22 June 2022. [Google Scholar] [CrossRef]
- Kermarrec, G. PALM_AMT_output, 2024. 11:46 PM (UTC+01:00). Available online: https://data.uni-hannover.de/dataset/2a6fe351-5a8a-4c1f-839c-abe8800a2d33 (accessed on 19 September 2024).

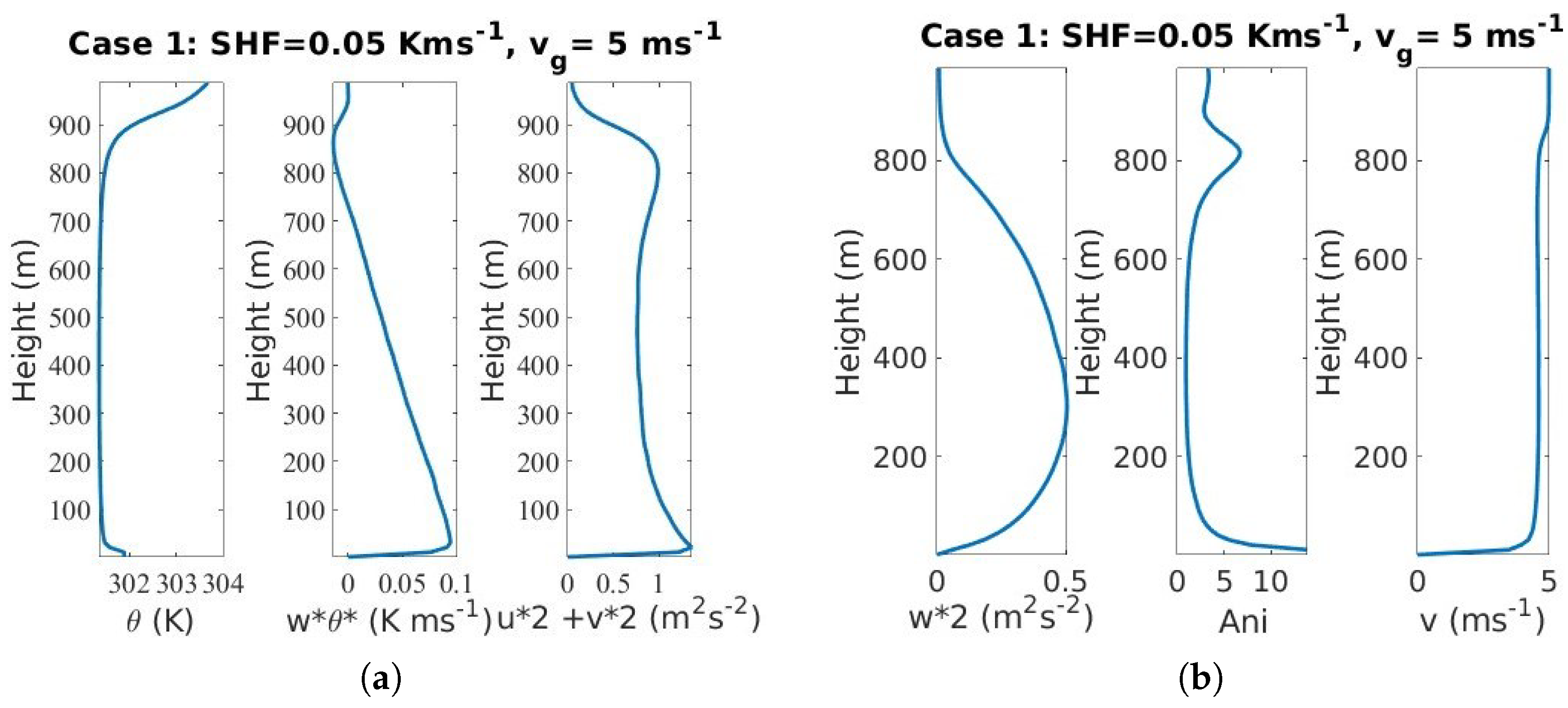
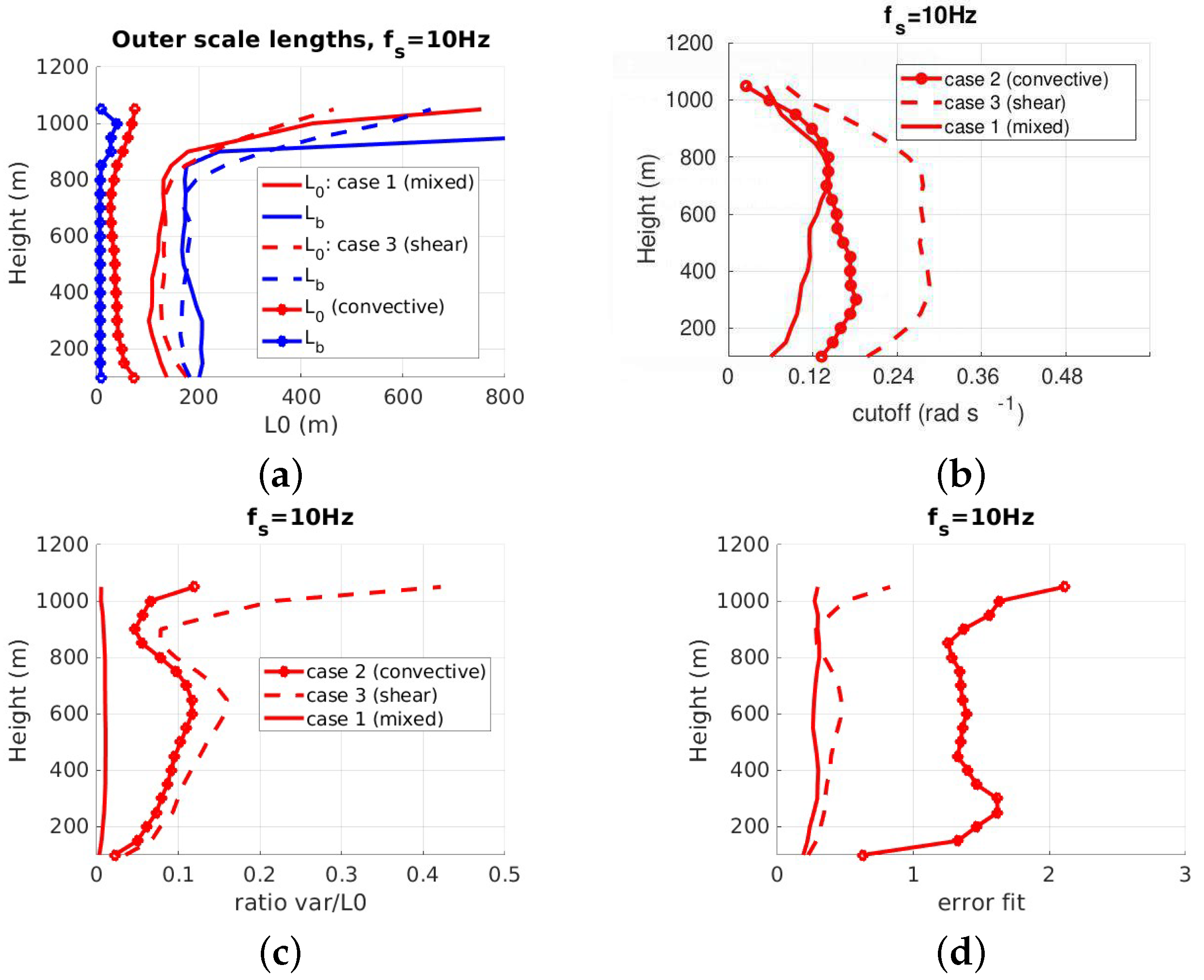
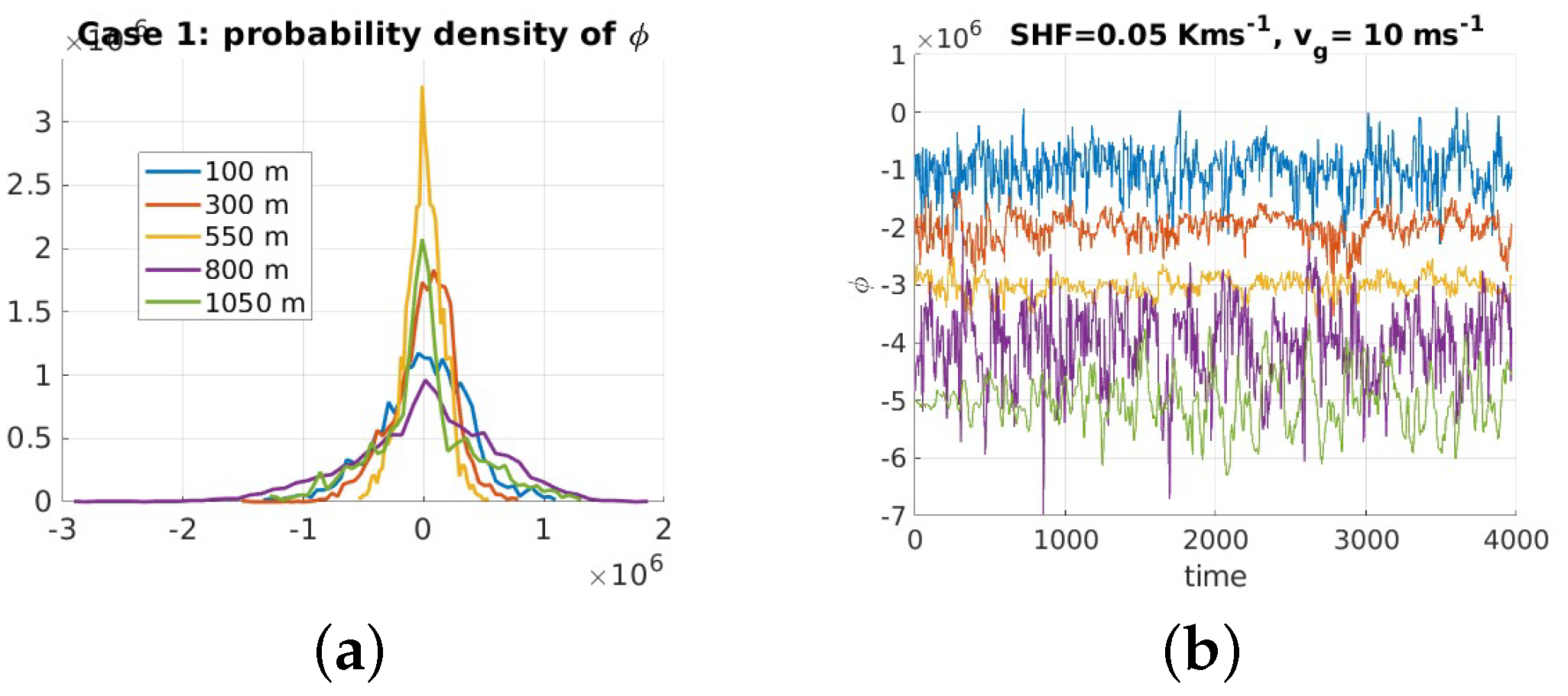

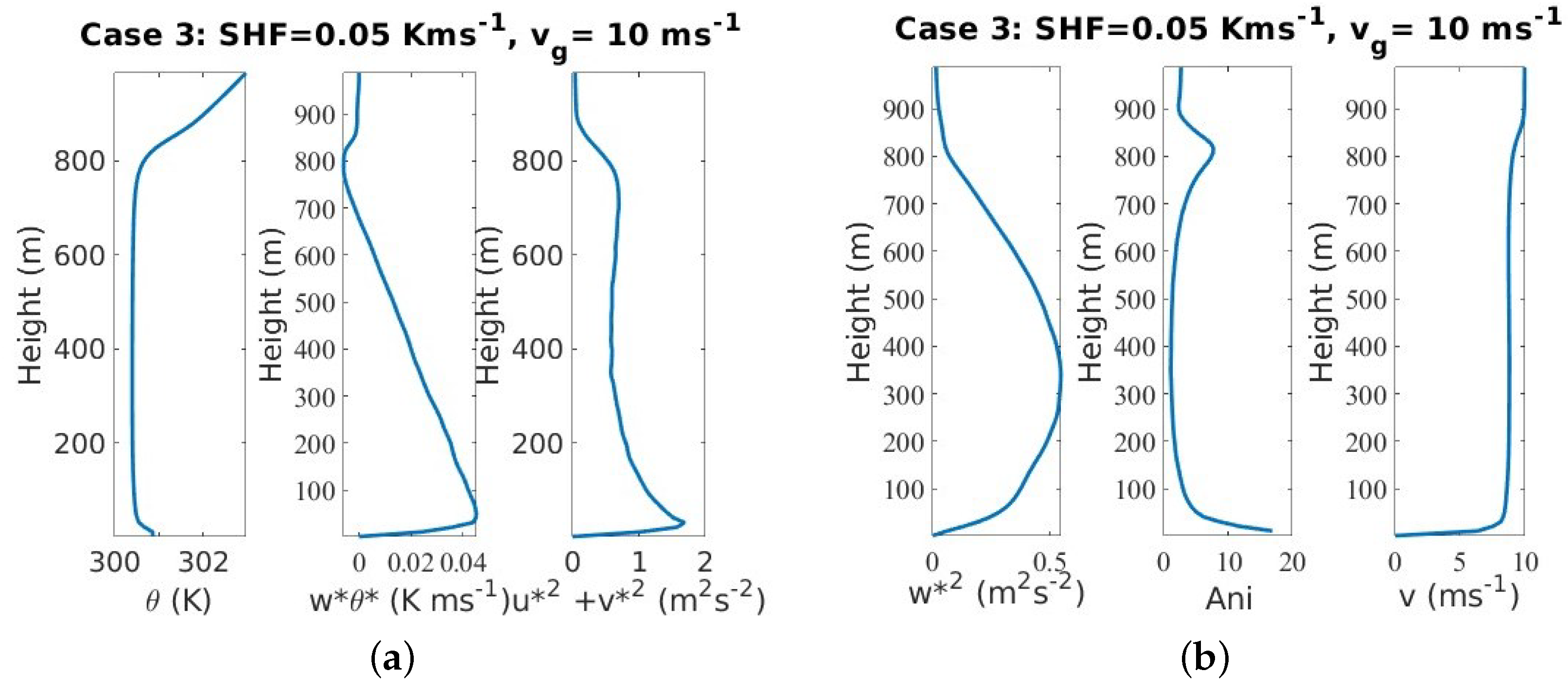
| Case | (K m s−1) | (m s−1) | Description |
|---|---|---|---|
| 1 | 0.05 | 5 | mixed: shear + buoyancy |
| 2 | 0.1 | 1 | buoyancy |
| 3 | 0.05 | 10 | shear |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kermarrec, G. On a Correlation Model for Laser Scanners: A Large Eddy Simulation Experiment. Remote Sens. 2024, 16, 3545. https://doi.org/10.3390/rs16193545
Kermarrec G. On a Correlation Model for Laser Scanners: A Large Eddy Simulation Experiment. Remote Sensing. 2024; 16(19):3545. https://doi.org/10.3390/rs16193545
Chicago/Turabian StyleKermarrec, Gaël. 2024. "On a Correlation Model for Laser Scanners: A Large Eddy Simulation Experiment" Remote Sensing 16, no. 19: 3545. https://doi.org/10.3390/rs16193545
APA StyleKermarrec, G. (2024). On a Correlation Model for Laser Scanners: A Large Eddy Simulation Experiment. Remote Sensing, 16(19), 3545. https://doi.org/10.3390/rs16193545






