Underwater Acoustic Scattering from Multiple Ice Balls at the Ice–Water Interface
Abstract
1. Introduction
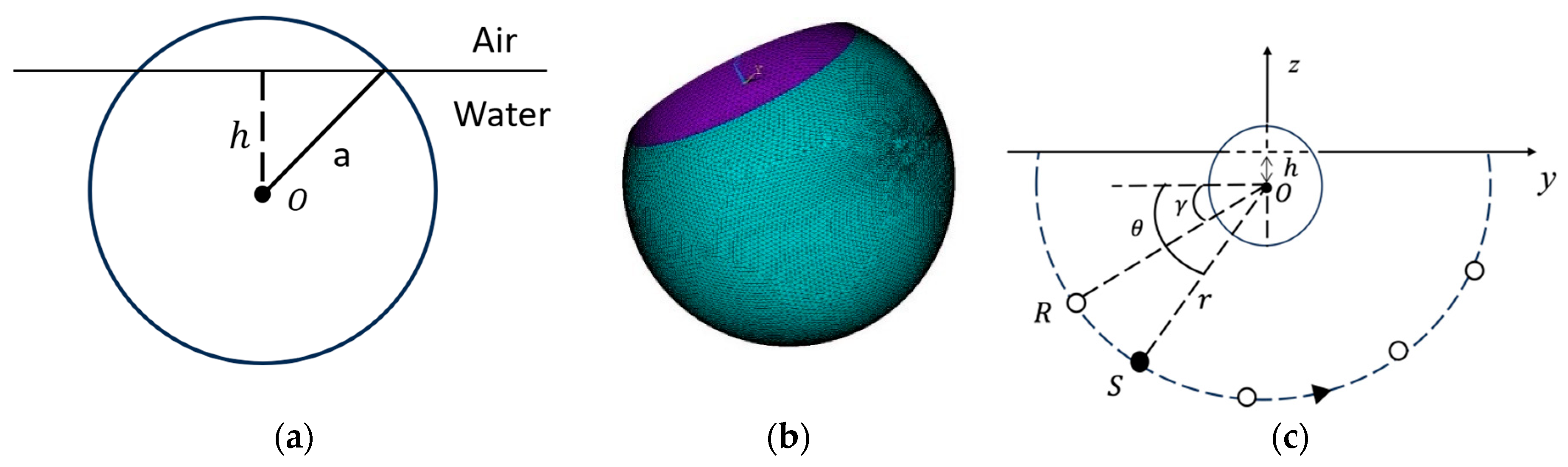
2. Single Ice Ball Model
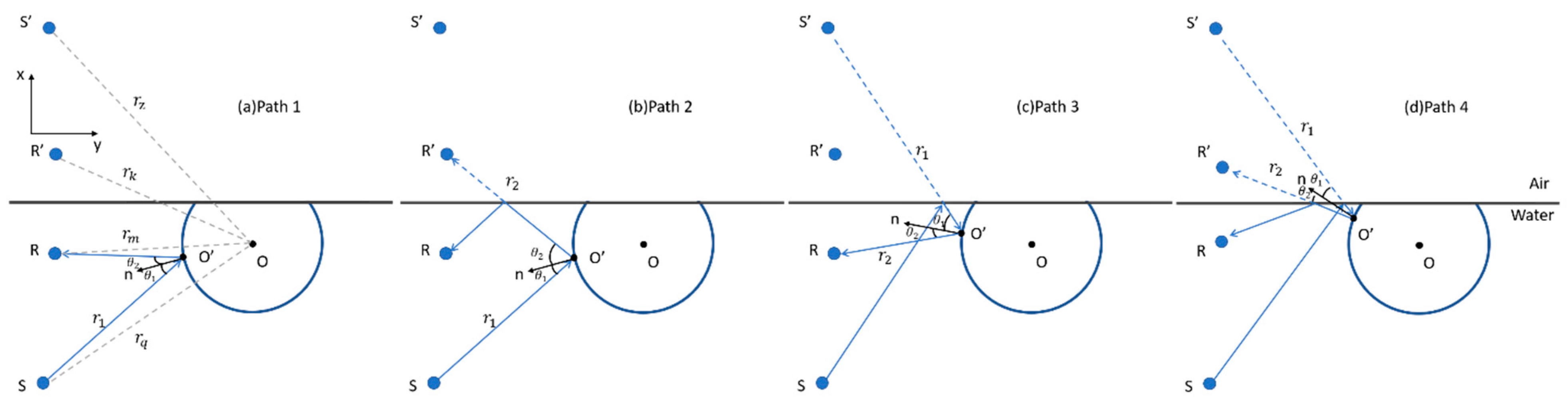
2.1. Acoustic Scattering under Ice–Water Interface with a Single Ice Ball
- Path 2: A sound wave from S initially reaches the water surface, then contacts the target surface following reflection off the water, and then reverberates back to S after the spherical reflection; alternatively, the sound wave from S first reaches the spherical surface of the target, and subsequently the water surface, before finally reaching S. Both trajectories exemplify double-station scattering from S to S’.
- Path 3: A sound wave from S is reflected by the water surface to reach the spherical surface of the ice ball. Subsequently, it is reflected from the spherical surface to the water surface and then back to S. This path represents a single-station echo of S’ relative to the ice ball.
- Path 1: considering the target echo in the free field without interface effect, that is, the sound wave from S reaches R after being reflected by the ice ball.
- Path 2: Starting from S, the wave reaches the ice ball surface, then the water surface, and finally R. This is equivalent to the path arriving at the mirror image point of the receiving position R’ through the water surface. The reflection coefficient is added due to the reflection at the water surface.
- Path 3: Similar to the path 2, is considered. The path can be viewed as the starting point at the S’ and reaching R after being reflected by the ice ball surface.
- Path 4: Both the incident and reflected waves from the ice ball are reflected by the water surface, which can be viewed as waves emitted from S’ to R’ after being reflected by the ice ball surface. Due to the twice reflections at the water surface, the interface reflection coefficient becomes a quadratic term .
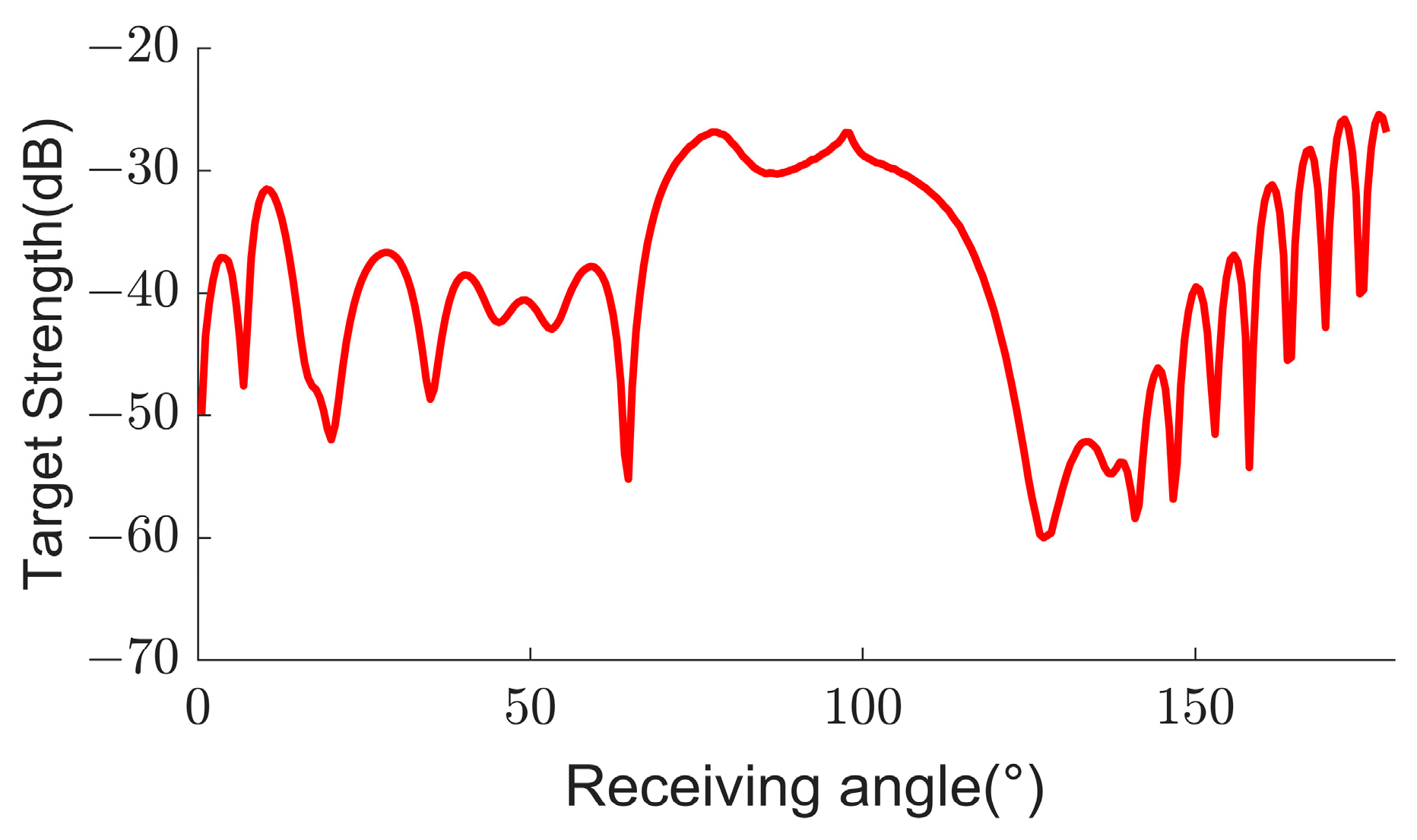
2.2. Simulation of the Signal Ice Ball Model
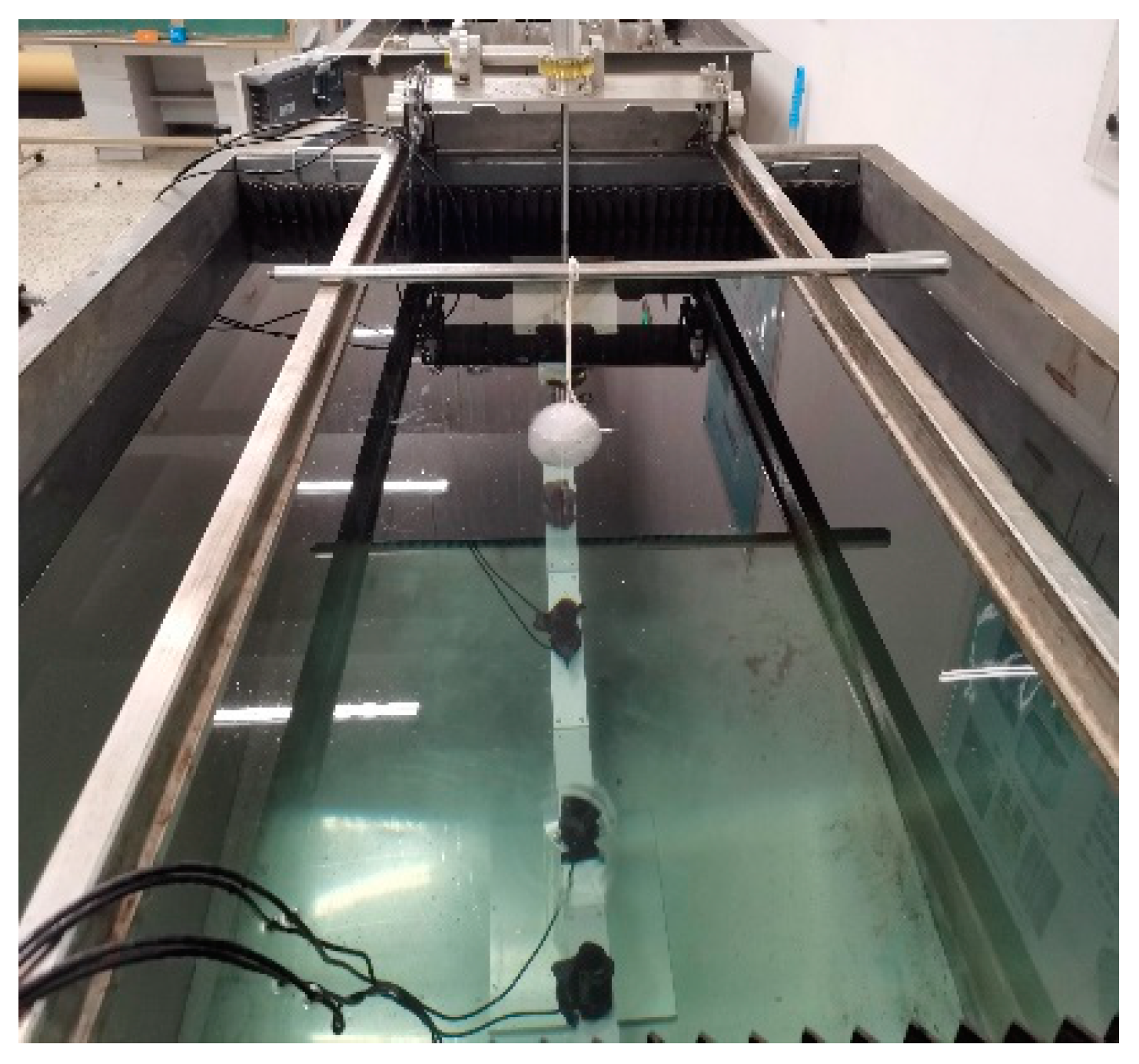
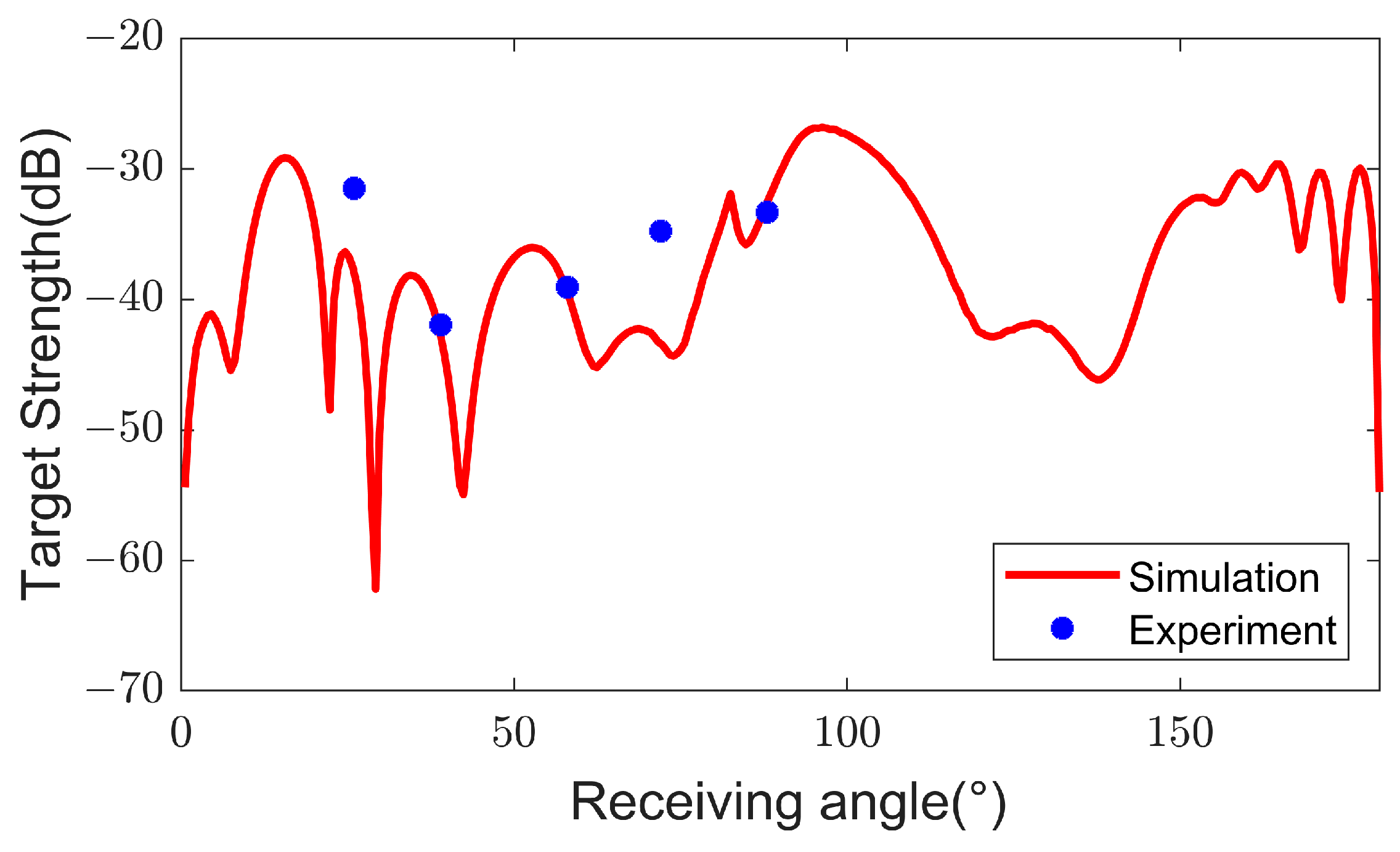
2.3. Water Tank Experiment
3. Acoustic Scattering under Ice Water Interface with Multiple Ice Balls
3.1. Theory of Multiple Ice Balls Model
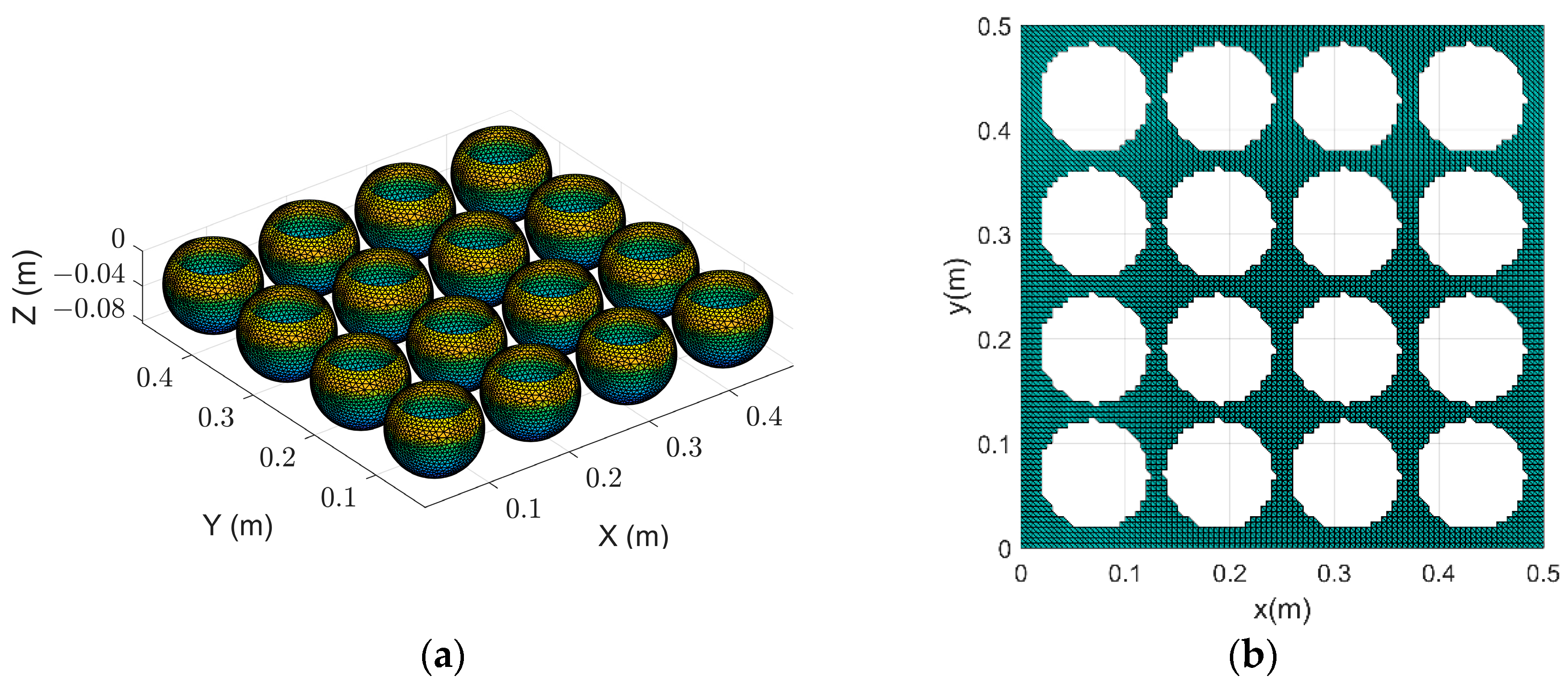
3.2. Simulation of Multiple Ice Balls Floating in Water
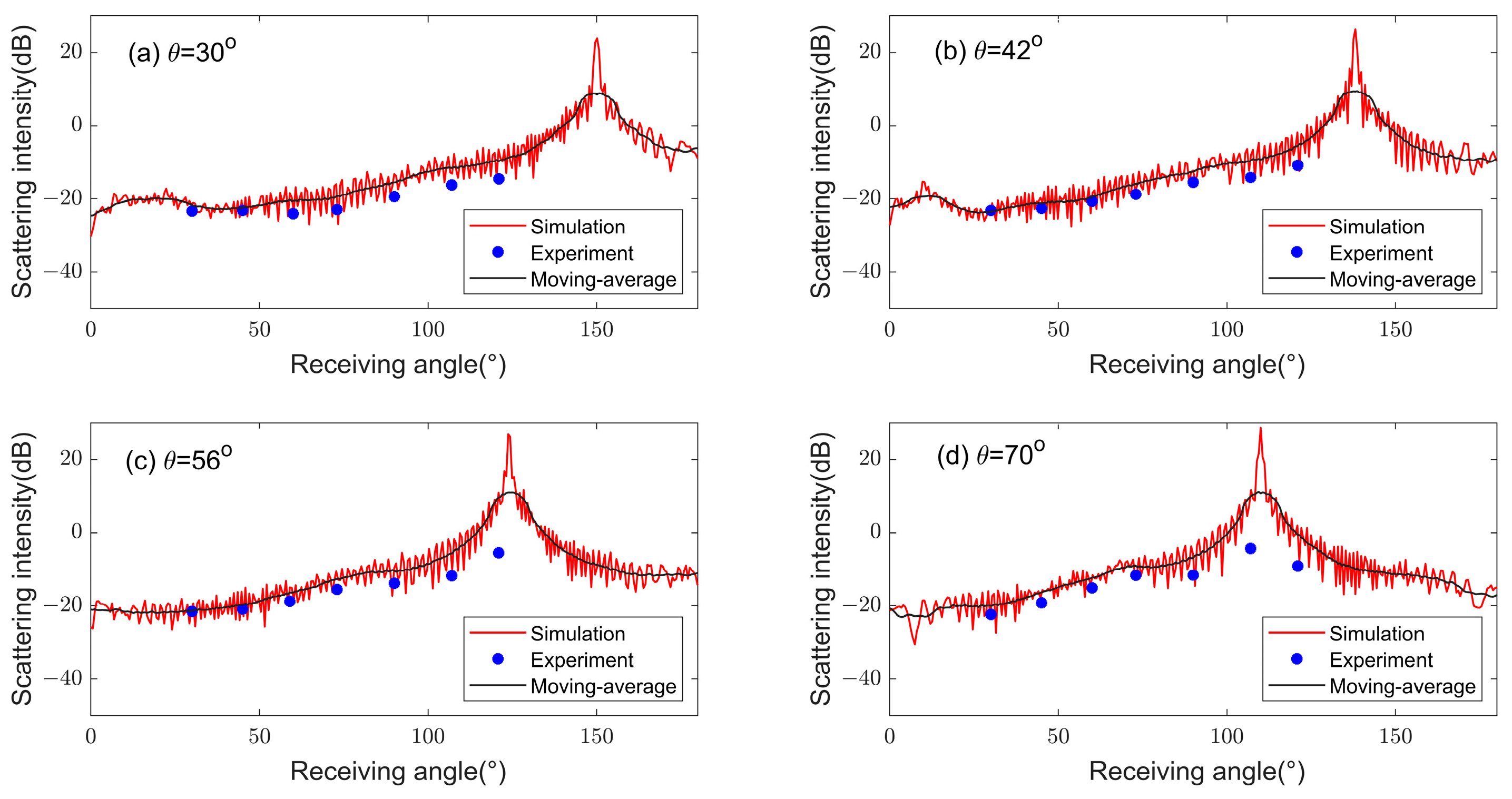
3.3. Experiments of Multiple Ice Balls Floating in a Water Tank
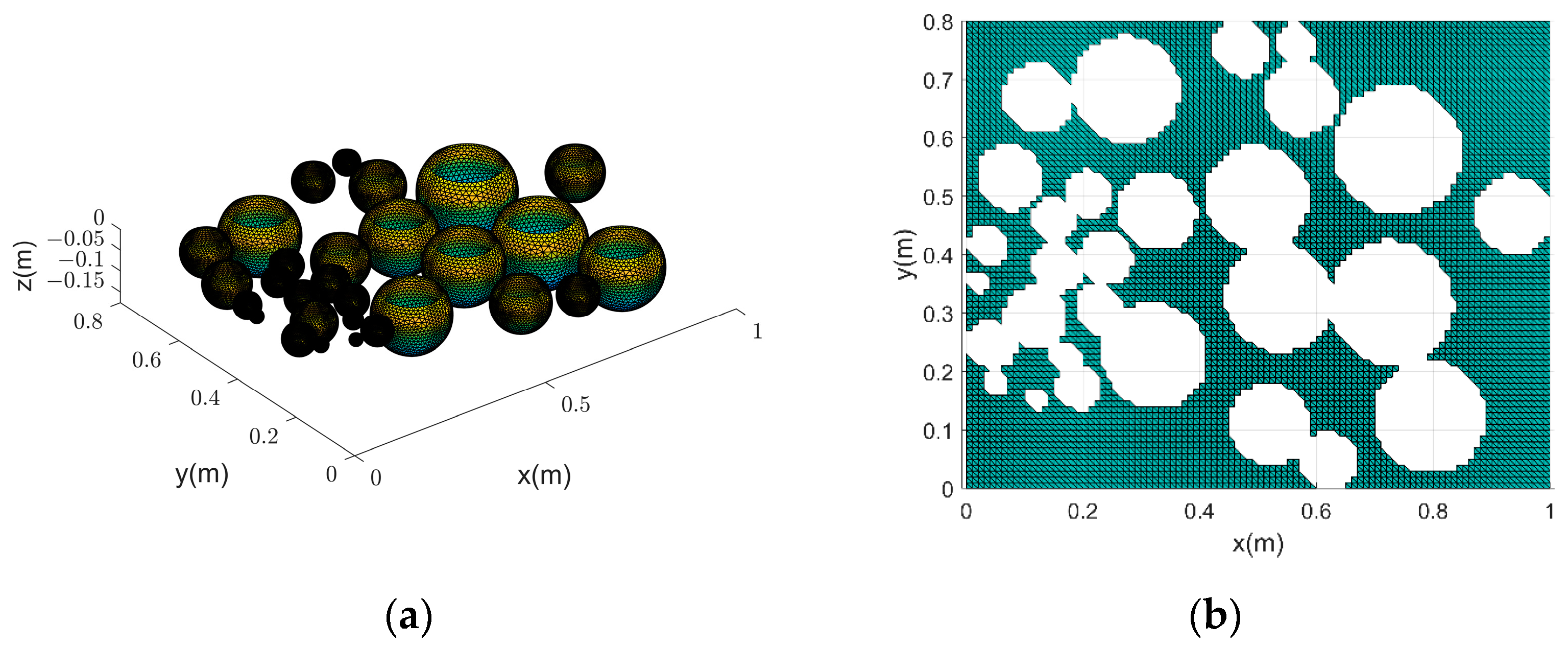
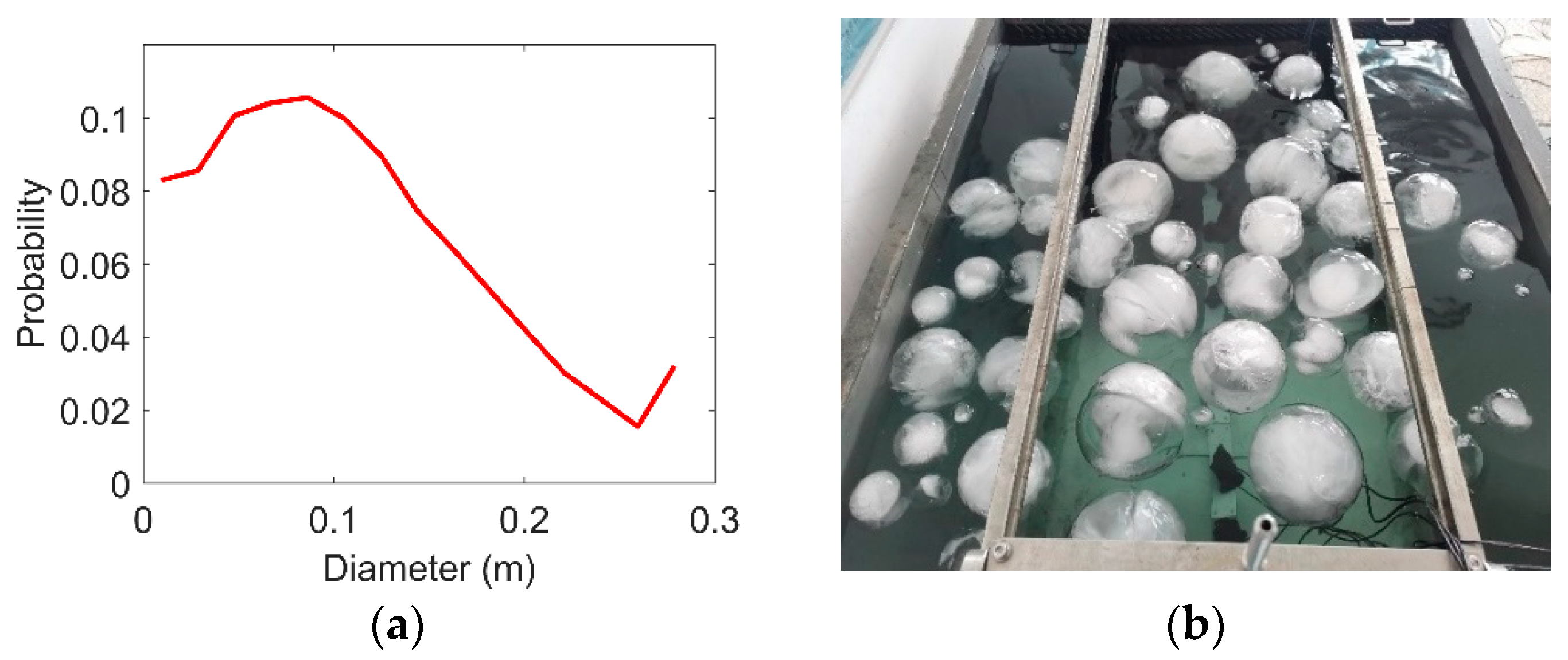
3.4. Experiments of Randomized Ice Balls Floating in a Water Tank
4. Discussion and Conclusions
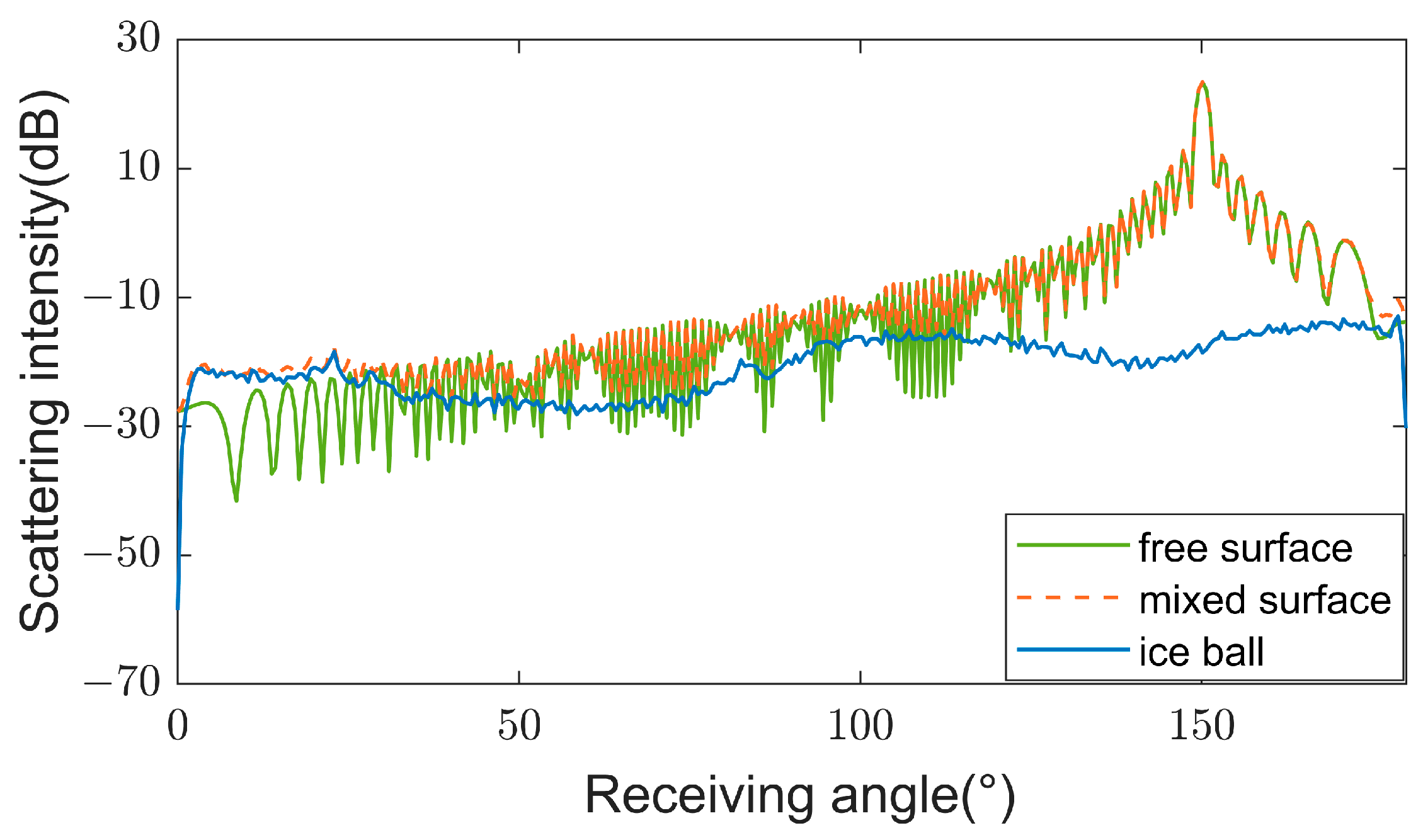
- The underwater acoustic field in the presence of ice floes on the water surface is discussed. The multiple scatterings between the ice balls and the free surface lead to a complex echo structure. We analyze the resulting acoustic field by selecting the primary contributors, such as the direct wave in the free field, the echo due to the virtual source, and two scattering waves between the ice ball and water surfaces in a single ice ball model.
- Based on the analysis of the scattering acoustic field, we establish a single ice ball model to calculate the target strength. The model is then extended to two cases with multiple ice balls in water: one model is established for uniformly distributed ice balls, and another model is established for non-uniformly distributed ice balls. The scattering intensity for the corresponding ice–water interfaces is calculated, including reflections from the surfaces of submerged ice balls and the free water surface. The results indicate that when the receiving angle is close to the incident acoustic wave’s mirror reflection angle, the scattering intensity positively correlates with the reflection component of the water surface.
- The scattering intensity measured in a water tank is used to validate the theoretical models. For the uniformly distributed ice–water interface, the average error over the receiving angles is found to be within 3 dB, thereby verifying the validity of the model while considering the analysis error. For the non-uniformly distributed ice–water interface, the experimental and simulation results show consistent trends, and the average error is also within 3 dB. The best agreement between the measured and simulated results occurs when the receiving angle is far from the mirror reflection angle, whereas larger errors are observed when the receiving angle is near the mirror reflection angle.
- The overall good agreement between the experimental and theoretical results supports the effectiveness of our methods for analyzing complex acoustic scattering in the presence of ice–water interfaces. We acknowledge the bias from non-uniform distribution and sizes of the ice balls from both modeling and experimental perspectives. From a modeling perspective, we simplified an ice ball as a sphere with resistance and simulated wave scattering in a semi-infinite domain. From an experimental perspective, ice balls cannot be precisely located in the water tank and may move or melt during the experiment. The ice balls randomly generated by the simulation are approximated by the limited number of molds used to freeze ice balls. Moreover, the number of experiments might be insufficient to obtain unbiased statistical variables.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Frequency | Transducer No. | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| 90 kHz | Sound source level (dB) | 179.48 | 178.46 | 178.14 | 178.02 |
| Frequency | Transducer No. | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| 90 kHz | Free field sensitivity (dB) | −201.68 | −197.02 | −199.33 | −200.27 |
| Transducer No. | 5 | 6 | 7 | 8 | |
| Free field sensitivity (dB) | −199.90 | −199.29 | −201.96 | −199.11 |
References
- Zhu, G.; Yin, J.; Chen, W.; Hu, S.; Zhou, H.; Guo, L. Modeling and characteristics of acoustic channel under typical arctic ice. Acoust. Acta Sin. 2017, 42, 152–158. [Google Scholar]
- Hutt, D. An overview of Arctic Ocean acoustics. In AIP Conference Proceedings; American Institute of Physics: College Park, MD, USA, 2012; Volume 1495. [Google Scholar]
- Morozov, A.K.; Altshuler, T.W.; Jones, T.C.P.; Freitag, L.E.; Koski, P.A.; Singh, S. Underwater acoustic technologies for long-range navigation and communications in the Arctic. In Proceedings of the International Conference on Underwater Acoustic Measurements, Kos, Greece, 20–24 June 2011; Papadakis, J.S., Bjorno, L., Eds.; Technologies & Results. 2011. [Google Scholar]
- Alexander, P.; Duncan, A.; Bose, N.; Smith, D. Modelling Acoustic Transmission Loss Due to Sea Ice Cover. Acoust. Aust. 2013, 41, 79–87. [Google Scholar]
- Freitag, L.; Koski, P.; Singh, S.; Maksym, T.; Singh, H. Acoustic communications under shallow shore-fast Arctic ice. In Proceedings of the OCEANS 2017-Anchorage, Anchorage, AK, USA, 18–21 September 2017; pp. 1–5. [Google Scholar]
- Mccammon, D.F.; Mcdaniel, S.T. The influence of the physical properties of ice on reflectivity. Acoust. Soc. Am. J. 1985, 77, 499–507. [Google Scholar] [CrossRef]
- Liu, S.; Li, Z. Reflection and scattering characteristics of sea ice on sound waves. Acta Phys. Sin. 2017, 66, 206–213. [Google Scholar]
- Lucieer, V.; Nau, A.W.; Forrest, A.L.; Hawes, I. Fine-Scale Sea Ice Structure Characterized Using Underwater Acoustic Methods. Remote Sens. 2016, 8, 821. [Google Scholar] [CrossRef]
- Jiang, M.; Chen, X.; Xu, L.; Clausi, D.A. IceGCN: An Interactive Sea Ice Classification Pipeline for SAR Imagery Based on Graph Convolutional Network. Remote Sens. 2024, 16, 2301. [Google Scholar] [CrossRef]
- Garrison, G.; Early, E. Acoustic backscattering from uniform Arctic Sea ice. In Proceedings of the IEEE International Conference on Engineering in the Ocean Environment, Halifax, NS, Canada, 21–23 August 1974. [Google Scholar]
- Ito, M.; Ohshima, K.I.; Fukamachi, Y.; Mizuta, G.; Kusumoto, Y.; Nishioka, J. Observations of frazil ice formation and upward sediment transport in the Sea of Okhotsk: A possible mechanism of iron supply to sea ice. J. Geophys. Res. Ocean. 2017, 122, 788–802. [Google Scholar] [CrossRef]
- Huang, H.; Gaunaurd, G. Exact analysis of the acoustic scattering by an arbitrarily insonified spherical body near a flat boundary. J. Acoust. Soc. Am. 1994, 95, 2946. [Google Scholar] [CrossRef]
- Huang, H.; Gaunaurd, G. Scattering of a plane acoustic wave by a spherical elastic shell near a free surface. Int. J. Solids Struct. 1997, 34, 591–602. [Google Scholar] [CrossRef]
- Gaunaurd, G.; Huang, H. Sound scattering by bubble clouds near the sea surface. J. Acoust. Soc. Am. 2000, 107, 95–102. [Google Scholar] [CrossRef] [PubMed]
- Richardson, M.E.; Briggs, K.B.; Williams, K.L.; Jackson, D.R.; Naval Research Lab Stennis Space Center Ms Marine Geosciences Div. Scattering of High-Frequency Acoustic Energy from Discrete Scatterers on the Seafloor: Glass Spheres and Shells. Proc.-Inst. Acoust. 2001, 23, 369–374. [Google Scholar]
- Kungl, A.F.; Schumayer, D.; Frazer, E.K.; Langhorne, P.J.; Leonard, G.H. An oblate spheroidal model for multi-frequency acoustic back-scattering of frazil ice. Cold Reg. Sci. Technol. 2020, 177, 103122. [Google Scholar] [CrossRef]
- Simon, B.; Isakson, M.; Ballard, M. Modeling acoustic wave propagation and reverberation in an ice covered environment using finite element analysis. In Proceedings of the Meetings on Acoustics 175ASA, Minneapolis, MN, USA, 7–11 May 2018; Acoustical Society of America: Melville, NY, USA, 2018; Volume 33, p. 070002. [Google Scholar]
- Chen, W.; Hu, S.; Wang, Y.; Yin, J.; Korochensev, V.I.; Zhang, Y. Study on acoustic reflection characteristics of layered sea ice based on boundary condition method. Waves Random Complex Media 2021, 31, 2177–2196. [Google Scholar] [CrossRef]
- Chen, W.; Sun, H.; Wang, W. Study on calculation method of target strength in waveguide. In Proceedings of the 2016 IEEE/OES China Ocean Acoustics (COA), Harbin, China, 9–11 January 2016; pp. 1–4. [Google Scholar]
- Peng, X. The effects of the sail on acoustic scattering characteristics of the underwater structure. In Proceedings of the INTER-NOISE and NOISE-CON Congress and Conference, New Orleans, LA, USA, 29 June–1 July 2020; Institute of Noise Control Engineering: Wakefield, MA, USA, 2020; Volume 261, pp. 4382–4388. [Google Scholar]
- Abawi, A.T. Kirchhoff scattering from underwater targets modeled as an assembly of triangular facets. J. Acoust. Soc. Am. 2009, 125, 2608. [Google Scholar] [CrossRef]
- Lavia, E.F.; Gonzalez, J.D.; Blanc, S. Modeling high-frequency backscattering from a mesh of curved surfaces using Kirchhoff Approximation. J. Theor. Comput. Acoust. 2019, 27, 1850057. [Google Scholar] [CrossRef]
- Seybert, A.F.; Wu, T.W. Modified Helmholtz integral equation for bodies sitting on an infinite plane. J. Acoust. Soc. Am. 1989, 85, 19–23. [Google Scholar] [CrossRef]
- Tang, W. Calculation of acoustic scattering from object near an interface using physical acoustic method. Acta Acust.-Peking- 1999, 24, 1–5. [Google Scholar]
- Williams, K.L.; Marston, P.L. Synthesis of backscattering from an elastic sphere using the Sommerfeld–Watson transformation and giving a Fabry–Perot analysis of resonances. J. Acoust. Soc. Am. 1986, 79, 1702–1708. [Google Scholar] [CrossRef]
- Gurcan, M.; Creasey, D.J.; Gazey, B.K. Development of a sonar to measure the size of sea-bed material. In Proceedings of the Acoustics and the Sea-Bed Conference, Bath, UK, 6–8 April 1983; pp. 357–365. [Google Scholar]


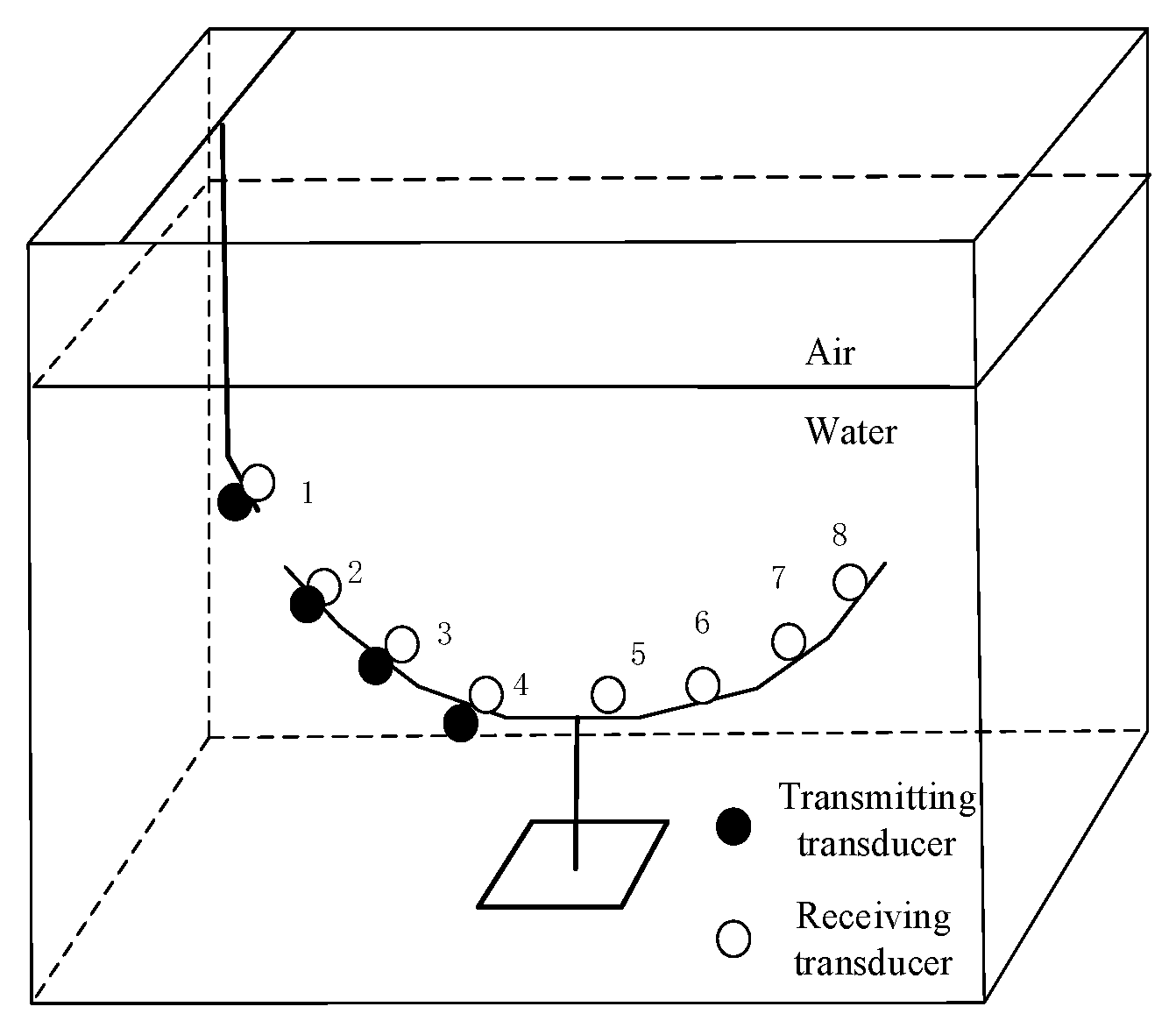


| Transducers | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Angle (°) | 30 | 45 | 60 | 73 | 90 |
| Distance (m) | 0.65 | 0.75 | 0.80 | 0.81 | 0.80 |
| (°) | 30 | 42 | 56 | 70 |
| Error (dB) | 3.1 | 2.13 | 3.68 | 2.92 |
| Diameter (cm) | 3 | 5 | 6 | 8 | 9 | 10 | 12 |
| Amount | 5 | 5 | 6 | 6 | 7 | 6 | 6 |
| Diameter (cm) | 14 | 16 | 18 | 20 | 22 | 24 | 26 |
| Amount | 5 | 4 | 3 | 3 | 3 | 2 | 4 |
| Grazing angle (°) | 30 | 42 | 56 | 70 |
| Error (dB) | 2.64 | 1.83 | 3.64 | 3.73 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, S.; Chen, W.; Sun, H.; Zhou, S.; Yin, J. Underwater Acoustic Scattering from Multiple Ice Balls at the Ice–Water Interface. Remote Sens. 2024, 16, 3113. https://doi.org/10.3390/rs16173113
Hu S, Chen W, Sun H, Zhou S, Yin J. Underwater Acoustic Scattering from Multiple Ice Balls at the Ice–Water Interface. Remote Sensing. 2024; 16(17):3113. https://doi.org/10.3390/rs16173113
Chicago/Turabian StyleHu, Siwei, Wenjian Chen, Hui Sun, Shunbo Zhou, and Jingwei Yin. 2024. "Underwater Acoustic Scattering from Multiple Ice Balls at the Ice–Water Interface" Remote Sensing 16, no. 17: 3113. https://doi.org/10.3390/rs16173113
APA StyleHu, S., Chen, W., Sun, H., Zhou, S., & Yin, J. (2024). Underwater Acoustic Scattering from Multiple Ice Balls at the Ice–Water Interface. Remote Sensing, 16(17), 3113. https://doi.org/10.3390/rs16173113






