A Phase-Only Optimization Null Control Method for FDA-MIMO Based on ADMM
Abstract
1. Introduction
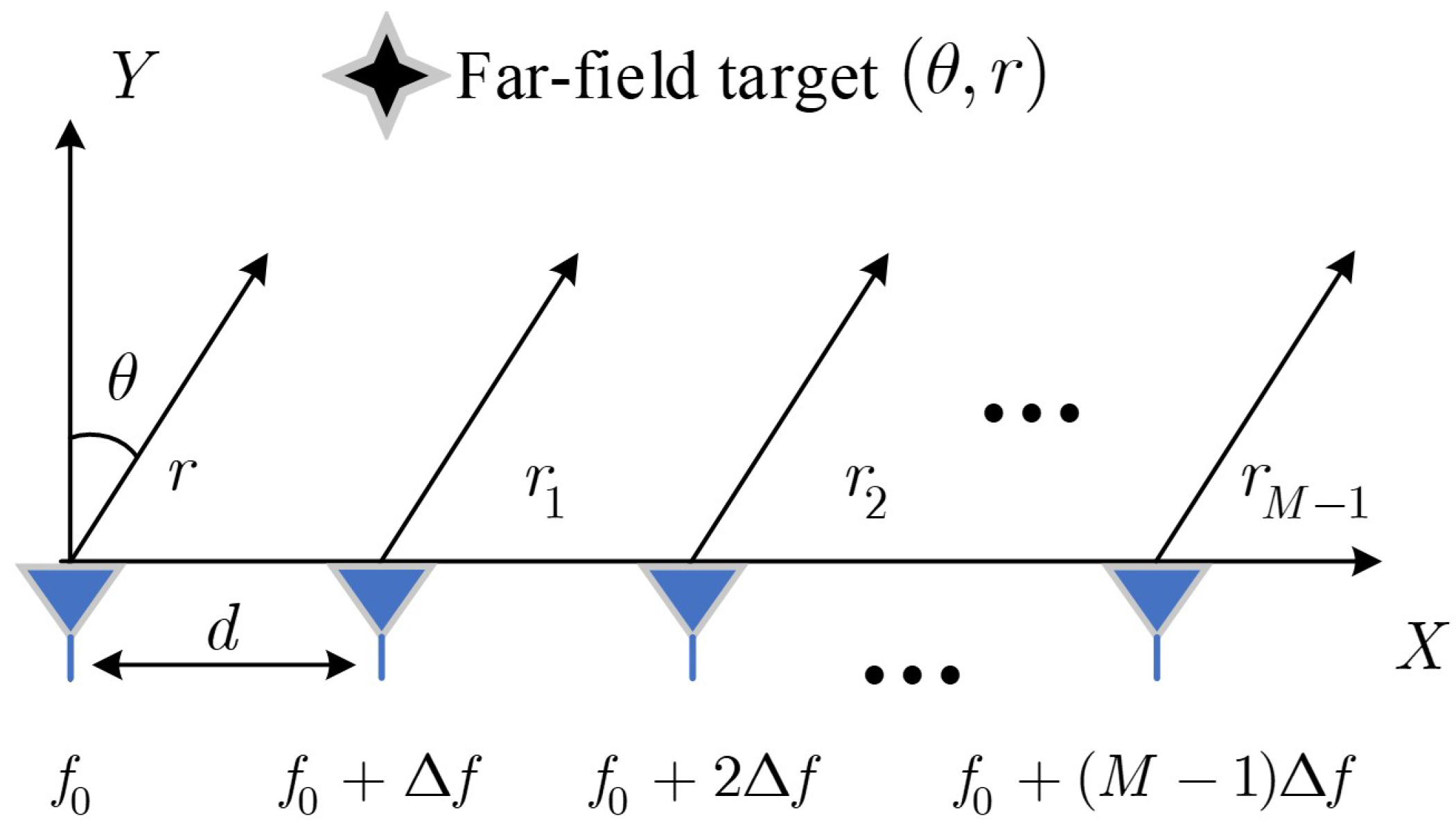
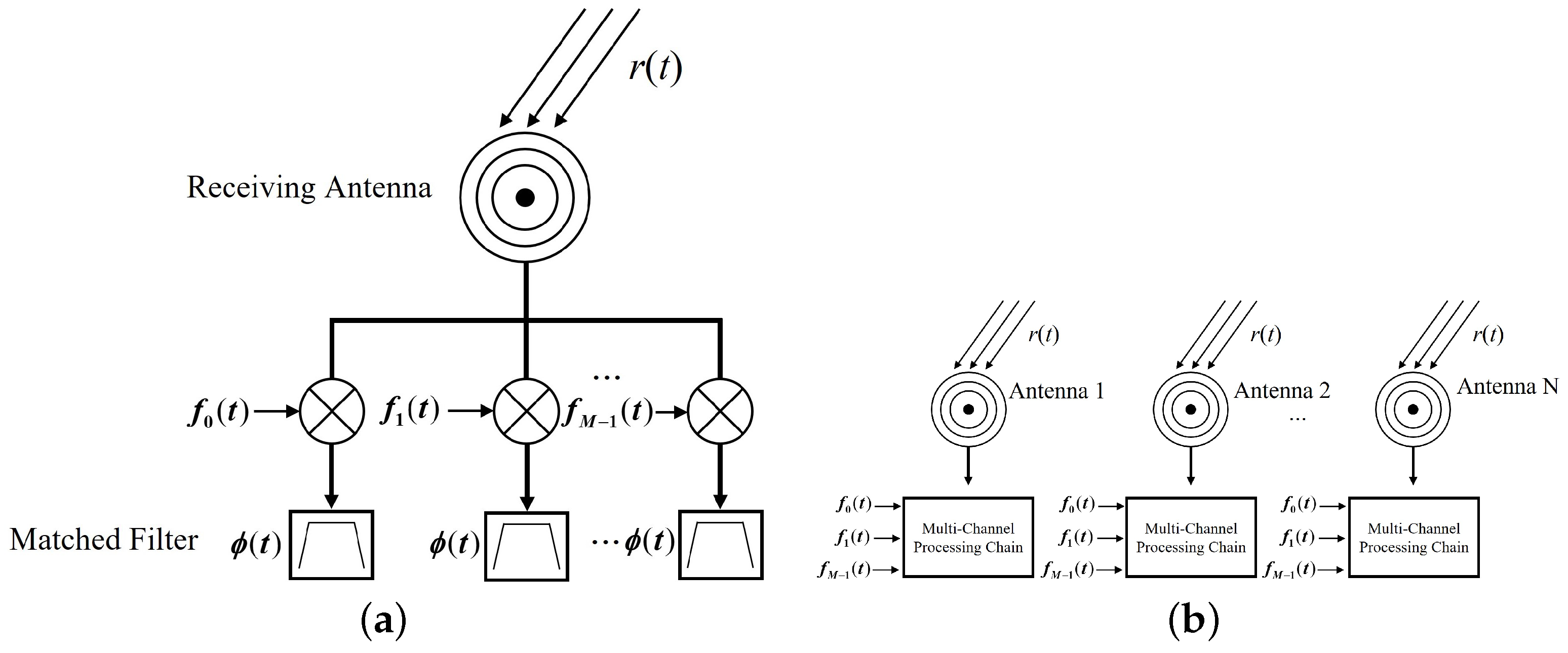
2. FDA-MIMO Transmit–Receive Signal Model
3. Null Control for FDA-MIMO Transmit–Receive Beampattern
3.1. Problem Statement
3.2. Solution for Based on ADMM
3.3. Solution for Based on DADMM
Algorithm Summary and Computational Complexity Analysis
| Algorithm 1 Algorithm of ADMM |
| Input: M, N, , , , , , , , , Output: |
| Algorithm 2 Algorithm of DADMM |
Input:
M, N, , , , , , , , , Output:
|
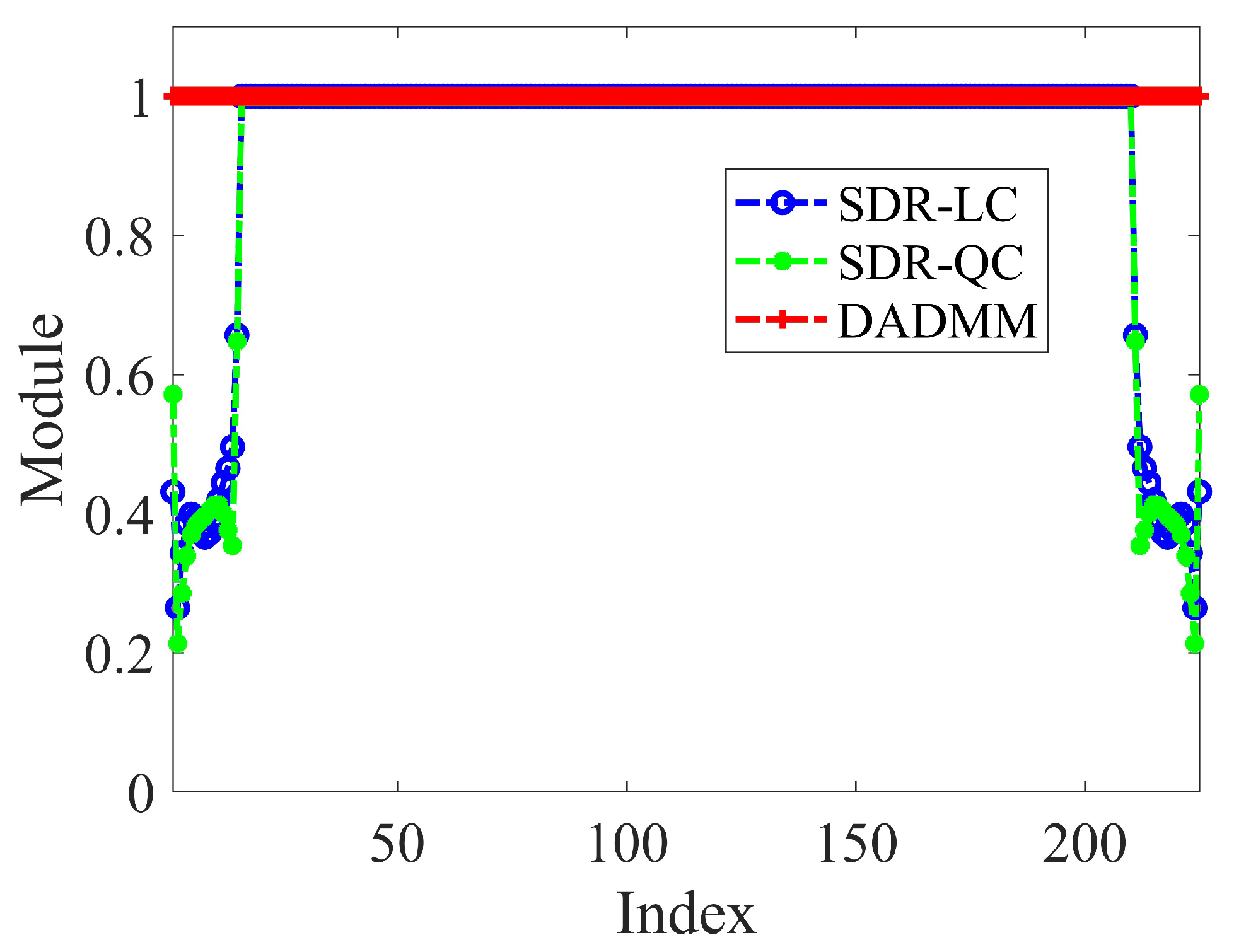
4. Simulation Results
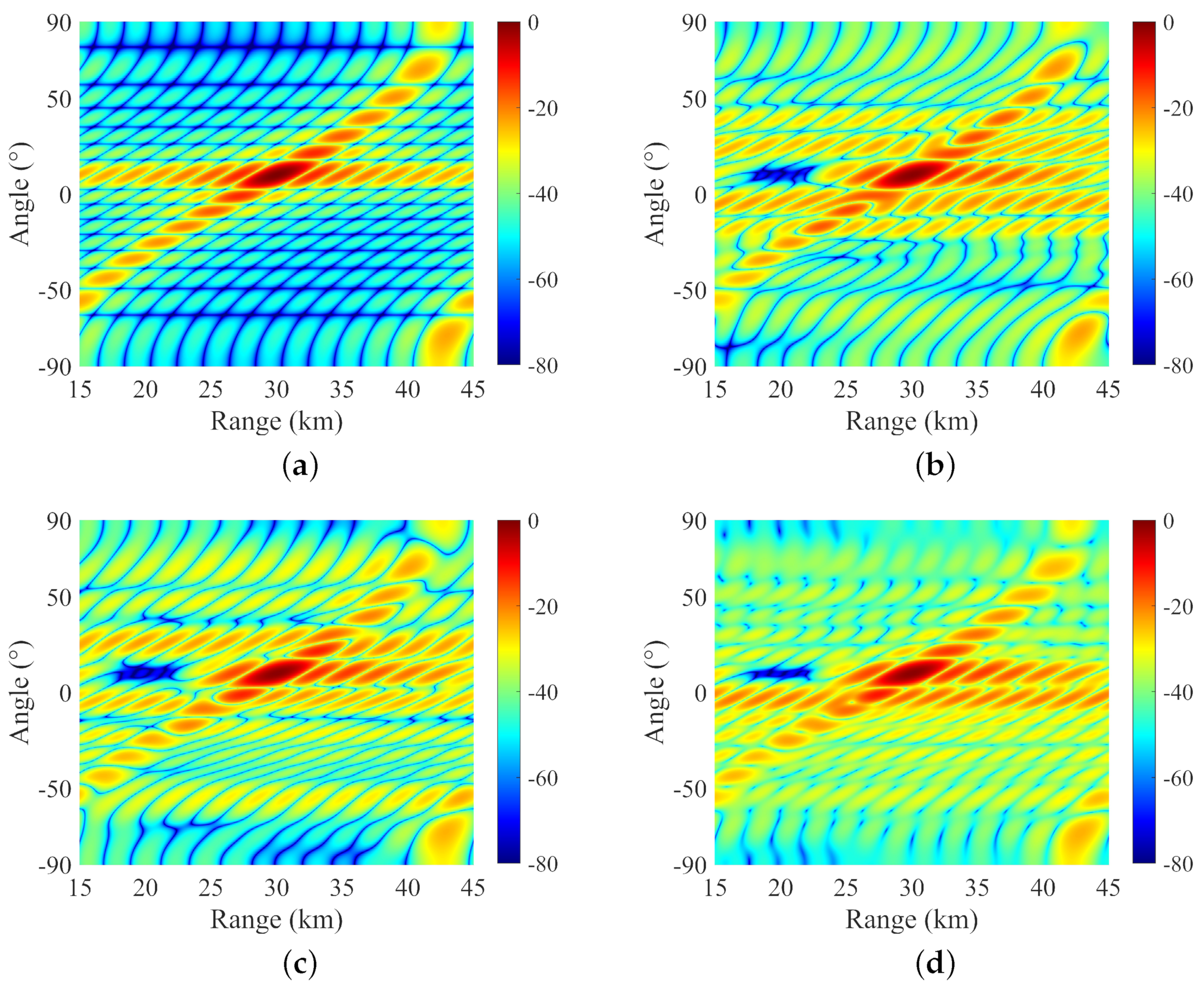
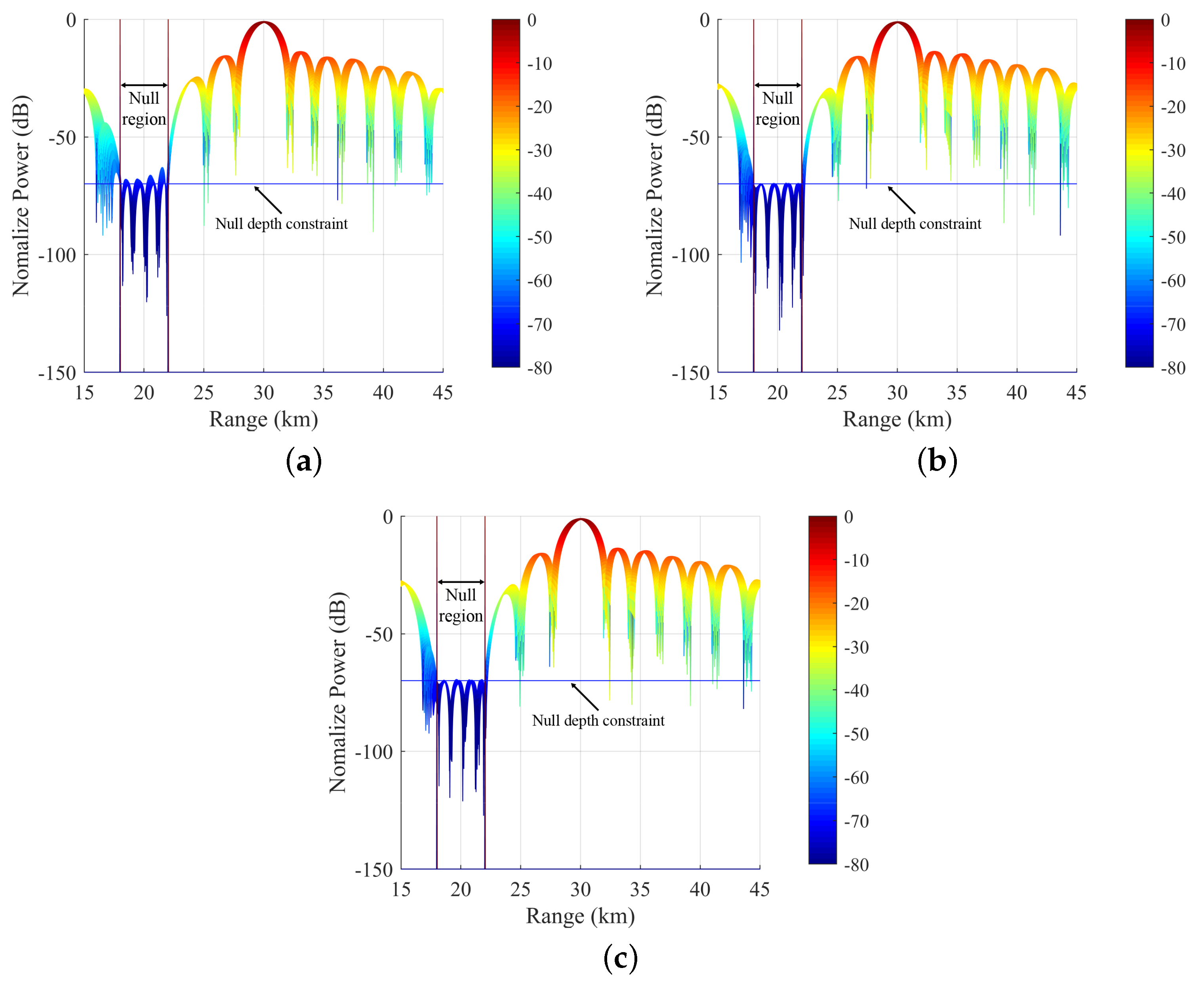
4.1. Given Null Depth Constraints to Maximize the Main Lobe Gain ( Based on ADMM)
4.2. Maximizing Null Depth under Given Main Lobe Gain Constraint ( Based on DADMM)
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Steyskal, H.; Shore, R.; Haupt, R. Methods for null control and their effects on the radiation pattern. IEEE Trans. Antennas Propag. 1986, 34, 404–409. [Google Scholar] [CrossRef]
- Lan, L.; Liao, L.; Xu, J.; Zhu, S.; Zeng, C.; Zhang, Y. Control and utilization of range-dependent beampattern with waveform diverse array radars. Chin. J. Aeronaut. 2022, 35, 1–31. [Google Scholar] [CrossRef]
- Liu, Z.; Zhao, S.; Zhang, G.; Jiao, B. Robust adaptive beamforming for sidelobe canceller with null widening. IEEE Sensors J. 2019, 19, 11213–11220. [Google Scholar] [CrossRef]
- Liu, Z.; Zhao, S.; Zhang, C.; Zhang, G. Flexible robust adaptive beamforming method with null widening. IEEE Sensors J. 2021, 21, 10579–10586. [Google Scholar] [CrossRef]
- Zhang, L.; Li, B.; Huang, L.; Kirubarajan, T.; So, H.C. Robust minimum dispersion distortionless response beamforming against fast-moving interferences. Signal Process. 2017, 140, 190–197. [Google Scholar] [CrossRef]
- Amar, A.; Doron, M.A. A linearly constrained minimum variance beamformer with a pre-specified suppression level over a pre-defined broad null sector. Signal Process. 2015, 109, 165–171. [Google Scholar] [CrossRef]
- Mao, X.; Li, W.; Li, Y.; Sun, Y.; Zhai, Z. Robust adaptive beamforming against signal steering vector mismatch and jammer motion. Int. J. Antennas Propag. 2015, 2015, 780296. [Google Scholar] [CrossRef]
- Antonik, P.; Wicks, M.C.; Griffiths, H.D.; Baker, C.J. Frequency diverse array radars. In Proceedings of the 2006 IEEE Conference on Radar, Verona, NY, USA, 24–27 April 2006; p. 3. [Google Scholar]
- Wang, W.Q. Frequency diverse array antenna: New opportunities. IEEE Antennas Propag. Mag. 2015, 57, 145–152. [Google Scholar] [CrossRef]
- Tan, M.; Wang, C.; Li, Z. Correction analysis of frequency diverse array radar about time. IEEE Trans. Antennas Propag. 2020, 69, 834–847. [Google Scholar] [CrossRef]
- Gong, P.; Wang, W.Q.; Li, F.; So, H.C. Sparsity-aware transmit beamspace design for FDA-MIMO radar. Signal Process. 2018, 144, 99–103. [Google Scholar] [CrossRef]
- Wen, C.; Huang, Y.; Peng, J.; Wu, J.; Zheng, G.; Zhang, Y. Slow-time FDA-MIMO technique with application to STAP radar. IEEE Trans. Aerosp. Electron. Syst. 2021, 58, 74–95. [Google Scholar] [CrossRef]
- Huang, B.; Basit, A.; Gui, R.; Wang, W.Q. Adaptive moving target detection without training data for FDA-MIMO radar. IEEE Trans. Veh. Technol. 2021, 71, 220–232. [Google Scholar] [CrossRef]
- Basit, A.; Wang, W.Q.; Wali, S.; Nusenu, S.Y. Transmit beamspace design for FDA–MIMO radar with alternating direction method of multipliers. Signal Process. 2021, 180, 107832. [Google Scholar] [CrossRef]
- Xu, Y.; Luk, K.M. Enhanced transmit–receive beamforming for frequency diverse array. IEEE Trans. Antennas Propag. 2020, 68, 5344–5352. [Google Scholar] [CrossRef]
- Xu, Y.; Wang, A.; Xu, J. Range–Angle Transceiver Beamforming Based on Semicircular-FDA Scheme. IEEE Trans. Aerosp. Electron. Syst. 2021, 58, 834–843. [Google Scholar] [CrossRef]
- Wang, C.; Zhu, X.; Huang, S. Coherent receiver design and aperture expansion beamforming for frequency diverse array radar. Digit. Signal Process. 2022, 128, 103631. [Google Scholar] [CrossRef]
- Gui, R.; Wang, W.Q.; Cui, C.; So, H.C. Coherent pulsed-FDA radar receiver design with time-variance consideration: SINR and CRB analysis. IEEE Trans. Signal Process. 2017, 66, 200–214. [Google Scholar] [CrossRef]
- Lan, L.; Rosamilia, M.; Aubry, A.; De Maio, A.; Liao, G. FDA-MIMO Transceiver Design under the Uniform Frequency Increment Constraint. IEEE Trans. Radar Syst. 2024, 2, 446–459. [Google Scholar] [CrossRef]
- Liao, Y.; Tang, H.; Chen, X.; Wang, W.Q.; Xing, M.; Zheng, Z.; Wang, J.; Liu, Q.H. Antenna beampattern with range null control using weighted frequency diverse array. IEEE Access 2020, 8, 50107–50117. [Google Scholar] [CrossRef]
- Ding, Z.; Xie, J.; Wang, B.; Zhang, H. Robust adaptive null broadening method based on FDA-MIMO radar. IEEE Access 2020, 8, 177976–177983. [Google Scholar] [CrossRef]
- Lan, L.; Xu, J.; Liao, G.; Zhang, Y.; Fioranelli, F.; So, H.C. Suppression of mainbeam deceptive jammer with FDA-MIMO radar. IEEE Trans. Veh. Technol. 2020, 69, 11584–11598. [Google Scholar] [CrossRef]
- Lan, L.; Liao, G.; Xu, J.; Zhang, Y.; Liao, B. Transceive beamforming with accurate nulling in FDA-MIMO radar for imaging. IEEE Trans. Geosci. Remote. Sens. 2020, 58, 4145–4159. [Google Scholar] [CrossRef]
- Lan, L.; Liao, G.; Xu, J.; Zhu, S.; Zhang, Y. Range-angle-dependent beamforming for FDA-MIMO radar using oblique projection. Sci. China Inf. Sci. 2022, 65, 152305. [Google Scholar] [CrossRef]
- Smith, S.T. Optimum phase-only adaptive nulling. IEEE Trans. Signal Process. 1999, 47, 1835–1843. [Google Scholar] [CrossRef]
- Kajenski, P.J. Phase only antenna pattern notching via a semidefinite programming relaxation. IEEE Trans. Antennas Propag. 2012, 60, 2562–2565. [Google Scholar] [CrossRef]
- Pallotta, L.; Farina, A.; Smith, S.T.; Giunta, G. Phase-only space-time adaptive processing. IEEE Access 2021, 9, 147250–147263. [Google Scholar] [CrossRef]
- Tan, M.; Wang, C. Reply to Comments on “Correction Analysis of ‘Frequency Diverse Array Radar about Time’”. IEEE Trans. Antennas Propag. 2022, 71, 2899–2902. [Google Scholar] [CrossRef]
- Chen, G.; Wang, C.; Gong, J.; Tan, M.; Liu, Y. Data-Independent Phase-Only Beamforming of FDA-MIMO Radar for Swarm Interference Suppression. Remote Sens. 2023, 15, 1159. [Google Scholar] [CrossRef]
- Boyd, S.; Parikh, N.; Chu, E.; Peleato, B.; Eckstein, J. Distributed optimization and statistical learning via the alternating direction method of multipliers. Found. Trends® Mach. Learn. 2011, 3, 1–122. [Google Scholar]
- Karush, W. Minima of Functions of Several Variables with Inequalities as Side Constraints. Master’s Thesis, Department of Mathematics, The University of Chicago, Chicago, IL, USA, 1939. [Google Scholar]
- Luo, Z.Q.; Ma, W.K.; So, A.M.C.; Ye, Y.; Zhang, S. Semidefinite relaxation of quadratic optimization problems. IEEE Signal Process. Mag. 2010, 27, 20–34. [Google Scholar] [CrossRef]
- He, X.; Wang, J. QCQP with extra constant modulus constraints: Theory and application to SINR constrained mmwave hybrid beamforming. IEEE Trans. Signal Process. 2022, 70, 5237–5250. [Google Scholar] [CrossRef]
- Liu, Y.; Jiu, B.; Liu, H. ADMM-based transmit beampattern synthesis for antenna arrays under a constant modulus constraint. Signal Process. 2020, 171, 107529. [Google Scholar] [CrossRef]
- Aubry, A.; De Maio, A.; Carotenuto, V.; Farina, A. Radar phase noise modeling and effects-part I: MTI filters. IEEE Trans. Aerosp. Electron. Syst. 2016, 52, 698–711. [Google Scholar] [CrossRef]
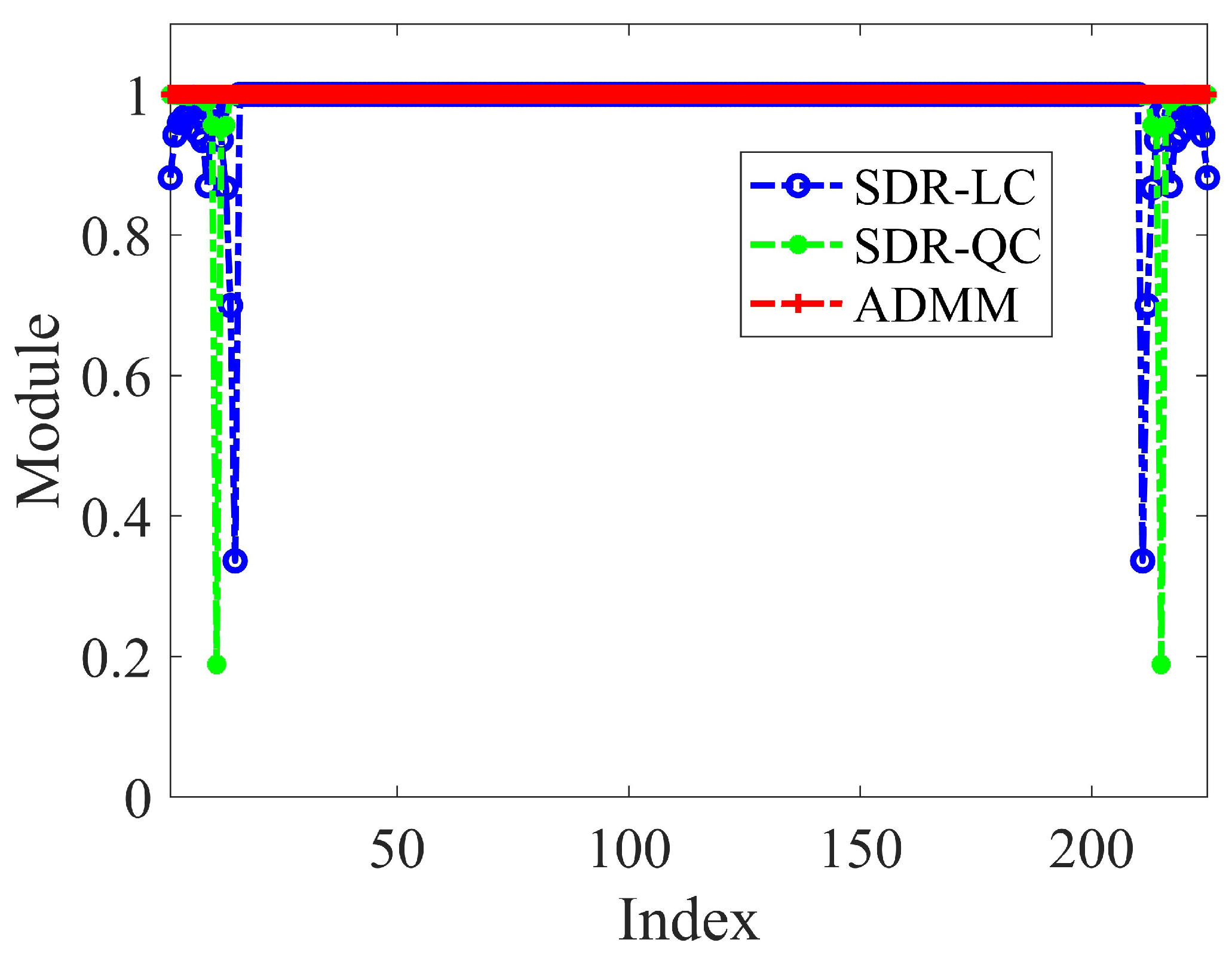
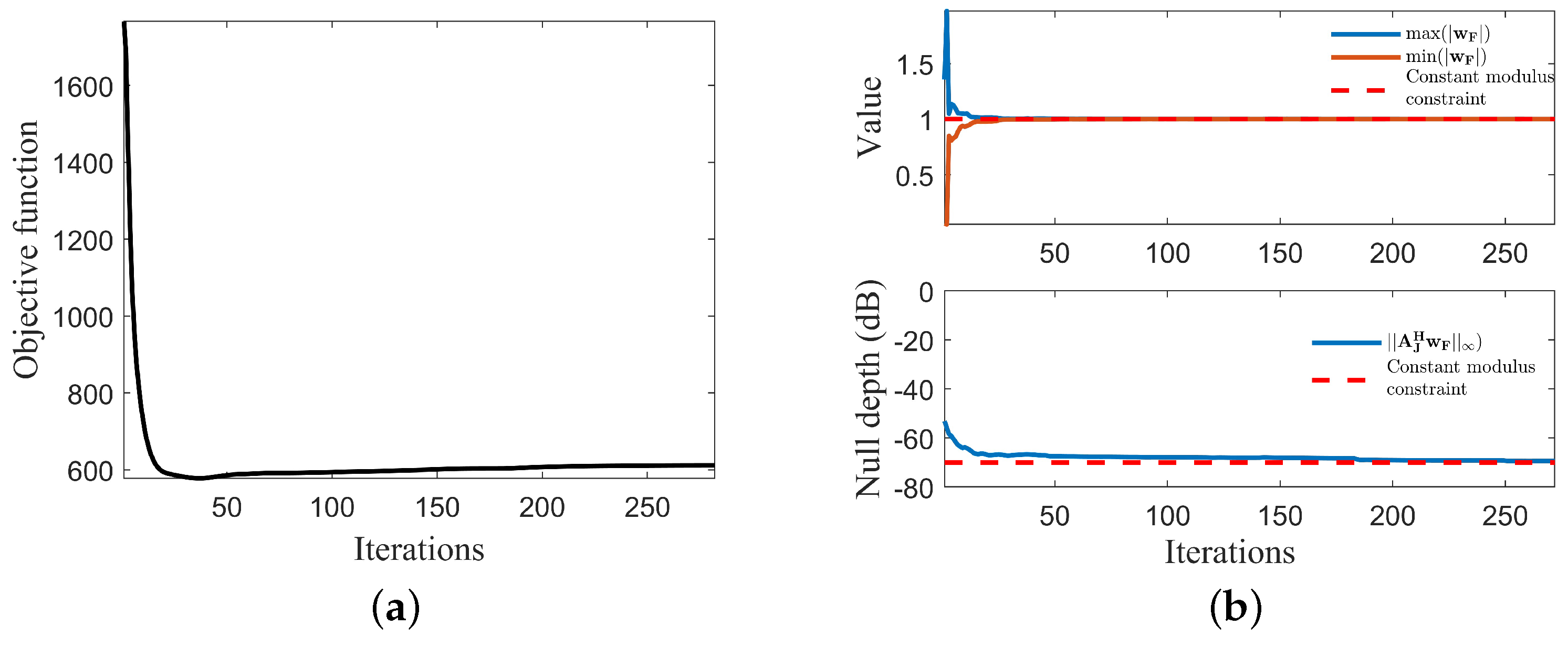
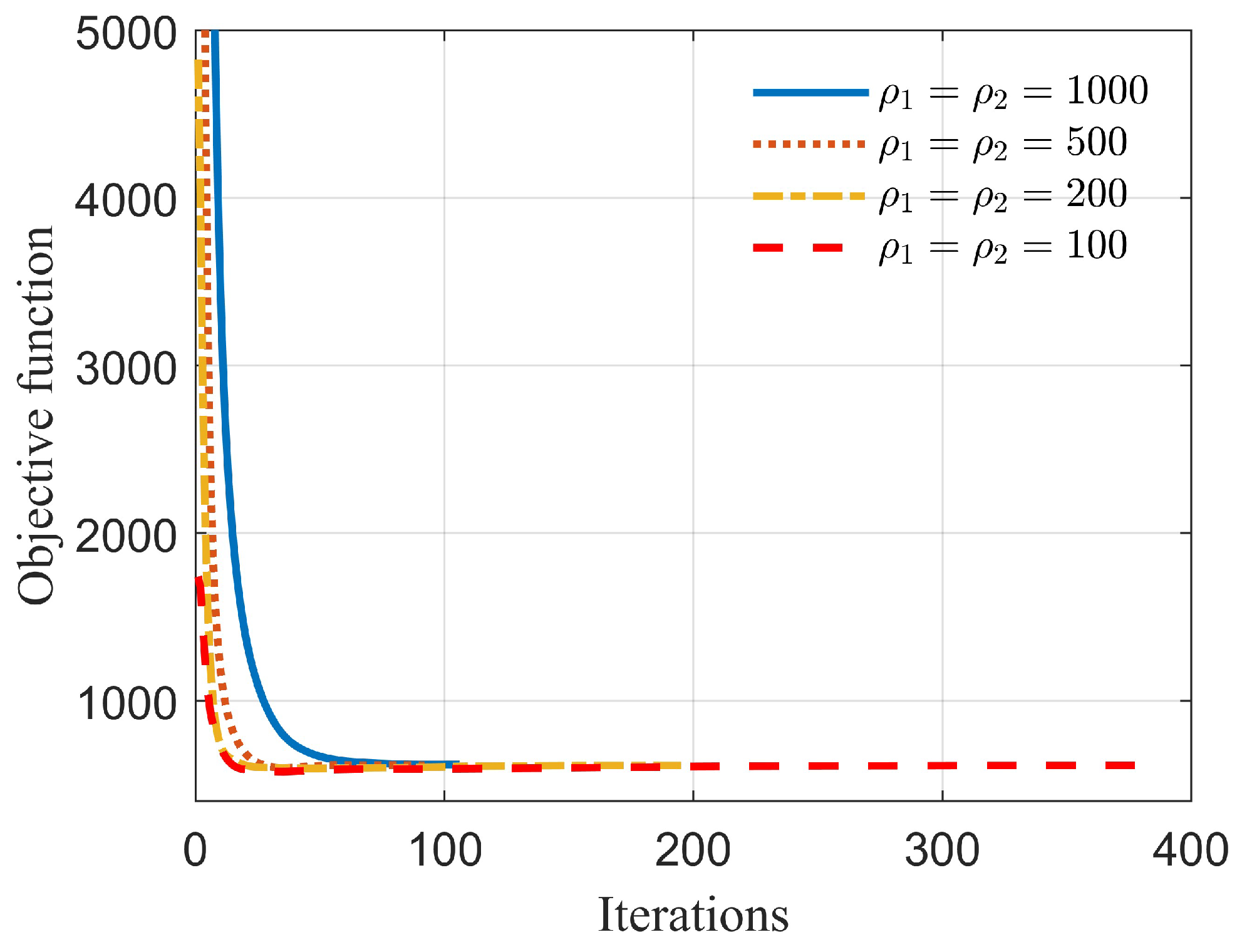
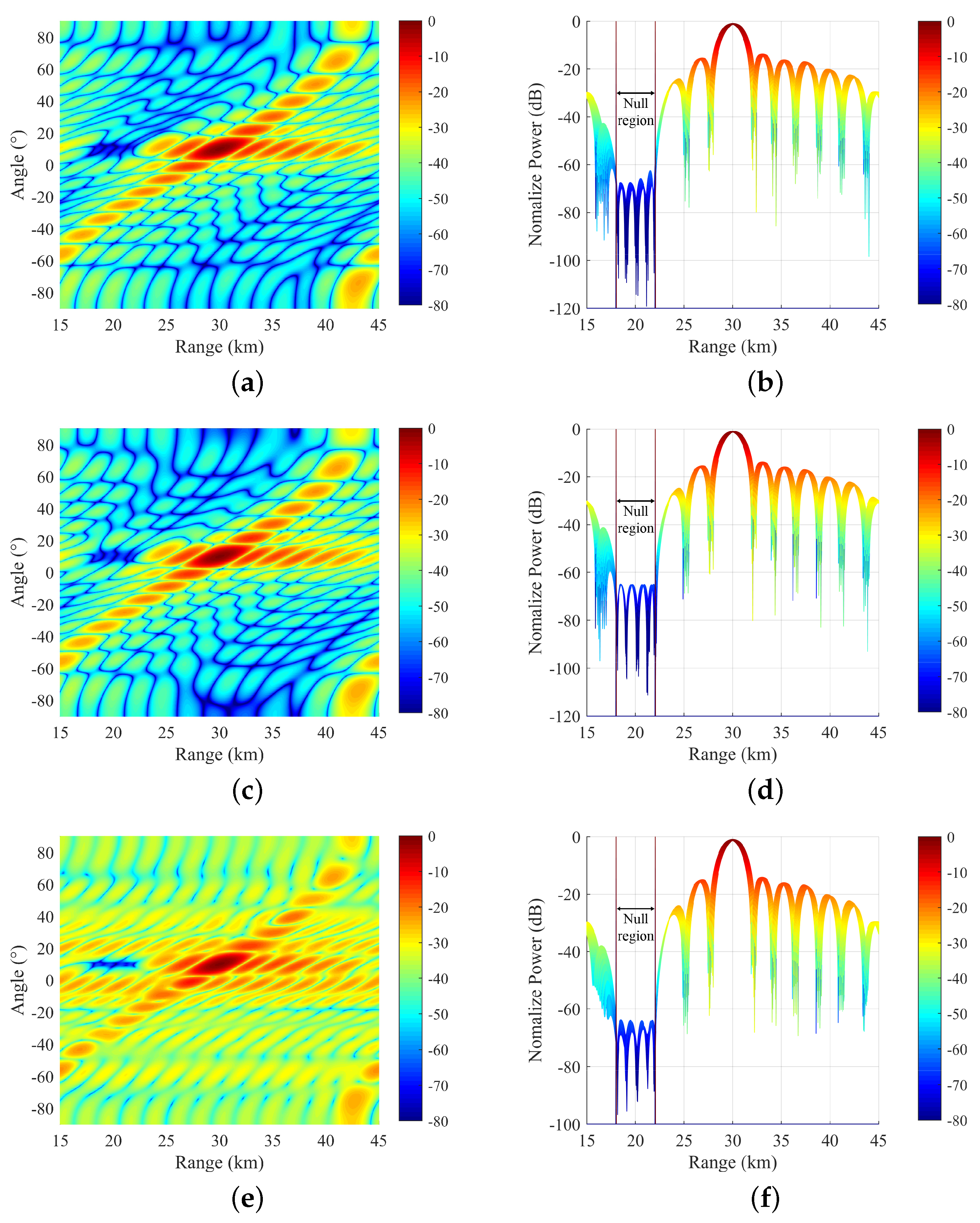
| Algorithms | Null Depth (dB) | Main Lobe Gain Loss (dB) | Execution Time (s) | VCMC |
|---|---|---|---|---|
| SDR−QC | −62.92 | 0.92 | 10.2 | 1.1523 |
| SDR−LC | −69.68 | 1.02 | 842 | 1.0946 |
| Proposed Method | −69.76 | 1.01 | 0.4 | 0 |
| Algorithm | Null Depth After Projection (dB) | Main Lobe Gain Loss After Projection (dB) |
|---|---|---|
| SDR−QC | −42.41 | 0.87 |
| SDR−LC | −46.87 | 0.99 |
| Proposed Method | −69.76 | 1.01 |
| Algorithm | Null Depth (dB) | Main Lobe Gain Loss (dB) | Execution Time (s) | VCMC |
|---|---|---|---|---|
| SDR−QC | −62.47 | 0.92 | 9.28 | 3.3468 |
| SDR−LC | −65.06 | 0.92 | 560 | 3.2437 |
| Proposed Method | −64.02 | 0.92 | 1.2 | 0 |
| Algorithm | Null Depth After Projection (dB) | Main Lobe Gain Loss After Projection (dB) |
|---|---|---|
| SDR−QC | −22.31 | 0.40 |
| SDR−LC | −22.51 | 0.41 |
| Proposed Method | −64.02 | 0.92 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiao, M.; Hu, T.; Shao, X.; Wu, Y.; Xiao, Z. A Phase-Only Optimization Null Control Method for FDA-MIMO Based on ADMM. Remote Sens. 2024, 16, 2865. https://doi.org/10.3390/rs16152865
Xiao M, Hu T, Shao X, Wu Y, Xiao Z. A Phase-Only Optimization Null Control Method for FDA-MIMO Based on ADMM. Remote Sensing. 2024; 16(15):2865. https://doi.org/10.3390/rs16152865
Chicago/Turabian StyleXiao, Mengxuan, Taiyang Hu, Xiaolang Shao, Yifan Wu, and Zelong Xiao. 2024. "A Phase-Only Optimization Null Control Method for FDA-MIMO Based on ADMM" Remote Sensing 16, no. 15: 2865. https://doi.org/10.3390/rs16152865
APA StyleXiao, M., Hu, T., Shao, X., Wu, Y., & Xiao, Z. (2024). A Phase-Only Optimization Null Control Method for FDA-MIMO Based on ADMM. Remote Sensing, 16(15), 2865. https://doi.org/10.3390/rs16152865







