A Comprehensive Signal Quality Assessment for BDS/Galileo/GPS Satellites and Signals
Abstract
1. Introduction
2. Data and Methods
2.1. Data
2.2. Carrier-to-Noise Density Ratio Evaluation Model
2.3. Noise Assessment Model
2.4. Code Multipath Analysis
3. Experiment Results
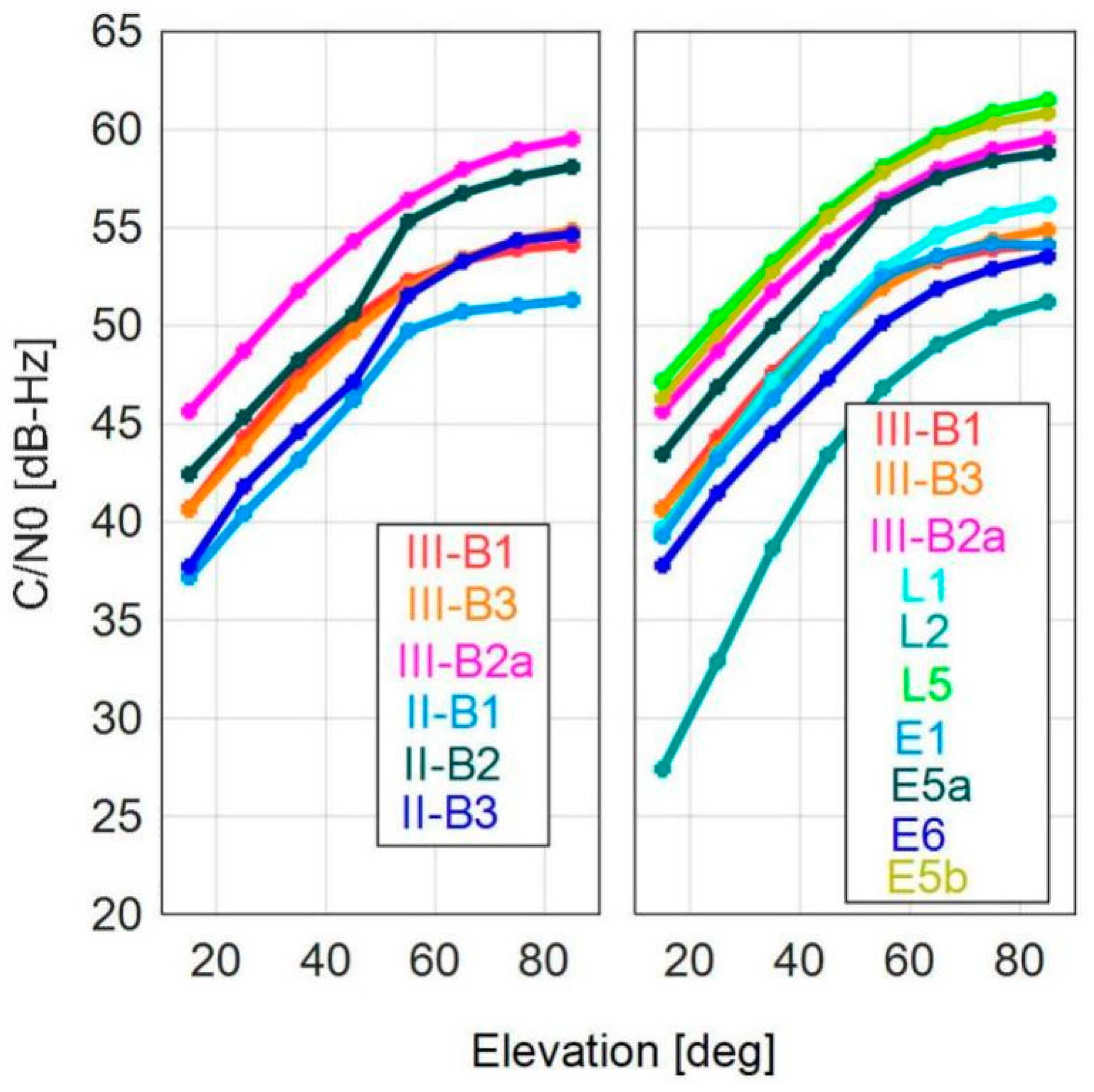
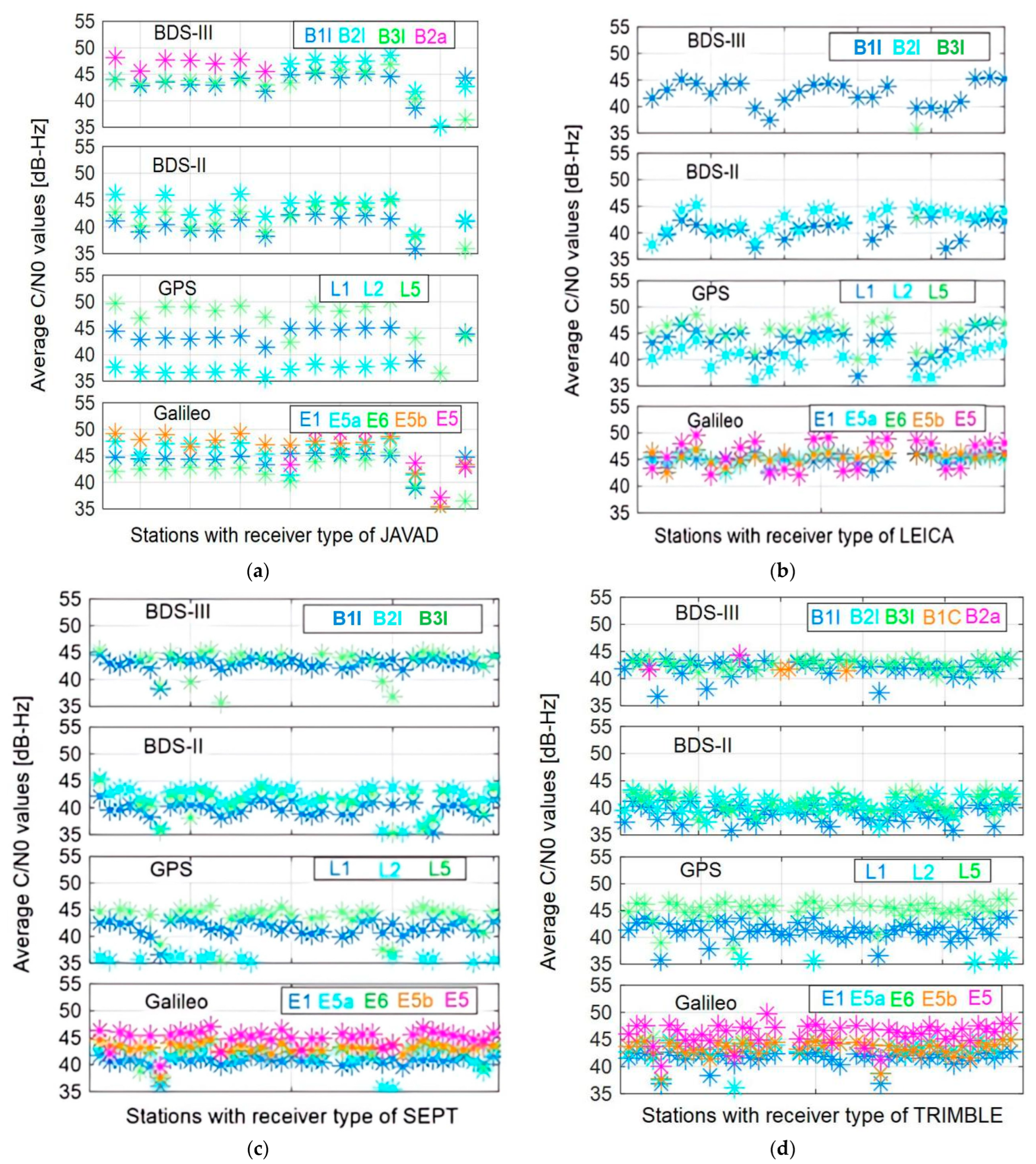
3.1. Experimental Analysis of Signal Power
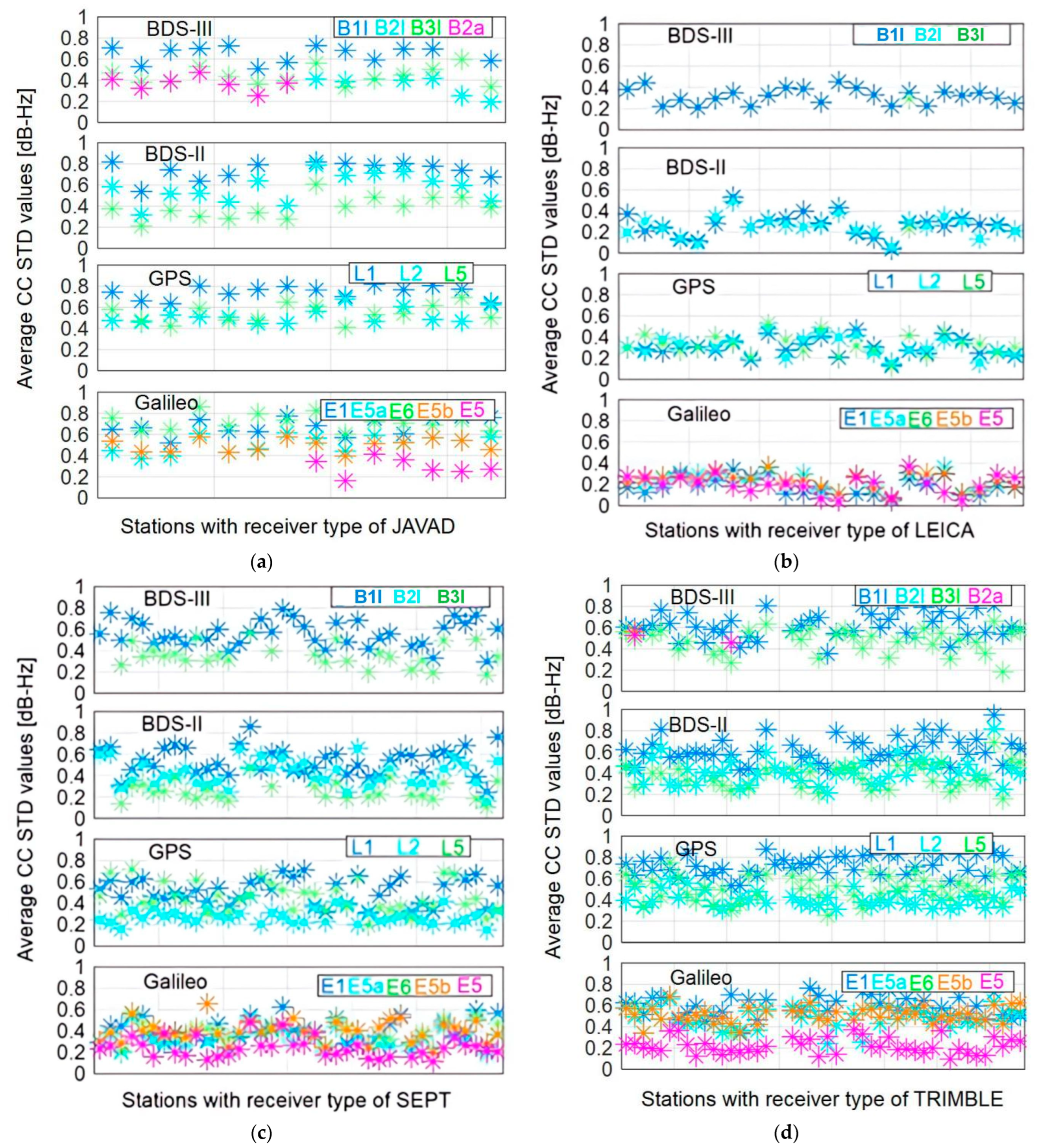
3.2. Experimental Analysis of Pseudorange Noise
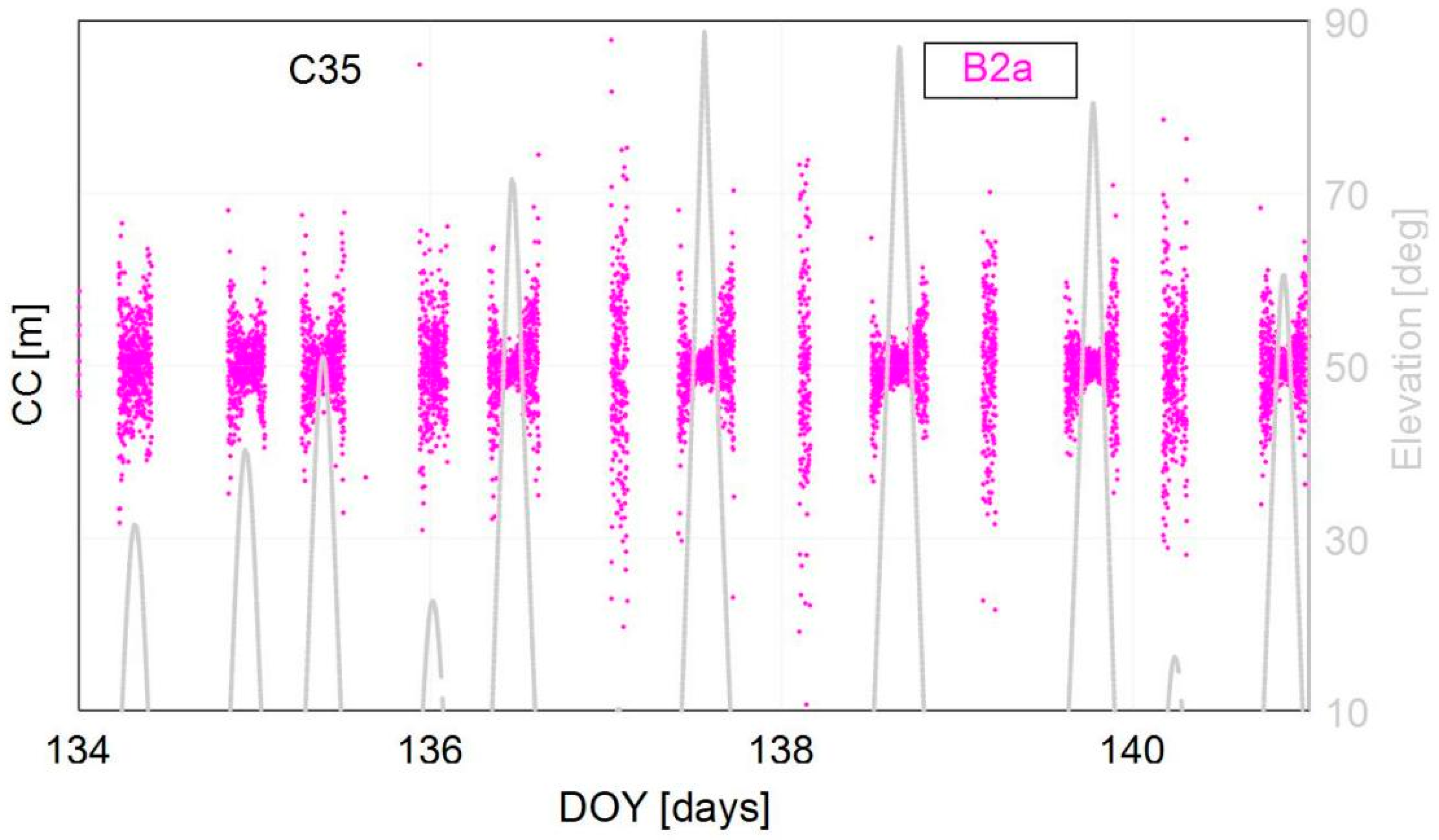
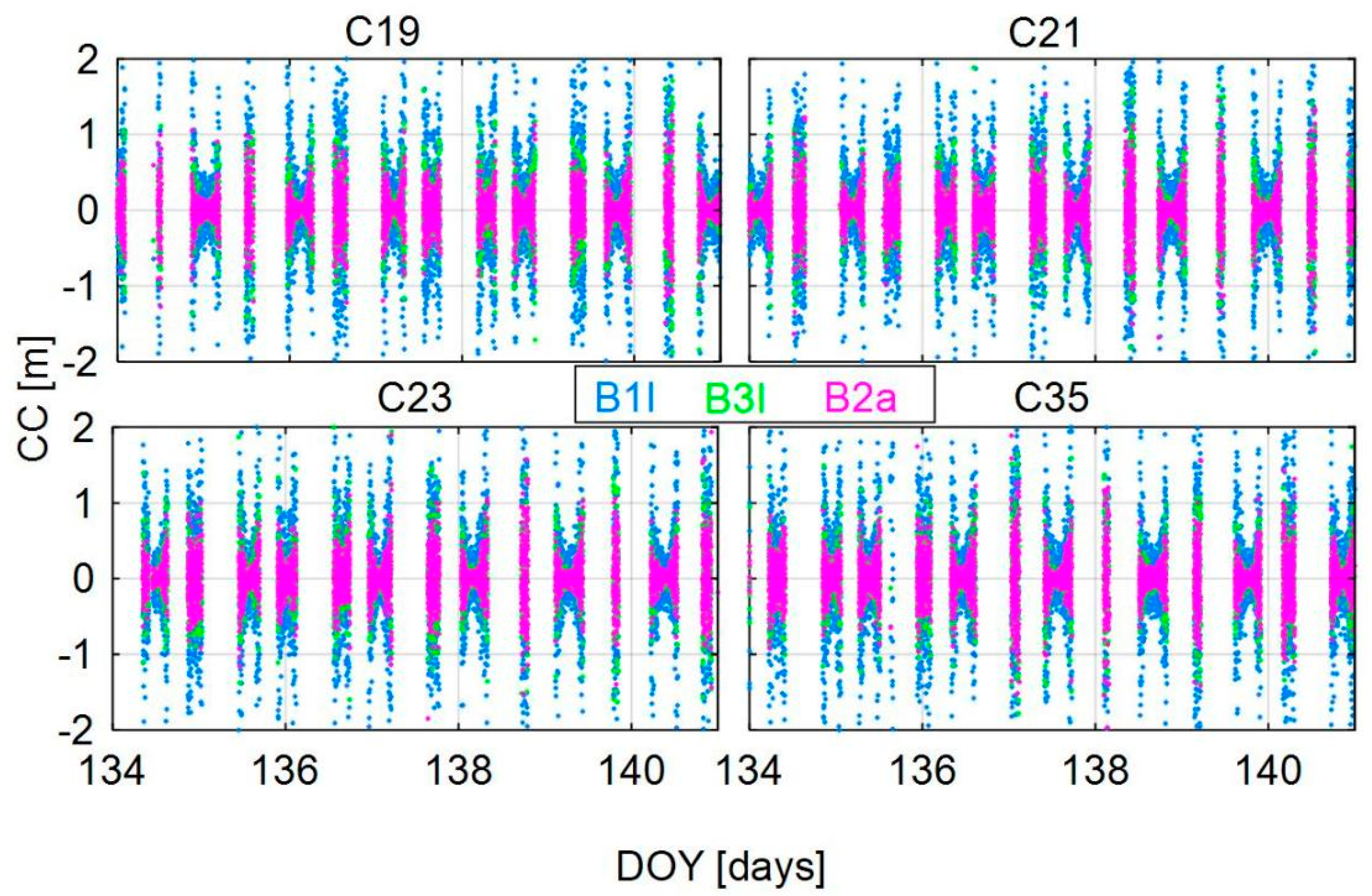
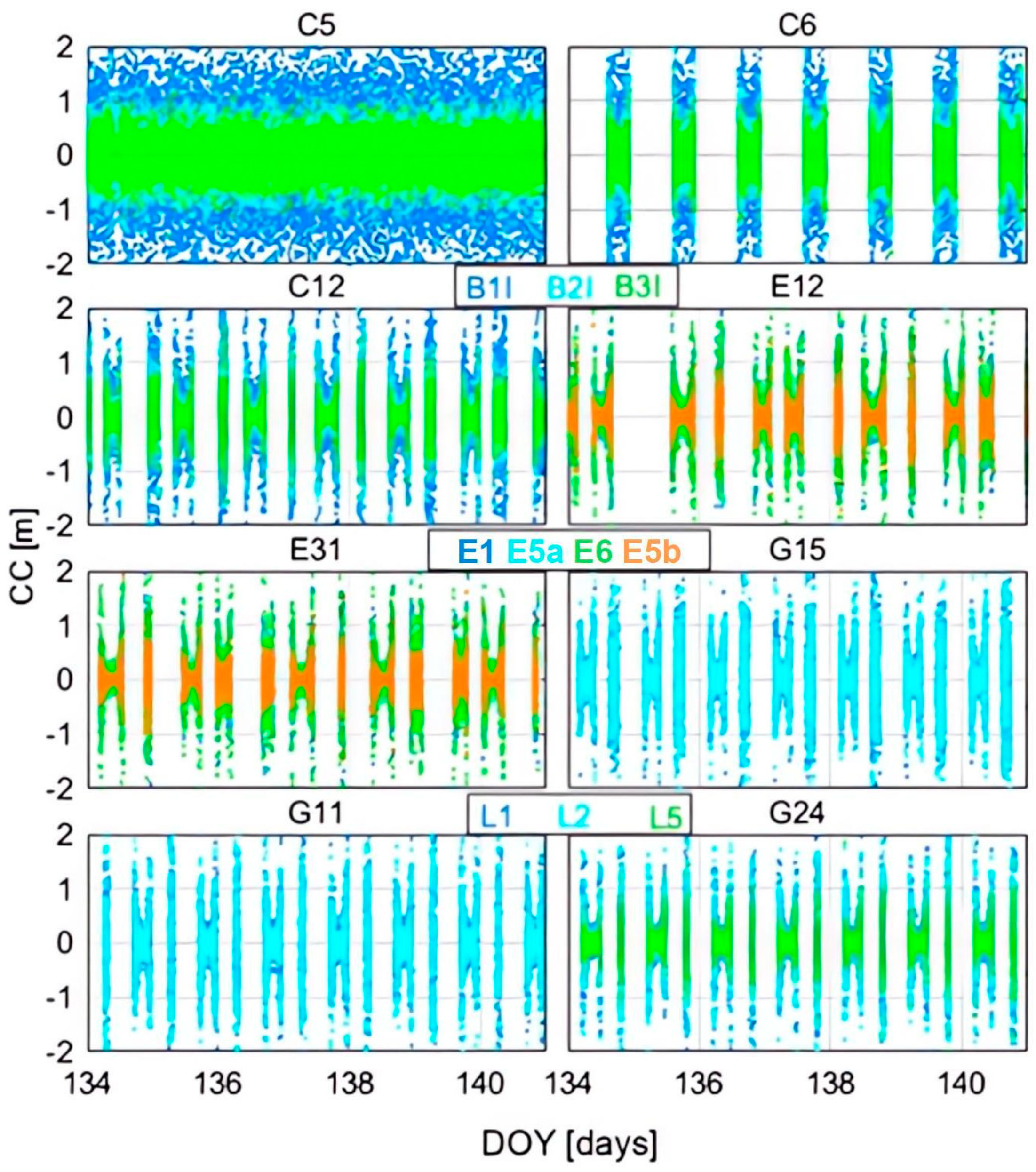
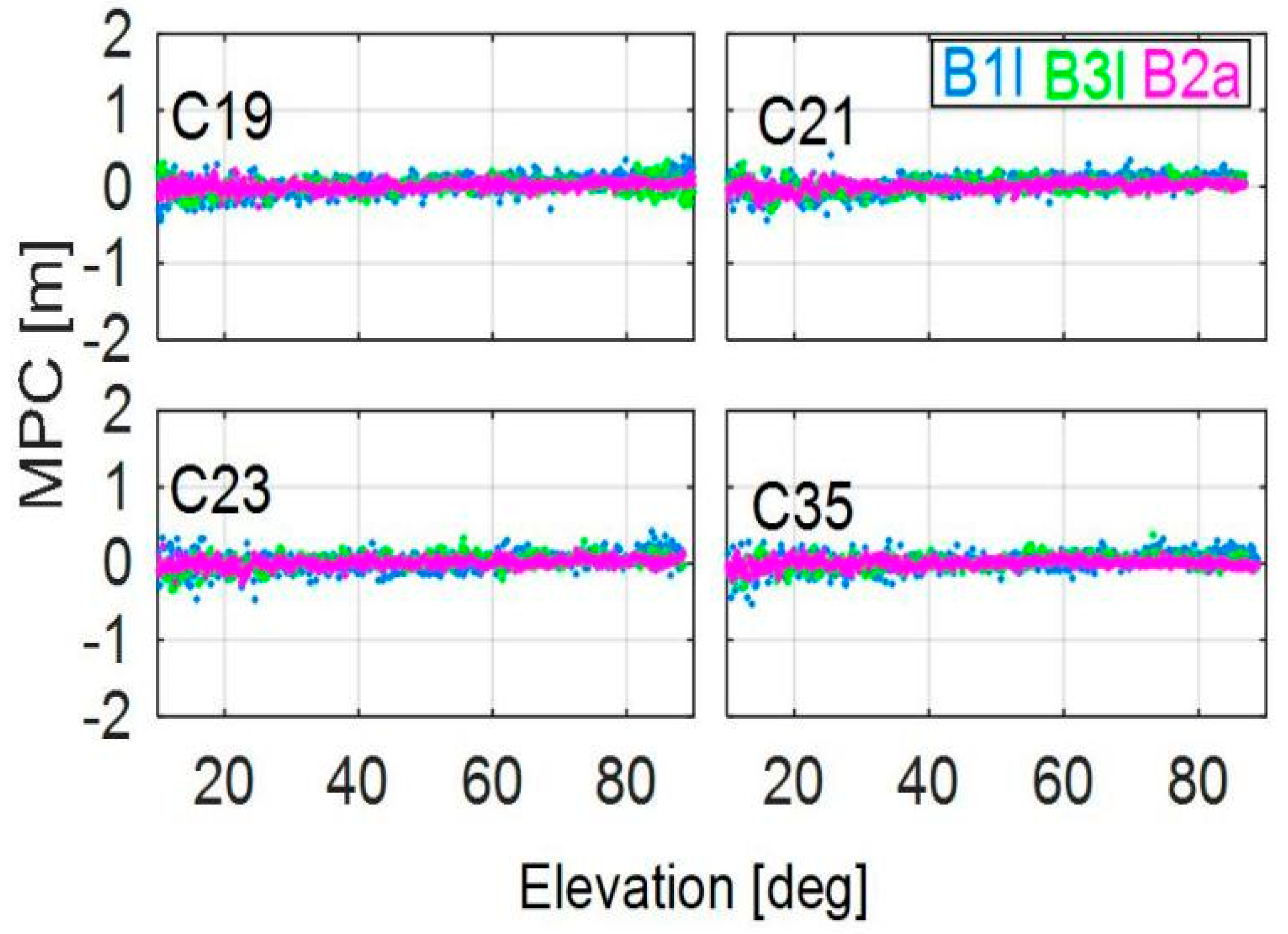
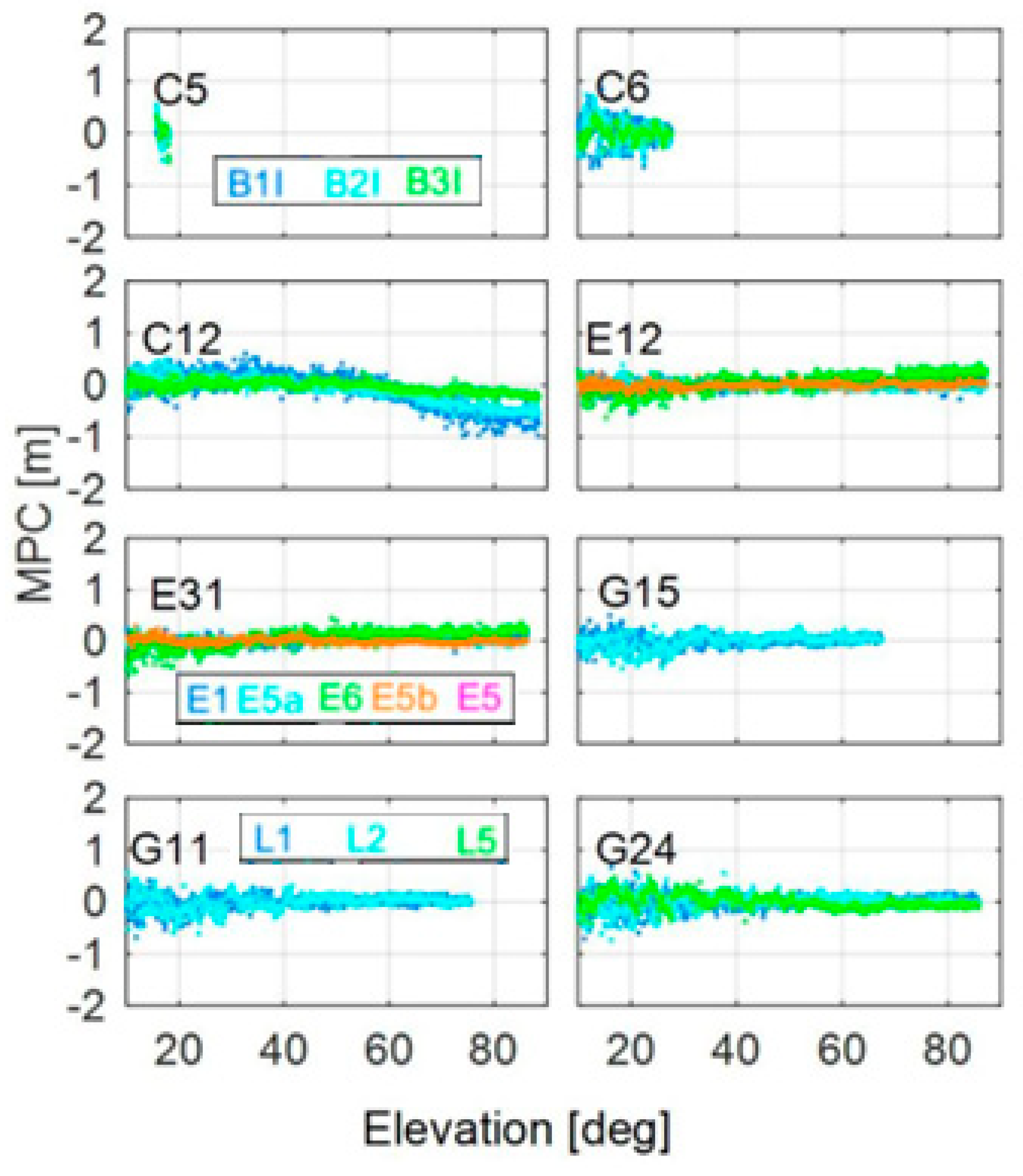
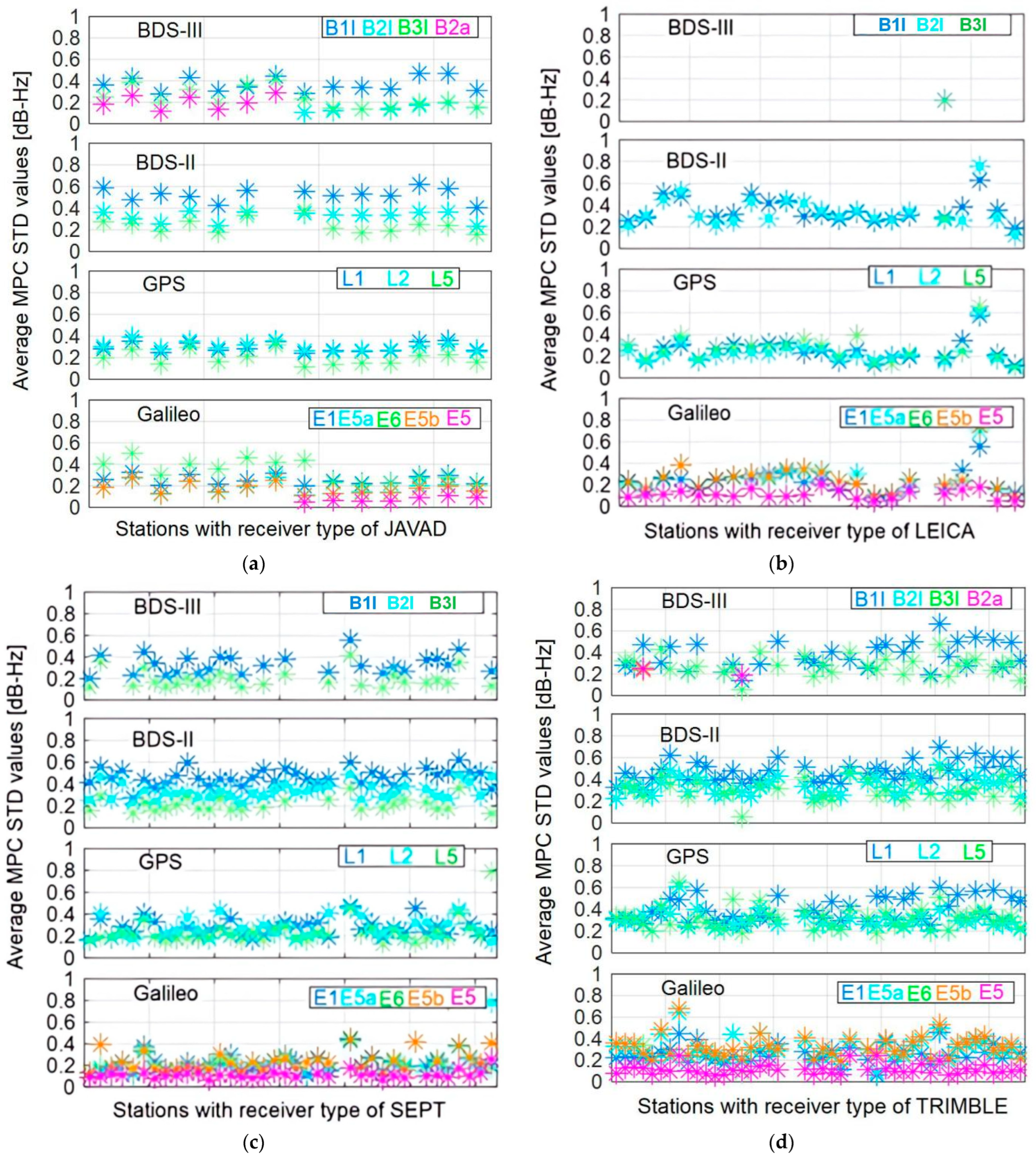
3.3. Experimental Analysis of Multipath
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- ILRS BeiDou (COMPASS) Center of Mass Information. Available online: https://ilrs.gsfc.nasa.gov/missions/satellite_missions/current_missions/bm13_general.html (accessed on 12 April 2024).
- Yang, Y.; Yang, Y.; Hu, X.; Tang, C.; Guo, R.; Zhou, Z.; Xu, J.; Pan, J.; Su, M. BeiDou-3 broadcast clock estimation by integration of observations of regional tracking stations and inter-satellite links. GPS Solut. 2021, 25, 57. [Google Scholar] [CrossRef]
- CSNO. Report on the Development of BeiDou Navigation Satellite System (Version 2.1). Available online: http://www.beidou.gov.cn/xt/gfxz/201805/P020180509588857259014.pdf (accessed on 10 December 2021).
- Gu, S.; Wang, Y.; Zhao, Q.; Zheng, F.; Gong, X. BDS-3 differential code bias estimation with undifferenced uncombined model based on triple-frequency observation. J. Geod. 2020, 94, 45. [Google Scholar] [CrossRef]
- CSNO. Development of BeiDou Navigation Satellite System (Version 4.0). Available online: http://www.beidou.gov.cn/xt/gfxz/201912/P020191227430565455478.pdf (accessed on 10 December 2021).
- Li, X.; Li, X.; Liu, G.; Yuan, Y.; Freeshah, M.; Zhang, K.; Zhou, F. BDS multi-frequency PPP ambiguity resolution with new B2a/B2b/B2a + b signals and legacy B1I/B3I signals. J. Geod. 2020, 94, 107. [Google Scholar] [CrossRef]
- Zhang, X.; Li, X.; Lu, C.; Wu, M.; Pan, L. A comprehensive analysis of satellite-induced code bias for BDS-3 satellites and signals. Adv. Space Res. 2019, 63, 2822–2835. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, B.; Nie, L.; Wei, C.; Jia, S.; Jiang, S. Initial assessment of BeiDou-3 global navigation satellite system: Signal quality, RTK and PPP. GPS Solut. 2019, 23, 1–12. [Google Scholar] [CrossRef]
- Lu, M.; Li, W.; Yao, Z. Overview of BDS III new signals. Navigation 2019, 66, 19–35. [Google Scholar] [CrossRef]
- China Satellite Navigation System Administration Office. BDS Satellite Navigation System Space Signal Interface Control Document Open Service Signal B1C (Version 1.0) [EB/OL][R]. 27 December 2017. Available online: http://www.beidou.gov.cn/xt/gfxz/201712/P020171226741342013031.pdf (accessed on 18 May 2022).
- China Satellite Navigation System Administration Office (CSNO). BDS Satellite Navigation System Space Signal Interface Control Document Open Service Signal B2a (Version 1.0) [EB/OL][R]. 27 December 2017. Available online: http://www.beidou.gov.cn/xt/gfxz/201712/P020171226742357364174.pdf (accessed on 18 May 2022).
- China Satellite Navigation System Administration Office (CSNO). BDS Satellite Navigation System Space Signal Interface Control Document Open Service Signal B3I (Version 1.0) [EB/OL][R]. 9 February 2018. Available online: http://www.beidou.gov.cn/xt/gfxz/201802/P020180209623601401189.pdf (accessed on 18 May 2022).
- China Satellite Navigation System Administration Office (CSNO). BDS Satellite Navigation System Space Signal Interface Control Document Open Service Signal B1I (Version 1.0) [EB/OL][R]. 27 February 2019. Available online: http://www.beidou.gov.cn/xt/gfxz/201902/P020190227593621142475.pdf (accessed on 18 May 2022).
- Gaglione, S.; Angrisano, A.; Castaldo, G.; Freda, P.; Gioia, C.; Innac, A. The first Galileo FOC satellites: From useless to essential. In Proceedings of the 2015 IEEE International Geoscience and Remote Sensing Symposium, Milan, Italy, 26–31 July 2015; IEEE: Piscataway, NJ, USA, 2015. [Google Scholar]
- Montenbruck, O.; Hauschild, A.; Steigenberger, P.; Hugentobler, U.; Teunissen, P.; Nakamura, S. Initial assessment of the compass/BDS-2 regional navigation satellite system. GPS Solut. 2013, 17, 211–222. [Google Scholar] [CrossRef]
- Hauschild, A.; Montenbruck, O.; Sleewaegen, J.; Huisman, L.; Teunissen, P. Characterization of Compass M-1 Signals. GPS Solut. 2012, 16, 117–126. [Google Scholar] [CrossRef]
- Wang, G.; Jong, K.; Zhao, Q.; Hu, Z.; Guo, J. Multipath analysis of code measurements for BDS geostationary satellites. GPS Solut. 2015, 19, 129–139. [Google Scholar] [CrossRef]
- Wanninger, L.; Beer, S. BDS satellite-induced code pseudorange variations: Diagnosis and therapy. GPS Solut. 2015, 19, 639–648. [Google Scholar] [CrossRef]
- Zaminpardaz, S.; Teunissen, P. Analysis of Galileo IOV + FOC signals and E5 RTK performance. GPS Solut. 2017, 21, 1855–1870. [Google Scholar] [CrossRef]
- Pan, L.; Zhang, X.; Liu, J.; Li, X.; Li, X. Analysis and correction of the inter-frequency clock bias for BDS satellites. In China Satellite Navigation Conference (CSNC) 2016 Proceedings; Springer: Singapore, 2016; Volume II, pp. 115–128. [Google Scholar]
- Yang, Y.; Li, J.; Wang, A.; Xu, J.; He, H.; Guo, H.; Shen, J.; Dai, X. Preliminary assessment of the navigation and positioning performance of BDS regional navigation satellite system. Sci. China Earth Sci. 2014, 57, 144–152. [Google Scholar] [CrossRef]
- Tian, Y.; Sui, L.; Xiao, G.; Zhao, D.; Tian, Y. Analysis of BDS/Galileo/GPS signals and RTK performance. GPS Solut. 2019, 23, 37. [Google Scholar] [CrossRef]
- Simsky, A.; Sleewaegen, J.; Crisci, M. Performance assessment of Galileo ranging signals transmitted by GSTB-v2 satellites. In Proceedings of the 19th International Technical Meeting of the Satellite Division of the Institute of Navigation (ION GNSS 2006), Fort Worth, TX, USA, 26–29 September 2006; pp. 1547–1559. [Google Scholar]
- Zhang, X.; Wu, M.; Liu, W.; Li, X.; Yu, S.; Lu, C. Initial assessment of the COMPASS/BDS-3: New-generation navigation signals. J. Geod. 2017, 91, 1225–1240. [Google Scholar] [CrossRef]
- Fu, Z.; Gong, X.; Gu, S.; Lou, Y.; Shi, S. Accounting for biases between BDS-3 and BDS-2 overlapping B1I/B3I signals in BeiDou global ionospheric modeling and DCB determination. Adv. Space Res. 2022, 69, 3677–3691. [Google Scholar]
- Mi, X.; Sheng, C.; ElMowafy, A.; Zhang, B. Characteristics of receiver-related biases between BDS-3 and BDS-2 for five frequencies including inter-system biases, differential code biases, and differential phase biases. GPS Solut. 2021, 25, 3. [Google Scholar] [CrossRef]
- Liang, Y.; Xu, J.; Wu, M.; Li, F. Analysis of the Long-Term Characteristics of BDS On-Orbit Satellite Atomic Clock: Since BDS-3 Was Officially Commissioned. Remote Sens. 2022, 14, 4535. [Google Scholar] [CrossRef]
- Wang, E.; Song, W.; Zhang, Y.; Shi, X.; Wang, Z.; Xu, S.; Shu, W. Evaluation of BDS/GPS Multi-Frequency RTK Positioning Performance under Different Baseline Lengths. Remote Sens. 2022, 14, 3561. [Google Scholar] [CrossRef]
- Yang, Y.; Mao, Y.; Sun, B. Basic performance and future developments of BeiDou global navigation satellite system. Satell. Navig. 2020, 1, 1. [Google Scholar] [CrossRef]
- Shi, J.; Ouyang, C.; Huang, Y.; Peng, W. Assessment of BDS-3 global positioning service: Ephemeris, SPP, PPP, RTK, and new signal. GPS Solut. 2020, 24, 81. [Google Scholar] [CrossRef]
- Gu, S.; Dai, C.; Fang, W.; Zheng, F.; Wang, Y.; Zhang, Q.; Lou, Y.; Niu, X. Multi-GNSS PPP/INS tightly coupled integration with atmospheric augmentation and its application in urban vehicle navigation. J. Geod. 2021, 95, 64. [Google Scholar] [CrossRef]
- Ma, Z.; Cui, J.; Liu, Z.; Su, X.; Xiang, Y.; Xu, Y.; Deng, C.; Hui, M.; Li, Q. Influence of Inter-System Biases on Combined Single-Frequency BDS-2 and BDS-3 Pseudorange Positioning of Different Types of Receivers. Remote Sens. 2024, 16, 1710. [Google Scholar] [CrossRef]
- Dang, X.; Yin, X.; Zhang, Y.; Gao, C.; Wu, J.; Liu, Y. Improved Medium Baseline RTK Positioning Performance Based on BDS/Galileo/GPS Triple-Frequency only Observations. Remote Sens. 2023, 15, 5198. [Google Scholar] [CrossRef]
- Xiao, K.; Sun, F.; Zhu, X.; Zhou, P.; Ma, Y.; Wang, Y. Assessment of overlapping triple-frequency BDS-3/BDS-2/INS tightly coupled integration model in kinematic surveying. GPS Solut. 2024, 28, 2. [Google Scholar] [CrossRef]
- Shu, B.; Tian, Y.; Qu, X.; Li, W.; Huang, G.; Du, Y.; Zhang, Q. Estimation of BDS-2/3 phase observable-specific signal bias aided by double-differenced model: An exploration of fast BDS-2/3 real-time PPP. GPS Solut. 2024, 28, 88. [Google Scholar] [CrossRef]


| Signal Component | Frequency (MHz) | Bandwidth (MHz) | Code Rate (Mcps) | Modulation | Broadcast Satellite | Compatible Frequency |
|---|---|---|---|---|---|---|
| B1I | 1561.09 | 4.092 | 2.046 | BPSK (2) | All | |
| B3I | 1268.52 | 20.46 | 10.23 | BPSK (10) | All | |
| B1C_data | 1575.42 | 32.736 | 1.023 | BOC (1,1) | IGSO MEO | L1, E1 |
| B1C_pilot | QMBOC (6,1,4/33) | |||||
| B2a_data | 1176.45 | 20.46 | 10.23 | BPSK (10) | IGSO MEO | L5, E5a |
| B2a_pilot | ||||||
| B2b_I | 1207.14 | — | 10.23 | QPSK (10) | IGSO MEO | E5b |
| B2b_Q |
| Receiver Type | Firmware Version | Observation Signal | Selection of Stations |
|---|---|---|---|
| JAVAD | TRE_3 3.7.6 | G: L1, L2, L5 E: E1, E5a, E5b, E5 C: B1I, B2I, B3I, B2a | POTS, SGOC, SUTM, ULAB, URUM, WIND, WUH2 |
| Others | G: L1, L2, L5 E: E1, E5a, E5b, E5 C: B1I, B2I, B3I | 9 measuring stations, such as BSHM, KOKV | |
| LEICA | GR10, GR25, GR30, GR50 | G: L1, L2, L5 E: E1, E5a, E5b, E5 C: B1I, B2I | 25 measuring stations, such as ALIC, EBRE |
| TRIMBLE | NETR9 | G: L1, L2, L5 E: E1, E5a, E5b, E5 C: B1I, B2I, B3I, | 48 measuring stations, such as BOR1, CUT0 |
| SEPT | POLARX5 | G: L1, L2, L5 E: E1, E5a, E5b, E5, E6 C: B1I, B2I, B3I | 40 measuring stations, such as CEBR, HOB2 |
| System | Galileo | BDS-2 | BDS-3 | GPS | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| E1 | E5a | E5b | E5 | E6 | B1I | B2I | B3I | B1I | B3I | B2a | L1 | L2 | L5 | |
| E5a | E1 | E1 | E1 | E1 | B2I | B1I | B1I | B3I | B1I | B1I | L2 | L1 | L1 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Y.; Xiao, G.; Guo, R.; Zhao, D.; Zhang, L.; Xin, J.; Guo, J.; Han, Y.; Du, X.; He, D.; et al. A Comprehensive Signal Quality Assessment for BDS/Galileo/GPS Satellites and Signals. Remote Sens. 2024, 16, 2277. https://doi.org/10.3390/rs16132277
Tian Y, Xiao G, Guo R, Zhao D, Zhang L, Xin J, Guo J, Han Y, Du X, He D, et al. A Comprehensive Signal Quality Assessment for BDS/Galileo/GPS Satellites and Signals. Remote Sensing. 2024; 16(13):2277. https://doi.org/10.3390/rs16132277
Chicago/Turabian StyleTian, Yijun, Guorui Xiao, Rui Guo, Dongqing Zhao, Lu Zhang, Jie Xin, Jinglei Guo, Yuechao Han, Xuefan Du, Donghan He, and et al. 2024. "A Comprehensive Signal Quality Assessment for BDS/Galileo/GPS Satellites and Signals" Remote Sensing 16, no. 13: 2277. https://doi.org/10.3390/rs16132277
APA StyleTian, Y., Xiao, G., Guo, R., Zhao, D., Zhang, L., Xin, J., Guo, J., Han, Y., Du, X., He, D., & Qin, Z. (2024). A Comprehensive Signal Quality Assessment for BDS/Galileo/GPS Satellites and Signals. Remote Sensing, 16(13), 2277. https://doi.org/10.3390/rs16132277






