Examining the Capability of the VLF Technique for Nowcasting Solar Flares Based on Ground Measurements in Antarctica
Abstract
1. Introduction
2. Measurements and Methodology
2.1. VLF Measurements of Solar Flares at GWS
2.2. Identifying Solar Flare Events
2.3. VLF Data Processing
3. Analysis Results
3.1. The Effects of the Solar Zenith Angle
3.2. Solar Flare Effects on Dayside Paths
3.3. Solar Flare Effects on Mixed Paths
4. Discussion
5. Conclusions
- (1)
- For the dayside paths, the time delay between the maxima of a solar flare and the VLF maximum change can vary by several minutes, and it is negatively correlated with the fluxes of flare X-rays. As for the mixed paths, the time delay for the NAA-GWS path is always inversely correlated with the maximum X-ray flux of the associated solar flares, while the time delay in the phase for the NPM-GWS and HWU-GWS paths has positive correlations.
- (2)
- The VLF response in both the amplitude and phase is linearly proportional to the logarithm of the flare X-ray fluxes for the day–night mixed propagation paths. The curve-fitting coefficients between the VLF maximum change and flare X-rays for the NPM-GWS and NAA-GWS paths during the day–night mixed conditions are found to be larger than those of the dayside conditions, in contrast to the HWU-GWS path.
- (3)
- The curve-fitting coefficients are found to be consistent with those reported in George et al. [41] for the dayside paths. However, these coefficients are notably different for the day–night mixed paths, suggesting the need to update the previously developed nowcasting technique for day–night mixed propagation paths.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Inan, U.S.; Cummer, S.A.; Marshall, R.A. A survey of ELF and VLF research on lightning-ionosphere interactions and causative discharges. J. Geophys. Res. 2010, 115, A00E36. [Google Scholar] [CrossRef]
- Clilverd, M.A.; Rodger, C.J.; Thomson, N.R.; Lichtenberger, J.; Steinbach, P.; Cannon, P.; Angling, M.J. Total solar eclipse effects on VLF signals: Observations and modeling. Radio Sci. 2001, 36, 773–788. [Google Scholar] [CrossRef]
- Pal, S.; Chakrabarti, S.K.; Mondal, S.K. Modeling of sub-ionospheric VLF signal perturbations associated with total solar eclipse, 2009 in Indian subcontinent. Adv. Space Res. 2012, 50, 196–204. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A. Characteristics of energetic electron precipitation estimated from simulated bremsstrahlung X-ray distributions. J. Geophys. Res. Space Phys. 2019, 124, 2831–2843. [Google Scholar] [CrossRef]
- Cheng, W.; Xu, W.; Gu, X.; Wang, S.; Wang, Q.; Ni, B.; Lu, Z.; Xiao, B.; Meng, X. A Comparative Study of VLF Transmitter Signal Measurements and Simulations during Two Solar Eclipse Events. Remote Sens. 2023, 15, 3025. [Google Scholar] [CrossRef]
- Basak, T.; Hobara, Y.; Pal, S.; Nakamura, T.; Izutsu, J.; Minatohara, T. Modeling of Solar Eclipse effects on the sub-ionospheric VLF/LF signals observed by multiple stations over Japan. Adv. Space Res. 2024, 73, 736–746. [Google Scholar] [CrossRef]
- Thomson, N.R.; Clilverd, M.A. Solar flare induced ionospheric D-region enhancements from VLF amplitude observations. J. Atmos. Sol.-Terr. Phys. 2001, 63, 1729–1737. [Google Scholar] [CrossRef]
- Singh, A.K.; Singh, A.K.; Singh, R.; Singh, R.P. Solar flare induced D-region ionospheric perturbations evaluated from VLF measurements. Astrophys. Space Sci. 2014, 350, 1–9. [Google Scholar] [CrossRef]
- Bouderba, Y.; NaitAmor, S.; Tribeche, M. Study of the solar flares effect on VLF radio signal propagating along NRK-ALG path using LWPC code. J. Geophys. Res. 2016, 121, 6799–6807. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Kero, A.; Turunen, E.; Drob, D.; Sojka, J.; Rice, D. VLF measurements and modeling of the D-region response to the 2017 total solar eclipse. IEEE Trans. Geosci. Remote Sens. 2019, 57, 7613–7622. [Google Scholar] [CrossRef]
- Gu, X.; Luo, F.; Peng, R.; Li, G.; Chen, H.; Wang, S.; Yi, J.; Li, Z.; Ni, B.; Zhao, Z.; et al. Response characteristics of very low frequency signals from JJI transmitter to solar flare events. Chin. J. Geophys. 2021, 64, 1508–1517. (In Chinese) [Google Scholar] [CrossRef]
- Greatorex, H.; Milligan, R.; Chamberlin, P. Observational Analysis of Lyα Emission in Equivalent-magnitude Solar Flares. Astrophys. J. 2023, 954, 120. [Google Scholar] [CrossRef]
- Moler, W.F. VLF propagation effects of a D-region layer produced by cosmic rays. J. Geophys. Res. 1960, 65, 1459–1468. [Google Scholar] [CrossRef]
- Kolarski, A.; Veselinović, N.; Srećković, V.; Mijić, Z.; Savić, M.; Dragić, A. Impacts of Extreme Space Weather Events on September 6, 2017 on Ionosphere and Primary Cosmic Rays. Remote Sens. 2023, 15, 1403. [Google Scholar] [CrossRef]
- Marshall, R.A.; Xu, W.; Sousa, A.; McCarthy, M.; Millan, R. X-ray signatures of lightning-induced electron precipitation. J. Geophys. Res. Space Phys. 2019, 124, 10230–10245. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Kero, A.; Sousa, A. Chemical response of the upper atmosphere due to lightning-induced electron precipitation. J. Geophys. Res. Atmos. 2021, 126, e2021JD034914. [Google Scholar] [CrossRef]
- Chand, A.; Kumar, A.; Kumar, S. VLF and Ionospheric D-Region Perturbations Associated With WWLLN-Detected Lightning in the South Pacific Region. J. Geophys. Res. Space Phys. 2023, 128, e2022JA030964. [Google Scholar] [CrossRef]
- Tomicic, M.; Chanrion, O.; Farges, T.; Mlynarczyk, J.; Kolmašová, I.; Soula, S.; Lapierre, J.; Köhn, C.; Neubet, T. Observations of Elves and Radio Wave Perturbations by Intense Lightning. J. Geophys. Res. Atmos. 2023, 128, e2022JD036541. [Google Scholar] [CrossRef]
- Ni, B.; Hua, M.; Gu, X.; Fu, S.; Xiang, Z.; Cao, X.; Ma, X. Artificial modification of Earth’s radiation belts by ground-based very-low-frequency (VLF) transmitters. Sci. China Earth Sci. 2022, 65, 391–413. [Google Scholar] [CrossRef]
- Hu, J.; Xiang, Z.; Ma, X.; Liu, Y.; Dong, J.; Guo, D.; Ni, B. Long-Term Variations of Energetic Electrons Scattered by Signals From the North West Cape Transmitter. Space Weather 2024, 22, e2023SW003827. [Google Scholar] [CrossRef]
- Chen, Z.; Xie, T.; Li, H.; Ouyang, Z.; Deng, X. The Global Variation of Low Ionosphere Under Action of Energetic Electron Precipitation. J. Geophys. Res. Space Phys. 2023, 128, e2023JA031930. [Google Scholar] [CrossRef]
- Wait, J.R.; Spies, K.P. Characteristics of the Earth-Ionosphere Waveguide for VLF Radio Waves; Note 300; NBS Tech.: Boulder, CO, USA, 1964. [Google Scholar]
- Cummer, S.A.; Inan, U.S.; Bell, T.F. Ionospheric D region remote sensing using vlf radio atmospherics. Radio Sci. 1998, 33, 1781–1792. [Google Scholar] [CrossRef]
- Han, F.; Cummer, S.A. Midlatitude daytime D region ionosphere variations measured from radio atmospherics. J. Geophys. Res. 2010, 115, A10314. [Google Scholar] [CrossRef]
- Xu, W.; Marshall, R.A.; Bortnik, J.; Bonnell, J.W. An electron density model of the D-and E-region ionosphere for transionospheric VLF propagation. J. Geophys. Res. Space Phys. 2021, 126, e2021JA029288. [Google Scholar] [CrossRef]
- Rodger, C.J.; Enell, C.-F.; Turunen, E.; Clilverd, M.A.; Thomson, N.R.; Verronen, P.T. Lightning-driven inner radiation belt energy deposition into the atmosphere: Implications for ionisation-levels and neutral chemistry. Ann. Geophys. 2007, 25, 1745–1757. [Google Scholar] [CrossRef]
- Inan, U.S.; Lehtinen, N.G.; Moore, R.C.; Hurley, K.; Boggs, S.; Smith, D.M.; Fishman, G.J. Massive disturbance of the daytime lower ionosphere by the giant γ-ray flare from magnetar SGR 1806-20. Geophys. Res. Lett. 2007, 34, L08103. [Google Scholar] [CrossRef]
- Grubor, D.P.; Šulić, D.M.; Žigman, V. Classification of X-ray solar flares regarding their effects on the lower ionosphere electron density profile. Ann. Geophys. 2008, 26, 1731–1740. [Google Scholar] [CrossRef]
- Raulin, J.-P.; Bertoni, F.C.P.; Gavilán, H.R.; Guevara-Day, W.; Rodriguez, R.; Fernandez, G.; Correia, E.; Kaufmann, P.; Pacini, A.; Stekel, T.R.C.; et al. Solar flare detection sensitivity using the South America VLF Network (SAVNET). J. Geophys. Res. 2010, 115, A07301. [Google Scholar] [CrossRef]
- Šulić, D.M.; Srećković, V.A. A comparative study of measured amplitude and phase perturbations of VLF and LF radio signals induced by solar flares. Serb. Astron. J. 2014, 188, 45–54. [Google Scholar] [CrossRef]
- Neal, J.J.; Rodger, C.J.; Clilverd, M.A.; Thomson, N.R.; Raita, T.; Ulich, T. Long-term determination of energetic electron precipitation into the atmosphere from AARDDVARK subionospheric VLF observations. J. Geophys. Res. Space Phys. 2015, 120, 2194–2211. [Google Scholar] [CrossRef]
- Mitra, A.P. Ionospheric Effects of Solar Flares; D. Reidel: Boston, MA, USA, 1974; Volume 46. [Google Scholar]
- Xiong, B.; Wan, W.; Liu, L.; Withers, P.; Zhao, B.; Ning, B.; Wei, Y.; Le, H.; Ren, Z.; Chen, Y.; et al. Ionospheric response to the X-class solar flare on September 7 2005. J. Geophys. Res. 2011, 116, A11317. [Google Scholar] [CrossRef]
- Qian, L.; Wang, W.; Burns, A.G.; Chamberlin, P.C.; Solomon, S.C. Responses of the thermosphere and ionosphere system to concurrent solar flares and geomagnetic storms. J. Geophys. Res. Space Phys. 2020, 125, e2019JA027431. [Google Scholar] [CrossRef]
- Žigman, V.; Grubor, D.; Šulić, D. D-region electron density evaluated from VLF amplitude time delay during X-ray solar flares. J. Atmos. Sol.-Terr. Phys. 2007, 69, 775–792. [Google Scholar] [CrossRef]
- Xu, W.; Gu, X.; Ni, B.; Wang, S.; Yang, Z.; Cheng, W.; Hu, Z.; He, F.; Li, B.; Chen, X.; et al. Measurements and Modeling of the Responses of VLF Transmitter Signals to X-Class Solar Flares at the Great Wall Station in Antarctica. Space Weather 2023, 21, e2022SW003249. [Google Scholar] [CrossRef]
- Gu, X.; Yi, J.; Wang, S.; Hu, Z.; Xu, W.; Ni, B.; Li, B.; He, F.; Chen, X.; Hu, H. Comparison of VLF Signal Responses to Solar Flares along Daytime and Nighttime Propagation Paths. Remote Sens. 2023, 15, 1018. [Google Scholar] [CrossRef]
- McRae, W.M.; Thomson, N.R. Solar flare induced ionospheric D-region enhancements from VLF phase and amplitude observations. J. Atmos. Sol.-Terr. Phys. 2004, 66, 77–87. [Google Scholar] [CrossRef]
- Žigman, V.; Dominique, M.; Grubor, D.; Rodger, C.; Clilverd, M. Lower-ionosphere electron density and effective recombination coefficients from multi-instrument space observations and ground VLF measurements during solar flares. J. Atmos. Sol.-Terr. Phys. 2023, 247, 106074. [Google Scholar] [CrossRef]
- Wenzel, D.; Jakowski, N.; Berdermann, J.; Mayer, C.; Valladares, C.; Heber, B. Global Ionospheric Flare Detection System (GIFDS). J. Atmos. Sol.-Terr. Phys. 2016, 138–139, 233–242. [Google Scholar] [CrossRef]
- George, H.E.; Rodger, C.J.; Clilverd, M.A.; Cresswell-Moorcock, K.; Brundell, J.B.; Thomson, N.R. Developing a Nowcasting Capability for X-Class Solar Flares Using VLF Radiowave Propagation Changes. Space Weather 2019, 17, 1783–1799. [Google Scholar] [CrossRef]
- Belcher, S.R.G.; Clilverd, M.A.; Rodger, C.J.; Cook, S.; Thomson, N.R.; Brundell, J.B.; Raita, T. Solar flare X-ray impacts on long subionospheric VLF paths. Space Weather 2021, 19, e2021SW002820. [Google Scholar] [CrossRef]
- Hayes, L.A.; Gallagher, P.T.; McCauley, J.; Dennis, B.R.; Ireland, J.; Inglis, A. Pulsations in the Earth’s lower ionosphere synchronized with solar flare emission. J. Geophys. Res. Space Phys. 2017, 122, 9841–9847. [Google Scholar] [CrossRef]
- Kumar, A.; Kumar, S. Solar flare effects on D-region ionosphere using VLF measurements during low-and high-solar activity phases of solar cycle 24. Earth Planets Space 2018, 70, 29. [Google Scholar] [CrossRef]
- Chen, Y.; Yang, G.; Ni, B.; Zhao, Z.; Gu, X.; Zhou, C.; Wang, F. Development of ground-based elf/vlf receiver system in wuhan and its first results. Adv. Space Res. 2016, 57, 1871–1880. [Google Scholar] [CrossRef]
- Gu, X.; Chen, H.; Wang, S.; Lu, Z.; Ni, B.; Li, G.; Cheng, W. Extraction of Alpha transmitter signals from single-station observations using the direction-finding method. Sci. China Technol. Sci. 2022, 65, 1727–1737. [Google Scholar] [CrossRef]
- Gu, X.; Li, G.; Pang, H.; Wang, S.; Ni, B.; Luo, F.; Peng, R.; Chen, L. Statistical analysis of very low frequency atmospheric noise caused by the global lightning using ground-based observations in China. J. Geophys. Res. Space Phys. 2021, 126, e2020JA029101. [Google Scholar] [CrossRef]
- Gu, X.; Peng, R.; Wang, S.; Ni, B.; Luo, F.; Li, G.; Li, Z. Responses of the very low frequency transmitter signals during the solar eclipse on December 26, 2019 over a North-South propagation path. IEEE Trans. Geosci. Remote Sens. 2022, 60, 2000207. [Google Scholar] [CrossRef]
- Chen, Y.; Ni, B.; Gu, X.; Zhao, Z.; Yang, G.; Zhou, C.; Zhang, Y. First observations of low latitude whistlers using whu ELF/VLF receiver system. Sci. China Technol. Sci. 2017, 60, 166–174. [Google Scholar] [CrossRef]
- Wang, S.; Gu, X.; Luo, F.; Peng, R.; Chen, H.; Li, G.; Ni, B.; Zhao, Z.; Yuan, D. Observations and analyses of the sunrise effect for NWC VLF transmitter signals. Chin. J. Geophys. 2020, 63, 4300–4311. (In Chinese) [Google Scholar]
- Wang, S.; Ni, B.; Gu, X.; Lin, R.; Li, G.; Luo, F.; Peng, R.; Chen, H. Sunrise effect of very-low-frequency JJI transmitter signal propagating over an east-west path. Chin. J. Geophys. 2022, 65, 145–156. (In Chinese) [Google Scholar]
- Pasupathy, S. Minimum shift keying: A spectrally efficient modulation. IEEE Commun. Mag. 1979, 17, 14–22. [Google Scholar] [CrossRef]
- Gross, N.C.; Cohen, M.B.; Said, R.K.; Gołkowski, M. Polarization of Narrowband VLF Transmitter Signals as an Ionospheric Diagnostic. J. Geophys. Res. Space Phys. 2018, 123, 901–917. [Google Scholar] [CrossRef]
- Gu, X.; Wang, Q.; Ni, B.; Xu, W.; Wang, S.; Yi, J.; Hu, Z.; Li, B.; He, F.; Chen, X.; et al. First results of the wave measurements by the WHU VLF wave detection system at the Chinese Great Wall station in Antarctica. J. Geophys. Res. Space Phys. 2022, 127, e2022JA030784. [Google Scholar] [CrossRef]
- Veronig, A.; Temmer, M.; Hanslmeier, A.; Otruba, W.; Messerotti, M. Temporal aspects and frequency distributions of solar soft X-ray flares. Astron. Astrophys. 2002, 382, 1070–1080. [Google Scholar] [CrossRef]
- Lotz, S.I.; Clilverd, M.A. Demonstrating the use of a class of min-max smoothers for D region event detection in narrow band VLF phase. Radio Sci. 2019, 54, 233–244. [Google Scholar] [CrossRef]

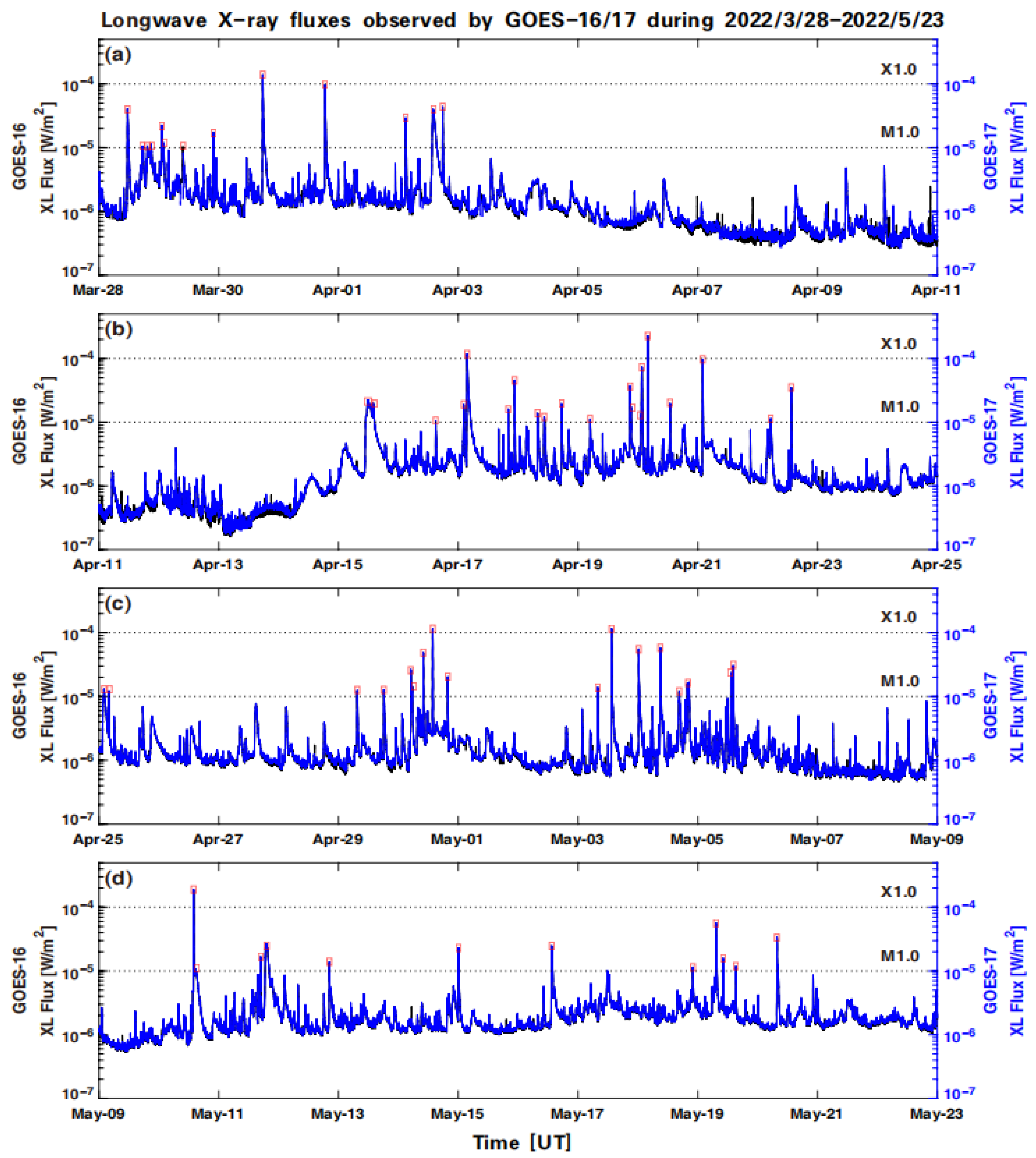
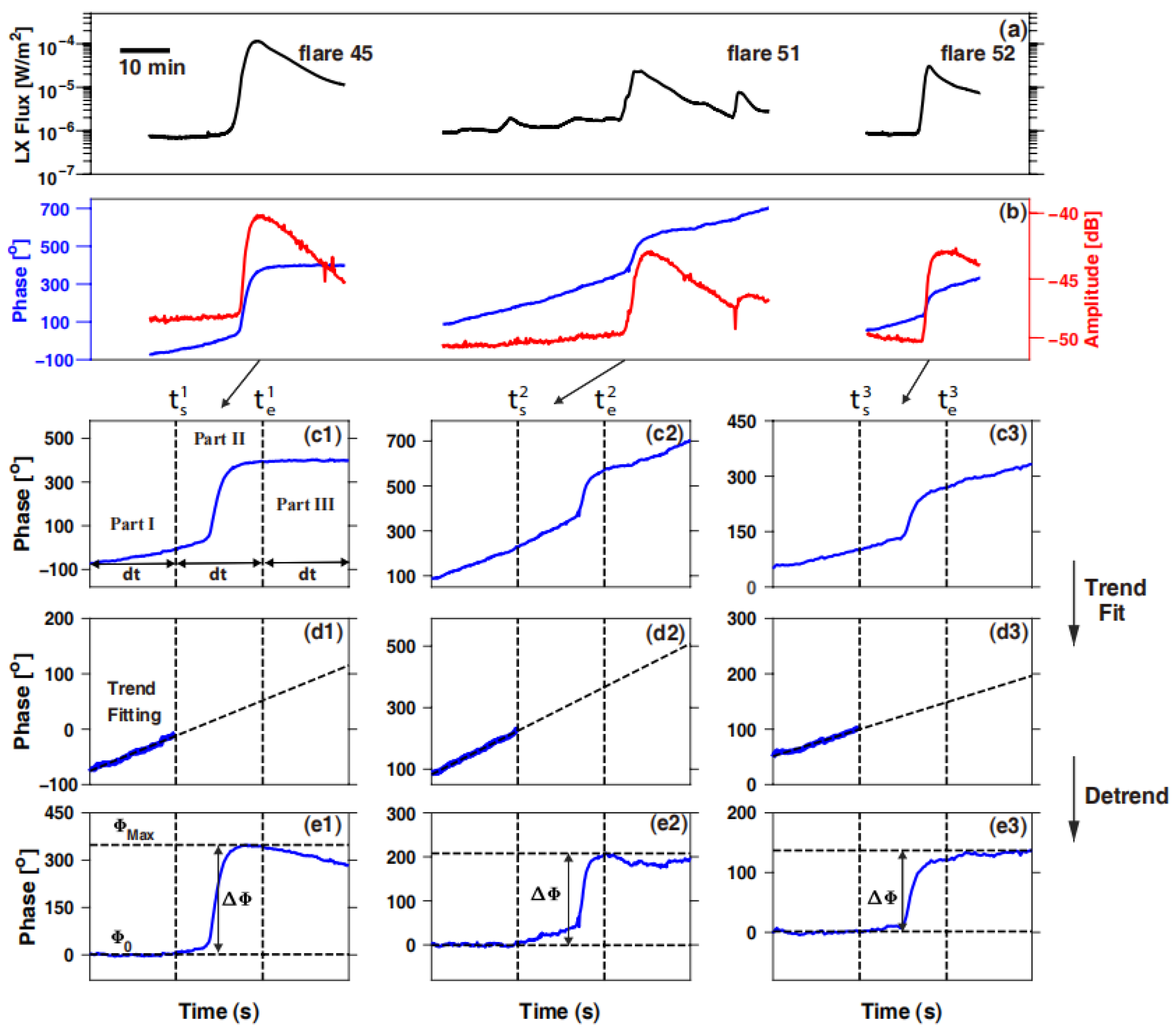



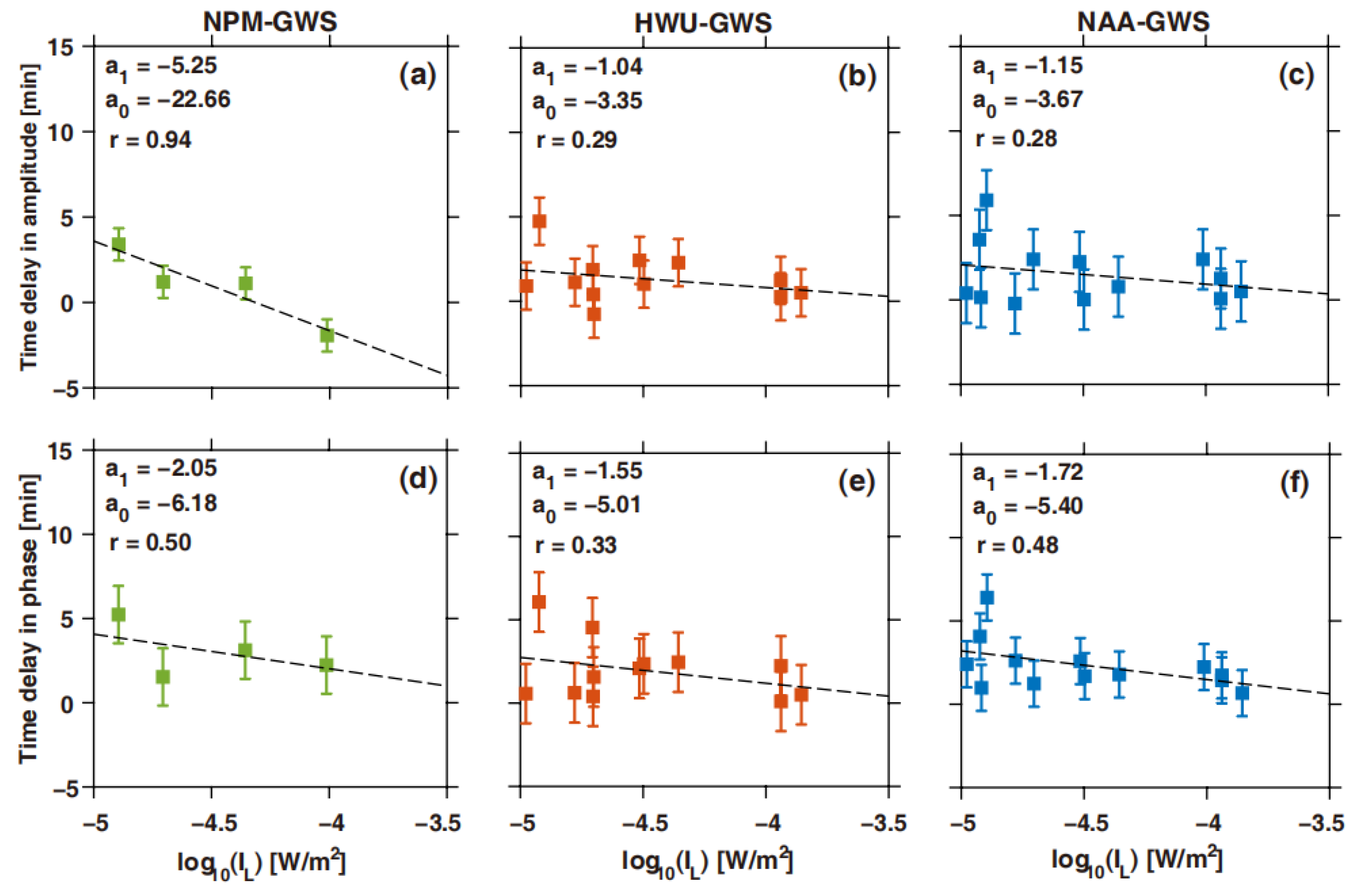

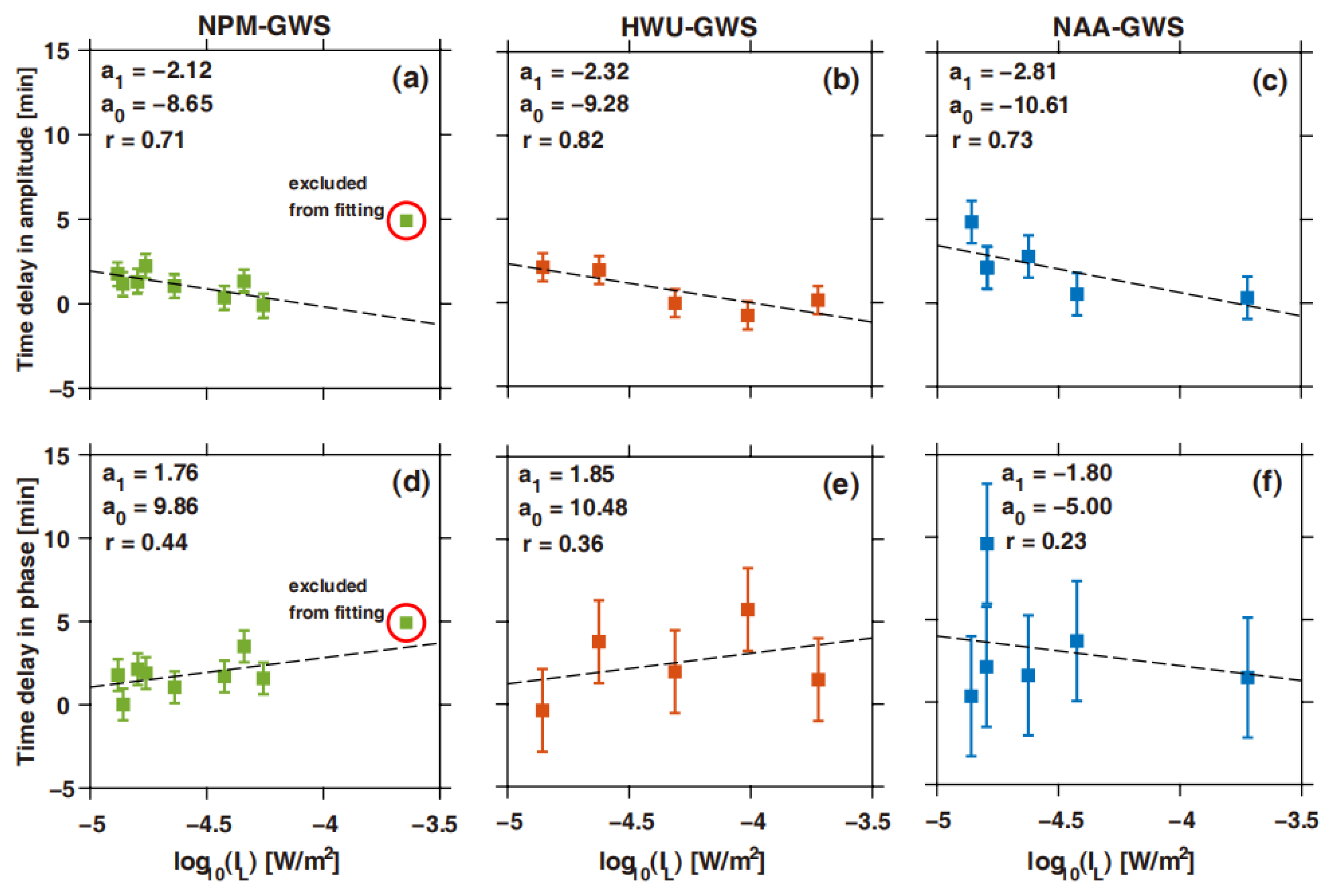
| Path Name | Transmitter Location | Midpoint Location | Path Length | Frequency |
|---|---|---|---|---|
| NPM-GWS | 21.42°N, 158.15°W | 28.12°S, 129.91°W | ~12.6 Mm | 21.4 kHz |
| NAA-GWS | 44.64°N, 67.28°W | 8.81°S, 63.99°W | ~11.9 Mm | 24.0 kHz |
| HWU-GWS | 46.71°N, 1.25°E | 8.89°S, 22.55°W | ~13.2 Mm | 21.75 kHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Zhou, R.; Gu, X.; Xu, W.; Hu, Z.; Ni, B.; Cheng, W.; Feng, J.; Ma, W.; Xu, H.; et al. Examining the Capability of the VLF Technique for Nowcasting Solar Flares Based on Ground Measurements in Antarctica. Remote Sens. 2024, 16, 2092. https://doi.org/10.3390/rs16122092
Wang S, Zhou R, Gu X, Xu W, Hu Z, Ni B, Cheng W, Feng J, Ma W, Xu H, et al. Examining the Capability of the VLF Technique for Nowcasting Solar Flares Based on Ground Measurements in Antarctica. Remote Sensing. 2024; 16(12):2092. https://doi.org/10.3390/rs16122092
Chicago/Turabian StyleWang, Shiwei, Ruoxian Zhou, Xudong Gu, Wei Xu, Zejun Hu, Binbin Ni, Wen Cheng, Jingyuan Feng, Wenchen Ma, Haotian Xu, and et al. 2024. "Examining the Capability of the VLF Technique for Nowcasting Solar Flares Based on Ground Measurements in Antarctica" Remote Sensing 16, no. 12: 2092. https://doi.org/10.3390/rs16122092
APA StyleWang, S., Zhou, R., Gu, X., Xu, W., Hu, Z., Ni, B., Cheng, W., Feng, J., Ma, W., Xu, H., Pan, Y., Li, B., He, F., Chen, X., & Hu, H. (2024). Examining the Capability of the VLF Technique for Nowcasting Solar Flares Based on Ground Measurements in Antarctica. Remote Sensing, 16(12), 2092. https://doi.org/10.3390/rs16122092







