Preliminary Exploration of Coverage for Moon-Based/HEO Spaceborne Bistatic SAR Earth Observation in Polar Regions
Abstract
1. Introduction
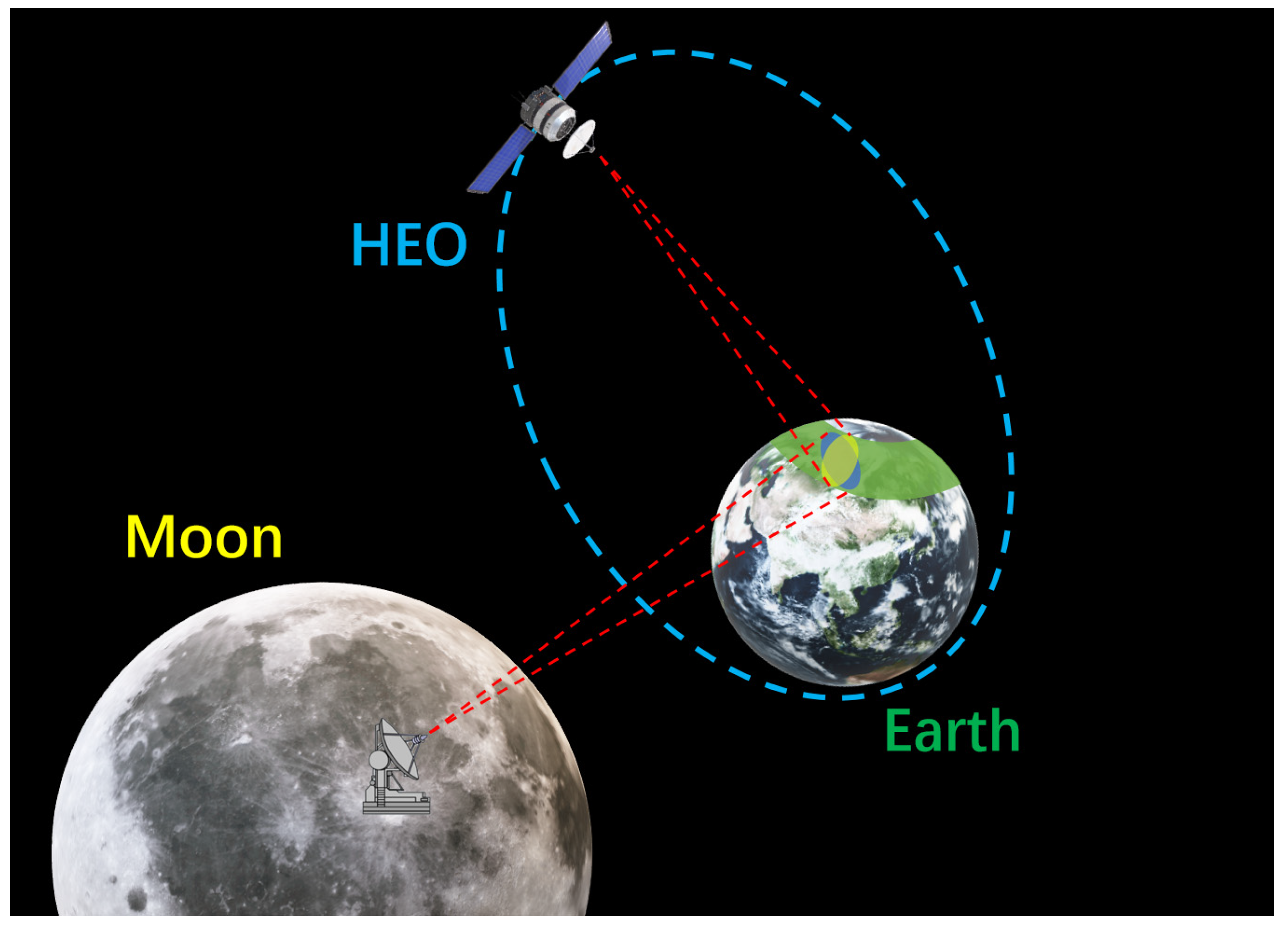
2. Observation Geometry
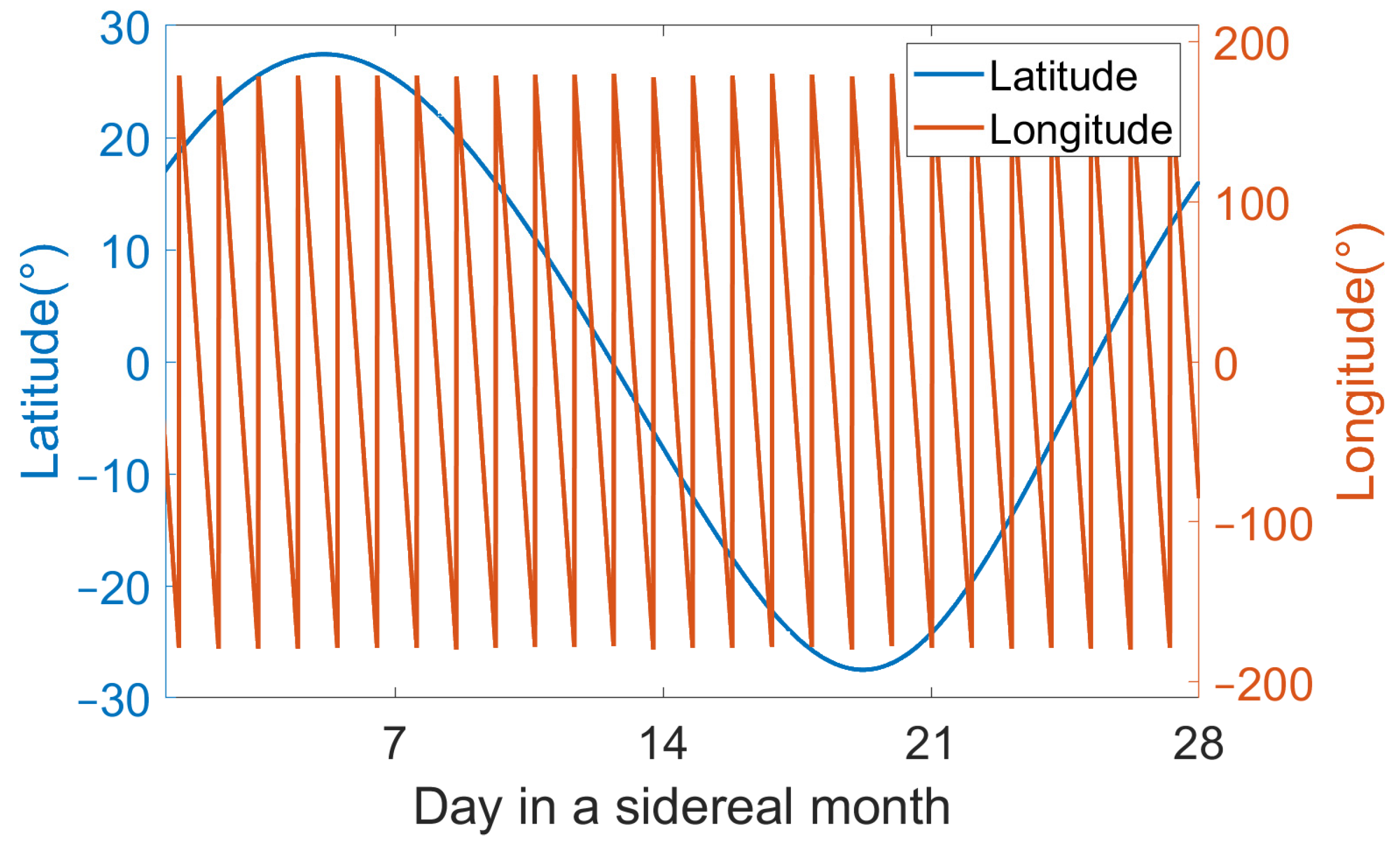
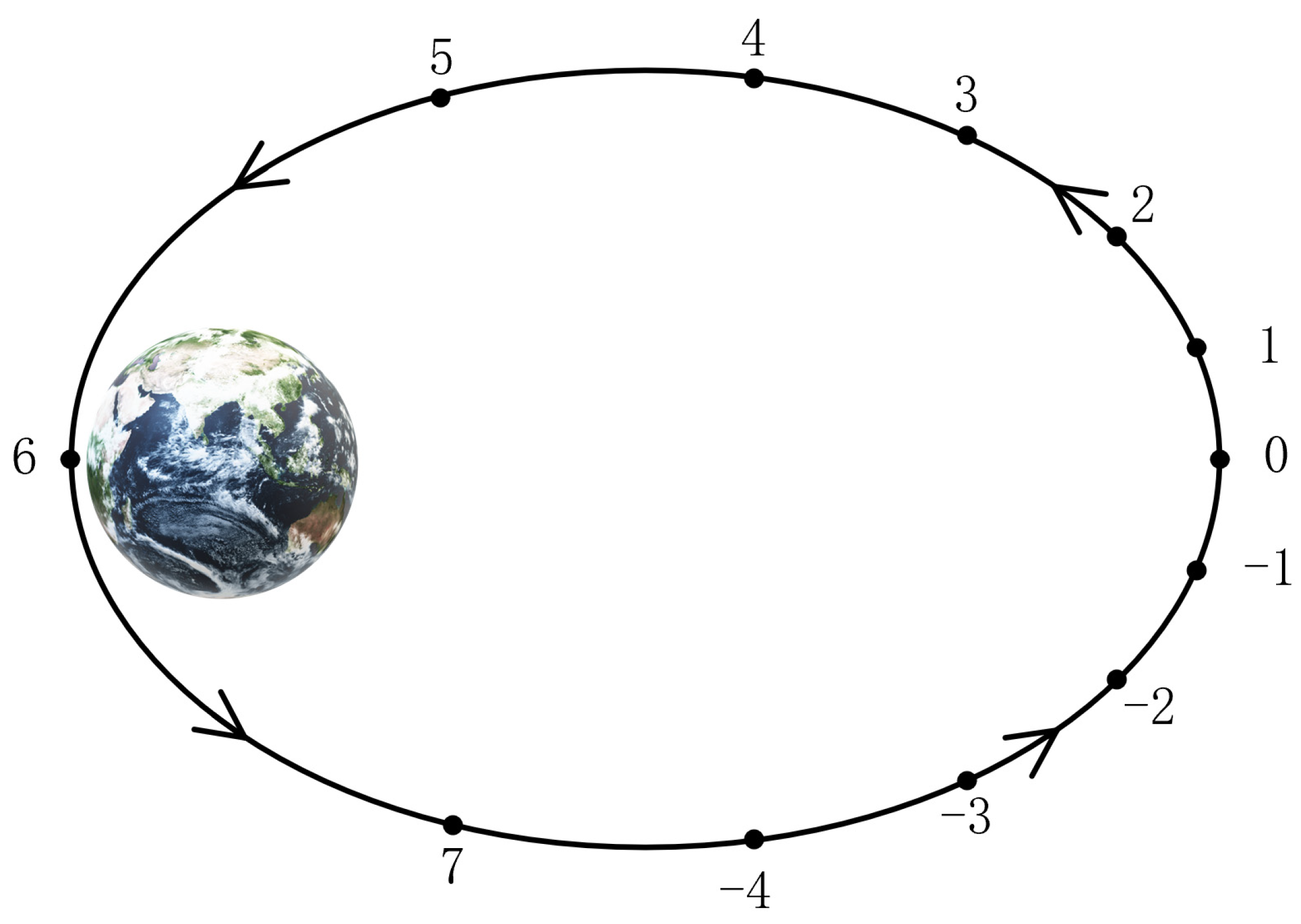
2.1. Dynamics of the Moon and HEO Satellites
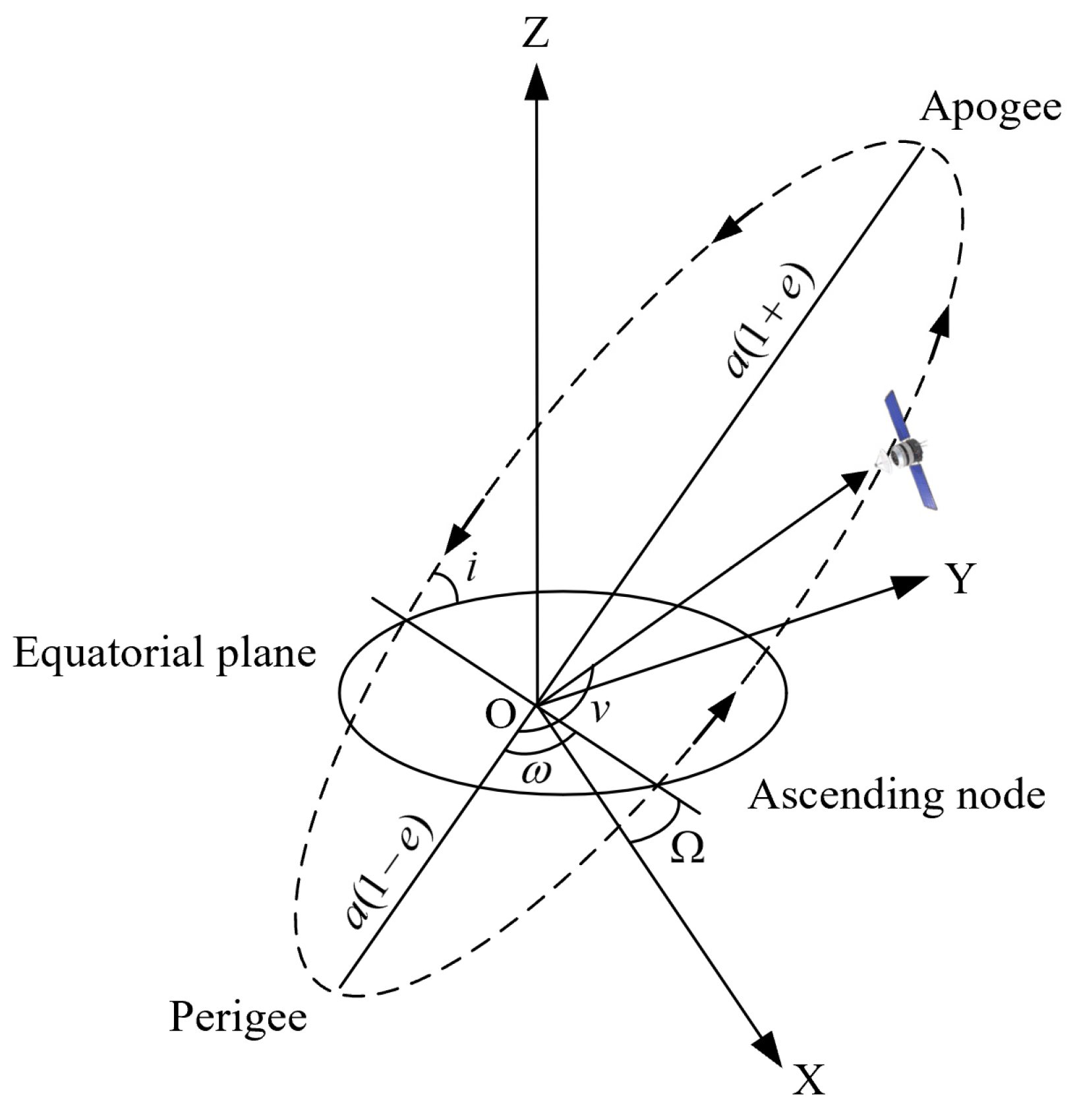
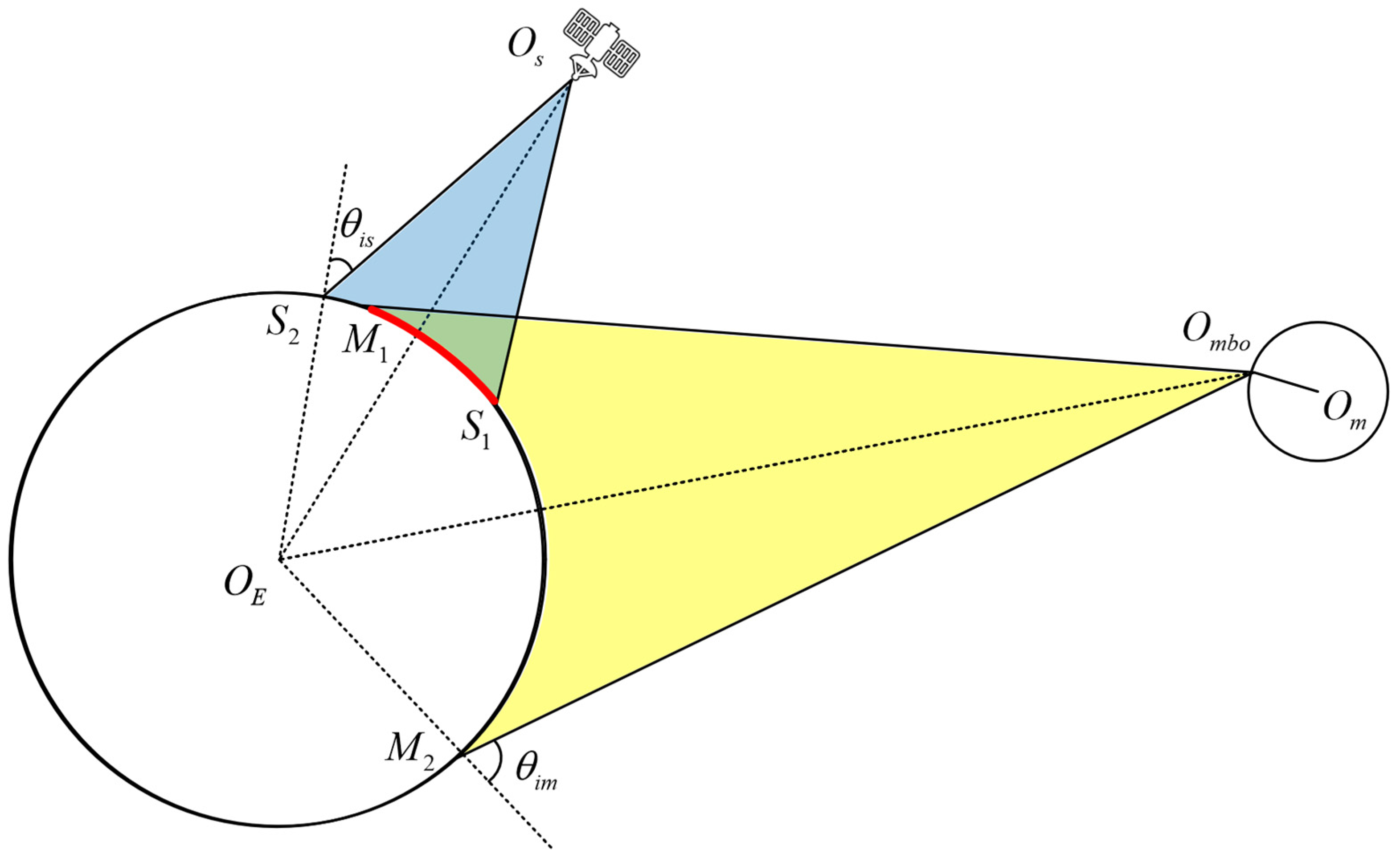
2.2. Observation Geometric Model
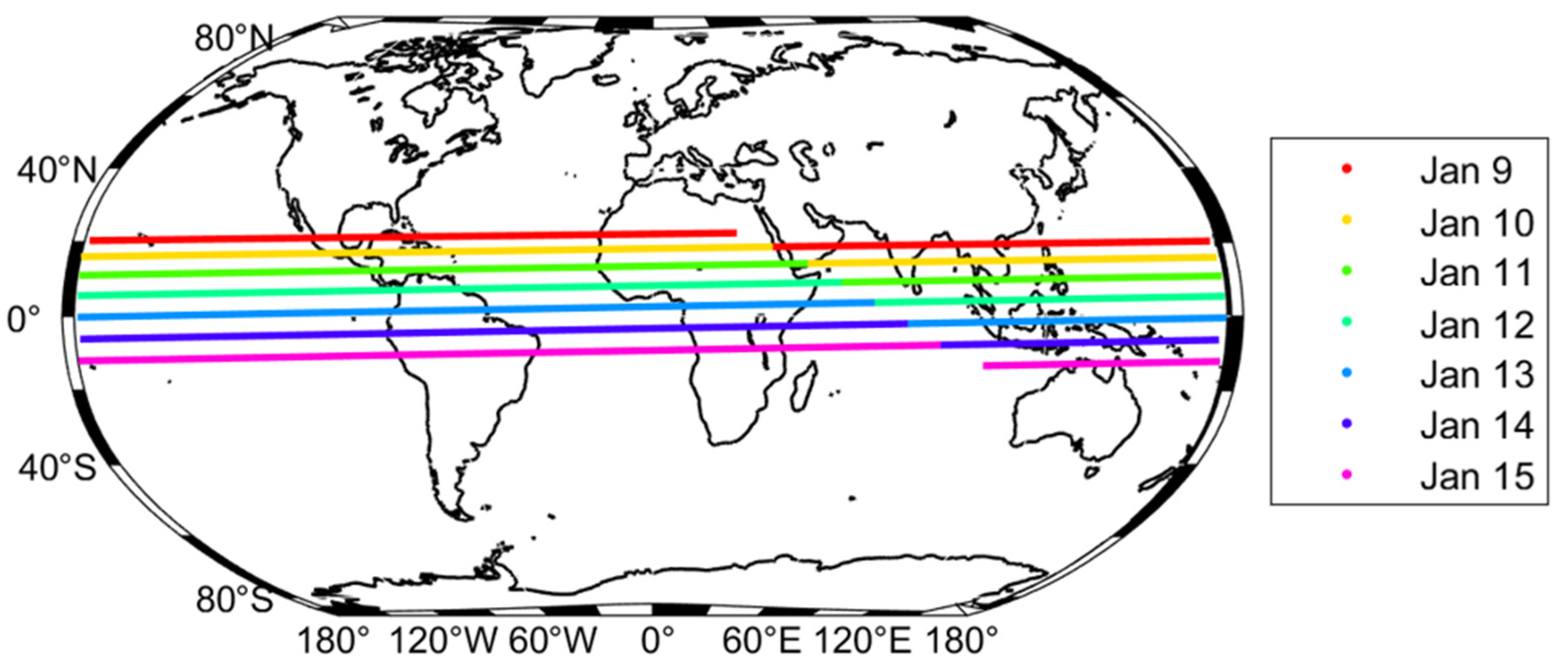
3. Coverage Characteristics of Polar Regions
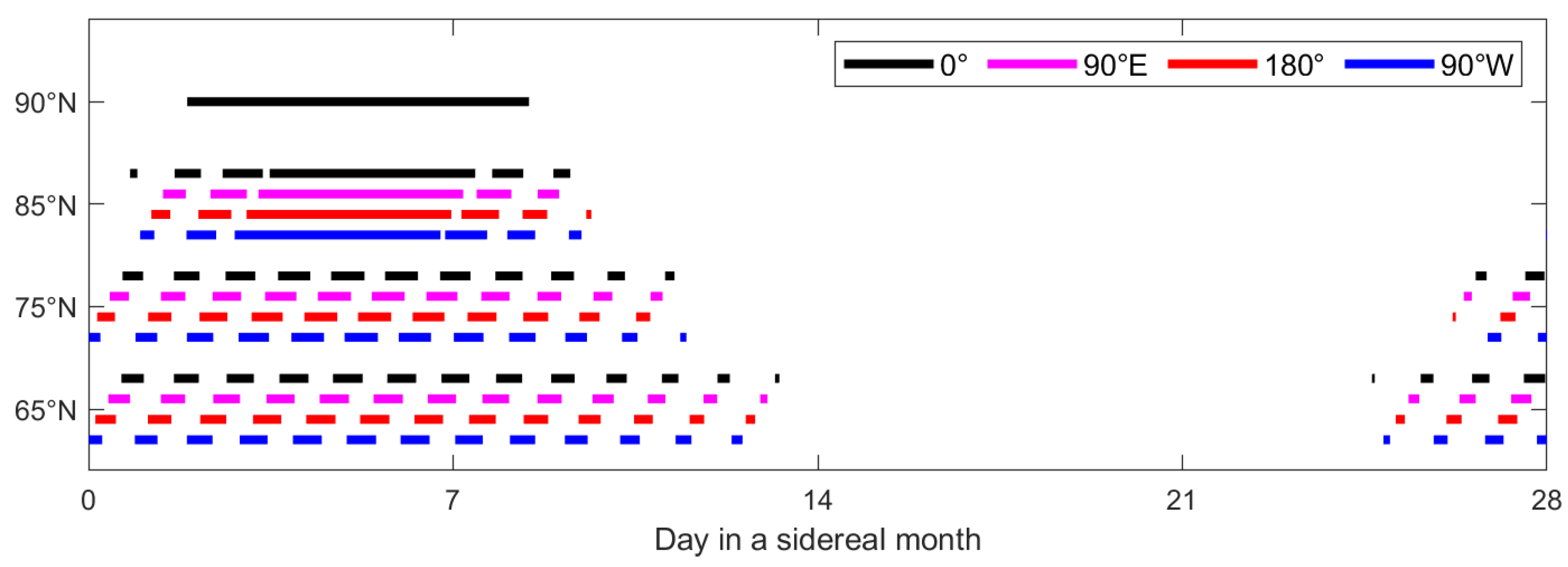
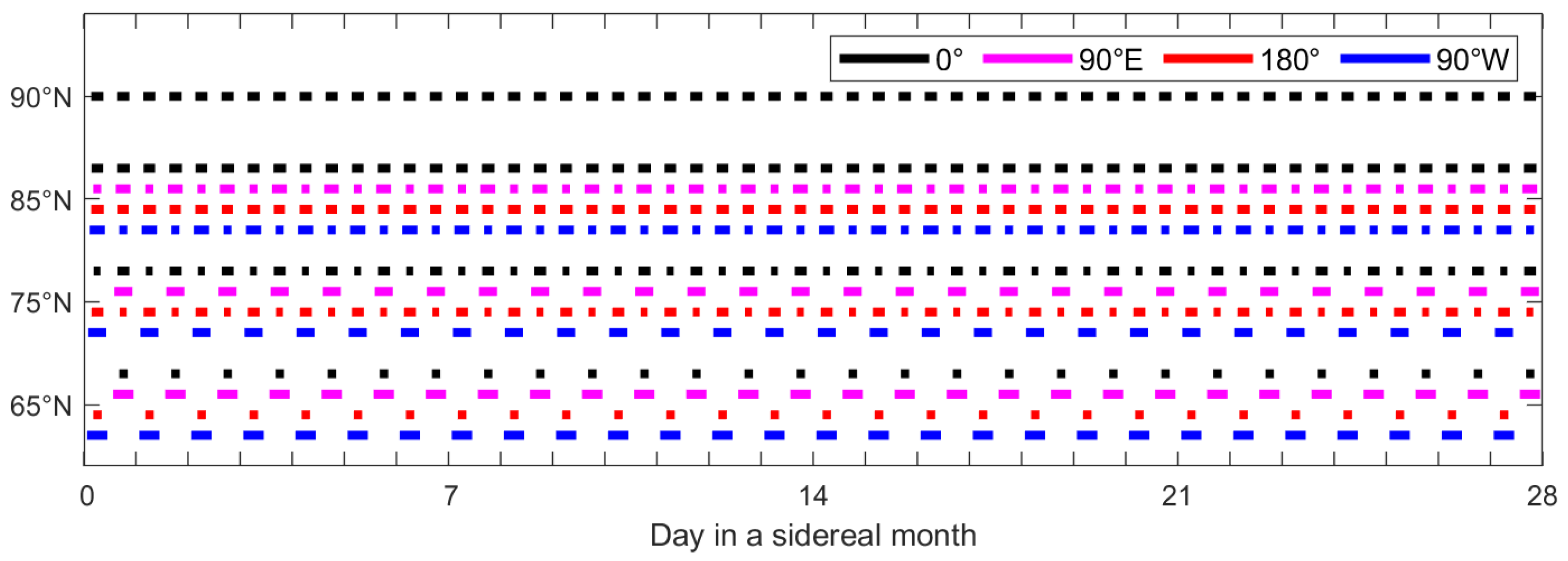
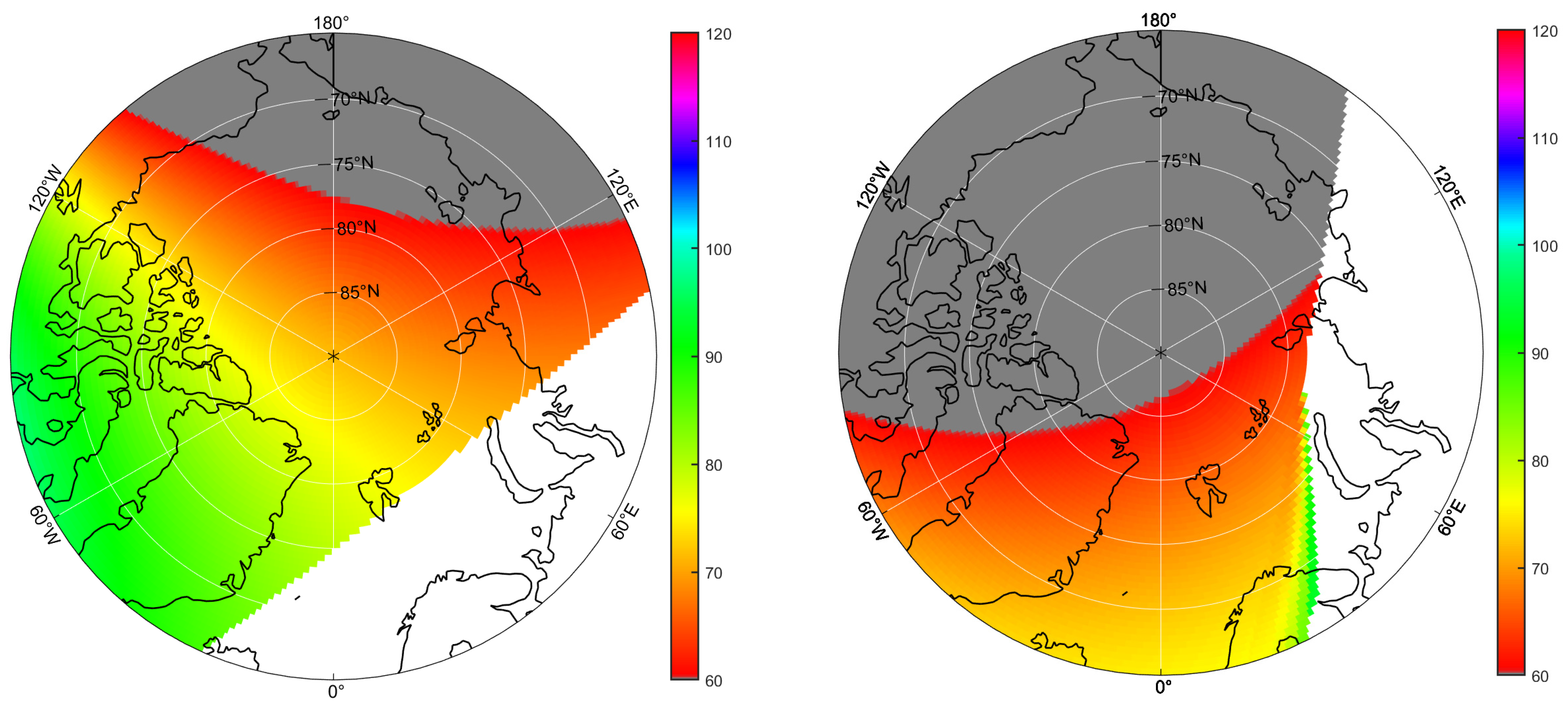
3.1. Coverage Characteristics of Moon-Based and HEO Platforms
3.2. Coverage Characteristics of MH-BiSAR
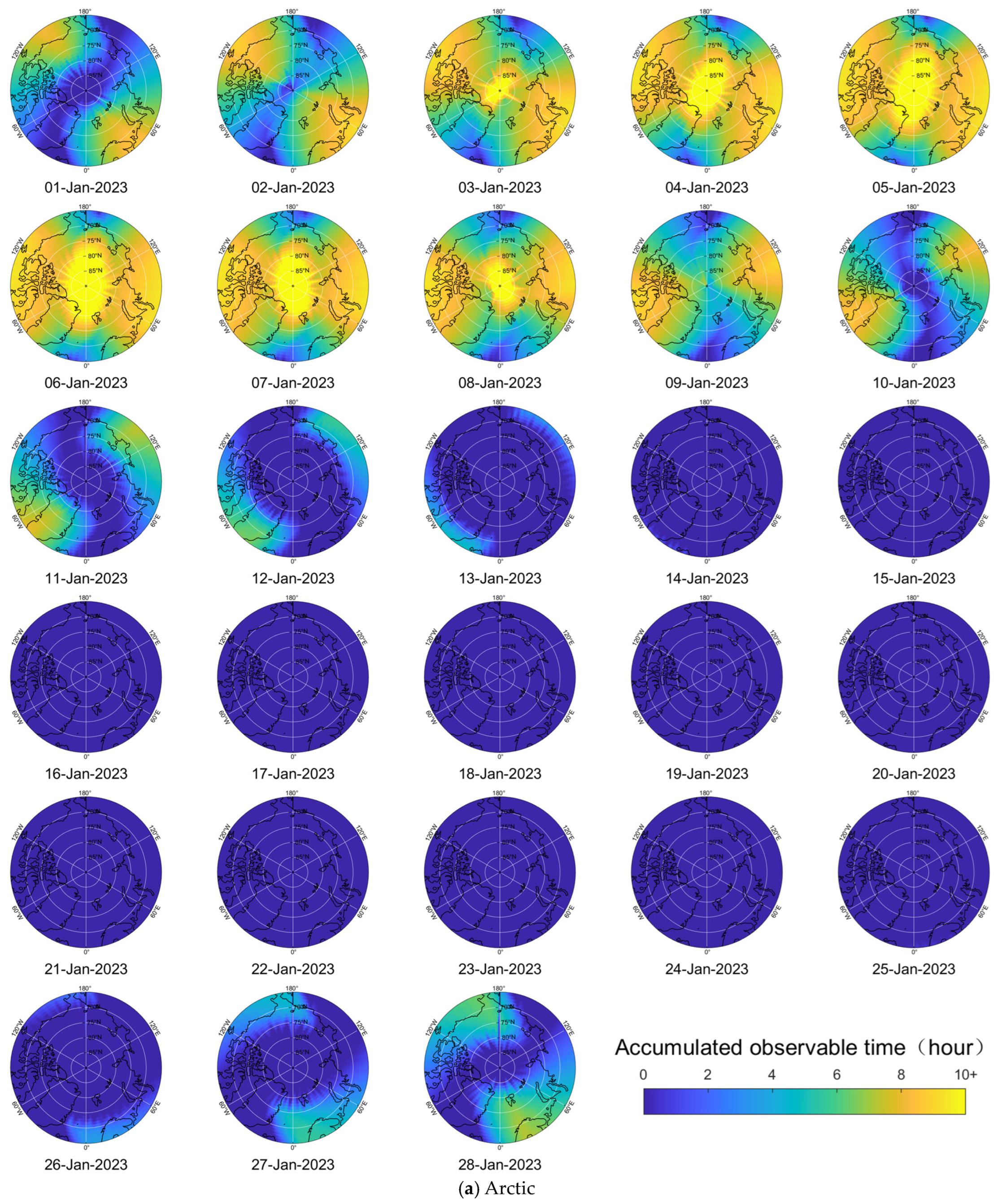
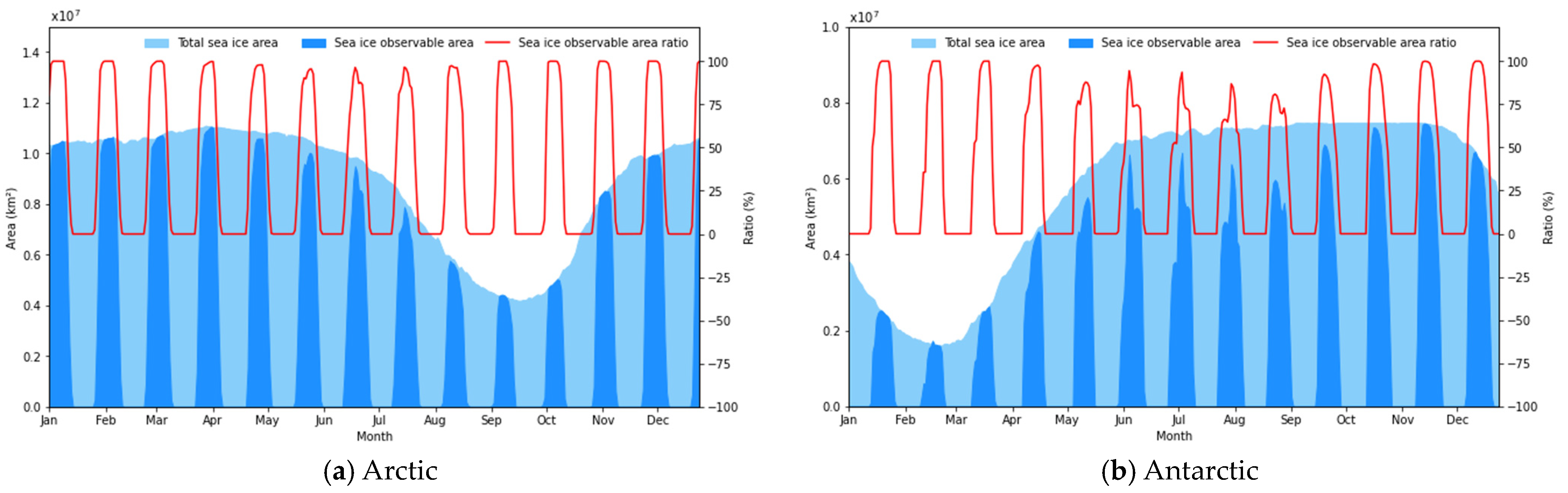
3.3. Potential Applications in Sea Ice Monitoring
4. Limitations of Coverage Analysis Based on Observation Geometry
4.1. Spatial Resolution
4.2. Radiometric Resolution
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Torres, R.; Snoeij, P.; Geudtner, D.; Bibby, D.; Davidson, M.; Attema, E.; Potin, P.; Rommen, B.; Floury, N.; Brown, M.; et al. GMES Sentinel-1 mission. Remote Sens. Environ. 2012, 120, 9–24. [Google Scholar] [CrossRef]
- Pan, L.; Powell, E.M.; Latychev, K.; Mitrovica, J.X.; Creveling, J.R.; Gomez, N.; Hoggard, M.J.; Clark, P.U. Rapid postglacial rebound amplifies global sea level rise following West Antarctic Ice Sheet collapse. Sci. Adv. 2021, 7, eabf7787. [Google Scholar] [CrossRef] [PubMed]
- Rounce, D.R.; Hock, R.; Maussion, F.; Hugonnet, R.; Kochtitzky, W.; Huss, M.; Berthier, E.; Brinkerhoff, D.; Compagno, L.; Copland, L.; et al. Global glacier change in the 21st century: Every increase in temperature matters. Science 2023, 379, 78–83. [Google Scholar] [CrossRef]
- Hwang, B.; Aksenov, Y.; Blockley, E.; Tsamados, M.; Brown, T.; Landy, J.; Stevens, D.; Wilkinson, J. Impacts of climate change on Arctic sea ice. MCCIP Sci. Rev. 2020, 2020, 208–227. [Google Scholar]
- Trishchenko, A.P.; Garand, L. Observing polar regions from space: Advantages of a satellite system on a highly elliptical orbit versus a constellation of low Earth polar orbiters. Can. J. Remote Sens. 2012, 38, 12–24. [Google Scholar] [CrossRef]
- Asmus, V.V.; Milekhin, O.E.; Kramareva, L.S.; Khailov, M.N.; Shirshakov, A.E.; Shumakov, I.A. Arktika-M: The World’s First Highly Elliptical Orbit Hydrometeorological Space System. Russ. Meteorol. Hydrol. 2021, 46, 805–816. [Google Scholar] [CrossRef]
- Li, C.; Liu, J.; Duan, C. Imaging characteristic for large elliptical orbit SAR. In Proceedings of the 2019 6th Asia-Pacific Conference on Synthetic Aperture Radar (APSAR), Xiamen, China, 26–29 November 2019; pp. 1–5. [Google Scholar]
- Hu, X.; Wang, P.; Zeng, H.; Guo, Y. An Improved Equivalent Squint Range Model and Imaging Approach for Sliding Spotlight SAR Based on Highly Elliptical Orbit. Remote Sens. 2021, 13, 4883. [Google Scholar] [CrossRef]
- Hobbs, S.; Mitchell, C.; Forte, B.; Holley, R.; Snapir, B.; Whittaker, P. System Design for Geosynchronous Synthetic Aperture Radar Missions. IEEE Trans. Geosci. Remote Sens. 2014, 52, 7750–7763. [Google Scholar] [CrossRef]
- Moussessian, A.; Chen, C.; Edelstein, W.; Madsen, S.; Rosen, P. System concepts and technologies for high orbit SAR. In Proceedings of the IEEE MTT-S International Microwave Symposium Digest, 2005, Long Beach, CA, USA, 17 June; 2005; pp. 1623–1626. [Google Scholar]
- Lu, Y.F.; Shao, Q.; Yue, H.H.; Yang, F. A Review of the Space Environment Effects on Spacecraft in Different Orbits. IEEE Access 2019, 7, 93473–93488. [Google Scholar] [CrossRef]
- Trichtchenko, L.D.; Nikitina, L.V.; Trishchenko, A.P.; Garand, L. Highly Elliptical Orbits for Arctic observations: Assessment of ionizing radiation. Adv. Space Res. 2014, 54, 2398–2414. [Google Scholar] [CrossRef][Green Version]
- Xu, L.; Li, H.; Pei, Z.; Zou, Y.; Wang, C. A Brief Introduction to the International Lunar Research Station Program and the Interstellar Express Mission. Chin. J. Space Sci. 2022, 42, 511–513. [Google Scholar] [CrossRef]
- Weiren, W. International Lunar Research Station. Aerosp. China 2023, 24, 10–14. [Google Scholar]
- Guo, H.D.; Ding, Y.X.; Liu, G. Moon-based Earth observation. Sci. Bull. 2022, 67, 2036–2039. [Google Scholar] [CrossRef]
- Guo, H.D.; Liu, G.; Ding, Y.X. Moon-based Earth observation: Scientific concept and potential applications. Int. J. Digit. Earth 2018, 11, 546–557. [Google Scholar] [CrossRef]
- Fornaro, G.; Franceschetti, G.; Lombardini, F.; Mori, A.; Calamia, M. Potentials and Limitations of Moon-Borne SAR Imaging. IEEE Trans. Geosci. Remote Sens. 2010, 48, 3009–3019. [Google Scholar] [CrossRef]
- Liu, H.Y.; Guo, H.D.; Liu, G.; Ding, Y.X. An exploratory study on moon-based observation coverage of sea ice from the geometry. Int. J. Remote Sens. 2020, 41, 6089–6098. [Google Scholar] [CrossRef]
- Behner, F.; Reuter, S.; Nies, H.; Loffeld, O. Synchronization and Processing in the HITCHHIKER Bistatic SAR Experiment. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2016, 9, 1028–1035. [Google Scholar] [CrossRef]
- Cai, Y.H.; Li, J.F.; Yang, Q.Y.; Liang, D.; Liu, K.Y.; Zhang, H.; Lu, P.P.; Wang, R. First Demonstration of RFI Mitigation in the Phase Synchronization of LT-1 Bistatic SAR. IEEE Trans. Geosci. Remote Sens. 2023, 61, 5217319. [Google Scholar] [CrossRef]
- Sun, Z.C.; Wu, J.J.; Huang, Y.L.; Yang, J.Y.; Yang, H.G.; Yang, X.B. Performance Analysis and Mission Design for Inclined Geosynchronous Spaceborne-Airborne Bistatic SAR. In Proceedings of the 2015 IEEE Radar Conference (RadarCon), Arlington, VA, USA, 10–15 May 2015; pp. 1177–1181. [Google Scholar]
- Sun, Z.C.; Wu, J.J.; Pei, J.F.; Li, Z.Y.; Huang, Y.L.; Yang, J.Y. Inclined Geosynchronous Spaceborne-Airborne Bistatic SAR: Performance Analysis and Mission Design. IEEE Trans. Geosci. Remote Sens. 2016, 54, 343–357. [Google Scholar] [CrossRef]
- Zhang, K.; Guo, H.; Jiang, D.; Han, C. Analysis of Geometric Characteristics and Coverage for Moon-Based/Spaceborne Bistatic SAR Earth Observation. Remote Sens. 2023, 15, 2151. [Google Scholar] [CrossRef]
- Folkner, W.M.; Williams, J.G.; Boggs, D.H.; Park, R.S.; Kuchynka, P. The planetary and lunar ephemerides DE430 and DE431. Interplanet. Netw. Prog. Rep. 2014, 196, 42–196. [Google Scholar]
- Bizouard, C.; Lambert, S.; Gattano, C.; Becker, O.; Richard, J.-Y. The IERS EOP 14C04 solution for Earth orientation parameters consistent with ITRF 2014. J. Geod. 2019, 93, 621–633. [Google Scholar] [CrossRef]
- Xu, Z.; Chen, K.S.; Liu, G.; Guo, H.D. Spatiotemporal Coverage of a Moon-Based Synthetic Aperture Radar: Theoretical Analyses and Numerical Simulations. IEEE Trans. Geosci. Remote Sens. 2020, 58, 8735–8750. [Google Scholar] [CrossRef]
- Kidder, S.Q.; Vonderhaar, T.H. On the Use of Satellites in Molniya Orbits for Meteorological Observation of Middle and High-Latitudes. J. Atmos. Ocean. Technol. 1990, 7, 517–522. [Google Scholar] [CrossRef]
- Bonin, G.; King, J.; Brett, M.; Wilson, S. Antarctic Broadband: Ka-Band Communications for the Bottom of the Earth. In Proceedings of the 30th AIAA International Communications Satellite System Conference (ICSSC), Ottawa, ON, Canada, 24–27 September 2012; p. 15191. [Google Scholar]
- Moccia, A.; Renga, A. Synthetic Aperture Radar for Earth Observation from a Lunar Base: Performance and Potential Applications. IEEE Trans. Aerosp. Electron. Syst. 2010, 46, 1034–1051. [Google Scholar] [CrossRef]
- Trishchenko, A.P.; Garand, L. Spatial and Temporal Sampling of Polar Regions from Two-Satellite System on Molniya Orbit. J. Atmos. Ocean. Technol. 2011, 28, 977–992. [Google Scholar] [CrossRef]
- Fetterer, F.; Knowles, K.; Meier, W.N.; Savoie, M.; Windnagel, A.K. Sea Ice Index; Version 3; Data Set; National Snow and Ice Data Center: Boulder, CO, USA, 2017. [Google Scholar] [CrossRef]
- Wu, J.; Sun, Z.; Yang, J.; Lyu, Z.; Li, D.; Miao, Y.; Chen, T.; Zuo, W.; Li, C.; Hai, Y.; et al. Spaceborne Airborne Bistatic SAR Using GF-3 Illumination—Technology and Experiment. Radar Science and Technology. Radar Sci. Technol. 2021, 19, 241–247. [Google Scholar]
- Wu, T.D.; Chen, K.S.; Shi, J.C.; Lee, H.W.; Fung, A.K. A study of an AIEM model for bistatic scattering from randomly rough surfaces. IEEE Trans. Geosci. Remote Sens. 2008, 46, 2584–2598. [Google Scholar] [CrossRef]







| Parameter | Value |
|---|---|
| Semi-major axis (km) | 26,500 |
| Argument of Perigee (deg) | 270 |
| Eccentricity | 0.741 |
| Inclination (deg) | 63.4 |
| Perigee altitude (km) | ~600 |
| Apogee altitude (km) | ~39,750 |
| Orbital period (day) | ~0.5 |
| Orbital revisit period (day) | ~1 |
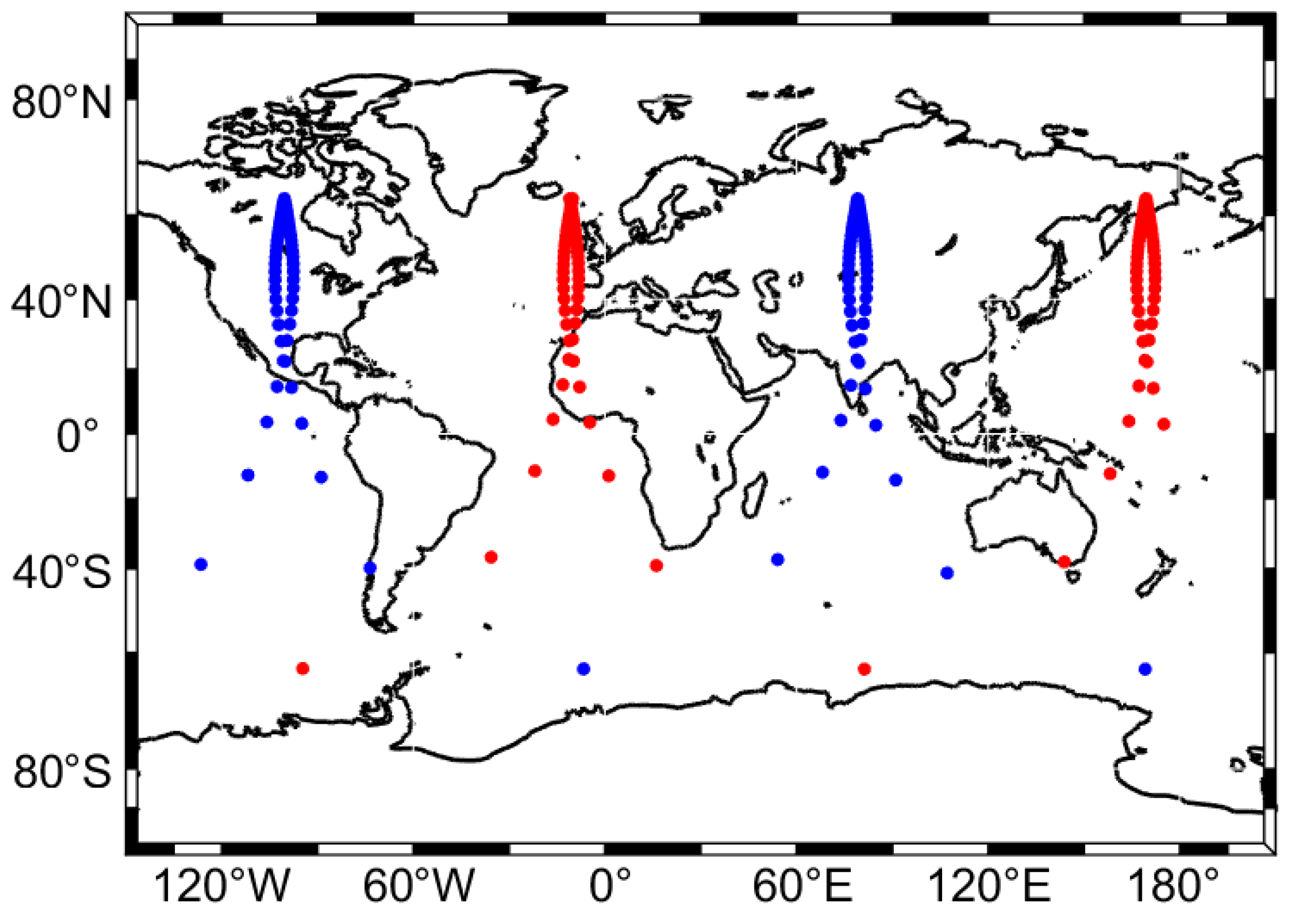
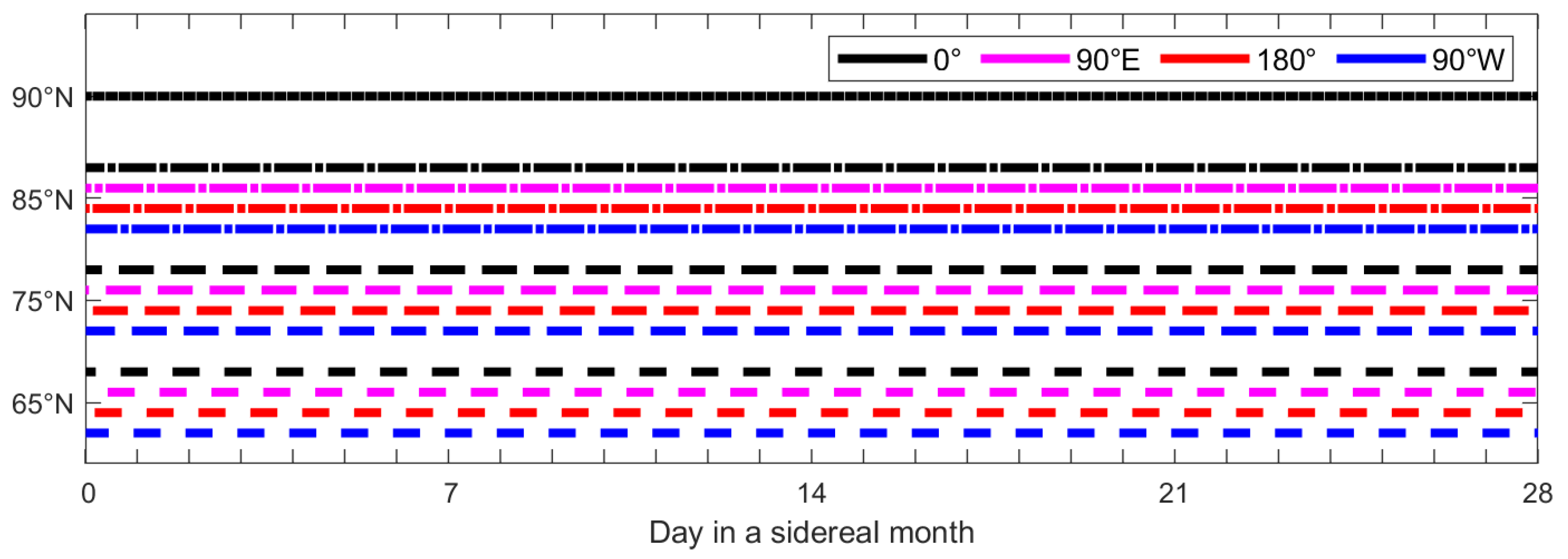
| Sample Points | Annual Accumulated Observable Time (Hours) | CV | ||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 2005 | 2006 | 2007 | 2008 | 2009 | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 | 2016 | 2017 | 2018 | 2019 | 2020 | 2021 | 2022 | 2023 | ||
| (90°N) | 6039 | 6178 | 6331 | 6389 | 5348 | 4945 | 4499 | 3735 | 2862 | 290 | 0 | 0 | 0 | 0 | 0 | 1673 | 3292 | 4756 | 5419 | 0.781 |
| (85°N, 0°E) | 5696 | 5925 | 6075 | 6090 | 5101 | 4641 | 3944 | 3101 | 2766 | 2081 | 1596 | 1255 | 1075 | 1227 | 1679 | 2121 | 2817 | 4102 | 5010 | 0.518 |
| (85°N, 90°E) | 5334 | 5559 | 5706 | 5734 | 4828 | 4360 | 3710 | 2961 | 2655 | 1959 | 1418 | 1238 | 941 | 1100 | 1623 | 1956 | 2640 | 3854 | 4688 | 0.521 |
| (85°N, 180°) | 5599 | 5790 | 5980 | 6026 | 5042 | 4539 | 3832 | 3049 | 2756 | 2041 | 1556 | 1221 | 1069 | 1218 | 1671 | 2088 | 2717 | 4058 | 4910 | 0.518 |
| (85°N, 90°W) | 5428 | 5622 | 5778 | 5847 | 4895 | 4381 | 3754 | 3022 | 2671 | 2039 | 1462 | 1267 | 960 | 1093 | 1654 | 2014 | 2704 | 3923 | 4732 | 0.518 |
| (75°N, 0°) | 4104 | 4145 | 4231 | 4233 | 3618 | 3490 | 3288 | 3061 | 3256 | 2997 | 2848 | 2860 | 2593 | 2510 | 2725 | 2693 | 3000 | 3563 | 3748 | 0.174 |
| (75°N, 90°E) | 3968 | 3845 | 3936 | 4094 | 3409 | 3327 | 3208 | 2883 | 3082 | 2847 | 2548 | 2750 | 2308 | 2245 | 2667 | 2486 | 2790 | 3485 | 3494 | 0.185 |
| (75°N, 180°) | 4122 | 4115 | 4231 | 4241 | 3620 | 3488 | 3298 | 3089 | 3263 | 3006 | 2850 | 2872 | 2591 | 2504 | 2722 | 2691 | 2972 | 3574 | 3730 | 0.173 |
| (75°N, 90°W) | 3939 | 3874 | 3929 | 4110 | 3396 | 3270 | 3238 | 2891 | 3063 | 2871 | 2552 | 2731 | 2336 | 2252 | 2668 | 2476 | 2755 | 3499 | 3481 | 0.184 |
| (65°N, 0°) | 2064 | 2001 | 2143 | 2040 | 1824 | 1878 | 1708 | 1671 | 1904 | 1716 | 1767 | 1831 | 1645 | 1655 | 1661 | 1577 | 1740 | 1840 | 1901 | 0.088 |
| (65°N, 90°E) | 4974 | 4773 | 4838 | 5029 | 4297 | 4286 | 4290 | 3993 | 4377 | 4277 | 3983 | 4322 | 3851 | 3694 | 4067 | 3722 | 3965 | 4597 | 4481 | 0.093 |
| (65°N, 180°) | 2088 | 1995 | 2116 | 2063 | 1837 | 1866 | 1717 | 1676 | 1896 | 1717 | 1749 | 1846 | 1633 | 1650 | 1660 | 1573 | 1732 | 1833 | 1898 | 0.089 |
| (65°N, 90°W) | 4968 | 4789 | 4810 | 5044 | 4317 | 4259 | 4305 | 4011 | 4332 | 4300 | 3988 | 4297 | 3866 | 3686 | 4061 | 3732 | 3931 | 4628 | 4493 | 0.093 |
| Illuminator | Band | Power Density (dbW·m−2) | Required Transmit Power (kW) | |
|---|---|---|---|---|
| 160 m | 80 m | |||
| LEO-SAR | C | −50.3 | 256.3 | 1044.2 |
| MEO-SAR | C | −64.5 | 9.7 | 39.7 |
| GEO-SAR | L | −74.3 | 19.0 | 77.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, K.; Guo, H.; Jiang, D.; Han, C.; Chen, G. Preliminary Exploration of Coverage for Moon-Based/HEO Spaceborne Bistatic SAR Earth Observation in Polar Regions. Remote Sens. 2024, 16, 2086. https://doi.org/10.3390/rs16122086
Zhang K, Guo H, Jiang D, Han C, Chen G. Preliminary Exploration of Coverage for Moon-Based/HEO Spaceborne Bistatic SAR Earth Observation in Polar Regions. Remote Sensing. 2024; 16(12):2086. https://doi.org/10.3390/rs16122086
Chicago/Turabian StyleZhang, Ke, Huadong Guo, Di Jiang, Chunming Han, and Guoqiang Chen. 2024. "Preliminary Exploration of Coverage for Moon-Based/HEO Spaceborne Bistatic SAR Earth Observation in Polar Regions" Remote Sensing 16, no. 12: 2086. https://doi.org/10.3390/rs16122086
APA StyleZhang, K., Guo, H., Jiang, D., Han, C., & Chen, G. (2024). Preliminary Exploration of Coverage for Moon-Based/HEO Spaceborne Bistatic SAR Earth Observation in Polar Regions. Remote Sensing, 16(12), 2086. https://doi.org/10.3390/rs16122086







