Investigation of the Global Spatio-Temporal Characteristics of Astronomical Seeing
Abstract
1. Introduction
2. Data and Methodology
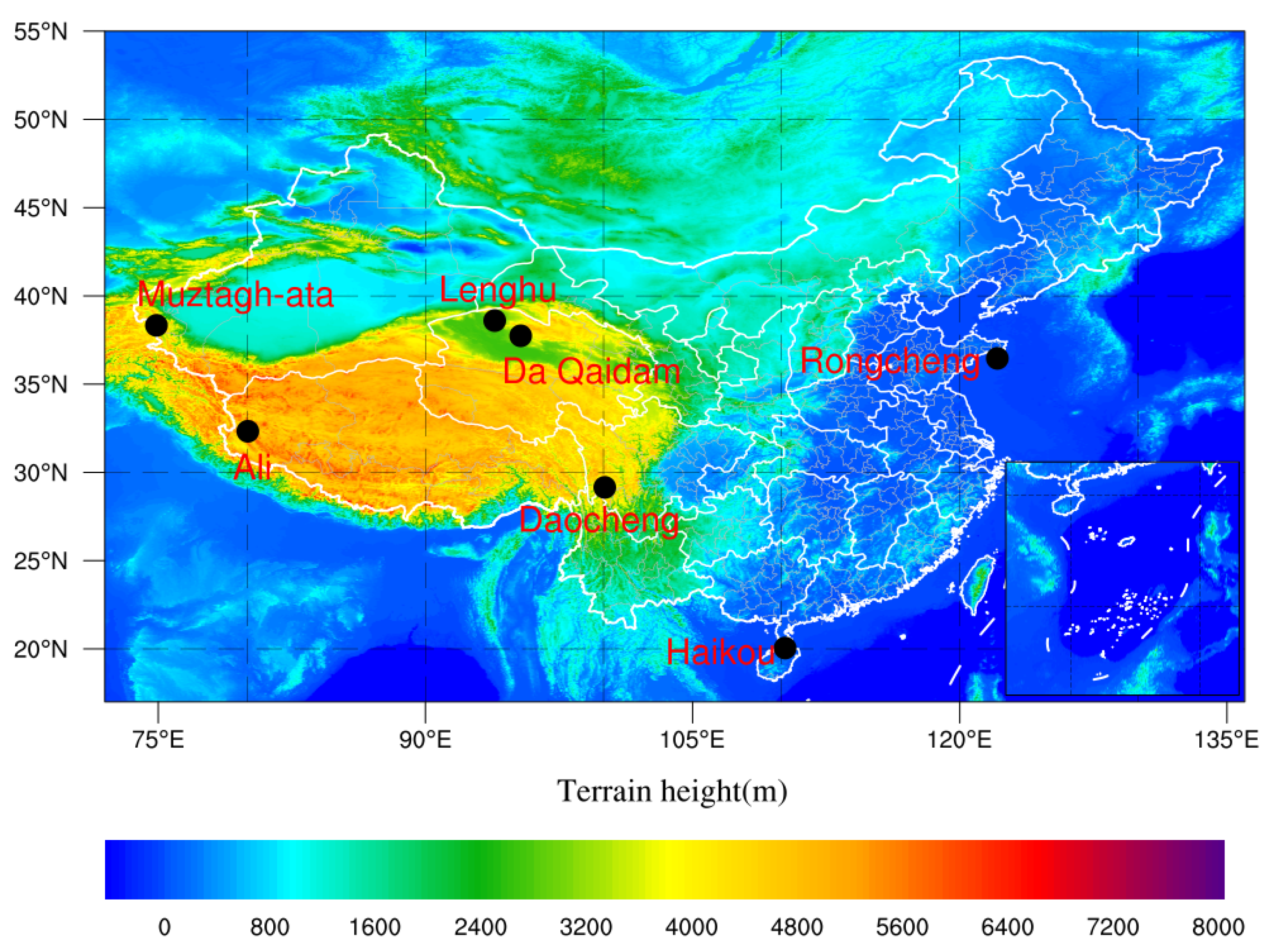
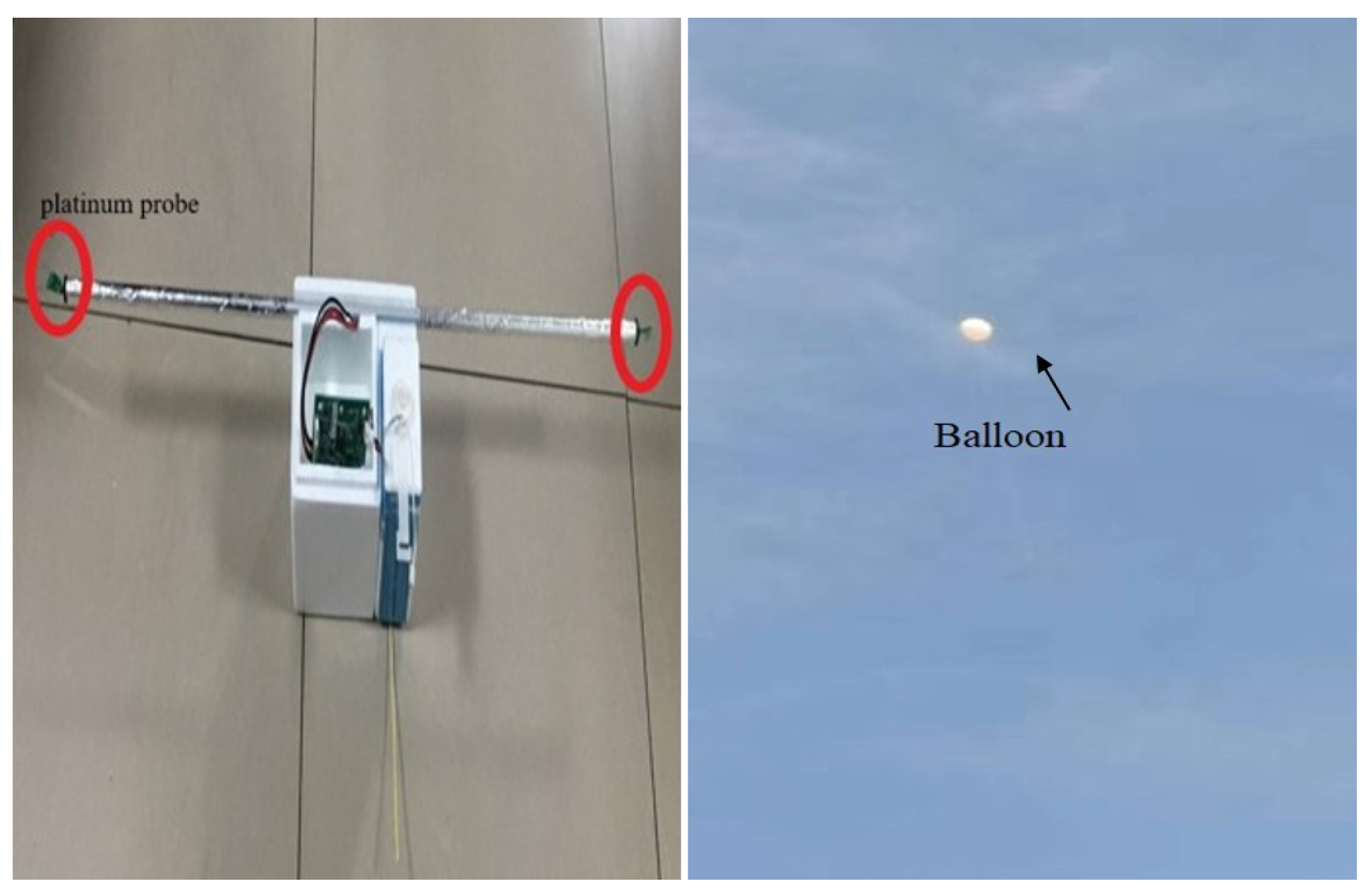
2.1. Balloon-Borne Microthermal and DIMM Measurements
2.2. Methodology
2.3. ERA5 Data
3. Results and Discussion
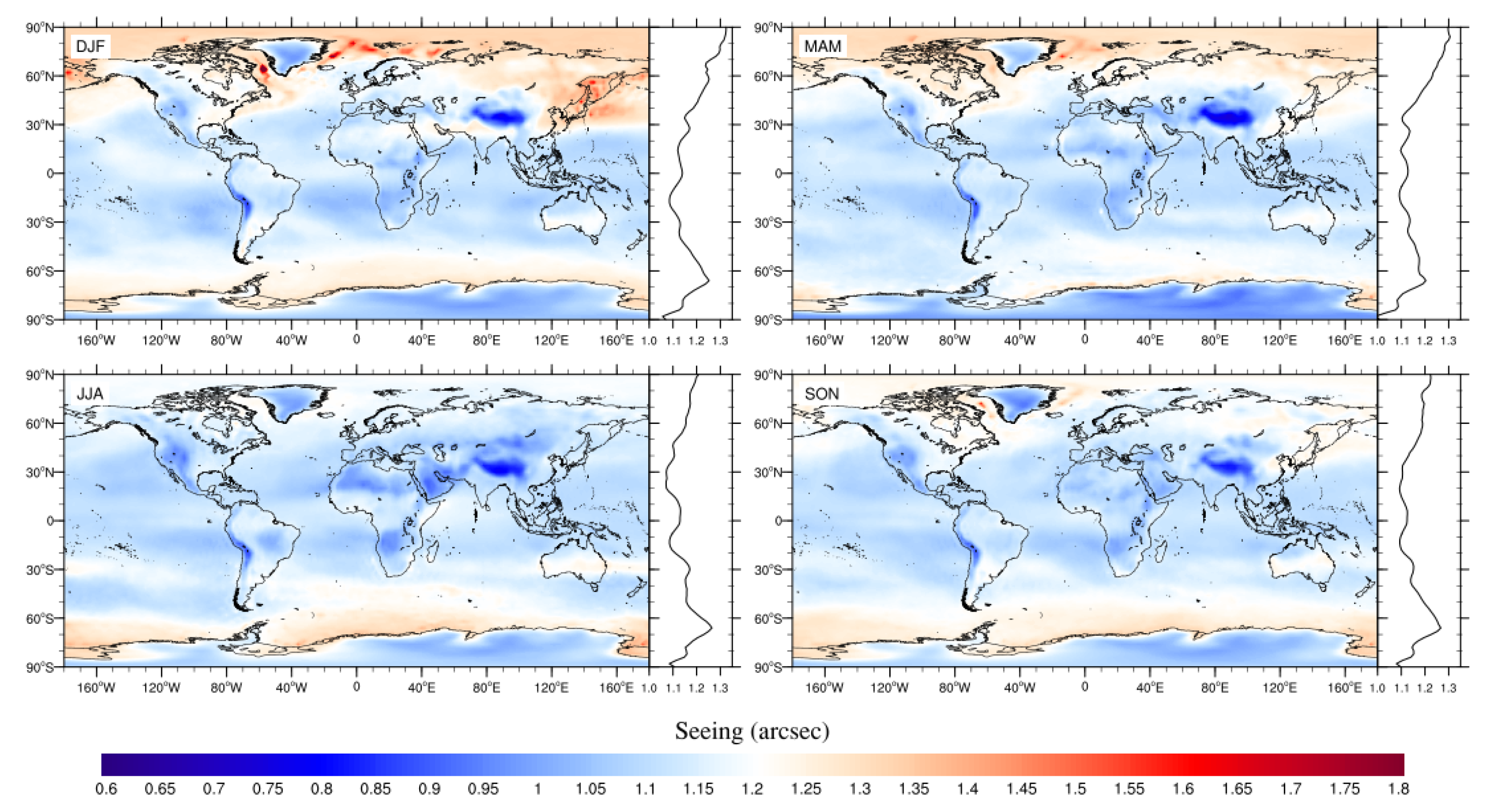
3.1. Global Distribution Characteristics of Seeing
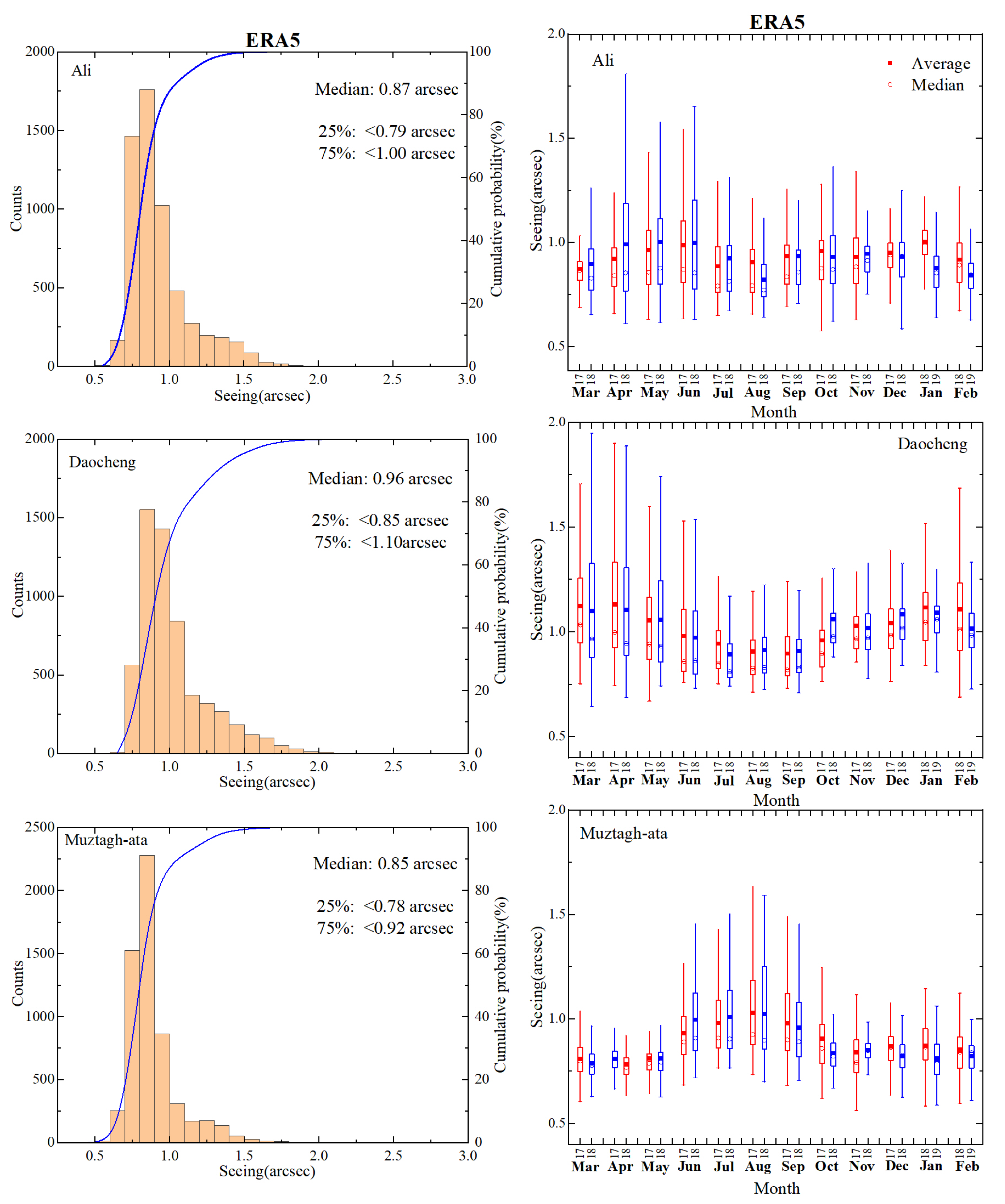
3.2. Verification of the Example Sites
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tatarskii, V.I. Wave Propagation in a Turbulent Medium; McGraw-Hill: New York, NY, USA, 1961. [Google Scholar]
- Hutt, D.L. Modeling and measurement of atmospheric optical turbulence over land. Opt. Eng. 1999, 38, 1288–1295. [Google Scholar] [CrossRef]
- Roddier, F. V the effects of atmospheric turbulence in optical astronomy. In Progress in Optics; Elsevier: Amsterdam, The Netherlands, 1981; Volume 19, pp. 281–376. [Google Scholar]
- Yang, Q.K.; Wu, X.Q.; Han, Y.J.; Qing, C.; Wu, S.; Su, C.D.; Wu, P.F.; Luo, T.; Zhang, S.T. Estimating the astronomical seeing above Dome A using Polar WRF based on the Tatarskii equation. Opt. Express 2021, 29, 44000–44011. [Google Scholar] [CrossRef]
- Deng, L.; Yang, F.; Chen, X.; He, F.; Liu, Q.; Zhang, B.; Zhang, C.; Wang, K.; Liu, N.; Ren, A. Lenghu on the Tibetan Plateau as an astronomical observing site. Nature 2021, 596, 353–356. [Google Scholar] [CrossRef]
- Vernin, J.; Roddier, F. Experimental determination of two-dimensional spatiotemporal power spectra of stellar light scintillation Evidence for a multilayer structure of the air turbulence in the upper troposphere. J. Opt. Soc. Am. 1973, 63, 270–273. [Google Scholar] [CrossRef]
- Avila, R.; Vernin, J.; Masciadri, E. Whole atmospheric-turbulence profiling with generalized scidar. Appl. Opt. 1997, 36, 7898–7905. [Google Scholar] [CrossRef]
- Butterley, T.; Wilson, R.W.; Sarazin, M. Determination of the profile of atmospheric optical turbulence strength from SLODAR data. Mon. Not. R. Astron. Soc. 2006, 369, 835–845. [Google Scholar] [CrossRef]
- Kornilov, V.; Tokovinin, A.; Shatsky, N.; Voziakova, O.; Potanin, S.; Safonov, B. Combined MASS–DIMM instruments for atmospheric turbulence studies. Mon. Not. R. Astron. Soc. 2007, 382, 1268–1278. [Google Scholar] [CrossRef]
- Gimmestad, G.G.; Roberts, D.W.; Stewart, J.M.; Wood, J.W.; Eaton, F.D. Testing of LIDAR system for turbulence profiles. In Proceedings of the SPIE Defense and Security Symposium, Orlando, FL, USA, 18 April 2008; SPIE: Orlando, FL, USA, 2008; p. 695109. [Google Scholar]
- Carlisle, E.; Schmidt, D.; Marino, J.; Guesalaga, A. Use of SLODAR for daytime turbulence profiling. In Proceedings of the Adaptive Optics for Extremely Large Telescopes, Tenerife, Spain, 25–30 June 2017. [Google Scholar]
- Odintsov, S.L.; Gladkikh, V.A.; Kamardin, A.P.; Nevzorova, I.V. Determination of the Structural Characteristic of the Refractive Index of Optical Waves in the Atmospheric Boundary Layer with Remote Acoustic Sounding Facilities. Atmosphere 2019, 10, 711. [Google Scholar] [CrossRef]
- Azouit, M.; Vernin, J. Optical Turbulence Profiling with Balloons Relevant to Astronomy and Atmospheric Physics. Publ. Astron. Soc. Pac. 2005, 117, 536–543. [Google Scholar] [CrossRef]
- Qing, C.; Wu, X.Q.; Li, X.B.; Luo, T.; Su, C.D.; Zhu, W.Y. Mesoscale optical turbulence simulations above Tibetan Plateau: First attempt. Opt. Express 2020, 28, 4571–4586. [Google Scholar] [CrossRef]
- Han, Y.J.; Wu, X.Q.; Luo, T.; Qing, C.; Yang, Q.K.; Jin, X.M.; Liu, N.N.; Wu, S.; Su, C.D. New () statistical model based on first radiosonde turbulence observation over Lhasa. J. Opt. Soc. Am. A 2020, 37, 995–1001. [Google Scholar] [CrossRef] [PubMed]
- Sarazin, M.; Roddier, F. The ESO differential image motion monitor. Astron. Astrophys. 1990, 227, 294–300. [Google Scholar]
- Vernin, J.; Munoz-Tunon, C. Measuring astronomical seeing: The DA/IAC DIMM. Publ. Astron. Soc. Pac. 1995, 107, 265. [Google Scholar] [CrossRef]
- Bally, J.; Theil, D.; Billawala, Y.; Potter, D.; Loewenstein, R.F.; Mrozek, F.; Lloyd, J.P. A Hartmann differential image motion monitor (H-DIMM) for atmospheric turbulence characterisation. Publ. Astron. Soc. Aust. 1996, 13, 22–27. [Google Scholar] [CrossRef]
- Tokovinin, A. From differential image motion to seeing. Publ. Astron. Soc. Pac. 2002, 114, 1156. [Google Scholar] [CrossRef]
- McHugh, J.P.; Jumper, G.Y.; Chun, M. Balloon Thermosonde Measurements over Mauna Kea and Comparison with Seeing Measurements. Publ. Astron. Soc. Pac. 2008, 120, 1318–1324. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, L.; Kong, L.; Bao, H.; Guo, Y.; Rao, X.; Zhong, L.; Zhu, L.; Rao, C. A modified S-DIMM+: Applying additional height grids for characterizing daytime seeing profiles. Mon. Not. R. Astron. Soc. 2018, 478, 1459–1467. [Google Scholar] [CrossRef]
- Tokovinin, A.; Kornilov, V. Accurate seeing measurements with MASS and DIMM. Mon. Not. R. Astron. Soc. 2007, 381, 1179–1189. [Google Scholar] [CrossRef]
- Lyman, R.; Cherubini, T.; Businger, S. Forecasting seeing for the Maunakea Observatories. Mon. Not. R. Astron. Soc. 2020, 496, 4734–4748. [Google Scholar] [CrossRef]
- Han, Y.J.; Yang, Q.K.; Liu, N.N.; Zhang, K.; Qing, C.; Li, X.B.; Wu, X.Q.; Luo, T. Analysis of wind-speed profiles and optical turbulence above Gaomeigu and the Tibetan Plateau using ERA5 data. Mon. Not. R. Astron. Soc. 2021, 501, 4692–4702. [Google Scholar] [CrossRef]
- Kuo, C.L. Assessments of Ali, Dome A, and Summit Camp for mm-wave observations using MERRA-2 reanalysis. Astrophys. J. 2017, 848, 64. [Google Scholar] [CrossRef]
- Kobayashi, S.; Ota, Y.; Harada, Y.; Ebita, A.; Moriya, M.; Onoda, H.; Onogi, K.; Kamahori, H.; Kobayashi, C.; Endo, H. The JRA-55 reanalysis: General specifications and basic characteristics. J. Meteorol. Soc. Japan. Ser. II 2015, 93, 5–48. [Google Scholar] [CrossRef]
- Saha, S.; Moorthi, S.; Wu, X.; Wang, J.; Nadiga, S.; Tripp, P.; Behringer, D.; Hou, Y.T.; Chuang, H.Y.; Iredell, M. The NCEP climate forecast system version 2. J. Clim. 2014, 27, 2185–2208. [Google Scholar] [CrossRef]
- Hersbach, H.; Bell, B.; Berrisford, P.; Hirahara, S.; Horányi, A.; Muñoz-Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Schepers, D.; et al. The ERA5 global reanalysis. Q. J. R. Meteorol. Soc. 2020, 146, 1999–2049. [Google Scholar] [CrossRef]
- Guo, J.P.; Zhang, J.; Yang, K.; Liao, H.; Zhang, S.D.; Huang, K.M.; Lv, Y.M.; Shao, J.; Yu, T.; Tong, B. Investigation of near-global daytime boundary layer height using high-resolution radiosondes: First results and comparison with ERA5, MERRA-2, JRA-55, and NCEP-2 reanalyses. Atmos. Chem. Phys. 2021, 21, 17079–17097. [Google Scholar] [CrossRef]
- Miller, M.G.; Zieske, P.L. Turbulence Environment Characterization; Interim Report; Avco-Everett Research Lab.: Everett, MA, USA, 1979; p. 135. [Google Scholar]
- Good, R.E.; Beland, R.R.; Murphy, E.A.; Brown, J.H.; Dewan, E.M. Atmospheric models of optical turbulence. In Proceedings of the Modeling of the Atmosphere, Orlando, FL, USA, 24 August 1988; SPIE: Orlando, FL, USA, 1988; pp. 165–186. [Google Scholar]
- Jumper, G.; Beland, R. Progress in the understanding and modeling of atmospheric optical turbulence. In Proceedings of the 31st Plasmadynamics and Lasers Conference, Denver, CO, USA, 19 June 2000; p. 2355. [Google Scholar]
- Abahamid, A.; Jabiri, A.; Vernin, J.; Benkhaldoun, Z.; Azouit, M.; Agabi, A. Optical turbulence modeling in the boundary layer and free atmosphere using instrumented meteorological balloons. Astron. Astrophys. 2004, 416, 1193–1200. [Google Scholar] [CrossRef]
- Nath, D.; Venkat Ratnam, M.; Patra, A.; Krishna Murthy, B.; Bhaskar Rao, S.V. Turbulence characteristics over tropical station Gadanki (13.5°N, 79.2°E) estimated using high-resolution GPS radiosonde data. J. Geophys. Res. 2010, 115, D07102. [Google Scholar] [CrossRef]
- Dewan, E.M.; Good, R.E.; Beland, B.; Brown, J. A Model for (Optical Turbulence) Profiles Using Radiosonde Data; Phillips Laboratory Technical Report, PL-TR-93-2043; ADA 279399; Phillips Laboratory: Springfield, VA, USA, 1993. [Google Scholar]
- Ruggiero, F.H.; DeBenedictis, D.A. Forecasting optical turbulence from mesoscale numerical weather prediction models. In Proceedings of the DoD High Performance Modernization Program Users Group Conference, Austin, TX, USA, 10–14 June 2002; pp. 10–14. [Google Scholar]
- Basu, S. A simple approach for estimating the refractive index structure parameter () profile in the atmosphere. Opt. Lett. 2015, 40, 4130–4133. [Google Scholar] [CrossRef]
- Bi, C.C.; Qian, X.M.; Liu, Q.; Zhu, W.Y.; Li, X.B.; Luo, T.; Wu, X.Q.; Qing, C. Estimating and measurement of atmospheric optical turbulence according to balloon-borne radiosonde for three sites in China. J. Opt. Soc. Am. A 2020, 37, 1785–1794. [Google Scholar] [CrossRef]
- Bi, C.C.; Qing, C.; Wu, P.F.; Jin, X.M.; Liu, Q.; Qian, X.M.; Zhu, W.Y.; Weng, N.Q. Optical turbulence profile in marine environment with artificial neural network model. Remote Sens. 2022, 14, 2267. [Google Scholar] [CrossRef]
- Bufton, J.L. Correlation of microthermal turbulence data with meteorological soundings in the troposphere. J. Atmos. Sci. 1973, 30, 83–87. [Google Scholar] [CrossRef]
- Beland, R.R. Propagation through atmospheric optical turbulence. Atmos. Propag. Radiat. 1993, 2, 157–232. [Google Scholar]
- Qing, C.; Luo, T.; Bi, C.C.; Li, X.B.; Cui, S.C.; Yang, Q.K.; Su, C.D.; Wu, S.; Qian, X.M.; Wu, X.Q. Optical turbulence and wind speed distributions above the Tibetan Plateau from balloon-borne microthermal measurements. Mon. Not. R. Astron. Soc. 2021, 508, 4096–4105. [Google Scholar] [CrossRef]
- Liu, L.; Yao, Y.; Vernin, J.; Wang, H.; Yin, J.; Qian, X. Multi-instrument characterization of optical turbulence at the Ali observatory. J. Phys. Conf. Ser. 2015, 595, 012019. [Google Scholar] [CrossRef]
- Cao, Z.H.; Hao, J.X.; Feng, L.; Jones, H.R.; Li, J.; Xu, J.; Liu, L.Y.; Song, T.F.; Wang, J.F.; Chen, H.L. Data processing and data products from 2017 to 2019 campaign of astronomical site testing at Ali, Daocheng and Muztagh-ata. Res. Astron. Astrophys. 2020, 20, 082. [Google Scholar] [CrossRef]
- Feng, L.; Hao, J.X.; Cao, Z.H.; Bai, J.M.; Yang, J.; Zhou, X.; Yao, Y.Q.; Hou, J.L.; Zhao, Y.H.; Liu, Y. Site testing campaign for the Large Optical/infrared Telescope of China: Overview. Res. Astron. Astrophys. 2020, 20, 080. [Google Scholar] [CrossRef]
- Song, T.F.; Liu, Y.; Wang, J.X.; Zhang, X.F.; Liu, S.Q.; Zhao, M.Y.; Li, X.B.; Cai, Z.C.; Song, Q.W.; Cao, Z.H.; et al. Site testing campaign for the Large Optical/infrared Telescope of China: General introduction of the Daocheng site. Res. Astron. Astrophys. 2020, 20, 085. [Google Scholar] [CrossRef]


| Horizontal Coverage | Horizontal Resolution | Vertical Resolution | Vertical Coverage | Temporal Coverage | Temporal Resolution |
|---|---|---|---|---|---|
| Global | 0.25 × 0.25 | 137 level | 1000 hPa to 1 hPa | 1959 to present | Hourly |
| Name | Site (lon, lat) | ERA5 (lon, lat) | Time (UTC) |
|---|---|---|---|
| Da Qaidam | 95.35E, 37.74N | 95.25E, 37.75N | Aug. 2020 |
| Haikou | 110.19E, 20.05N | 110.25E, 20.00N | Apr. 2018 |
| Rongcheng | 122.11E, 36.46N | 122.00E, 36.50N | Nov. 2018 |
| Ali | 80.06E, 32.31N | 80.00E, 32.25N | Mar. 2017 to Feb. 2019 |
| Daocheng | 100.11E, 29.11N | 100.00E, 29.00N | Mar. 2017 to Feb. 2019 |
| Muztagh-ata | 74.90E, 38.33N | 75.00E, 38.25N | Mar. 2017 to Feb. 2019 |
| Lenghu | 93.89E, 38.61N | 94.00E, 38.50N | Oct. 2018 to 2020 |
| Site Name | Seeing (Arcseconds) (Median [25%, 75%]) | |
|---|---|---|
| Measurement | ERA5 | |
| Da Qaidam | 1.06 [0.72, 1.75] | 0.88 [0.85, 0.93] |
| Haikou | 1.15 [0.74, 2.32] | 1.17 [1.15, 1.20] |
| Rongcheng | 1.09 [0.98, 1.38] | 1.23 [1.17, 1.30] |
| Ali | 1.08 [0.88, 1.39] [44] | 0.87 [0.79, 1.00] |
| Daocheng | 1.01 [0.84, 1.22] [44] | 0.96 [0.85, 1.10] |
| Muztagh-ata | 0.82 [0.64, 1.06] [44] | 0.85 [0.78, 0.92] |
| Lenghu | 0.75 [0.61, 1.03] [5] | 0.96 [0.88, 1.07] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bi, C.; Qing, C.; Qian, X.; Luo, T.; Zhu, W.; Weng, N. Investigation of the Global Spatio-Temporal Characteristics of Astronomical Seeing. Remote Sens. 2023, 15, 2225. https://doi.org/10.3390/rs15092225
Bi C, Qing C, Qian X, Luo T, Zhu W, Weng N. Investigation of the Global Spatio-Temporal Characteristics of Astronomical Seeing. Remote Sensing. 2023; 15(9):2225. https://doi.org/10.3390/rs15092225
Chicago/Turabian StyleBi, Cuicui, Chun Qing, Xianmei Qian, Tao Luo, Wenyue Zhu, and Ningquan Weng. 2023. "Investigation of the Global Spatio-Temporal Characteristics of Astronomical Seeing" Remote Sensing 15, no. 9: 2225. https://doi.org/10.3390/rs15092225
APA StyleBi, C., Qing, C., Qian, X., Luo, T., Zhu, W., & Weng, N. (2023). Investigation of the Global Spatio-Temporal Characteristics of Astronomical Seeing. Remote Sensing, 15(9), 2225. https://doi.org/10.3390/rs15092225







