A Comparative Study of the Temperature Change in a Warm Eddy Using Multisource Data
Abstract
1. Introduction
2. Data and Methodology
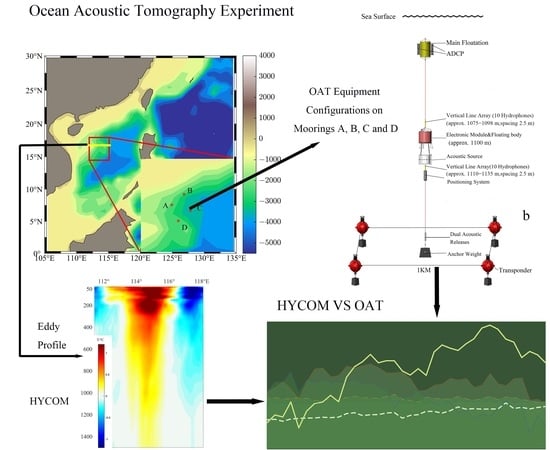
2.1. 2021 SCS OAT Experiment Data
2.2. Satellite Altimeter and HYCOM Data
2.3. Data Processing Methods
2.3.1. Sound Speed/Temperature Conversion
2.3.2. OAT Data Processing
2.3.3. Processing of HYCOM Data
3. Movement of the Eddy from Altimeters and HYCOM Data
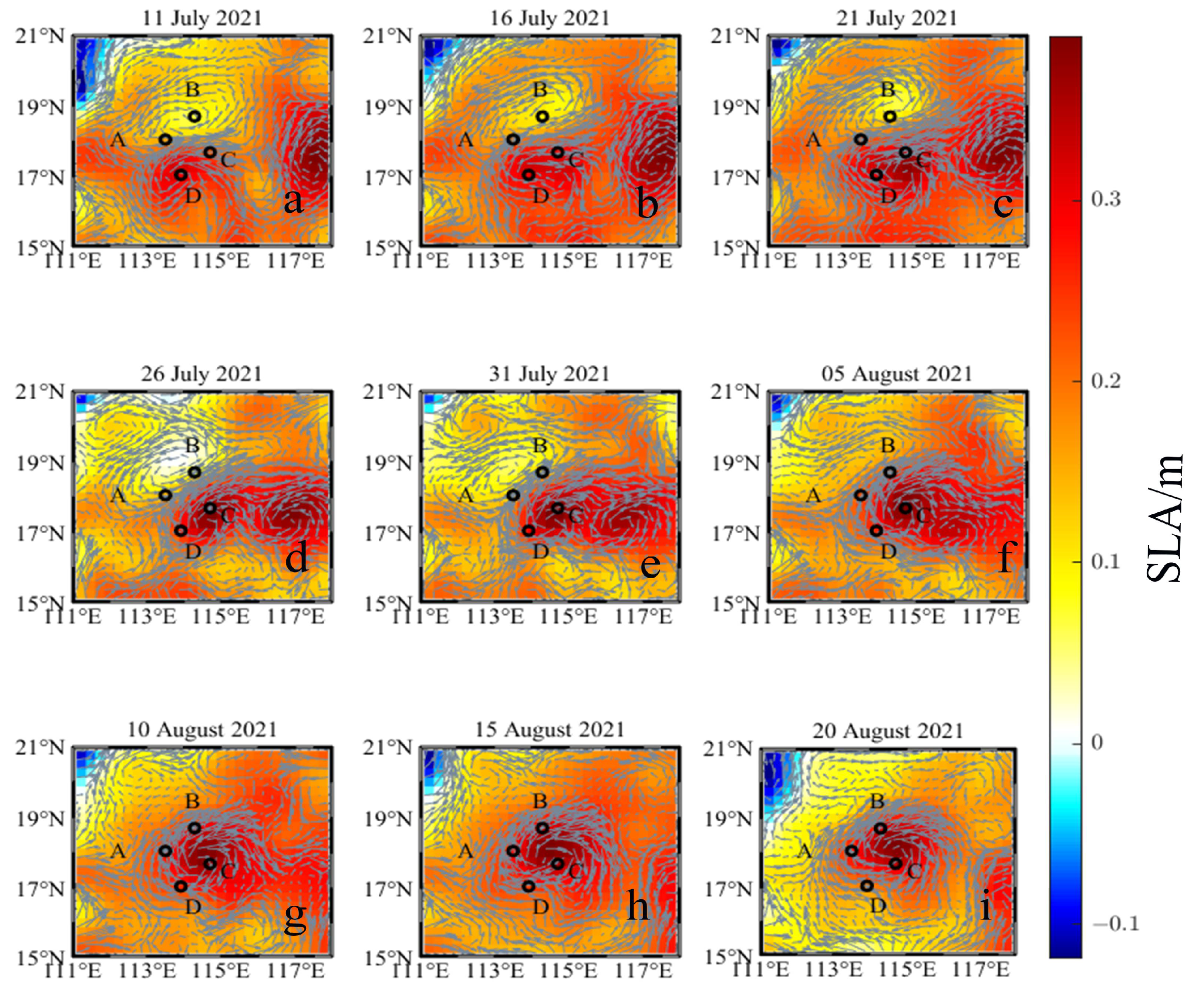
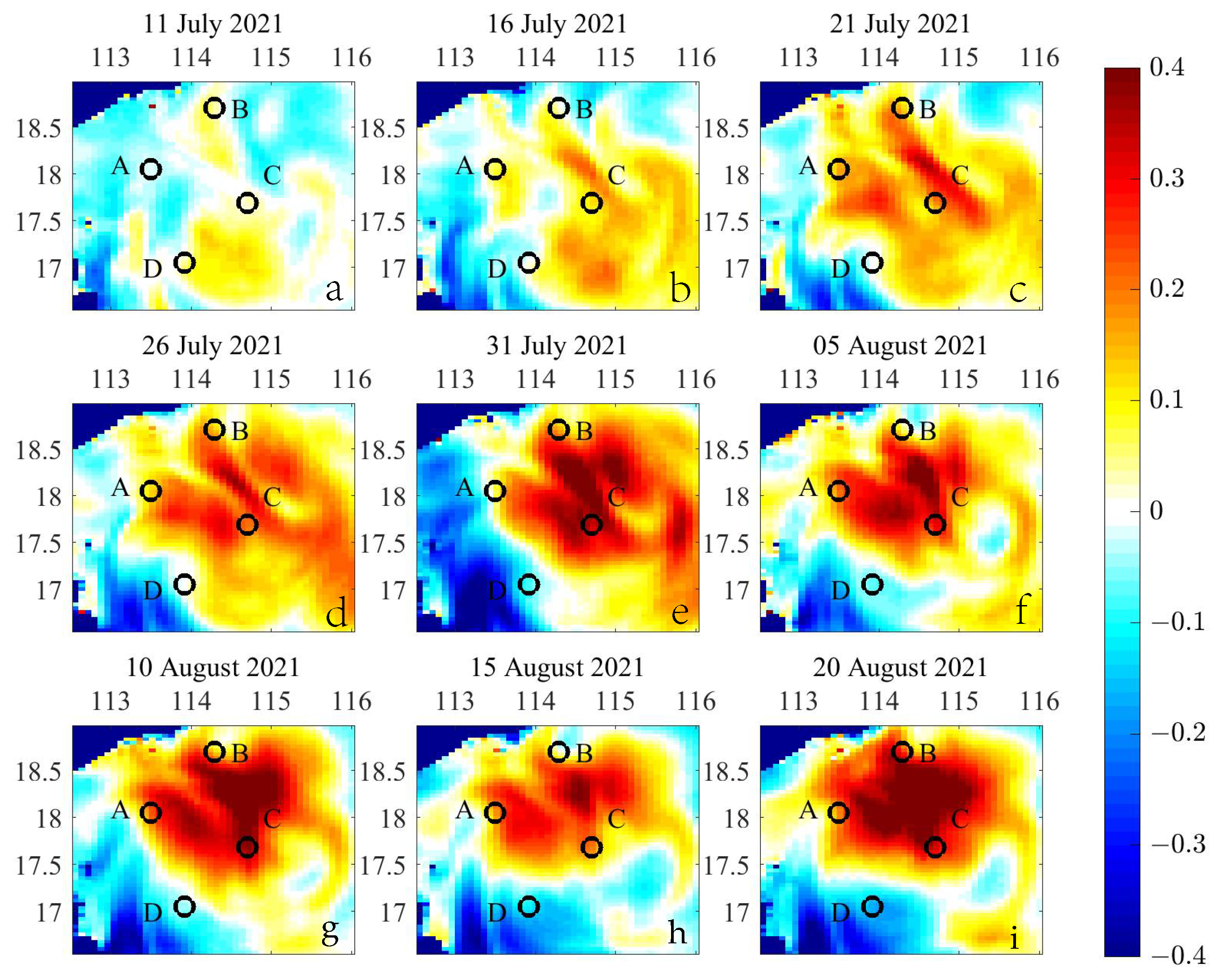
3.1. The Movement of the Eddy Imaged by Altimeters
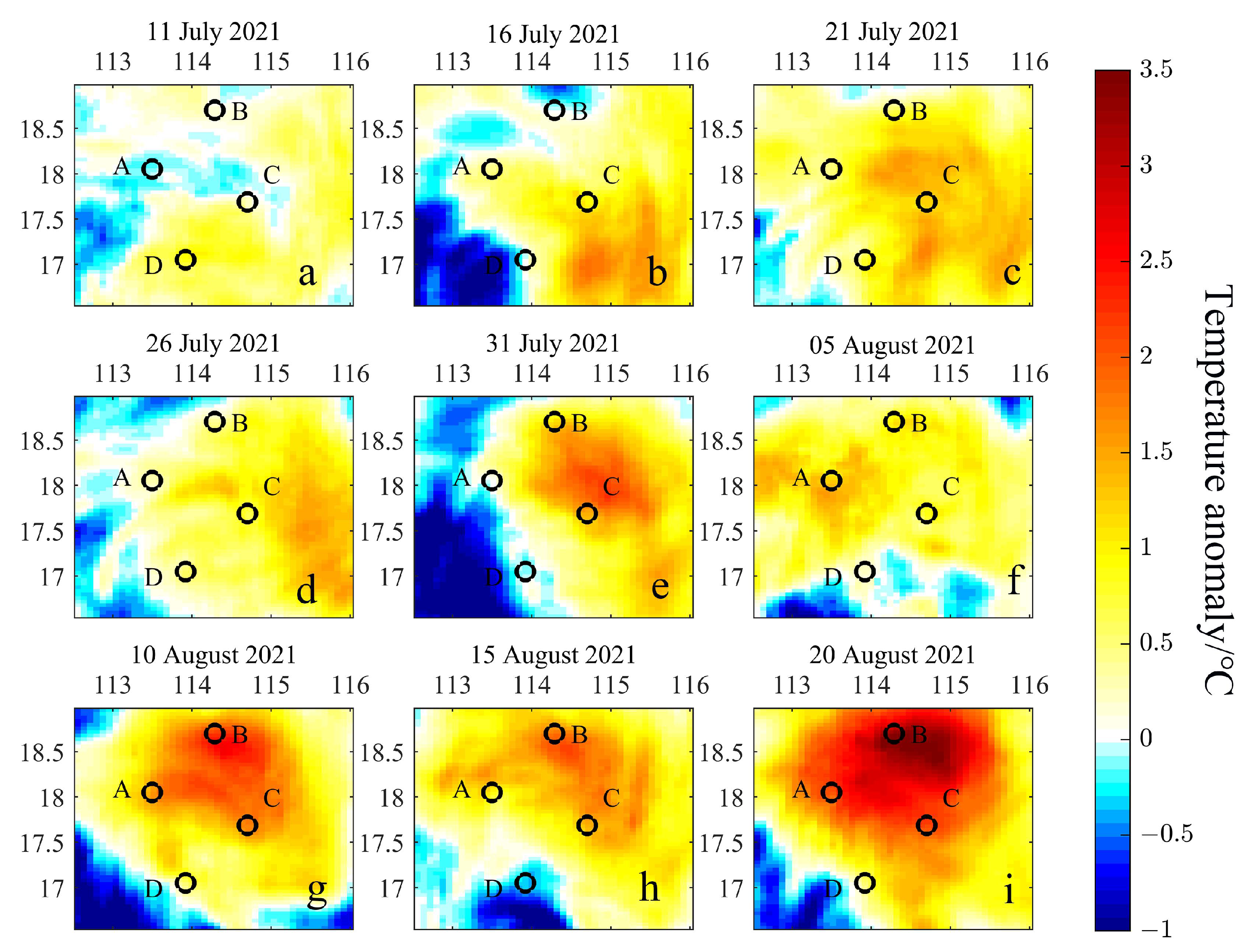
3.2. The Warm Eddy Captured by HYCOM Data
4. Results of the OAT Experiment
5. Comparison of the Eddy Using Multisource Data
5.1. Comparison of the Eddy Movement between the MSLA and HYCOM Data
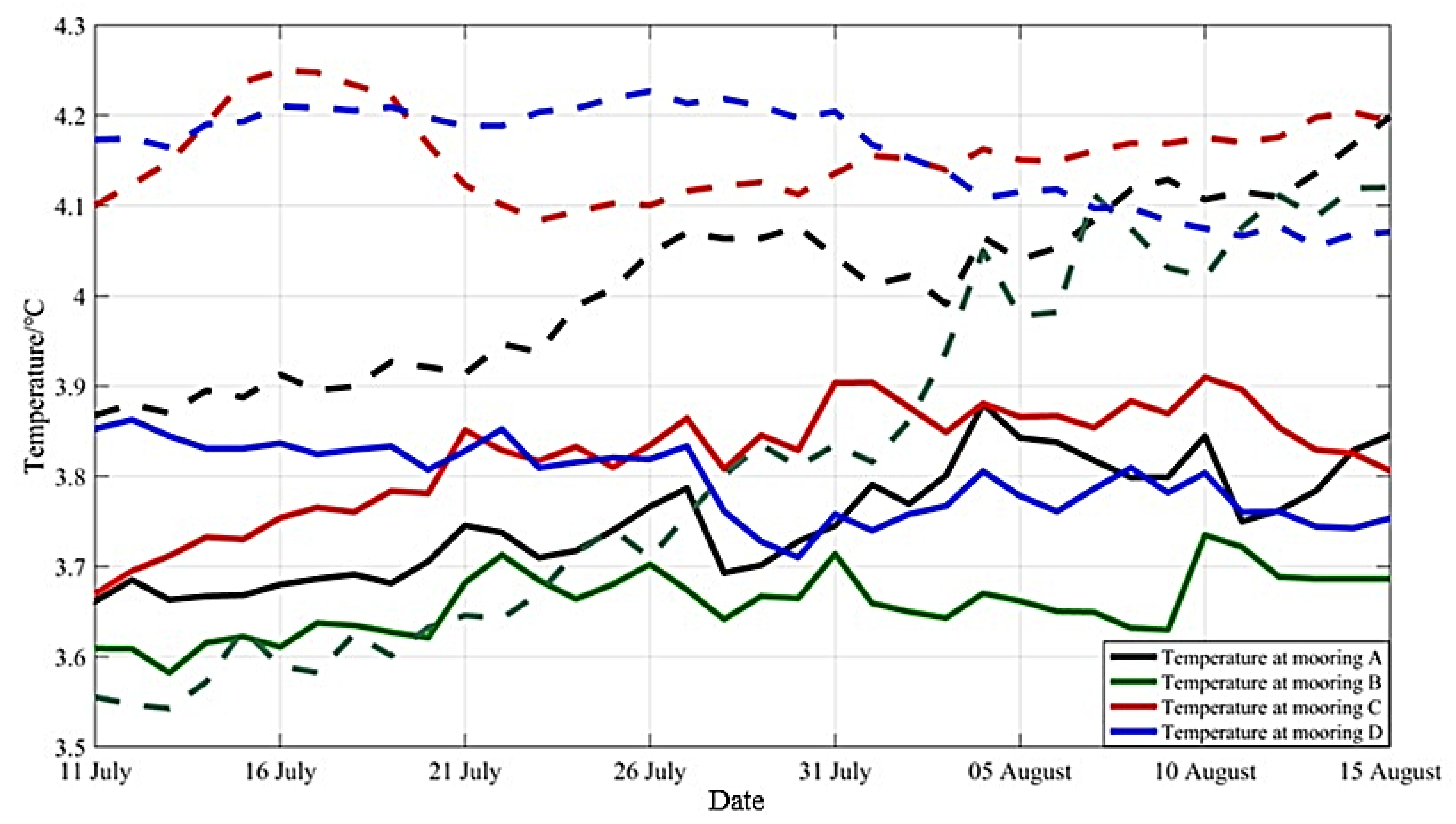
5.2. Comparison of Temperature between MSLA and TD/CTD
5.3. Comparison of Temperature between TD/CTD and HYCOM
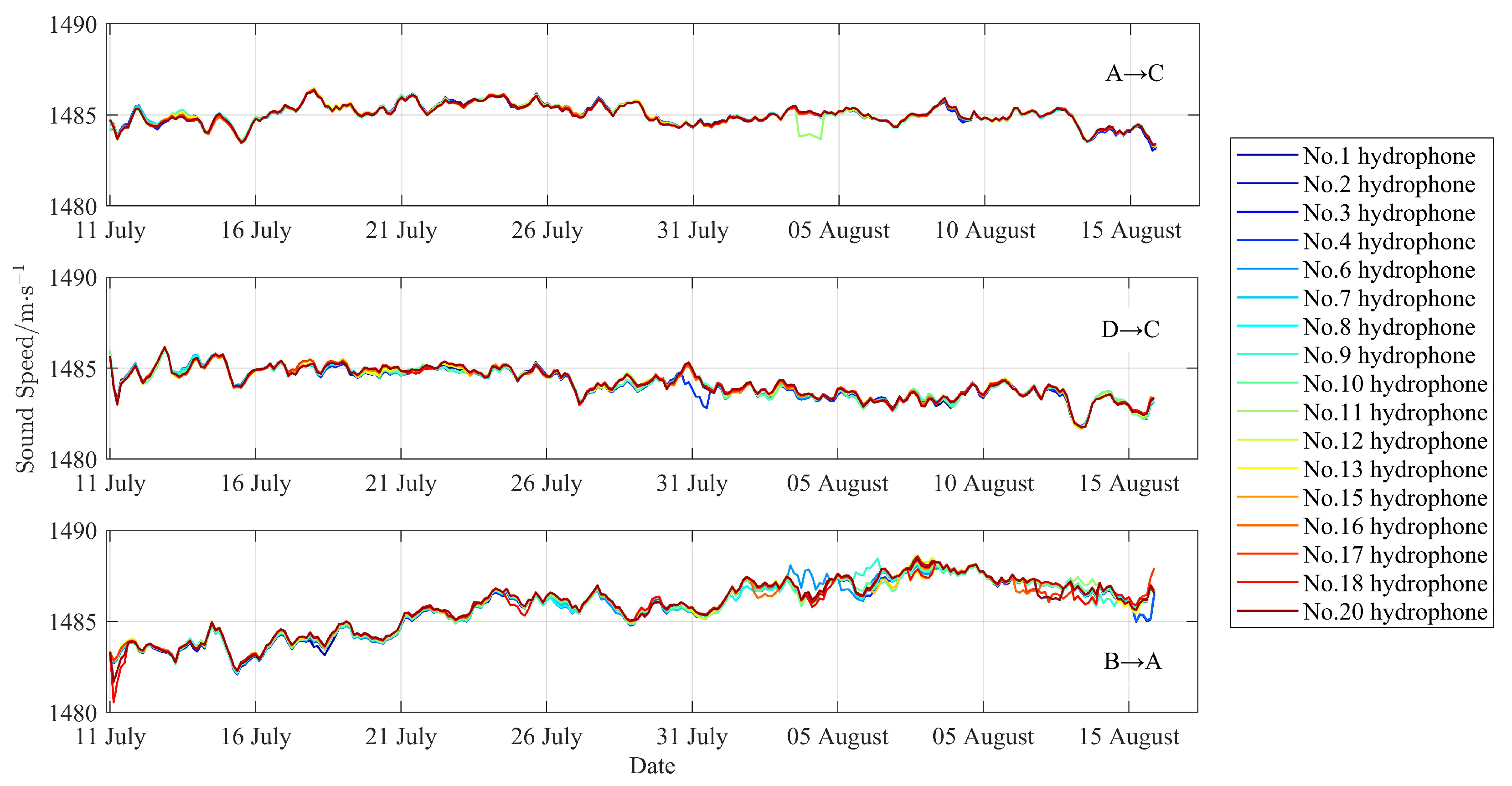
5.4. Comparison of the Eddy Temperature between the OAT and HYCOM Data
5.4.1. Comparison of the Eddy Movement at the Sound Channel Axis Depth
5.4.2. Difference in the Eddy Temperature at the Sound Channel Axis Depth between OAT and HYCOM
6. Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cai, S.; Xie, J.; He, J. An Overview of Internal Solitary Waves in the South China Sea. Surv. Geophys. 2012, 33, 927–943. [Google Scholar] [CrossRef]
- Hong, D.-B.; Yang, C.-S.; Ouchi, K. Estimation of Internal Wave Velocity in the Shallow South China Sea Using Single and Multiple Satellite Images. Remote Sens. Lett. 2015, 6, 448–457. [Google Scholar] [CrossRef]
- Chu, P.C.; Fan, C.; Lozano, C.J.; Kerling, J.L. An Airborne Expendable Bathythermograph Survey of the South China Sea, May 1995. J. Geophys. Res. Oceans 1998, 103, 21637–21652. [Google Scholar] [CrossRef]
- Xiu, P.; Chai, F.; Shi, L.; Xue, H.; Chao, Y. A Census of Eddy Activities in the South China Sea during 1993–2007. J. Geophys. Res. 2010, 115, C03012. [Google Scholar] [CrossRef]
- Lin, X.; Dong, C.; Chen, D.; Liu, Y.; Yang, J.; Zou, B.; Guan, Y. Three-Dimensional Properties of Mesoscale Eddies in the South China Sea Based on Eddy-Resolving Model Output. Deep Sea Res. Part Oceanogr. Res. Pap. 2015, 99, 46–64. [Google Scholar] [CrossRef]
- Klein, P.; Lapeyre, G.; Siegelman, L.; Qiu, B.; Fu, L.-L.; Torres, H.; Su, Z.; Menemenlis, D.; Gentil, S.L. Ocean-Scale Interactions from Space. Earth Space Sci. 2019, 6, 795–817. [Google Scholar] [CrossRef]
- Ferrari, R.; Wunsch, C. Ocean Circulation Kinetic Energy: Reservoirs, Sources, and Sinks. Annu. Rev. Fluid Mech. 2008, 4, 253–282. [Google Scholar] [CrossRef]
- Wolfe, C.L.; Cessi, P.; McClean, J.L.; Maltrud, M.E. Vertical Heat Transport in Eddying Ocean Models. Geophys. Res. Lett. 2008, 35. [Google Scholar] [CrossRef]
- McGillicuddy, D.J. Mechanisms of Physical-Biological-Biogeochemical Interaction at the Oceanic Mesoscale. Annu. Rev. Mar. Sci. 2016, 8, 125–159. [Google Scholar] [CrossRef]
- Vastano, A.C.; Owens, G.E. On the Acoustic Characteristics of a Gulf Stream Cyclonic Ring. J. Phys. Oceanogr. 1973, 3, 470–478. [Google Scholar] [CrossRef]
- Weinberg, N.L.; Zabalgogeazcoa, X. Coherent Ray Propagation through a Gulf Stream Ring. J. Acoust. Soc. Am. 1977, 62, 888. [Google Scholar] [CrossRef]
- Mikryukov, A.V.; Popov, O.E. Penetration of Sound into the Cold Eddy of the Sargasso Sea. Acoust. Phys. 2008, 54, 77–83. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, W.; Qiu, B. Oceanic Mass Transport by Mesoscale Eddies. Science 2014, 345, 322–324. [Google Scholar] [CrossRef] [PubMed]
- Shang, E.C.; Wang, Y.Y. ACOUSTIC TRAVEL TIME COMPUTATION BASED ON PE SOLUTION. J. Comput. Acoust. 1993, 1, 91–100. [Google Scholar] [CrossRef]
- Baer, R.N. Calculations of Sound Propagation through an Eddy. J. Acoust. Soc. Am. 1980, 67, 1180–1185. [Google Scholar] [CrossRef]
- Jian, Y.J.; Zhang, J.; Liu, Q.S.; Wang, Y.F. Effect of Mesoscale Eddies on Underwater Sound Propagation. Appl. Acoust. 2009, 70, 432–440. [Google Scholar] [CrossRef]
- Munk, W.H. Horizontal Deflection of Acoustic Paths by Mesoscale Eddies. J. Phys. Oceanogr. 1980, 10, 596–604. [Google Scholar] [CrossRef]
- Weinberg, N.L.; Clark, J.G. Horizontal Acoustic Refraction through Ocean Mesoscale Eddies and Fronts. J. Acoust. Soc. Am. 1980, 68, 703–705. [Google Scholar] [CrossRef]
- Mellberg, L.E.; Robinson, A.R.; Botseas, G. Azimuthal Variation of Low-Frequency Acoustic Propagation through Asymmetric Gulf Stream Eddies. J. Acoust. Soc. Am. 1991, 89, 2157–2167. [Google Scholar] [CrossRef]
- Lawrence, M.W. Modeling of Acoustic Propagation across Warm-core Eddies. J. Acoust. Soc. Am. 1983, 73, 474–485. [Google Scholar] [CrossRef]
- Munk, W.; Wunsch, C. Ocean Acoustic Tomography: A Scheme for Large Scale Monitoring. Deep Sea Res. Part Oceanogr. Res. Pap. 1979, 26, 123–161. [Google Scholar] [CrossRef]
- Munk, W.; Worcester, P.; Wunsch, C. Ocean Acoustic Tomography; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Elisseeff, P.; Schmidt, H.; Wen, X. Ocean Acoustic Tomography as a Data Assimilation Problem. IEEE J. Ocean. Eng. 2002, 27, 275–282. [Google Scholar] [CrossRef]
- Skarsoulis, E.K.; Cornuelle, B.D. Travel-Time Sensitivity Kernels in Ocean Acoustic Tomography. J. Acoust. Soc. Am. 2004, 116, 227–238. [Google Scholar] [CrossRef]
- Duda, T.F.; Flatté, S.M.; Colosi, J.A.; Cornuelle, B.D.; Hildebrand, J.A.; Hodgkiss, W.S.; Worcester, P.F.; Howe, B.M.; Mercer, J.A.; Spindel, R.C. Measured Wave-front Fluctuations in 1000-km Pulse Propagation in the Pacific Ocean. J. Acoust. Soc. Am. 1992, 92, 939–955. [Google Scholar] [CrossRef]
- Skarsoulis, E.K.; Athanassoulis, G.A.; Send, U. Ocean Acoustic Tomography Based on Peak Arrivals. J. Acoust. Soc. Am. 1996, 100, 797–813. [Google Scholar] [CrossRef]
- Colosi, J.A.; Van Uffelen, L.J.; Cornuelle, B.D.; Dzieciuch, M.A.; Worcester, P.F.; Dushaw, B.D.; Ramp, S.R. Observations of Sound-Speed Fluctuations in the Western Philippine Sea in the Spring of 2009. J. Acoust. Soc. Am. 2013, 134, 3185–3200. [Google Scholar] [CrossRef] [PubMed]
- Worcester, P.F.; Dzieciuch, M.A.; Mercer, J.A.; Andrew, R.K.; Dushaw, B.D.; Baggeroer, A.B.; Heaney, K.D.; D’Spain, G.L.; Colosi, J.A.; Stephen, R.A.; et al. The North Pacific Acoustic Laboratory Deep-Water Acoustic Propagation Experiments in the Philippine Sea. J. Acoust. Soc. Am. 2013, 134, 3359–3375. [Google Scholar] [CrossRef]
- Sagen, H.; Dushaw, B.D.; Skarsoulis, E.K.; Dumont, D.; Dzieciuch, M.A.; Beszczynska-Möller, A. Time Series of Temperature in Fram Strait Determined from the 2008–2009 DAMOCLES Acoustic Tomography Measurements and an Ocean Model. J. Geophys. Res. Oceans 2016, 121, 4601–4617. [Google Scholar] [CrossRef]
- Liu, J.; Piao, S.; Gong, L.; Zhang, M.; Guo, Y.; Zhang, S. The Effect of Mesoscale Eddy on the Characteristic of Sound Propagation. J. Mar. Sci. Eng. 2021, 9, 787. [Google Scholar] [CrossRef]
- Ji, X.; Zhao, H. Three-Dimensional Sound Speed Inversion in South China Sea Using Ocean Acoustic Tomography Combined with Pressure Inverted Echo Sounders. In Proceedings of the Global Oceans 2020: Singapore-U.S. Gulf Coast, Biloxi, MS, USA, 5 October 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 1–6. [Google Scholar]
- Ji, X.; Cheng, L.; Zhao, H. Physics-Guided Reduced-Order Representation of Three-Dimensional Sound Speed Fields with Ocean Mesoscale Eddies. Remote Sens. 2022, 14, 5860. [Google Scholar] [CrossRef]
- Worcester, P.F.; Cornuelle, B.D.; Dzieciuch, M.A.; Munk, W.H.; Howe, B.M.; Mercer, J.A.; Spindel, R.C.; Colosi, J.A.; Metzger, K.; Birdsall, T.G.; et al. A Test of Basin-Scale Acoustic Thermometry Using a Large-Aperture Vertical Array at 3250-Km Range in the Eastern North Pacific Ocean. J. Acoust. Soc. Am. 1999, 105, 3185–3201. [Google Scholar] [CrossRef]
- Wu, L. Ocean Models Adaptability Comparison and Improvement in the South China Sea. Master’s Thesis, Wuhan University of Technology, Wuhan, China, 2013. [Google Scholar]
- Mackenzie, K.V. Nine-term Equation for Sound Speed in the Oceans. J. Acoust. Soc. Am. 1981, 70, 807–812. [Google Scholar] [CrossRef]
- Dushaw, B.D.; Sagen, H.; Beszczynska-Möller, A. Sound Speed as a Proxy Variable to Temperature in Fram Strait. J. Acoust. Soc. Am. 2016, 140, 622–630. [Google Scholar] [CrossRef] [PubMed]
- Gaube, P.; McGillicuddy, D.J.; Chelton, D.B.; Behrenfeld, M.J.; Strutton, P.G. Regional Variations in the Influence of Mesoscale Eddies on Near-Surface Chlorophyll. J. Geophys. Res. Oceans 2014, 119, 8195–8220. [Google Scholar] [CrossRef]
- Zu, Y.; Sun, S.; Zhao, W.; Li, P.; Liu, B.; Fang, Y.; Samah, A.A. Seasonal Characteristics and Formation Mechanism of the Thermohaline Structure of Mesoscale Eddy in the South China Sea. Acta Oceanol. Sin. 2019, 38, 29–38. [Google Scholar] [CrossRef]
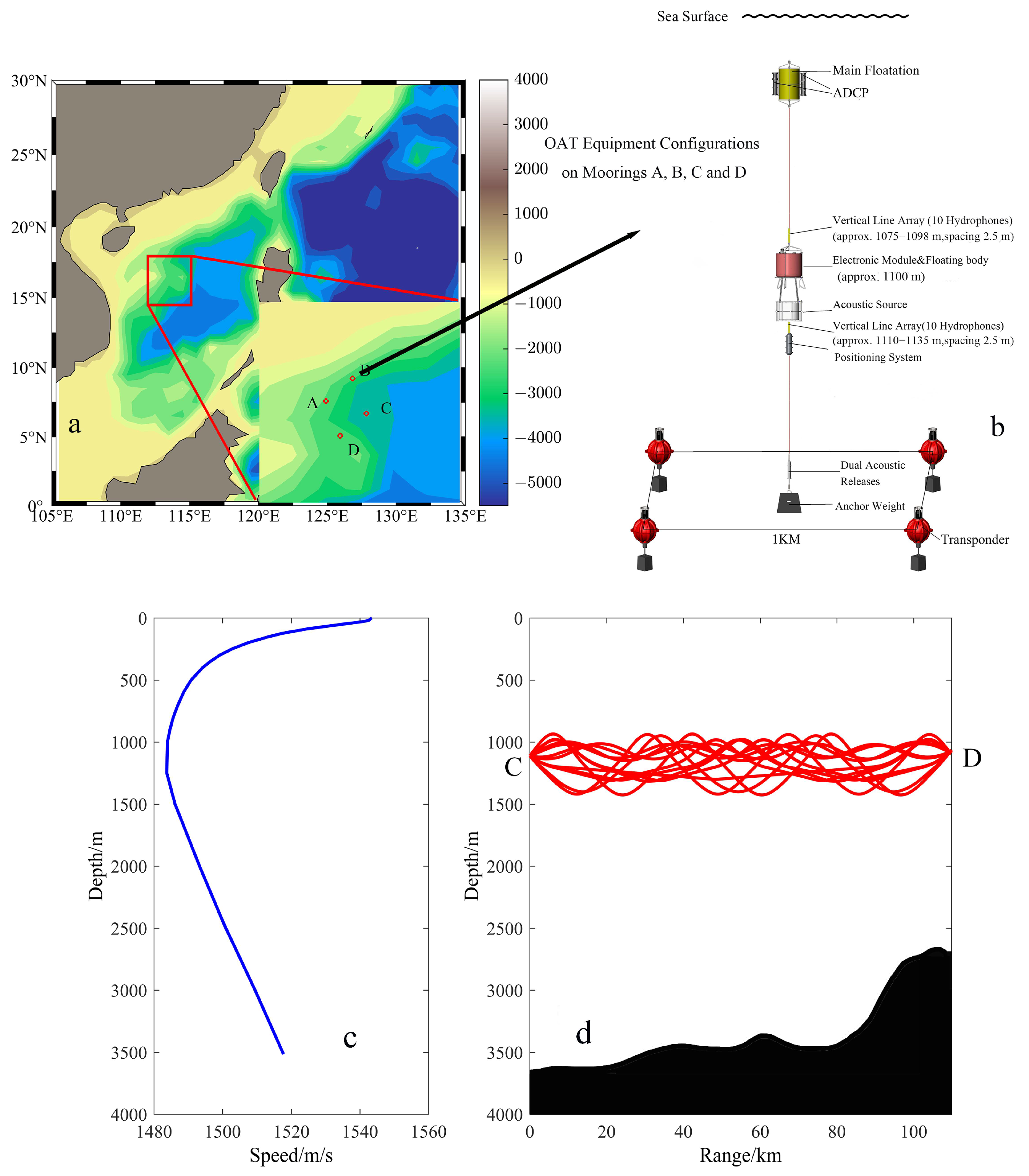




| Mooring | Water Depth/m | Location | Depth Range of Hydrophones/m | Spacing/m | Numbers of Hydrophones |
|---|---|---|---|---|---|
| A | 2168 | 18.06°N, 113.51°E | 1075–1097.5 | 2.5 | 10 |
| 1102.5–1125 | 2.5 | 10 | |||
| B | 2490 | 18.70°N, 114.29°E | 1065–1087.5 | 2.5 | 10 |
| 1102.5–1125 | 2.5 | 10 | |||
| C | 3620 | 17.69°N, 114.71°E | 1076–1098.5 | 2.5 | 10 |
| 1113.5–1136 | 2.5 | 10 | |||
| D | 2700 | 17.05°N, 113.92°E | 1076–1098.5 | 2.5 | 10 |
| 1113.5–1136 | 2.5 | 10 |
| Mooring Pair | Distance between Moorings/km |
|---|---|
| A–B | 109.81 |
| A–C | 133.26 |
| A–D | 120.12 |
| B–C | 120.72 |
| B–D | 187.80 |
| C–D | 109.39 |
| D→C | B→A | A→C | |||||
|---|---|---|---|---|---|---|---|
| Difference | Sound Speed/ms−1 | Temperature/°C | Sound Speed | Temperature | Sound Speed | Temperature | |
| Hydrophone | |||||||
| No. 1 | 4.23 | 1.02 | 6.02 | 1.44 | 3.20 | 0.77 | |
| No. 2 | 4.28 | 1.03 | 6.02 | 1.44 | 3.33 | 0.80 | |
| No. 3 | 4.28 | 1.03 | 5.93 | 1.42 | 3.18 | 0.76 | |
| No. 4 | 4.25 | 1.02 | 5.94 | 1.43 | 3.15 | 0.75 | |
| No. 5 | - | - | 6.03 | 1.44 | - | - | |
| No. 6 | 4.24 | 1.02 | 5.93 | 1.42 | 3.06 | 0.74 | |
| No. 7 | 4.25 | 1.02 | 6.01 | 1.44 | 3.13 | 0.75 | |
| No. 8 | 4.28 | 1.03 | 6.02 | 1.44 | 3.13 | 0.75 | |
| No. 9 | 4.23 | 1.01 | 6.24 | 1.50 | 3.15 | 0.76 | |
| No. 10 | 4.20 | 1.01 | 6.00 | 1.44 | 3.16 | 0.76 | |
| No. 11 | 4.50 | 1.08 | 6.18 | 1.48 | 3.21 | 0.77 | |
| No. 12 | 4.48 | 1.07 | 6.40 | 1.53 | 3.19 | 0.77 | |
| No. 13 | 4.47 | 1.07 | 5.96 | 1.43 | 3.16 | 0.756 | |
| No. 14 | - | - | 6.05 | 1.45 | - | - | |
| No. 15 | 4.47 | 1.07 | 6.09 | 1.46 | 3.12 | 0.75 | |
| No. 16 | 4.45 | 1.07 | 6.07 | 1.46 | 3.02 | 0.72 | |
| No. 17 | 4.37 | 1.05 | 4.37 | 1.05 | 3.03 | 0.73 | |
| No. 18 | 4.37 | 1.05 | 4.37 | 1.05 | 3.04 | 0.73 | |
| No. 19 | - | - | 6.87 | 1.65 | - | - | |
| No. 20 | 4.40 | 1.05 | 4.40 | 1.05 | 2.98 | 0.72 | |
| Mean Value | 4.34 | 1.04 | 5.85 | 1.40 | 3.13 | 0.75 | |
| HYCOM Result | NA | 0.19 | NA | 0.13 | NA | 0.04 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, X.; Yang, Y.; Weng, J. A Comparative Study of the Temperature Change in a Warm Eddy Using Multisource Data. Remote Sens. 2023, 15, 1650. https://doi.org/10.3390/rs15061650
Yang X, Yang Y, Weng J. A Comparative Study of the Temperature Change in a Warm Eddy Using Multisource Data. Remote Sensing. 2023; 15(6):1650. https://doi.org/10.3390/rs15061650
Chicago/Turabian StyleYang, Xiaohong, Yanming Yang, and Jinbao Weng. 2023. "A Comparative Study of the Temperature Change in a Warm Eddy Using Multisource Data" Remote Sensing 15, no. 6: 1650. https://doi.org/10.3390/rs15061650
APA StyleYang, X., Yang, Y., & Weng, J. (2023). A Comparative Study of the Temperature Change in a Warm Eddy Using Multisource Data. Remote Sensing, 15(6), 1650. https://doi.org/10.3390/rs15061650